Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike
Abstract
1. Introduction
1.1. Impact Damage
1.2. GLARE
1.3. Existing Knowledge
1.4. Critical Strain Energy Release Rate
1.5. Next Leap
2. Modeling Particulars
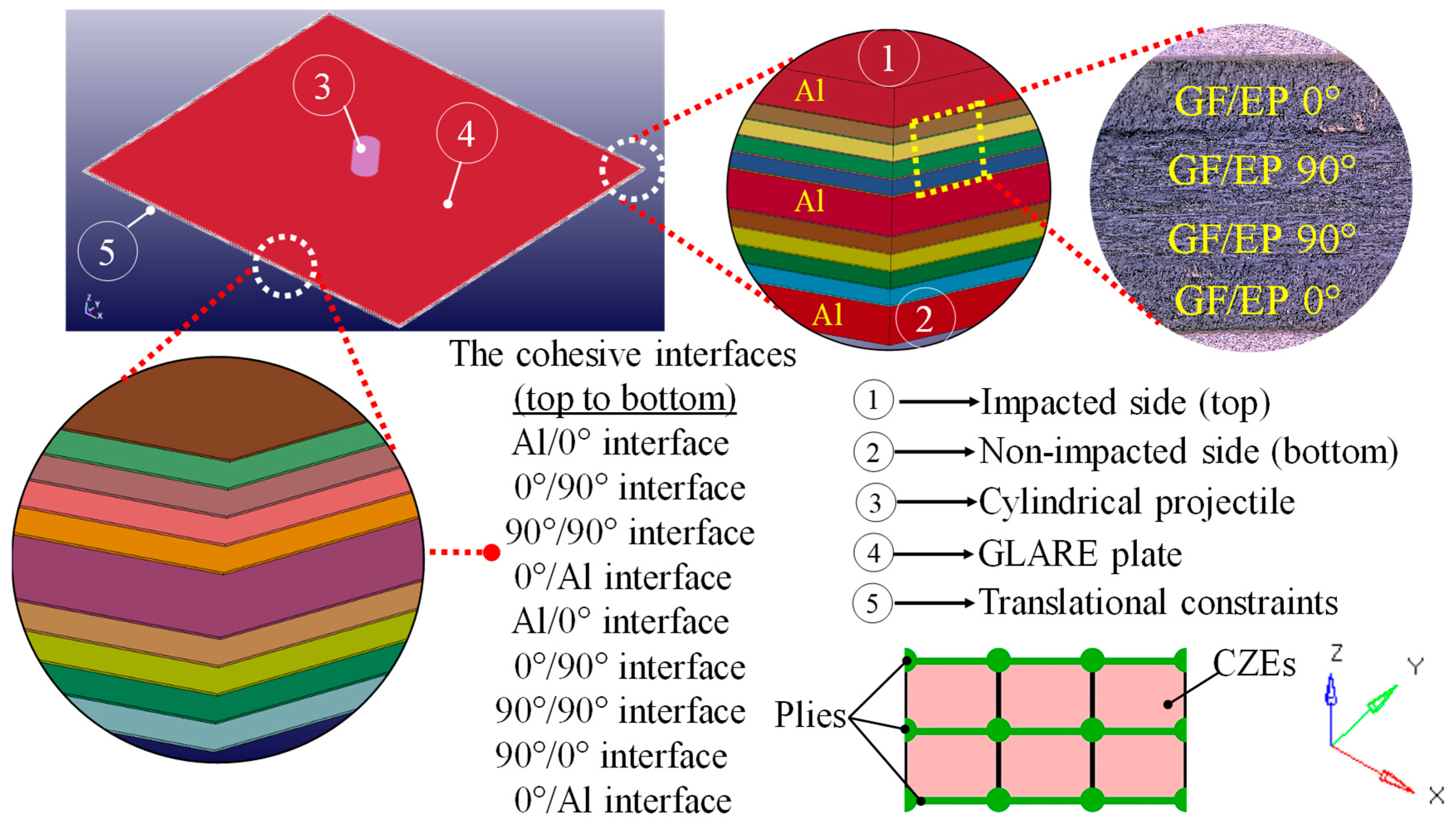
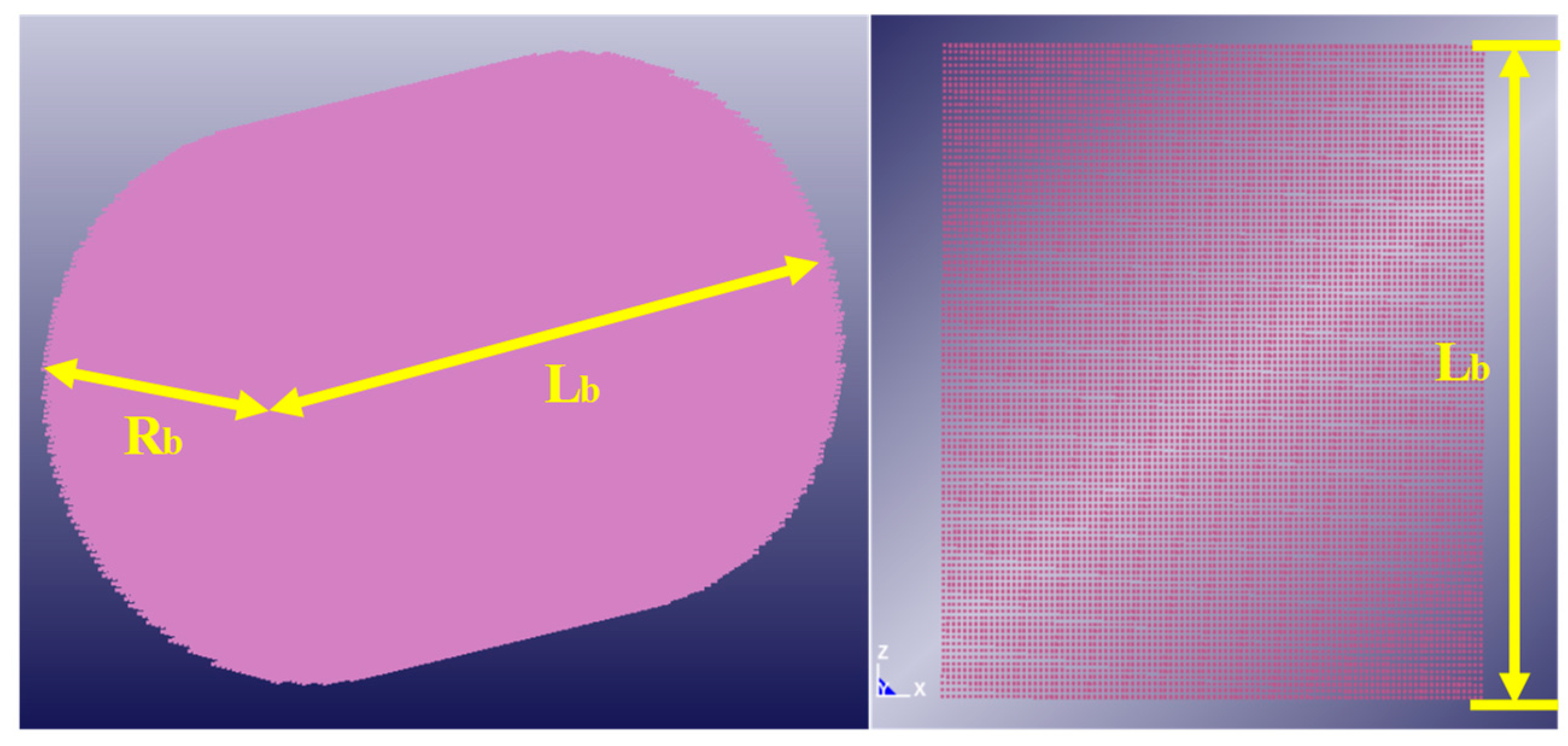
2.1. Geometry
2.2. Discretization
2.3. Material Properties
2.3.1. GLARE Material
2.3.2. Cohesive Zone Material
2.3.3. Projectile Material
2.4. Projectile Hydrodynamics
2.5. Constitutive Equations
2.5.1. GF/EP Composites
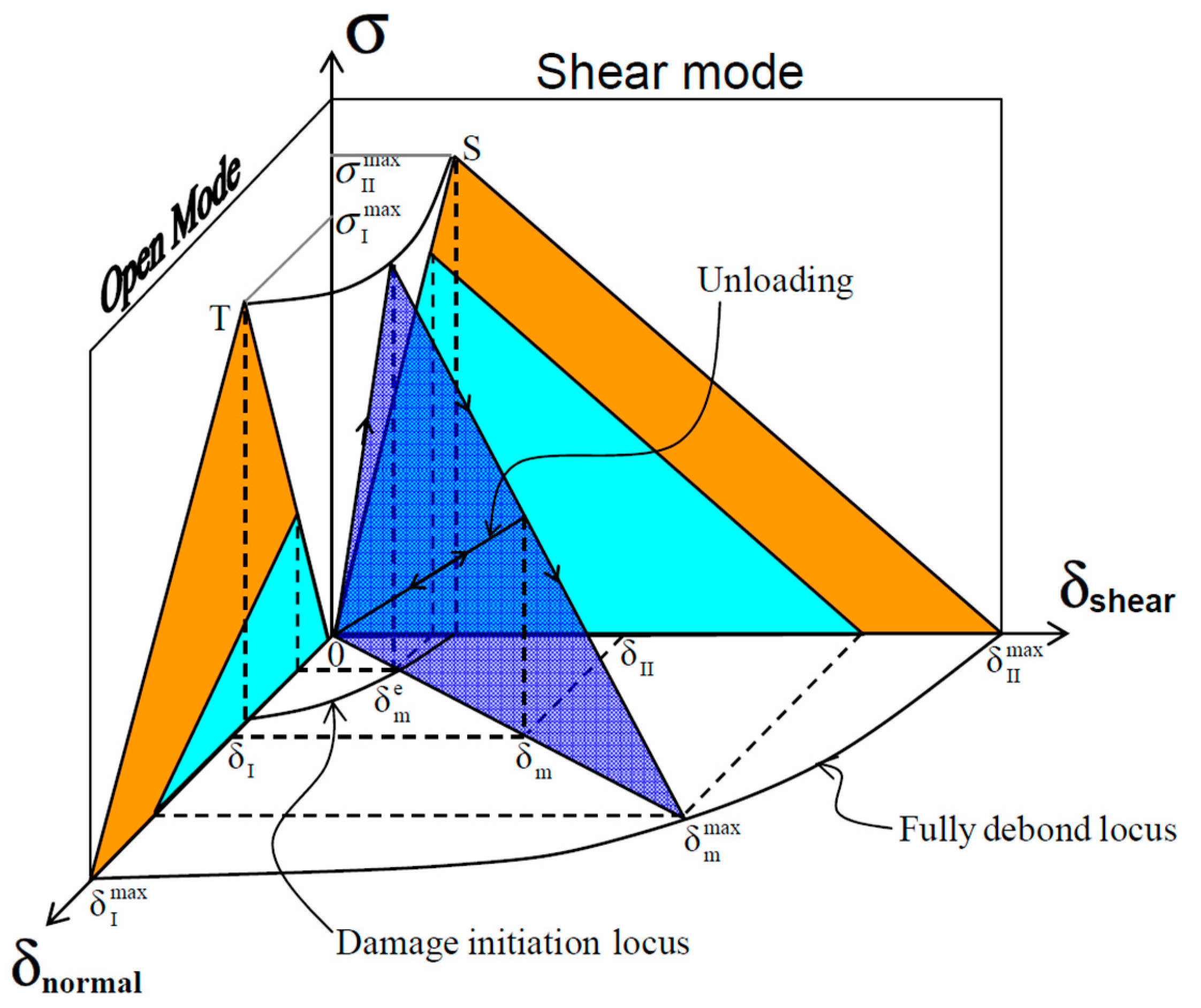
2.5.2. Cohesive Zone
2.6. Boundary Conditions
2.6.1. Translational Constraints
2.6.2. Projectile/Target Interaction
2.7. Numerical Implementation
3. Computational Predictions
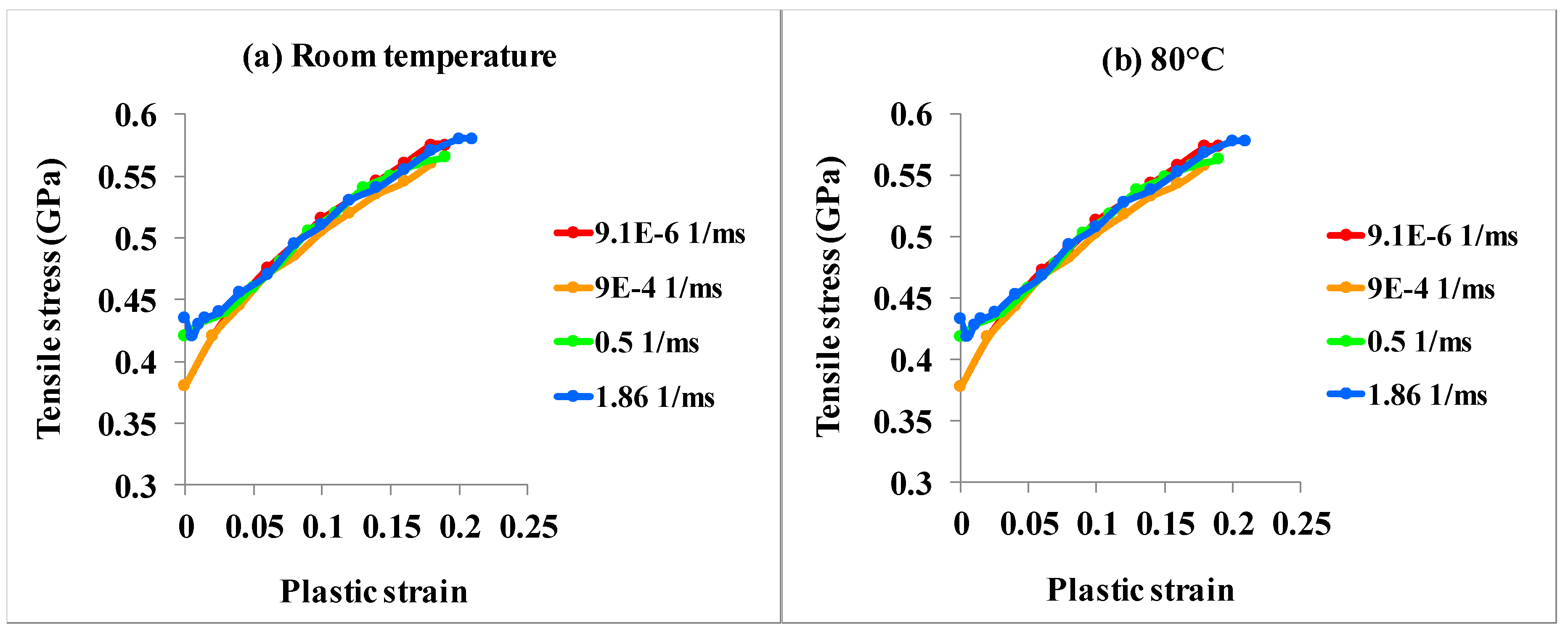
3.1. Elastoplastic Response of GLARE
3.1.1. Room Temperature (Gc0)
3.1.2. Model Verification
3.1.3. 80 °C (Gc80 = Gc0 + (39%*Gc0))
3.1.4. 80 °C (Gc80 = Gc0 + (45%*Gc0))
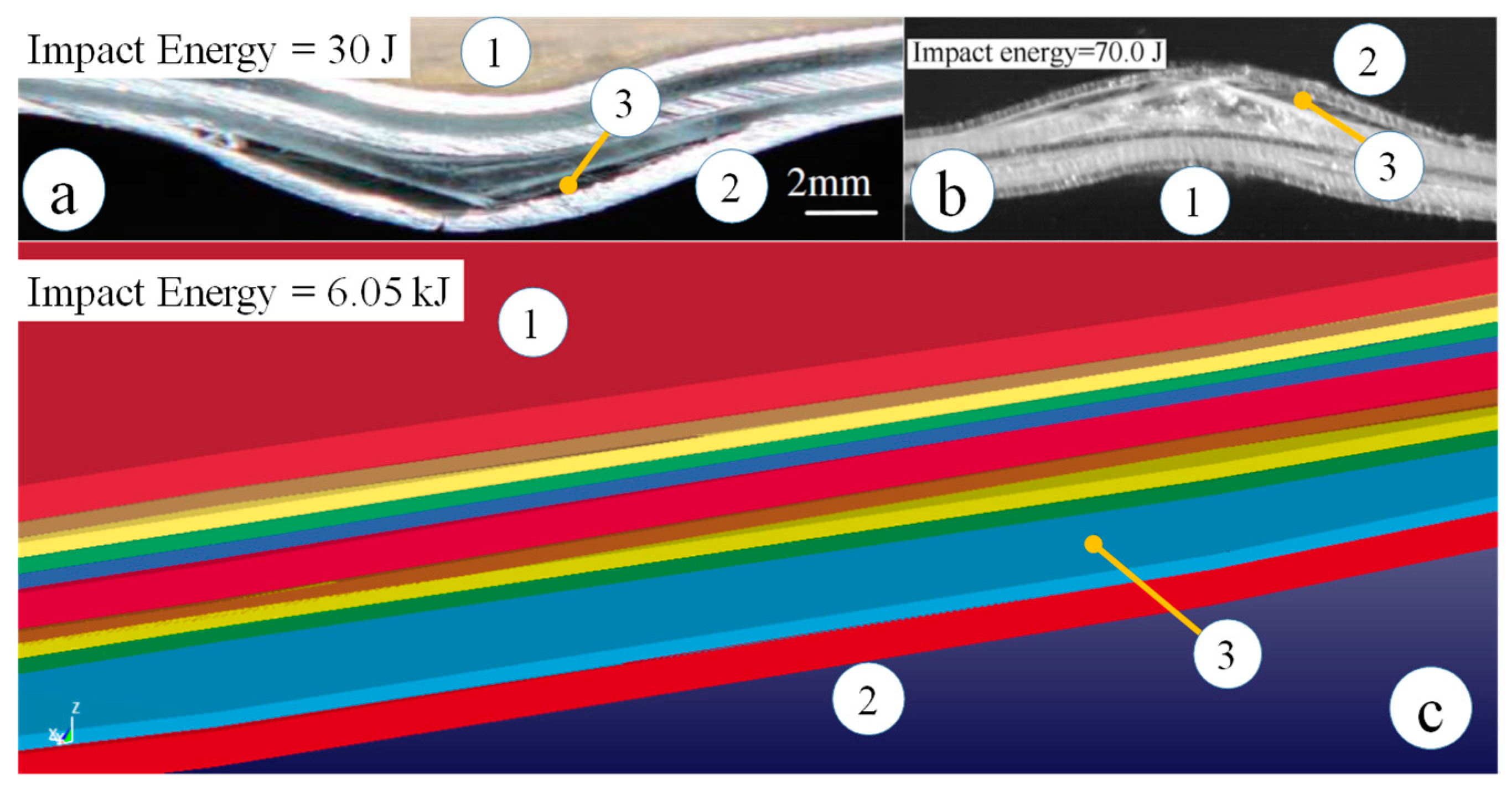
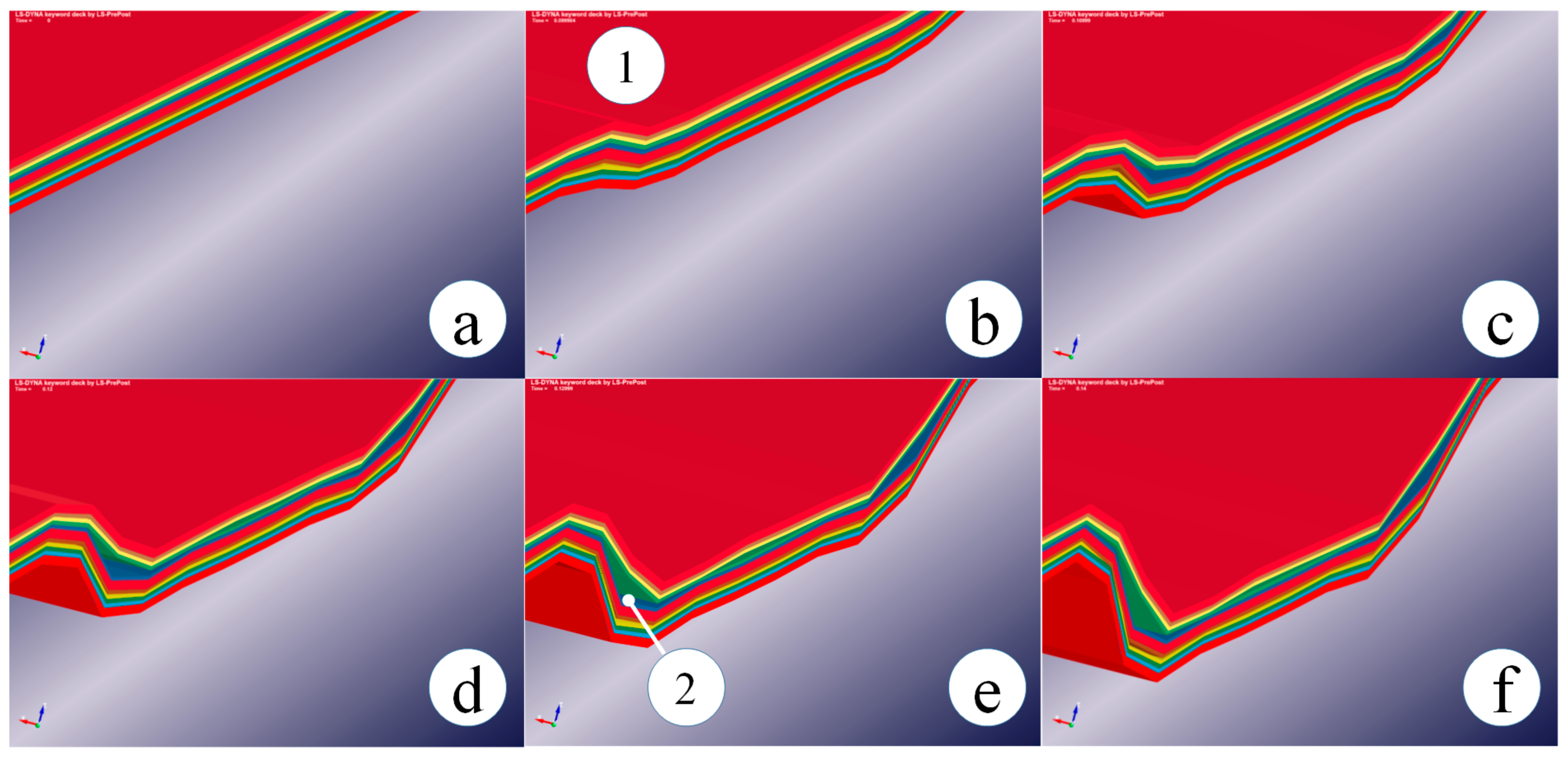
3.2. Delamination of Cohesive Interfaces
4. Experimental Procedures
4.1. Manufacturing of GLARE Specimens
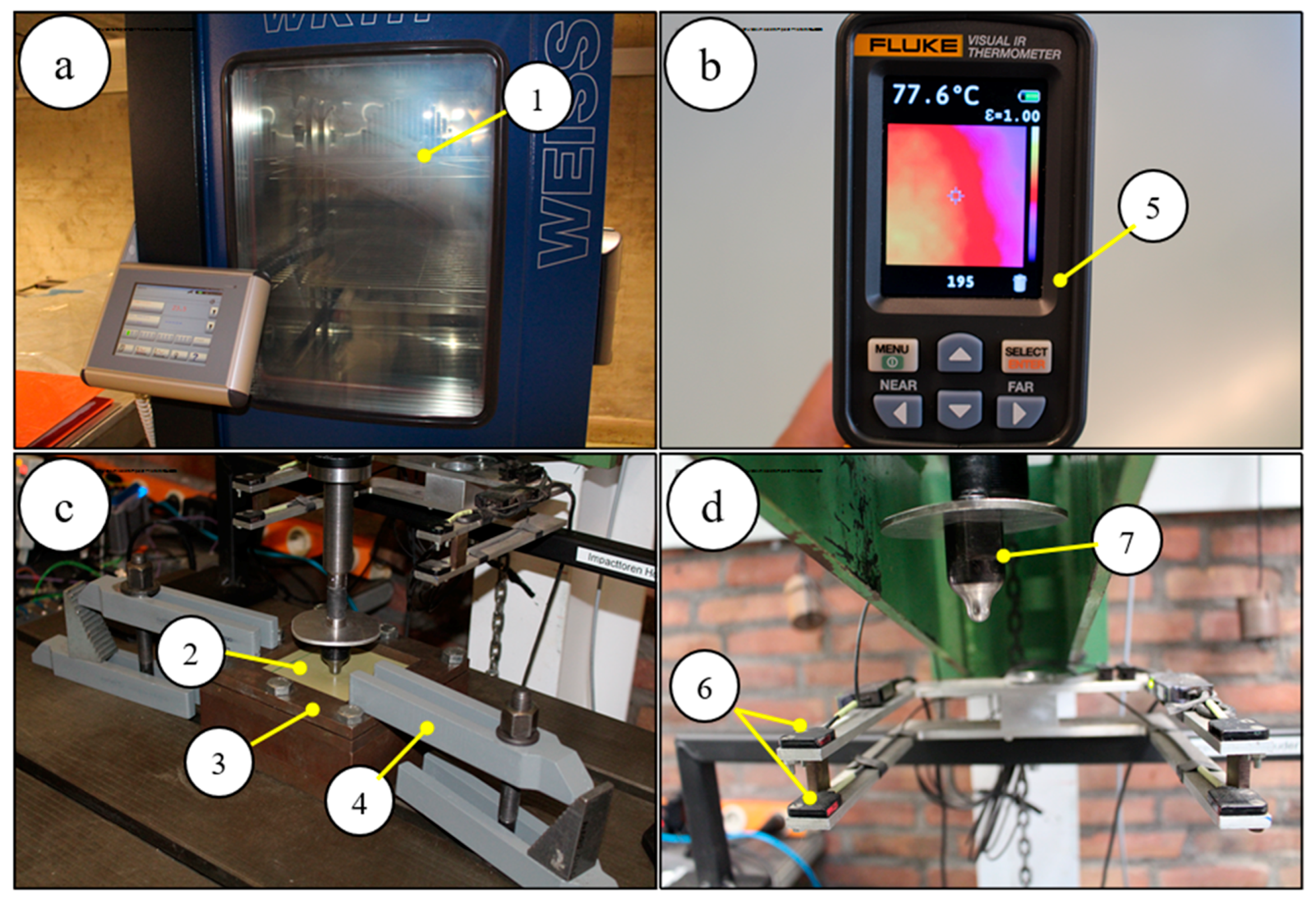
4.2. Impact Test Method
4.3. Test Set-Up
4.4. Test Matrix
4.5. Non-Destructive Inspection
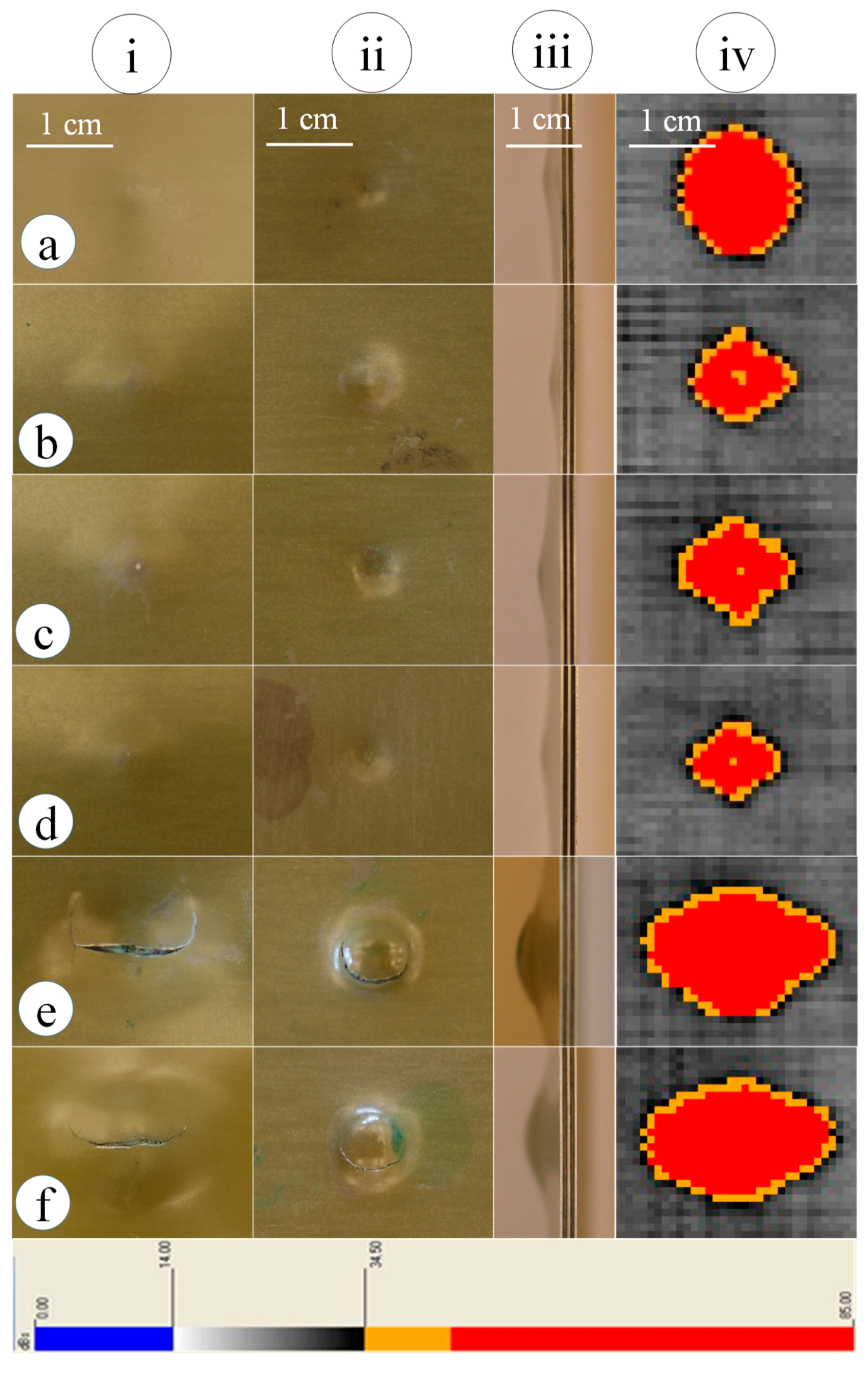
4.6. Experimental Outcomes
5. Discussion
6. Concluding Remarks
- In the non-penetration impact events at room temperature, first the GF/EP 90°/0° cohesive interface farthest from the impacted side of the GLARE model disintegrated. Because stress waves reverberated at the rear face, which augmented the cross-ply induced shear stress beyond the limit threshold upon impact. At room temperature, the brittle behavior of cohesive elements accelerated the inter-laminar interface degradation.
- At 80 °C, the GLARE model endured interface failure at the periphery of impact zone, though the panel remained coherent at the impact center. In analogy to the interface degradation at room temperature, the GF/EP 0°/90° interfaces suffered severe failures at 80 °C. Nonetheless, the delamination growth was delayed at 80 °C.
- As explicated in the experiments, FM94-epoxy behaved ductile at 75 °C. As a result, the critical strain energy release rate of FM94-epoxy was higher compared to that at room temperature. It is believed that the higher energy release rate to cohesive failure changed the stick-slip nature of interface degradation to a stable one at 75 °C. The numerical analysis, when implemented a 39% higher Gc of cohesive interfaces by contrast to that at room temperature, corroborated the stable failure of the inter-laminar interface.
- Thermal conditioning at circa 85 °C for 45 minutes reinforced the bending stiffness of GLARE. As a result, the GLARE plate deflected only locally and offered a higher impact resistance, which restricted the impact damage with the support of resin plasticization.
- For the sake of brevity, stress-based intra-ply fiber failure and matrix failure criteria were not included in the composite material model, although intra-ply matrix cracks can be the embryos of inter-laminar interface failure [74]. Scopes are there to complement the proposed model. However, if composite plies fail and are eroded prior to the failure of cohesive elements, convergence difficulties are inevitable.
- This study illustrates that a lower scale of interface failure at an elevated temperature may ascertain the residual strength of GLARE at the acceptable level. Therefore, the risk of a total collapse of a thin-walled GLARE airfoil at an anti/de-icing temperature under a soft body impact is not unexpectedly high.
- The literature review gives hundreds if not thousands of scientific articles interrogated the impact resistance and the related interface failure of GLARE and other fiber-metal laminates. Most of the studies accommodated experiments at room temperature. Thus, the question arises of whether the delineated energy partition between the different damage modes of GLARE holds also for higher operating temperatures. This study clearly shows the necessity to re-evaluate the impact performance of different GLARE grades based on the operating temperature, than just focusing on the cross-ply GLARE variant.
- The outcomes of this study allocate new fields of application for GLARE, e.g., the energy storage of aircraft or shielding against moderate temperature. The thermal energy stored in GLARE could be converted in electric power to execute the secondary operations of an aircraft. In such cases, the landing impact should not impair the interface integrity of GLARE, if the findings of this study are extrapolated in a conservative manner.
Funding
Conflicts of Interest
References
- Kim, H.; Kedward, K.T. Modeling Hail Ice Impacts and Predicting Impact Damage Initiation in Composite Structures. AIAA J. 2000, 38, 1278–1288. [Google Scholar] [CrossRef]
- McCarthy, M.A.; Xiao, J.R.; McCarthy, C.T.; Kamoulakos, A.; Ramos, J.; Gallard, J.P.; Melito, V. Modelling of Bird Strike on an Aircraft Wing Leading Edge Made from Fibre Metal Laminates – Part 2: Modelling of Impact with SPH Bird Model. Appl. Compos. Mater. 2004, 11, 317–340. [Google Scholar] [CrossRef]
- Smojver, I.; Ivančević, D. Numerical simulation of bird strike damage prediction in airplane flap structure. Compos. Struct. 2010, 92, 2016–2026. [Google Scholar] [CrossRef]
- Takeda, S.; Minakuchi, S.; Okabe, Y.; Takeda, N. Delamination monitoring of laminated composites subjected to low-velocity impact using small-diameter FBG sensors. Compos. Part A Appl. Sci. Manuf. 2005, 36, 903–908. [Google Scholar] [CrossRef]
- Vlot, A.; Gunnink, J.W. Fibre Metal Laminates. An Introduction; Springer: Dordrecht, The Netherlands, 2001; ISBN 978-94-010-0995-9. [Google Scholar]
- Guan, Z.W.; Cantwell, W.J.; Abdullah, R. Numerical modeling of the impact response of fiber-metal laminates. Polym. Compos. 2009, 30, 603–611. [Google Scholar] [CrossRef]
- Wu, G.; Yang, J.-M. The mechanical behavior of GLARE laminates for aircraft structures. JOM 2005, 57, 72–79. [Google Scholar] [CrossRef]
- Morency, F.; Tezok, F.; Paraschivoiu, I. Heat and Mass Transfer in the Case of Anti-Icing System Simulation. J. Aircr. 2000, 37, 245–252. [Google Scholar] [CrossRef]
- Morency, F.; Tezok, F.; Paraschivoiu, I. Anti-Icing System Simulation Using CANICE. J. Aircr. 1999, 36, 999–1006. [Google Scholar] [CrossRef]
- Hagenbeek, M. Characterisation of Fiber metal laminates under Thermo-mechanical Loadings. Available online: https://www.elibrary.ru/item.asp?id=9358929 (accessed on 9 March 2020).
- Zhong, Y.; Joshi, S.C. Impact behavior and damage characteristics of hygrothermally conditioned carbon epoxy composite laminates. Mater. Design (1980–2015) 2015, 65, 254–264. [Google Scholar] [CrossRef]
- Zimmerman, R.S.; Adams, R.D. (Eds.) Impact Performance of Various Fiber Reinforced Composites as a Function of Temperature; Int SAMPE Symp Exhib.: Anaheim, CA, USA, 1987. [Google Scholar]
- Levin, K. (Ed.) Effect of Low-Velocity Impact on Compression Strength of Quasi-Isotropic Laminate; 1st Technical Conference, Dayton, Ohio.; Technomic Publishing Co.: Lanchester, NH, USA, 1986. [Google Scholar]
- Botelho, E.C.; Almeida, R.S.; Pardini, L.C.; Rezende, M.C. Elastic properties of hygrothermally conditioned glare laminate. Int. J. Eng. Sci. 2007, 45, 163–172. [Google Scholar] [CrossRef]
- Botelho, E.C.; Pardini, L.C.; Rezende, M.C. Hygrothermal effects on the shear properties of carbon fiber/epoxy composites. J. Mater. Sci. 2006, 41, 7111–7118. [Google Scholar] [CrossRef]
- Liotier, P.-J.; Vautrin, A.; Beraud, J.-M. Microcracking of composites reinforced by stitched multiaxials subjected to cyclical hygrothermal loadings. Compos. Part A Appl. Sci. Manuf. 2011, 42, 425–437. [Google Scholar] [CrossRef]
- Zhong, Y.; Joshi, S.C. Impact resistance of hygrothermally conditioned composite laminates with different lay-ups. J. Compos. Mater. 2015, 49, 829–841. [Google Scholar] [CrossRef]
- Khashaba, U.A.; Othman, R. Low-velocity impact of woven CFRE composites under different temperature levels. Int. J. Impact Eng. 2017, 108, 191–204. [Google Scholar] [CrossRef]
- Hirai, Y.; Hamada, H.; Kim, J.-K. Impact response of woven glass-fabric composites—II. Effect of temperature. Compos. Sci. Technol. 1998, 58, 119–128. [Google Scholar] [CrossRef]
- Johnson, W. (Ed.) Delamination and Debonding of Materials; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1985; ISBN 978-0-8031-0414-3. [Google Scholar]
- Johnson, W.S.; Masters, J.E.; Karasek, M.L.; Strait, L.H.; Amateau, M.F.; Runt, J.P. Effect of Temperature and Moisture on the Impact Behavior of Graphite/Epoxy Composites: Part II—Impact Damage. J. Compos. Technol. Res. 1995, 17, 11. [Google Scholar] [CrossRef]
- Johnson, W.S.; Masters, J.E.; Karasek, M.L.; Strait, L.H.; Amateau, M.F.; Runt, J.P. Effect of Temperature and Moisture on the Impact Behavior of Graphite/Epoxy Composites: Part I—Impact Energy Absorption. J. Compos. Technol. Res. 1995, 17, 3. [Google Scholar] [CrossRef]
- Li, G.; Pang, S.-S.; Helms, J.E.; Ibekwe, S.I. Low velocity impact response of GFRP laminates subjected to cycling moistures. Polym. Compos. 2000, 21, 686–695. [Google Scholar] [CrossRef]
- Wyatt, R.C.; Ashbee, K.H.G. Debonding in carbon fibre/polyester resin composites exposed to water: Comparison with ‘E’ glass fibre composites. Fibre Science and Technology 1969, 2, 29–40. [Google Scholar] [CrossRef]
- Antoon, M.K.; Koenig, J.L.; Serafini, T. Fourier-transform infrared study of the reversible interaction of water and a crosslinked epoxy matrix. J. Polym. Sci. Polym. Phys. Ed. 1981, 19, 1567–1575. [Google Scholar] [CrossRef]
- Chamis, C.C. (Ed.) Test Methods and Design Allowables for Fibrous Composites; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1981; ISBN 978-0-8031-0700-7. [Google Scholar]
- Porter, T.R. Environmental Effects on Composite Fracture Behavior. In Test Methods and Design Allowables for Fibrous Composites; Chamis, C.C., Ed.; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1981; 396-396-15; ISBN 978-0-8031-0700-7. [Google Scholar]
- Shen, C.-H.; Springer, G.S. Effects of Moisture and Temperature on the Tensile Strength of Composite Materials. J. Compos. Mater. 1977, 11, 2–16. [Google Scholar] [CrossRef]
- Sun, P.; Zhao, Y.; Luo, Y.; Sun, L. Effect of temperature and cyclic hygrothermal aging on the interlaminar shear strength of carbon fiber/bismaleimide (BMI) composite. Mater. Design (1980-2015) 2011, 32, 4341–4347. [Google Scholar] [CrossRef]
- Ray, B.C. Temperature effect during humid ageing on interfaces of glass and carbon fibers reinforced epoxy composites. J. Colloid Interface Sci. 2006, 298, 111–117. [Google Scholar] [CrossRef] [PubMed]
- Asp, L.E. The effects of moisture and temperature on the interlaminar delamination toughness of a carbon/epoxy composite. Compos. Sci. Technol. 1998, 58, 967–977. [Google Scholar] [CrossRef]
- Coronado, P.; Argüelles, A.; Viña, J.; Mollón, V.; Viña, I. Influence of temperature on a carbon–fibre epoxy composite subjected to static and fatigue loading under mode-I delamination. Int. J. Solids Struct. 2012, 49, 2934–2940. [Google Scholar] [CrossRef]
- Banea, M.D.; Silva, L.F.M.d.; Campilho, R.D.S.G. Effect of Temperature on Tensile Strength and Mode I Fracture Toughness of a High Temperature Epoxy Adhesive. J. Adhes. Sci. Technol. 2012, 26, 939–953. [Google Scholar] [CrossRef]
- Charalambous, G.; Allegri, G.; Hallett, S.R. Temperature effects on mixed mode I/II delamination under quasi-static and fatigue loading of a carbon/epoxy composite. Compos. Part A Appl. Sci. Manuf. 2015, 77, 75–86. [Google Scholar] [CrossRef]
- Russell, A.J.; Street, K.N. Moisture and Temperature Effects on the Mixed-Mode Delamination Fracture of Unidirectional Graphite/Epoxy. In Delamination and Debonding of Materials; Johnson, W.S., Ed.; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1985; 349-349-22; ISBN 978-0-8031-0414-3. [Google Scholar]
- Ashcroft, I.A.; Hughes, D.J.; Shaw, S.J. Mode I fracture of epoxy bonded composite joints: 1. Quasi-static loading. Int. J. Adhes. Adhes. 2001, 21, 87–99. [Google Scholar] [CrossRef]
- Aliyu, A.A.; Daniel, I.M. Effects of Strain Rate on Delamination Fracture Toughness of Graphite/Epoxy. In Delamination and Debonding of Materials; Johnson, W.S., Ed.; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1985; 336-336-13; ISBN 978-0-8031-0414-3. [Google Scholar]
- Kim, B.W.; Mayer, A.H. Influence of fiber direction and mixed-mode ratio on delamination fracture toughness of carbon/epoxy laminates. Compos. Sci. Technol. 2003, 63, 695–713. [Google Scholar] [CrossRef]
- Benzeggagh, M.L.; Kenane, M. Measurement of mixed-mode delamination fracture toughness of unidirectional glass/epoxy composites with mixed-mode bending apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]
- Hashemi, S.; Kinloch, A.J.; Williams, G. Mixed-Mode Fracture in Fiber-Polymer Composite Laminates. In Composite Materials: Fatigue and Fracture (Third Volume); O’Brien, T.K., Ed.; ASTM International: West Conshohocken, PA, USA, 19428-2959; 1991; 143-143-26; ISBN 978-0-8031-1419-7. [Google Scholar]
- O’Brien, T.K. (Ed.) Composite Materials: Fatigue and Fracture (Third Volume); ASTM International: West Conshohocken, PA, USA, 19428-2959; 1991; ISBN 978-0-8031-1419-7. [Google Scholar]
- Feng, W.W.; Reifsnider, K.L.; Sendeckyj, G.P.; Chiao, T.T.; Rodericks, G.L.; Stinchcomb, W.W.; de Vore, L.; Hunston, D.L.; Bascom, W.D. Effects of Lay-Up, Temperature, and Loading Rate in Double Cantilever Beam Tests of Interlaminar Crack Growth. J. Compos. Technol. Res. 1983, 5, 118. [Google Scholar] [CrossRef]
- Fan, J.; Guan, Z.W.; Cantwell, W.J. Numerical modelling of perforation failure in fibre metal laminates subjected to low velocity impact loading. Compos. Struct. 2011, 93, 2430–2436. [Google Scholar] [CrossRef]
- Laliberté, J.; Straznicky, P.V.; Poon, C. Impact Damage in Fiber Metal Laminates, Part 1: Experiment. AIAA J. 2005, 43, 2445–2453. [Google Scholar] [CrossRef]
- Lu, X.; Ridha, M.; Chen, B.Y.; Tan, V.B.C.; Tay, T.E. On cohesive element parameters and delamination modelling. Eng. Fract. Mech. 2019, 206, 278–296. [Google Scholar] [CrossRef]
- Guida, M.; Marulo, F.; Meo, M.; Russo, S. Experimental Tests Analysis of Fiber Metal Laminate under Birdstrike. Mech. Adv. Mater. Struct. 2012, 19, 376–395. [Google Scholar] [CrossRef]
- Guida, M. Study Design and Testing of Structural Configurations for the Bird-Strike Compliance of Aeronautical Components; CUEN: Roma, Italy, 2008; ISBN 978-8871467658. [Google Scholar]
- Hedayati, R.; Ziaei-Rad, S. A new bird model and the effect of bird geometry in impacts from various orientations. Aerosp. Sci. Technol. 2013, 28, 9–20. [Google Scholar] [CrossRef]
- Morinière, F.D.; Alderliesten, R.C.; Sadighi, M.; Benedictus, R. An integrated study on the low-velocity impact response of the GLARE fibre-metal laminate. Compos. Struct. 2013, 100, 89–103. [Google Scholar] [CrossRef]
- Seidt, J.D.; Gilat, A. Plastic deformation of 2024-T351 aluminum plate over a wide range of loading conditions. Int. J. Solids Struct. 2013, 50, 1781–1790. [Google Scholar] [CrossRef]
- Dogan, F.; Hadavinia, H.; Donchev, T.; Bhonge, P. Delamination of impacted composite structures by cohesive zone interface elements and tiebreak contact. Open Eng. 2012, 2, 96. [Google Scholar] [CrossRef]
- Da Silva, L.F.M.; Adams, R.D. Measurement of the mechanical properties of structural adhesives in tension and shear over a wide range of temperatures. J. Adhes. Sci. Technol. 2005, 19, 109–141. [Google Scholar] [CrossRef]
- Mao, R.H.; Meguid, S.A.; Ng, T.Y. Effects of incidence angle in bird strike on integrity of aero-engine fan blade. Int. J. Crashworthiness 2009, 14, 295–308. [Google Scholar] [CrossRef]
- Monaghan, J.J. Smoothed Particle Hydrodynamics. Annu. Rev. Astron. Astrophys. 1992, 30, 543–574. [Google Scholar] [CrossRef]
- Guida, M.; Marulo, F.; Meo, M.; Grimaldi, A.; Olivares, G. SPH – Lagrangian study of bird impact on leading edge wing. Compos. Struct. 2011, 93, 1060–1071. [Google Scholar] [CrossRef]
- Harding, M.M.; Ruiz, C. The Mechanical Behaviour of Composite Materials under Impact Loading. KEM 1997, 141–143, 403–426. [Google Scholar] [CrossRef]
- Vural, M.; Ravichandran, G. Transverse Failure in Thick S2-Glass/ Epoxy Fiber-Reinforced Composites. J. Compos. Mater. 2004, 38, 609–623. [Google Scholar] [CrossRef]
- Lässig, T.; Nguyen, L.; May, M.; Riedel, W.; Heisserer, U.; van der Werff, H.; Hiermaier, S. A non-linear orthotropic hydrocode model for ultra-high molecular weight polyethylene in impact simulations. Int. J. Impact Eng. 2015, 75, 110–122. [Google Scholar] [CrossRef]
- Vo, T.P.; Guan, Z.W.; Cantwell, W.J.; Schleyer, G.K. Low-impulse blast behaviour of fibre-metal laminates. Compos. Struct. 2012, 94, 954–965. [Google Scholar] [CrossRef]
- Johnson, A.F.; Holzapfel, M. Influence of delamination on impact damage in composite structures. Compos. Sci. Technol. 2006, 66, 807–815. [Google Scholar] [CrossRef]
- Fan, C.; Jar, P.-Y.B.; Cheng, J.R. Cohesive zone with continuum damage properties for simulation of delamination development in fibre composites and failure of adhesive joints. Eng. Fract. Mech. 2008, 75, 3866–3880. [Google Scholar] [CrossRef]
- Soutis, C.; Mohamed, G.; Hodzic, A. Modelling the structural response of GLARE panels to blast load. Compos. Struct. 2011, 94, 267–276. [Google Scholar] [CrossRef]
- Yaghoubi, A.S.; Liaw, B. Effect of lay-up orientation on ballistic impact behaviors of GLARE 5 FML beams. Int. J. Impact Eng. 2013, 54, 138–148. [Google Scholar] [CrossRef]
- Yaghoubi, A.S.; Liu, Y.; Liaw, B. Low-Velocity Impact on GLARE 5 Fiber-Metal Laminates: Influences of Specimen Thickness and Impactor Mass. J. Aerosp. Eng. 2012, 25, 409–420. [Google Scholar] [CrossRef]
- Naghipour, P.; Bartsch, M.; Voggenreiter, H. Simulation and experimental validation of mixed mode delamination in multidirectional CF/PEEK laminates under fatigue loading. Int. J. Solids Struct. 2011, 48, 1070–1081. [Google Scholar] [CrossRef]
- General Civil Aviation Authority. Runway Impact during Attempted Go-Around; Preliminary report, Operator; AAIS Case no. AIFN/0008/2016; Emirates: Abu Dhabi, Arab, 2016.
- Anderson, C.E. An overview of the theory of hydrocodes. Int. J. Impact Eng. 1987, 5, 33–59. [Google Scholar] [CrossRef]
- Seo, H.; Hundley, J.; Hahn, H.T.; Yang, J.-M. Numerical Simulation of Glass-Fiber-Reinforced Aluminum Laminates with Diverse Impact Damage. AIAA J. 2010, 48, 676–687. [Google Scholar] [CrossRef]
- Caprino, G.; Spataro, G.; Del Luongo, S. Low-velocity impact behaviour of fibreglass–aluminium laminates. Compos. Part A Appl. Sci. Manuf. 2004, 35, 605–616. [Google Scholar] [CrossRef]
- Kassapoglou, C. Modeling the Effect of Damage in Composite Structures. Simplified Approaches/Christos Kassapoglou, Delft University of Technology, The Netherlands; Wiley: Chichester, West Sussex, UK, 2015; ISBN 978-1-119-01321-1. [Google Scholar]
- Chen, Q.; Guan, Z.; Li, Z.; Ji, Z.; Zhuo, Y. Experimental investigation on impact performances of GLARE laminates. Chin. J. Aeronaut. 2015, 28, 1784–1792. [Google Scholar] [CrossRef]
- Bikakis, G.S.E.; Savaidis, A.; Zalimidis, P.; Tsitos, S. Influence of the Metal Volume Fraction on the permanent dent depth and energy absorption of GLARE plates subjected to low velocity impact. IOP Conf. Ser.: Mater. Sci. Eng. 2016, 161, 12055. [Google Scholar] [CrossRef]
- Bellini, C.; Di Cocco, V.; Iacoviello, F.; Sorrentino, L. Influence of structural characteristics on the interlaminar shear strength of CFRP/Al fibre metal laminates. Procedia Struct. Integr. 2019, 18, 373–378. [Google Scholar] [CrossRef]
- Zheng, S.; Sun, C.T. A double-plate finite-element model for the impact-induced delamination problem. Compos. Sci. Technol. 1995, 53, 111–118. [Google Scholar] [CrossRef]





| Mechanical Properties of GF/EP (unidirectional ply) | ||
|---|---|---|
| Properties | at Room Temperature | at 80 °C |
| Density (kg/mm3) | 1.98 × 10−6 | 1.98 × 10−6 |
| Tensile modulus, E11 (GPa) | 54 | 42.22 |
| Tensile modulus, E22 (GPa) | 9.4 | 0.15 |
| Tensile modulus, E33 (GPa) | 9.4 | 0.15 |
| Poisson’s ratio (21) | 0.0574 | 0.0011 |
| Poisson’s ratio (31) | 0.0574 | 0.0011 |
| Poisson’s ratio (32) | 0.43 | 0.25 |
| Shear modulus, G12 (GPa) | 5.5 | 0.1 |
| Shear modulus, G13 (GPa) | 5.5 | 0.1 |
| Shear modulus, G23 (GPa) | 3.3 | 0.06 |
| Mechanical Properties of Aluminum (Al 2024-T3) | ||
|---|---|---|
| Properties | at Room Temperature | at 80 °C |
| Density (kg/mm3) | 2.78 × 10−6 | 2.78 × 10−6 |
| E-modulus (GPa) | 73.1 | 71 |
| Shear modulus (GPa) | 28 | 26 |
| Material Properties of a Cohesive Interface in a Mixed-Mode Loading Condition | ||
|---|---|---|
| Properties | At Room Temperature | At 80 °C |
| Density (kg/mm3) | 1.20 × 10−6 | 1.20 × 10−6 |
| E-modulus (GPa) | 3.9 | 2.12 |
| Critical strain energy release rate GIc, (J/mm2) | 0.0025 | 0.00363 |
| Critical strain energy release rate GIIc, (J/mm2) | 0.009 | 0.01309 |
| Peak traction in peel (T), (GPa) | 0.0082 | 0.0046 |
| Peak traction in shear (S), (GPa) | 0.0466 | 0.0348 |
| εv | 1 | 0 | −0.095 | −0.104 | −0.112 | −0.117 | −0.125 | −0.131 | −0.148 | −0.232 |
| C (GPa) | −5 | 0 | 0.294 | 1.47 | 2.94 | 4.41 | 5.88 | 7.35 | 14.7 | 73.5 |
| Specimen No. | Areal Density (mg/mm2) | Drop Height (m) | Temperature (°C) | Impact Velocity (m/s) | Actual Impact Energy (J) |
|---|---|---|---|---|---|
| (a) | 5.6 | 2 | room | 4.77 | 12.4 |
| (b) | 5.6 | 2 | 75 | 4.6 | 11.53 |
| (c) | 5.6 | 3 | room | 6.18 | 20.81 |
| (d) | 5.6 | 3 | 77.4 | 5.98 | 19.48 |
| (e) | 5.6 | 4 | room | 8.08 | 35.58 |
| (f) | 5.6 | 4 | 77 | 8.07 | 35.49 |
| Specimen No. | Temperature(°C) | Impact Velocity (m/s) | Damage Shape | Major Radius (mm) | Minor Radius (mm) | Area (mm2) | Impact Force (N) |
|---|---|---|---|---|---|---|---|
| (a) | room | 4.77 | circular | 7.6 | 7.2 | 171.87 | 4099 |
| (b) | 75 | 4.6 | pyramid | 5.6 | 5.4 | 94.98 | 4910 |
| (c) | room | 6.18 | pyramid | 6.8 | 6.4 | 136.69 | 5800 |
| (d) | 77.4 | 5.98 | pyramid | 5.6 | 4.6 | 80.91 | 7246 |
| (e) | room | 8.08 | elliptical | 11.4 | 7.6 | 272.13 | 6371 |
| (f) | 77 | 8.07 | elliptical | 11.4 | 7.2 | 257.81 | 6721 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasan, M.Z. Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike. Aerospace 2020, 7, 28. https://doi.org/10.3390/aerospace7030028
Hasan MZ. Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike. Aerospace. 2020; 7(3):28. https://doi.org/10.3390/aerospace7030028
Chicago/Turabian StyleHasan, Md.Zahid. 2020. "Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike" Aerospace 7, no. 3: 28. https://doi.org/10.3390/aerospace7030028
APA StyleHasan, M. Z. (2020). Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike. Aerospace, 7(3), 28. https://doi.org/10.3390/aerospace7030028




