Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation
Abstract
1. Introduction
2. Problem Formulation
2.1. Uncoupled Natural Vibration Modes
2.2. Unsteady Aerodynamic Model
2.3. Modal Approach and Stability Analysis
2.4. Sensitivity Analysis
3. Lower-Fidelity Model
Aero-Structural Parametric Derivatives
4. Higher-Fidelity Model
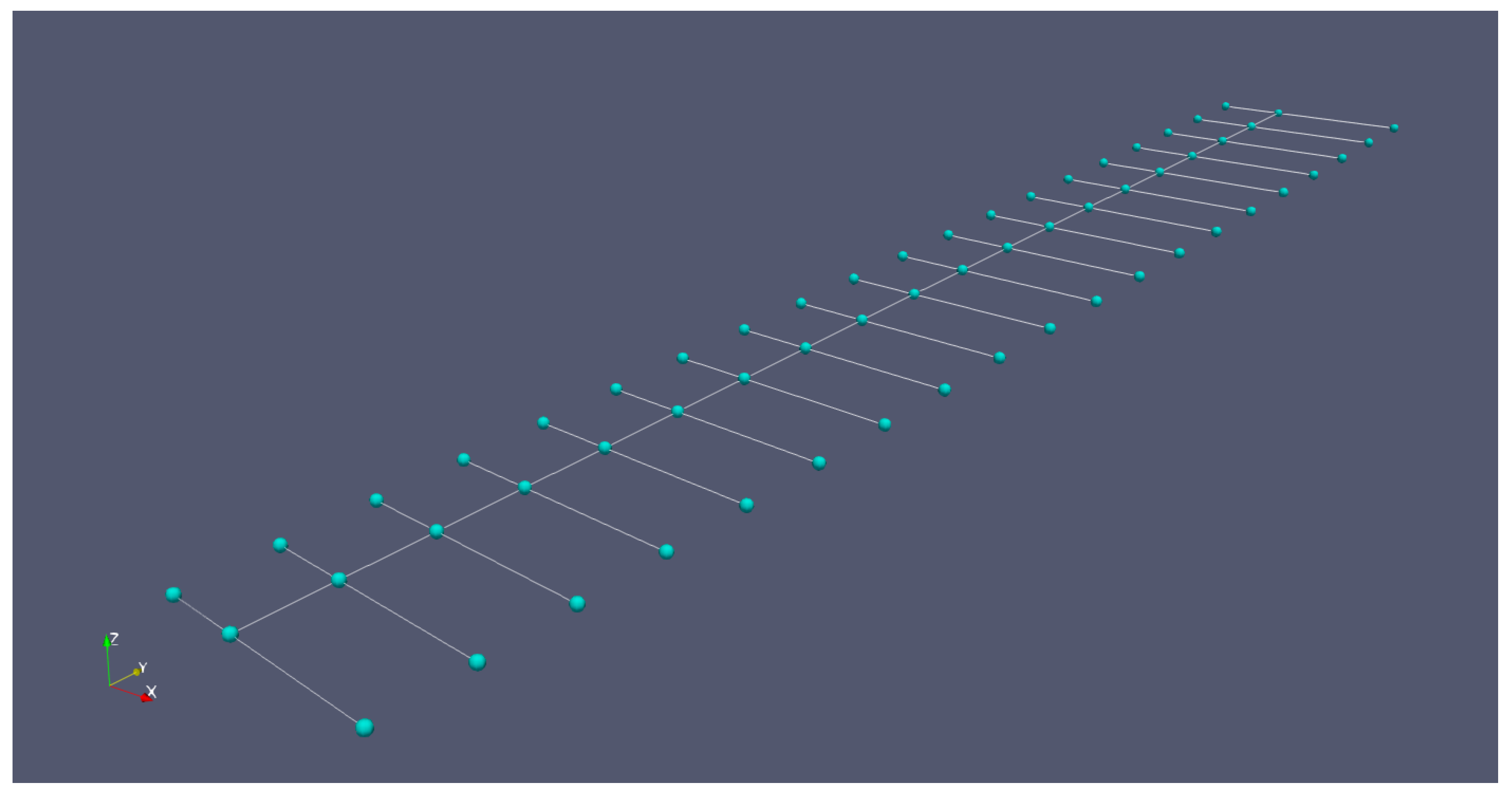
4.1. Structural Model
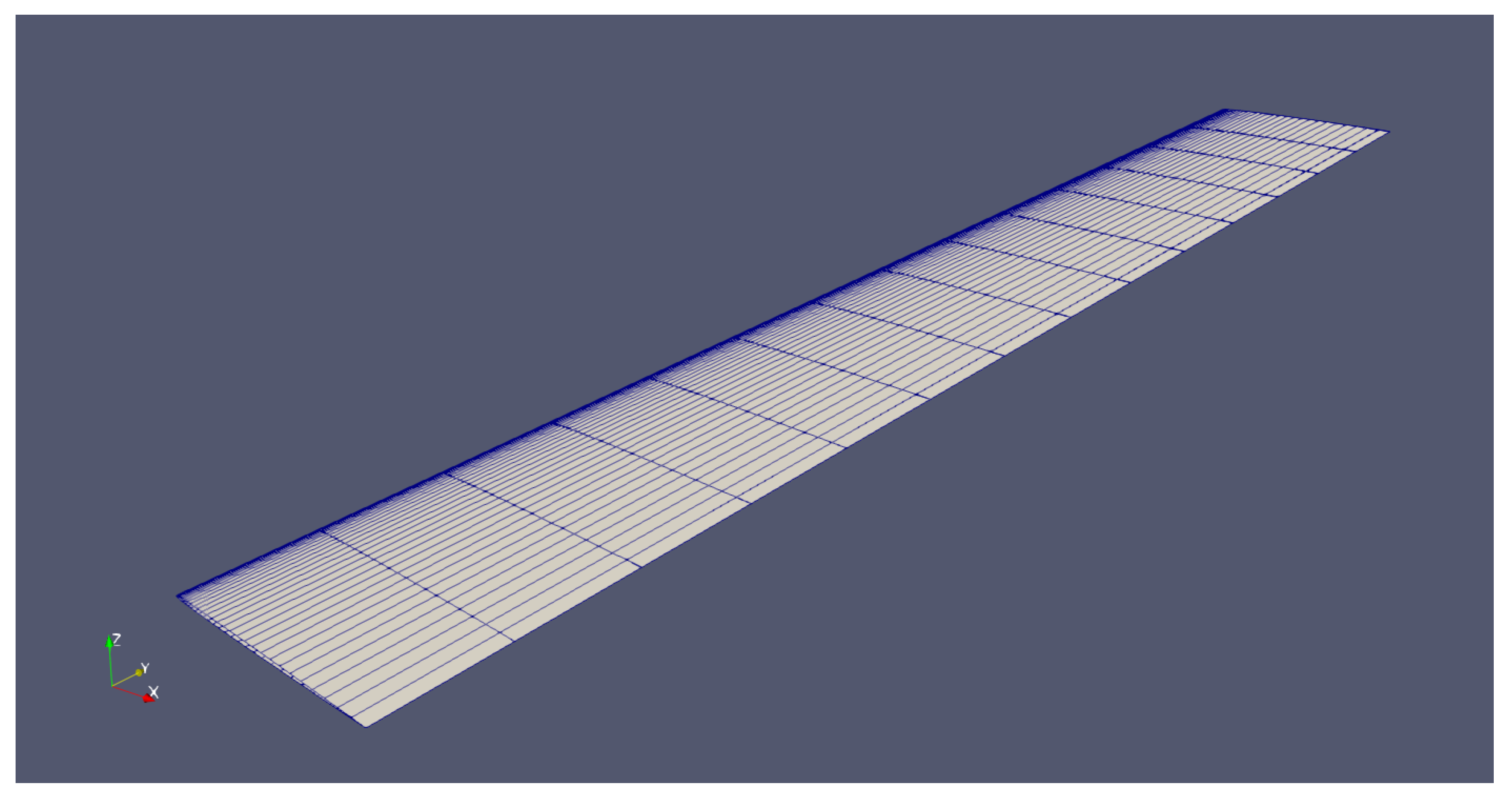
4.2. Aerodynamic Model
4.3. Aeroelastic Model
4.4. Aero-Structural Parametric Derivatives
5. Results and Discussion
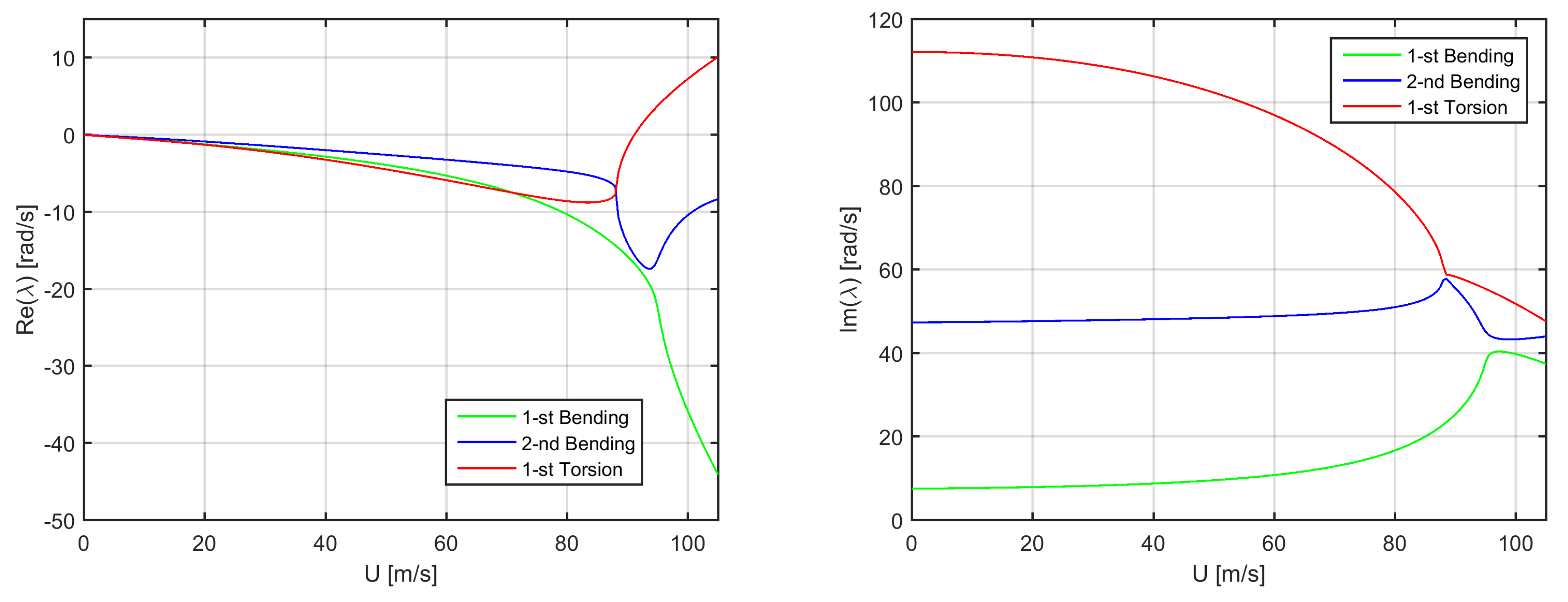
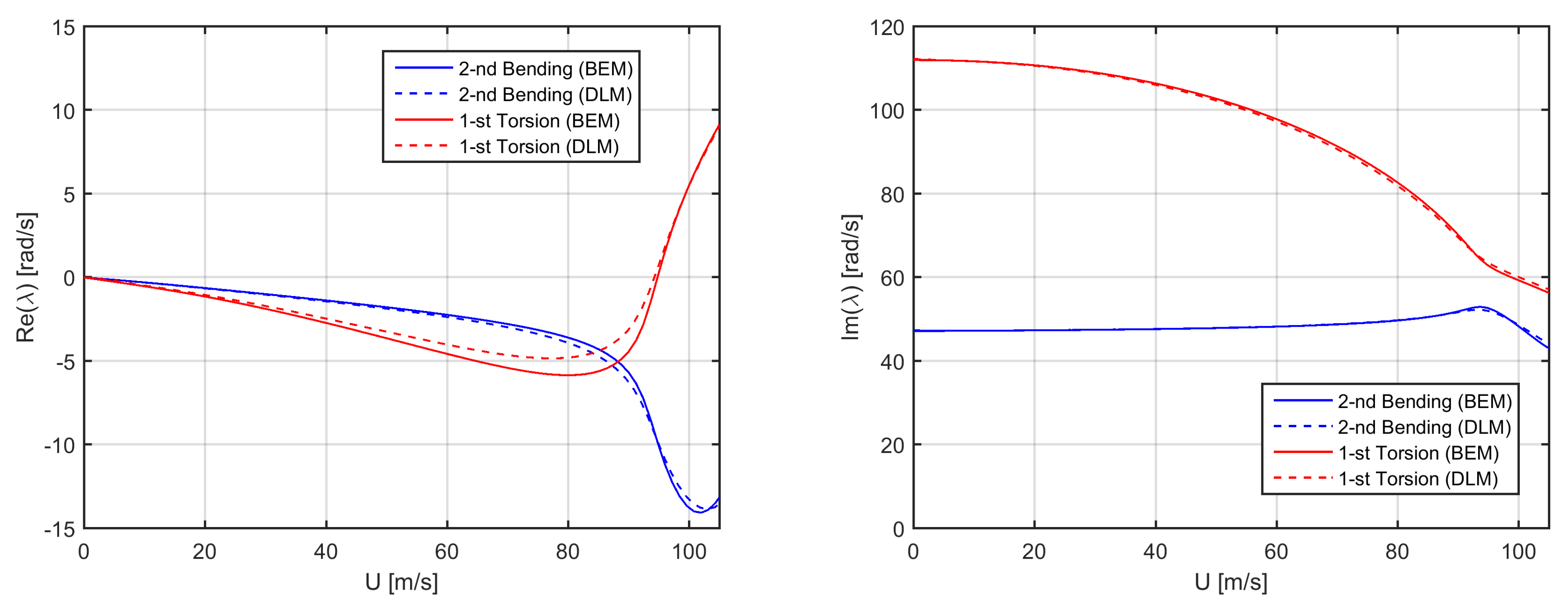
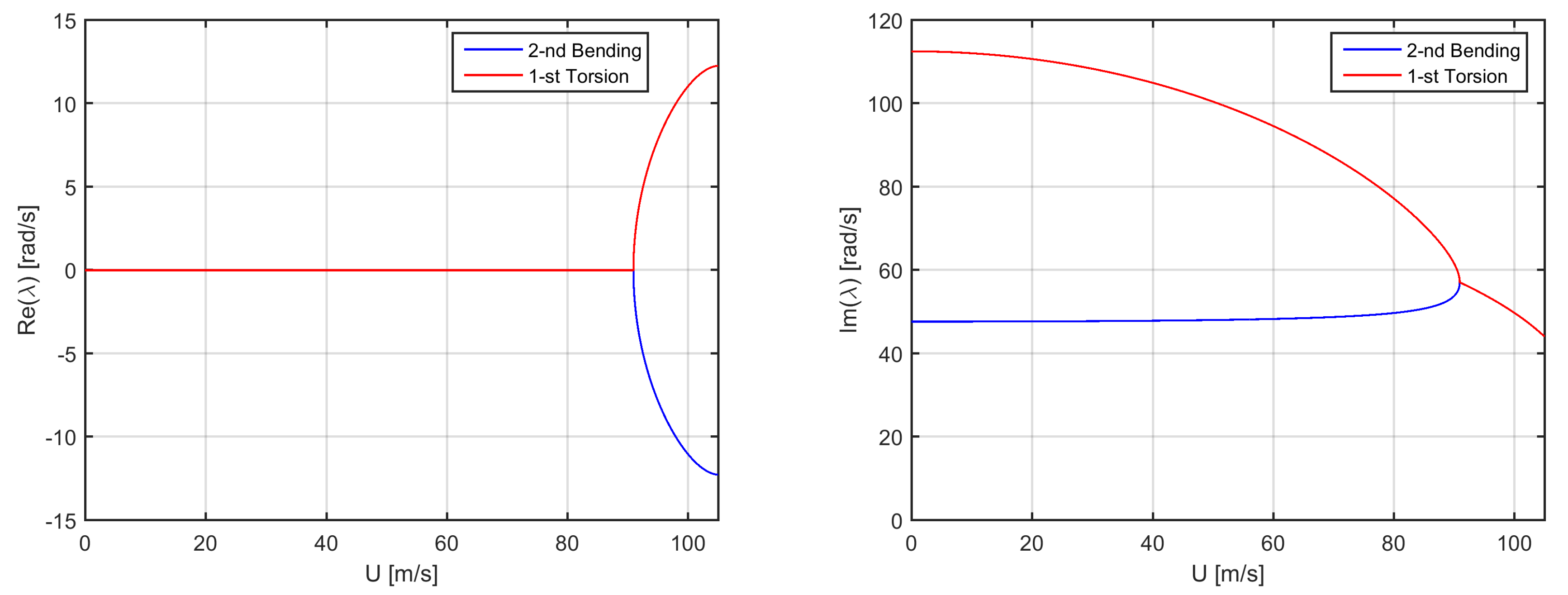
5.1. Aeroelastic Analyses
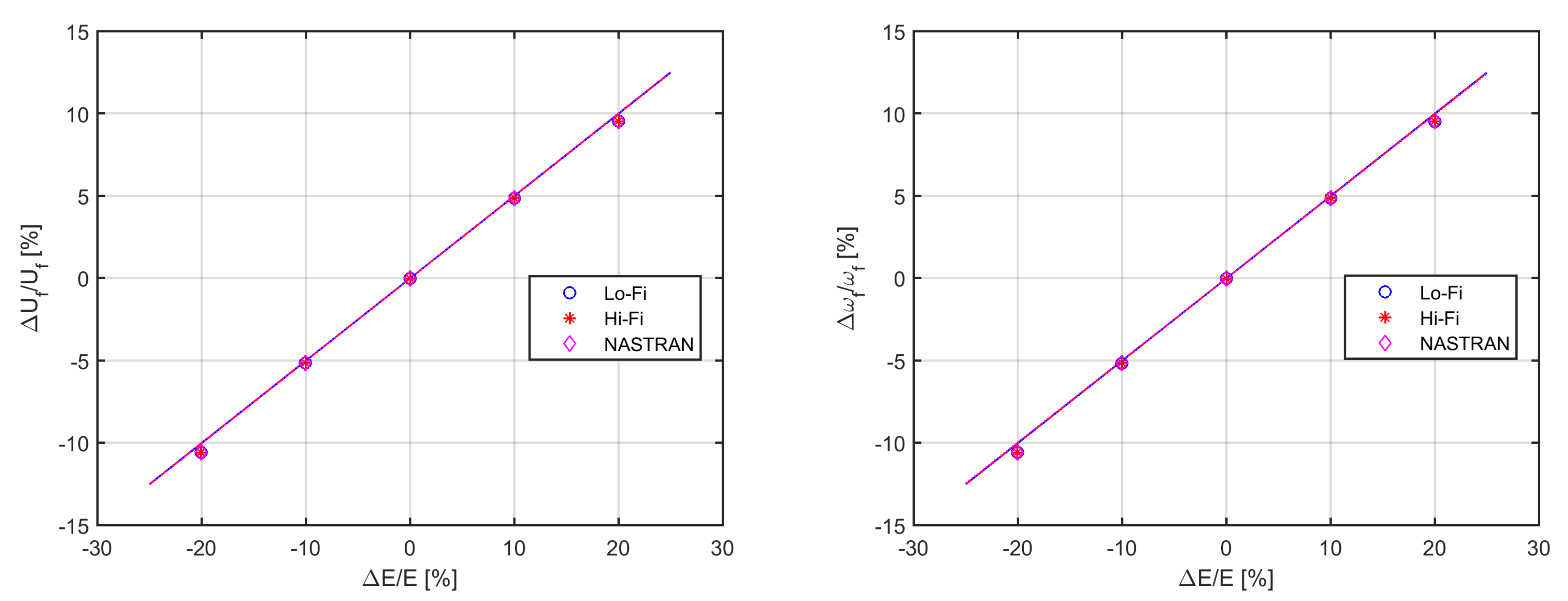
5.2. Sensitivity Study
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbols | |
| A | aerodynamic panel area |
| c | section chord |
| section lift | |
| section lift derivative | |
| wing lift derivative | |
| pressure coefficient | |
| Theodorsen’s function | |
| generalised damping matrix | |
| aerodynamic approximation matrix (fraction denominator) | |
| e | semiperimeter-to-span ratio |
| E | section Young’s elastic modulus |
| f | cross-projection of first bending and first torsion modes |
| generalised load vector | |
| g | cross-projection of second bending and first torsion modes |
| G | section shear elastic modulus |
| h | section flexural (plunge) displacement |
| Hankel’s functions of the second type and n-th order | |
| I | section flexural area moments of inertia |
| J | section torsional area moments of inertia |
| k | reduced frequency |
| k | equivalent spring stiffness |
| generalised stiffness matrix | |
| l | wing semi-span |
| section aerodynamic force | |
| m | section mass |
| section aerodynamic moment | |
| generalised mass matrix | |
| aerodynamic panel normal vector | |
| aerodynamic approximation matrix (fraction numerator) | |
| p | design parameter |
| generalised aerodynamic forces matrix | |
| r | squared ratio of second and first flexural vibration frequencies |
| s | complex reduced frequency |
| system matrix | |
| t | time |
| aerodynamic influence coefficients matrix | |
| eigenvector | |
| U | horizontal airspeed |
| V | vertical airspeed |
| x | chordwise coordinate |
| y | spanwise coordinate |
| w | section vertical displacement |
| Greek | |
| angle of attack | |
| generalised coordinates | |
| aerofoil thickness ratio | |
| natural vibration mode shape | |
| flexural natural vibration constant | |
| wing aspect ratio | |
| aerodynamic load scaling function | |
| eigenvalue | |
| section mass moment of inertia | |
| torsional natural vibration constant | |
| section torsional (pitch) displacement | |
| normal wash matrix | |
| air density | |
| material density | |
| aerodynamic potential matrix | |
| reduced time | |
| natural vibration frequency | |
| Subscripts | |
| A | aerodynamic |
| c | critical |
| f | flutter |
| d | divergence |
| h | flexural |
| S | structural |
| torsional | |
| Acronyms | |
| AC | aerodynamic centre |
| AIC | aerodynamic influence coefficient |
| BEM | boundary element method |
| CFD | computational fluid dynamics |
| CG | centre of gravity |
| CP | control point |
| CSRD | closely-spaced rigid diaphragm |
| DLM | doublet lattice method |
| EA | elastic axis |
| FEM | finite element method |
| FSI | fluid-structure interaction |
| GAF | generalised aerodynamic forces |
| IPS | infinite plate spline |
| MAC | modal assurance criterion |
| MC | mid-chord |
| MDO | multidisciplinary design and optimisation |
| MFA | matrix fraction approach |
| MST | modified strip theory |
| ODE | ordinary differential equation |
| PDE | partial differential equation |
| QST | quasi-steady theory |
| RFA | rational function approximation |
| ROM | reduced order model |
| SST | standard strip theory |
| TST | tuned strip theory |
Appendix A. Aeroelastic Stability of the Typical Section with Steady Aerodynamics
Appendix B. Higher-Fidelity Model Results Convergence Study



References
- Alexandrov, N.; Hussaini, M. Multidisciplinary Design Optimization: State of the Art; SIAM: Philadelphia, PA, USA, 1997. [Google Scholar]
- Martins, J.; Lambe, A. Multidisciplinary Design Optimization: A Survey of Architectures. AIAA J. 2013, 51, 2049–2075. [Google Scholar] [CrossRef]
- Quarteroni, A.; Rozza, G. Reduced Order Methods for Modeling and Computational Reduction; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Qu, Z. Model Order Reduction Techniques with Applications in Finite Element Analysis; Springer: London, UK, 2004. [Google Scholar]
- Ghoreyshi, M.; Jirasek, A.; Cummings, R. Reduced Order Unsteady Aerodynamic Modeling for Stability and Control Analysis Using Computational Fluid Dynamics. Prog. Aerosp. Sci. 2014, 71, 167–217. [Google Scholar] [CrossRef]
- Livne, E. Integrated Aeroservoelastic Optimization: Status and Direction. J. Aircr. 1999, 36, 122–145. [Google Scholar] [CrossRef]
- Cavagna, L.; Ricci, S.; Travaglini, L. NeoCASS: An Integrated Tool for Structural Sizing, Aeroelastic Analysis and MDO at Conceptual Design Level. Prog. Aerosp. Sci. 2011, 47, 621–635. [Google Scholar] [CrossRef]
- Silva, W. AEROM: NASA’s Unsteady Aerodynamic and Aeroelastic Reduced-Order Modeling Software. Aerospace 2018, 5, 41. [Google Scholar] [CrossRef]
- Cardani, C.; Mantegazza, P. Calculation of Eigenvalue and Eigenvector Derivatives for Algebraic Flutter and Divergence Eigenproblems. AIAA J. 1979, 17, 408–412. [Google Scholar] [CrossRef]
- Crema, L.B.; Mastroddi, F.; Coppotelli, G. Aeroelastic Sensitivity Analyses for Flutter Speed and Gust Response. J. Aircr. 2000, 37, 172–180. [Google Scholar] [CrossRef]
- Martins, J.; Hwang, J. Review and Unification of Methods for Computing Derivatives of Multidisciplinary Computational Models. AIAA J. 2013, 51, 2582–2599. [Google Scholar] [CrossRef]
- Hodges, D.; Pierce, G. Introduction to Structural Dynamics and Aeroelasticity; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Berci, M. Semi-Analytical Static Aeroelastic Analysis and Response of Flexible Subsonic Wings. Appl. Math. Comput. 2015, 267, 148–169. [Google Scholar] [CrossRef]
- Ripepi, M.; Görtz, S. Reduced Order Models for Aerodynamic Applications, Loads and MDO. In Proceedings of the Deutscher Luft- und Raumfahrtkongress, Braunschweig, Germany, 13–15 September 2016. [Google Scholar]
- Bungartz, H.; Schafer, M. Fluid-Structure Interaction: Modelling, Simulation, Optimization; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Dhatt, G.; Touzot, G.; Lefrancois, E. Finite Element Method; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Chung, T. Computational Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Farhat, C.; Lesoinne, M.; LeTallec, P. Load and Motion Transfer Algorithms for Fluid/Structure Interaction Problems with Non-Matching Discrete Interfaces: Momentum and Energy Conservation, Optimal Discretization and Application to Aeroelasticity. Comput. Methods Appl. Mech. Eng. 1998, 157, 95–114. [Google Scholar] [CrossRef]
- Cizmas, P.; Gargoloff, J. Mesh Generation and Deformation Algorithm for Aeroelasticity Simulations. J. Aircr. 2008, 45, 1062–1066. [Google Scholar] [CrossRef]
- Berci, M.; Toropov, V.V.; Hewson, R.W.; Gaskell, P.H. Multidisciplinary Multifidelity Optimisation of a Flexible Wing Aerofoil with Reference to a Small UAV. Struct. Multidiscip. Optim. 2014, 50, 683–699. [Google Scholar] [CrossRef]
- Berci, M.; Cavallaro, R. A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment. Aerospace 2018, 5, 76. [Google Scholar] [CrossRef]
- Bisplinghoff, R.; Ashley, H.; Halfman, R. Aeroelasticity; Dover: Mineola, NY, USA, 1996. [Google Scholar]
- Hancock, G.; Wright, J.; Simpson, A. On the Teaching of the Principles of Wing Flexure-Torsion Flutter. Aeronaut. J. 1985, 89, 285–305. [Google Scholar]
- Dennis, S. Undergraduate Aeroelasticity: The Typical Section Idealization Re-Examined. Int. J. Mech. Eng. Educ. 2013, 41, 72–91. [Google Scholar] [CrossRef]
- Loring, S. Use of Generalized Coordinates in Flutter Analysis. SAE Trans. 1944, 52, 113–132. [Google Scholar]
- Abbott, I.; von Doenhoff, A. Theory of Wing Sections: Including a Summary of Aerofoil Data; Dover: New York, NY, USA, 1945. [Google Scholar]
- Banerjee, J. Flutter Sensitivity Studies of High Aspect Ratio Aircraft Wings. WIT Trans. Built Environ. 1993, 2, 683–699. [Google Scholar]
- Issac, J.; Kapania, R.; Barthelemy, J. Sensitivity Analysis of Flutter Response of a Wing Incorporating Finite-Span Corrections; NASA-CR-202089; NASA: Washington, DC, USA, 1994. [Google Scholar]
- Livne, E. Future of Airplane Aeroelasticity. J. Aircr. 2003, 40, 1066–1092. [Google Scholar] [CrossRef]
- Wright, J.; Cooper, J. Introduction to Aircraft Aeroelasticity and Loads; Wiley: Chichester, UK, 2014. [Google Scholar]
- Yang, B. Strain, Stress and Structural Dynamics; Elsevier: London, UK, 2005. [Google Scholar]
- Guo, S. Aeroelastic Optimisation of an Aerobatic Aircraft Wing Structure. Aerosp. Sci. Technol. 2003, 11, 396–404. [Google Scholar] [CrossRef]
- Karamcheti, K. Principles of Ideal-Fluid Aerodynamics; Wiley: New York, NY, USA, 1967. [Google Scholar]
- Diederich, F. A Plan-Form Parameter for Correlating Certain Aerodynamic Characteristics of Swept Wings; NACA-TN-2335; NACA: Washington, DC, USA, 1951. [Google Scholar]
- Theodorsen, T. General Theory of Aerodynamic Instability and the Mechanism of Flutter; NACA-TR-496; NACA: Washington, DC, USA, 1935. [Google Scholar]
- Jones, R. Correction of the Lifting-Line Theory for the Effect of the Chord. In NACA-TN-817; NACA: Washington, DC, USA, 1941. [Google Scholar]
- Katz, J.; Plotkin, A. Low Speed Aerodynamics; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Stanford, B.K. Role of Unsteady Aerodynamics During Aeroelastic Optimization. AIAA J. 2015, 53, 3826–3831. [Google Scholar] [CrossRef]
- van Zyl, L. Aeroelastic Divergence and Aerodynamic Lag Roots. J. Aircr. 2001, 38, 586–588. [Google Scholar] [CrossRef]
- Leishman, J. Principles of Helicopter Aerodynamics; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Fung, Y. An Introduction to the Theory of Aeroelasticity; Dover: Mineola, NY, USA, 1993. [Google Scholar]
- van Zyl, L. Use of Eigenvectors in the Solution of the Flutter Equation. J. Aircr. 1993, 30, 553–554. [Google Scholar] [CrossRef]
- Dimitriadis, G. Introduction to Nonlinear Aeroelasticity; Wiley: Chichester, UK, 2017. [Google Scholar]
- Vanderplaats, G. Numerical Optimization Techniques for Engineering Design: With Applications; McGraw Hill: New York, NY, USA, 1984. [Google Scholar]
- Kennedy, G.; Martins, J. A Parallel Finite-Element Framework for Large-Scale Gradient-Based Design Optimization of High-Performance Structures. Finite Elem. Anal. Des. 2014, 87, 56–73. [Google Scholar] [CrossRef]
- Stanford, B.; Dunning, P. Optimal Topology of Aircraft Rib and Spar Structures Under Aeroelastic Loads. J. Aircr. 2015, 52, 1298–1311. [Google Scholar] [CrossRef]
- Kennedy, G.; Martins, J. A Parallel Aerostructural Optimization Framework for Aircraft Design Studies. Struct. Multidiscip. Optim. 2014, 50, 1079–1101. [Google Scholar] [CrossRef]
- Seyranian, A. Sensitivity Analysis and Optimization of Aeroelastic Stability. Int. J. Solids Struct. 1982, 18, 791–807. [Google Scholar] [CrossRef]
- Pedersen, P.; Seyranian, A. Sensitivity Analysis for Problems of Dynamic Stability. Int. J. Solids Struct. 1983, 19, 315–335. [Google Scholar] [CrossRef]
- Cardani, C.; Mantegazza, P. Continuation and Direct Solution of the Flutter Equation. Comput. Struct. 1978, 8, 185–192. [Google Scholar]
- Bindolino, G.; Mantegazza, P. Aeroelastic Derivatives as a Sensitivity Analysis of Nonlinear Equations. AIAA J. 1987, 25, 1145–1146. [Google Scholar] [CrossRef]
- Bisplinghoff, R.; Ashley, H. Principles of Aeroelasticity; Dover: Mineola, NY, USA, 2013. [Google Scholar]
- Rodden, W.; Johnson, E. MSC/NASTRAN Aeroelastic Analysis User’s Guide; MSC Software Corporation: Newport Beach, CA, USA, 1994. [Google Scholar]
- Rodden, W.; Harder, R.; Bellinger, E. Aeroelastic Addition to NASTRAN; NASA-CR-3094; NASA: Washington, DC, USA, 1979. [Google Scholar]
- Morino, L. A General Theory of Unsteady Compressible Potential Aerodynamics; NASA-CR-2464; NASA: Washington, DC, USA, 1974. [Google Scholar]
- Morino, L.; Chen, L.; Suciu, E. Steady and Oscillatory Subsonic and Supersonic Aerodynamics around Complex Configurations. AIAA J. 1975, 13, 368–374. [Google Scholar] [CrossRef]
- Megson, T. Aircraft Structures for Engineering Students; Elsevier: Oxford, UK, 2007. [Google Scholar]
- Lanczos, C. An Iteration Method for the Solution of the Eigenvalue Problem of Linear Differential and Integral Operators. J. Res. Natl. Bur. Stand. 1950, 45, 255–282. [Google Scholar] [CrossRef]
- Albano, E.; Rodden, W. A Doublet-Lattice Method for Calculating Lift Distributions on Oscillating Surfaces in Subsonic Flows. AIAA J. 1969, 7, 279–285. [Google Scholar] [CrossRef]
- Rodden, W.; Taylor, P.; McIntosh, S. Further Refinement of the Subsonic Doublet-Lattice Method. J. Aircr. 1998, 35, 720–727. [Google Scholar] [CrossRef]
- Whitham, G. Linear and Nonlinear Waves; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Bindolino, G.; Mantegazza, P. Improvements on a Green’s Function Method for the Solution of Linearized Unsteady Potential Flows. J. Aircr. 1987, 24, 355–361. [Google Scholar] [CrossRef]
- Chen, P. ZAERO User’s Manual; ZONA Technology Incorporated: Scottsdale, AZ, USA, 2018. [Google Scholar]
- Torrigiani, F.; Ciampa, P.D. Development of an Unsteady Aeroelastic Module for a Collaborative Aircraft MDO. In Proceedings of the Multidisciplinary Analysis and Optimization Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar]
- Miranda, I.; Soviero, P. Indicial Response of Thin Wings in a Compressible Subsonic Flow. In Proceedings of the 18th International Congress of Mechanical Engineering, Ouro Preto, Brazil, 6–11 November 2005. [Google Scholar]
- Maskew, B. Program VSAERO Theory Document; NASA-CR-4023; NASA: Washington, DC, USA, 1987. [Google Scholar]
- Murua, J.; Martínez, P.; Climent, H.; van Zylc, L.; Palaciosd, R. T-Tail Flutter: Potential-Flow Modelling, Experimental Validation and Flight Tests. Prog. Aerosp. Sci. 2014, 71, 54–84. [Google Scholar] [CrossRef]
- Rodden, W. Theoretical and Computational Aeroelasticity; Crest Publishing: Los Angeles, CA, USA, 2011. [Google Scholar]
- Walther, J.; Gastaldi, A.A.; Maierl, R. Integration Aspects of the Collaborative Aero-Structural Design of an Unmanned Aerial Vehicle. CEAS Aeronaut. J. 2020, 11, 217–227. [Google Scholar] [CrossRef]
- Jones, K.; Platzer, M. Airfoil Geometry and Flow Compressibility Effects on Wings and Blade Flutter. Aerosp. Res. Cent. 1998. [Google Scholar] [CrossRef]
- Morino, L.; Troia, R.D.; Ghiringhelli, G.L.; Mantegazza, P. Matrix Fraction Approach for Finite-State Aerodynamic Modeling. AIAA J. 1995, 33, 703–711. [Google Scholar] [CrossRef]
- Roger, K. Airplane Math Modelling Methods for Active Control Design. AGARD 1977, 28, 1–11. [Google Scholar]
- Torrigiani, F.; Walther, J.-N.; Bombardieri, R.; Cavallaro, R.; Ciampa, P.D. Flutter Sensitivity Analysis for Wing Planform Optimization. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics IFASD 2019, Savannah, GA, USA, 9–13 June 2019. [Google Scholar]
- Venkatesan, C.; Friedmann, P. New Approach to Finite-State Modeling of Unsteady Aerodynamics. AIAA J. 1986, 24, 1889–1897. [Google Scholar] [CrossRef]
- Allemang, R. The Modal Assurance Criterion—Twenty Years of Use and Abuse. Sound Vib. 2003, 37, 14–23. [Google Scholar]
- Harder, R.; Desmarais, R. Interpolation Using Surface Splines. J. Aircr. 1972, 9, 189–191. [Google Scholar] [CrossRef]
- Hassig, H. An Approximate True Damping Solution of the Flutter Equation by Determinant Iteration. J. Aircr. 1971, 8, 885–889. [Google Scholar] [CrossRef]
- Squire, W.; Trapp, G. Using Complex Variables to Estimate Derivatives of Real Functions. SIAM Rev. 1998, 40, 110–112. [Google Scholar] [CrossRef]
- Dowell, E. A Modern Course in Aeroelasticity; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
- Bellinger, D.; Pototzky, T. A Study of Aerodynamic Matrix Numerical Condition. In Proceedings of the 3rd MSC Worldwide Aerospace Conference and Technology Showcase, Toulouse, France, 16–24 September 2001. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berci, M.; Torrigiani, F. Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation. Aerospace 2020, 7, 161. https://doi.org/10.3390/aerospace7110161
Berci M, Torrigiani F. Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation. Aerospace. 2020; 7(11):161. https://doi.org/10.3390/aerospace7110161
Chicago/Turabian StyleBerci, Marco, and Francesco Torrigiani. 2020. "Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation" Aerospace 7, no. 11: 161. https://doi.org/10.3390/aerospace7110161
APA StyleBerci, M., & Torrigiani, F. (2020). Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation. Aerospace, 7(11), 161. https://doi.org/10.3390/aerospace7110161




