Analysis of the Synchronized Locking Dynamic Characteristics of a Dual-Sidestay Main Landing Gear Retraction Mechanism
Abstract
1. Introduction
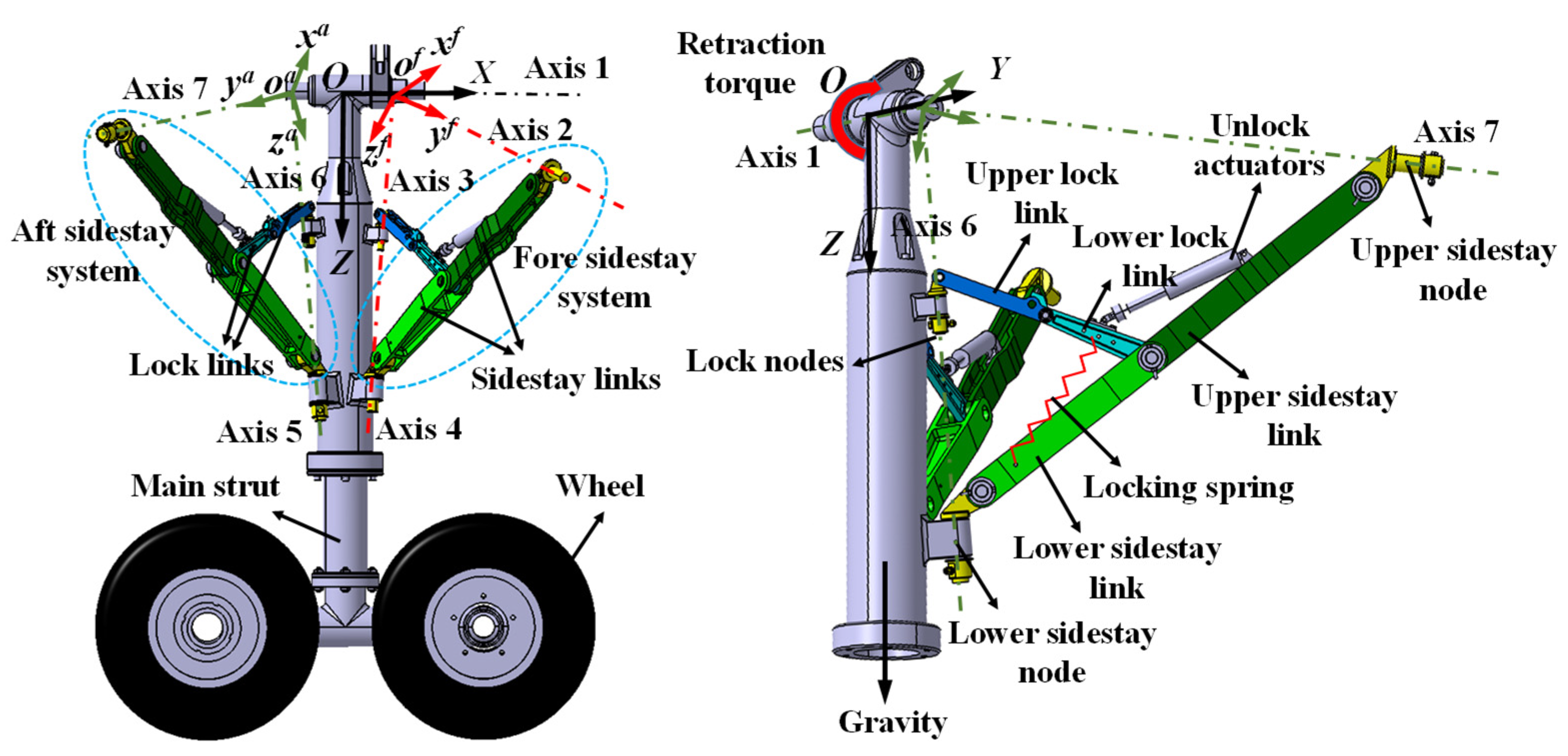
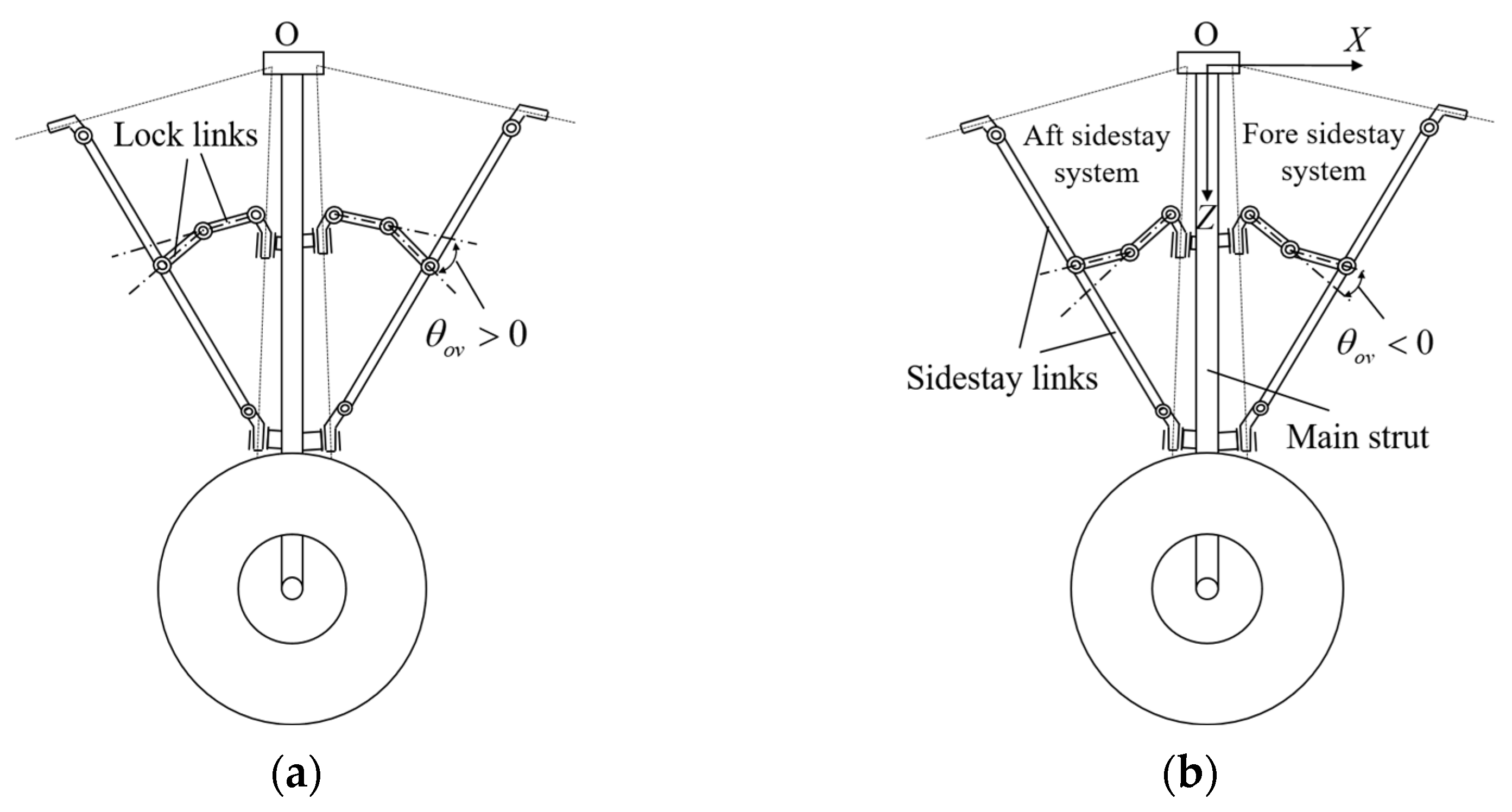
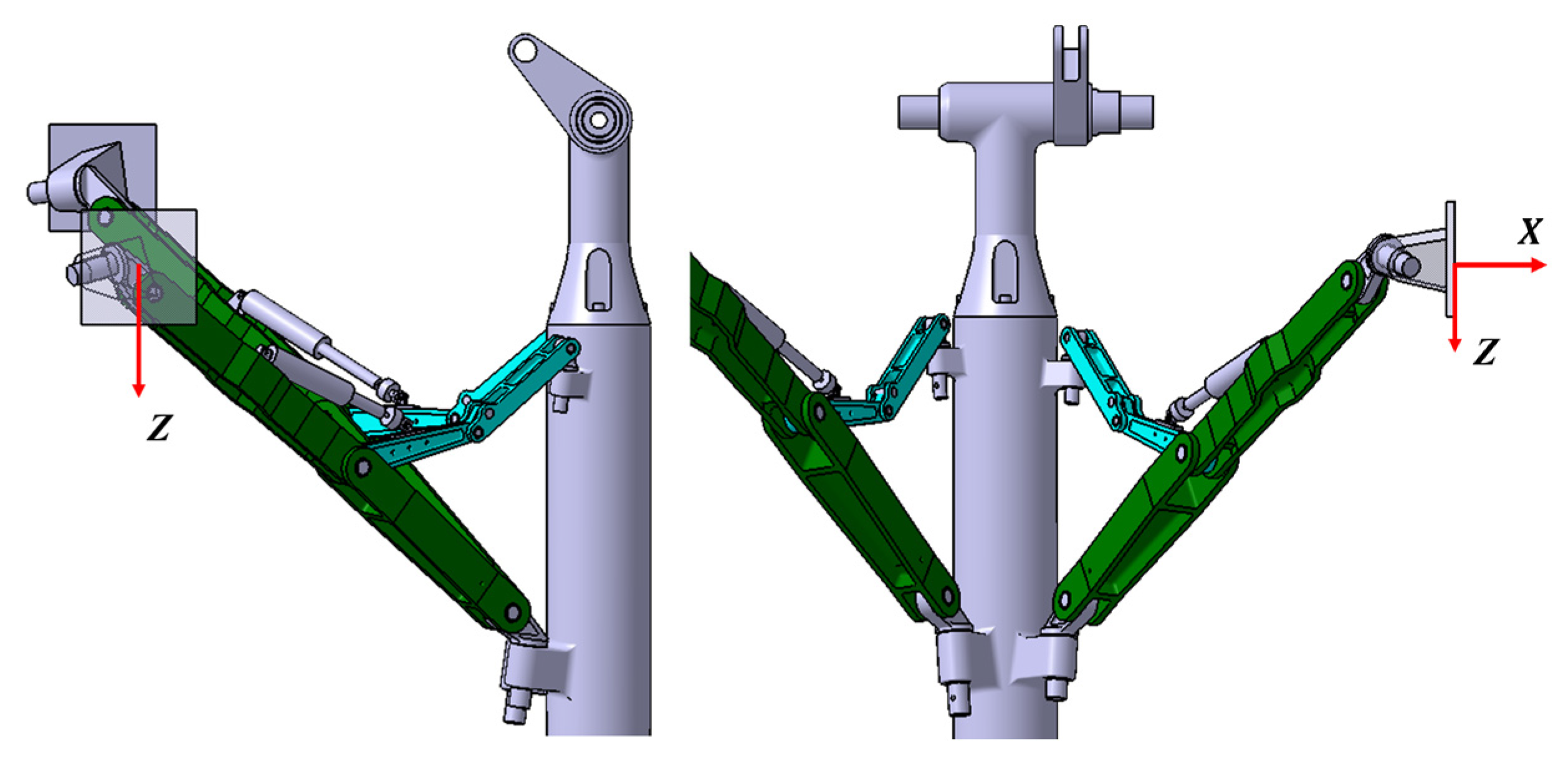
2. Composition and Principle of DSS Landing Gear
3. Dynamic Simulation and Experimental Verification of the Landing Gear Extension Process
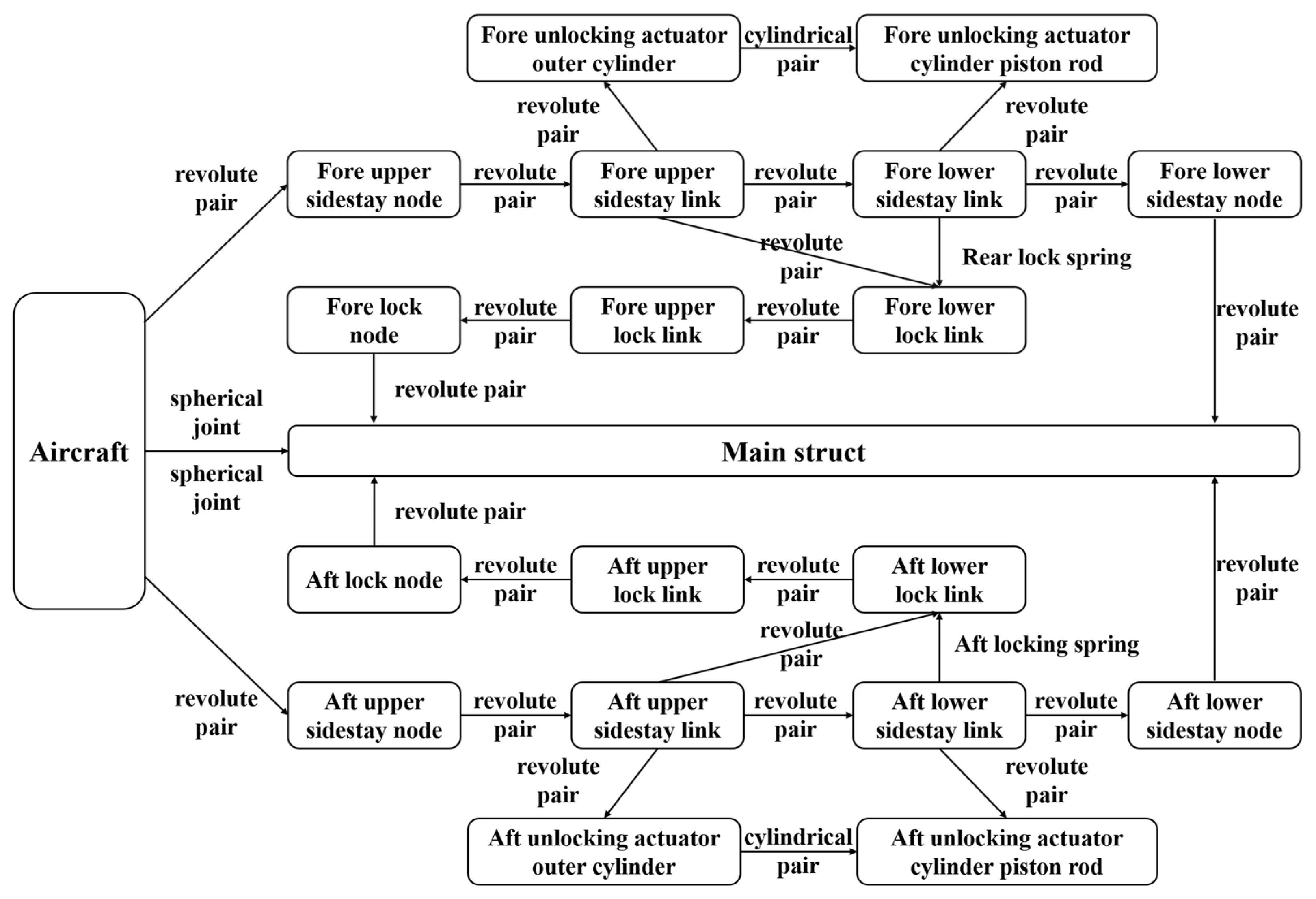
3.1. Dynamic Simulation Model of the Landing Gear Retraction Mechanism
3.1.1. Multi-Rigid-Body Dynamic Simulation Model
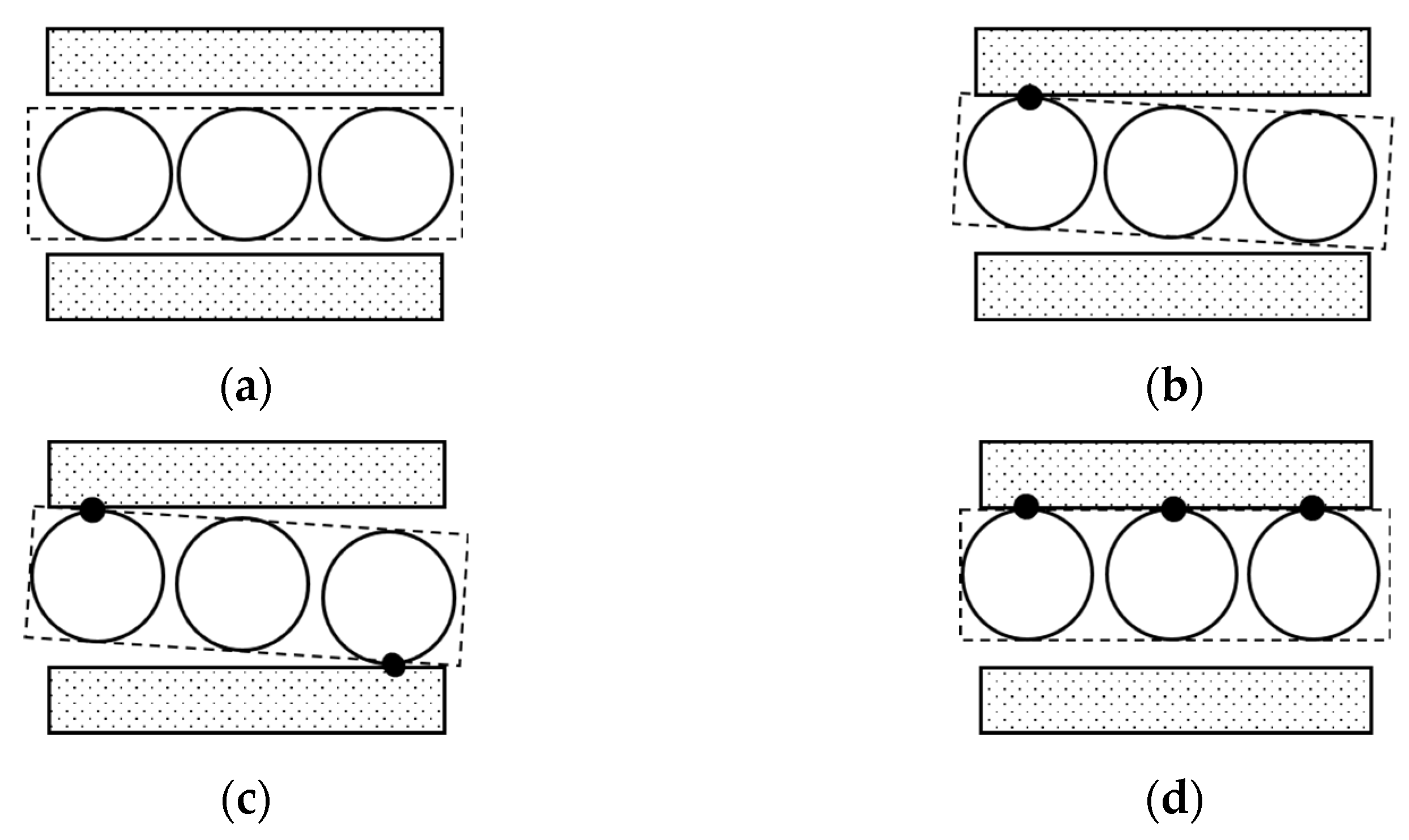
3.1.2. Landing Gear Joint Clearance Model
- Hole–shaft Clearance Model
- 2.
- Contact Force Model
- Normal contact force:
- Tangential friction force:
3.1.3. Rigid–Flexible-Coupling Model of Landing Gear Retraction Mechanism
- In the HyperMesh 2021 finite element analysis software, the finite element mesh of each component to be flexible is established, and the corresponding material properties are given. The MPC point is established at the constraint connection, the boundary conditions are defined, and the flexible file is derived.
- The flexible file is imported into the LMS Virtual.Lab software, and the Nastran Craig–Bampton modal set is established, respectively. The modal information of each flexible component is calculated by NX.Nastran for LMS 8.5 software, the first six free modes are cancelled, the last ten modes are selected, and the corresponding modal damping rate is given.
- The flexible and rigid components are assembled into a complete model in LMS Virtual.Lab, and the rigid–flexible-coupling dynamic simulation model of the DSS landing gear is obtained, as shown in Figure 6.
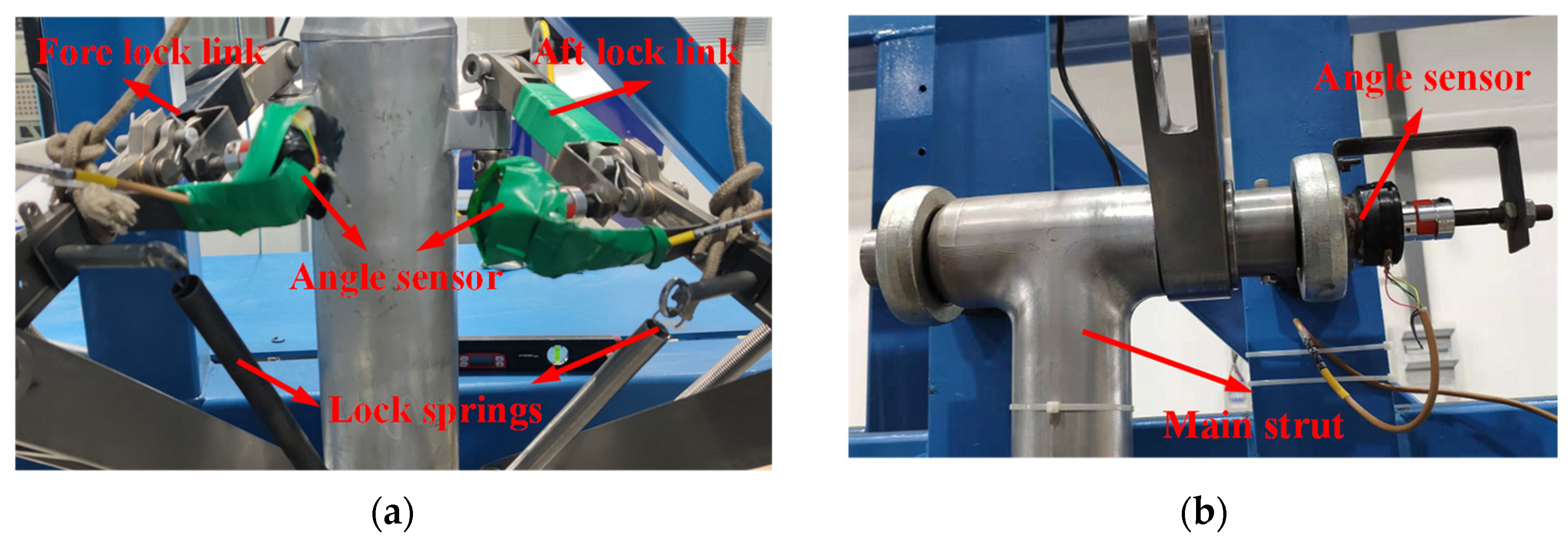
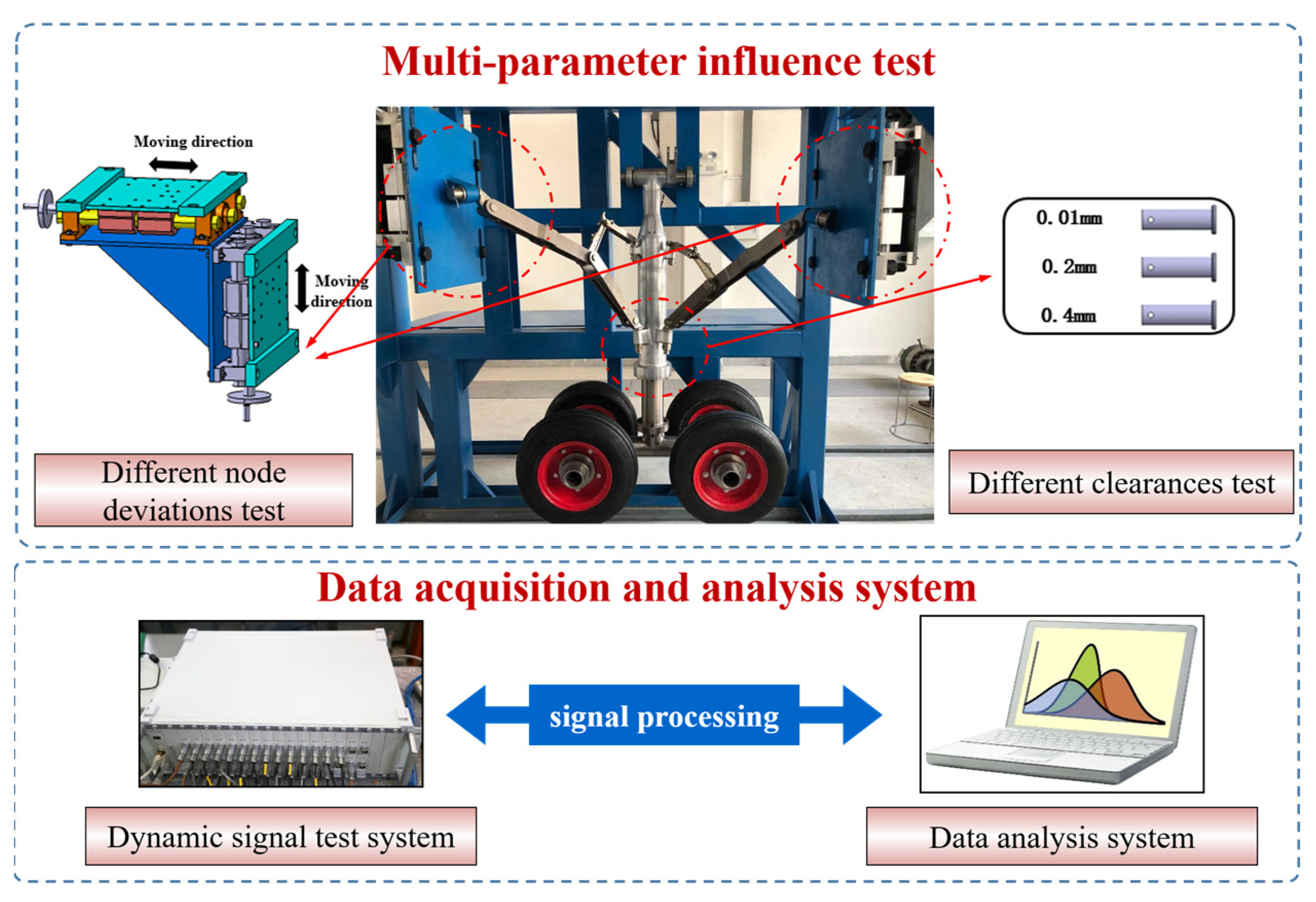
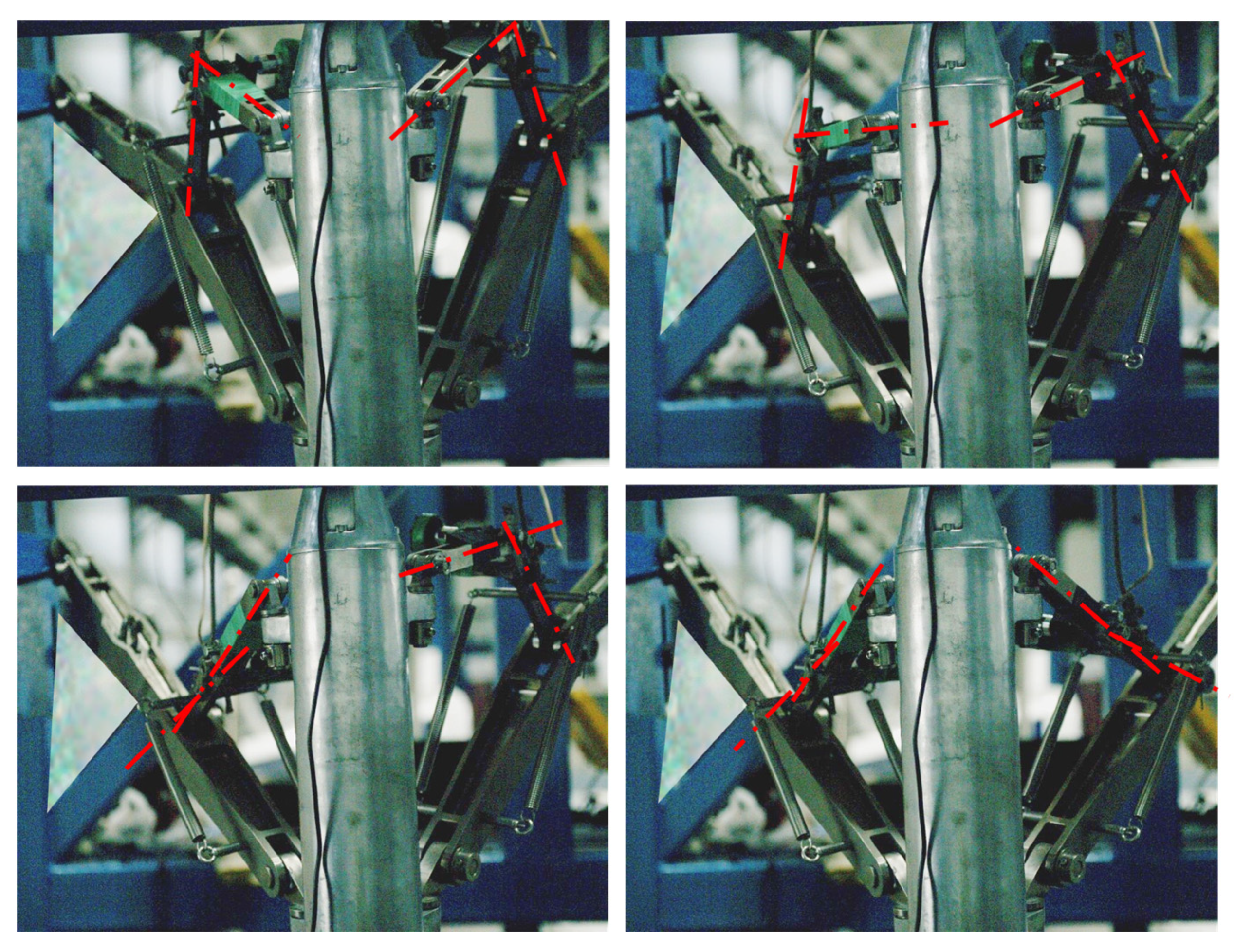
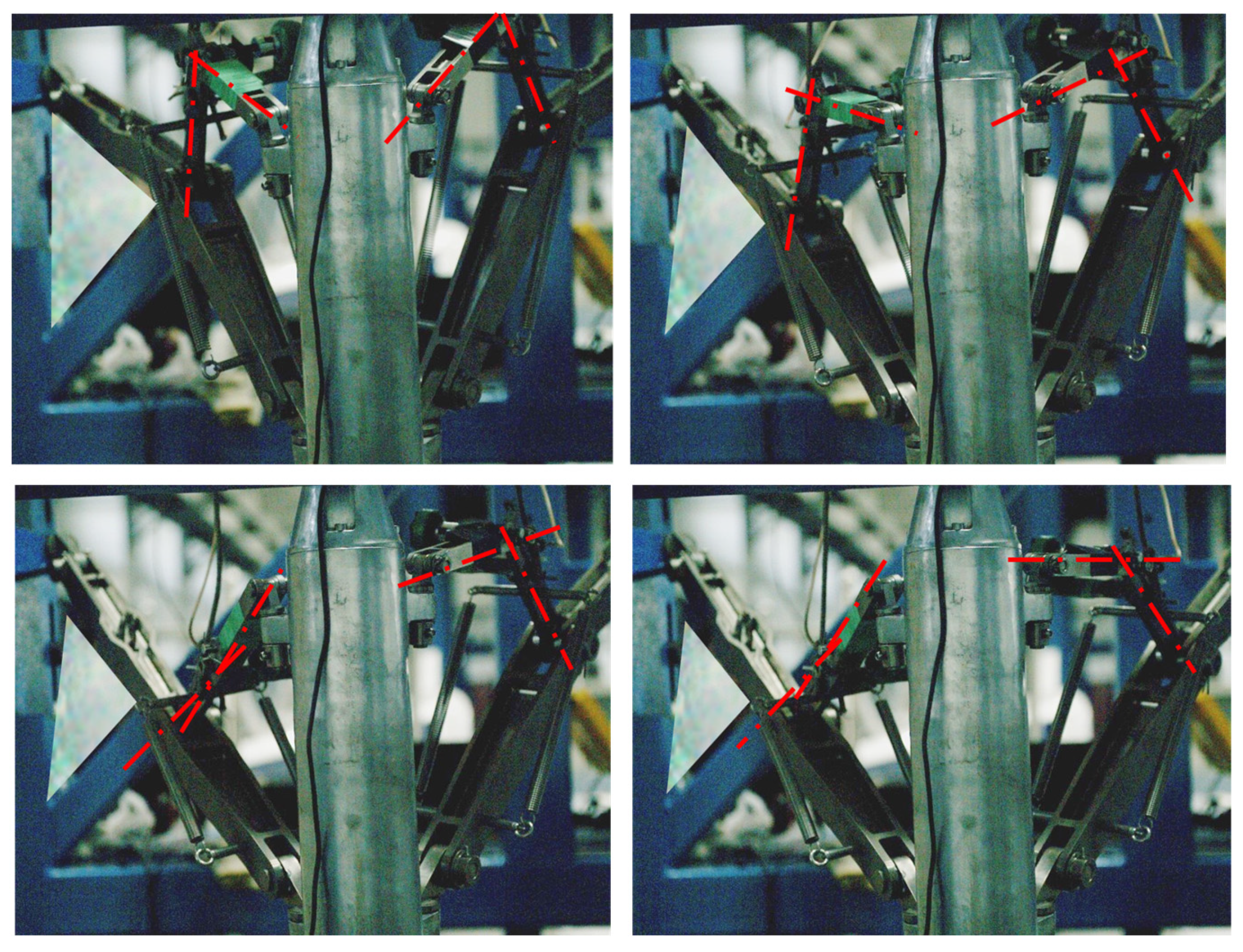
3.2. Synchronous Locking Test of Dual-Sidestay Landing Gear
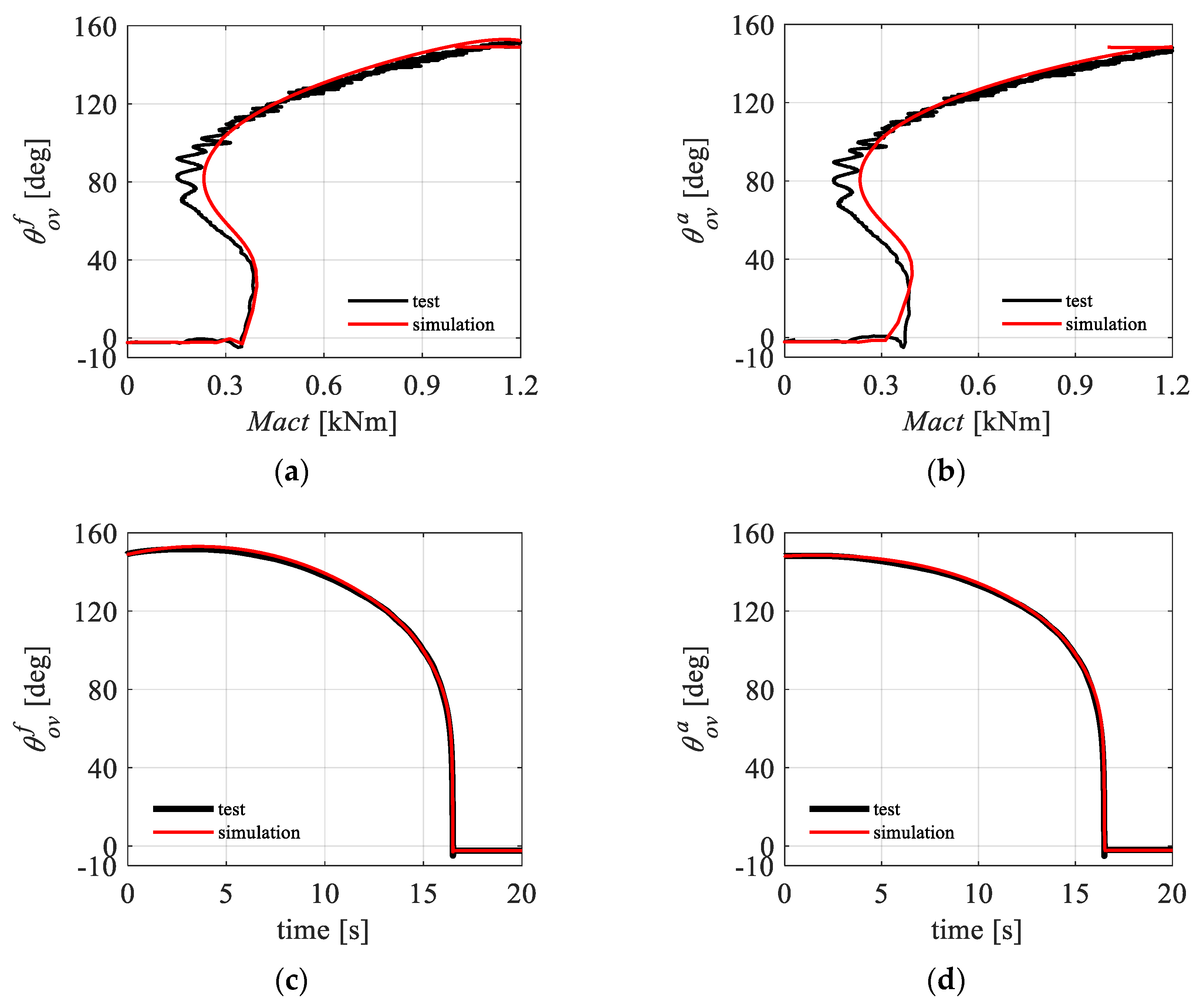
3.3. Comparison of Simulation and Test Results
4. Multiparameter Impact Analysis
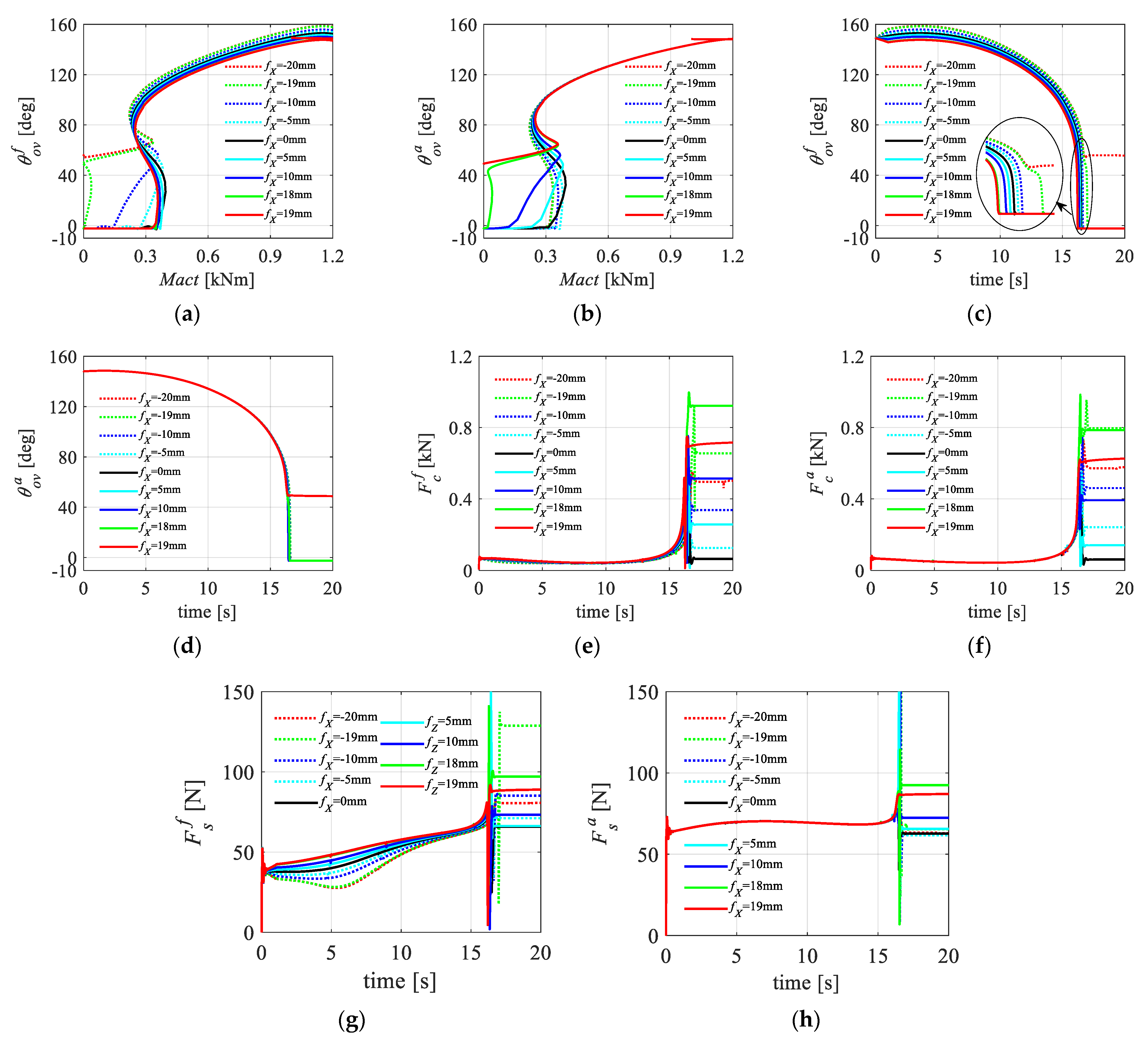
4.1. Analysis of the Impact of Node Deviation
4.1.1. Analysis of Simulation Results
4.1.2. Analysis of Test Results
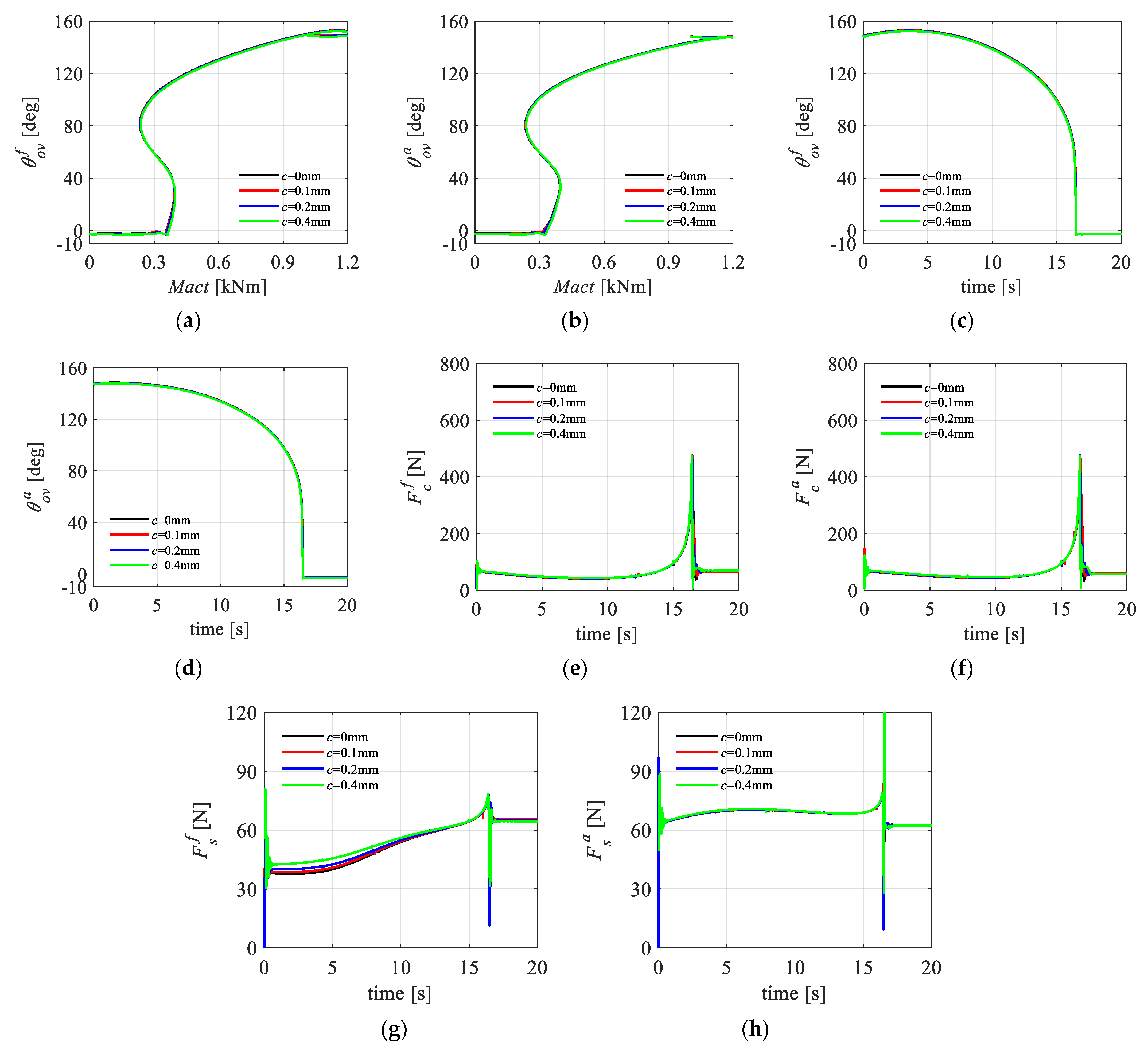
4.2. Analysis of the Impact of Joint Clearance
4.2.1. Analysis of Simulation Results
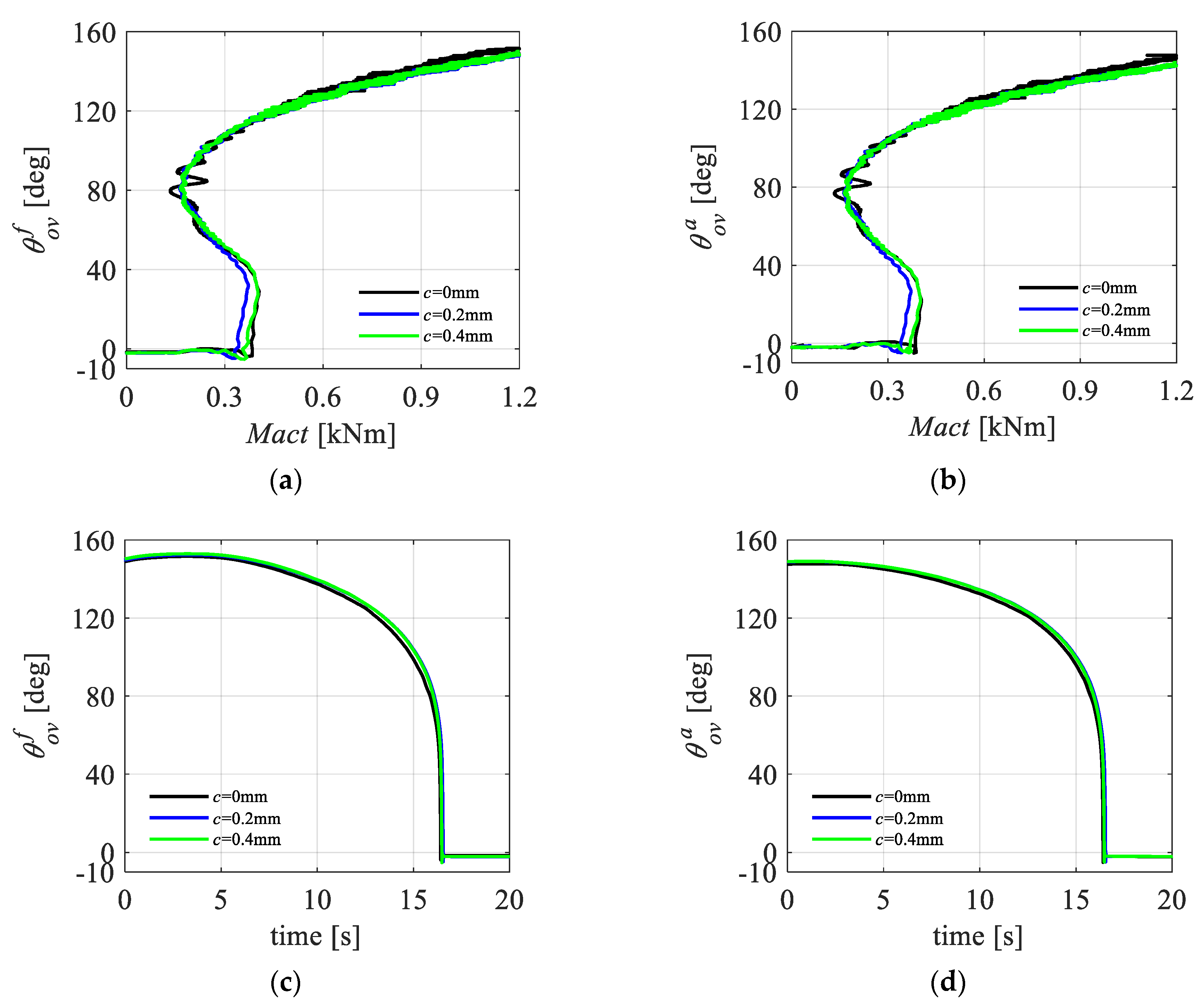
4.2.2. Analysis of Test Results
5. Conclusions
- In actual engineering, the hole–shaft clearance at the structural joint can eliminate the adverse effects caused by the deformation of the wing structure to a certain extent. Therefore, within a reasonable design range, this hyperstatic 3D retraction mechanism can successfully complete the retraction process without following the principle of “four axes intersecting at one point” and will not have a great impact on the final analysis results.
- An increase in node deviation makes the synchronous locking of the lock links on both sides more difficult. The sensitivity of the aft sidestay node deviation in the Z direction is the largest, and the sensitivity of the fore sidestay node deviation in the X direction is the smallest. Node deviation has a greater impact on the joint load of the sidestay node on the side of the adjusted deviation, mainly affecting its peak load and the position of the peak, and has a small impact on the remaining joint loads.
- Through a simulation analysis, the limit range of the fore and aft sidestay node deviation in different directions that can satisfy the complete locking of lock links on both sides is obtained. The deviation range of the fore sidestay node in the X direction is (−20 mm, 19 mm), and in the Z direction, it is (−17 mm, 18 mm); and the deviation range of the aft sidestay node in the X direction is (−20 mm, 19 mm), and in the Z direction, it is (−16 mm, 16 mm).
- For mechanisms with good a cooperative locking performance, during the slow extension process of the landing gear, the joint clearance has a very small impact on the retraction trajectory and joint load of the mechanism, and the general trend is that the load of the lock node and retraction torque decrease slightly as the joint clearance increases. Although appropriately increasing the joint clearance can increase the node deviation range corresponding to the complete locking of the DSS retraction mechanism, its size should be controlled strictly to avoid frequent collisions and severe vibrations of structural parts.
- The simulation results are in good agreement with the test results. The only difference is that the Mact in the simulation results is smaller than that in the test results when the lock links jump rapidly. The influence trend of the parameters on the synchronous locking is basically similar to the simulation conclusion. The difference is that the limit range of the node deviation measured by the test is larger. It is proved that the continuous contact force model and modified Coulomb friction model are suitable for the clearance modeling of the landing gear retraction mechanism. This modeling method can be applied to the design and analysis of the landing gear mechanism of wide-body aircraft.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tartaruga, I.; Cooper, J.E.; Lowenberg, M.H.; Lemmens, Y. Enhanced Evolutionary-Based Optimization Techniques Applied to a Nonlinear Landing Gear Design Problem. J. Aircr. 2019, 56, 2004–2018. [Google Scholar] [CrossRef]
- Currey, N.S. Aircraft Landing Gear Design: Principles and Practices; AIAA: Washington, DC, USA, 1988. [Google Scholar]
- Parat, C.; Li, Z.; Zhao, J.-S. Design and stiffness analysis of an overconstrained landing gear retraction mechanism with four side-stays. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 4421–4435. [Google Scholar] [CrossRef]
- Parat, C.; Li, Z.; Zhao, J. Design and Folding/Unfolding Dynamics of an Over-Constrained Airplane’s Landing Gear With Four Side Stays. J. Mech. Robot. 2019, 11, 011001. [Google Scholar] [CrossRef]
- Tinoco, E.N.; Brodersen, O.P.; Keye, S.; Laflin, K.R.; Feltrop, E.; Vassberg, J.C.; Mani, M.; Rider, B.; Wahls, R.A.; Morrison, J.H.; et al. Summary Data from the Sixth AIAA CFD Drag Prediction Workshop: CRM Cases. J. Aircr. 2017, 55, 1352–1379. [Google Scholar] [CrossRef]
- Veaux, J. New design procedures applied to landing gear development. J. Aircr. 1988, 25, 904–910. [Google Scholar] [CrossRef]
- Yin, Y.; Nie, H.; Ni, H.J.; Zhang, M. Reliability Analysis of Landing Gear Retraction System Influenced by Multifactors. J. Aircr. 2016, 53, 713–724. [Google Scholar] [CrossRef]
- Luo, H.T.; Zhao, J.S. Synthesis and kinematics of a double-lock overconstrained landing gear mechanism. Mech. Mach. Theory 2018, 121, 245–258. [Google Scholar] [CrossRef]
- Knowles, J.A.C.; Krauskopf, B.; Lowenberg, M. Numerical continuation analysis of a three-dimensional aircraft main landing gear mechanism. Nonlinear Dyn. 2013, 71, 331–352. [Google Scholar] [CrossRef][Green Version]
- Gao, C. Civil Aircraft Main Landing Gear Single Stay and Dual Stay Trade Off. Sci. Technol. Vis. 2017, 4, 2095–2457. [Google Scholar]
- Xu, K.; Yin, Y.; Yang, Y.; Nie, H.; Wei, X. Bifurcation analysis of dual-sidestay landing gear locking performance considering joint clearance. Chin. J. Aeronaut. 2022, 35, 209–226. [Google Scholar] [CrossRef]
- Fan, G.J.; Yuan, L.; Lu, L.J.; Jiang, B.Y. Kinematic Analysis of Landing Gear Spatial Retractable Mechanism. J. Zhengzhou Univ. (Eng. Sci.) 2012, 33, 88–92. [Google Scholar]
- Tian, J.J.; Jia, Y.H. Modeling and Simulation of Large Civil Aircraft Landing Gear System. Mach. Des. Manuf. 2016, 5, 48–52. [Google Scholar] [CrossRef]
- Cui, F.; Ma, D.L. Simulation analysis on dynamic performance of landing gear based on LMS Virtual. Lab. Comput.-Aided Eng. 2012, 21, 25–29. [Google Scholar]
- Huang, F.L.; Li, S.; Wang, Y.D. Study of Mechanical Efficiency of a Civil Aircraft’s Three Dimensional Landing Gear Operation Mechanism. J. Vib. Meas. Diagn. 2013, 33 (Suppl. S1), 188–191+230. [Google Scholar]
- Krakowska, A. Design of retraction mechanism of aircraft landing gear. Mech. Mech. Eng. 2008, 12, 357–373. [Google Scholar]
- Xin, Y.; Chen, X.J. Load design of landing gear brace lock spring of a new type civil aircraft. Mach. Des. Manuf. Eng. 2021, 50, 63–67. [Google Scholar]
- Knowles, J.A.C.; Krauskopf, B.; Lowenberg, M.H. Numerical Continuation Applied to Landing Gear Mechanism Analysis. J. Aircr. 2011, 48, 1254–1262. [Google Scholar] [CrossRef][Green Version]
- Yin, Y.; Neild, S.A.; Jiang, J.Z.; Knowles, J.A.C.; Nie, H. Optimization of a Main Landing Gear Locking Mechanism Using Bifurcation Analysis. J. Aircr. 2017, 54, 2126–2139. [Google Scholar] [CrossRef]
- Chinvorarat, S.; Vallikul, P. A novel retractable landing gear of a light amphibious airplane design, synthesis, analysis, and implementation. Aircr. Eng. Aerosp. Technol. 2021, 93, 1547–1558. [Google Scholar] [CrossRef]
- Knowles, J.A.C.; Krauskopf, B.; Lowenberg, M.H.; Neild, S.A.; Thota, P. Numerical Continuation Analysis of a Dual-Sidestay Main Landing Gear Mechanism. J. Aircr. 2014, 51, 129–143. [Google Scholar] [CrossRef][Green Version]
- Erkaya, S.; Uzmay, İ. Investigation on effect of joint clearance on dynamics of four-bar mechanism. Nonlinear Dyn. 2009, 58, 179–198. [Google Scholar] [CrossRef]
- Erkaya, S.; Doğan, S. A comparative analysis of joint clearance effects on articulated and partly compliant mechanisms. Nonlinear Dyn. 2015, 81, 323–341. [Google Scholar] [CrossRef]
- Zhang, Z.L.; Wu, S.J.; Zhao, W.Q.; Wang, X.S. Dynamic characteristics of high-speed multi-link transmission mechanisms with clearance. J. Vib. Shock 2014, 33, 66–71. [Google Scholar]
- Yin, Y.; Xu, K.; Nie, H.; Wei, X.H.; Wang, H.L. Dynamics Analysis of Spatial Landing-Gear Mechanism with Hinge Clearance and Axis Deviation. J. Aircr. 2020, 58, 30–42. [Google Scholar] [CrossRef]
- Yan, S.Z.; Xiang, W.W.; Huang, T.Q. Advances in Modeling of Clearance Joints and Dynamics of Mechanical Systems with Clearances. Acta Sci. Nat. Univ. Pekin. 2016, 52, 741–755. [Google Scholar]
- Lankarani, H.M.; Nikravesh, P.E. A Contact Force Model With Hysteresis Damping for Impact Analysis of Multibody Systems. J. Mech. Des. 1990, 112, 369–376. [Google Scholar] [CrossRef]
- Rooney, G.T.; Deravi, P. Coulomb friction in mechanism sliding joints. Mech. Mach. Theory 1982, 17, 207–211. [Google Scholar] [CrossRef]
- Ambrósio, J.A.C. Impact of Rigid and Flexible Multibody Systems: Deformation Description and Contact Models. In Virtual Nonlinear Multibody Systems; Schiehlen, W., Valášek, M., Eds.; Springer: Dordrecht, The Netherlands, 2003; pp. 57–81. [Google Scholar]
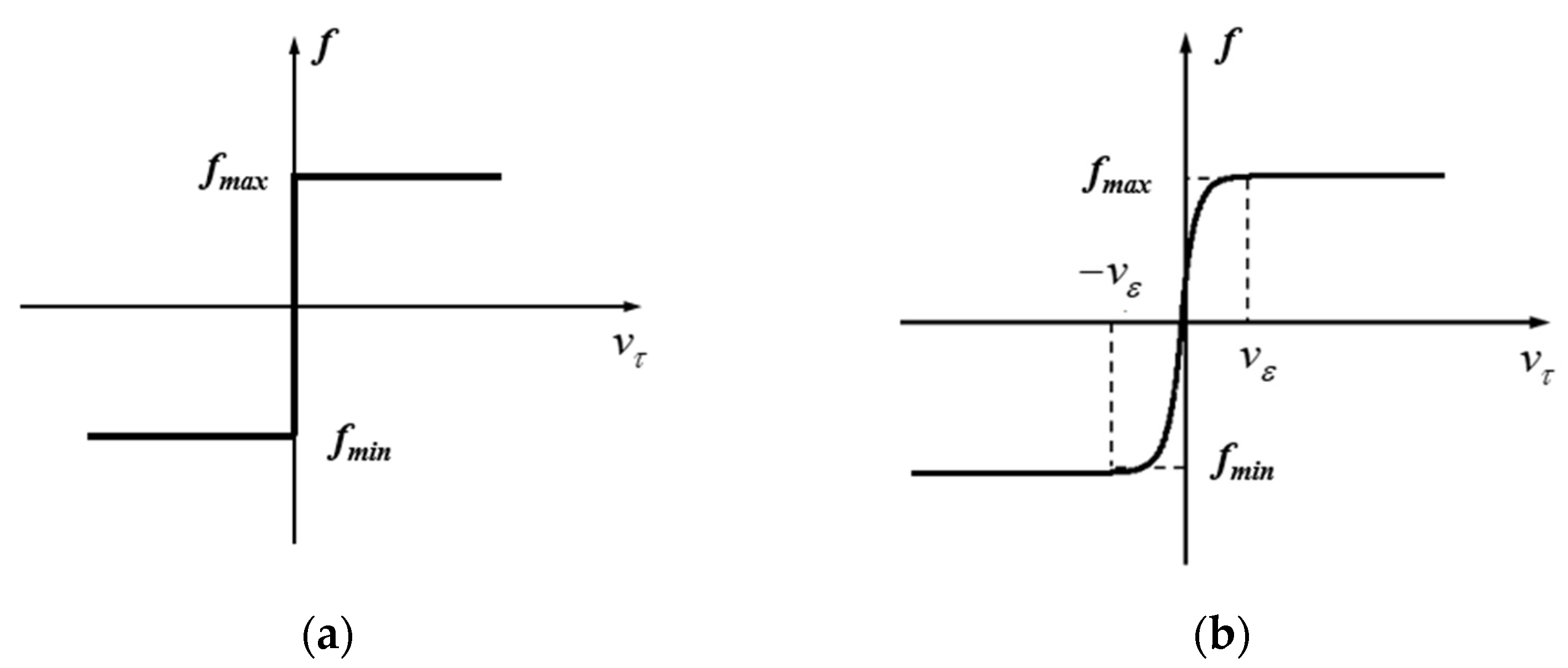





| Node Deviation | X Direction | Z Direction |
|---|---|---|
| Fore sidestay | (−20 mm, 19 mm) | (−17 mm, 18 mm) |
| Aft sidestay | (−20 mm, 19 mm) | (−16 mm, 16 mm) |
| Nodes | Fore Sidestay Node | Aft Sidestay Node | ||
|---|---|---|---|---|
| Deviation direction | X direction | Z direction | X direction | Z direction |
| Simulation results | (−20 mm, 19 mm) | (−17 mm, 18 mm) | (−20 mm, 19 mm) | (−16 mm, 16 mm) |
| Test results | (−22 mm, 21 mm) | (−19 mm, 20 mm) | (−20 mm, 20 mm) | (−18 mm,18 mm) |
| Clearances | 0 mm | 0.1 mm | 0.2 mm | 0.4 mm |
|---|---|---|---|---|
| Range of the node deviations | (−20 mm, 19 mm) | (−22 mm, 21 mm) | (−24 mm, 23 mm) | (−28 mm, 26 mm) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Wu, S.; Zhu, H.; Nie, H.; Wei, X. Analysis of the Synchronized Locking Dynamic Characteristics of a Dual-Sidestay Main Landing Gear Retraction Mechanism. Aerospace 2024, 11, 356. https://doi.org/10.3390/aerospace11050356
Zhang Z, Wu S, Zhu H, Nie H, Wei X. Analysis of the Synchronized Locking Dynamic Characteristics of a Dual-Sidestay Main Landing Gear Retraction Mechanism. Aerospace. 2024; 11(5):356. https://doi.org/10.3390/aerospace11050356
Chicago/Turabian StyleZhang, Zhipeng, Shengxiao Wu, He Zhu, Hong Nie, and Xiaohui Wei. 2024. "Analysis of the Synchronized Locking Dynamic Characteristics of a Dual-Sidestay Main Landing Gear Retraction Mechanism" Aerospace 11, no. 5: 356. https://doi.org/10.3390/aerospace11050356
APA StyleZhang, Z., Wu, S., Zhu, H., Nie, H., & Wei, X. (2024). Analysis of the Synchronized Locking Dynamic Characteristics of a Dual-Sidestay Main Landing Gear Retraction Mechanism. Aerospace, 11(5), 356. https://doi.org/10.3390/aerospace11050356







