Dynamic Behavior of Satellite and Its Solar Arrays Subject to Large-Scale Antenna Deployment Shock
Abstract
1. Introduction
2. Experimental Investigation
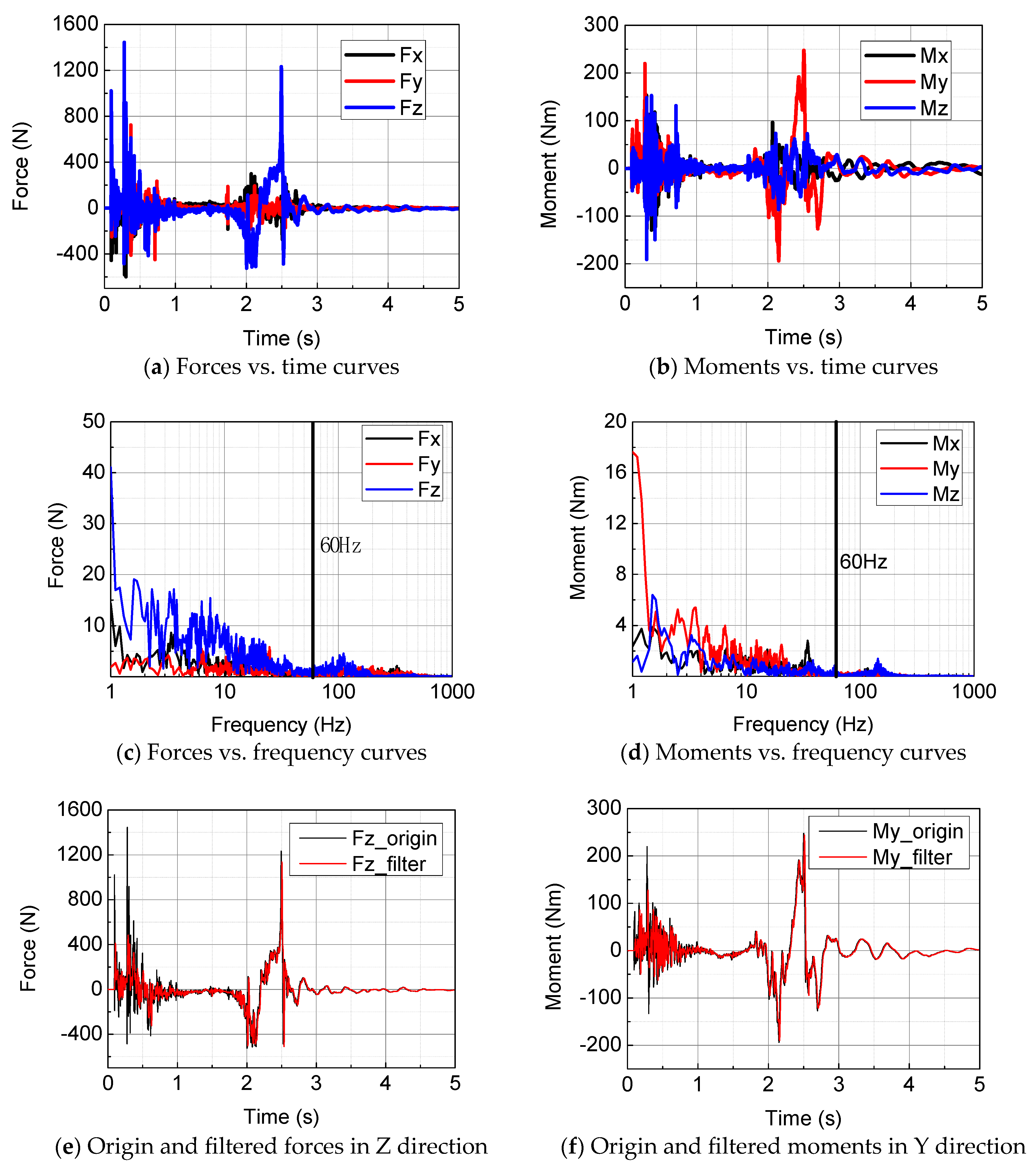
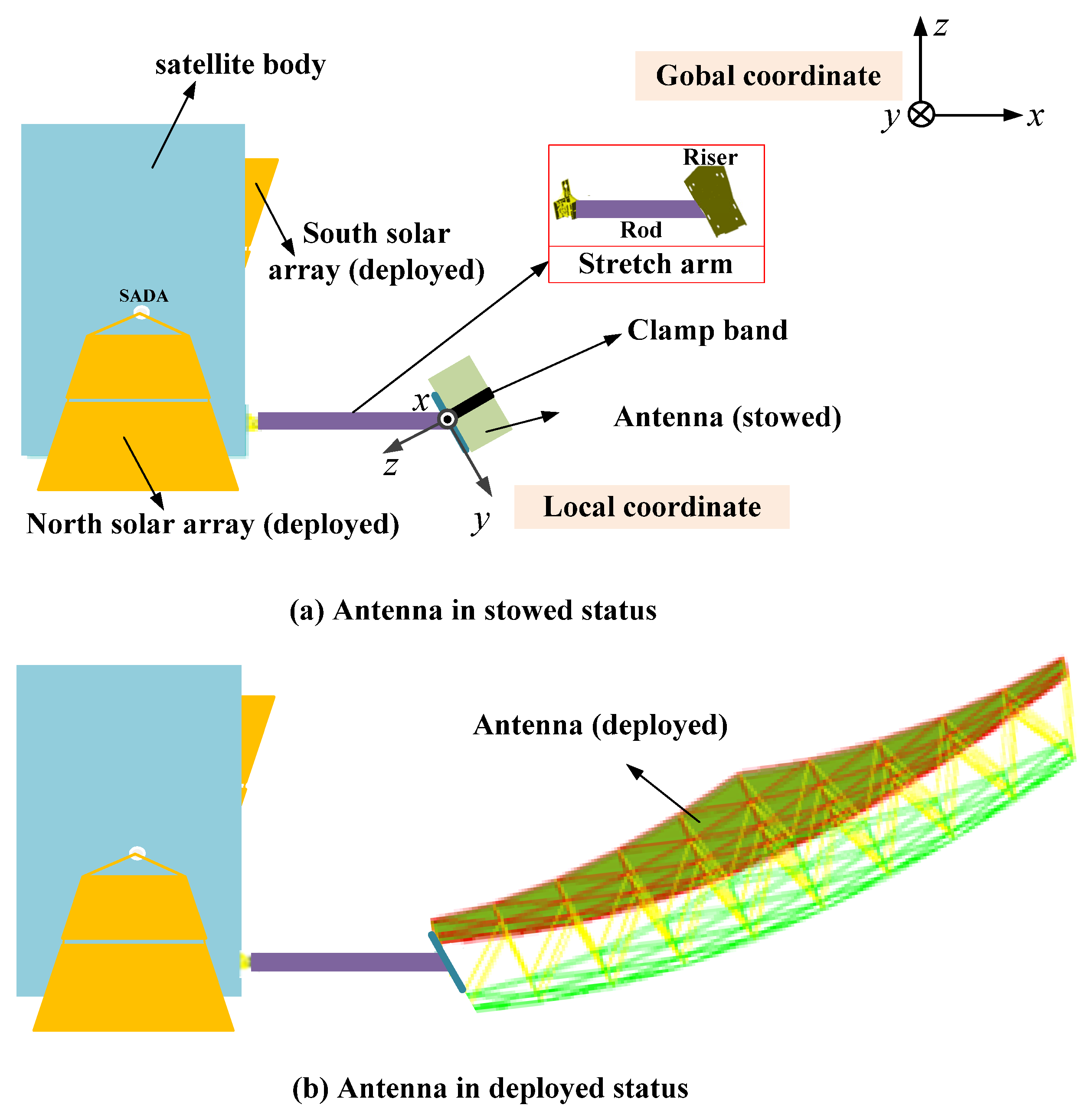
2.1. Deployment Test of Antenna Driven by Internal Spring Energy
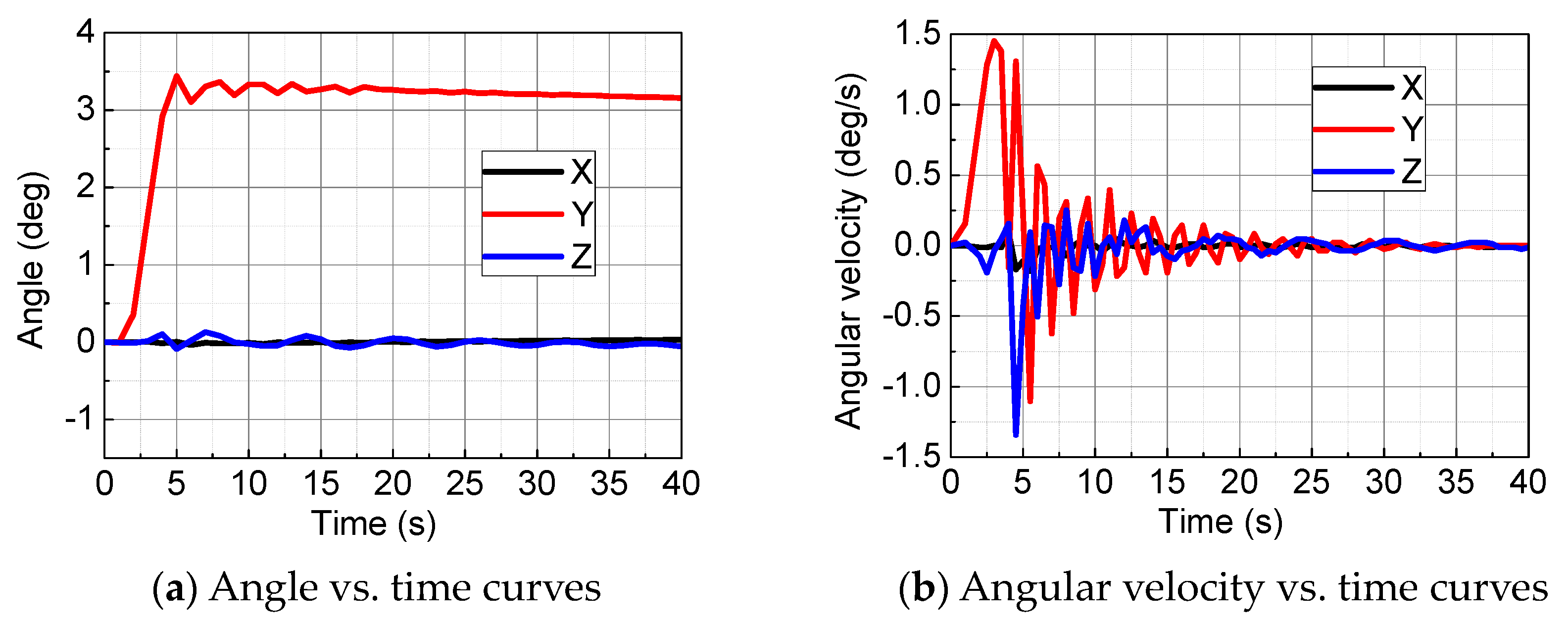
2.2. Attitude Test of Satellite during Antenna Shock Deployment
3. Dynamical Model
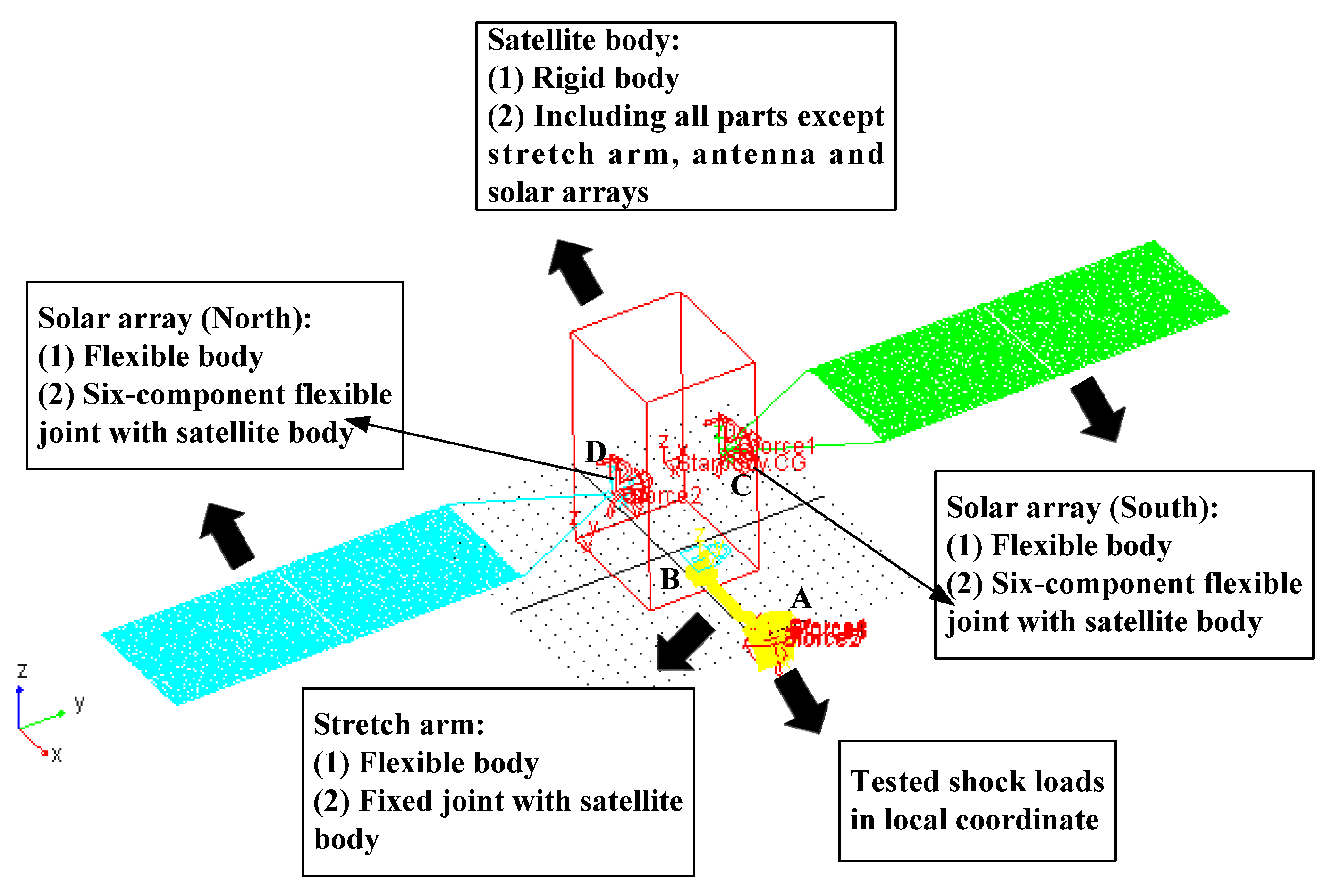
3.1. Modelling Details
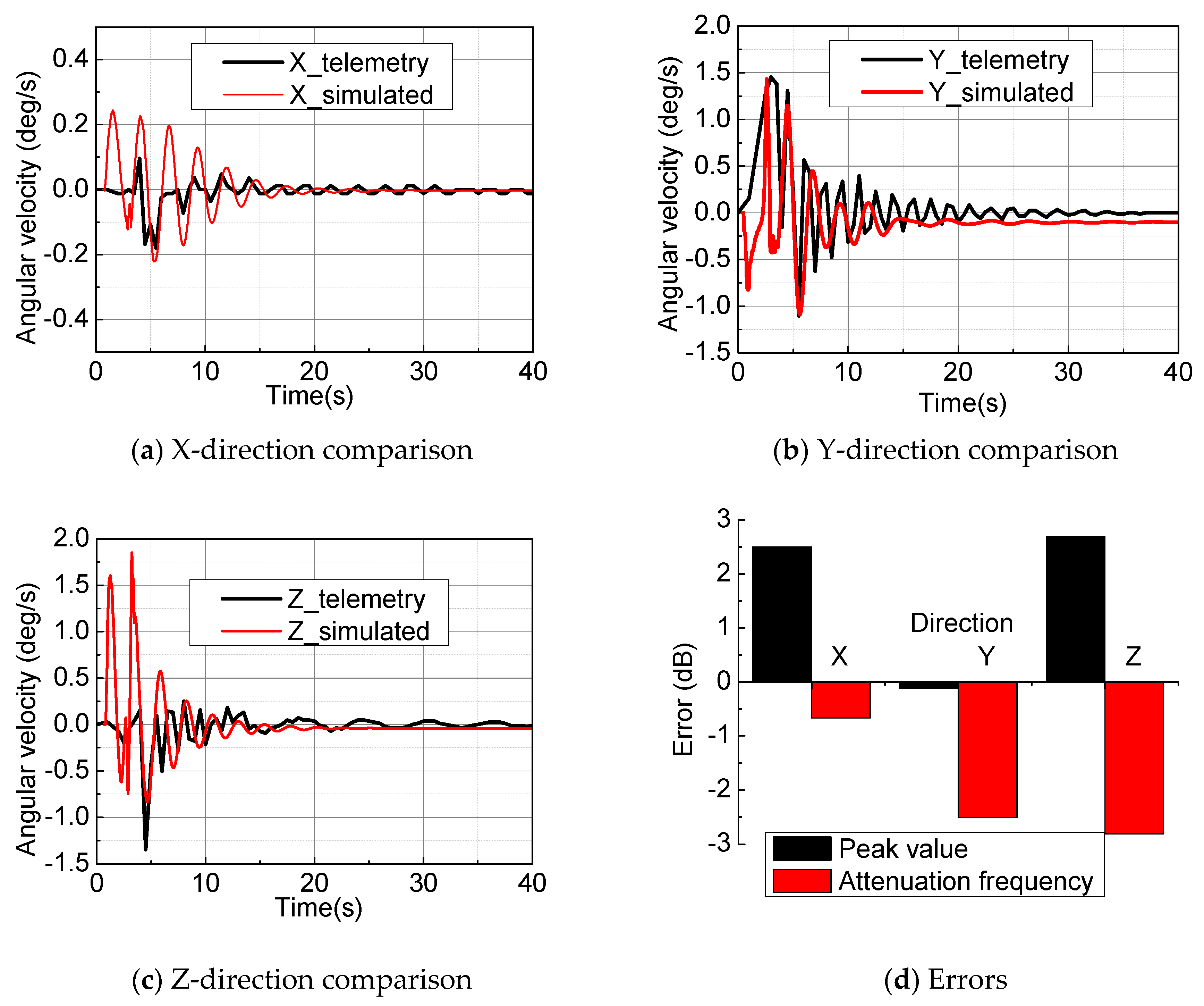
3.2. Prediction Verification
4. Discussion
4.1. Shock Mechanism Transmitted to SADA
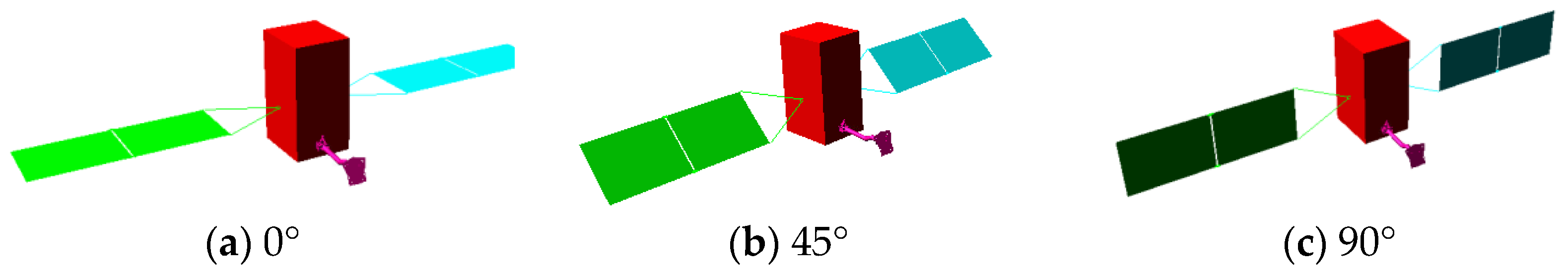
4.2. Effect of Solar Array Attitude
5. Conclusions
- (1)
- The deployment of the truss antenna includes four stages, sequentially, the pyro unlocking of the antenna, the antenna extension driven by spring energy, the locking at a specified location and the antenna vibration attenuating to stability. Pyro shock and spring-induced mechanical shock are dominant at more and less than 60 Hz, respectively, and the former are smaller than the latter.
- (2)
- The stability time of the satellite subjected to the truss antenna deployment (40 s) is much larger than that of the truss antenna deployment by itself (5 s) due to the damped vibration of the solar arrays. In the dynamical model of the satellite, the simulated method of antenna deployment, equivalent to the shock loads tested, was thought to be efficient, though it could cause a small non-zero constant of the simulated Y-direction angular velocities.
- (3)
- The shock-induced moments, except the SADA rotation direction, should be highlighted for the antenna deployment dynamic design of satellites. The solar array attitude affects the X-direction and Z-direction loads at the SADAs but not their composed loads and Y-direction loads. Therefore, it can be inferred that there is no need to pay special attention to the design of the solar array attitude during antenna deployment.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Du, X.; Du, J.; Bao, H.; Sun, G. Deployment analysis of deployable antennas considering cable net and truss flexibility. Aerosp. Sci. Technol. 2018, 82, 557–565. [Google Scholar] [CrossRef]
- Zhao, J.; Yang, J.; Xiao, Y.; Ma, X. Deployment Strategy and Dynamic Analysis of Large Ring Truss Antenna. Int. J. Aerosp. Eng. 2022, 2022, 4725423. [Google Scholar] [CrossRef]
- Dai, L.; Xiao, R. Optimal design and analysis of deployable antenna truss structure based on dynamic characteristics restraints. Aerosp. Sci. Technol. 2020, 106, 106086. [Google Scholar] [CrossRef]
- Sun, Z.; Yang, D.; Duan, B.; Kong, L.; Zhang, Y. Structural design, dynamic analysis, and verification test of a novel double-ring deployable truss for mesh antennas. Mech. Mach. Theory 2021, 165, 104416. [Google Scholar] [CrossRef]
- Zhang, W.; Wu, R.Q.; Behdinan, K. Nonlinear dynamic analysis near resonance of a beam-ring structure for modeling circular truss antenna under time-dependent thermal excitation. Aerosp. Sci. Technol. 2019, 86, 296–311. [Google Scholar] [CrossRef]
- Freeman, M. On-orbit deployment anamolies, What can be done? In Proceedings of the the Seventeenth Space Simulation Conference. Terrestrial Test for Space Success, Baltimore, MD, USA, 9–12 November 1992; NASA Goddard Space Flight Center: Baltimore, MD, USA, 1992. [Google Scholar]
- Xu, Y.; Guan, F.L. Structure–electronic synthesis design of deployable truss antenna. Aerosp. Sci. Technol. 2013, 26, 259–267. [Google Scholar] [CrossRef]
- Takano, T.; Natori, M.; Miyoshi, K. A tension-truss deployable antenna for space-use and its obtainable characteristics. In Proceedings of the IEEE Antennas and Propagation Society International Symposium and URSI National Radio Science Meeting, Seattle, WA, USA, 20–24 June 1994; Volume 2, pp. 878–881. [Google Scholar]
- Wang, Y.; Liu, R.; Yang, H.; Cong, Q.; Guo, H. Design and deployment analysis of modular deployable structure for large antennas. J. Spacecr. Rocket. 2015, 52, 1101–1111. [Google Scholar] [CrossRef]
- Meng, Q.; Liu, X.J.; Xie, F. Structure design and kinematic analysis of a class of ring truss deployable mechanisms for satellite antennas based on novel basic units. Mech. Mach. Theory 2022, 174, 104881. [Google Scholar] [CrossRef]
- Han, B.; Xu, Y.; Yao, J.; Zheng, D.; Guo, L.; Zhao, Y. Type synthesis of deployable mechanisms for ring truss antenna based on constraint-synthesis method. Chin. J. Aeronaut. 2020, 33, 2445–2460. [Google Scholar] [CrossRef]
- Siriguleng, B.; Zhang, W.; Liu, T.; Liu, Y. Vibration modal experiments and modal interactions of a large space deployable antenna with carbon fiber material and ring-truss structure. Eng. Struct. 2020, 207, 109932. [Google Scholar] [CrossRef]
- Liu, R.Q.; Tian, D.K.; Deng, Z.Q.; Guo, H.W.; Liu, Z.J. Kinematics modeling and driving spring design of truss structure for deployable truss antenna. In Advanced Materials Research; Trans Tech Publications Ltd.: Wollerau, Switzerland, 2012; Volume 457, pp. 1337–1341. [Google Scholar]
- Wang, D.; Liu, R.; Wang, Y.; Guo, H.; Cong, Q.; Zhang, C. Deployment analysis of a planar deployable support truss structure. In Proceedings of the 2013 IEEE International Conference on Mechatronics and Automation, Takamatsu, Japan, 4–7 August 2013; pp. 1287–1292. [Google Scholar]
- Guo, J.; Zhao, Y.; Zhang, G.; Liu, E.; Liu, B.; Xu, Y. Configuration synthesis and unfolding stiffness characteristics analysis of a truss antenna connecting mechanism based on URU-RR-URU hexagonal deployable unit. Mech. Mach. Theory 2022, 177, 105047. [Google Scholar] [CrossRef]
- Guo, J.; Zhao, Y.; Xu, Y.; Zhang, G. Mechanics analysis and structural design of a truss deployable antenna mechanism based on 3RR-3URU tetrahedral unit. Mech. Mach. Theory 2022, 171, 104749. [Google Scholar] [CrossRef]
- Takamatsu, K.A.; Onoda, J. New deployable truss concepts for large antenna structures or solar concentrators. J. Spacecr. Rocket. 1991, 28, 330–338. [Google Scholar] [CrossRef]
- Tian, D.; Liu, R.; Deng, Z. Spatial geometry modeling of truss structure and analysis of connection deviation for deployable truss antenna. In Proceedings of the 2010 International Conference on Measuring Technology and Mechatronics Automation, Changsha, China, 13–14 March 2010; Volume 3, pp. 427–432. [Google Scholar]
- Tian, D.; Deng, Z.; Liu, R.; Guo, H. Analysis on dynamic response of truss structure for deployable truss antenna. In Proceedings of the 2011 9th World Congress on Intelligent Control and Automation, Taipei, Taiwan, 21–25 June 2011; pp. 1185–1188. [Google Scholar]
- Li, D.X.; Liu, W.; Fan, C.Z. Dynamic characteristics of satellite solar arrays under the deployment shock in orbit. Shock. Vib. 2018, 2018, 6519748. [Google Scholar] [CrossRef]
- Santoni, F. Dynamics of spring-deployed solar panels for agile nanospacecraft. J. Aerosp. Eng. 2015, 28, 04014122. [Google Scholar] [CrossRef]
- Sureda, M.; Sobrino, M.; Millan, O.; Aguilella, A.; Solanellas, A.; Badia, M.; Munoz-Martin, J.F.; Fernandez, L.; Ruiz-De-Azua, J.A.; Camps, A. Design and testing of a helix antenna deployment system for a 1U CubeSat. IEEE Access 2021, 9, 66103–66114. [Google Scholar] [CrossRef]
- Liu, L.; Shan, J.; Zhang, Y. Dynamics modeling and analysis of spacecraft with large deployable hoop-truss antenna. J. Spacecr. Rocket. 2016, 53, 471–479. [Google Scholar] [CrossRef]
- Zheng, S.; Li, T.; Zhao, J.; Ma, X.; Zhu, J.; Huang, Z.; Lang, Y. Deployment Impact Experiment and Dynamic Analysis of Modular Truss Antenna. Int. J. Aerosp. Eng. 2022, 2022, 2038932. [Google Scholar] [CrossRef]
- Safak, O. Structural Design and Analysis of a Solar Array Substrate for a GEO Satellite. Master’s Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2013. [Google Scholar]
- Adams Dynamic Software; MSC Software Corporation: Santa Ana, CA, USA, 2016.



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Wu, P.; Han, Q.; Wei, X.; Duan, Y. Dynamic Behavior of Satellite and Its Solar Arrays Subject to Large-Scale Antenna Deployment Shock. Aerospace 2024, 11, 349. https://doi.org/10.3390/aerospace11050349
Zhang J, Wu P, Han Q, Wei X, Duan Y. Dynamic Behavior of Satellite and Its Solar Arrays Subject to Large-Scale Antenna Deployment Shock. Aerospace. 2024; 11(5):349. https://doi.org/10.3390/aerospace11050349
Chicago/Turabian StyleZhang, Jie, Pengfei Wu, Qinghu Han, Xin Wei, and Yi Duan. 2024. "Dynamic Behavior of Satellite and Its Solar Arrays Subject to Large-Scale Antenna Deployment Shock" Aerospace 11, no. 5: 349. https://doi.org/10.3390/aerospace11050349
APA StyleZhang, J., Wu, P., Han, Q., Wei, X., & Duan, Y. (2024). Dynamic Behavior of Satellite and Its Solar Arrays Subject to Large-Scale Antenna Deployment Shock. Aerospace, 11(5), 349. https://doi.org/10.3390/aerospace11050349





