Numerical Study on Pressure Oscillations in a Solid Rocket Motor with Backward Step Configuration Under Two-Phase Flow Interactions
Abstract
1. Introduction
2. Numerical Methodology
2.1. Large Eddy Simulation Method
2.2. Numerical Model of Two-Phase Flow
2.3. Physical Model and Numerical Methodology
3. Verification of Numerical Methodology
3.1. Grid Independence Analysis
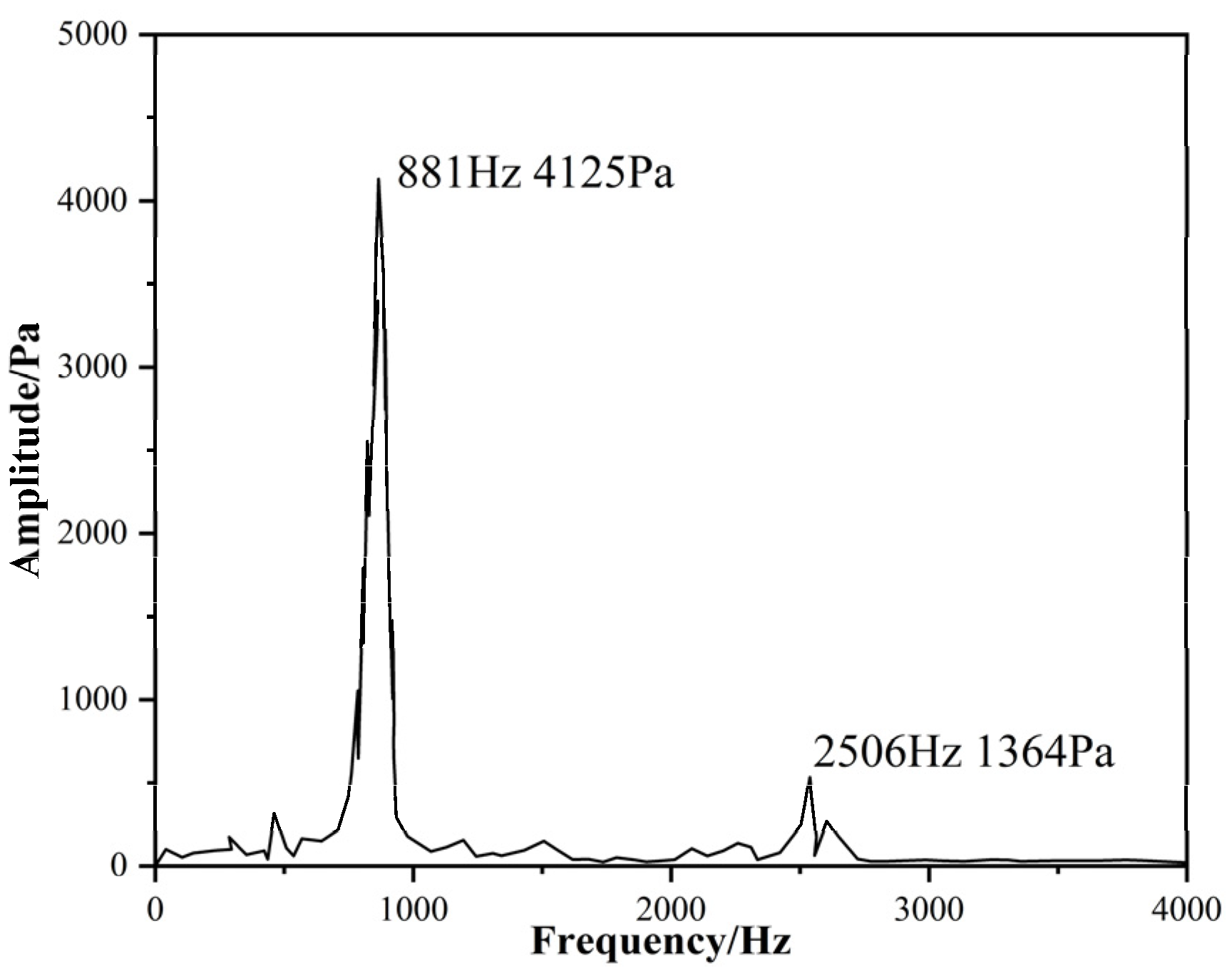
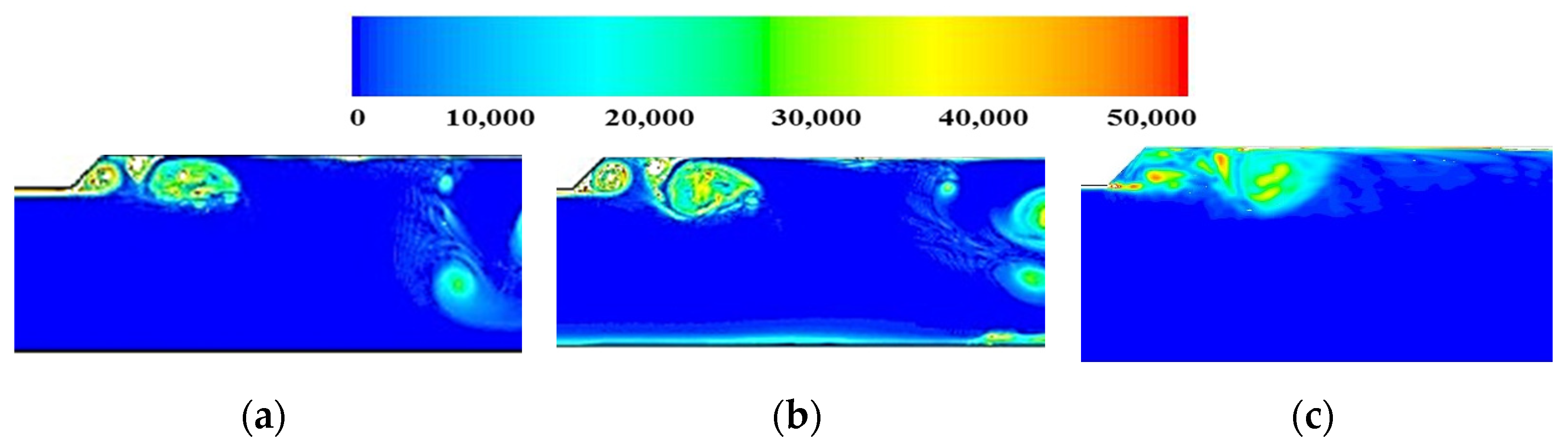
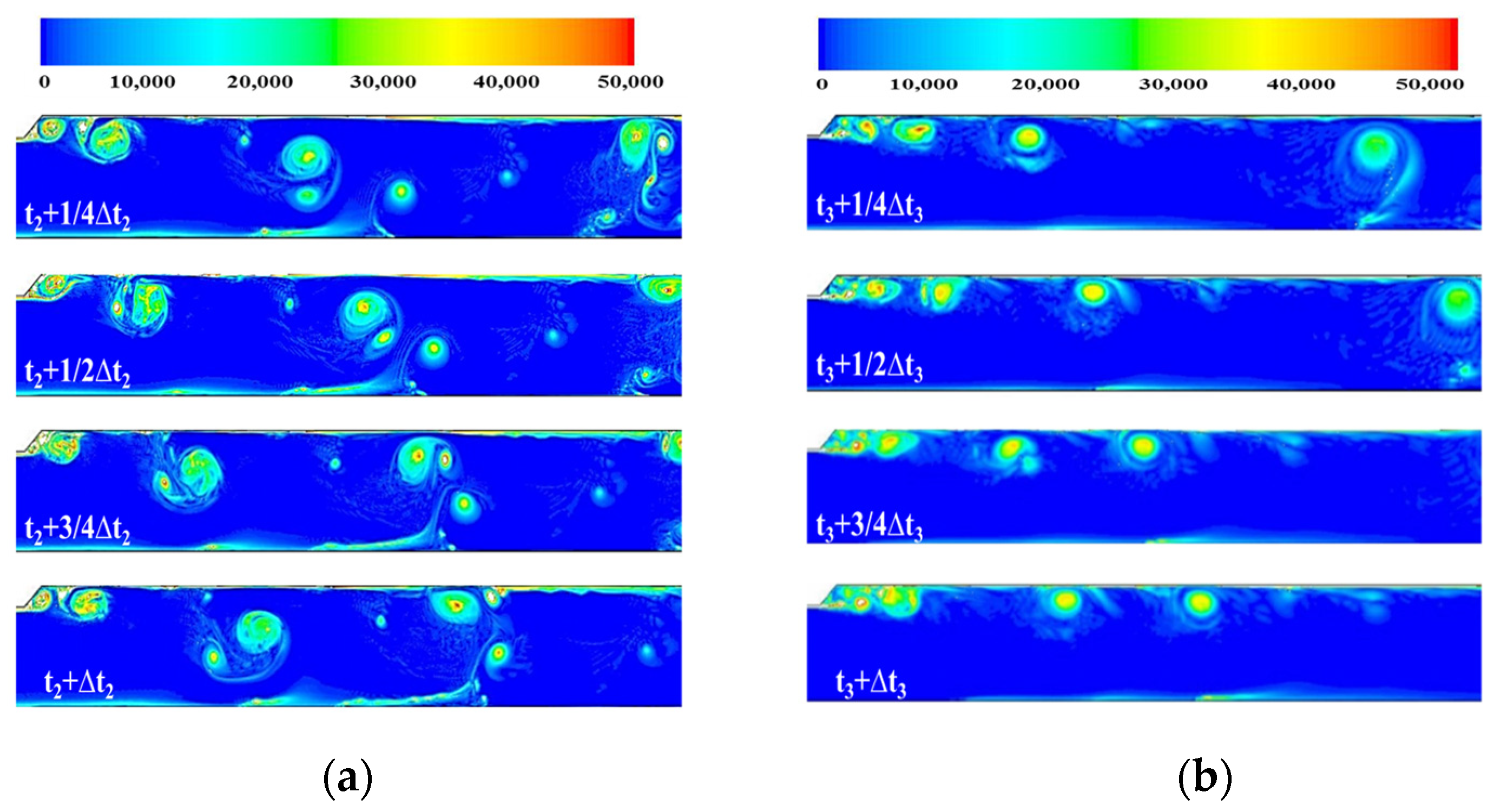
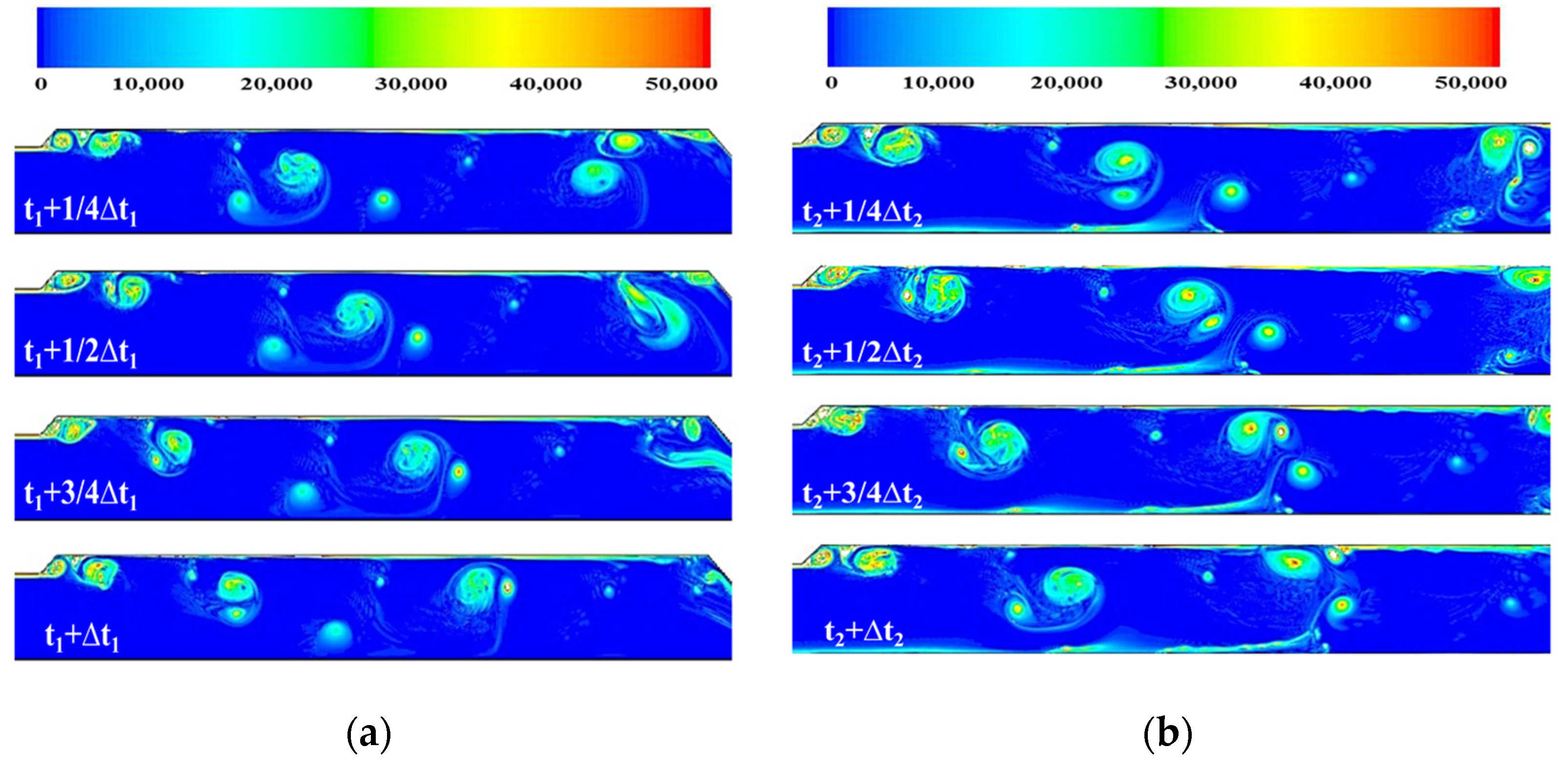
3.2. Verification of the Numerical Method
4. Numerical Results and Discussion
4.1. Analysis of Key Parameters of the Particle Phase
4.1.1. Particle Diameter
4.1.2. Particle Concentration
4.2. Analysis of Key Geometric Parameters of the Backward Step
4.2.1. Upstream Aspect Ratio of the Step
4.2.2. Expansion Ratio
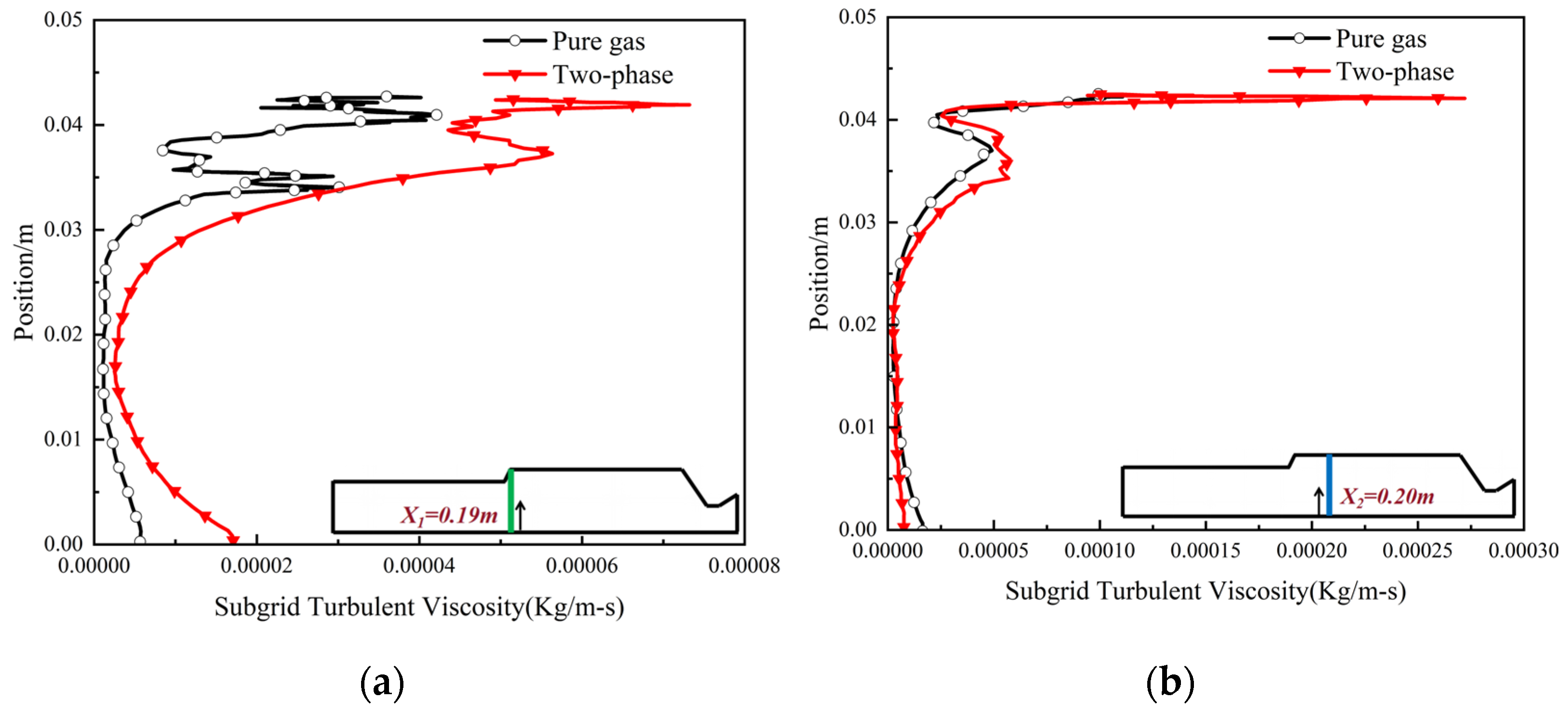
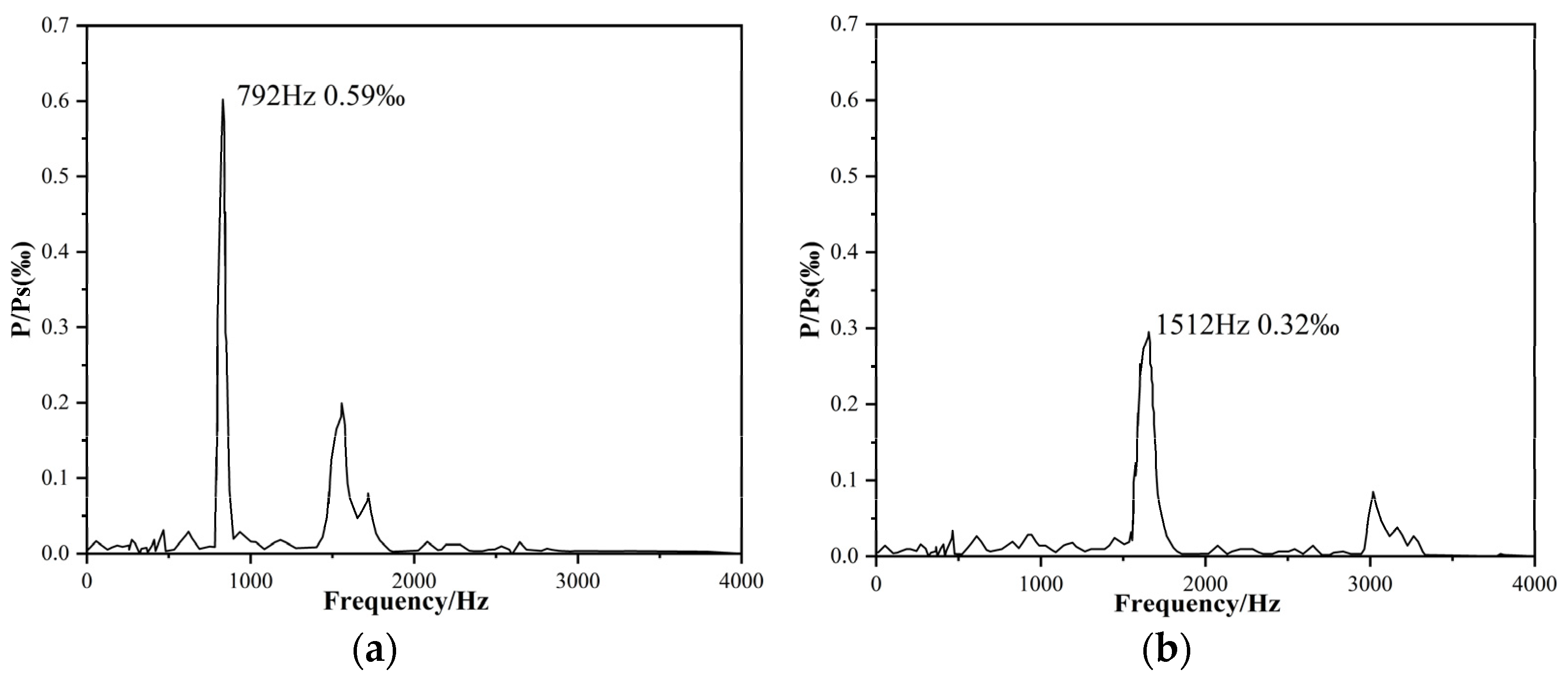
4.2.3. Backward Step Location
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| LES | large eddy simulation |
| DPM | discrete phase model |
| RANS | Reynolds-averaged Navier–Stokes equation |
| DNS | direct numerical simulation |
| SGS | subgrid-scale model |
| ONERA | Office National d’Etudes et de Recherches |
| FFT | fast Fourier transformation |
References
- Vacher, D.; Dudeck, M.A.; André, P.; Silva, M.L.D.; Faure, G.E.; Dubois, M.; Hamwi, A.; Menecier, S.; Rochette, D. Radiation from an ICP plasma torth in the near-UV to near-IR spectral region for a Tiran-type N2-CH4 mixture. Tech. Phys. 2009, 50, 213–231. [Google Scholar]
- Scippa, S.; Pascal, P.; Zanier, F. Ariane 5-MPS-Chamber Pressure Oscillations Full Scale Firing Results: Analysis and Further Studies. In Proceedings of the 30th Joint Propulsion Conference and Exhibit, Indianapolis, IN, USA, 27–29 June 1994; p. 3068. [Google Scholar]
- Zhang, Q.; Wei, Z.; Su, W.; Li, J.; Wang, N. Theoretical modeling and numerical study for thrust-oscillation characteristics in solid rocket motors. J. Propuls. Power 2012, 28, 312–322. [Google Scholar] [CrossRef]
- Blomshield, F. Lessons Learned in Solid Rocket Combustion Instability. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 8–11 July 2007. [Google Scholar]
- Brown, R.; Dunlap, R.; Young, S.; Waugh, R. Vortex shedding as a source of acoustic energy in segmented solid rockets. J. Spacecr. Rocket. 1981, 18, 312–319. [Google Scholar] [CrossRef]
- Flatau, A.; VanMoorhem, W. Prediction of vortex shedding responses in segmented solid rocket motors. In Proceedings of the 26th Joint Propulsion Conference, Orlando, FL, USA, 16–18 July 1990; p. 2073. [Google Scholar]
- Miao, Q.; Shen, Z.; Zhang, H.; Sun, H. Multiobjective Optimization Method of Solid Rocket Motor Finocyl Grain Based on Surrogate Model. Aerospace 2022, 9, 679. [Google Scholar] [CrossRef]
- Hyun, W.; Kim, J.; Chae, H.; Lee, C. Passive Control of Low-Frequency Instability in Hybrid Rocket Combustion. Aerospace 2021, 8, 204. [Google Scholar] [CrossRef]
- Flandro, G.; Jacobs, H. Vortex generated sound in cavities. In Proceedings of the Aeroacoustics Conference, Seattle, WA, USA, 15–17 October 1973; p. 1014. [Google Scholar]
- Dunlap, R.; Brown, R.S. Exploratory experiments on acoustic oscillations driven by periodic vortex shedding. AIAA J. 1981, 19, 408–409. [Google Scholar] [CrossRef]
- Culick, F.E.C.; Yang, V. Prediction of the stability of unsteady motions in solid-propellant rocket motors. Nonsteady Burn. Combust. Stab. Solid Propellants 1992, 143, 719–779. [Google Scholar]
- Dotson, K.W.; Koshigoe, S.; Pace, K.K. Vortex Shedding in a Large Solid Rocket Motor Without Inhibitors at the Segment Interfaces. J. Propuls. Power 1997, 13, 197–206. [Google Scholar] [CrossRef]
- Apte, S.; Yang, V. Unsteady flow evolution in porous chamber with surface mass injection, part 1: Free oscillation. AIAA J. 2001, 39, 1577–1586. [Google Scholar] [CrossRef]
- Apte, S.; Yang, V. Unsteady flow evolution in porous chamber with surface mass injection, part 2: Acoustic excitation. AIAA J. 2002, 40, 244–253. [Google Scholar] [CrossRef]
- Cai, W.; Ma, F.; Yang, V. Two-Phase Vorticoacoustic Flow Interactions in Solid-Propellant Rocket Motors. J. Propuls. Power 2003, 19, 385–396. [Google Scholar] [CrossRef]
- Chen, L.; Gao, Y.; Wang, D.; Zou, Q.; Zhang, S. Numerical simulation on acoustic mode and pressure-oscillation decay in finocyl-and axil-grain combustion chambers. Aerosp. Sci. Technol. 2020, 107, 106351. [Google Scholar] [CrossRef]
- Jalili, B.; Jalili, P. Numerical analysis of airflow turbulence intensity effect on liquid jet trajectory and breakup in two-phase cross flow. Alex. Eng. J. 2023, 68, 577–585. [Google Scholar] [CrossRef]
- Zhang, Q.; Wang, N.; Li, J.; Su, W.; Zhang, Y. Effect of the head cavity on pressure oscillation suppression characteristics in large solid rocket motors. Sci. China Technol. Sci. 2015, 58, 1250–1262. [Google Scholar] [CrossRef]
- Wang, D.; Yang, Y.; Fan, W.; Li, X.; Gao, Y. Simulation of pressure oscillations in a combustion chamber under periodic inlet disturbances. Acta Astronaut. 2018, 152, 859–871. [Google Scholar] [CrossRef]
- Xingpeng, G.; Junwei, L.; Wensheng, Q.; Sheng, W.; Lei, H.; Qi, W.; Ningfei, W. Motion Trajectory of Solid Particles in Clxb Solid Rocket Motor. Acta Armamentarii 2022, 43, 489–502. [Google Scholar]
- Huo, C.; Luo, T.; Liu, P. Numerical Simulations of Vortex-Acoustic Coupling in Two-Phase Flow of VKI Motor. In Proceedings of the 2022 13th International Conference on Mechanical and Aerospace Engineering (ICMAE), Bratislava, Slovakia, 20–22 July 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 384–389. [Google Scholar]
- Ferretti, V. Numerical simulations of acoustic resonance of Solid Rocket Motor. In Proceedings of the 46th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Nashville, TN, USA, 25–28 July 2010. [Google Scholar]
- Aider, J.-L.; Danet, A. Large-eddy simulation study of upstream boundary conditions influence upon a backward-facing step flow. Comptes Rendus Mécanique 2006, 334, 447–453. [Google Scholar] [CrossRef]
- Neto, A.S.; Grand, D.; Metais, O.; Lesieur, M. Large-eddy simulation of the turbulent flow in the downstream region of a backward-facing step. Phys. Rev. Lett. 1991, 66, 2320. [Google Scholar] [CrossRef]
- Vuillot, F. Vortex-shedding phenomena in solid rocket motors. J. Propuls. Power 1995, 11, 626–639. [Google Scholar] [CrossRef]
- Ruifeng, T.; Pingan, L. Numerical Calculation of Heat Transfer and Fluid Flow; Harbin Engineering University Press: Harbin, China, 2015. [Google Scholar]
- Smagorinsky, J. General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather. Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Nicoud, F.; Ducros, F. Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Zahari, N.; Zawawi, M.; Sidek, L.; Mohamad, D.; Itam, Z.; Ramli, M.; Syamsir, A.; Abas, A.; Rashid, M. Introduction of discrete phase model (DPM) in fluid flow: A review. In Proceedings of the AIP Conference Proceedings, Ho Chi Minh, Vietnam, 29–30 April 2018. [Google Scholar]
- Wu, H.; Liu, T.; Yang, Y. Simulation of Particle Trajectory in T-shaped Pipes Based on the DPM Model. Sci. Technol. 2021, 17, 21–22. [Google Scholar]
- Kuipers, J.A.M.; van Duin, K.J.; van Beckum, F.P.H.; van Swaaij, W.P.M. A Numerical Model of Gas-fluidzed Beds. Chem. Eng. Sci. 1992, 47, 1913–1924. [Google Scholar] [CrossRef]
- Ergun, S. Fluid Flow through Packed Columns. Chem. Eng. Prog 1952, 48, 89–94. [Google Scholar]
- Wen, C.Y.; Yu, Y.H. Mechanics of Fluidization. Chem. Eng. Prog. Symp. Ser 1966, 162, 100–111. [Google Scholar]
- Lupoglazoff, N.; Vuillot, F. Numerical simulation of vortex shedding phenomenon in 2D test case solid rocket motors. In Proceedings of the 30th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992; p. 776. [Google Scholar]
- Patankar, S.V.; Spalding, D.B. Paper 5—A calculation proceduce for heat, mass and momentum transfer in three-dimensional parabolic flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Pergamon: Bergama, Turkey, 1983; pp. 54–73. [Google Scholar]
- Lupoglazoff, N.; Vuillot, F. Comparison between firing tests and numerical simulation of vortex shedding in a 2-D test solid motor. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993; p. 3066. [Google Scholar]
- Flatau, A.; Moorhem, W. Cold-flow investigation of vortex-shedding induced sound amplitude variations. In Proceedings of the 33rd Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 1995; p. 605. [Google Scholar]
- Zhang, X.Y.; He, G.Q.; Liu, P.J. Classification of corner vortex shedding in soild rocket motor. J. Aerosp. Power 2014, 29, 2003–2011. [Google Scholar]








| Part | Size |
|---|---|
| Upstream length of the step a | 350 mm |
| Downstream length of the step b | 350 mm |
| Nozzle length c | 50 mm |
| The angle of backward step α | 135° |
| Total Elements | Amplitude (Pa) | Frequency (Hz) |
|---|---|---|
| 520,000 | 4107 | 879.40 |
| 270,000 | 4125 | 881.74 |
| 140,000 | 4248 | 784.15 |
| Mode | Parameter | Simulation | Experiment | Error |
|---|---|---|---|---|
| 1 | Frequency of pressure oscillation/Hz | 881 | 850 | 3.57% |
| Amplitude of pressure oscillation/Pa | 4125 | 4254 | 3.13% | |
| 3 | Frequency of pressure oscillation/Hz | 2506 | 2540 | 1.32% |
| Amplitude of pressure oscillation/Pa | 1364 | 1328 | 2.74% |
| Case | Vortex Shedding Frequency (Hz) | Pressure Oscillation Frequency (Hz) | Pressure Oscillation Amplitude (‰) | |||
|---|---|---|---|---|---|---|
| Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | |
| L/D-4.2-1.7 | 1676 | 1471 | 1603 | 1406 | 0.59 | 0.54 |
| L/D-4.2-2.3 | 1557 | 1404 | 1327 | 1224 | 0.43 | 0.41 |
| L/D-4.2-3 | 1296 | 1192 | 1012 | 951 | 0.32 | 0.29 |
| L/D-4.2-3.5 | 1081 | 997 | 862 | 784 | 0.31 | 0.27 |
| Expansion Ratio | Vortex Shedding Frequency (Hz) | Pressure Oscillation Frequency (Hz) | Pressure Oscillation Amplitude (‰) | |||
|---|---|---|---|---|---|---|
| Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | |
| 1.4 | 1050 | 955 | 1528 | 1386 | 0.41 | 0.38 |
| 1.3 | 870 | 791 | 1224 | 1165 | 0.52 | 0.48 |
| 1.2 | 776 | 706 | 979 | 933 | 0.61 | 0.57 |
| 1.1 | 753 | 685 | 782 | 734 | 0.57 | 0.55 |
| Case | Upstream and Downstream Length Ratio of the Step | Vortex Shedding Frequency (Hz) | Pressure Oscillation Frequency (Hz) | Pressure Oscillation Amplitude (‰) | |||
|---|---|---|---|---|---|---|---|
| Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | Pure-Gas Flow | Two-Phase Flow | ||
| L/D-2-5 | 1/3 | 651 | 614 | 871 | 783 | 0.42 | 0.40 |
| L/D-2.8-4.7 | 1/2 | 693 | 643 | 926 | 833 | 0.31 | 0.29 |
| L/D-2.5-4 | 5/7 | 753 | 707 | 880 | 792 | 0.63 | 0.59 |
| L/D-4.2-3.5 | 1/1 | 1081 | 997 | 862 | 784 | 0.29 | 0.28 |
| L/D-5-3 | 7/5 | 1225 | 1151 | 1684 | 1512 | 0.33 | 0.32 |
| L/D-6.4-1.7 | 3/1 | 1256 | 1192 | 1728 | 1545 | 0.15 | 0.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huo, C.; Xu, H.; Hu, J.; Luo, T. Numerical Study on Pressure Oscillations in a Solid Rocket Motor with Backward Step Configuration Under Two-Phase Flow Interactions. Aerospace 2024, 11, 1054. https://doi.org/10.3390/aerospace11121054
Huo C, Xu H, Hu J, Luo T. Numerical Study on Pressure Oscillations in a Solid Rocket Motor with Backward Step Configuration Under Two-Phase Flow Interactions. Aerospace. 2024; 11(12):1054. https://doi.org/10.3390/aerospace11121054
Chicago/Turabian StyleHuo, Chao, Hongbo Xu, Jie Hu, and Tengfei Luo. 2024. "Numerical Study on Pressure Oscillations in a Solid Rocket Motor with Backward Step Configuration Under Two-Phase Flow Interactions" Aerospace 11, no. 12: 1054. https://doi.org/10.3390/aerospace11121054
APA StyleHuo, C., Xu, H., Hu, J., & Luo, T. (2024). Numerical Study on Pressure Oscillations in a Solid Rocket Motor with Backward Step Configuration Under Two-Phase Flow Interactions. Aerospace, 11(12), 1054. https://doi.org/10.3390/aerospace11121054





