The Impact of Battery Performance on Urban Air Mobility Operations
Abstract
1. Introduction
2. Models and Methods
2.1. Key Power Battery Parameters
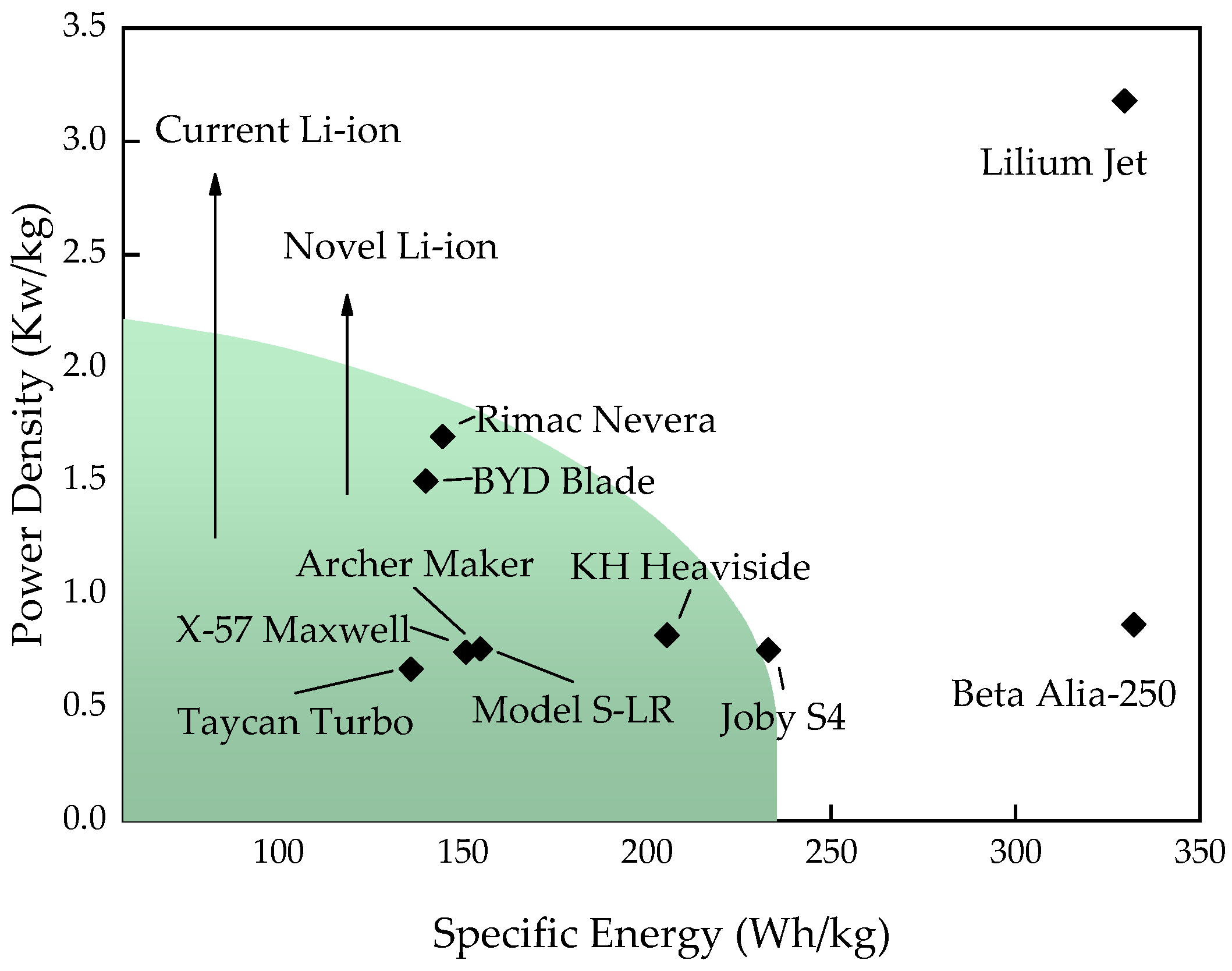
2.1.1. Specific Energy
2.1.2. Charge Rate
2.1.3. Other Parameters
2.2. Flight Profile
2.3. Direct Operating Cost
2.3.1. Acquisition Cost
2.3.2. Battery Cost
2.3.3. Depreciation Cost
2.3.4. Energy Cost
2.3.5. Maintenance Cost
2.3.6. Infrastructure Cost
2.3.7. Insurance and Service Fees
2.3.8. Pilot-Related Fees
2.4. DOC Calculation Formulae and Other Parameters
3. Results
3.1. Existing UAM Vehicle Results
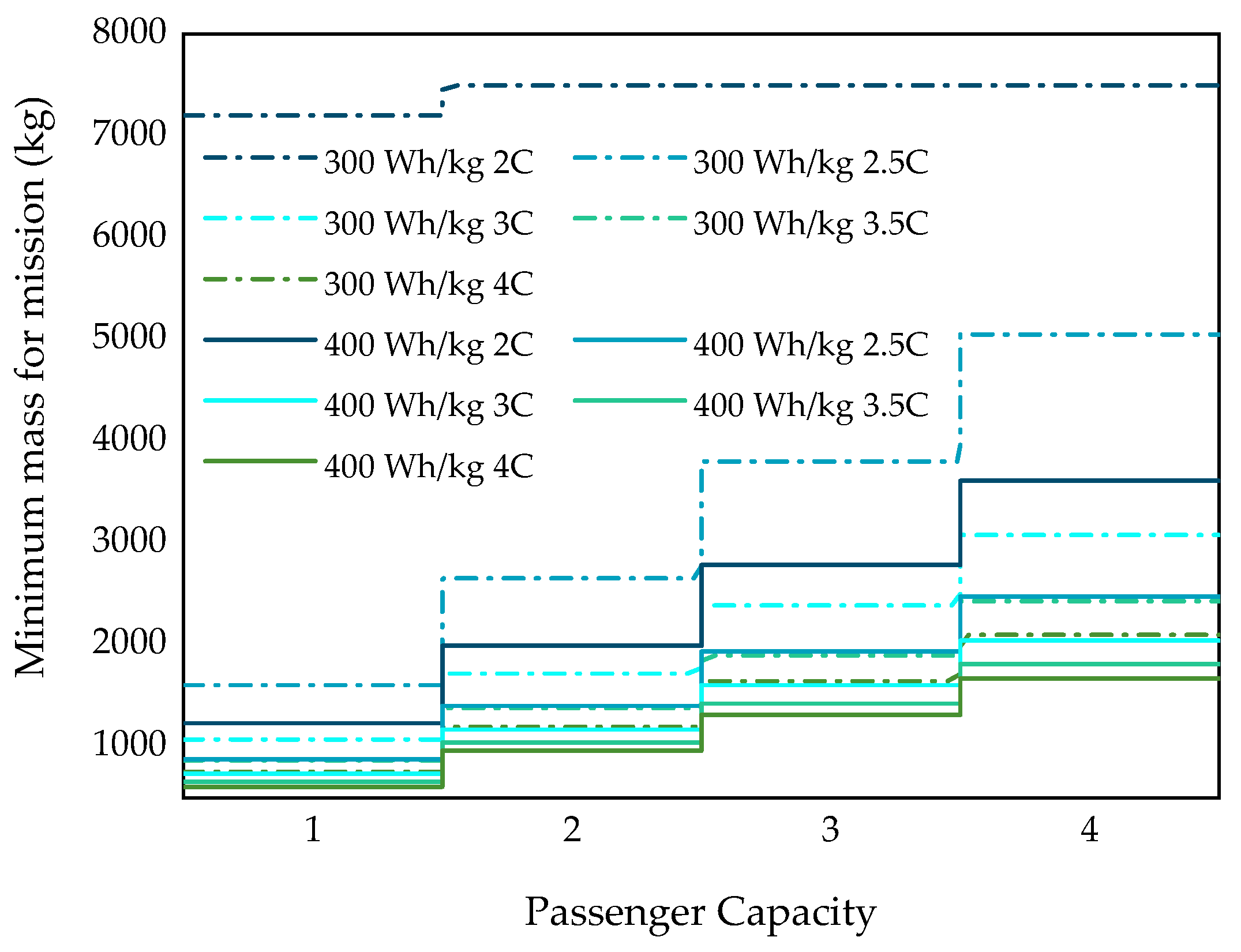
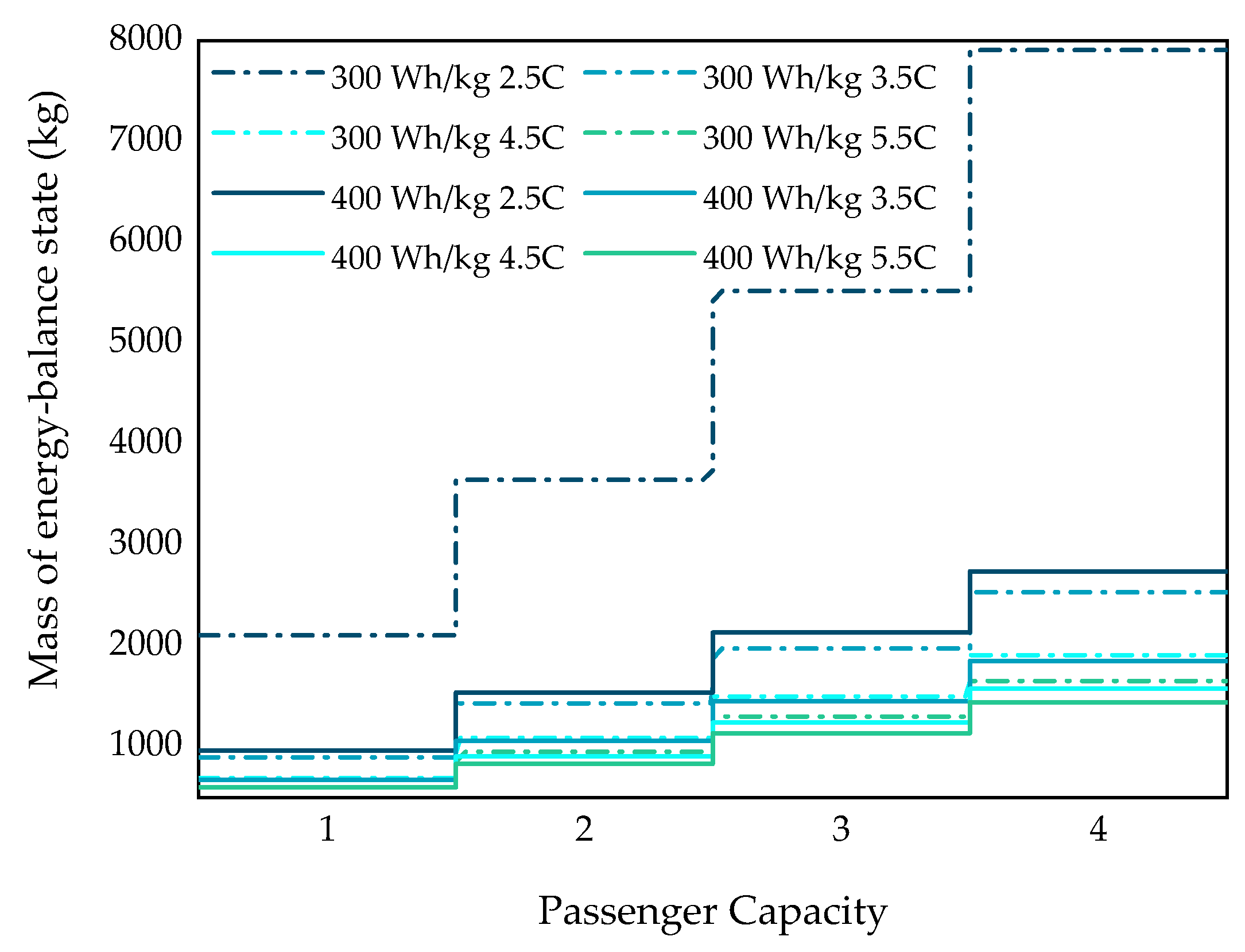
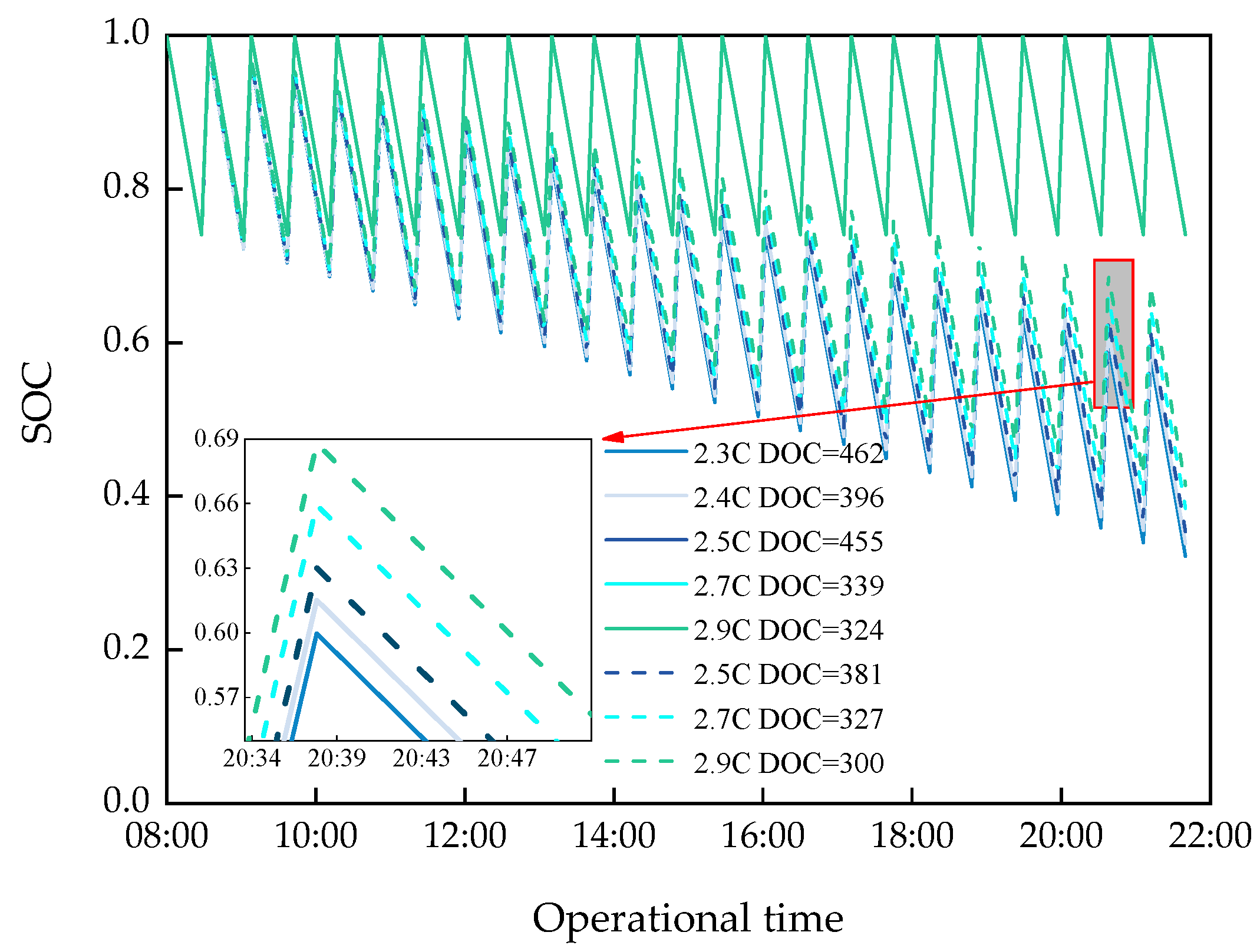
3.2. Effect of Charge Rate
3.3. Effect of Advances in Specific Energy
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Garrow, L.A.; German, B.J.; Leonard, C.E. Urban air mobility: A comprehensive review and comparative analysis with autonomous and electric ground transportation for informing future research. Transp. Res. Part C Emerg. Technol. 2021, 132, 103377. [Google Scholar] [CrossRef]
- Harish, A.; Perron, C.; Bavaro, D.; Ahuja, J.; Ozcan, M.; Justin, C.Y.; Briceno, S.; German, B.J.; Mavris, D.N. Economics of Advanced Thin-Haul Concepts and Operations. In Proceedings of the 16th AIAA Aviation Technology, Integration, and Operations Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar] [CrossRef]
- Howard, R.; Wright, E.; Mudumba, S.; Gunady, N.; Sells, B.; Maheshwari, A. Assessing the Suitability of Urban Air Mobility Vehicles for a Specific Aerodrome Network. In Proceedings of the Aiaa Aviation 2021 Forum, Online, 2–6 August 2021. [Google Scholar] [CrossRef]
- Goyal, R.; Reiche, C.; Fernando, C.; Cohen, A. Advanced Air Mobility: Demand Analysis and Market Potential of the Airport Shuttle and Air Taxi Markets. Sustainability 2021, 13, 7421. [Google Scholar] [CrossRef]
- Pertz, J.; Niklaß, M.; Swaid, M.; Gollnick, V.; Kopera, S.; Schunck, K.; Baur, S. Estimating the Economic Viability of Advanced Air Mobility Use Cases: Towards the Slope of Enlightenment. Drones 2023, 7, 75. [Google Scholar] [CrossRef]
- Kohlman, L.W.; Patterson, M.D.; Raabe, B.E. Urban Air Mobility Network and Vehicle Type—Modeling and Assessment. Available online: https://rotorcraft.arc.nasa.gov/Publications/files/Kohlman_TM-2019-220072_FINAL.pdf (accessed on 21 February 2022).
- Niklaß, M.; Dzikus, N.; Swaid, M.; Berling, J.; Lührs, B.; Lau, A.; Terekhov, I.; Gollnick, V. A Collaborative Approach for an Integrated Modeling of Urban Air Transportation Systems. Aerospace 2020, 7, 50. [Google Scholar] [CrossRef]
- Justin, C.Y.; Payan, A.P.; Briceno, S.I.; German, B.J.; Mavris, D.N. Power optimized battery swap and recharge strategies for electric aircraft operations. Transp. Res. Part C Emerg. Technol. 2020, 115, 102605. [Google Scholar] [CrossRef]
- Hamilton, T.; German, B.J. Optimal Airspeeds for Scheduled Electric Aircraft Operations. J. Aircr. 2019, 56, 545–555. [Google Scholar] [CrossRef]
- Kreimeier, M.; Stumpf, E.; Gottschalk, D. Economical assessment of air mobility on demand concepts with focus on Germany. In Proceedings of the 16th AIAA Aviation Technology, Integration, and Operations Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar] [CrossRef]
- Justin, C.Y.; Payan, A.P.; Briceno, S.I.; Mavris, D.N. Operational and Economic Feasibility of Electric Thin Haul Transportation. In Proceedings of the 17th AIAA Aviation Technology, Integration, and Operations Conference, Denver, Colorado, 5–9 June 2017. [Google Scholar] [CrossRef]
- Kreimeier, M.; Strathoff, P.; Gottschalk, D.; Stumpf, E. Economic Assessment of Air Mobility On-Demand Concepts. J. Air Transp. 2018, 26, 23–36. [Google Scholar] [CrossRef]
- Avanzini, G.; de Angelis, E.L.; Giulietti, F. Optimal performance and sizing of a battery-powered aircraft. Aerosp. Sci. Technol. 2016, 59, 132–144. [Google Scholar] [CrossRef]
- Traub, L.W. Optimal Battery Weight Fraction for Maximum Aircraft Range and Endurance. J. Aircr. 2016, 53, 1177–1179. [Google Scholar] [CrossRef]
- Gatti, M.; Giulietti, F.; Turci, M. Maximum endurance for battery-powered rotary-wing aircraft. Aerosp. Sci. Technol. 2015, 45, 174–179. [Google Scholar] [CrossRef]
- dos Reis, G.; Strange, C.; Yadav, M.; Li, S. Lithium-ion battery data and where to find it. Energy AI 2021, 5, 100081. [Google Scholar] [CrossRef]
- Hu, X.; Xu, L.; Lin, X.; Pecht, M. Battery Lifetime Prognostics. Joule 2020, 4, 310–346. [Google Scholar] [CrossRef]
- Sripad, S.; Viswanathan, V. The promise of energy-efficient battery-powered urban aircraft. Proc. Natl. Acad. Sci. USA 2021, 118, e2111164118. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Yang, X.-G.; Ge, S.; Leng, Y.; Wang, C.-Y. Ultrafast charging of energy-dense lithium-ion batteries for urban air mobility. eTransportation 2021, 7, 100103. [Google Scholar] [CrossRef]
- Tomaszewska, A.; Chu, Z.; Feng, X.; O’Kane, S.; Liu, X.; Chen, J.; Ji, C.; Endler, E.; Li, R.; Liu, L.; et al. Lithium-ion battery fast charging: A review. eTransportation 2019, 1, 100011. [Google Scholar] [CrossRef]
- Severson, K.A.; Attia, P.M.; Jin, N.; Perkins, N.; Jiang, B.; Yang, Z.; Chen, M.H.; Aykol, M.; Herring, P.K.; Fraggedakis, D.; et al. Data-driven prediction of battery cycle life before capacity degradation. Nat. Energy 2019, 4, 383–391. [Google Scholar] [CrossRef]
- Omar, N.; Monem, M.A.; Firouz, Y.; Salminen, J.; Smekens, J.; Hegazy, O.; Gaulous, H.; Mulder, G.; Van den Bossche, P.; Coosemans, T.; et al. Lithium iron phosphate based battery—Assessment of the aging parameters and development of cycle life model. Appl. Energy 2014, 113, 1575–1585. [Google Scholar] [CrossRef]
- Bocca, A.; Sassone, A.; Shin, D.; Macii, A.; Macii, E.; Poncino, M. An equation-based battery cycle life model for various battery chemistries. In Proceedings of the 2015 IFIP/IEEE International Conference on Very Large Scale Integration (VLSI-SoC), Daejeon, Republic of Korea, 5–7 October 2015; pp. 57–62. [Google Scholar]
- Lain, M.J.; Kendrick, E. Understanding the limitations of lithium ion batteries at high rates. J. Power Sources 2021, 493, 229690. [Google Scholar] [CrossRef]
- Ahn, B.; Hwang, H.-Y. Design Criteria and Accommodating Capacity Analysis of Vertiports for Urban Air Mobility and Its Application at Gimpo Airport in Korea. Appl. Sci. 2022, 12, 6077. [Google Scholar] [CrossRef]
- Boeing; Wisk. Concept of Operations for Uncrewed Urban Air Mobility. Available online: https://wisk.aero/wp-content/uploads/2022/09/Concept-of-Operations-for-Uncrewed-Urban-Air-Mobility.pdf (accessed on 22 September 2022).
- EASA. Prototype Technical Specifications for the Design of VFR Vertiports for Operation with Manned VTOL-Capable Aircraft Certified in the Enhanced Category. Available online: https://www.easa.europa.eu/downloads/136259/en (accessed on 24 March 2023).
- Uber. Fast-Forwarding to a Future of On-Demand Urban Air Transportation. Available online: https://evtol.news/__media/PDFs/UberElevateWhitePaperOct2016.pdf (accessed on 12 February 2023).
- Nathen, P.; Strohmayer, A.; Miller, R.; Grimshaw, S.D.; Taylor, J. Architectural Performance Assessment of an Electric Vertical Take-Off and Landing (e-VTOL) Aircraft Based on a Ducted Vectored Thrust Concept. Available online: https://lilium.com/files/redaktion/refresh_feb2021/investors/Lilium_7-Seater_Paper.pdf (accessed on 7 April 2023).
- Reiche, C.; Goyal, R.; Cohen, A.; Serrao, J.; Kimmel, S.; Fernando, C.; Shaheen, S. Urban Air Mobility Market Study. Natl. Aeronaut. Space Adm. 2018. [Google Scholar] [CrossRef]
- Brelje, B.J.; Martins, J.R.R.A. Electric, hybrid, and turboelectric fixed-wing aircraft: A review of concepts, models, and design approaches. Prog. Aerosp. Sci. 2019, 104, 1–19. [Google Scholar] [CrossRef]
- Lin, W.; Ren, H.; Peng, Q. A general analysis model of economical efficiency for regional aircraft operation. Civ. Aircr. Des. Res. 2019, 135, 21–30. [Google Scholar]
- Liao, L.; Ye, Y.; Tiehong, D. The Method and Application of the DOC Analysis in European Market. Civ. Aircr. Des. Res. 2013, 1, 1–4+22. [Google Scholar]
- Bower, G. Vahana Configuration Trade Study—Part II. Available online: https://acubed.airbus.com/blog/vahana/vahana-configuration-trade-study-part-ii/ (accessed on 1 February 2022).
- BloombergNEF. Electric Vehicle Outlook 2022. Available online: https://about.bnef.com/electric-vehicle-outlook/ (accessed on 23 May 2022).
- Kohlman, L.W.; Patterson, M.D. System-Level Urban Air Mobility Transportation Modeling and Determination of Energy-Related Constraints. In Proceedings of the 2018 Aviation Technology, Integration, and Operations Conference, Atlanta, Georgia, 25–29 June 2018. [Google Scholar] [CrossRef]
- Mathur, A.; Panesar, K.; Kim, J.; Atkins, E.M.; Sarter, N. Paths to Autonomous Vehicle Operations for Urban Air Mobility. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar] [CrossRef]
- EASA. Introduction of a Regulatory Framework for the Operation of Drones. Available online: https://unmanned-network.com/wp-content/uploads/elementor/forms/62c5abfdb275b.pdf (accessed on 1 June 2022).
- Howard, R.J.; Wright, E.C.; Mudumba, S.V.; Gunady, N.I.; Sells, B.E.; Maheshwari, A.; Delaurentis, D.A.; Crossley, W.A. Urban Air Mobility Vehicle Dataset. Purdue Univ. Res. Repos. 2021. [Google Scholar] [CrossRef]
- Ehang. The Future of Transportation: White Paper on Urban Air Mobility Systems. Available online: https://www.ehang.com/app/en/EHang%20White%20Paper%20on%20Urban%20Air%20Mobility%20Systems.pdf (accessed on 15 January 2022).
- Balli, M.E. EVTOL aircraft conceptual design and optimization. Sc. Di Ing. Ind. E Dell’informazione 2020. Available online: http://hdl.handle.net/10589/154226 (accessed on 17 April 2023).
- Liu, M.; Hao, H.; Lin, Z.; He, X.; Qian, Y.; Sun, X.; Geng, J.; Liu, Z.; Zhao, F. Flying cars economically favor battery electric over fuel cell and internal combustion engine. PNAS Nexus 2023, 2, pgad019. [Google Scholar] [CrossRef]
- Wu, D.; Wu, F. Toward better batteries: Solid-state battery roadmap 2035+. eTransportation 2023, 16, 100224. [Google Scholar] [CrossRef]
- Bloomberg, N.E.F. Hitting the EV Inflection Point. Available online: https://www.transportenvironment.org/wp-content/uploads/2021/08/2021_05_05_Electric_vehicle_price_parity_and_adoption_in_Europe_Final.pdf (accessed on 23 May 2022).


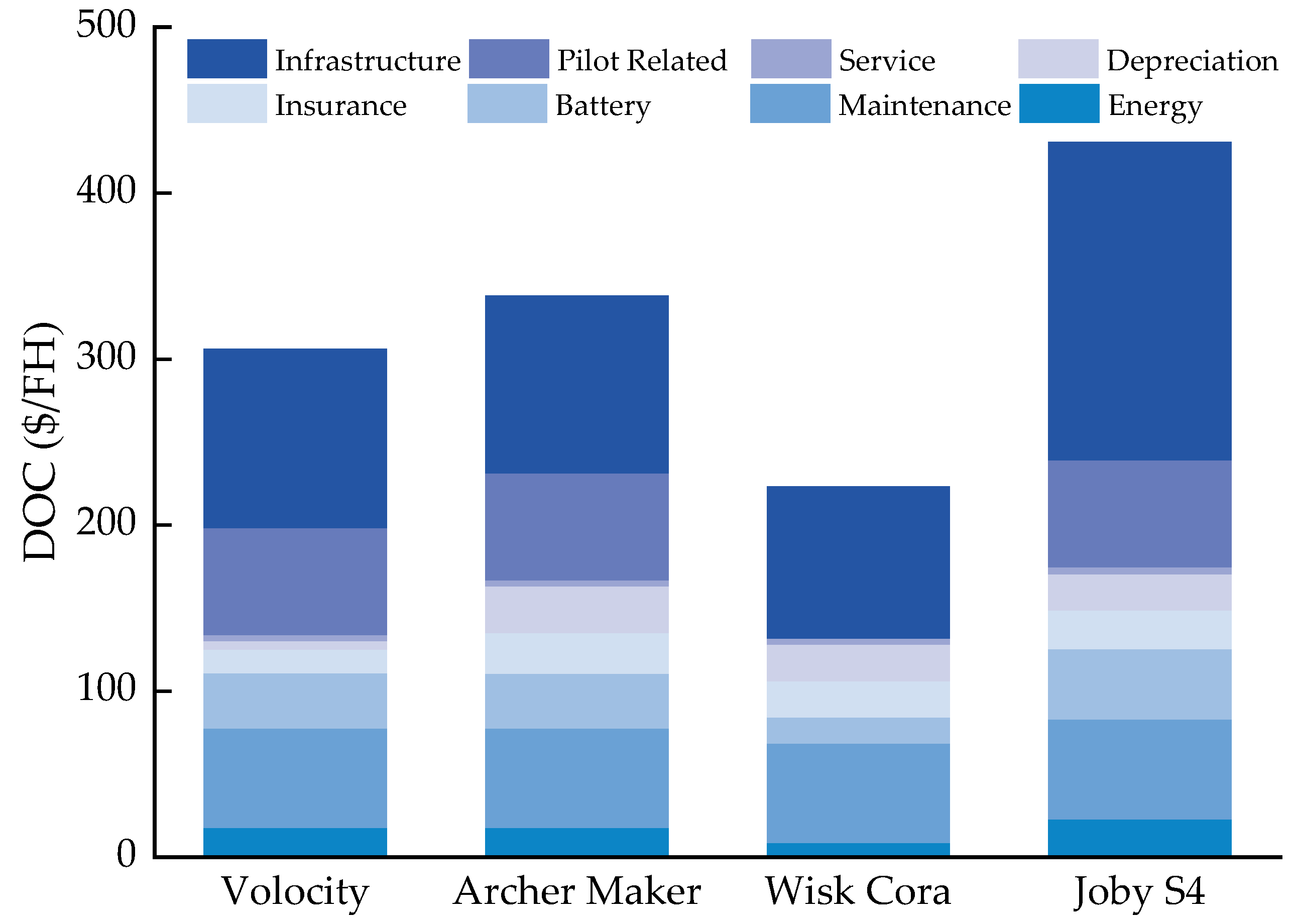
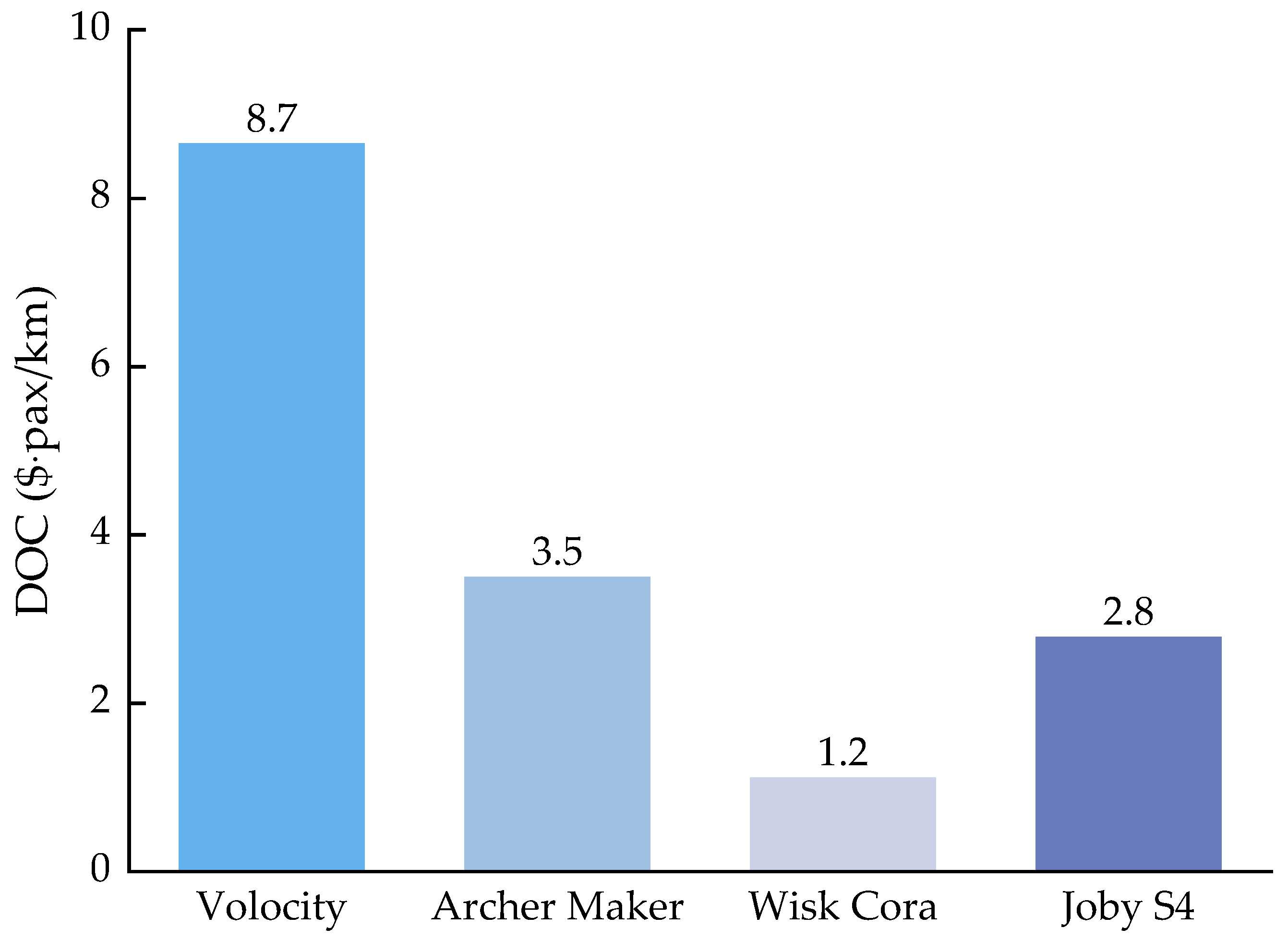
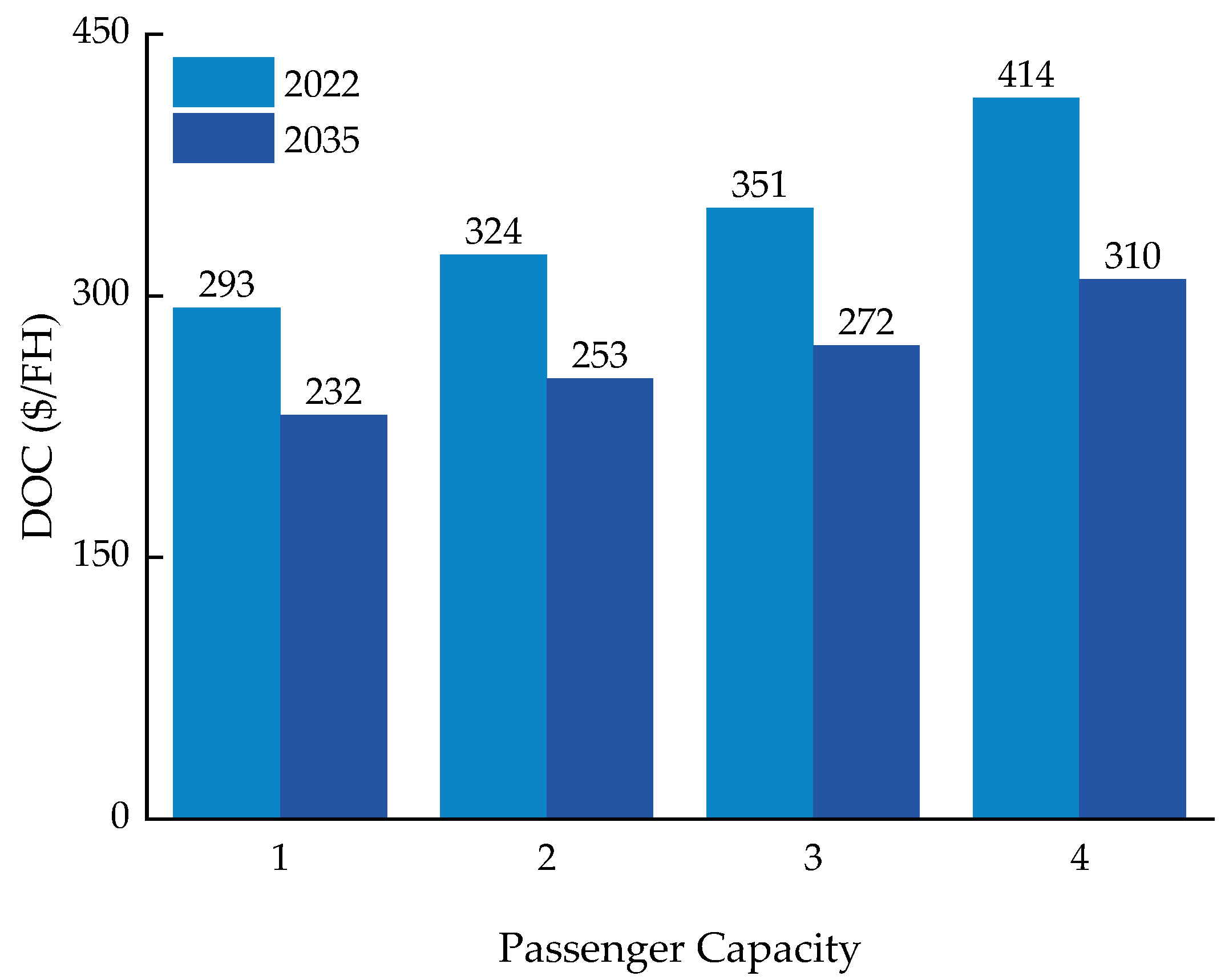
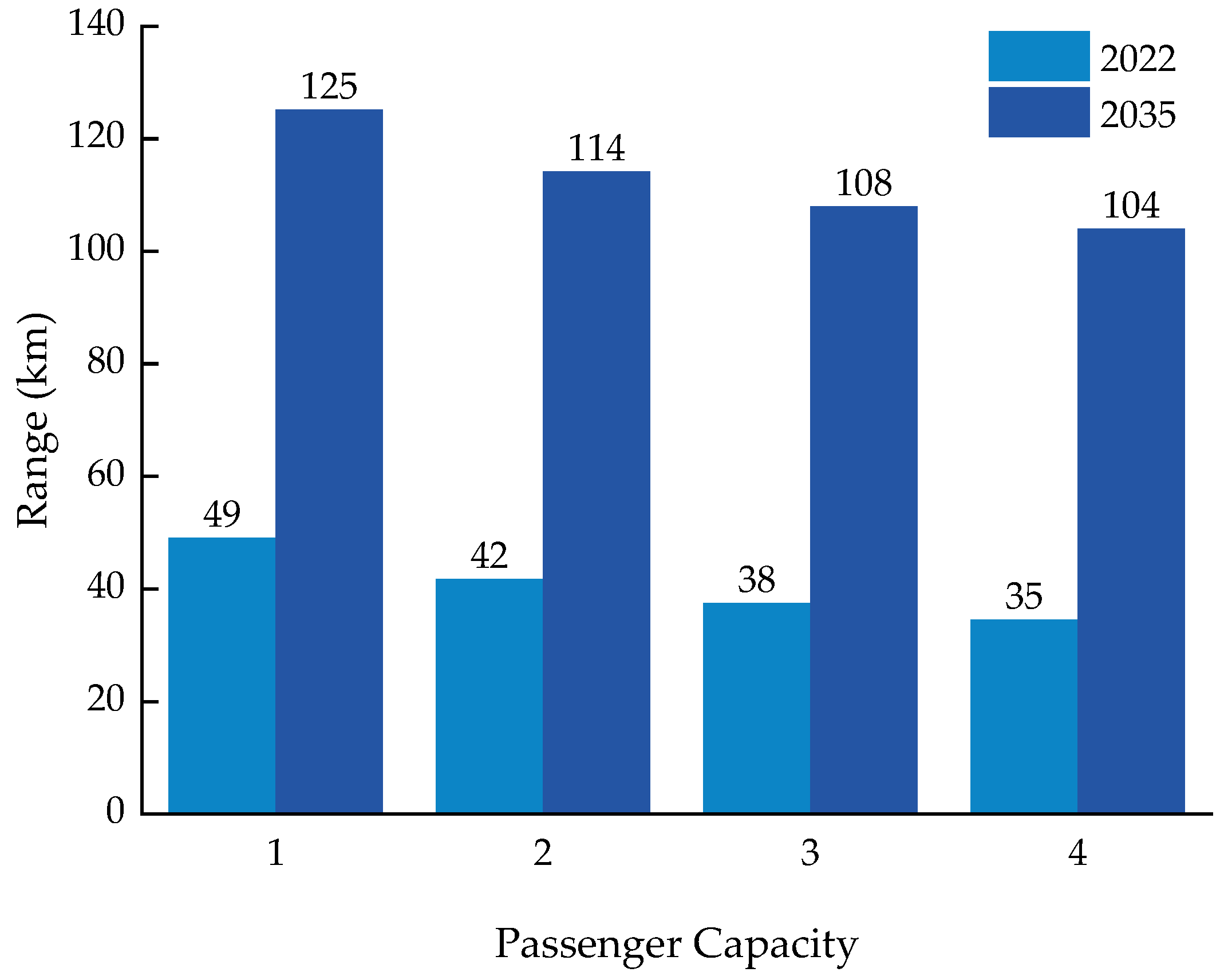
| Phase | Vertical Speed (m/s) | Horizontal Speed (m/s) | End Altitude (m) |
|---|---|---|---|
| A | 0–2.5 | 0 | 15 |
| B | 2.5 | 0–1.2 | 91 |
| C | 0 | 1.2 | 91 |
| D | 2.5 | 1.2 – | 457 |
| E | 0 | 457 | |
| F | 2.5 | –1.2 | 91 |
| G | 0 | 1.2 | 91 |
| H | 2.5–1.5 | 1.2 –0 | 15 |
| I | 1.5–0 | 0 | 0 |
| MTOW (kg) | Takeoff Fee ($) | Parking Fee ($/h) |
|---|---|---|
| 0–1814 | 50 | 30 |
| 1814–2721 | 64 | 30 |
| 2721–3629 | 74 | 300 |
| 3629–4536 | 90 | 40 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Empty weight factor | 0.6 | Hovering efficiency | 0.893 |
| Daily planning operation hours | 12 | FOM of vertical flight | 0.8 |
| Annual planning operation rate | 0.9 | Aerodynamic interference coefficient of vertical flight | 1.03 |
| Actual operating rate | 0.9 | L/D of cruise | 16 |
| Parameter | Volocity | Archer Maker | Wisk Cora | Joby S4 |
|---|---|---|---|---|
| Passengers | 1 | 1 | 1 | 4 |
| Cruise Speed (m/s) | 49.2 | 67.1 | 25.0 | 73.8 |
| Range (km) | 35.4 | 96.6 | 99.8 | 241.4 |
| MTOW (kg) | 900.0 | 1508.0 | 1224.0 | 2177.0 |
| Battery Capacity (kWh) | 83.3 | 74.0 | 63.0 | 200.0 |
| L/D | 2.5 | 11.3 | 13.9 | 13.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiao, X.; Chen, G.; Lin, W.; Zhou, J. The Impact of Battery Performance on Urban Air Mobility Operations. Aerospace 2023, 10, 631. https://doi.org/10.3390/aerospace10070631
Qiao X, Chen G, Lin W, Zhou J. The Impact of Battery Performance on Urban Air Mobility Operations. Aerospace. 2023; 10(7):631. https://doi.org/10.3390/aerospace10070631
Chicago/Turabian StyleQiao, Xiaotao, Guotao Chen, Weichao Lin, and Jun Zhou. 2023. "The Impact of Battery Performance on Urban Air Mobility Operations" Aerospace 10, no. 7: 631. https://doi.org/10.3390/aerospace10070631
APA StyleQiao, X., Chen, G., Lin, W., & Zhou, J. (2023). The Impact of Battery Performance on Urban Air Mobility Operations. Aerospace, 10(7), 631. https://doi.org/10.3390/aerospace10070631





