Flight Control Law for Stabilizing Transient Response of the Aircraft during Gun Firing
Abstract
1. Introduction
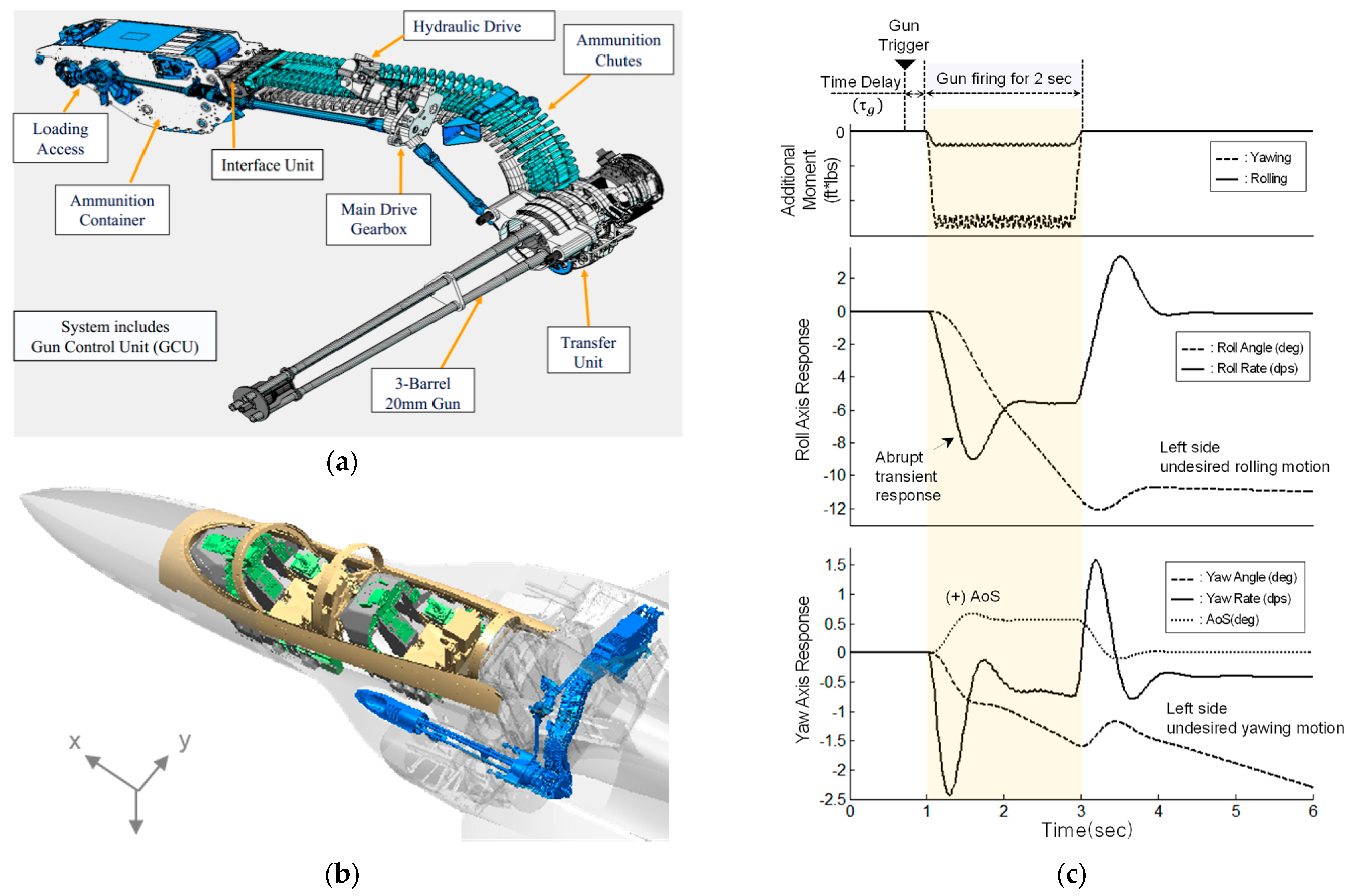
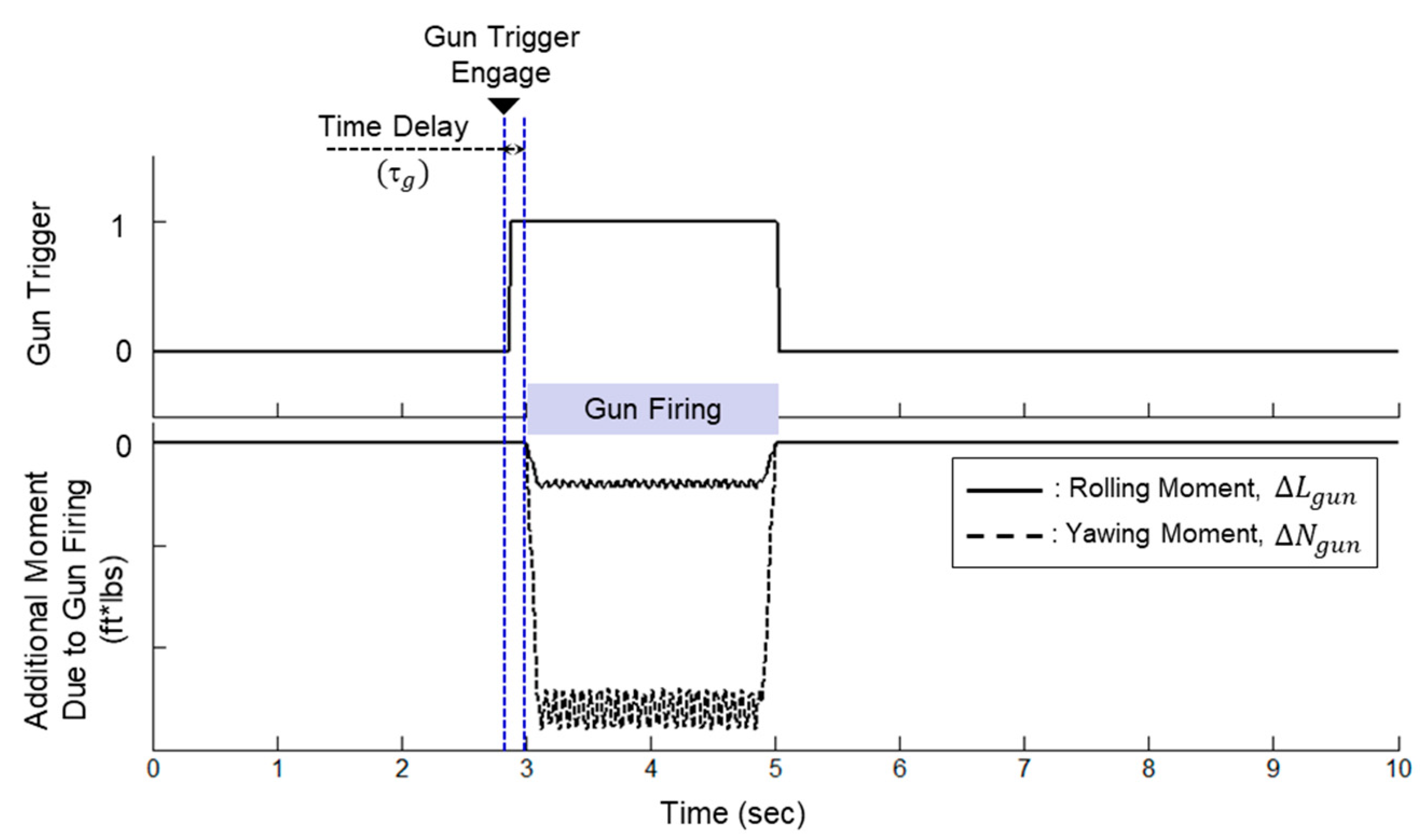
2. Gun Firing Effect
3. Flight Control Law Design
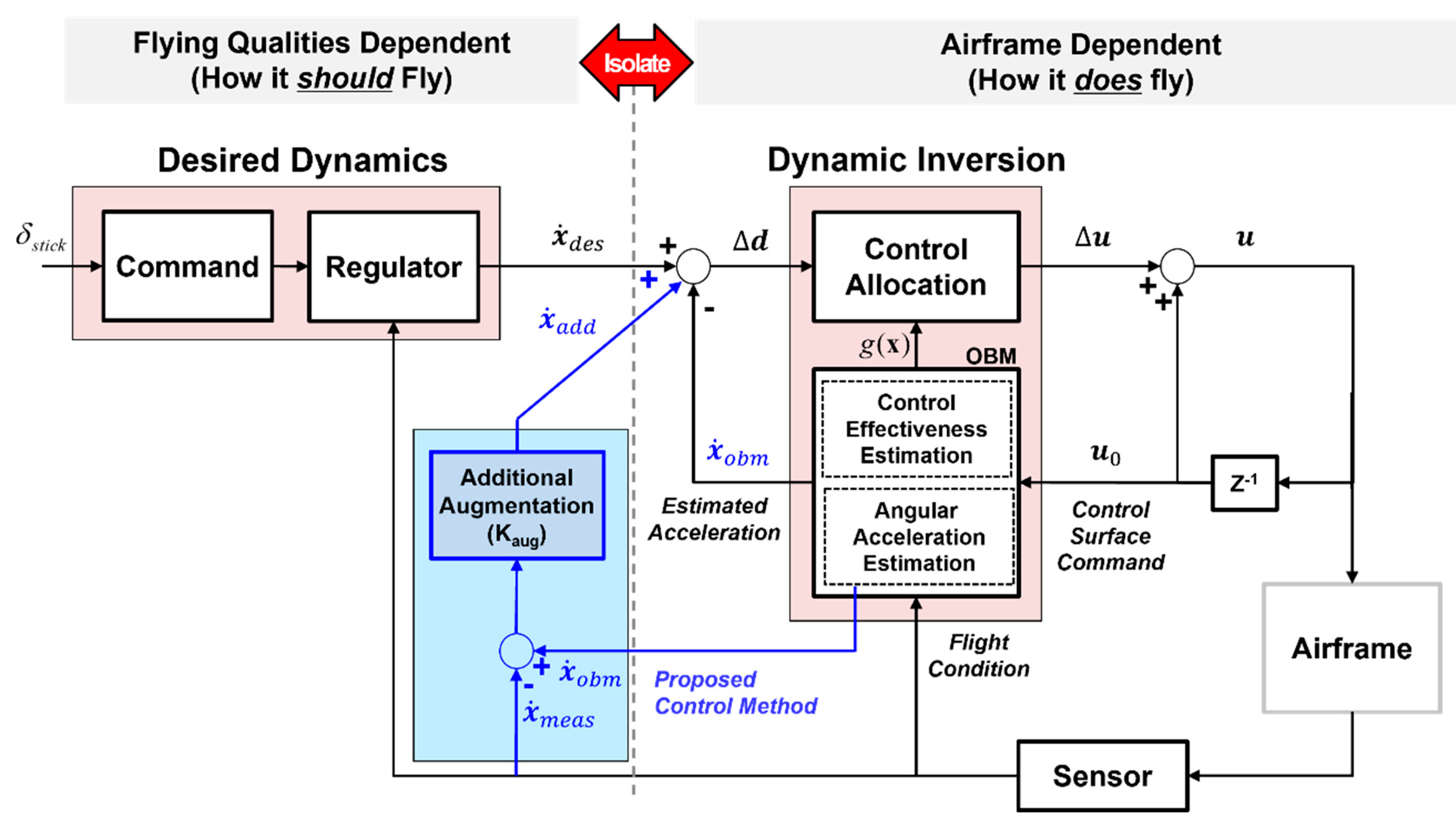
3.1. Fundamental INDI Control Methodology
3.2. Model-Based INDI
3.2.1. General
3.2.2. Control Surface Command Synchronization
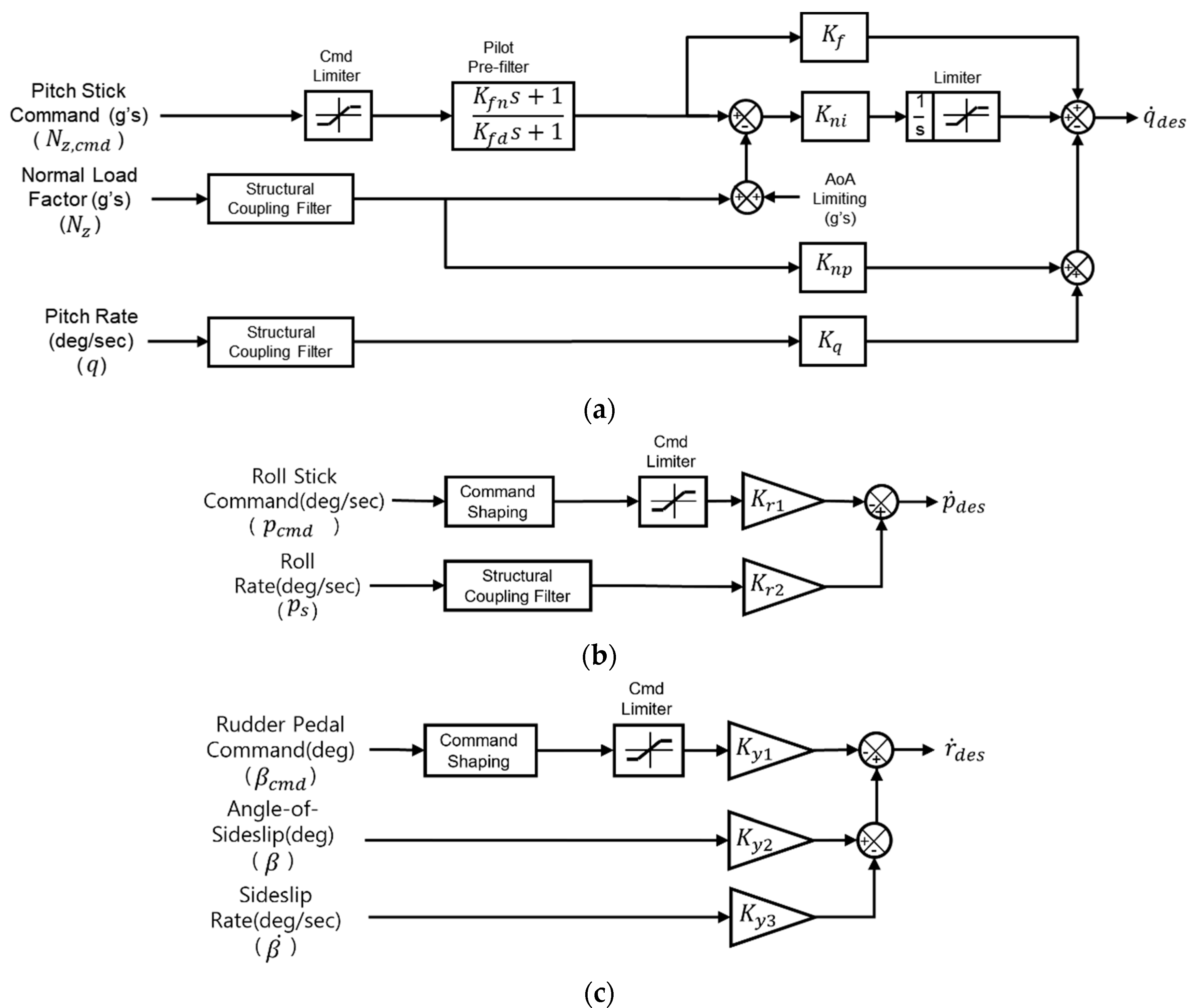
3.2.3. Desired Dynamics
3.2.4. Gun Compensation Control
3.3. Sensor-Based INDI
3.4. Angular Acceleration Estimation
3.5. Additional Augmention Control
4. Analysis and Evaluation Result
4.1. Evaluation Points and Method
4.2. Modeling of Additional Moments Due to Reaction Force
4.3. Flying Quality Analysis
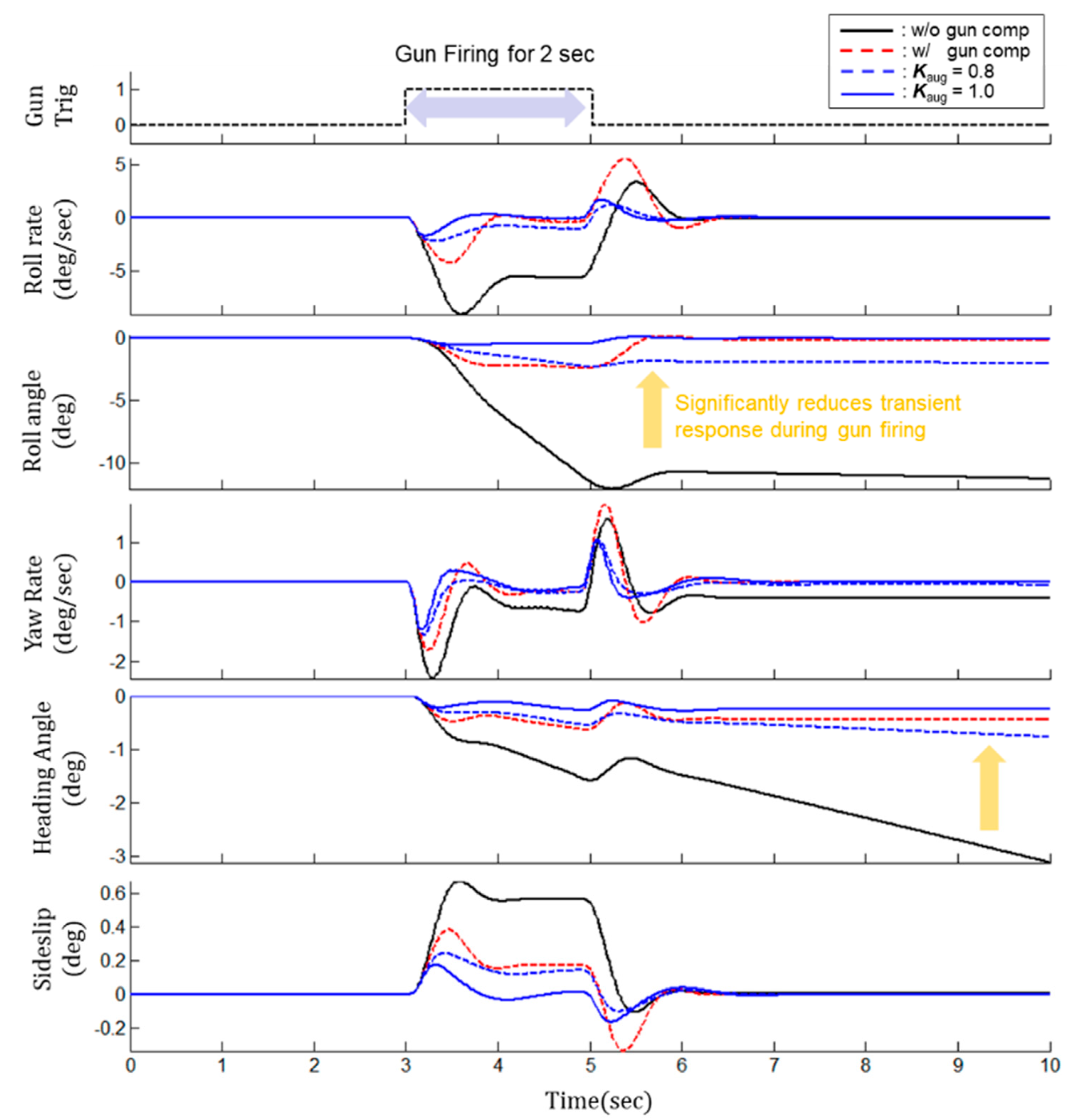
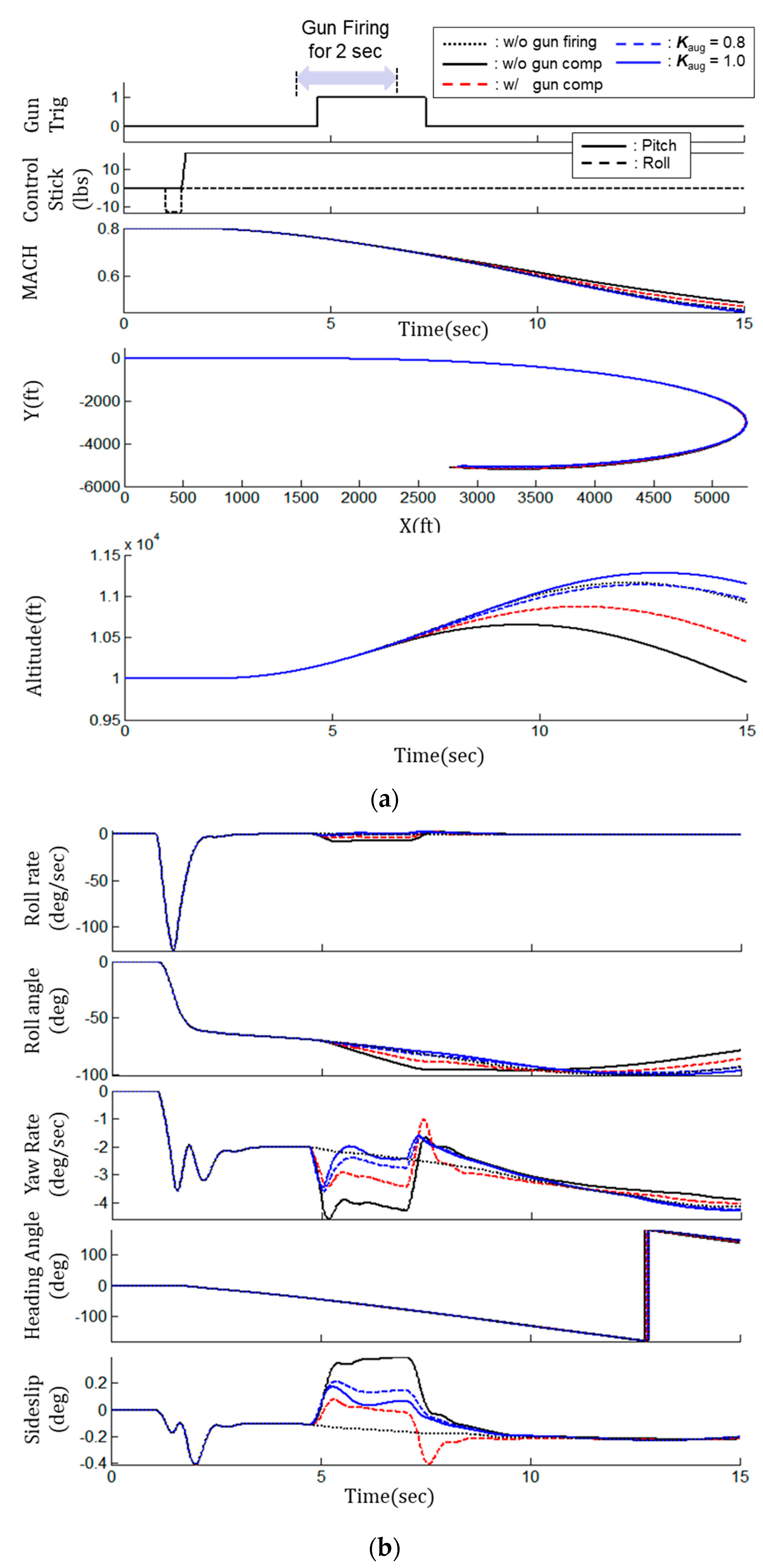
4.4. Transient Response Evaluation during Gun Firing
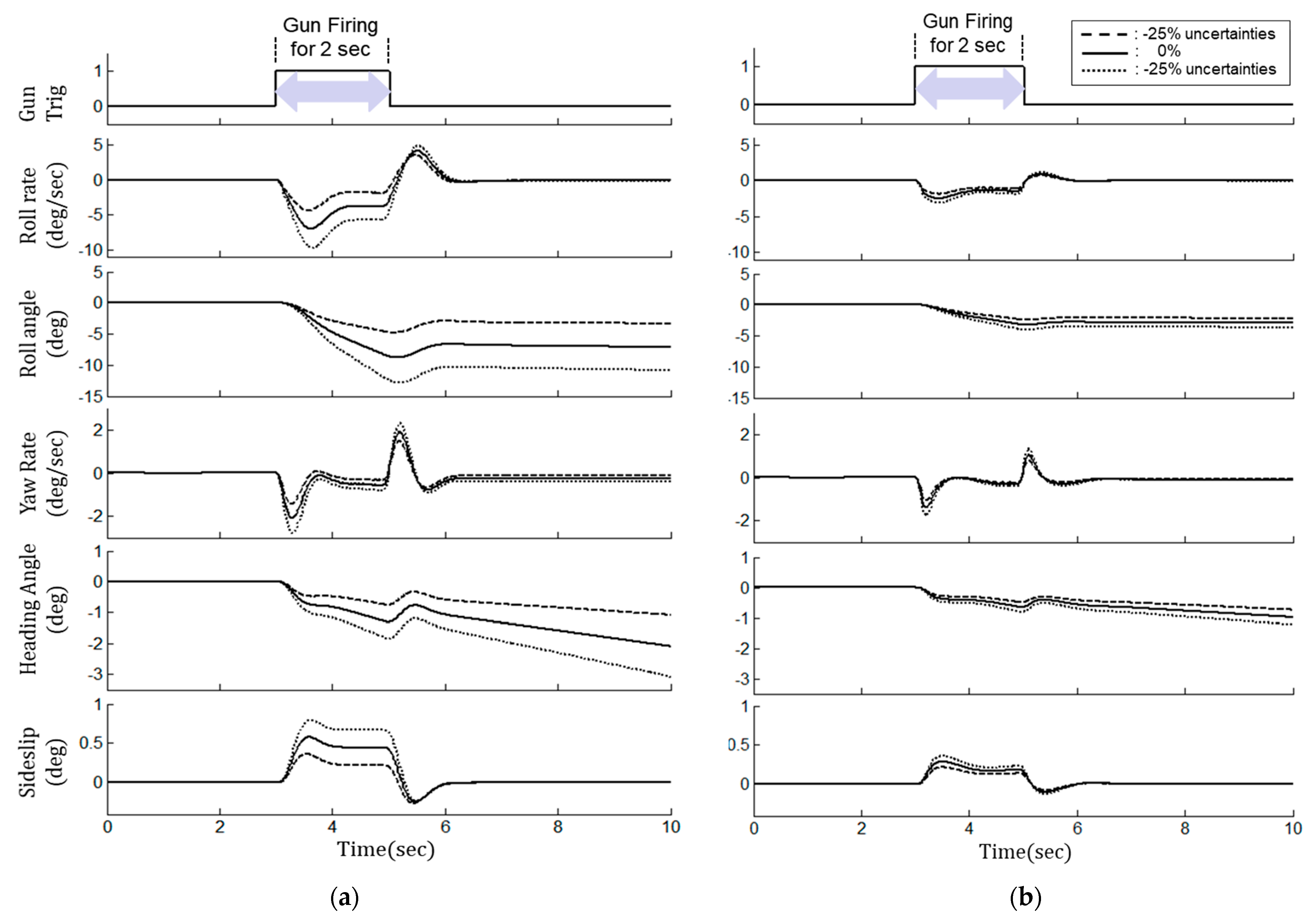
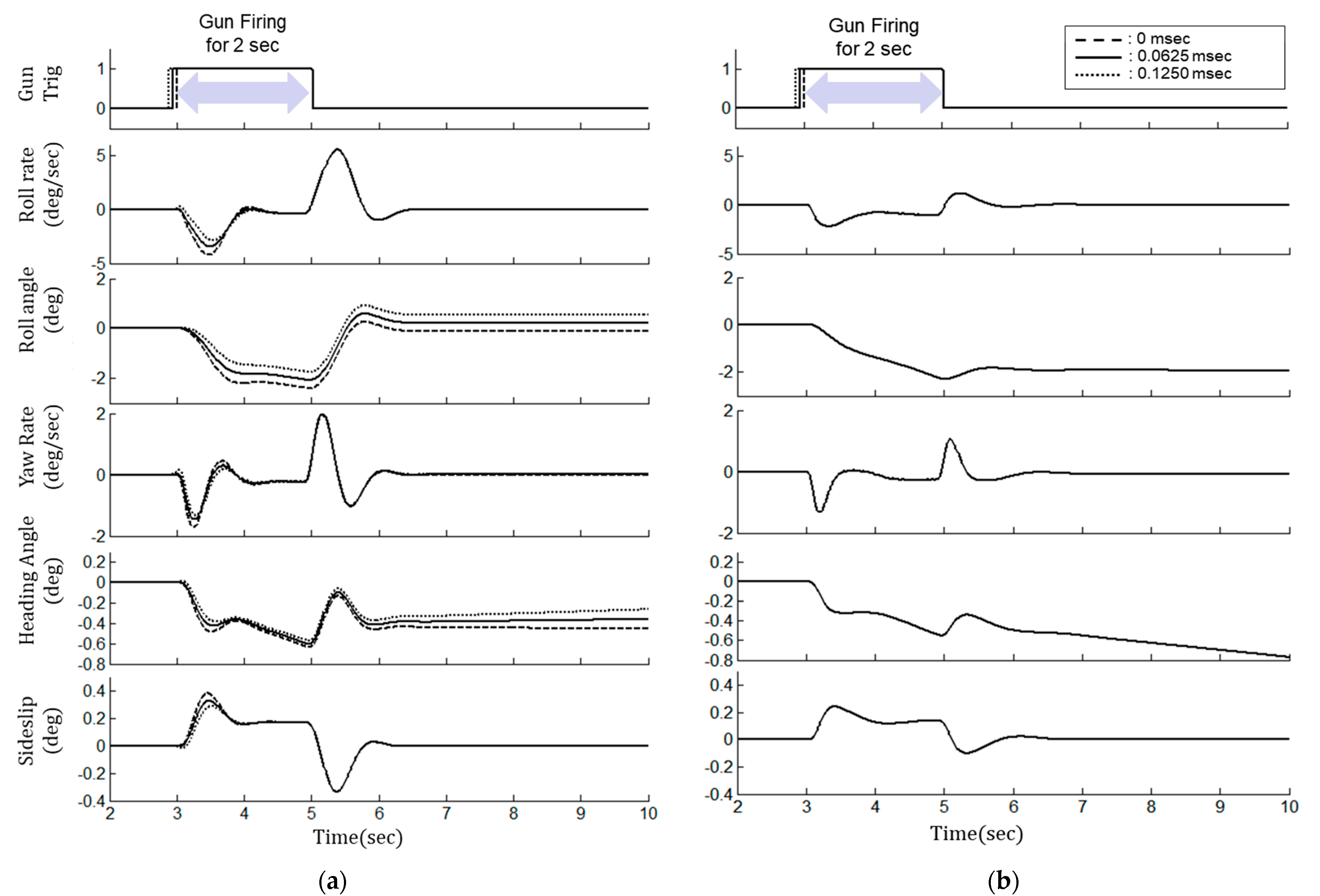
4.5. Robustness Analysis for Various Uncertainties
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| state vector | |
| control input vector | |
| nonlinear state dynamic function | |
| nonlinear control distribution function | |
| incremental control command (°) | |
| virtual control command (°) | |
| previous control command (°) | |
| additional augmentation control gains | |
| nonlinear state dynamic function of OBM | |
| nonlinear control distribution function of OBM | |
| rate of desired state vector (°/s2) | |
| rate of state vector calculated from OBM (°/s2) | |
| rate of state vector of additional augmentation control sensor (°/s2) | |
| pilot prefilter numerator gain | |
| pilot prefilter denominator gain | |
| forward gain | |
| integral gain to normal acceleration | |
| proportional gain to normal acceleration feedback | |
| proportional gain to pitch rate feedback | |
| flying quality parameter of roll command | |
| flying quality parameter of roll rate feedback | |
| flying quality parameter of yaw command | |
| flying quality parameter of sideslip feedback | |
| flying quality parameter of sideslip rate feedback | |
| stability axis roll rate (°/s) | |
| stability axis roll rate command (°/s) | |
| roll time constant (s) | |
| angle of sideslip (°) | |
| angle of sideslip command (°) | |
| Dutch roll frequency (rad) | |
| Dutch roll damping ratio | |
| pitch angular acceleration (°/s2) | |
| roll angular acceleration (°/s2) | |
| yaw angular acceleration (°/s2) | |
| desired pitch angular acceleration (°/s2) | |
| desired roll angular acceleration (°/s2) | |
| desired yaw angular acceleration (°/s2) | |
| roll rate (°/s) | |
| pitch rate (°/s) | |
| yaw rate (°/s) | |
| principal moment of inertia (slug-ft2) () | |
| production moment of inertia (slug-ft2) () | |
| rolling moment of the aircraft | |
| yawing moment of the aircraft | |
| rolling moment for () | |
| yawing moment for () | |
| control surface deflection for () | |
| additional augmentation control gains | |
| aircraft plant dynamics for control surface | |
| total angular acceleration feedback (°/s2) | |
| synchronization filter matrix | |
| damping ratio of 2nd order synchronization filter | |
| natural frequency of 2nd order synchronization filter (rad) | |
| pitch attitude time constant | |
| desired pitch attitude time constant | |
| resultant additional rolling moment from gun burst | |
| resultant additional pitching moment from gun burst | |
| resultant additional yawing moment from gun burst | |
| additional required symmetric horizontal control surface deflections | |
| additional required asymmetric horizontal control surface deflections | |
| additional required aileron control surface deflections | |
| additional required rudder control surface deflections | |
| control gain ratio of asymmetric horizontal control surface deflections | |
| gun burst signal | |
| time lag from gun burst signal to actual firing of the gun | |
| control gains of gun compensation control |
List of Acronyms
| AMRAAM | advanced medium-range air-to-air missile |
| HUD | head-up display |
| HQ | handling quality |
| INDI | incremental nonlinear dynamic inversion |
| IMU | inertial measurement unit |
| OML | outer mold line |
| AoA | angle of attack |
| AoS | angle-of-sideslip |
| CA | control allocation |
| RLSN | recursive linear smoothed Newton |
| DLR | German Aerospace Center |
| HARV | high angle-of-attack research |
| HOS | high-order system |
| LOES | low-order equivalent system |
| FLCC | flight control computer |
| JSF | joint strike fighter |
| LOES | low-order equivalent system |
| N/A | not applicable |
| NASA | National Aeronautics and Space Administration |
| NLR | Netherlands Aerospace Centre |
| OBM | onboard model |
| RESTORE | reconfigurable control for tailless aircraft |
| RSRI | rolling surface-to-rudder interconnect |
| SDT | slow down turn |
| STOVL | short take-off/vertical landing |
| VAAC | vectored thrust aircraft advanced control |
References
- Sobel, J.; U.S. Air Force Eglin AFB United States. AIM-120 Advanced Medium Range Air-to-Air Missile (AMRAAM). Defense Acquisition Management Information Retrieval (DAMIR), DoD, USA. 2015. Available online: https://www.esd.whs.mil/Portals/54/Documents/FOID/Reading%20Room/Selected_Acquisition_Reports/FY_2015_SARS/16-F-0402_DOC_14_AMRAAM_DEC_2015_SAR.pdf (accessed on 9 February 2023).
- Ji, C.; Kim, C.; Kim, B. A Hybrid Incremental Nonlinear Dynamic Inversion Control for Improving Flying Qualities of Asymmetric Store Configuration Aircraft. Aerospace 2021, 8, 126. [Google Scholar] [CrossRef]
- Ahmadi, K.; Asadi, D.; Nabavi-Chashmi, S.Y.; Tutsoy, O. Modified adaptive discrete-time incremental nonlinear dynamic inversion control for quad-rotors in the presence of motor faults. Mech. Syst. Signal Process. 2023, 188, 109989. [Google Scholar] [CrossRef]
- Anonymous. General Dynamics Awarded $10.2 Million For Super Hornet Guns. Def. Dly. 2003, 218, 1. [Google Scholar]
- Anonymous. GD Awarded $18.9 Million Contract to Supply F-15K Gun Systems. Def. Dly. Int. 2003, 4, 1. [Google Scholar]
- Anonymous. Lockheed Martin Completes F-22 Gun Check. Def. Dly. 2000, 205, 1. [Google Scholar]
- Anonymous. Second T-50 Advanced Jet Trainer Prototype Conducts Initial Flight. Def. Dly. Int. 2002, 3, 1. [Google Scholar]
- Choi, D.; Lee, E.; Cho, H.; Song, J. A Study of Enhanced Air-to-Air Gunnery Algorithm Development. J. Korean Soc. Aeronaut. Space Sci. 2007, 2007, 537–540. [Google Scholar]
- Joe, H.; Shin, D.; Jo, J.; Yang, S.; Ryu, K. Air-to-air and air-to-ground engagement modeling for the KAI embedded training system. In Proceedings of the AIAA Modeling and Simulation Technologies Conference and Exhibit, AIAA-2008-6859, Reston, VA, USA, 18–21 August 2008; pp. 18–21. [Google Scholar] [CrossRef]
- Hankins, M.W. The Phantom menace: The F-4 in Air Combat in Vietnam. Master Thesis, History Department, North Texas University, Denton, TX, USA, 2013. [Google Scholar]
- MIL-STD-1797A; Anon. Military Standard Flying Qualities of Piloted Aircraft. 1990.
- Kim, B.; Kim, Y. T-50 20mm Gun System Design. In Proceeding of The Korean Society for Aeronautical and Space Sciences; The Korean Society for Aeronautical and Space Sciences: Seoul, Republic of Korea, 2004; Volume 2004, pp. 754–757. [Google Scholar]
- Harris, J.J.; Standfords, J.R. F-35 flight control law design, development, and verification. In Proceedings of the American Institute of Aeronautics and Astronautics Aviation Forum, 2018 Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar] [CrossRef]
- Smith, P.; Berry, A. Flight test experience of a nonlinear dynamic inversion control law on the VAAC harrier. In Proceedings of the Atmospheric flight Mechanics Conference, Denver, CO, USA, 14–17 August 2000. [Google Scholar] [CrossRef]
- Smith, P.R. A simplified approach to non-linear dynamic inversion based flight control. In Proceedings of the 1998 American Institute of Aeronautics and Astronautics, Atmospheric Flight Mechanics Conference, Boston, MA, USA, 10–12 August 1998; pp. 762–770. [Google Scholar] [CrossRef]
- Buffington, J.M. Modular Control Law Design for the Innovative Control Effectors (ICE) Tailless Fighter Aircraft Configuration; Wright-Patterson Air Force Base: Patterson, OH, USA, 1999. [Google Scholar]
- Grondman, F.; Looye, G.; Kuchar, R.O.; Chu, Q.P.; Van Kampen, E.-J. Design and Flight Testing of Incremental Nonlinear Dynamic Inversion Based Control Laws for a Passenger Aircraft; AIAA Sci-Tech Forum: Kissimmee, FL, USA, 2018. [Google Scholar]
- Veld, R.V.; Van Kampen, E.-J.; Chu, Q.P. Analysis and Robustness Analysis and Improvements for Incremental Nonlinear Dynamic Inversion Control. In Proceedings of the 2018 AIAA Guidance, Navigation, and Control Conference, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- Wang, X.; Van Kampen, E.J.; Chu, Q.; Lu, P. Stability Analysis for Incremental Nonlinear Dynamic Inversion Control. In Proceedings of the 2019 American Institute of Aeronautics and Astronautics (AIAA), 4 March 2019; Volume 42, pp. 1116–1129. [Google Scholar] [CrossRef]
- Kim, C.-S.; Ji, C.-H.; Kim, B.S. Development of Flight Control Law for Improvement of Uncommanded Lateral Motion of the Fighter Aircraft. Int. J. Aeronaut. Space Sci. 2020, 21, 1059–1077. [Google Scholar] [CrossRef]
- Kim, C.-S.; Jin, T.; Koh, G.-O.; Kim, B.S. Control law design to improve the unexpected pitch motion in slow down turn maneuver. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2021, 236, 123–139. [Google Scholar] [CrossRef]
- Yang, J.; Zhu, J. Prop-hanging Control of a Thrust Vector Vehicle with Hybrid Nonlinear Dynamic Inversion Method. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 2999–3005. [Google Scholar] [CrossRef]
- Yang, J.; Zhu, J. A Hybrid NDI Control Method for the High-alpha Super-Maneuver Flight Control. In Proceedings of the 2016 American Control Conference (ACC), Boston, MA, USA, 6–8 July 2016; pp. 6747–6753. [Google Scholar]
- Walker, G.; Allen, D. X-35B STOVL flight control law design and flying qualities. In Proceedings of the 2002 Biennial International Powered Lift Conference and Exhibit, Wiliamsbrg, VA, USA, 5–7 November 2002. [Google Scholar] [CrossRef]
- Gola, A.; Chiesa, E.; Lasalandra, E.; Pasolini, F.; Tronconi, M.; Ungaretti, T.; Baschirotto, A. Interface for MEMS-based rotational accelerometer for HDD applications with 2.5 rad/s/sup 2/ resolution and digital output. IEEE Sensors J. 2003, 3, 383–392. [Google Scholar] [CrossRef]
- Godler, I.; Akahane, A.; Ohnishi, K.; Yamashita, T. A novel rotary acceleration sensor. IEEE Control Syst. 1995, 15, 56–60. [Google Scholar]
- Smeur, E.; de Croon, G.; Chu, Q. Cascaded incremental nonlinear dynamic inversion for MAV disturbance rejection. Control. Eng. Pract. 2018, 73, 79–90. [Google Scholar] [CrossRef]
- Gary, B.; Garrard, W.; Reiner, J. Robust dynamic inversion control laws for aircraft control. In Proceedings of the Guidance, Navigation and Control Conference, Hilton Head Island, SC, USA, 17 August 1992; p. 4329. [Google Scholar] [CrossRef]
- Honeywell Inc Minneapolis Mn. Application of multivariable control theory to aircraft control laws. In Final Report: Multivariable Control Design Guidelines; Flight Dynamics Directorate Wright Laboratory Air Force Materiel Command Wright-Patterson Air Force Base: Patterson, OH, USA, 1996. [Google Scholar]
- Daigoro, I.; Georgie, J.; Valasek, J.; Ward, D.T. Reentry Vehicle Flight Controls Design Guidelines: Dynamic Inversion; NASA Center for AeroSpace Information: Hanover, MD, USA, 2002.
- Gibson, J.C. Development of a Methodology for Excellence in Handling Qualities Design for Fly by Wire Aircraft; TU Delft: Delft, The Netherlands, 1999. [Google Scholar]
- Koschorke, J.; Falkena, W.; Van Kampen, E.J.; Chu, Q.P. Time delayed incremental nonlinear control. In Proceedings of the AIAA Guidance, Navigation, and Control (GNC) Conference, Boston, MA, USA, 19–22 August 2013; p. 4929. [Google Scholar] [CrossRef]
- Wu, X.S.; Chen, W.Y. Review on angular accelerometer development. J. Chin. Inert. Technol. 2007, 15, 458–463. [Google Scholar]
- Hong-Xing, D.; Wang, X.-B.; Liu, K. Design of an angular acceleration boundary observer. J. Harbin Inst. Technol. 2010, 42, 1504–1508. [Google Scholar]
- Wang, X.; Zhang, W.; Li, W. Inertia electrical emulation and angular acceleration estimation for transmission test rig. J. Southeast Univ. (Nat. Sci. Ed.) 2012, 42, 62–66. [Google Scholar]
- Brown, R.H.; Schneider, S.C.; Mulligan, M.G. Analysis of algorithms for velocity estimation from discrete position versus time data. IEEE Trans. Ind. Electron. 1992, 39, 11–19. [Google Scholar] [CrossRef]
- Ovaska, S.; Valiviita, S. Angular acceleration measurement: A review. In Proceedings of the IMTC/98 Conference Proceedings, IEEE Instrumentation and Measurement Technology Conference, St. Paul, MN, USA, 18–21 May 1998; Volume 2, pp. 875–880. [Google Scholar] [CrossRef]
- Han, J.; He, Y.; Xu, W. Angular acceleration estimation and feedback control: An experimental investigation. Mechatronics 2007, 17, 524–532. [Google Scholar] [CrossRef]
- Belanger, P.; Dobrovolny, P.; Helmy, A.; Zhang, X. Estimation of Angular Velocity and Acceleration from Shaft-Encoder Measurements. Int. J. Robot. Res. 1998, 17, 1225–1233. [Google Scholar] [CrossRef]
- Yang, J.; Zhu, J. An angular acceleration estimation method based on the complementary filter theory. In Proceedings of the 2016 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Taipei, Taiwan, 23–26 May 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Kim, C.-S.; Ji, C.-H.; Koh, G.-O.; Kim, B.S. Stability Margin and Structural Coupling Analysis of a Hybrid INDI Control for the Fighter Aircraft. Int. J. Aeronaut. Space Sci. 2021, 22, 1154–1169. [Google Scholar] [CrossRef]
- Anonymous. MIL-HDBK-516B (W/CHANGE 1); Department of Defense Handbook: Airworthiness Certification Criteria. 2008. Available online: http://everyspec.com/MIL-HDBK/MIL-HDBK-0500-0599/MIL-HDBK-516B_CHANGE-1_10217/ (accessed on 9 February 2023).

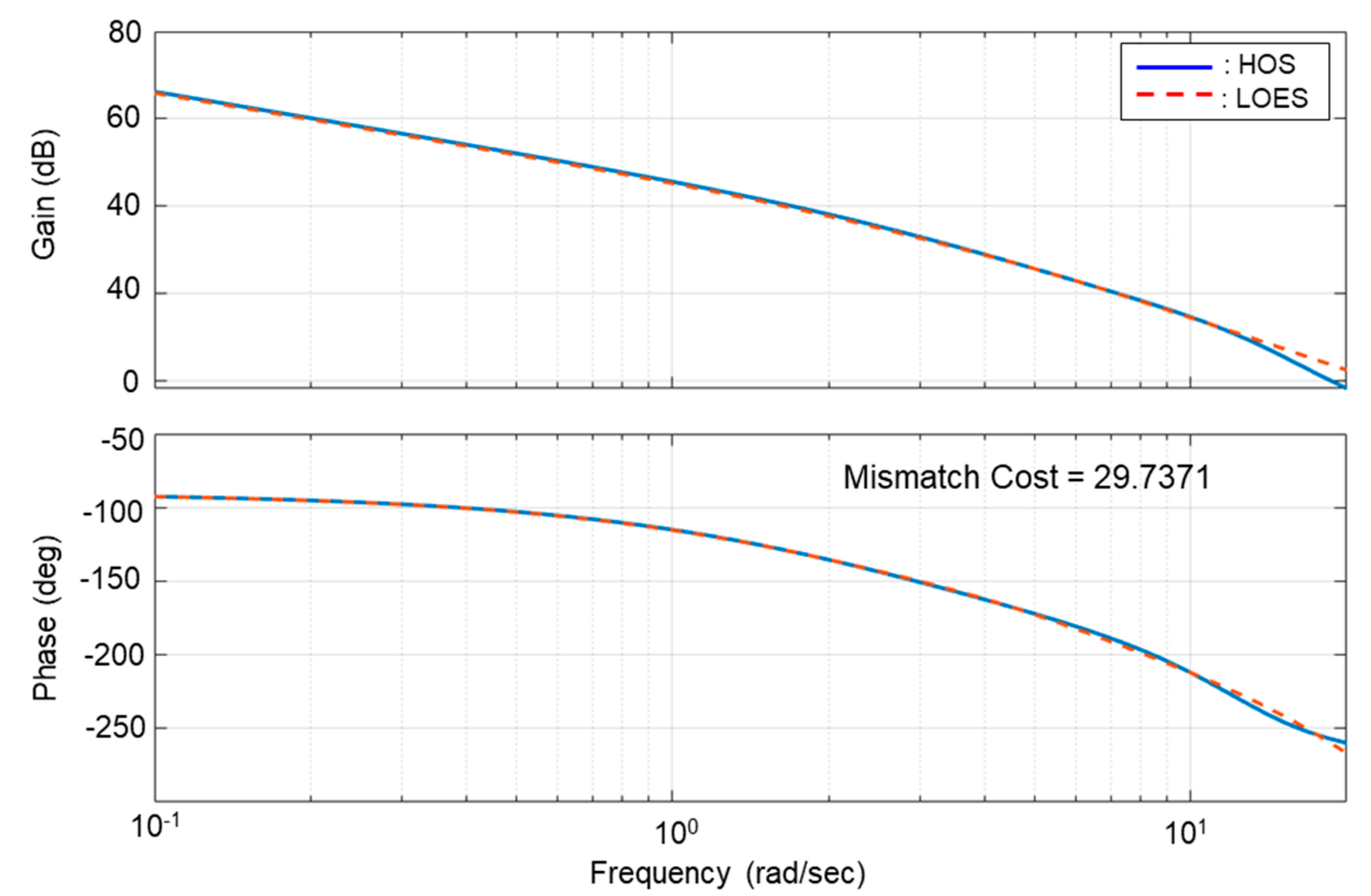
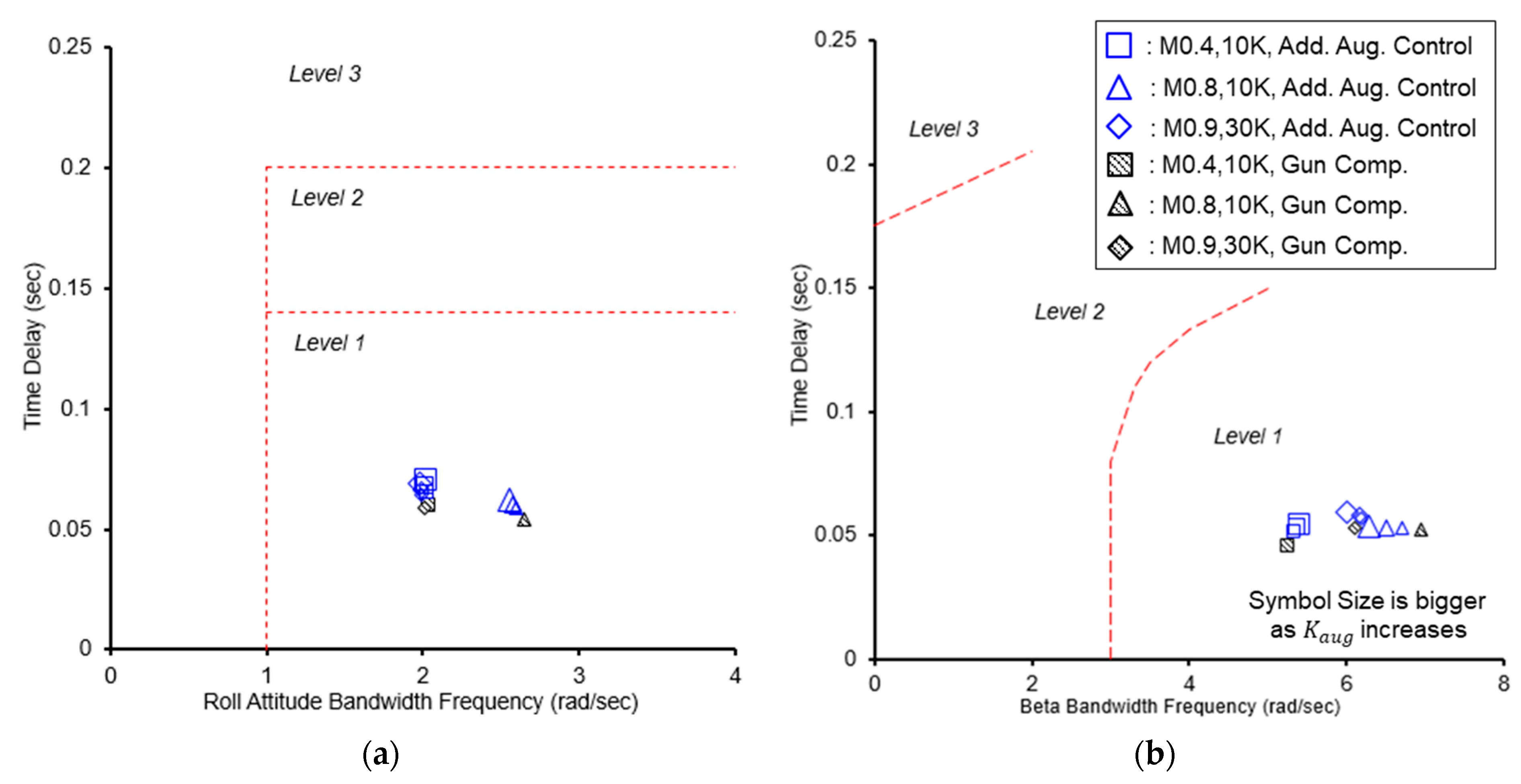
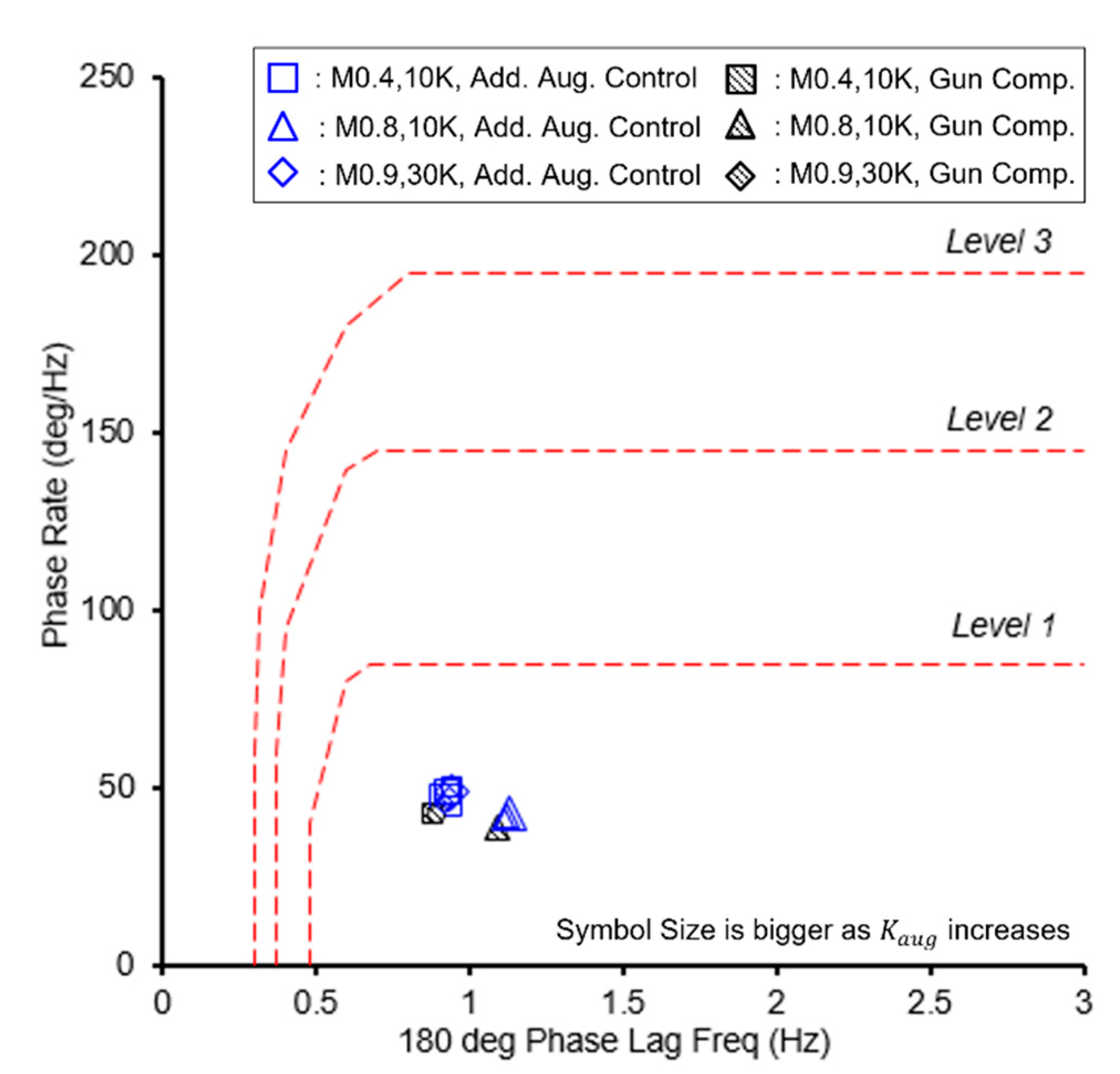

| Mach | Airspeed (knots) | Alt (kft) | Inner-Loop Control Gains | Gun Compensation Control Gain | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Kr1 | Kr2 | Ky1 | Ky2 | Ky3 | Kg1 | Kg2 | Kg3 | Kg4 | Kg5 | Kea | ||||
| 0.4 | 220 | 10 | 0.0 | −2.9 | −2.9 | −12.2 | 12.3 | −4.9 | 1.4 | 0.2 | 0.3 | 0.5 | 0.6 | 0.6 |
| 0.8 | 448 | 10 | −4.6 | −4.6 | −22.9 | 22.9 | −6.7 | 1.4 | 0.003 | 0.2 | 0.5 | 0.6 | 0.6 | |
| 0.8 | 346 | 30 | −2.9 | −2.9 | −16.7 | 16.7 | −5.7 | 2.8 | 0.04 | 0.1 | 0.4 | 0.6 | 0.6 | |
| 0.4 | 220 | 10 | 0.6~1.0 | −2.9 | −2.9 | −12.2 | 12.3 | −4.9 | N/A | N/A | N/A | N/A | N/A | N/A |
| 0.8 | 448 | 10 | −4.6 | −4.6 | −22.9 | 22.9 | −6.7 | N/A | N/A | N/A | N/A | N/A | N/A | |
| 0.8 | 346 | 30 | −2.9 | −2.9 | −16.7 | 16.7 | −5.7 | N/A | N/A | N/A | N/A | N/A | N/A | |
| Mach | Alt (kft) | Control Method | Dutch Roll Mode | Roll Time Const. (sec) | Spiral Root (sec−1) | Mismatch Cost | HQ Level | ||
|---|---|---|---|---|---|---|---|---|---|
| Freq. (rad/s) | Damping | ||||||||
| 0.4 | 10 | Gun Comp. | - | 3.91 | 0.65 | 0.42 | N/A | 4 | 1 |
| Additional Augmentation Control | 0.6 | 3.99 | 0.66 | 0.41 | N/A | 14 | 1 | ||
| 0.8 | 4.03 | 0.66 | 0.41 | N/A | 20 | 1 | |||
| 1.0 | 4.09 | 0.66 | 0.41 | N/A | 26 | 1 | |||
| 0.8 | Gun Comp. | - | 6.09 | 0.58 | 0.28 | N/A | 4 | 1 | |
| Additional Augmentation Control | 0.6 | 6.08 | 0.64 | 0.29 | N/A | 15 | 1 | ||
| 0.8 | 6.10 | 0.65 | 0.29 | N/A | 20 | 1 | |||
| 1.0 | 6.17 | 0.66 | 0.29 | N/A | 25 | 1 | |||
| 0.9 | 30 | Gun Comp. | - | 4.95 | 0.70 | 0.39 | N/A | 5 | 1 |
| Additional Augmentation Control | 0.6 | 5.00 | 0.70 | 0.41 | N/A | 18 | 1 | ||
| 0.8 | 5.04 | 0.70 | 0.42 | N/A | 23 | 1 | |||
| 1.0 | 5.09 | 0.70 | 0.42 | N/A | 30 | 1 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, C.-h.; Kim, C.; Kim, B.S. Flight Control Law for Stabilizing Transient Response of the Aircraft during Gun Firing. Aerospace 2023, 10, 365. https://doi.org/10.3390/aerospace10040365
Ji C-h, Kim C, Kim BS. Flight Control Law for Stabilizing Transient Response of the Aircraft during Gun Firing. Aerospace. 2023; 10(4):365. https://doi.org/10.3390/aerospace10040365
Chicago/Turabian StyleJi, Chang-ho, Chongsup Kim, and Byoung Soo Kim. 2023. "Flight Control Law for Stabilizing Transient Response of the Aircraft during Gun Firing" Aerospace 10, no. 4: 365. https://doi.org/10.3390/aerospace10040365
APA StyleJi, C.-h., Kim, C., & Kim, B. S. (2023). Flight Control Law for Stabilizing Transient Response of the Aircraft during Gun Firing. Aerospace, 10(4), 365. https://doi.org/10.3390/aerospace10040365






