Study on the Influence of Nozzle Ablation on the Performance of the Solid Rocket Motor
Abstract
1. Introduction
2. Calculation Model and Method
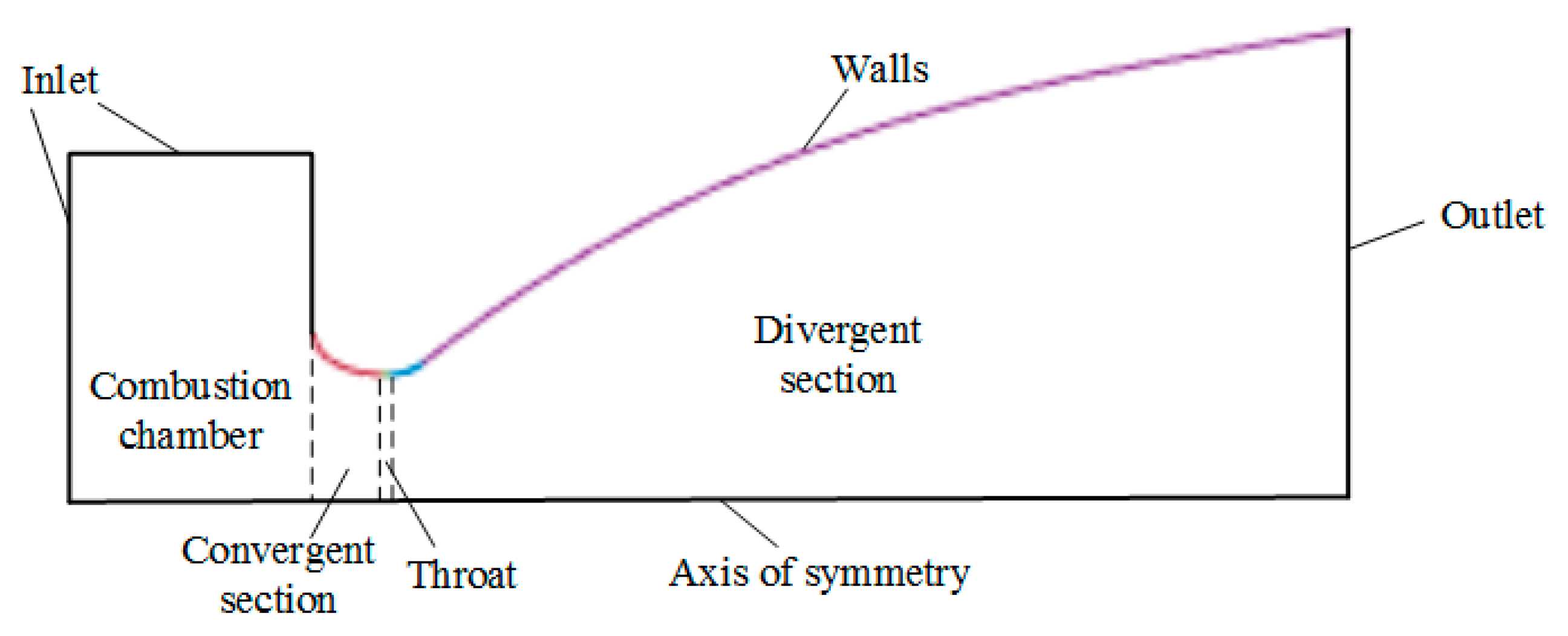
2.1. Physical Model
2.2. Governing Equation
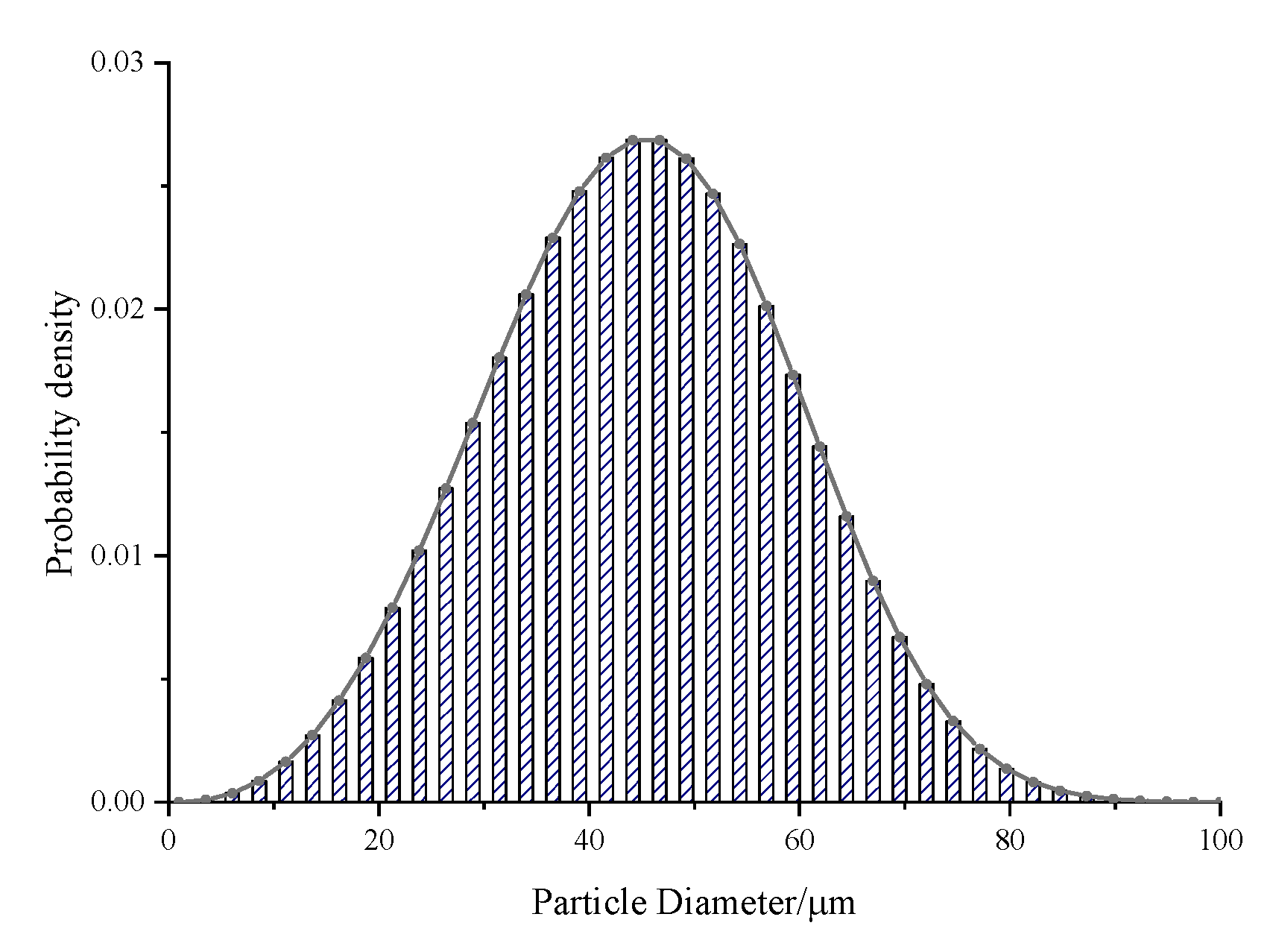
2.3. Computational Methods and Boundary Conditions
2.4. Model Verification
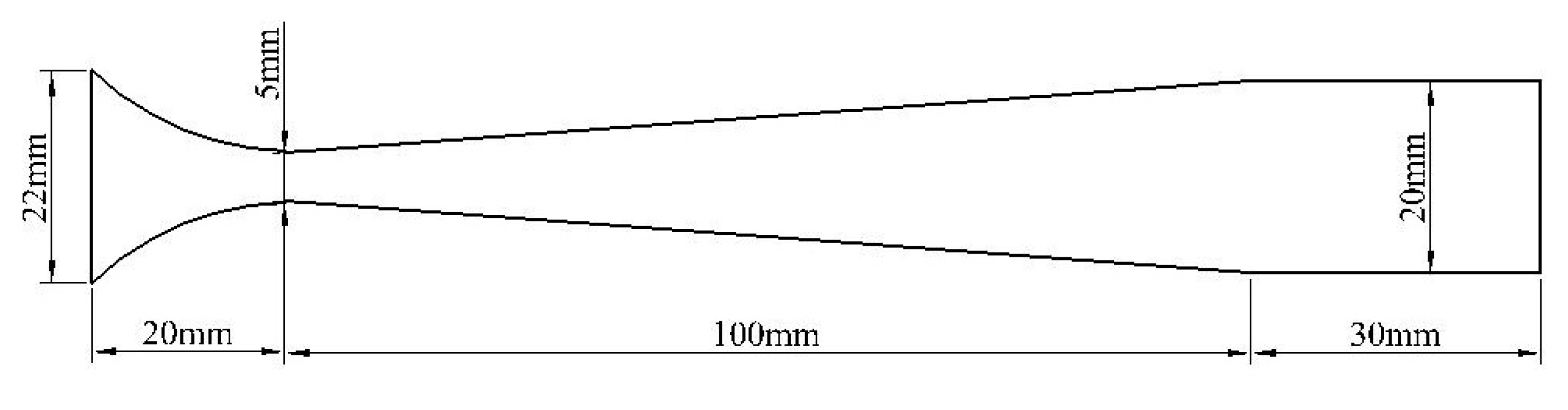
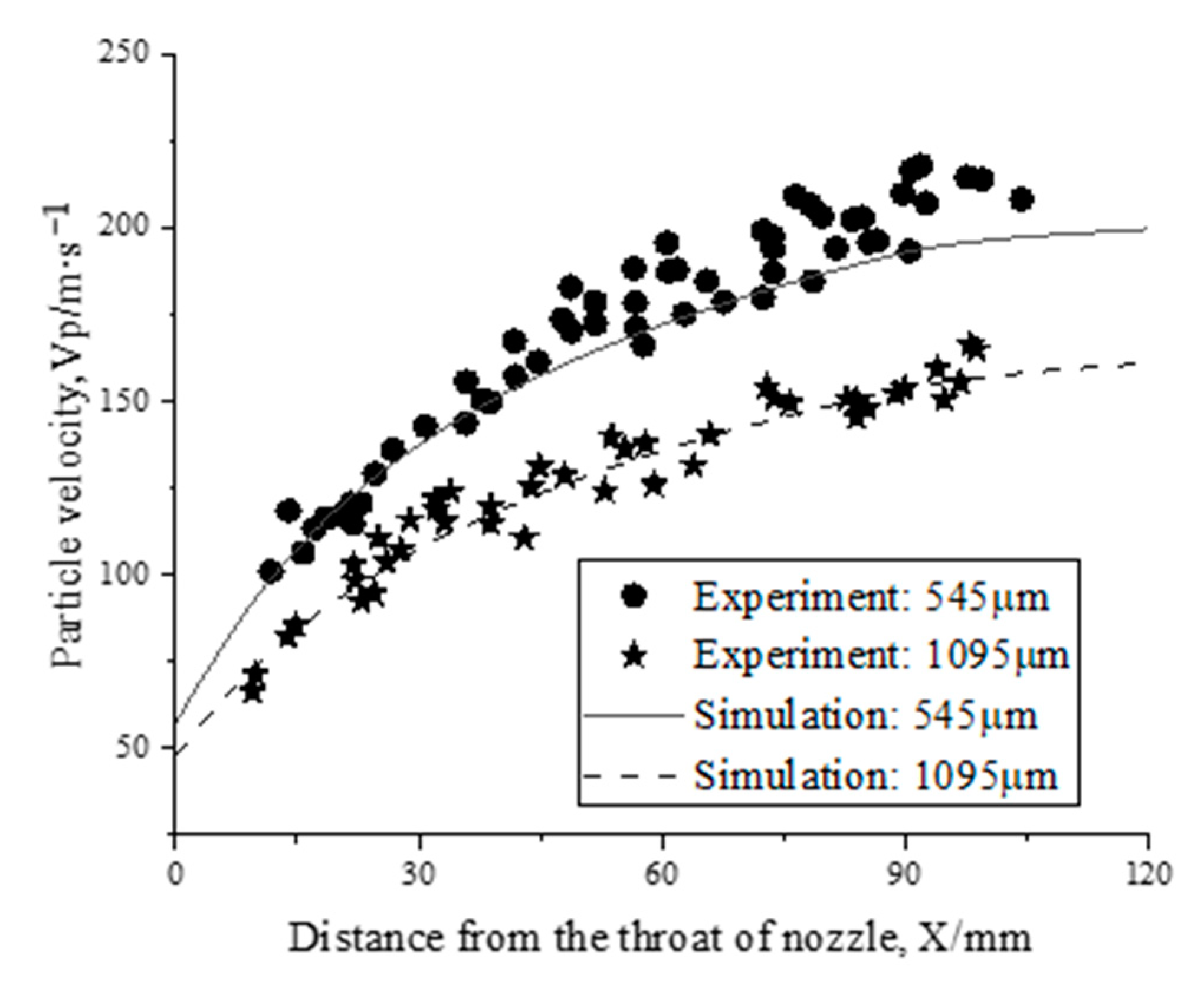
2.4.1. Verification of Two-Phase Flow Model
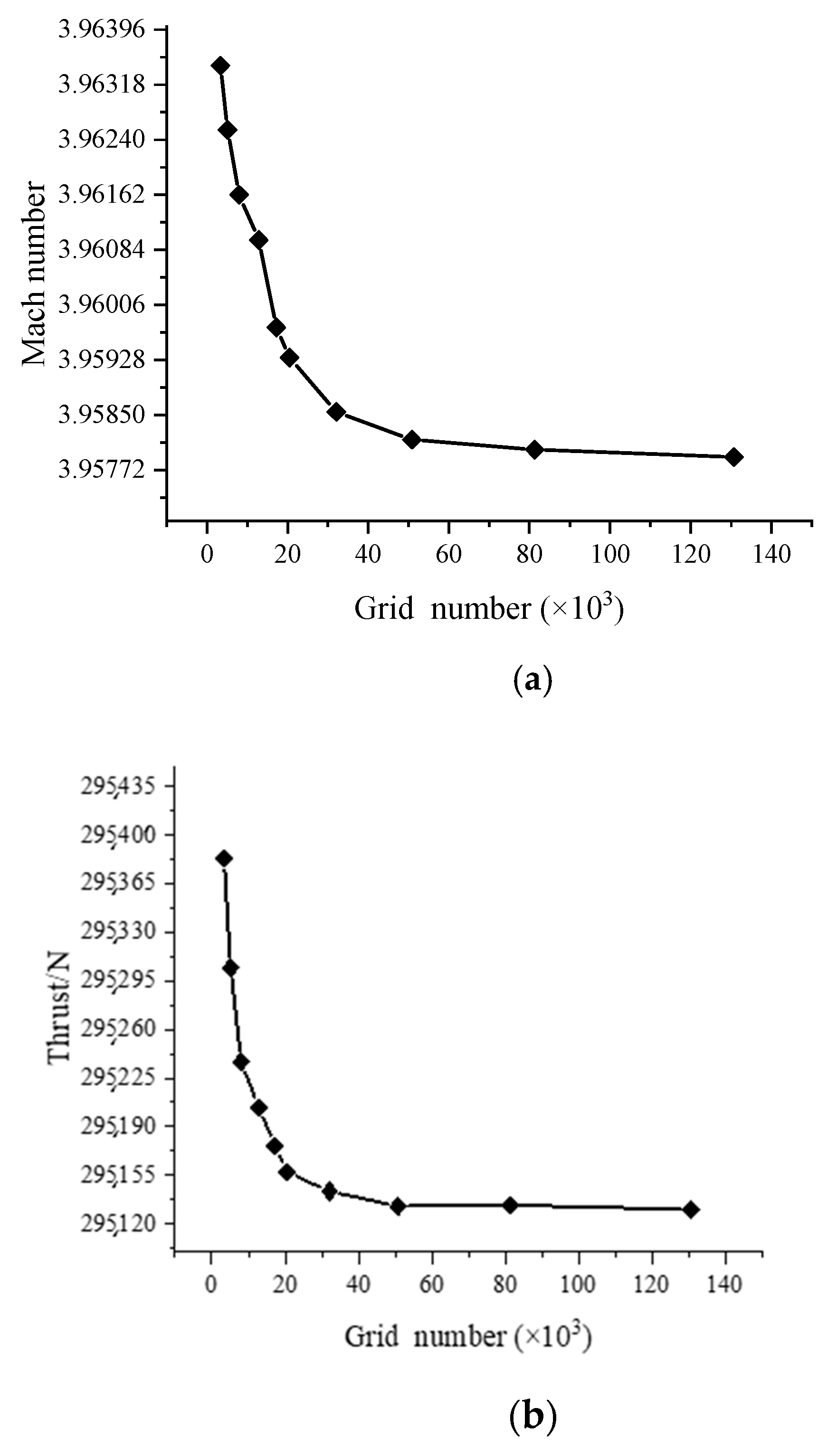
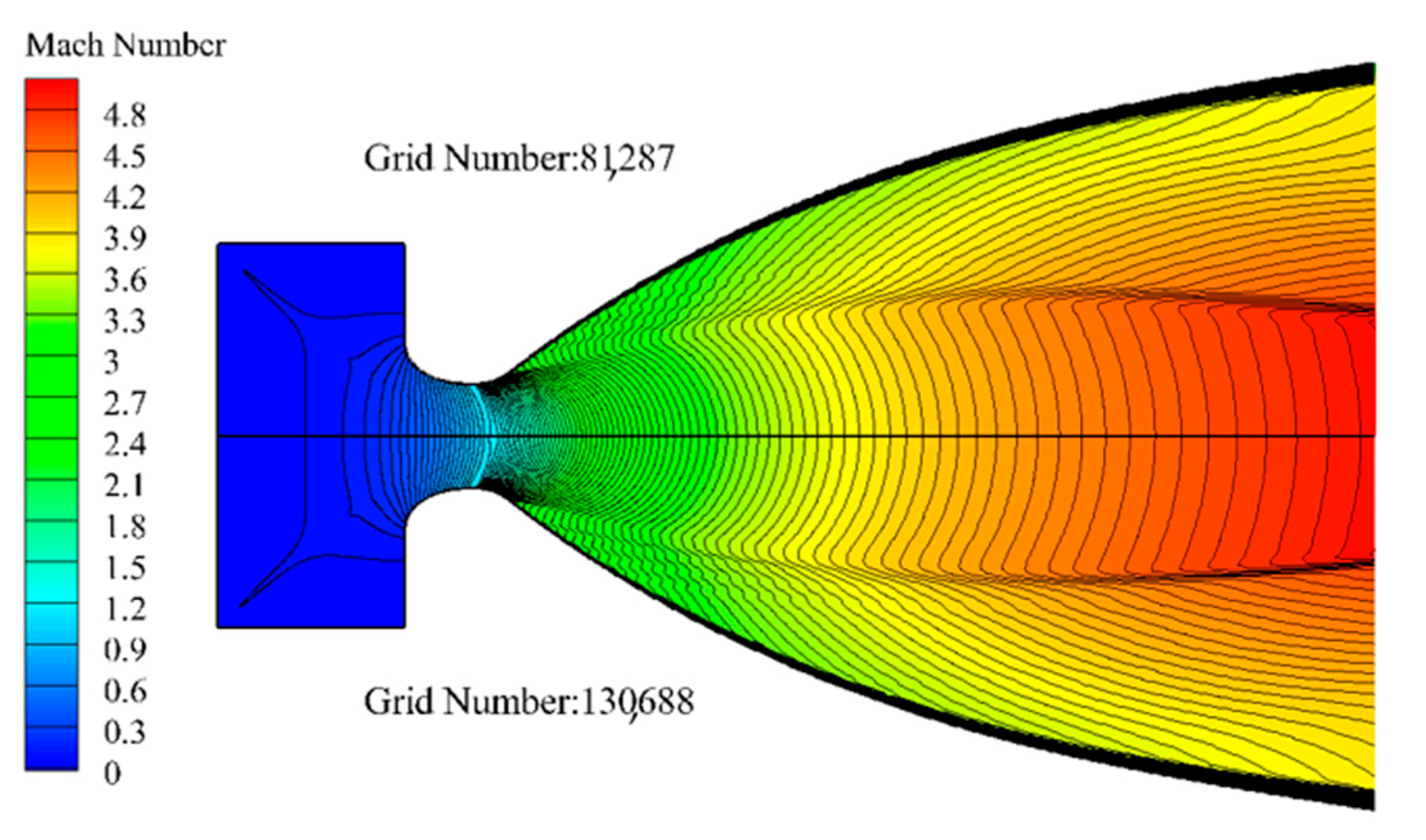
2.4.2. Verification of Grid Independence
3. Analysis of Calculation Results
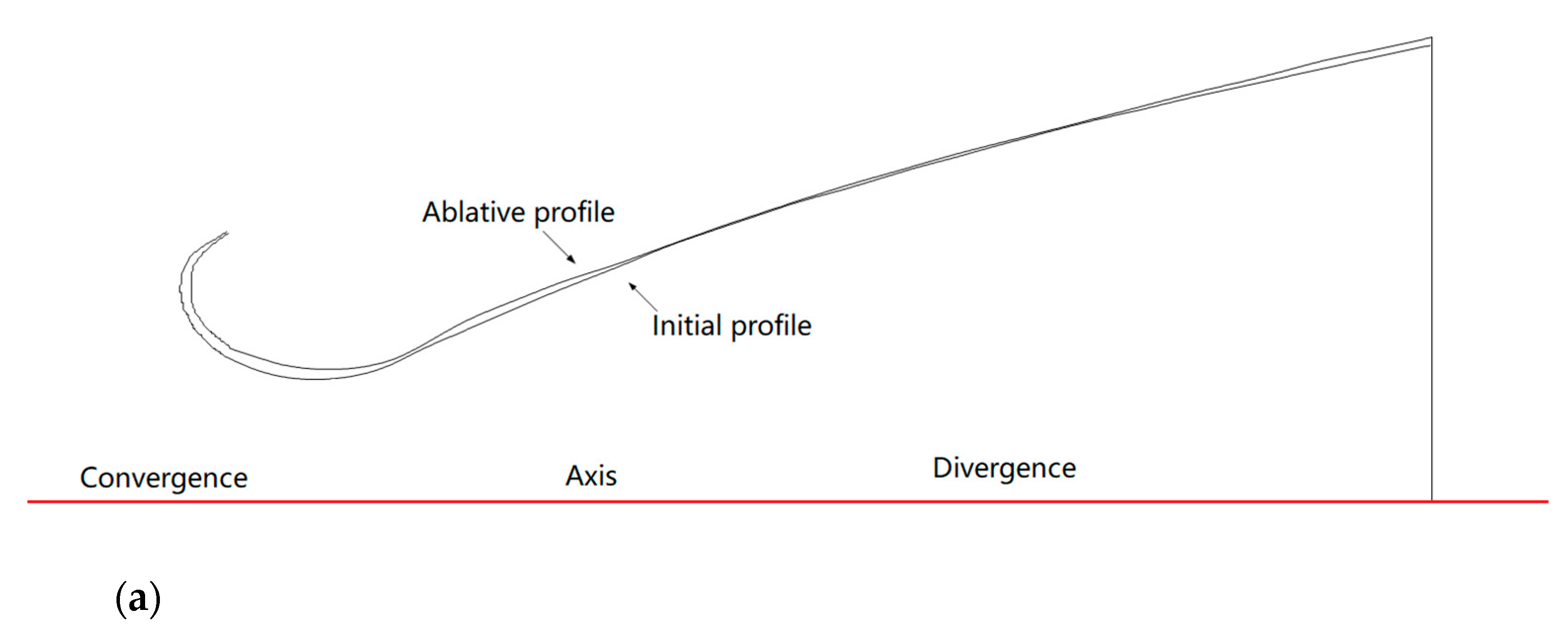
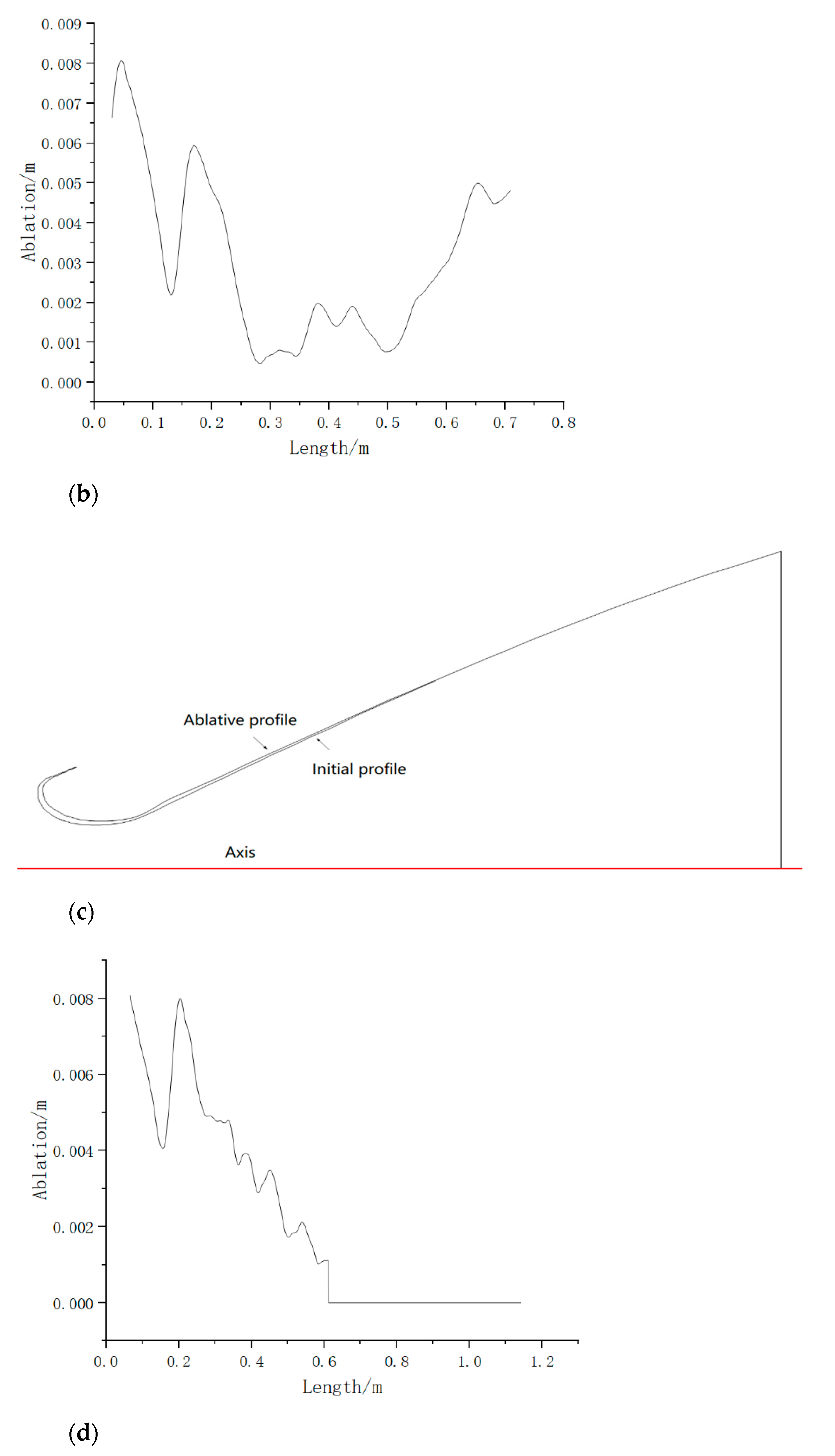
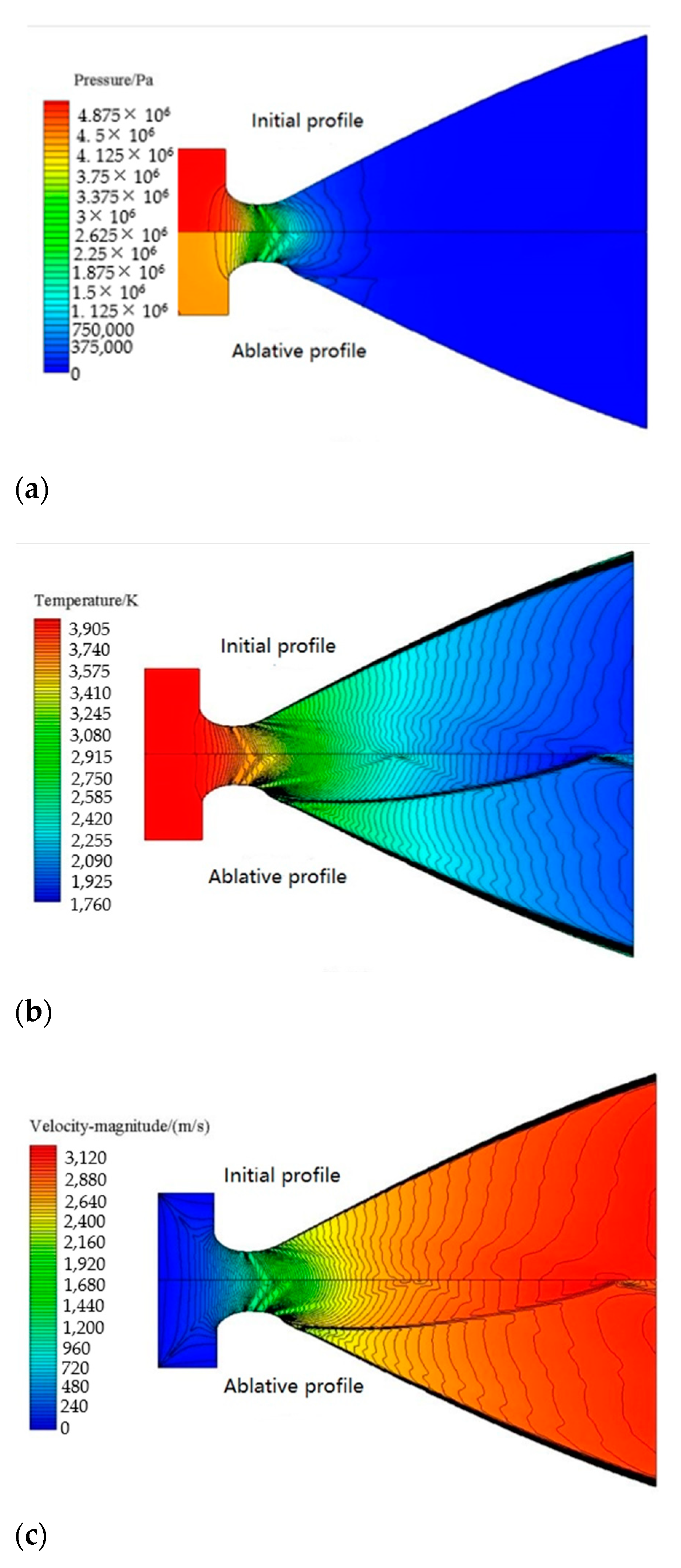
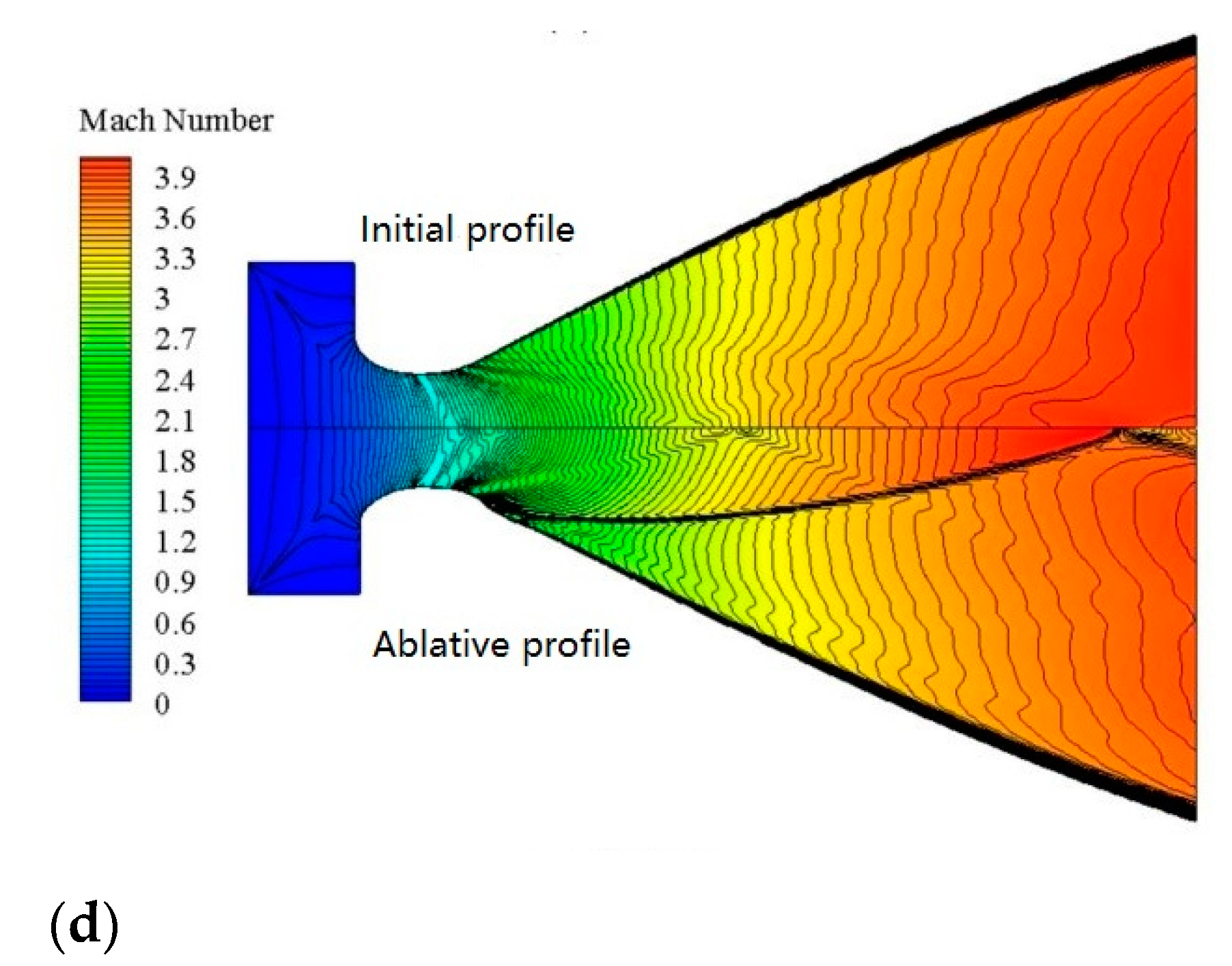
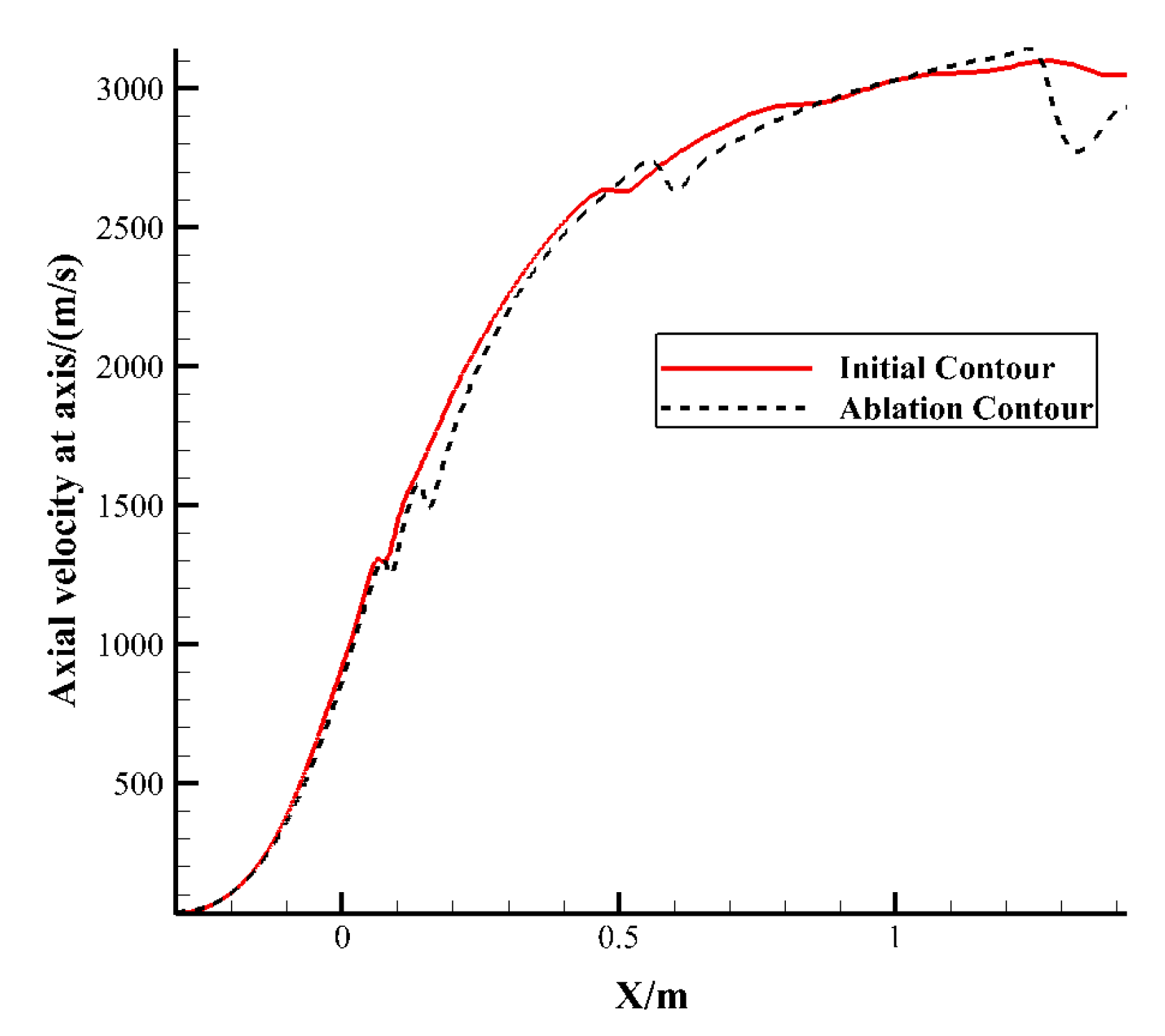
3.1. Analysis of Nozzle Ablation under Ground Condition
- (1)
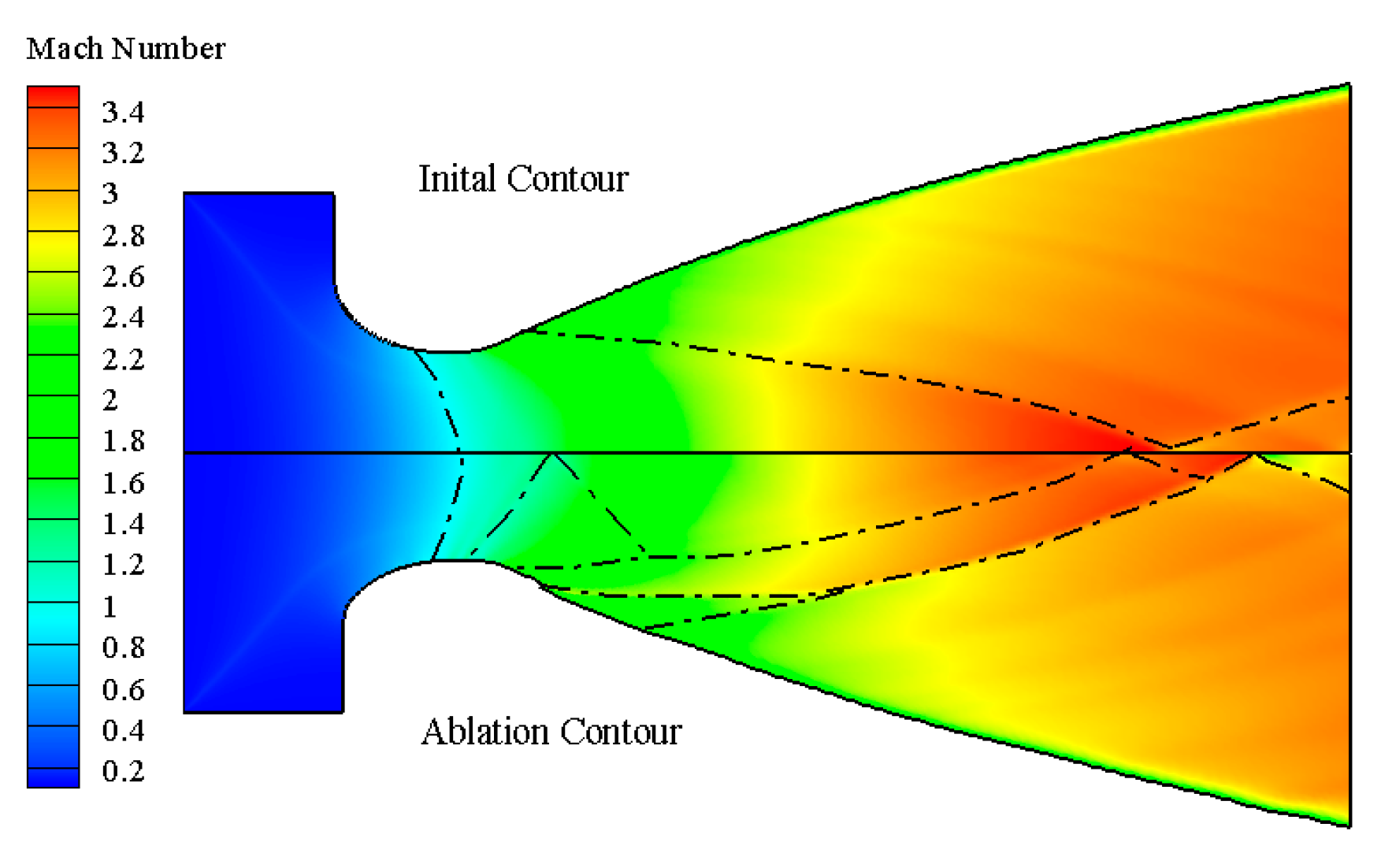
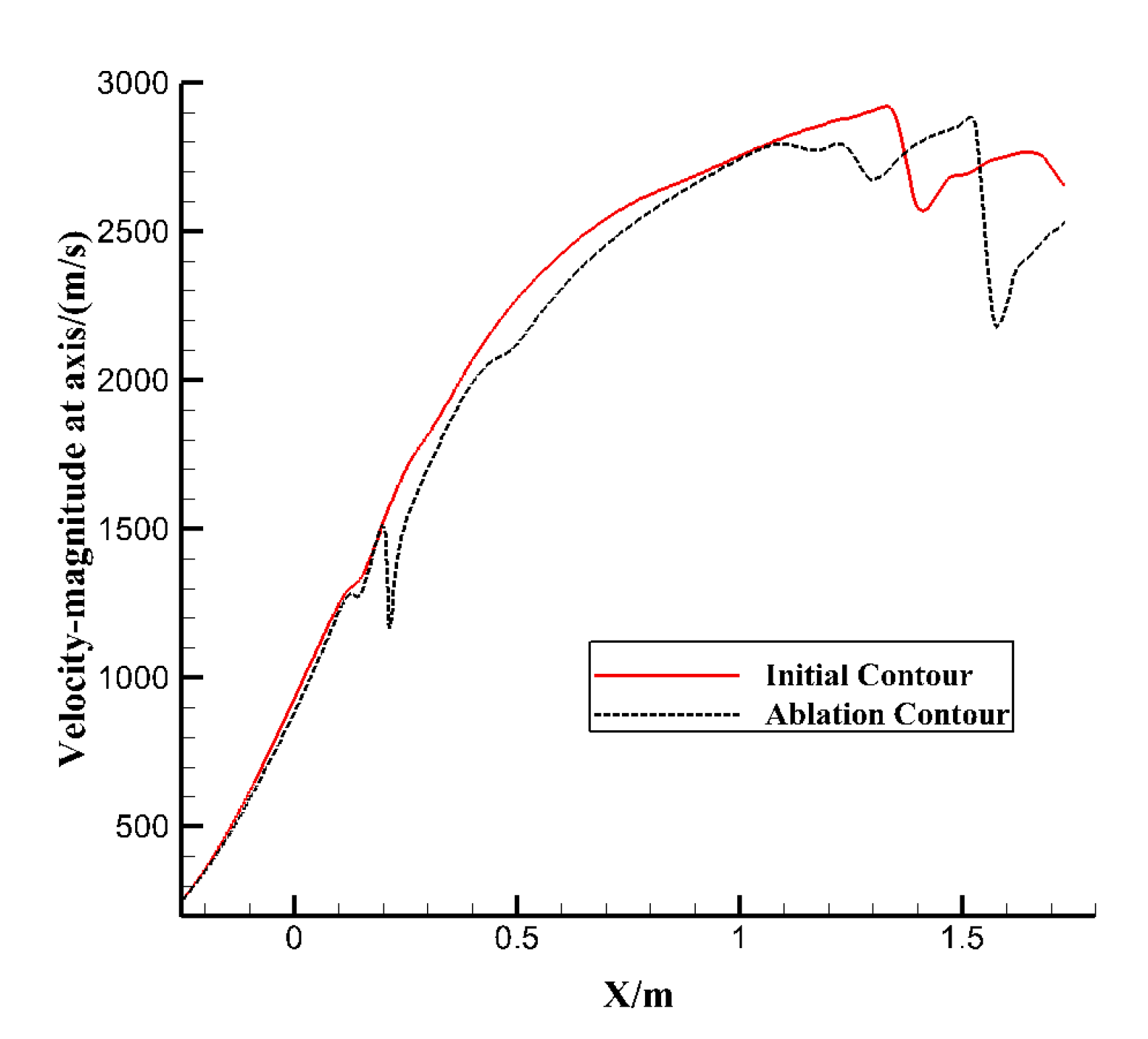
- Influence of profile ablation on velocity field
- (2)
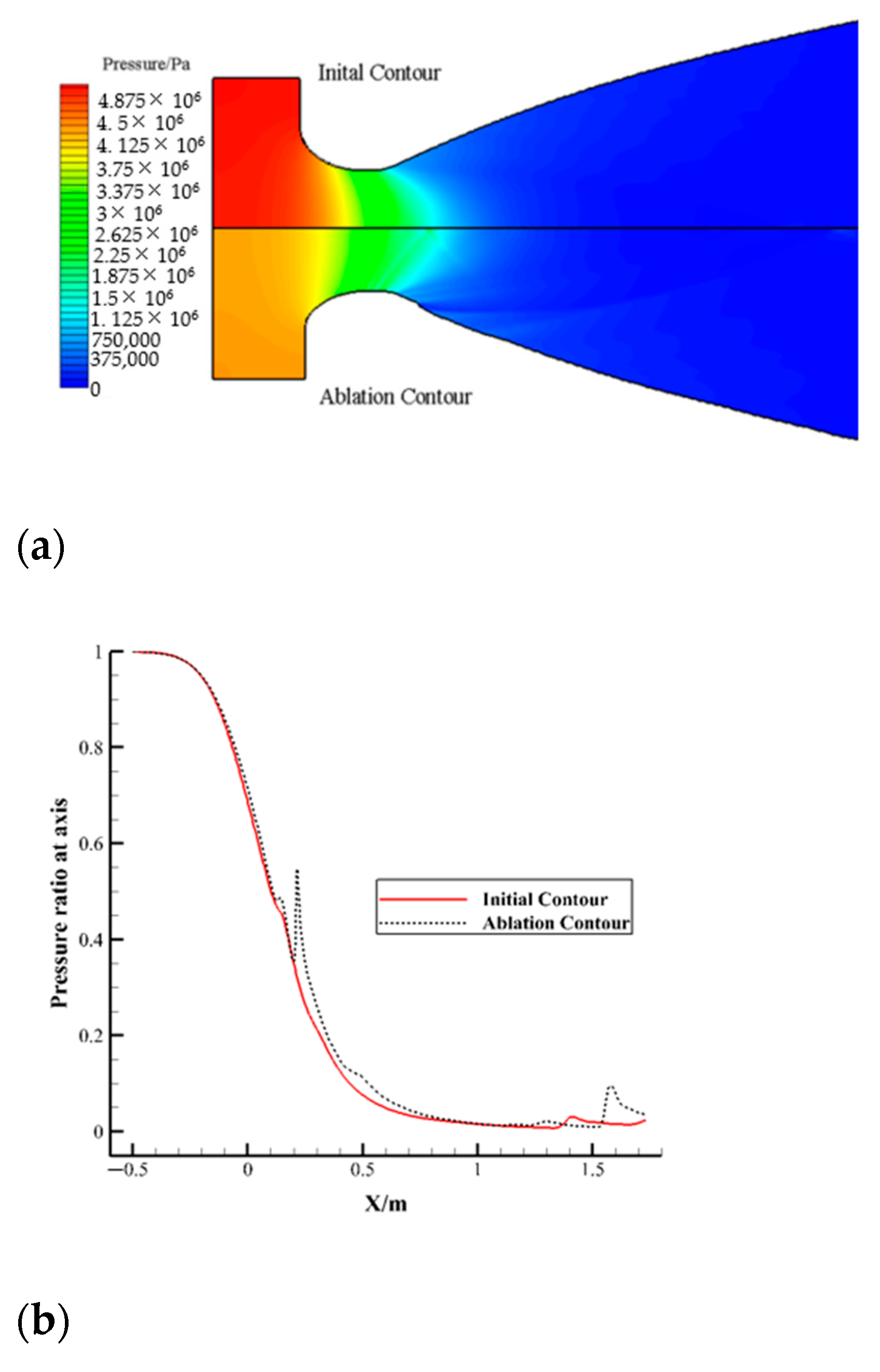
- Influence of profile ablation on the pressure field
- (3)
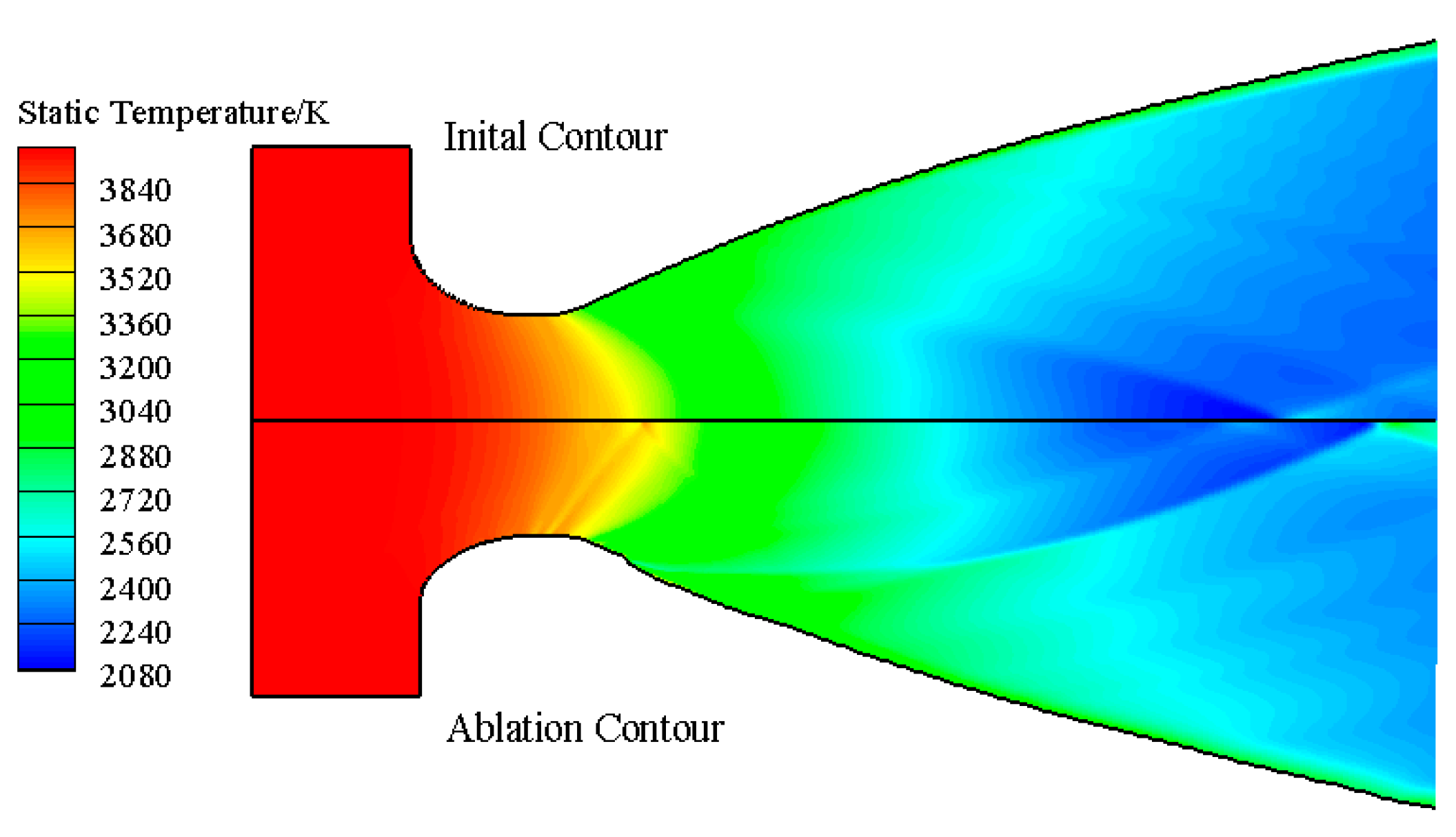
- Influence of profile ablation on the temperature field
- (4)
- Influence of profile ablation on nozzle performance
3.2. Analysis on the Influence of Nozzle Ablation at Vacuum State
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Helong, J.; Ruyi, T.; Hao, W. Research on flow field characteristics of nozzle plume for a solid rocket motor. J. Phys. Conf. Ser. 2022, 2336, 012013. [Google Scholar]
- Yang, L.; Hai-feng, W.; Dong, M.; Yong-gang, G.; Wei, Z. Numerical investigation of surface roughness effects on non-equilibrium flow in expansion section of rocket nozzle. Aerosp. Sci. Technol. 2022, 124, 107523. [Google Scholar]
- Zhao, S.; Tian, H.; Wang, P.; Yu, N.; Cai, G. Steady-state coupled analysis of flowfields and thermochemical erosion of C/C nozzles in hybrid rocket motors. Sci. Chin3.3Technol. Sci. 2015, 58, 574–586. [Google Scholar] [CrossRef]
- Wu, X.; Chen, J.; Wang, D. Numerical Simulation of Working Process of Solid Rocket Motor; Higher Education Press: Beijing, China, 2006. [Google Scholar]
- Rao, G.V.R. Exhaust Nozzle Contour for Optimum Thrust. J. Jet Propuls. 1958, 28, 377–382. [Google Scholar] [CrossRef]
- Lorenc, S.A.; Hoffman, J.D. Correlation of performance of conical and contoured nozzles for gas-particle flow. AIAA J. 1966, 4, 169–171. [Google Scholar] [CrossRef]
- Hoffman, J.D.; Thompson, H.D. Optimum thrust-nozzle contours for gas-particle flows. AIAA J. 1967, 5, 1886–1887. [Google Scholar] [CrossRef]
- Allman, J.G.; Hoffman, J.D. Design of Maximum Thrust Nozzle Contours by Direct Optimization Methods. AIAA J. 2012, 19, 750–751. [Google Scholar] [CrossRef]
- Liu, B.; Fang, D.; Xia, Z. Optimal design of rocket nozzle parameters with consideration of gas-particle two-phase flow effect. J. Propuls. Technol. 2013, 34, 8–14. [Google Scholar]
- Yu, K.; Yang, X.; Mo, Z. Profile Design and Multifidelity Optimization of Solid Rocket Motor Nozzle. J. Fluids Mot. 2014, 136, 031104. [Google Scholar] [CrossRef]
- Wang, C. Optimal design of internal contour of solid motor nozzle. J. Astronaut. 1996, 17, 64–67. [Google Scholar]
- Chen, L.; Li, Y.; Hou, X. Effects of nozzle contour in convergent section and throat on mass flow rate. J. Solid Rocket. Technol. 2002, 25, 10–11. [Google Scholar]
- Chen, L.; Li, Y.; Wang, J.; Hou, X. Effects of the nozzle divergent cone contour on solid rocket motor performance. J. Solid 2004, 27, 9–11. [Google Scholar]
- Wang, Y.; Liang, Y.; Zhao, Y. Numerical simulation of effect of parabolic nozzle contour parameters on flow separation. J. Aerosp. Power 2017, 32, 196–201. [Google Scholar]
- Mu, X.; Tian, W.; Dong, X. Study on the effect of divergence contour parameters on performance of solid rocke motor nozzle. J. Solid Rocket. 2021, 44, 254–263. [Google Scholar]
- Liu, R.; Chen, X.; Zhou, C.; Li, Y. Devolopment of interpolation Method for conjugate heat transfer in non-orthogonal interface. J. Propuls. Technol. 2016, 37, 90–97. [Google Scholar]
- Okuda, S.; Choi, W.S. Gas-particle mixture flow in various types of convergent-divergent nozzle. J. Chem. Eng. Jpn. 1978, 11, 432–438. [Google Scholar] [CrossRef]
| Parameters | Vacuum State Nozzle | Ground State Nozzle |
|---|---|---|
| Convergence ratio | 3 | 3 |
| Expansion ratio | 48 | 13.4 |
| Diameter of throat, m | 0.159 | 0.159 |
| Diameter of exit, m | 1.102 | 0.582 |
| Length of convergence, m | 0.095 | 0.095 |
| Length of throat, m | 0.01 | 0.01 |
| Length of divergence, m | 1.036 | 0.604 |
| Curvature radius of arc, m | 0.080 | 0.080 |
| Initial expansion half-angle, ° | 29 | 27 |
| Expansion half-angle of exit, ° | 17 | 15 |
| Working times, s | 50 | 50 |
| Parameter | Initial Profile | Ablative Profile | Deviation |
|---|---|---|---|
| Mach number | 3.15783 | 3.05630 | 3.215% |
| Total thrust, KN | 238.866 | 235.448 | 1.431% |
| Specific impulse, m·s−1 | 2512.430 | 2476.471 | 1.431% |
| Momentum thrust, KN | 196.750 | 192.706 | 2.055% |
| Static thrust, KN | 17.049 | 18.004 | −5.602% |
| Particle force, KN | 25.068 | 24.738 | 1.316% |
| Outlet static temperature, K | 2100.965 | 2143.818 | −2.040% |
| Parameter | Initial Profile | Ablative Profile | Deviation |
|---|---|---|---|
| Mach number | 4.024 | 3.897 | 3.156% |
| Total thrust, KN | 251.405 | 249.505 | 0.756% |
| Specific impulse, m·s−1 | 2644.288 | 2623.806 | 0.775% |
| Momentum thrust, KN | 212.566 | 210.107 | 1.157% |
| Static thrust, KN | 11.891 | 12.582 | −5.811% |
| Particle force, KN | 26.948 | 26.815 | 0.494% |
| Outlet static temperature, K | 1629.084 | 1673.599 | −2.733% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, W.; Wang, C.; Zhang, K.; Wang, Z.; Tian, W. Study on the Influence of Nozzle Ablation on the Performance of the Solid Rocket Motor. Aerospace 2023, 10, 156. https://doi.org/10.3390/aerospace10020156
Huang W, Wang C, Zhang K, Wang Z, Tian W. Study on the Influence of Nozzle Ablation on the Performance of the Solid Rocket Motor. Aerospace. 2023; 10(2):156. https://doi.org/10.3390/aerospace10020156
Chicago/Turabian StyleHuang, Weiqiang, Chunguang Wang, Kaining Zhang, Zhihong Wang, and Weiping Tian. 2023. "Study on the Influence of Nozzle Ablation on the Performance of the Solid Rocket Motor" Aerospace 10, no. 2: 156. https://doi.org/10.3390/aerospace10020156
APA StyleHuang, W., Wang, C., Zhang, K., Wang, Z., & Tian, W. (2023). Study on the Influence of Nozzle Ablation on the Performance of the Solid Rocket Motor. Aerospace, 10(2), 156. https://doi.org/10.3390/aerospace10020156






