1. Introduction
Gusts have very pronounced effects on aircraft flight. Gust load is often considered to be a serious load condition encountered during aircraft flight, and its influence must be taken into account in the aircraft structure design process. Additionally, gusts cause vibrations and attitude changes, reducing the ride and flight quality of the aircraft. As aircraft structures become more lightweight, the influence of gusts on aircraft becomes more severe. Gust load alleviation (GLA) technology can be employed to significantly reduce these negative influences.
With the development of aviation science and technology, many studies have been performed on GLA. Some of these studies focused on structure device design [
1,
2], and some used morphing wing technology to achieve GLA [
3,
4,
5]. Aside from these studies, gust alleviation through active control is a very important research branch. Depending on the type of the signal fed into the control system, the active control of the GLA can be divided into two categories: feedforward control, which uses the gust signal as the input, and feedback control, which uses the aircraft motion signal as the input. In the past few years, feedback control has been widely studied, including optimal control [
6], robust control [
7,
8], predictive control [
9], intelligent control [
10], etc. There are also many experimental studies [
11,
12,
13,
14]. At present, gust alleviation control system based on feedback control has been applied to operational aircraft [
15], such as the Airbus A380, Boeing B787, etc.
Although feedback control has been extensively studied and applied, it is a lag-compensated control method that only begins to work once the aircraft has been excited by the gust. Feedforward control system (FFCS) uses the gusts detected in advance as input signals and can drive control surface deflection before the gusts reach the aircraft’s main lifting surface, thus overcoming the above shortcoming. Another advantage of this control method is that it can maintain the dynamics and handling qualities of the aircraft unchanged and does not affect the stability of the existing flight control system.
To date, many feedforward controller design methods have been developed. As feedforward control is an open-loop control method, it is sensitive to changes in the flight condition and the aircraft’s variation. Therefore, some adaptive feedforward controller design methods [
16,
17] have been investigated. There are also other control methods that have been applied to feedforward gust alleviation, such as model predictive control (MPC) [
18] and robust control [
19]. In these studies, it is usually assumed that the gusts can be perfectly measured. There are also experimental studies on feedforward control, an adaptive feedforward controller flight test was conducted on an ATTAS aircraft [
20] using the signal from a flight log sensor as a reference for atmospheric turbulence. The results of this experiment showed that the feedforward controller reduced the power spectral density of the wing bending vibration acceleration by 20%. Some researchers [
21,
22] have used the response excited by aircraft control surface deflection to simulate the gust response and fed the excitation signal into FFCS to conduct gust alleviation flight tests. Similarly, several studies [
23,
24] used the command signal driving the gust generator as the input of FFCS for gust alleviation in wind tunnel tests. Obviously, feedforward gust alleviation wind tunnel test would be better conducted through direct gust measurement. The author’s team [
25] carried out a feedforward gust alleviation wind tunnel test on a single wing by using a five-hole probe to measure the gusts. The designed feedforward controller achieved a maximum alleviation rate of more than 80% for the wing root bending moment (WRBM). Zhao et al. [
26] carried out the wind tunnel test of feedforward control using a full aircraft model. However, these tests used a probe fixed inside the wind tunnel to measure the gusts and did not perform gust alleviation tests using the gust sensor mounted on the airframe. Thus, they missed the gust calculation step that considers aircraft motion, which is indispensable for actual feedforward gust alleviation.
It is very important to obtain accurate gust disturbance signals for feedforward control. There are two main types of gust signal acquisition equipment in the current research. The first is based on light detection and ranging (LIDAR) sensors which detect particles in the air to obtain wind field information. German researchers [
27,
28,
29] have carried out a lot of research on this kind of sensor. A newly developed direct detection Doppler wind LIDAR (DD-DWL) is capable of measuring wind speeds at close range with an accuracy of 0.5 m/s. The Japan Aerospace Exploration Agency also [
30,
31] carried out a similar project, called ”Safeavio”, which developed an airborne Doppler LIDAR capable of detecting turbulence at distances of 10–20 km to support pilots in bypassing turbulent areas. However, this detection distance is too large for gust alleviation control. This project will further reduce the LIDAR’ detection distance in subsequent research. Although LIDAR sensors can provide more accurate gust information, they are usually expensive, large, and heavy.
The second type of gust sensors are airflow sensors, such as multi-hole probe or flow vane. Multi-hole probe can measure the flow angle by sensing the pressure difference from different holes. Flow vane, also called pivoted vane, is a mass-balanced wind vane that automatically aligns itself with the direction of the incoming flow and then measures the angle between the flow vane and a reference line on the aircraft [
32]. At present, the technology of such sensors is relatively mature, and the equipment is inexpensive and small. There has been relatively little research on gust calculation method using LIDAR or airflow sensors. Nicolas from DLR [
33] studied the LIDAR-based wind field reconstruction algorithm, which can be realized through simple operation and therefore can obtain results with a low computational cost. Some gust calculation methods based on simplified flight dynamics [
34,
35] have also been studied. Through this kind of method, vertical gusts to trigger the FFCS are obtained. Through reviewing the existing research, it can be found that there are few in-depth studies on gust calculation. There is also a lack of experimental research combining gust calculation and feedforward gust alleviation. Such research is necessary to further promote the practical applications of feedforward gust alleviation.
In this paper, a gust calculation method based on the airflow sensor for the three-dimensional case is first described; On this basis, the gust calculation method in the longitudinal plane of aircraft motion, which is of major interest, is simplified. Based on the simplified method, a set of two degrees-of-freedom motion device was used to verify the gust calculation method in wind tunnel test. The effects of sensor time delays and measurement errors on the accuracy of gust calculation and the effect of gust alleviation are also investigated through numerical simulations. Finally, a gust alleviation wind tunnel test based on the gust calculation is carried out on a half-model aircraft.
2. Gust Calculation Method
The aerodynamic forces on an aircraft are mainly determined by the relative motion between the aircraft and the atmosphere. As shown in
Figure 1, consider an aircraft flying in the air with an arbitrary attitude, the airspeed
is at a certain angle with respect to the aircraft body. The body frame is
, the ground frame is
, and the attitude angles between the body frame and the ground frame are
,
, and
.
For a changing wind field, the change in wind speed can be regarded as the superposition of the average wind and the fluctuating wind. The average wind changes relatively slowly, and its direction and wind speed can be considered as basically unchanged within a certain period of time [
36]. For the problem of gust alleviation, the main study is the influence of the fluctuating wind, i.e., gusts, on the aircraft. The wind speed of the changing wind field is expressed as
, which can be written as
Each component of the above equation is expressed in the ground frame, where is the average wind speed, and is the gust velocity.
During the flight, the following relationship exists between the airspeed of the aircraft
, the ground speed
and the wind speed of the atmosphere
:
where all variables are expressed in the ground frame. The sum of the ground speed and the average wind speed is called the steady-state airspeed
:
To study the aerodynamic force of the aircraft, it is necessary to represent the airspeed under the body frame. The coordinate transformation matrix from the ground frame to the body frame, denoted as
, is expressed as follows:
The components of steady-state airspeed in the body frame can be obtained by
To simplify the problem, the effect of atmospheric disturbances on the aircraft can be decomposed into the longitudinal symmetry plane and the lateral plane of the aircraft. First, in the longitudinal plane, the aircraft’s angle of attack (AOA) sensor is usually mounted on the nose, and the measured aerodynamic AOA
incorporates the effects of the steady-state airspeed, gust disturbances and aircraft pitching motion. The influence of the steady-state airspeed is expressed as
and the components of the steady-state airspeed in the longitudinal plane are shown in
Figure 2. The influence of gusts on the aircraft is represented by the gust AOA
. Due to the rotational motion of the aircraft, the AOA measured by the sensor is
. Note that the influence of translation motion has been included in
. The AOA due to gusts in the longitudinal plane can then be obtained from the following relationship:
where
is the local AOA measured directly by the AOA sensor mounted on symmetrical plane of the aircraft nose, and
where
and
are the components of
along the
x-axis and
z-axis of the body frame, respectively. If the additional AOA caused by the pitch rate is small, there is
where
is the pitch rate and
is the distance from the AOA sensor to the aircraft center of gravity.
Similar to the derivation process for the longitudinal plane, the angle of sideslip (AOS) due to gusts in the lateral plane of the aircraft is:
where
is the component of
along the
y-axis of the body frame;
is the yaw rate, and
is the distance from the AOS sensor to the aircraft center of gravity.
When the aircraft is in flight, if the mean speed of the wind field is small compared to the speed of flight, then the effect of the mean wind can be ignored. Equation (3) can thus be written as
Assuming that the pitch angle of the aircraft is small and there is no roll motion (in fact, this is a very typical flight condition), then there is:
where
V is the projection of ground speed in the horizontal plane. Furthermore,
because the pitch angle is small and
is usually much smaller than
V. Thus, Equation (7) can be rewritten as
This equation implies that the steady-state AOA of the aircraft is composed of the pitch attitude of the aircraft and the AOA induced by the vertical motion. If the height of the aircraft is represented by
, then there is
. Thus, the final simplified method of gust calculation in the longitudinal plane of the aircraft can be expressed as
In this paper, the gust calculation method determined by this equation will be used as the basis for the research.
3. Wind Tunnel Test of Gust Calculation
As shown in
Figure 3, a wind tunnel test was designed to demonstrate the gust calculation method described in Equation (16). The main equipment includes a gust generator; a five-hole probe, which is used to measure flow field parameters such as AOA and wind speed, and a plunging–pitching motion device, which is used to provide rigid body motion to simulate the plunging and pitching motion of the aircraft. The motion speed could be freely controlled by a computer.
3.1. Gust Generator Design
In the wind tunnel testing, a gust generator was designed to disturb the flow field. The main components of the gust generator (
Figure 4) disturbing the flow field are four blades, which are divided into upper and lower groups. The blade profile is made of honeycomb composite material, and the blade shaft is a carbon-fiber cylinder. The application of composite material results in a lighter mass and a lower rotational inertia of the blades. Each blade has a span of 1.40 m, the airfoil follows the NACA0015 profile, the chord length is 0.30 m, and the total width of the gust generator is 2.80 m. A DC motor drives the blade deflection to disturb the flow field, and a tachometer to measure the rotational speed is installed near the motor. The designed gust generator was positioned 5.3 m ahead of the five-hole probe in the wind tunnel test. It can generate harmonic gusts with a frequency range of 1–6 Hz. The mathematical expression of such gusts is
where
is the gust amplitude and
is the gust frequency.
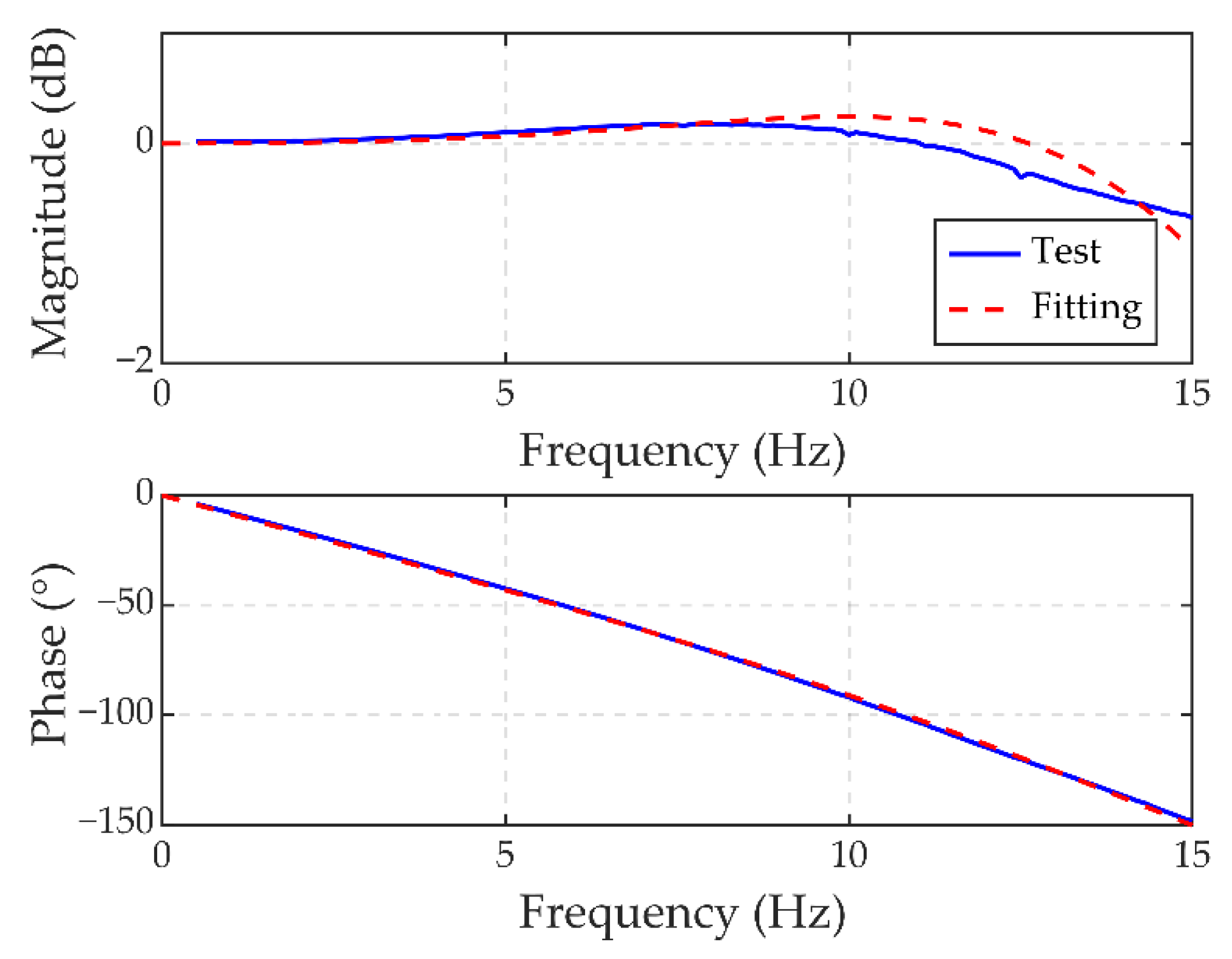
Figure 5 shows the ideal sine gust profile and measured gust profile with a frequency of 2.0 Hz. The actual gust variation trend is very close to a sine curve of the same frequency. The gust amplitude fluctuates, mainly because the incoming flow velocity of the wind tunnel is not strictly constant.
3.2. Gust Sensor and Plunging–Pitching Motion Device
During the test, a five-hole probe (
Figure 6) was used to measure the gusts in the wind tunnel flow field. The measured gusts were processed and used as the input signal for the FFCS. The five-hole probe is capable of measuring various aerodynamic parameters, such as the AOA, AOS, and wind speed. When compared with flow vane sensors, this type of device has no mechanical friction and rotation overshoot, so it has higher measurement accuracy and faster dynamic characteristics, making it more suitable for measuring rapidly changing gusts.
The longitudinal motion of the aircraft can be divided into plunging motion and pitching motion. The main objective of the gust calculation is to remove the AOA caused by these two types of motions from the measured AOA, thereby obtaining a pure gust AOA. To simulate both types of motion, a device with rotational and translational degrees-of-freedom was designed. To minimize the interference of the motion device on the flow field at the location of the five-hole probe, and to simulate the probe being installed on the nose of aircraft, the five-hole probe was connected to the two-degrees-of-freedom motion device using a bracket. The plunging–pitching motion device is shown in
Figure 7.
The plunging–pitching motion device is composed of a sliding platform and a rotating disc. A DC servo motor drives the sliding platform up and down through a screw, and the motion displacement is measured by a pull-wire displacement gauge. A disc is installed on the sliding platform and is driven by another motor. The angle of rotation is measured by a built-in angular displacement sensor. The device has a maximum travel of 450 mm in the plunging direction and a maximum speed of 60 mm/s; the pitch angle could be set arbitrarily as desired, with a maximum pitch rate of 30. The plunging motion and pitching motion could be controlled independently to enable different combinations of plunging movements and pitching movements.
At the same time, to calibrate the measurement accuracy of the five-hole probe and verify the gust calculation accuracy, a hot-wire anemometer was used. A double-wire X-type probe was installed on the motion device. It is capable of measuring the wind speed in two directions simultaneously: the horizontal incoming wind speed and the vertical wind speed. The hot-wire probe is at the same height as the head of the probe and at the same distance from the gust generator.
3.3. Sensors Signals Fusion Method
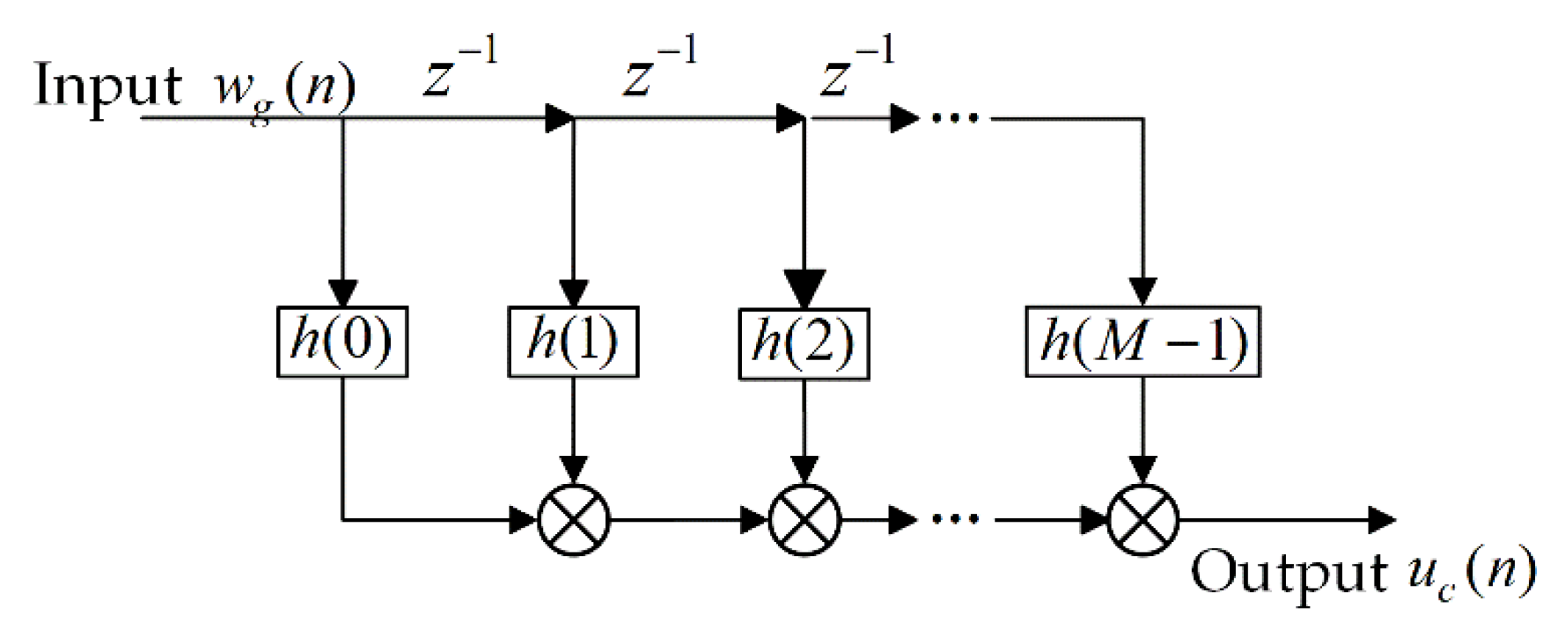
For the actual implementation of the gust calculation, the calculation method based on Equation (16) requires further processing.
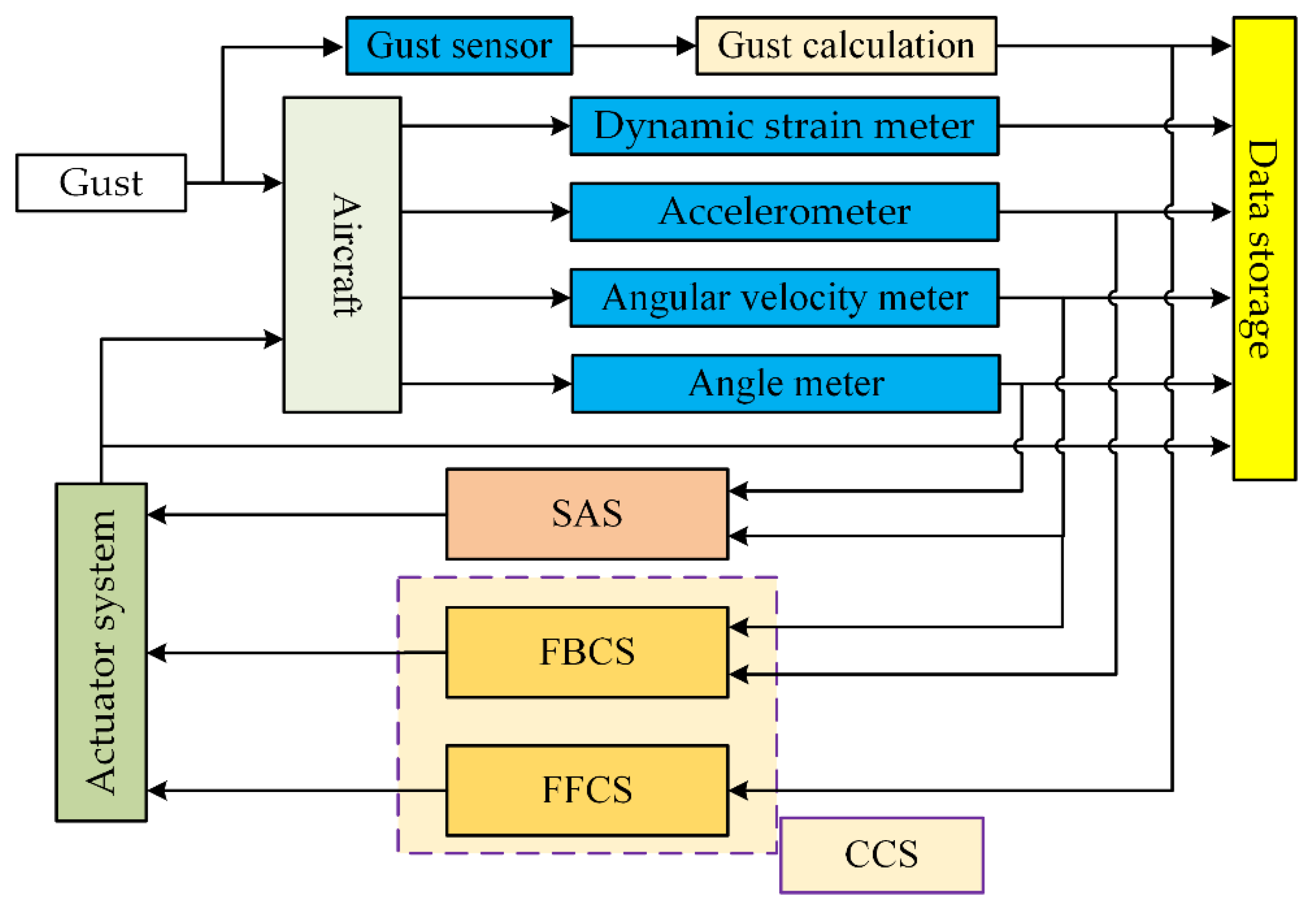
Figure 8 provides a detailed block diagram of the gust calculation, showing the physical quantities involved in the calculation and the corresponding measurement sensors. After obtaining data from the sensors, it is necessary to filter the signals and synchronize them in the temporal dimension.
represents the low-pass filter used to filter out unexpected high-frequency components, such as noise. The sensor used to detect the gusts is usually installed at the nose of the aircraft, so the measured signal may be affected by the bending mode of the fuselage. However, the frequency of the bending mode of the fuselage is generally higher than the gust frequency of concern. The influence of the bending mode of the fuselage can be eliminated by setting the appropriate parameters of the filter
.
Due to the independent acquisition of each channel of the measurement and control system, especially the different characteristics of various sensors, the collected signals will not be fully synchronized. For gust calculation, it is essential to synchronize the measured signals before fusing them for calculation. represents the delay module that coordinates the time difference between the signals collected by the individual sensors, by which the multiple signals measured remain synchronized in the computer system, thus ensuring the accuracy of the calculated gust signal.
In this study, the cross-correlation method is used to determine the delay between the various signals, and thus to synchronize the signals. For two related signals,
and
, where
is an integer, their cross-correlation function
[
37] is
where
The cross-correlation function reaches its maximum when
corresponds to the time-delay
between two signals. That is, the time delay can be estimated as
The closer the value of the cross-correlation coefficient is to 1, the more correlated the two signals are and the closer the signals are to being in phase.
3.4. Analysis of Gust Calculation Test Results
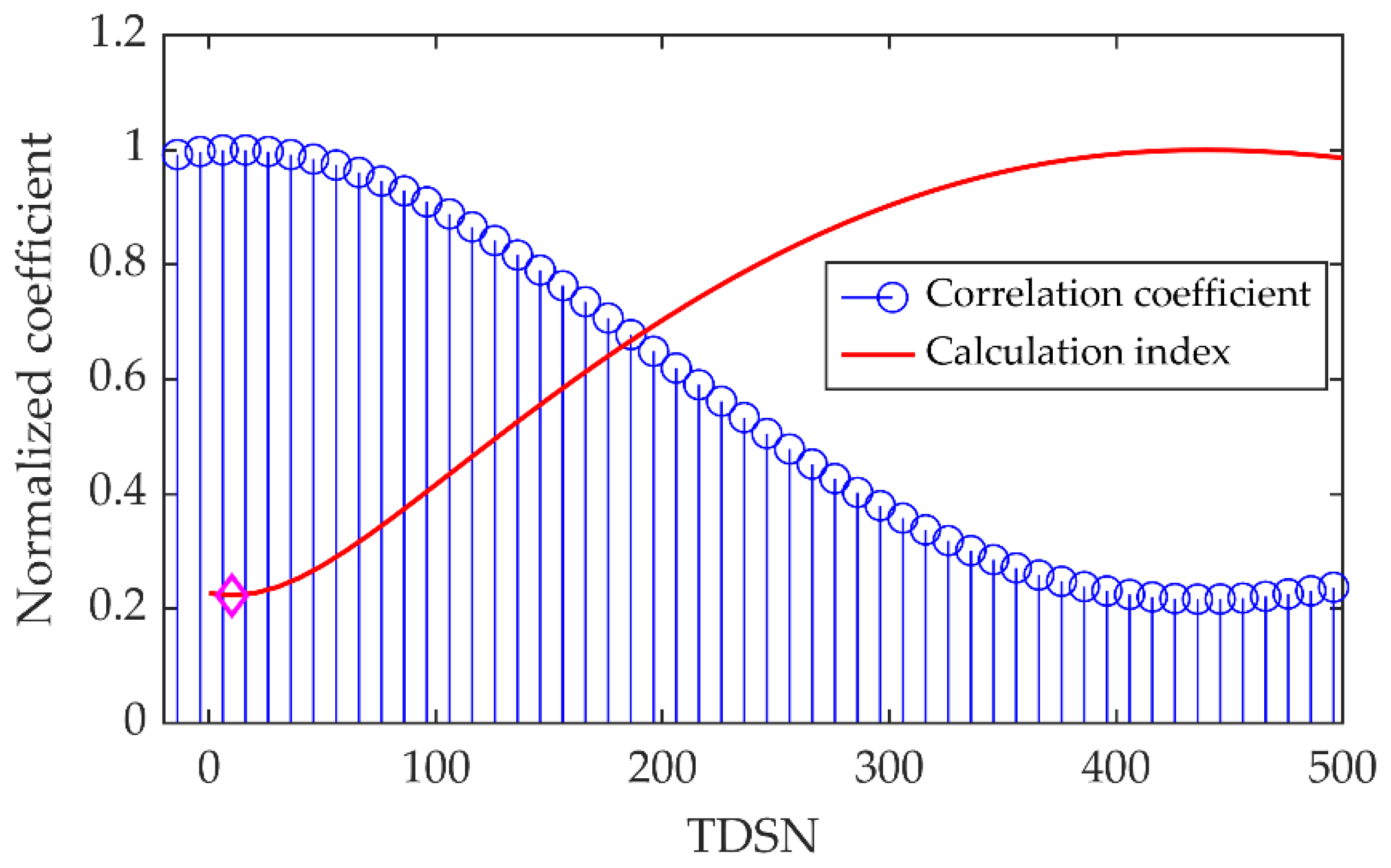
In the gust calculation testing, the five-hole probe transmits the pressure difference between the holes at the head of the probe into the internal pipe, and then converts the pressure signal to an electrical signal. This process leads to a relatively large delay. The delays of other sensors are generally small (of the order of microseconds), and the signals measured among these sensors can be considered synchronized. Due to pitch, the AOA in the test is the largest proportion of the total AOA measured by the probe, and the general trend of the change of the AOA measured by the probe is basically consistent with the pitch angle. From the perspective of phase, the AOA output by the probe lags behind the pitch angle output by the angular sensor. To determine this time difference exactly, it is necessary to calculate the correlation coefficients, between the pitch angle and the AOA, corresponding to different time delay step numbers (TDSN), as shown in
Figure 9.
Different TDSNs are assigned to the
in
Figure 8 to calculate the gust AOA, and the root mean square
of the calculated gust AOA in a certain time range is used as an index for measuring the quality of the calculation. Smaller value of the index indicates that the calculated gust AOA is closer to the true gust AOA. The index is obtained by:
where
is the calculated gust AOA at the
-th time step.
Figure 9 also shows the gust calculation index values corresponding to different TDSNs. Note that both the correlation coefficient and gust calculation index are normalized based on their respective maximum values. It can be seen that when the correlation coefficient is at its maximum, the corresponding TDSN is 10 (indicated by a magenta diamond marker); at this point, the gust calculation index is at its minimum, and the gust calculation quality is the best. At other time delays, as the correlation coefficient decreases, the calculation index becomes larger, and the gust calculation quality becomes worse.
When the undisturbed flow speed is 20 m/s and the gust frequency is 2.0 Hz, the pitch angle, the plunging displacement of the plunging–pitching motion device, the AOA measured by the probe, the calculated gust AOA, and the actual gust AOA measured by the hot-wire anemometer are shown in
Figure 10. From 16 to 72 s, the motion device only has pitching motion, and the five-hole probe is in different pitch conditions; From 78 to 108 s, the motion device is at different vertical positions; From 110 to 170 s, the device has both plunging motion and pitching motion. Since the hot-wire anemometer was fixed in the wind tunnel flow field, it directly measures the actual gust AOA, which changing trend is very close to a sinusoidal pattern with zero-mean value. The AOA measured by the probe varies with different motion states, while the calculated gust AOA remains almost unchanged at all times and is very close to the measured actual gust AOA. This demonstrates the accuracy of the gust calculation method.
Figure 11 presents a spectrum analysis of the measured AOA and the calculated gust AOA. It can be seen that the measured AOA has both the low-frequency components caused by the motion and the high-frequency components caused by the gust. The calculated gust AOA has no low-frequency component because the AOA caused by motion is eliminated by the gust calculation process, and only the gust component in the flow field is retained.
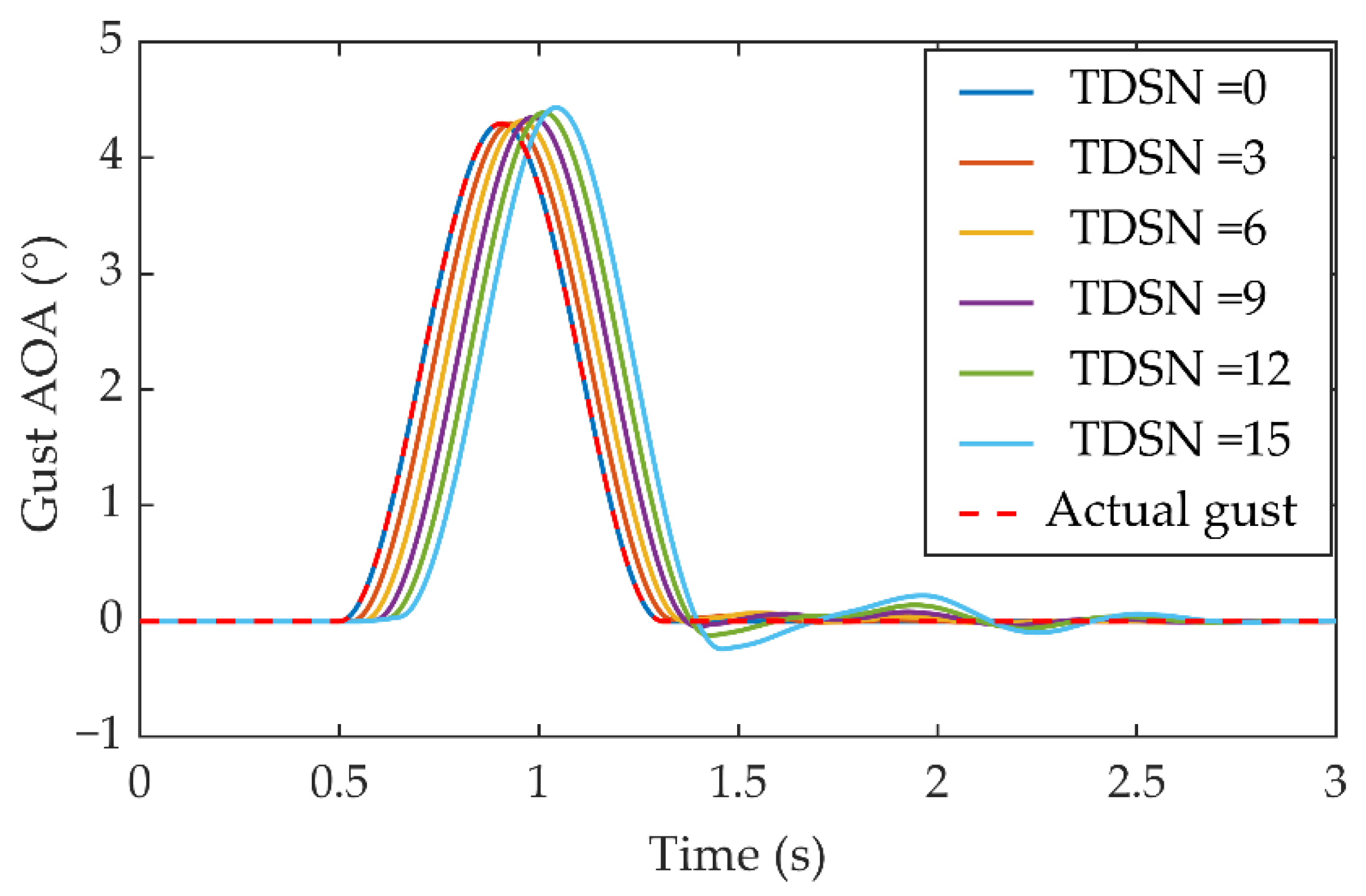
In the wind tunnel test, each signal related to the gust calculation can be measured with high quality by the sensors mounted on the motion device. High-quality gust information can be obtained through the gust calculation method in the wind tunnel environment. However, for the actual flights, the measured signal will contain more errors. For example, the vertical velocity of an aircraft is generally obtained by measuring atmospheric pressure or by a GPS, and the measured velocity is affected by multiple sensor errors. Also, when the aircraft flying with an arbitrary attitude that does not satisfy the simplification conditions, the gust information needs to be obtained by the non-simplified gust calculation method, which requires more signals, and the quality of the calculated result will be affected by more errors. The time delays of each signal also have a great influence on the gust calculation quality. These are important factors that require focus when applying the gust calculation method to actual flights. In this study, these problems are investigated through simulations.
6. Conclusions
This paper investigated the gust calculation method and feedforward gust alleviation. Firstly, the gust calculation method was derived and a simplified formula in the longitudinal axis was given. The influence of sensor characteristics on gust calculation accuracy and the gust alleviation effect was studied through numerical simulations. The test systems were also developed, including a gust generator, a two degrees-of-freedom motion device, an aircraft model, aircraft support device, and measurement and control system, based on which gust calculation and gust alleviation wind tunnel tests were carried out.
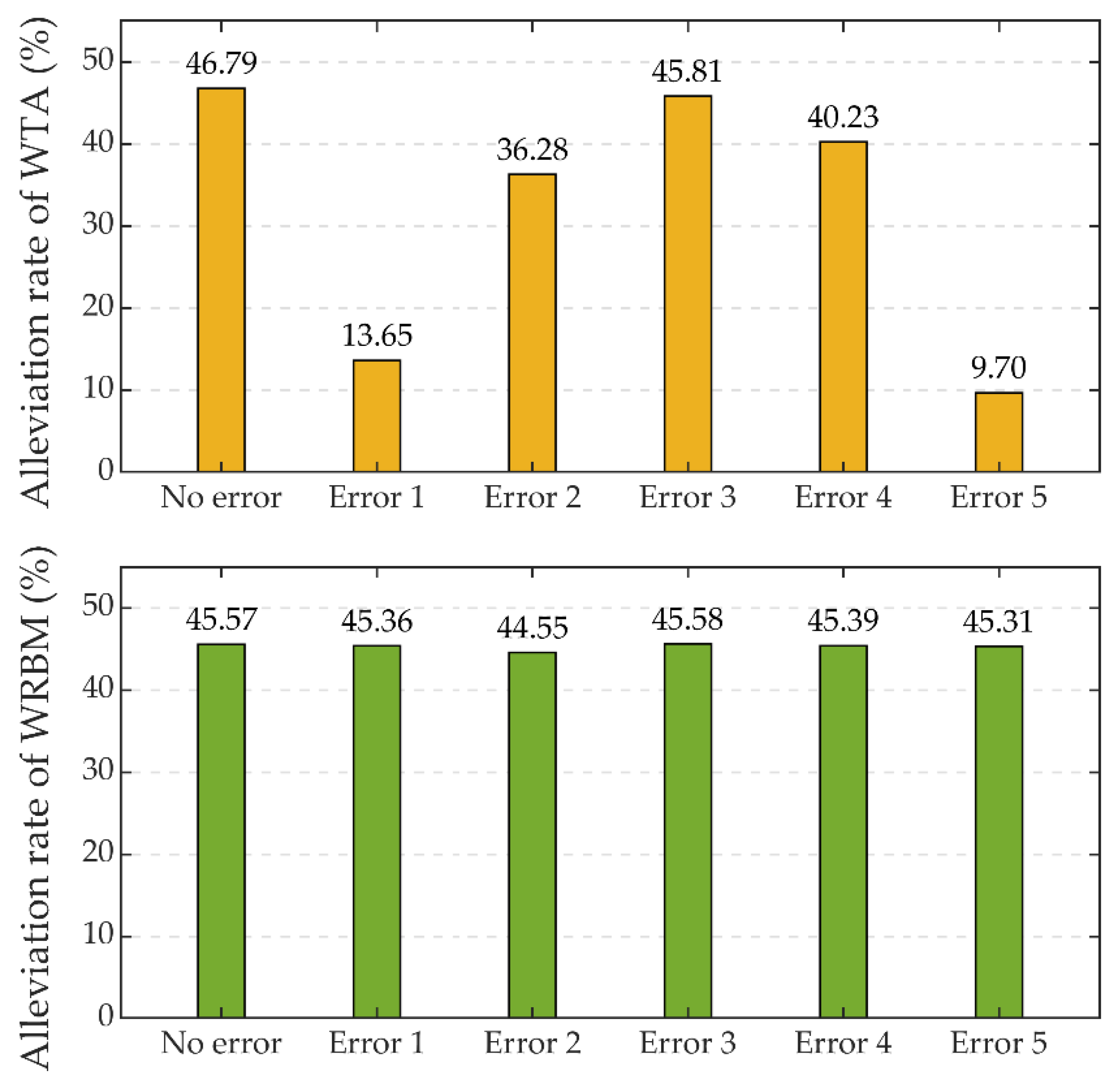
The gust calculation wind tunnel test results verified the effectiveness of the gust calculation method. The gust information obtained by the gust calculation system can truly reflect the actual gust in the wind tunnel flow field. Numerical simulations show that the sensor characteristics have an important influence on the accuracy of gust calculation and the effect of gust alleviation. In particular, excessive sensor delays will lead to a decrease of gust calculation accuracy and a deterioration of the gust alleviation effect. For example, a delay of nine sampling periods renders the gust alleviation system almost ineffective. The influence of sensor errors is relatively small. The FFCS based on gust calculation exhibits a good alleviation effect. For example, for the gust excitation at relevant frequencies (2.0 Hz and 2.5 Hz), the average alleviation rate of the WTA reaches 65.47%. Combining feedback control and feedforward control can achieve a better gust alleviation effect.
In the current work, the simplified gust calculation method has been verified by the wind tunnel test. In future work, it will be considered to verify the more complex three-dimensional gust calculation method through flight test, which is a more challenging and meaningful work and will lay a more solid foundation for practical applications. Second, the feedforward gust alleviation, based on gust calculation, is sensitive to the variation of the aircraft, and the adaptive feedforward controller design method also deserves in-depth study.