Mass Balance Sensitivity and Future Projections of Rabots Glaciär, Sweden
Abstract
1. Introduction
2. Materials and Methods
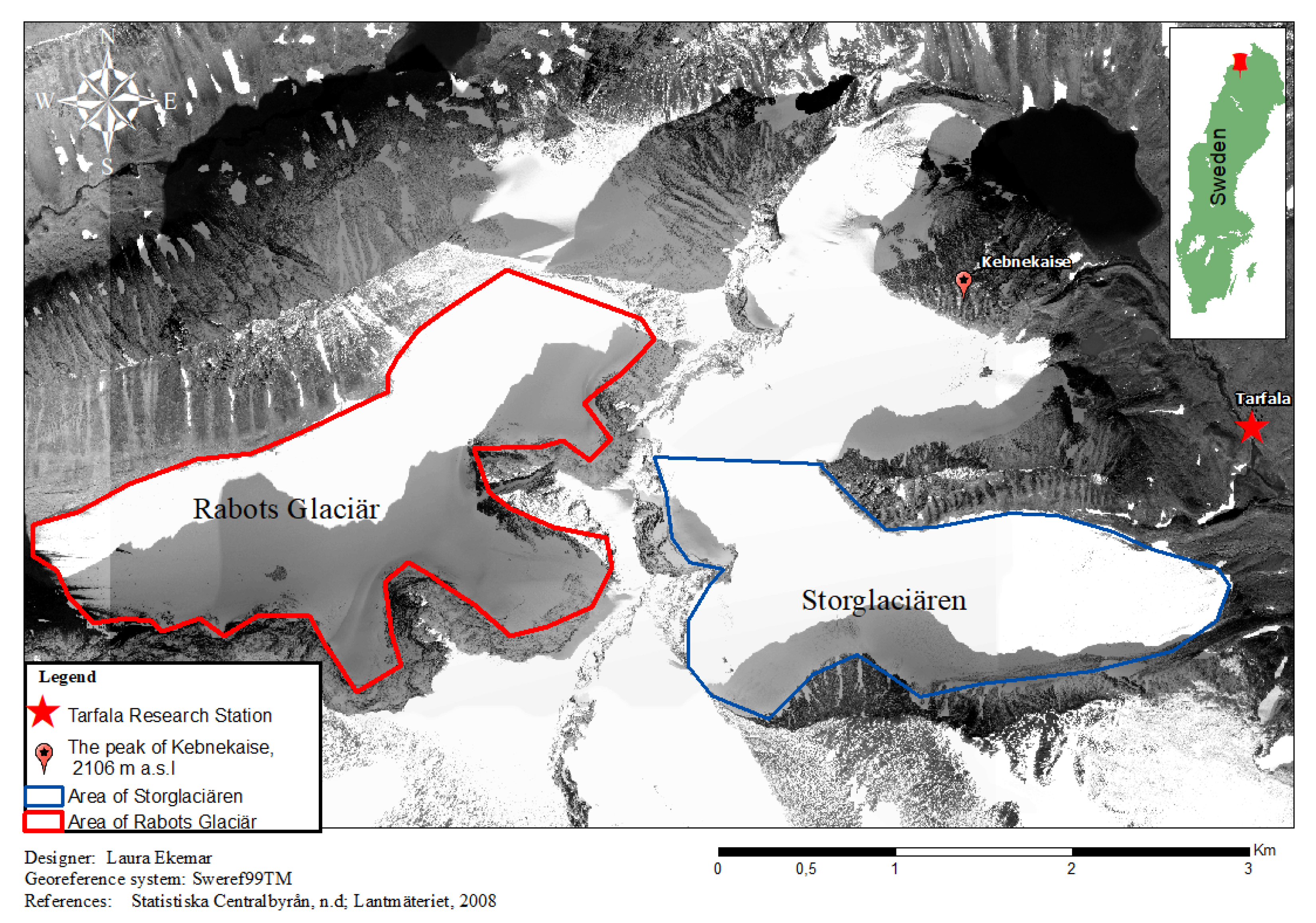
2.1. Study Area
2.2. Mass-Balance Sensitivity Computation
2.3. Future Projections of Mass Balance
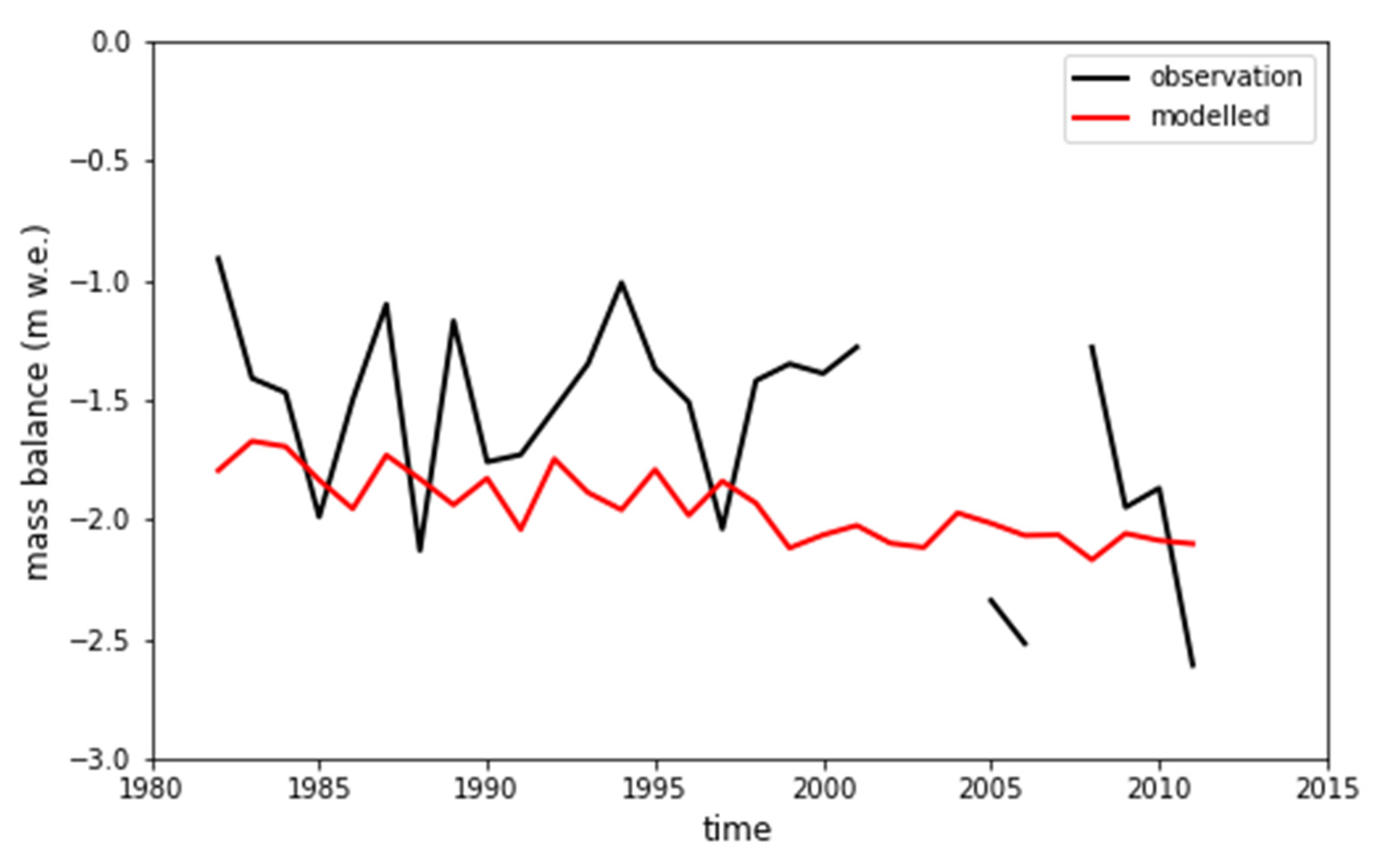
2.4. Validation
3. Results
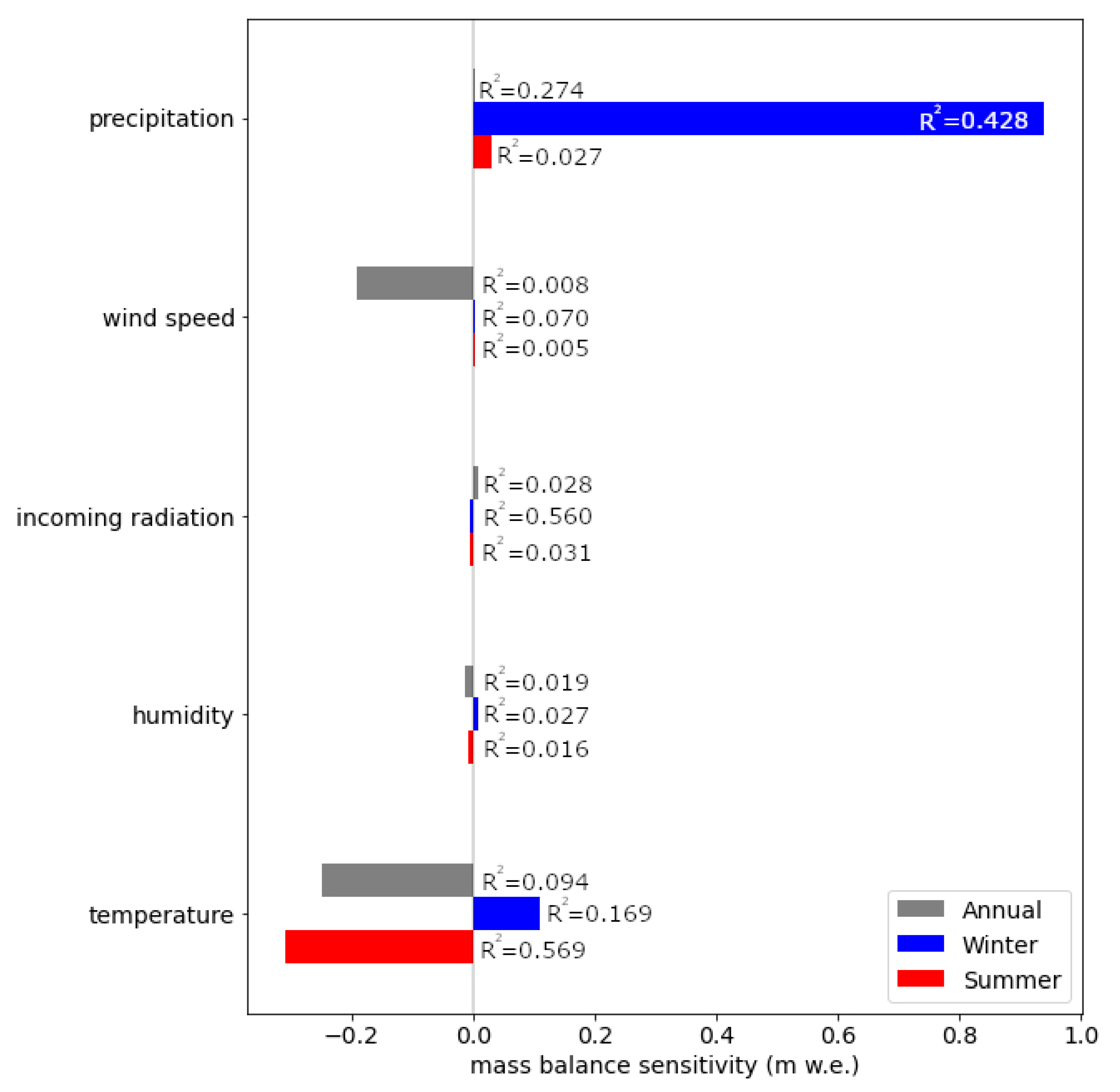
3.1. Sensitivity
3.1.1. Annual Mass Balance Sensitivity
3.1.2. Summer Mass Balance Sensitivity
3.1.3. Winter Mass Balance Sensitivity
3.2. Summer Mass Balance Prediction
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- NSIDC: Glaciers and Climate Change|National Snow and Ice Data Center. Available online: https://nsidc.org/cryosphere/glaciers/questions/climate.html (accessed on 15 December 2020).
- Cauvy-Fraunié, S.; Andino, P.; Espinosa, R.; Calvez, R.; Jacobsen, D.; Dangles, O. Ecological responses to experimental glacier-runoff reduction in alpine rivers. Nat. Commun. 2016, 7, 12025. [Google Scholar] [CrossRef]
- Brugger, K.A.; Pankratz, L. Changes in the geometry and volume of Rabots glaciär, Sweden, 2003–2011: Recent accelerated volume loss linked to more negative summer balances. Geogr. Ann. Ser. A Phys. Geogr. 2015, 97, 265–278. [Google Scholar] [CrossRef][Green Version]
- Seibert, J.; Jenicek, M.; Huss, M.; Ewen, T. Chapter 4—Snow and Ice in the Hydrosphere. In Snow and Ice-Related Hazards, Risks and Disasters; Shroder, J.F., Haeberli, W., Whiteman, C., Eds.; Academic Press: Boston, MA, USA, 2015; pp. 99–137. ISBN 978-0-12-394849-6. [Google Scholar]
- Baroni, C. Climate Change Impacts on Cold Climates. In Treatise on Geomorphology; Shroder, J.F., Ed.; Academic Press: San Diego, CA, USA, 2013; Volume 8, pp. 430–459. ISBN 978-0-08-088522-3. [Google Scholar]
- Curry, J.A.; Schramm, J.L.; Ebert, E.E. Sea Ice-Albedo Climate Feedback Mechanism. J. Clim. 1995, 8, 240–247. [Google Scholar] [CrossRef]
- Che, Y.; Zhang, M.; Zhongqin, L.; Wei, Y.; Nan, Z.; Li, H.; Wang, S.; Su, B. Energy balance model of mass balance and its sensitivity to meteorological variability on Urumqi River Glacier No.1 in the Chinese Tien Shan. Sci. Rep. 2019, 9. [Google Scholar] [CrossRef]
- Mills, S.C.; Brocq, A.M.L.; Winter, K.; Smith, M.; Hillier, J.; Ardakova, E.; Boston, C.M.; Sugden, D.; Woodward, J. Testing and application of a model for snow redistribution (Snow_blow) in the Ellsworth Mountains, Antarctica. J. Glaciol. 2019, 65, 957–970. [Google Scholar] [CrossRef]
- Schytt, V. The Glaciers of the Kebnekajse-Massif. Geogr. Ann. 1959, 41, 213–227. [Google Scholar] [CrossRef]
- Stroeven, A.; van der Wal, R. A Comparison of the Mass Balances and Flows of Rabots Glaciär and Storglaciären, Kebnekaise, Northern Sweden. Geogr. Ann. Ser. A Phys. Geogr. 1990, 72, 113–118. [Google Scholar] [CrossRef]
- Brugger, K.A. The non-synchronous responses of Rabots glaciär and Storglaciären, northern Sweden, to recent climate change: A comparative study. Ann. Glaciol. 2007, 46, 275–282. [Google Scholar] [CrossRef][Green Version]
- SMHI: Climate Scenarios|SMHI. Available online: https://www.smhi.se/en/climate/future-climate/climate-scenarios/sweden/district/norra-norrlands-fjalltrakter/rcp85/summer/temperature (accessed on 15 December 2020).
- Hock, R.; Radic, V.; de Would, M. Climate sensitivity of Storglaciaren, Sweden: An intercomparison of mass-balance models using ERA-40 re-analysis and regional climate model data. Int. Glaciol. Society 2007, 46, 342–348. [Google Scholar] [CrossRef]
- Brugger, K.A.; Refsnider, K.A.; Whitehill, M.F. Variation in glacier length and ice volume of Rabots Glaciär, Sweden, in response to climate change, 1910–2003. Ann. Glaciol. 2005, 42, 180–188. [Google Scholar] [CrossRef][Green Version]
- Tarfala Research Station. Mass Balance of Rabots Glaciär 2007/2008, Annual Report 2007/2008. Available online: https://bolin.su.se/data/tarfala/data/reports/2007-2008/Mass%20balance%20of%20Rabots%20glaci%C3%A4r%202007-2008.pdf (accessed on 29 July 2021).
- Bolin Centre Database. Tarfala Data: Rabots Glacier. Available online: https://bolin.su.se/data/tarfala/rabot.php (accessed on 3 December 2020).
- Rosqvist, G. Activity Report for Tarfala Research Station 2009. Available online: https://bolin.su.se/data/tarfala/data/reports/2008-2009/Activity%20report%202009.pdf (accessed on 20 December 2020).
- Bolin Center Database 2. Tarfala Data: Climate. Available online: https://bolin.su.se/data/tarfala/climate.php (accessed on 3 December 2020).
- Jonsell, U.; Hock, R.; Duguay, M. Recent air and ground temperature increases at Tarfala Research Station, Sweden. Polar Res. 2013, 32, 19807. [Google Scholar] [CrossRef]
- Dufresne, J.-L.; Foujols, M.-A.; Denvil, S.; Caubel, A.; Marti, O.; Aumont, O.; Balkanski, Y.; Bekki, S.; Bellenger, H.; Benshila, R.; et al. Climate change projections using the IPSL-CM5 Earth system model: From CMIP3 to CMIP5. Clim. Dyn. 2013, 40, 2123–2165. [Google Scholar] [CrossRef]
- Jacob, D.; Petersen, J.; Eggert, B.; Alias, A.; Christensen, O.B.; Bouwer, L.M.; Braun, A.; Colette, A.; Déqué, M.; Georgievski, G.; et al. EURO-CORDEX: New high-resolution climate change projections for European impact research. Reg. Environ. Chang. 2014, 14, 563–578. [Google Scholar] [CrossRef]
- IPCC. Climate Change 2014 Synthesis Report Summary for Policymakers; IPCC: Geneva, Switzerland, 2014; pp. 1–32. [Google Scholar]
- Chylek, P.; Li, J.; Dubey, M.K.; Wang, M.; Lesins, G. Observed and model simulated 20th century Arctic temperature variability: Canadian Earth System Model CanESM2. Atmos. Chem. Phys. 2011, 11, 22893–22907. [Google Scholar]
- Voldoire, A.; Sánchez-Gómez, E.; Salas y Mélia, D.; Decharme, B.; Cassou, C.; Sénési, S.; Valcke, S.; Beau, I.; Alias, A.; Chevallier, M.; et al. The CNRM-CM5.1 global climate model: Description and basic evaluation. Clim Dyn. 2012, 40, 2091–2121. [Google Scholar] [CrossRef]
- Jeffrey, S.; Rotstayn, L.M.; Collier, M.; Dravitzki, S.; Hamalainen, C.; Moeseneder, C.; Wong, W.; Syktus, J. Australia’s CMIP5 submission using the CSIRO-Mk3.6 model. Aust. Meteor. Oceanogr. J. 2013, 63, 1–13. [Google Scholar] [CrossRef]
- Dunne, J.P.; John, J.G.; Adcroft, A.J.; Griffies, S.M.; Hallberg, R.W.; Shevliakova, E.; Stouffer, R.J.; Cooke, W.; Dunne, K.A.; Harrison, M.J.; et al. GFDL’s ESM2 Global Coupled Climate-Carbon Earth System Models. Part I: Physical Formulation and Baseline Simulation Characteristics. J. Clim. 2012, 25, 6646–6665. [Google Scholar] [CrossRef]
- Watanabe, S.; Hajima, T.; Sudo, K.; Nagashima, T.; Takemura, T.; Okajima, H.; Nozawa, T.; Kawase, H.; Abe, M.; Yokohata, T.; et al. MIROC-ESM 2010: Model description and basic results of CMIP5-20c3m experiments. Geosci. Model. Dev. 2011, 4, 845–872. [Google Scholar] [CrossRef]
- Popke, D.; Stevens, B.; Voigt, A. Climate and climate change in a radiative- convective equilibrium version of ECHAM6. J. Adv. Modeling Earth Syst. 2013, 5, 1–14. [Google Scholar] [CrossRef]
- Bentsen, M.; Bethke, I.; Debernard, J.B.; Iversen, T.; Kirkevåg, A.; Seland, Ø.; Drange, H.; Roelandt, C.; Seierstad, I.A.; Hoose, C.; et al. The Norwegian earth system model, NorESM1-M. Part 1: Description and basic evaluation. Geosci. Mod. Dev. 2013, 6, 687–720. [Google Scholar] [CrossRef]
- Liu, L.; Jiang, L.; Sun, Y.; Wang, H.; Yi, C.; Hsu, H. Morphometric Controls on Glacier Mass Balance of the Puruogangri Ice Field, Central Tibetan Plateau. Water 2016, 8, 496. [Google Scholar] [CrossRef]
- Braithwaite, R.J.; Zhang, Y. Sensitivity of mass balance of five Swiss glaciers to temperature changes assessed by tuning a degree-day model. J. Glaciol. 2000, 46, 7–14. [Google Scholar] [CrossRef]
- Anderson, B.; Mackintosh, A.; Stumm, D.; George, L.; Kerr, T.; Winter-Billington, A.; Fitzsimons, S. Climate sensitivity of a high-precipitation glacier in New Zealand. J. Glaciol. 2010, 56, 114–128. [Google Scholar] [CrossRef]
- Woul, M.; Hock, R. Static mass-balance sensitivity of Arctic glaciers and ice caps using a degree-day approach. Ann. Glaciol. 2005, 42, 217–224. [Google Scholar] [CrossRef]
- Williamson, S.N.; Copland, L.; Thomson, L.; Burgess, D. Comparing simple albedo scaling methods for estimating Arctic glacier mass balance. Remote Sens. Environ. 2020, 246, 111858. [Google Scholar] [CrossRef]
- IPCC, 2000—Nebojsa Nakicenovic and Rob Swart (Eds.) Cambridge University Press, UK. pp. 570. Cambridge University Press, The Edinburgh Building Shaftesbury Road, Cambridge CB2 2RU ENGLAND. Available online: https://www.ipcc.ch/report/emissions-scenarios/ (accessed on 21 June 2021).


| Model Name | Modelling Centre | Reference |
|---|---|---|
| CanESM2 | Canadian Centre for Climate Modelling and Analysis | Chylek et al., 2011 [23] |
| CNRM-CM5 | Centre National de Recherches Météorologiques/Centre Européen de Recherche et Formation Avancée en Calcul Scientifique | Voldoire et al., 2012 [24] |
| CSIRO-Mk3.6 | CSIRO and Queensland Climate Change Centre of Excellence (QCCCE) | Jeffrey et al., 2011 [25] |
| GFDL-ESM2M | NOAA Geophysical Fluid Dynamics Laboratory | Dunne et al., 2012 [26] |
| IPSL-CM5A-MR | Institut Pierre-Simon Laplace | Dufresne et al., 2013 [20] |
| MIROC5 | Atmosphere and Ocean Research Institute (The University of Tokyo), National Institute for Environmental Studies, and Japan Agency for Marine-Earth Science and Technology | Watanabe et al., 2011 [27] |
| MPI-ESM-LR | Max Planck Institute for Meteorology | Popke et al., 2013 [28] |
| NorESM1-M | Norwegian Climate Centre | Bentsen et al., 2013 [29] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taveirne, M.; Ekemar, L.; González Sánchez, B.; Axelsson, J.; Zhang, Q. Mass Balance Sensitivity and Future Projections of Rabots Glaciär, Sweden. Climate 2021, 9, 126. https://doi.org/10.3390/cli9080126
Taveirne M, Ekemar L, González Sánchez B, Axelsson J, Zhang Q. Mass Balance Sensitivity and Future Projections of Rabots Glaciär, Sweden. Climate. 2021; 9(8):126. https://doi.org/10.3390/cli9080126
Chicago/Turabian StyleTaveirne, Moon, Laura Ekemar, Berta González Sánchez, Josefine Axelsson, and Qiong Zhang. 2021. "Mass Balance Sensitivity and Future Projections of Rabots Glaciär, Sweden" Climate 9, no. 8: 126. https://doi.org/10.3390/cli9080126
APA StyleTaveirne, M., Ekemar, L., González Sánchez, B., Axelsson, J., & Zhang, Q. (2021). Mass Balance Sensitivity and Future Projections of Rabots Glaciär, Sweden. Climate, 9(8), 126. https://doi.org/10.3390/cli9080126







