Climatology of Three-Dimensional Eliassen–Palm Wave Activity Fluxes in the Northern Hemisphere Stratosphere from 1981 to 2020
Abstract
1. Introduction
2. Materials and Methods
3. Results
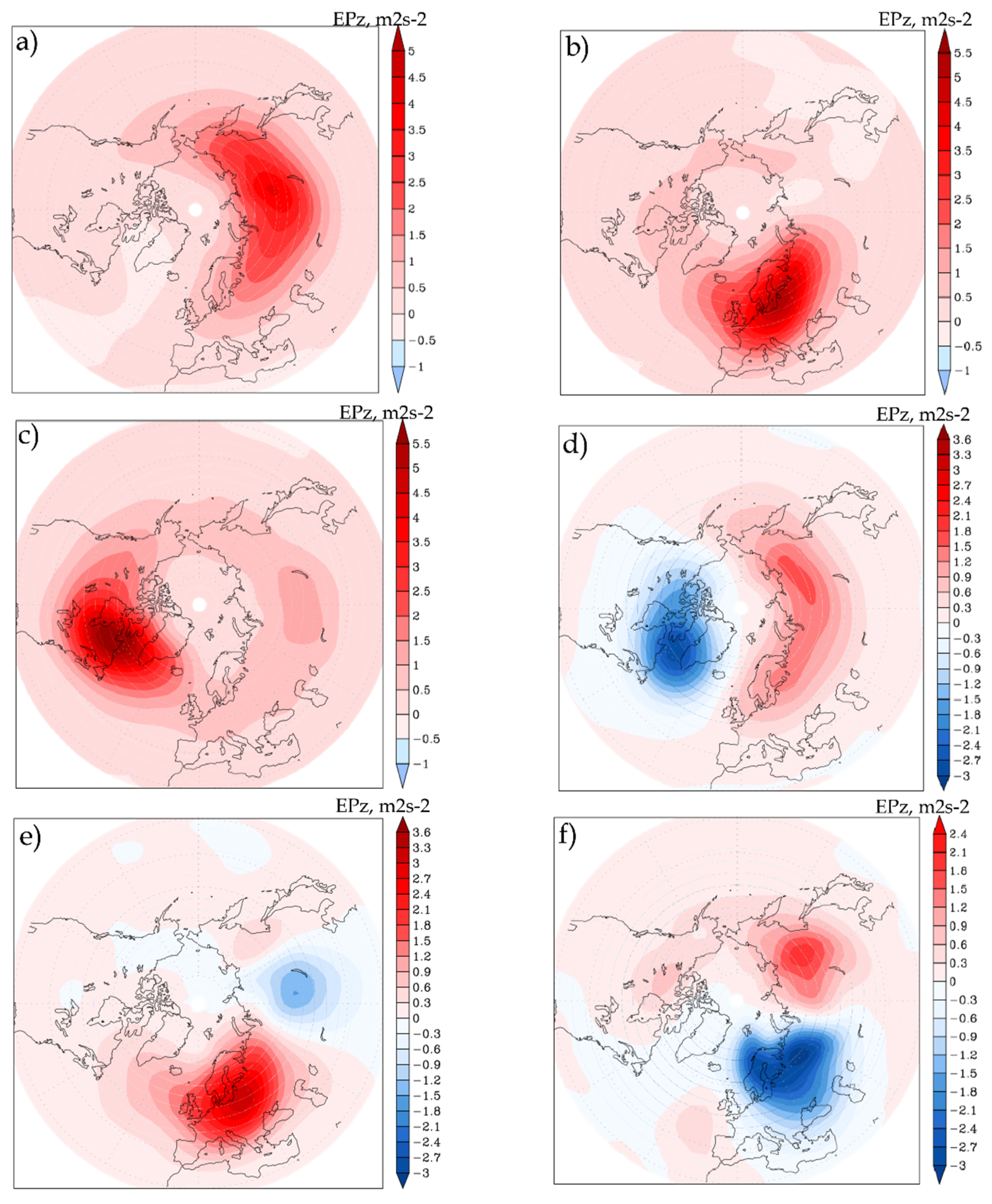
3.1. EPz WAF Climatology
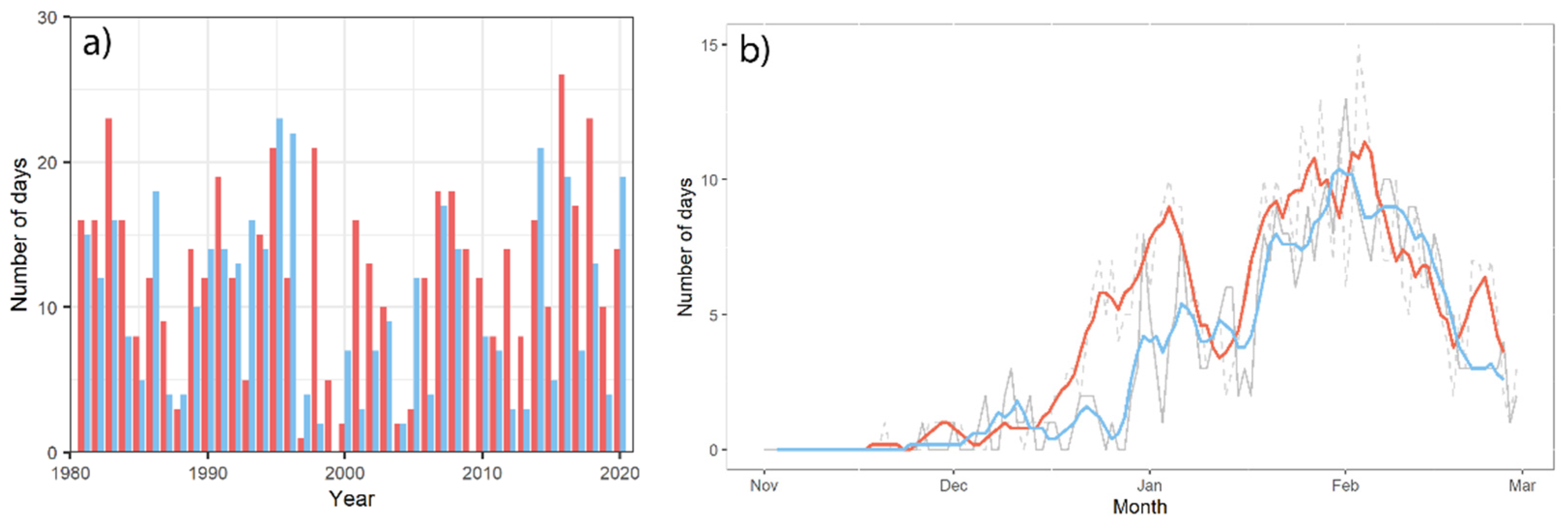
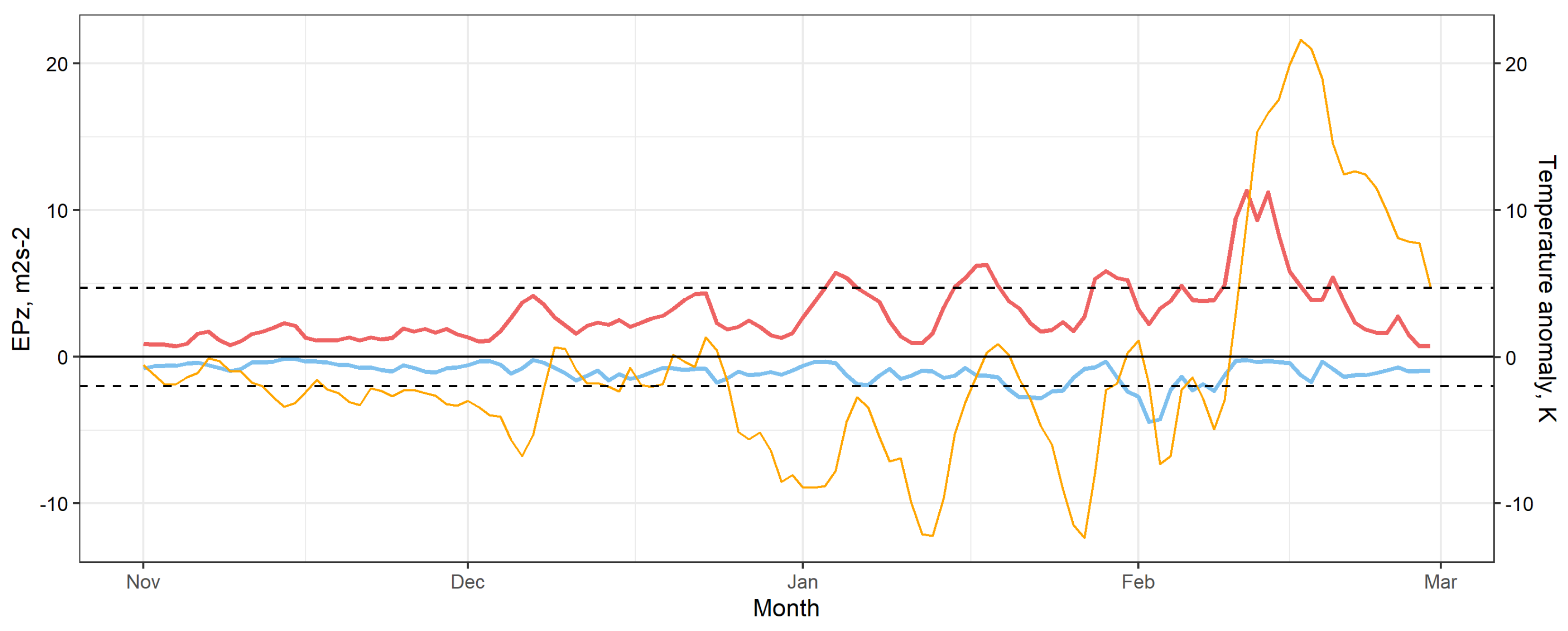
3.2. EPz WAF Tendencies over the Last 40 Years
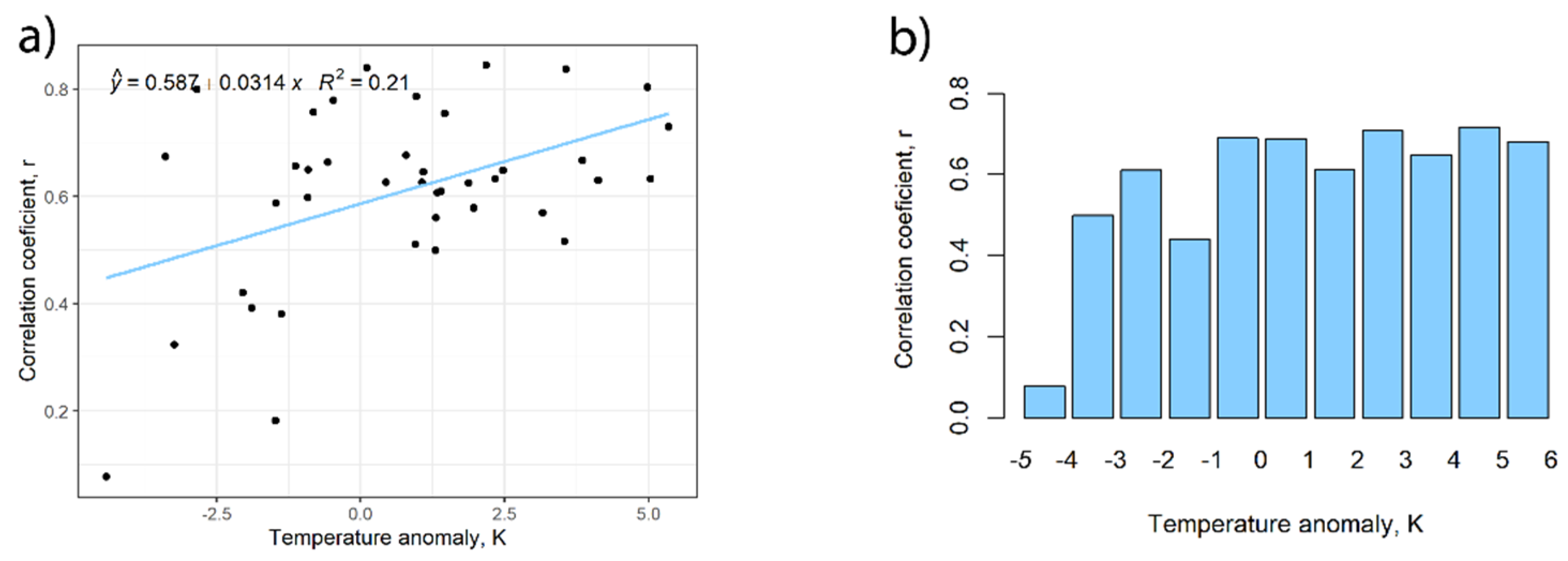
3.3. Statistical Relationships between EPzm and Stratospheric Temperature Anomalies
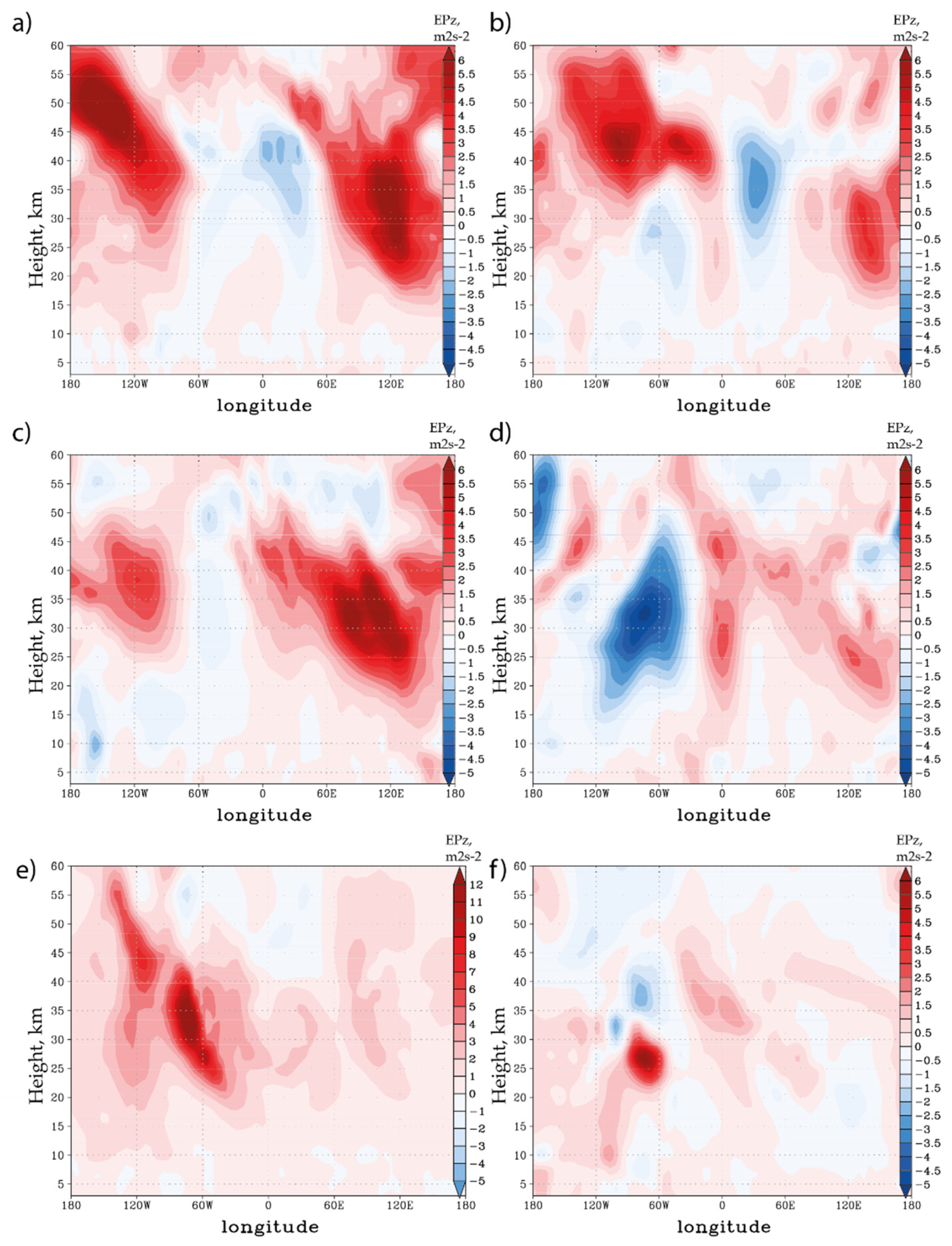
3.4. Geographical Characteristics of EPzm
4. Summary and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ↑EPzm | maximum of the upward vertical component of Eliassen–Palm wave activity flux; |
| ↓EPzm | minimum of the downward vertical component of Eliassen–Palm wave activity flux; |
| ↑EPze | 90th percentile of the maximum of the upward vertical component of Eliassen–Palm wave activity flux; |
| ↓EPze | 10th percentile of the minimum of the downward vertical component of Eliassen–Palm wave activity flux. |
References
- Baldwin, M.P.; Dunkerton, T.J. Propagation of the Arctic Oscillation from the stratosphere to the troposphere. J. Geophys. Res. 1999, 104, 30937–30946. [Google Scholar] [CrossRef]
- Perlwitz, J.; Harnik, N. Downward coupling between the stratosphere and troposphere: The relative roles of wave and zonal mean processes. J. Clim. 2004, 17, 4902–4909. [Google Scholar] [CrossRef]
- Kodera, K.; Mukougawa, H.; Itoh, S. Tropospheric impact of reflected planetary waves from the stratosphere. Geophys. Res. Lett. 2008, 35, L16806. [Google Scholar] [CrossRef]
- Cohen, J.; Screen, J.A.; Furtado, J.C.; Barlow, M.; Whittleston, D.; Coumou, D.; Francis, J.; Dethloff, K.; Entekhabi, D.; Overland, J.; et al. Recent Arctic amplification and extreme mid-latitude weather. Nat. Geosci. 2014, 7, 627–637. [Google Scholar] [CrossRef]
- Kidston, J.; Scaife, A.; Hardiman, S.; Mitchell, D.M.; Butchart, N.; Baldwin, M.P.; Gray, L.J. Stratospheric influence on tropospheric jet streams, storm tracks and surface weather. Nat. Geosci. 2015, 8, 433–440. [Google Scholar] [CrossRef]
- Cai, M.; Yu, Y.; Deng, Y.; van den Dool, H.M.; Ren, R.; Saha, S.; Wu, X.; Huang, J. Feeling the Pulse of the Stratosphere: An Emerging Opportunity for Predicting Continental-Scale Cold-Air Outbreaks 1 Month in Advance. Bull. Am. Meteorol. Soc. 2016, 97, 1475–1489. [Google Scholar] [CrossRef]
- Karpechko, A.Y.; Hitchcock, P.; Peters, D.H.W.; Schneidereit, A. Predictability of downward propagation of major sudden stratospheric warmings. Q. J. R. Meteorol. Soc. 2017, 143, 1459–1470. [Google Scholar] [CrossRef]
- Kretschmer, M.; Coumou, D.; Agel, L.; Barlow, M.; Tziperman, E.; Cohen, J. More-Persistent Weak Stratospheric Polar Vortex States Linked to Cold Extremes. Bull. Am. Meteor. Soc. 2018, 99, 49–60. [Google Scholar] [CrossRef]
- Kretschmer, M.; Cohen, J.; Matthias, V.; Runge, J.; Coumou, D. The different stratospheric influence on cold-extremes in Eurasia and North America. NPJ Clim. Atmos. Sci. 2014, 1, 44. [Google Scholar] [CrossRef]
- Lee, S.H.; Charlton-Perez, A.J.; Furtado, J.C.; Woolnough, S.J. Abrupt stratospheric vortex weakening associated with North Atlantic anticyclonic wave breaking. J. Geophys. Res. Atmos. 2019, 124, 8563–8575. [Google Scholar] [CrossRef]
- Zhou, X.; Chen, Q.; Wang, Z.; Xu, M.; Zhao, S.; Cheng, Z.; Feng, F. Longer Duration of the Weak Stratospheric Vortex During Extreme El Nino Events Linked to Spring Eurasian Coldness. J. Geophys. Res. Atmos. 2020, 125, e2019JD032331. [Google Scholar] [CrossRef]
- Cagnazzo, C.; Manzini, E.; Calvo, N.; Douglass, A.; Akiyoshi, H.; Bekki, S.; Chipperfield, M.; Dameris, M.; Deushi, M.; Fischer, A.M.; et al. Northern winter stratospheric temperature and ozone responses to ENSO inferred from an ensemble of Chemistry Climate Models. Atmos. Chem. Phys. 2009, 9, 8935–8948. [Google Scholar] [CrossRef]
- Xie, F.; Li, J.; Tian, W.; Feng, J.; Huo, Y. The Signals of El Niño Modoki in the Tropical Tropopause Layer and Stratosphere. Atmos. Chem. Phys. 2012, 12, 5259–5273. [Google Scholar] [CrossRef]
- Garfinkel, C.I.; Hartmann, D.L. Effects of El Nino—Southern Oscillation and the Quasi-Biennial Oscillation on polar temperatures in the stratosphere. J. Geophys. Res. 2007, 112, D19112. [Google Scholar] [CrossRef]
- Charlton, A.; Polvani, L. A new look at stratospheric sudden warmings. Part I: Climatology and modeling benchmarks. J. Clim. 2007, 20, 449–469. [Google Scholar] [CrossRef]
- Tripathi, O.P.; Baldwin, M.; Charlton-Perez, A.; Charron, M.; Cheung, J.C.H.; Eckermann, S.D.; Gerber, E.; Jackson, D.R.; Kuroda, Y.; Lang, A.; et al. Examining the Predictability of the Stratospheric Sudden Warming of January 2013 Using Multiple NWP Systems. Mon. Weather Rev. 2016, 144, 1935–1960. [Google Scholar] [CrossRef]
- Jucker, M.; Reichler, T. Dynamical precursors for statistical prediction of stratospheric sudden warming events. Geophys. Res. Lett. 2018, 45, 13–124. [Google Scholar] [CrossRef]
- Nath, D.; Chen, W.; Zelin, C.; Pogoreltsev, A.I.; Wei, K. Dynamics of 2013 Sudden Stratospheric Warming event and its impact on cold weather over Eurasia: Role of planetary wave reflection. Sci. Rep. 2016, 6, 24174. [Google Scholar] [CrossRef] [PubMed]
- Thuburn, J.; Craig, G.C. Stratospheric Influence on Tropopause Height: The Radiative Constraint. J. Atmos. Sci. 2000, 57, 17–28. [Google Scholar] [CrossRef]
- Karpechko, A.Y.; Charlton-Perez, A.; Balmaseda, M.; Tyrrell, N.; Vitart, F. Predicting sudden stratospheric warming 2018 and its climate impacts with a multimodel ensemble. Geophys. Res. Lett. 2018, 45, 13538–13546. [Google Scholar] [CrossRef]
- Polvani, L.M.; Waugh, D.W. Upward Wave Activity Flux as a Precursor to Extreme Stratospheric Events and Subsequent Anomalous Surface Weather Regimes. J. Clim. 2004, 17, 3548–3554. [Google Scholar] [CrossRef]
- Jaiser, R.; Dethloff, K.; Handorf, D. Stratospheric response to Arctic sea ice retreat and associated planetary wave propagation change. Tellus A Dyn. Meteorol. Oceanogr. 2013, 65, 1. [Google Scholar] [CrossRef]
- Solomon, A. Wave Activity Events and the Variability of the Stratospheric Polar Vortex. J. Clim. 2014, 27, 7796–7806. [Google Scholar] [CrossRef]
- Martineau, P.; Chen, G.; Son, S.-W.; Kim, J. Lower-stratospheric control of the frequency of sudden stratospheric warming events. J. Geophys. Res. Atmos. 2018, 123, 3051–3070. [Google Scholar] [CrossRef]
- Martineau, P.; Son, S. Onset of Circulation Anomalies during Stratospheric Vortex Weakening Events: The Role of Planetary-Scale Waves. J. Clim. 2015, 28, 7347–7370. [Google Scholar] [CrossRef]
- Nakagawa, K.I.; Yamazaki, K. What kind of stratospheric sudden warming propagates to the troposphere? Geophys. Res. Lett. 2006, 33, L04801. [Google Scholar] [CrossRef]
- Rao, J.; Garfinkel, C.I.; White, I.P. Predicting the downward and surface influence of the February 2018 and January 2019 sudden stratospheric warming events in subseasonal to seasonal (S2S) models. J. Geophys. Res. Atmos. 2020, 125, e2019JD031919. [Google Scholar] [CrossRef]
- Lee, S.H.; Furtado, J.C.; Charlton-Perez, A.J. Wintertime North American weather regimes and the Arctic stratospheric polar vortex. Geophys. Res. Lett. 2019, 46, 14892–14900. [Google Scholar] [CrossRef]
- Polvani, L.M.; Sun, L.; Butler, A.H.; Richter, J.H.; Deser, C. Distinguishing Stratospheric Sudden Warmings from ENSO as Key Drivers of Wintertime Climate Variability over the North Atlantic and Eurasia. J. Clim. 2017, 30, 1959–1969. [Google Scholar] [CrossRef]
- Butler, A.H.; Sjoberg, J.P.; Seidel, D.J.; Rosenlof, K.H. A sudden stratospheric warming compendium. Earth Syst. Sci. Data 2017, 9, 63–76. [Google Scholar] [CrossRef]
- King, A.D.; Butler, A.H.; Jucker, M.; Earl, N.O.; Rudeva, I. Observed relationships between sudden stratospheric warmings and European climate extremes. J. Geophys. Res. Atmos. 2019, 124, 13943–13961. [Google Scholar] [CrossRef]
- Domeisen, D.I.V.; Grams, C.M.; Papritz, L. The role of North Atlantic–European weather regimes in the surface impact of sudden stratospheric warming events. Weather Clim. Dynam. 2020, 1, 373–388. [Google Scholar] [CrossRef]
- Overland, J.; Hall, R.; Hanna, E.; Karpechko, A.; Vihma, T.; Wang, M.; Zhang, X. The Polar Vortex and Extreme Weather: The Beast from the East in Winter 2018. Atmosphere 2020, 11, 664. [Google Scholar] [CrossRef]
- Ke, W.; Wen, C. Northern Hemisphere Stratospheric Polar Vortex Extremes in February under the Control of Downward Wave Flux in the Lower Stratosphere. Atmos. Ocean. Sci. Lett. 2012, 5, 183–188. [Google Scholar] [CrossRef]
- Zyulyaeva, Y.A.; Zhadin, E.A. Analysis of three-dimensional Eliassen-Palm fluxes in the lower stratosphere. Russian Meteor. Hydrol. 2009, 34, 483–490. [Google Scholar] [CrossRef]
- Wei, K.; Ma, J.; Chen, W.; Vargin, P. Longitudinal peculiarities of planetary waves-zonal flow interactions and their role in stratosphere-troposphere dynamical coupling. Clim. Dyn. 2021, 1–20. [Google Scholar] [CrossRef]
- Plumb, R.A. On the three-dimensional propagation of stationary waves. J. Atmos. Sci. 1985, 42, 217–229. [Google Scholar] [CrossRef]
- Fletcher, C.G.; Kushner, P.J.; Cohen, J. Stratospheric control of the extratropical circulation response to surface forcing. Geophys. Res. Lett. 2007, 34, L21802. [Google Scholar] [CrossRef]
- Takaya, K.; Nakamura, H. A Formulation of a Phase-Independent Wave-Activity Flux for Stationary and Migratory Quasigeostrophic Eddies on a Zonally Varying Basic Flow. J. Atmos. Sci. 2001, 58, 608–627. [Google Scholar] [CrossRef]
- Domeisen, D.I.V.; Martius, O.; Jiménez-Esteve, B. Rossby wave propagation into the Northern Hemisphere stratosphere: The role of zonal phase speed. Geophys. Res. Lett. 2018, 45, 2064–2071. [Google Scholar] [CrossRef]
- Sussman, H.S.; Raghavendra, A.; Roundy, P.E.; Dai, A. Trends in northern midlatitude atmospheric wave power from 1950 to 2099. Clim. Dyn. 2020, 54, 2903–2918. [Google Scholar] [CrossRef]
- Cohen, J.; Furtado, J.C.; Jones, J.; Barlow, M.; Whittleston, D.; Entekhabi, D. Linking Siberian Snow Cover to Precursors of Stratospheric Variability. J. Clim. 2014, 27, 5422–5432. [Google Scholar] [CrossRef]
- Petoukhov, V.; Semenov, V.A. A link between reduced Barents-Kara sea ice and cold winter extremes over northern continents. J. Geophys. Res. 2010, 115, D21111. [Google Scholar] [CrossRef]
- Peings, Y. Ural blocking as a driver of early-winter stratospheric warmings. Geophys. Res. Lett. 2019, 46, 5460–5468. [Google Scholar] [CrossRef]
- Garfinkel, C.I.; Hartmann, D.L.; Sassi, F. Tropospheric Precursors of Anomalous Northern Hemisphere Stratospheric Polar Vortices. J. Clim. 2010, 23, 3282–3299. [Google Scholar] [CrossRef]
- Zhang, R.; Tian, W.; Wang, T. Role of the quasi-biennial oscillation in the downward extension of stratospheric northern annular mode anomalies. Clim. Dyn. 2020, 55, 595–612. [Google Scholar] [CrossRef]
- Petoukhov, V.; Rahmstorf, S.; Petri, S.; Schellnhuber, H.J. Quasiresonant amplification of planetary waves and recent Northern Hemisphere weather extremes. Proc. Natl. Acad. Sci. USA 2013, 110, 5336–5341. [Google Scholar] [CrossRef]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.A.; Balsamo, G.; Bauer, P.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Uppala, S.M.; Kållberg, P.W.; Simmons, A.J.; Andrae, U.; da Costa Bechtold, V.; Fiorino, M.; Gibson, J.K.; Haseler, J.; Hernandez, A.; Kelly, G.A.; et al. The ERA-40 re-analysis. Q. J. R. Meteorol. Soc. 2005, 131, 2961–3012. [Google Scholar] [CrossRef]
- Kistler, R.; Collins, W.; Saha, S.; White, G.; Woollen, J.; Kalnay, E.; Chelliah, M.; Ebisuzaki, W.; Kanamitsu, M.; Kousky, V.; et al. The NCEP–NCAR 50-year reanalysis: Monthly means CD-ROM and documentation. Bull. Am. Meteorol. Soc. 2001, 82, 247–267. [Google Scholar] [CrossRef]
- Gelaro, R.; McCarty, W.; Suárez, M.J.; Todling, R.; Molod, A.; Takacs, L.; Randles, C.A.; Darmenov, A.; Bosilovich, M.G.; Reichle, R.; et al. The Modern-Era Retrospective Analysis for Research and Applications, Version 2 (MERRA-2). J. Clim. 2017, 30, 5419–5454. [Google Scholar] [CrossRef] [PubMed]
- Wargan, K.; Coy, L. Strengthening of the Tropopause Inversion Layer during the 2009 Sudden Stratospheric Warming: A MERRA-2 Study. J. Atmos. Sci. 2016, 73, 1871–1887. [Google Scholar] [CrossRef]
- Huang, C.; Li, W.; Zhang, S.; Chen, G.; Huang, K.; Gong, Y.; Dai, A. Investigation of dominant traveling 10-day wave components using long-term MERRA-2 database. Earth Planets Space 2021, 73, 85. [Google Scholar] [CrossRef]
- Coy, L.; Newman, P.A.; Pawson, S.; Lait, L.R. Dynamics of the Disrupted 2015-16 Quasi-Biennial Oscillation. J. Clim. 2017, 30, 5661–5674. [Google Scholar] [CrossRef]
- Attard, H.E.; Lang, A.L. Troposphere–Stratosphere Coupling Following Tropospheric Blocking and Extratropical Cyclones. Mon. Weather Rev. 2019, 147, 1781–1804. [Google Scholar] [CrossRef]
- Steiner, A.K.; Ladstädter, F.; Randel, W.J.; Maycock, A.C.; Fu, Q.; Claud, C.; Gleisner, H.; Haimberger, L.; Ho, S.-P.; Keckhut, P.; et al. Observed Temperature Changes in the Troposphere and Stratosphere from 1979 to 2018. J. Clim. 2020, 33, 8165–8194. [Google Scholar] [CrossRef]
- Karpechko, A.Y.; Maycock, A.C.; Abalos, M.; Akiyoshi, H.; Arblaster, J.M.; Garfinkel, C.I.; Rosenlof, K.H.; Sigmond, M. Stratospheric Ozone Changes and Climate. In Scientific Assessment of Ozone Depletion; Fahey, D.W., Newman, P.A., Pyle, J.A., Safari, B., Eds.; World Meteorological Organization: Geneva, Switzerland, 2018; Volume 58. [Google Scholar]
- Perlwitz, J.; Harnik, N. Observational evidence of a stratospheric influence on the troposphere by planetary wave reflection. J. Clim. 2003, 16, 3011–3026. [Google Scholar] [CrossRef]
- Jadin, E.A. Stratospheric “wave hole” and interannual variations of the stratospheric circulation in late winter. Nat. Sci. 2011, 3, 259–267. [Google Scholar] [CrossRef][Green Version]

| Month | Average ↑EPzm | SD ↑EPzm | Absolute ↑EPzm Monthly Maxima | SD abs. Maxima ↑EPzm | Average ↓EPzm | SD ↓EPzm | Absolute ↓EPzm Monthly Minima | SD abs. Minima ↓EPzm |
|---|---|---|---|---|---|---|---|---|
| November | 1.45 | 0.36 | 5.67 | 1.03 | −0.49 | 0.13 | −2.18 | 0.43 |
| December | 2.51 | 0.64 | 8.33 | 1.43 | −0.87 | 0.19 | −3.37 | 0.58 |
| January | 3.18 | 0.85 | 9.21 | 1.79 | −1.24 | 0.42 | −6.39 | 1.26 |
| February | 2.85 | 1.00 | 11.33 | 2.33 | −1.21 | 0.48 | −7.42 | 1.57 |
| NDJF season | 2.50 | 1.56 | 11.33 | 1.56 | –0.95 | 0.18 | –7.42 | 1.28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gečaitė, I. Climatology of Three-Dimensional Eliassen–Palm Wave Activity Fluxes in the Northern Hemisphere Stratosphere from 1981 to 2020. Climate 2021, 9, 124. https://doi.org/10.3390/cli9080124
Gečaitė I. Climatology of Three-Dimensional Eliassen–Palm Wave Activity Fluxes in the Northern Hemisphere Stratosphere from 1981 to 2020. Climate. 2021; 9(8):124. https://doi.org/10.3390/cli9080124
Chicago/Turabian StyleGečaitė, Indrė. 2021. "Climatology of Three-Dimensional Eliassen–Palm Wave Activity Fluxes in the Northern Hemisphere Stratosphere from 1981 to 2020" Climate 9, no. 8: 124. https://doi.org/10.3390/cli9080124
APA StyleGečaitė, I. (2021). Climatology of Three-Dimensional Eliassen–Palm Wave Activity Fluxes in the Northern Hemisphere Stratosphere from 1981 to 2020. Climate, 9(8), 124. https://doi.org/10.3390/cli9080124





