Kinetic Isotope Effect in the Unfolding of a Protein Secondary Structure: Calculations for Beta-Sheet Polyglycine Dimers as a Model
Abstract
1. Introduction
2. Methods
2.1. Quantum Chemical Calculations
2.2. Rate Constant Calculations
3. Results
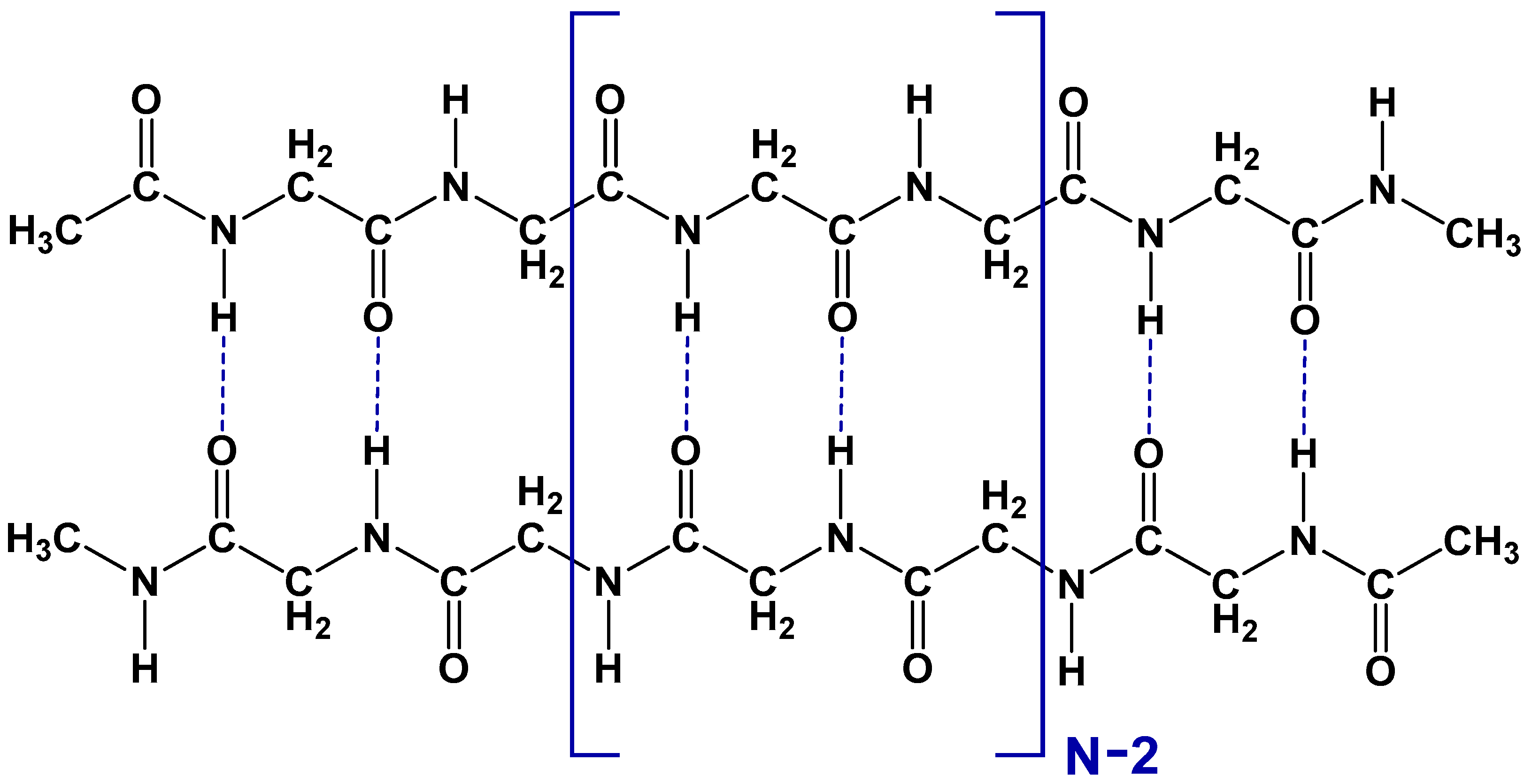
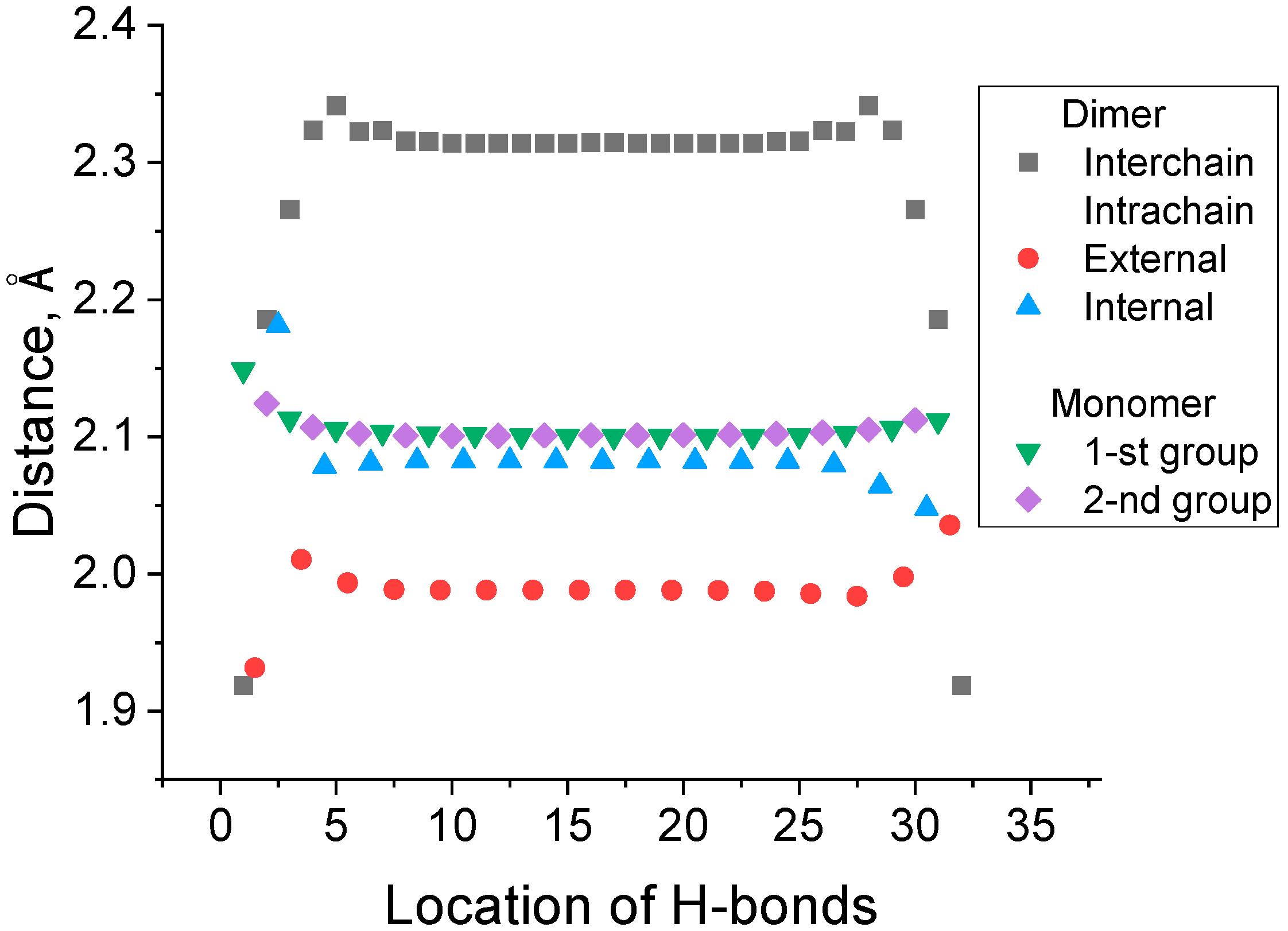
3.1. The Types of Hydrogen Bonds in Polyglycine Dimers and Monomers
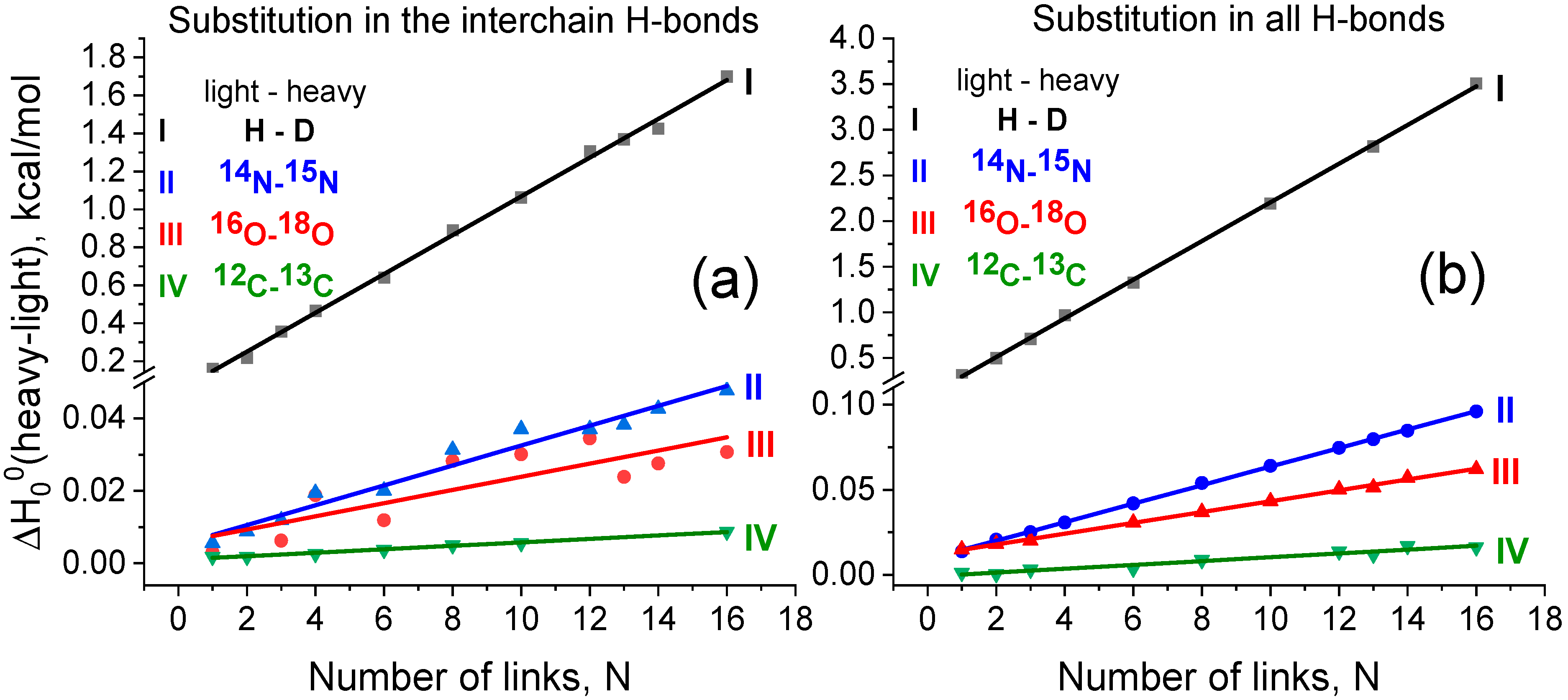
3.2. Effect of Isotope Substitution on the Strength of the Interchain H-Bond and Total Binding Energy of the Secondary Structure of the Dimer
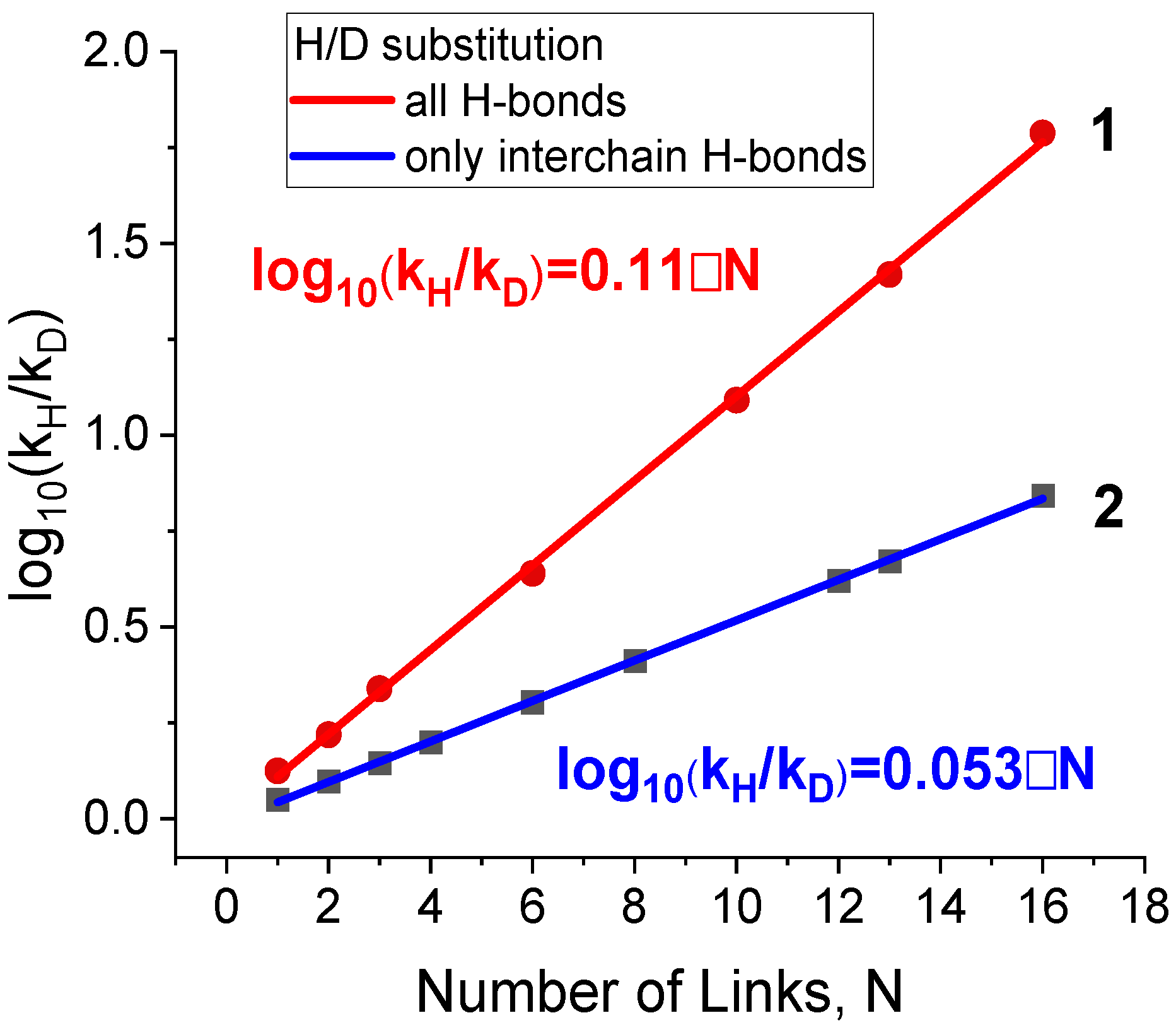
3.3. Effect of Isotope Substitution on the Rate Constant of Unfolding
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lewis, G.N. The biology of heavy water. Science 1934, 79, 151–153. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.H. (Ed.) Deuterium Oxide and Deuteration in Biosciences; Springer Nature Switzerland AG: Cham, Switzerland, 2022. [Google Scholar]
- Guild, W.R.; van Tubergen, R.P. Heat inactivation of catalase in deuterium oxide. Science 1957, 125, 939. [Google Scholar] [CrossRef] [PubMed]
- Ben-Naim, A. The Protein Folding Problem and Its Solutions; World Scientific: Singapore, 2013. [Google Scholar]
- Bahar, I.; Jernigan, R.L.; Dill, K. Protein Actions: Principles and Modeling; Garland Science: New York, NY, USA, 2017. [Google Scholar]
- Alexandrov, V.Y.; Vitvitskii, V.N.; Levdikova, G.A. The influence of D2O on the resistance of the procollagen to heat denaturation and to the collagenase action. Dokl. Akad. Nauk SSSR (Biochem.) 1975, 220, 1445–1448. [Google Scholar]
- Lemm, U.; Wenzel, M. Stabilisation of Enzymes and Antisera by Heavy Water. Eur. J. Biochem. 1981, 116, 441–445. [Google Scholar] [CrossRef] [PubMed]
- Heyde, C.; Wenzel, M. Heavy water (D2O) as a protective protein containing medium. Example: Human cholinesterase. Z. Naturforsch. C J. Biosci. 1991, 46, 789–793. [Google Scholar] [CrossRef]
- Chakrabarti, G.; Kim, S.; Gupta, M.L., Jr.; Barton, J.S.; Himes, R.H. Stabilization of tubulin by deuterium oxide. Biochemistry 1999, 38, 3067–3072. [Google Scholar] [CrossRef] [PubMed]
- Efimova, Y.M.; Haemers, S.; Wierczinski, B.; Norde, W.; van Well, A.A. Stability of globular proteins in H2O and D2O. Biopolymers 2007, 85, 264–273. [Google Scholar] [CrossRef]
- Mizuno, K.; Bachinger, H.P. The effect of deuterium oxide on the stability of the collagen model peptides H-(Pro-Pro-Gly)(10)-OH, H-(Gly-Pro-4(R)Hyp)(9)-OH, and Type I collagen. Biopolymers 2010, 93, 93–101. [Google Scholar] [CrossRef]
- Fu, L.; Villette, S.; Petoud, S.; Fernandez-Alonso, F.; Saboungi, M.-L. H/D isotope effects in protein thermal denaturation: The case of bovine serum albumin. J. Phys. Chem. B 2011, 115, 1881–1888. [Google Scholar] [CrossRef]
- Singha, H.; Goyal, S.K.; Malik, P.; Singh, R.K. Use of heavy water (D2O) in developing thermostable recombinant p26 protein based enzyme-linked immunosorbent assay for serodiagnosis of equine infectious anemia virus infection. Sci. World J. 2014, 2014, 620906. [Google Scholar] [CrossRef] [PubMed]
- Cho, Y.; Sagle, L.B.; Iimura, S.; Zhang, Y.; Kherb, J.; Chilkoti, A.; Scholtz, J.M.; Cremer, P.S. Hydrogen Bonding of β-turn structure is stabilized in D2O. J. Am. Chem. Soc. 2009, 131, 15188–15193. [Google Scholar] [CrossRef]
- Wu, R.; Georgescu, M.M.; Delpeyroux, F.; Guillot, S.; Balanant, J.; Simpson, K.; Crainic, R. Thermostabilization of live virus vaccines by heavy water (D2O). Vaccine 1995, 13, 1058–1063. [Google Scholar] [CrossRef]
- Ikizler, M.R.; Wright, P.F. Thermostabilization of egg grown influenza viruses. Vaccine 2002, 20, 1393–1399. [Google Scholar] [CrossRef] [PubMed]
- Adebayo, A.A.; Sim-Brandenburg, J.W.; Emmel, H.; Olaleye, D.O.; Niedrig, M. Stability of 17D Yellow Fever Virus Vaccine Using Different Stabilizers. Biologicals 1998, 26, 309–316. [Google Scholar] [CrossRef]
- Gandge, R.; Londhe, S.P.; Sherikar, A.A. Thermostabilization of live Newcastle disease virus (La Sota) vaccine using deuterium oxide (D2O). Indian J. Anim. Sci. 2012, 82, 1518–1520. [Google Scholar] [CrossRef]
- Alexandrov, V.Y.; Ponomarenko, V.V.; Ivanova, G.O. The influence of heavy water (D2O) on the thermopreferendum in Drosophila melanogaster. J. Therm. Biol. 1985, 10, 205–207. [Google Scholar] [CrossRef]
- Li, X.; Snyder, M.P. Can heavy isotopes increase lifespan? Studies of relative abundance in various organisms reveal chemical perspectives on aging. Bioessays 2016, 38, 1093–1101. [Google Scholar] [CrossRef][Green Version]
- Li, X.; Snyder, M.P. Yeast longevity promoted by reversing aging-associated decline in heavy isotope content. Npj Aging Mech. Dis. 2016, 2, 16004. [Google Scholar] [CrossRef] [PubMed]
- Filiou, M.D.; Varadarajulu, J.; Teplytska, L.; Reckow, S.; Maccarrone, G.; Turck, C.W. The 15N isotope effect in Escherichia coli: A neutron can make the difference. Proteomics 2012, 12, 736–747. [Google Scholar] [CrossRef]
- Andriukonis, E.; Gorokhova, E. Kinetic 15N-isotope effects on algal growth. Sci. Rep. 2017, 7, 44181. [Google Scholar] [CrossRef]
- Melander, L.; Saunders, W.H. Reaction Rates of Isotopic Molecules; Wiley: New York, NY, USA, 1980. [Google Scholar]
- Sverdlov, L.M.; Kovner, M.A.; Krainov, E.P. Vibrational Spectra of Polyatomic Molecules; Wiley: New York, NY, USA; Toronto, UK, 1974. [Google Scholar]
- Scheiner, S.; Čuma, M. Relative stability of hydrogen and deuterium bonds. J. Am. Chem. Soc. 1996, 118, 1511–1521. [Google Scholar] [CrossRef]
- Baklanov, A.V.; Kiselev, V.G. The Nature of the Enthalpy–Entropy Compensation and “Exotic” Arrhenius Parameters in the Denaturation Kinetics of Proteins. Int. J. Mol. Sci. 2023, 24, 10630. [Google Scholar] [CrossRef] [PubMed]
- Moore, W.H.; Krimm, S. Vibrational analysis of peptides, polypeptides, and proteins. I. Polyglycine I. Biopolymers 1976, 15, 2439–2464. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision, C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed]
- Mundlapati, V.R.; Imani, Z.; D’mello, V.C.; Brenner, V.; Gloaguen, E.; Baltaze, J.P.; Robin, S.; Mons, M.; Aitken, D.J. N–H/X interactions stabilize intra-residue C5 hydrogen bonded conformations in heterocyclic aamino acid derivatives. Chem. Sci. 2021, 12, 14826–14832. [Google Scholar] [CrossRef]
- Rosman, K.J.R.; Taylor, P.D.P. Isotopic compositions of the elements 1997 (Technical Report). Pure Appl. Chem. 1998, 70, 217–235. [Google Scholar] [CrossRef]
- Cioni, P.; Strambini, G.B. Effect of heavy water on protein flexibility. Biophys. J. 2002, 82, 3246–3253. [Google Scholar] [CrossRef]
- Mendonca, L.; Steinbacher, A.; Bouganne, R.; Hache, F. Comparative Study of the Folding/Unfolding Dynamics of Poly(glutamic acid) in Light and Heavy Water. J. Phys. Chem. B 2014, 118, 5350–5356. [Google Scholar] [CrossRef]
- Boese, A.D. Density Functional Theory and Hydrogen Bonds: Are We There Yet? ChemPhysChem 2015, 16, 978–985. [Google Scholar] [CrossRef] [PubMed]
- McGuire, R.F.; Momany, F.A.; Scheraga, H.A. Energy Parameters in Polypeptides. V. Empirical Hydrogen Bond Potential Function Based on Molecular Orbital Calculations. J. Phys. Chem. 1972, 76, 375–393. [Google Scholar] [CrossRef]
- Herzberg, G. Molecular Spectra and Molecular Structure. I. Spectra of Diatomic Molecules; Van Nostrand: Princeton, NJ, USA, 1950. [Google Scholar]

| Isotope-Substituted Hydrogen Bond | Interchain Hydrogen Bonds | All Hydrogen Bonds | Heavy Isotope Natural Abundance, % [33] | |
|---|---|---|---|---|
cal/mol | cal/mol | cal/mol | ||
| 102.3 ± 1.6 | 51.1 ± 0.8 | 211.9 ± 1.9 | 0.0115 (D) (in water) | |
| 2.74 ± 0.17 | 1.4 ± 0.1 | 5.44 ± 0.03 | 0.368 (15N) | |
| 1.8 ± 0.4 | 0.9 ± 0.2 | 3.18 ± 0.05 | 0.205 (18O) | |
| 0.48 ± 0.02 | 0.24 ± 0.01 | 1.12 ± 0.10 | 1.07 (13C) | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yanshin, A.O.; Kiselev, V.G.; Baklanov, A.V. Kinetic Isotope Effect in the Unfolding of a Protein Secondary Structure: Calculations for Beta-Sheet Polyglycine Dimers as a Model. Biomolecules 2025, 15, 92. https://doi.org/10.3390/biom15010092
Yanshin AO, Kiselev VG, Baklanov AV. Kinetic Isotope Effect in the Unfolding of a Protein Secondary Structure: Calculations for Beta-Sheet Polyglycine Dimers as a Model. Biomolecules. 2025; 15(1):92. https://doi.org/10.3390/biom15010092
Chicago/Turabian StyleYanshin, Alexey O., Vitaly G. Kiselev, and Alexey V. Baklanov. 2025. "Kinetic Isotope Effect in the Unfolding of a Protein Secondary Structure: Calculations for Beta-Sheet Polyglycine Dimers as a Model" Biomolecules 15, no. 1: 92. https://doi.org/10.3390/biom15010092
APA StyleYanshin, A. O., Kiselev, V. G., & Baklanov, A. V. (2025). Kinetic Isotope Effect in the Unfolding of a Protein Secondary Structure: Calculations for Beta-Sheet Polyglycine Dimers as a Model. Biomolecules, 15(1), 92. https://doi.org/10.3390/biom15010092







