Tutorial for Stopped-Flow Water Flux Measurements: Why a Report about “Ultrafast Water Permeation through Nanochannels with a Densely Fluorous Interior Surface” Is Flawed

Abstract

Millions of years of evolution have produced proteinaceous water channels (aquaporins) that combine perfect selectivity with a transport rate at the edge of the diffusion limit. However, Itoh et al. recently claimed in Science that artificial channels are 100 times faster and almost as selective. The published deflation kinetics of vesicles containing channels or channel elements indicate otherwise, since they do not demonstrate the facilitation of water transport. In an illustrated tutorial on the experimental basis of stopped-flow measurements, we point out flaws in data processing. In contrast to the assumption voiced in Science, individual vesicles cannot simultaneously shrink with two different kinetics. Moreover, vesicle deflation within the dead time of the instrument cannot be detected. Since flawed reports of ultrafast water channels in Science are not a one-hit-wonder as evidenced by a 2018 commentary by Horner and Pohl in Science, we further discuss the achievable limits of single-channel water permeability. After analyzing (i) diffusion limits for permeation through narrow channels and (ii) hydrodynamics in the surrounding reservoirs, we conclude that it is unlikely to fundamentally exceed the evolutionarily optimized water-channeling performance of the fastest aquaporins while maintaining near-perfect selectivity.

1. Introduction

Water scarcity is a global problem expected to become increasingly pressing in the future, as no globally affordable technical solutions for water separation have yet been identified. Mimicking the water-selective membranes of biological systems may appeal as the desired solution: in every kingdom of life, nature employs very narrow channels that are only about one water molecule wide, chief among them aquaporins. Such geometry enables perfect selectivity [1] because hydrated ions are too bulky to pass [2].

Unfortunately, hitherto aquaporin-containing membranes for water separation represent neither an affordable nor scalable solution to the problem of water scarcity. Among many reasons, the comparatively thick aquaporin wall renders the ratio of the cross-sectional area of the pore lumen to the total area occupied by the channel unfavorable for technical applications [3]. Nanofiltration membranes from aquaporins might only reach good porosities if the channels were packed in 2D crystals, which thus far appears unattainable on industrial scales. Widening the channel lumen above 3–4 Å would also increase porosity. Yet, natural channels with such a lumen are no longer water selective [4,5,6].

Consideration of this background motivates the search for artificial water channels. In 2017, a report in Science claimed to have found the solution: extremely narrow carbon nanotubes [7]. Due to their thin walls and extremely high unitary water permeability, p_f, these single-walled tubes appeared to be an excellent solution. Yet, their reported p_f—surpassing the fastest aquaporins—came along with exceedingly high activation energy, E_A, for water flow across these tubes. The combination of these two observations constituted a problem [8]. The well-known Arrhenius equation predicts that E_A and p_f are correlated. E_A reflects the energetic barrier to water transport: if E_A is large, p_f is small, and vice versa (Figure 1A). An exception would only be possible if a gain in entropy compensated for the high expense in enthalpy. Yet, we found no significant entropy difference between intraluminal and bulk water molecules [9]. Accordingly, transition state theory allows calculating p_f from E_A and vice versa [8,9]. In conclusion, the carbon nanotube study could not provide sound experimental evidence that very narrow carbon nanotubes are superior to nature’s aquaporins.

In 2022, Science published another promising report: a team of Japanese researchers synthesized fluorous oligoamide nanorings, which were supposed to polymerize in phospholipid bilayer membranes to form nanochannels [10]. Given its 0.9-nm wide channel lumen, the artificial ^F12NC₄ channel showed an astonishing preference for water over ions. Moreover, with a reported p_f of 5.5 × 10⁻¹⁰ cm³ s⁻¹, water allegedly crossed this nanochannel at a 100 times higher rate than the fastest aquaporin (GlpF [11]).

Unfortunately, upon inspection, we realized that instead of miraculously efficient channels, flawed analysis of the underlying stopped-flow data yielded those high p_f values. The experimental data, which became available only months after the original publication and after repeated requests, do not support the claim regarding rapid ^F12NC₄-facilitated water transport, whereby ^F12NC₄ has the reportedly highest p_f among the supramolecular nanochannels presented by Itoh et al. [10]. This conclusion, drawn from our reassessment of the experimental data, comes as no surprise, because the extreme p_f falsely attributed to the 0.9-nm wide ^F12NC₄ channel would have violated the limits set by diffusion across narrow channels. Moreover, additional consideration of hydrodynamics outside the channel shows that the adjacent aqueous solution could not feed ^F12NC₄ with enough water molecules to allow for such rapid water conductance.

We approach the reassessment of the experimental data by first outlining the fundamental idea behind water flux measurements in vesicular systems using the stopped-flow technique, namely the monitoring of water flux across the vesicular membrane after introducing an osmotic gradient. As we will later discuss data from both stopped-flow light scattering and fluorescence self-quenching experiments, we introduce these approaches at reading out the change in intravesicular volume due to transmembrane water flux. Throughout, we note several critical requirements for accurate determinations of p_f. Eventually, in the Results section, we present our interpretation of the experimental data from both experimental approaches that Itoh et al. [10] intended to affirm the exceptional water-channeling properties of ^F12NC₄ and demonstrate where the authors went wrong. Finally, the Discussion is dedicated to a theoretical analysis of what water permeabilities narrow water channels may achieve, which concludes that aquaporins may not be surpassed by much.

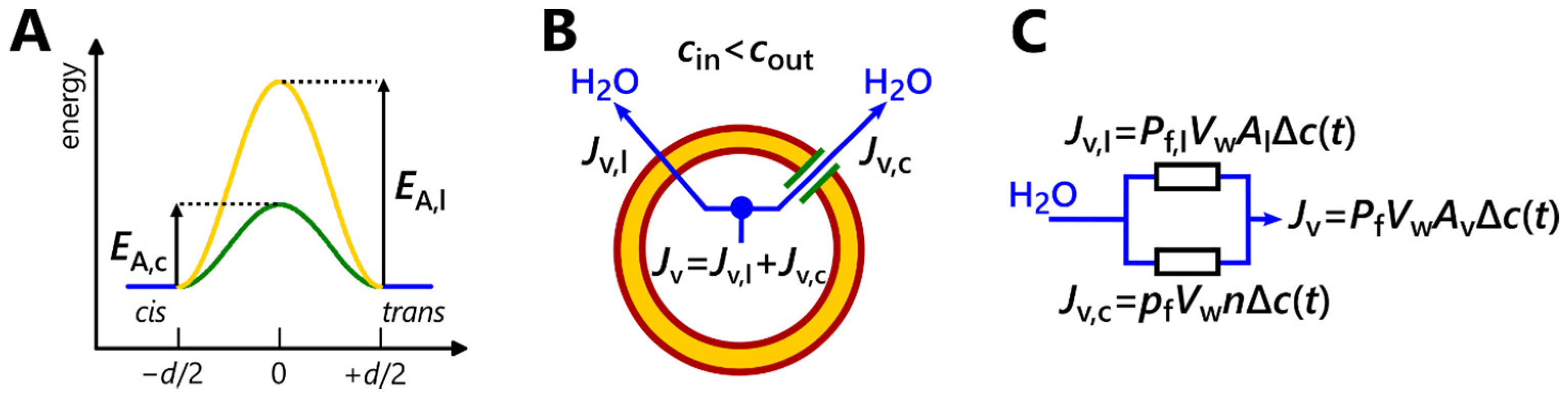
2. A Brief Tutorial on Water Flux Measurements Using Stopped-Flow (Materials and Methods)

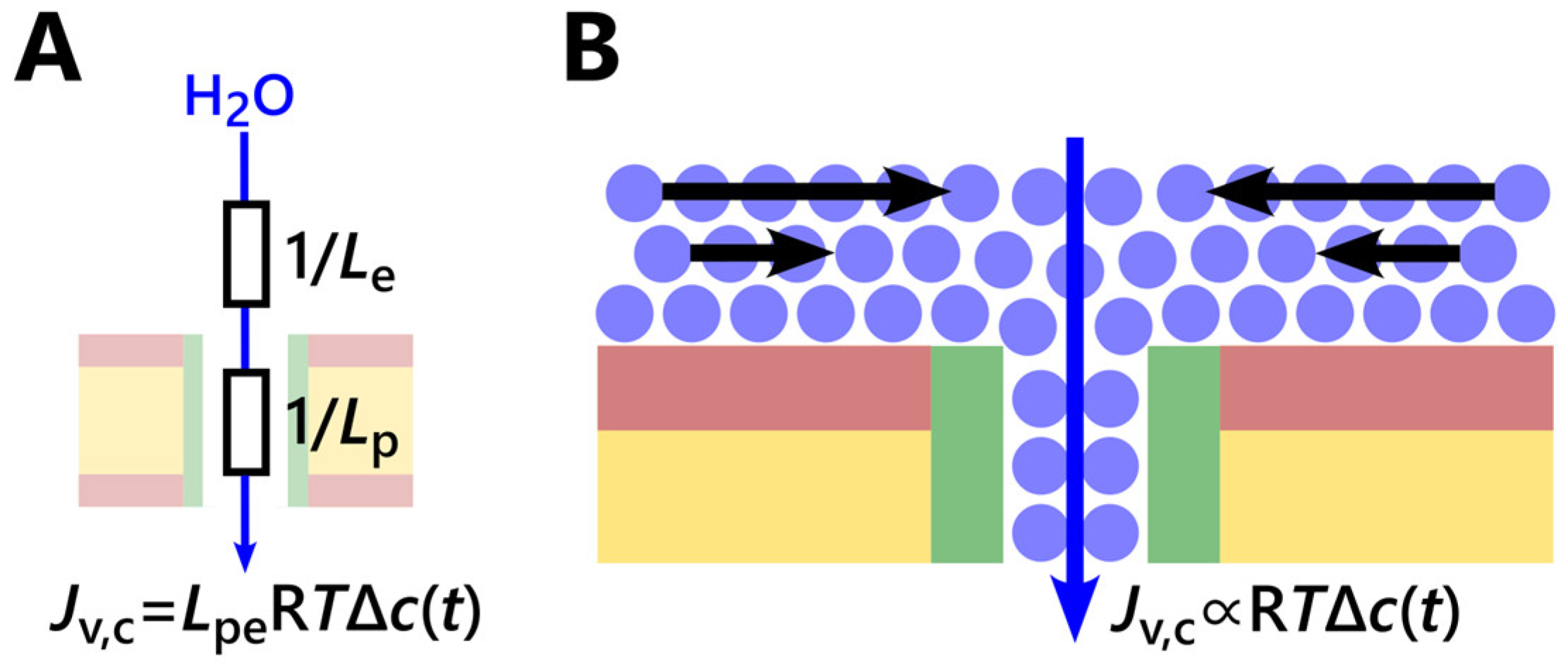
2.1. Vesicles in an Osmotic Gradient

Large unilamellar vesicles (LUVs) built from self-assembling amphiphilic lipids—herein simply referred to as vesicles—represent a convenient means for assessing unitary channel permeability, p_f. Under appropriate conditions, the liposomes behave like perfect osmometers [12,13]. They increase or decrease their volume under the influence of osmotic gradients. The changes in volume can be assessed in real-time by monitoring (i) the intensity of light scattered by the LUVs [14] or (ii) the concentration-dependent quenching of fluorophores encapsulated in the LUVs [15]. The stopped-flow technique ensures the rapid exchange of extravesicular osmolarity, which effectively synchronizes the individual vesicle shrinkage kinetics and allows for monitoring fast changes in volume (Figure 2).

The rate of change of intravesicular volume, dV(t)/dt, upon exposing a vesicle with initial intravesicular osmolyte concentration c₀ to hyperosmotic conditions reflects the overall volumetric flux of water, J_v (in units of cm³/s), across the vesicular membrane. Here, we are concerned with the case where the vesicular membrane, consisting of the lipid bilayer and any embedded water-conducting channels, is not permeable to the respective osmolyte in the time frame relevant for the stopped-flow experiment. Consequentially, the number of intravesicular osmolyte particles remains constant, yet their concentration depends on V(t). Upon denoting the incremental osmolyte concentration in the extravesicular volume as c_s, we can write for the osmotic gradient Δc(t):

$$\mathsf{\Delta }c\left(t\right)=\frac{V_0}{V\left(t\right)}{c}_0-\left(c_0+c_{\mathrm{s}}\right)$$

(1)

where V₀ is the intravesicular volume at time zero. J_v may comprise a relevant contribution from (a) the bare lipid bilayer, J_v,l, which, depending on the lipid composition, can be substantial, and (b) any membrane-embedded water-conducting channels, whereby we refer to the channel-awarded increment in volumetric flux as J_v,c. Experimentally, one obtains J_v and is subsequently tasked with determining J_v,l and J_v,c, whereby J_v = J_v,l + J_v,c.

J_v,l, the volumetric flux of water across the lipid bilayer, is given by the following expression:

$$J_{\mathrm{v},\mathrm{l}}=P_{\mathrm{f},\mathrm{l}}{V}_{\mathrm{w}}{A}_{\mathrm{l}}\mathsf{\Delta }c\left(t\right)$$

(2)

where P_f,l (in units of cm/s) is the lipid bilayer’s osmotic permeability coefficient, V_w the molar volume of water, and A_l the vesicular surface area occupied by the lipid bilayer. J_v,c, the total volumetric flux of water through all membrane-embedded water-conducting channels, is defined as follows [16]:

$$J_{\mathrm{v},\mathrm{c}}=p_{\mathrm{f}}{V}_{\mathrm{w}}n\mathsf{\Delta }c\left(t\right)$$

(3)

where p_f (in units of cm³/s) is the channel’s unitary water permeability coefficient. Since J_v,l and J_v,c add up, we can write:

$$J_{\mathrm{v}}=J_{\mathrm{v},\mathrm{l}}+J_{\mathrm{v},\mathrm{c}}=\left(P_{\mathrm{f},\mathrm{l}}{A}_{\mathrm{l}}+p_{\mathrm{f}}n\right)V_{\mathrm{w}}\mathsf{\Delta }c\left(t\right).$$

(4)

For practicality, we choose to define P_f,c, which reflects the contribution of membrane-embedded water-conducting channels to the experimentally observed vesicle shrinkage kinetics, as [16,17]:

$$P_{\mathrm{f},\mathrm{c}}=p_{\mathrm{f}}n/A_{\mathrm{v}}$$

(5)

While A_l may deviate from the vesicle’s surface area A_v in case a sizeable fraction of the membrane was occupied by channels, in the experiments we are concerned with here, this is not the case. Itoh et al. indicated n values well below 10 and an outer diameter between 5.2 nm and 6.3 nm for their fluorous nanochannels. Even if 10 of their channels were reconstituted into a 100-nm diameter vesicle, A_l would differ from A_v by <1%. This assumption of a negligible difference between A_v and A_l is commonly also made for vesicles containing reconstituted protein channels, since the external diameter of many water-conducting channels is in the range of a few nanometers, e.g., a typical aquaporin monomer measures roughly 2.5 nm, the respective tetramer somewhat more than twice that [18] and n seldomly exceeds 20. If it does, the background permeability of the lipid is much smaller than the combined water permeability of the channels so that J_v,l << J_v,c, i.e., J_v = J_v,c [19]. Thus, we approximate A_l with A_v, which is experimentally accessible, e.g., using dynamic light scattering.

Dividing both sides of Equation (4) by A_l = A_v yields:

$$J_{\mathrm{v}}/A_v=\left(P_{\mathrm{f},\mathrm{l}}+p_{\mathrm{f}}n/A_{\mathrm{v}}\right)V_{\mathrm{w}}\mathsf{\Delta }c\left(t\right)$$

(6)

Rearranging the above equation and inserting Equation (5) yields:

$$P_{\mathrm{f},\mathrm{l}}+P_{\mathrm{f},\mathrm{c}}=\frac{J_{\mathrm{v}}}{A_{\mathrm{v}}{V}_{\mathrm{w}}\mathsf{\Delta }c\left(t\right)}=P_{\mathrm{f}}$$

(7)

Upon substitution of Equations (1) and (5) into Equation (4), we can rewrite the latter as:

$$\frac{dV\left(t\right)}{dt}=A_{\mathrm{v}}{P}_{\mathrm{f}}{V}_{\mathrm{w}}\left(\frac{V_0}{V\left(t\right)}{c}_0^{}-\left(c_0^{}+c_{\mathrm{s}}\right)\right)$$

(8)

Equation (8) describes the time course of osmotically-induced vesicle volume changes. We want to highlight that the additivity of P_f,l and P_f,c is limited to cases where A_l ≈ A_v. Equation (8) has the analytical solution [14]:

$$V\left(t\right)=V_0\frac{c_0^{}}{c_0^{}+c_{\mathrm{s}}}\left\{1+L\left(\frac{c_{\mathrm{s}}}{c_0^{}}\exp \left(\frac{c_{\mathrm{s}}}{c_0^{}}-\frac{A_{\mathrm{v}}{P}_{\mathrm{f}}{V}_{\mathrm{w}}{\left(c_0^{}+c_{\mathrm{s}}\right)}^2}{V_0{c}_0^{}}t\right)\right)\right\}$$

(9)

where L is the Lambert function, defined as $L\left(x\right){\mathrm{e}}^{L\left(x\right)}=x$.

Itoh et al. [10] assumed a homogenous distribution of channels between vesicles. This assumption is reasonable since the hydrophobic nanorings which supposedly formed the supramolecular nanochannels were already added to the lipids prior to vesicle formation. Variable channel densities per vesicle are often found upon reconstitution of natural protein channels into lipid bilayers [20] (Figure 2).

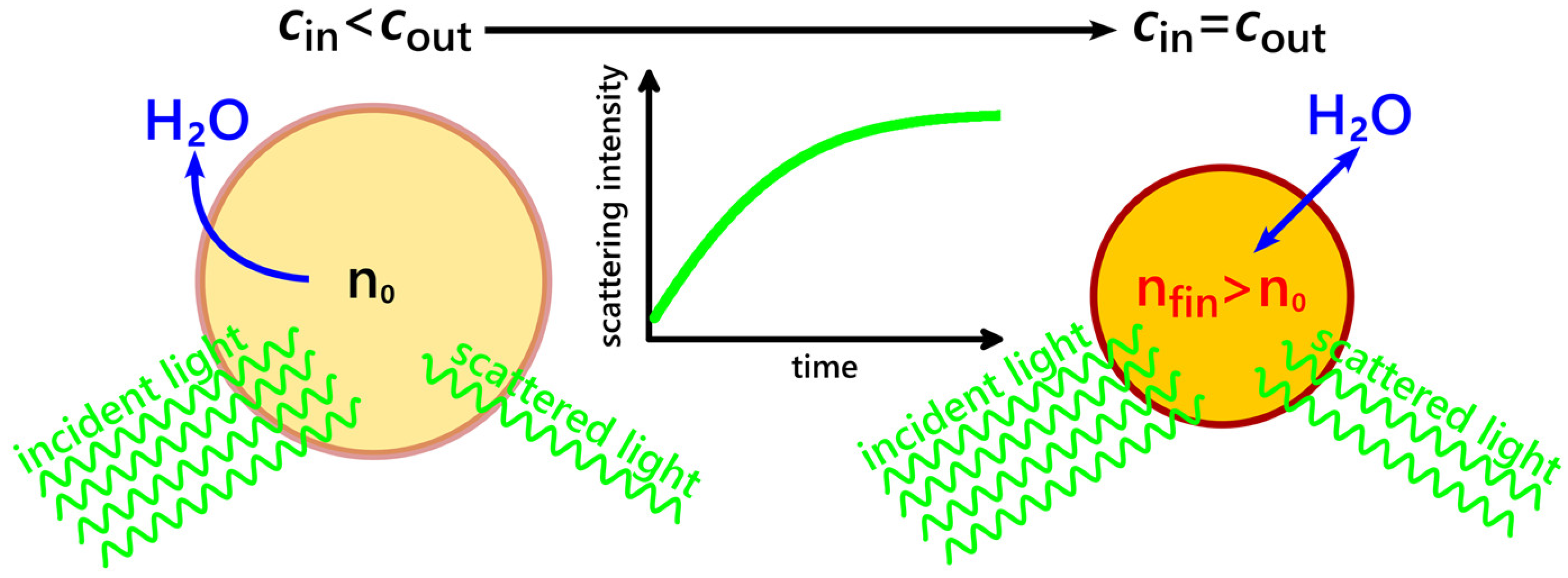
2.2. Assessing Vesicle Deflation Kinetics in a Liposome Suspension by Measuring the Intensity of Scattered Light

V(t) is experimentally accessible by measuring the intensity of light scattered by the vesicles I(t) (Figure 3). The Rayleigh–Gans–Debye relation describes the dependence of I(t) on vesicle radius R [21]:

$$I\sim \left(\frac{{\lambda }^2}{4{\pi }^2}\right){\left(\frac{m^2-1}{m^2+2}\right)}^2{\delta }^6\left(\frac{1+{\cos }^2\theta }{2}\right)P\left(\theta \right)$$

(10)

where λ, θ, and m are the effective wavelength (i.e., the ratio of the wavelength of incident light λ₀ and the refractive index n_s of the aqueous solution), the angle at which the intensity I of scattered light is measured, and the relative refractive index (m = n_p/n_s, where n_p is the average refractive index of the particle). R enters the equation via the size parameter $\delta =2\pi R/\lambda$ and the form factor $P\left(\theta \right)={(3\left(\sin u-u\cos u\right)/u^3)}^2$, where $u=2\delta \sin \left(\theta /2\right)$.

Using the explicit expression for I is rather tedious [14]. Indeed, its Taylor expansion provides a sufficient level of accuracy:

$$I\left(t\right)=a+bV\left(t\right)+d{V}^2\left(t\right)$$

(11)

Although it is possible to derive the coefficients b and d from the solution of Equation (10), they are commonly found by fitting a second-degree polynomial (Equation (11)) to I(t) [14].

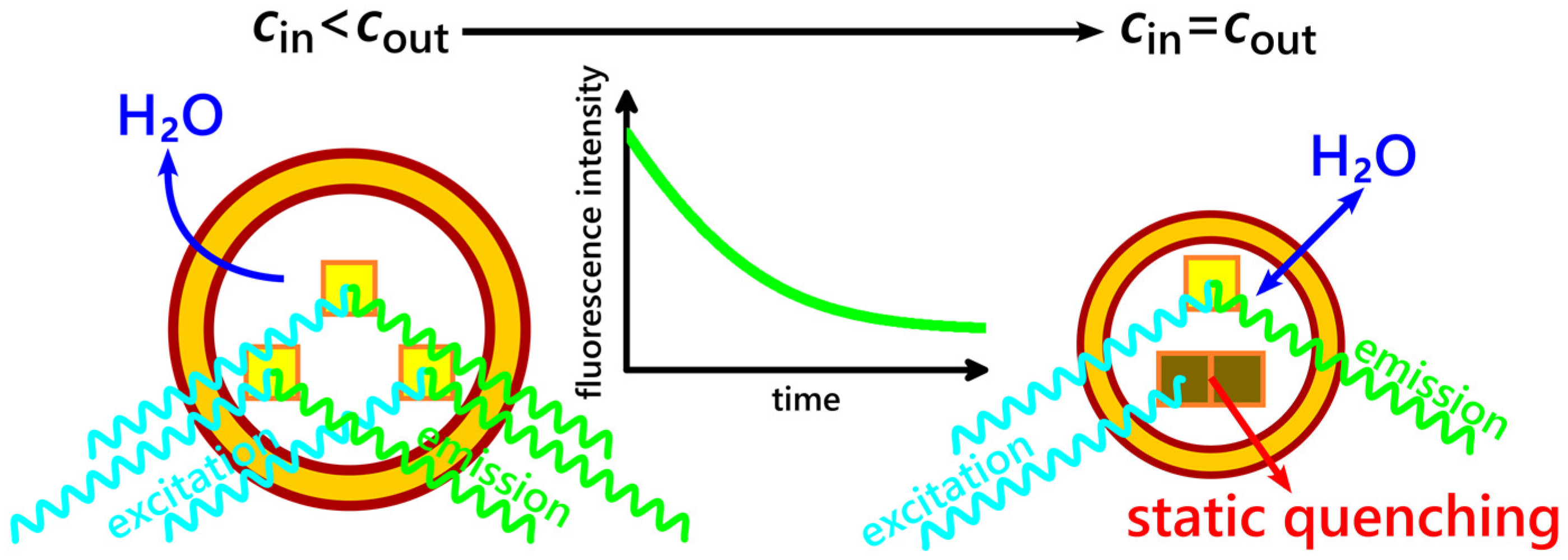
2.3. Evaluating Vesicle Deflation Kinetics in a Liposome Suspension by Measuring the Fluorescence Intensity of Encapsulated Aqueous Dyes

Self-quenching of aqueous dyes is distance-, and thus, concentration-dependent. This observation can be used to follow the upconcentration of a dye during vesicle deflation (Figure 4). Since vesicles behave like perfect osmometers, a linear dependence between fluorescent light intensity, I_f(t), and vesicle volume is attainable. This requires a sufficiently high fluorophore concentration. For the frequently used carboxyfluorescein, the initial intravesicular concentration should be above 10 mM [13,22].

3. Results

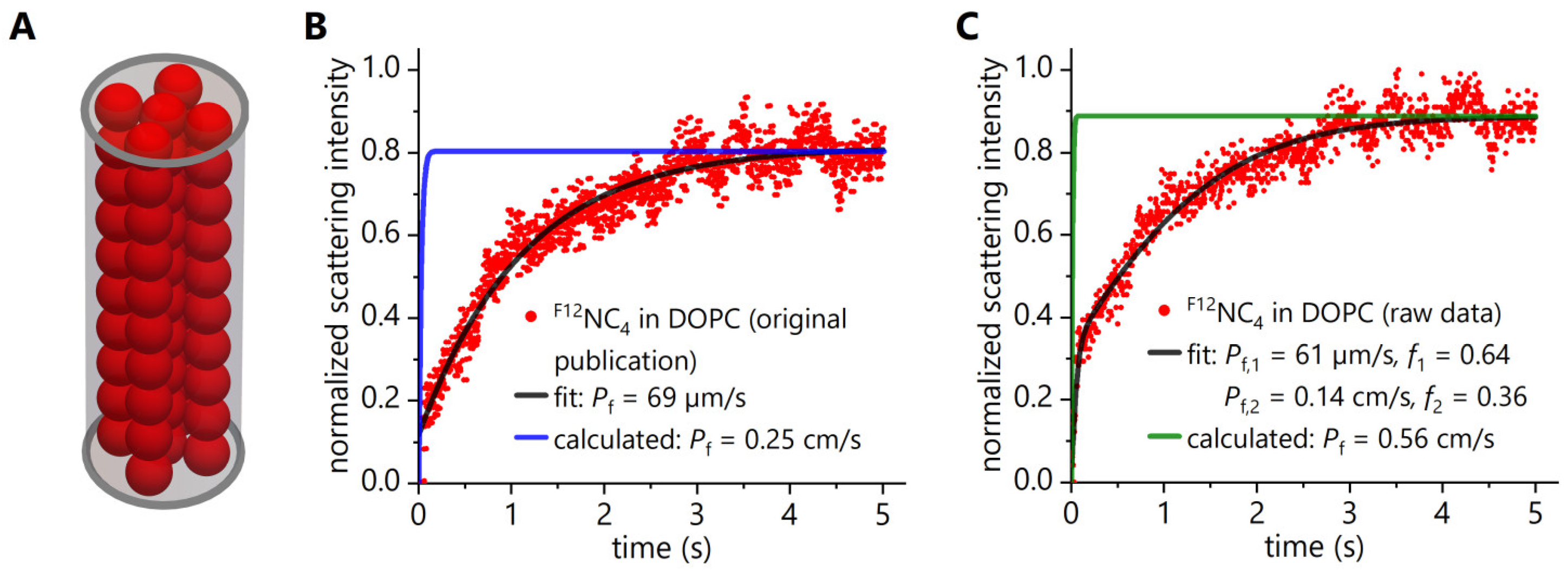
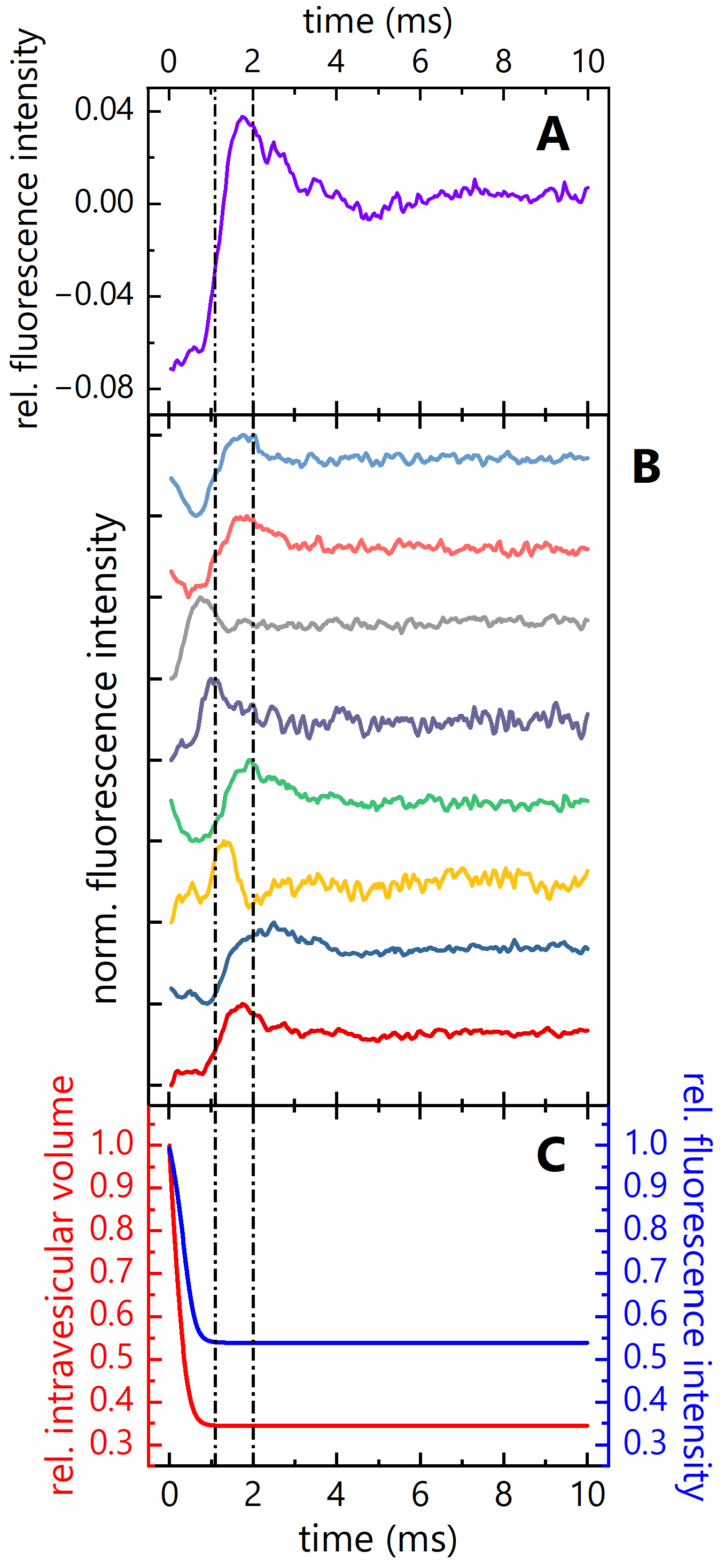
3.1. Reassessment of Experimental Data Obtained in Stopped-Flow Light Scattering Experiments with ^F12NC₄

We focused our data evaluation on the narrowest described channel, ^F12NC₄, built from ^F12NR₄ rings [10]. Since the original data became available only months after the original paper appeared, we digitized the published stopped-flow light scattering curve (Figure 5B, red points) and used Equation (9) to infer P_f. After the raw data had become publicly available, we repeated the same fitting procedure. To our surprise, the published graph and the raw data do not match (Figure 5B,C, red points).

For the published plot of ^F12NC₄ channels in DOPC (dioleoyl phosphatidylcholine) vesicles, we find P_f = 69 µm/s (Figure 5B, black line). This value corresponds to the permeability of a bare DOPC lipid bilayer [23,24], and hence, P_f = P_f,l. It follows that P_f,c = 0 (or P_f,c << P_f,l), which agrees with the observation that the time course of scattered light intensity shown in ref. [10] for bare DOPC liposomes is practically identical to that in the presence of ^F12NC₄. If P_f,c was equal to 0.25 cm/s, as claimed in [10], we would expect the measured scattering trace (Figure 5B, red points) to resemble the blue trace in Figure 5B—yet, there is no resemblance.

The analysis of the raw data (https://doi.org/10.5061/dryad.h18931zq1) yields a different picture (Figure 5C, red points): two apparently different deflation kinetics indicate sample inhomogeneity, i.e., the presence of two distinct vesicle populations. Accordingly, we adopted the fitting algorithm described in the caption to Figure 5. We can assign the major fraction f₁ = 64% to channel-free DOPC vesicles since P_f,1 = 61 µm/s (Figure 5C). The origin of the minor fraction (f₂ = 36%) with fast kinetic is unclear. It may originate from vesicle lysis upon osmotic challenge, aggregates in the sample, or other shortages in sample preparation or device application. In any case, ^F12NC₄ channel activity cannot be involved. In disregard of the apparent inability of an individual vesicle to simultaneously shrink with two distinct time constants, Itoh et al. proceeded to calculate P_f,c and P_f,l from the two time constants extracted by a two-exponent fit. Figure 2D illustrates under which conditions such kinetics may be observable, given the assumption of a homogeneous channel-containing vesicle ensemble: all channels must have been concertedly blocked shortly after the onset of the osmotic challenge, so that water may pass afterward solely through the lipid bilayer, utterly undisturbed by any channel activity.

A more realistic scenario that could explain the experimental data is illustrated in Figure 2C. It specifies that only a fraction of vesicles may contain ^F12NC₄ channels. Since channel-containing and empty liposomes deflate at different rates, the scattering trace obtained from the inhomogeneous vesicle ensemble will exhibit two distinct kinetics. Unfortunately, the experimental data presented by Itoh et al. [10] render the applicability of this scenario in their experiments unwarranted. It would require (a) independent proof that only ≈1/3 of the vesicles contain the functionally-assembled channel and/or (b) deflation experiments where the functional channel is present in most vesicles. Yet, Itoh et al. indicated that on average, about 1.9 channels should be active per vesicle for the experiment shown in Figure 5. We calculated that number from the mentioned % mol fraction of 0.0053 ^F12NR₄, the number of 3.5 × 10⁵ lipids per vesicle, and the involvement of 10 ^F12NR₄ molecules per channel. We computed the number of lipids from the vesicle diameter of 200 nm, a lipid area of 0.72 nm² [25], and the presence of 2 leaflets per vesicle. The data leave no room for empty vesicles, thereby ruling out (a). Furthermore, Itoh et al. provided experimental data in which they gradually increased the concentration of ^F12NR₄. Yet, the fraction with fast kinetic, f₂, did not increase, rendering option (b) obsolete as well (Figure 6).

3.2. Reassessment of Experimental Data Obtained in Stopped-Flow Fluorescence Self-Quenching Experiments with ^F12NC₄

Eventually, Itoh et al. [10] postulated that DOPC is poorly suited for studying water permeation through their nanochannels due to “spontaneous water leakage through the fluidic vesicular membrane”. The argument is incorrect because the extreme p_f values reported for their nanochannels should render P_f,l of DOPC negligible (Section 3.1). However, Itoh et al. felt that p_f of their supramolecular channels is better assessable in DPPC (dipalmitoyl phosphatidylcholine) liposomes since P_f,l of DPPC in its gel phase is nearly two orders of magnitude lower than DOPC’s P_f,l in the fluid phase [26].

With radii as small as ~24 nm, as read from the published raw data (https://doi.org/10.5061/dryad.h18931zq1), the DPPC vesicles were much smaller than the DOPC vesicles. From the % mol fraction of 0.0018 indicated for ^F12NR₄ (Figure 7), we find 0.54 ^F12NR₄ molecules per vesicle. Channel formation appears very unlikely, since (i) ^F12NR₄ distribution between the vesicles should be homogenous and (ii) ten ^F12NR₄ molecules would be required per channel. We base our conclusion on the finding of only 3 × 10⁴ lipids per vesicle, as computed from the lipid area of 0.479 nm² [27] and the presence of 2 leaflets per vesicle.

Despite these problematic settings, Itoh et al. [10] reported channel activity in DPPC vesicles. Instead of measuring light scattering, they now assessed V(t) via the fluorescence intensity of encapsulated carboxyfluorescein (Figure 7). Within 10 ms, as reported in their Science paper, bare DPPC vesicles do not shrink. Only channel-containing vesicles may respond to the osmotic challenge. The “representative” curve in the Science paper (reproduced in Figure 7) may falsely suggest that such vesicles exist, despite the above estimate of only 0.054 channels per vesicle. However, a glimpse at how the curve was obtained leads to a different conclusion.

In contrast to the exemplary curve in the Science paper, which displays the fluorescence intensity in the interval between 2 and 10 ms, Figure 7A shows the same trace starting from 0 ms. Fluorescence intensity increases in the interval between 0 and 2 ms—in contrast to the expected fluorescence decrease due to self-quenching upon vesicle shrinkage. As an explanation, Itoh et al. [10] offered that they used a “monochromator with a measured dead time of 1.1 ms”. We do not know why a monochromator should have a dead time. Yet, even if we attributed this dead time to sample mixing, we would still be left with a 0.9 ms interval of unexplained fluorescence increase. Since Figure 7A averages several stopped flow shots, we hoped to find an explanation by looking at the individual curves (Figure 7B). Only one out of eight traces exhibits a fluorescence decrease starting with the end of the dead time. The seemingly stochastic pattern of these traces raises the question of whether averaging is warranted.

To illustrate how fluorescence intensity should have changed given the reported experimental parameters, we calculated the expected volume change from the published value for P_f,c and used the result to compute the corresponding relative fluorescence intensity (Figure 7C). Thus, we found that the vesicles should have been largely deflated within the 1.1 ms dead time of the stopped-flow device and even more so within the first 2 ms. Thus, the very design of the stopped-flow self-quenching experiment does not allow for concluding that ^F12NC₄ facilitates water transport at the reported rate.

4. Discussion

The ultrafast water channels surpassing aquaporins’ p_f by a factor of 100 while retaining excellent selectivity do not exist. The study of Itoh et al. [10] did not provide compulsory experimental evidence. It relied on ill-designed stopped-flow approaches. The DPPC experiments were flawed for at least two reasons: (i) the vesicles contained only trace amounts of ^F12NC₄—insufficient to form channels—and (ii) the small vesicles should have been deflated within the dead (mixing) time of the device if they possessed the alleged water permeability. The DOPC experiments were faulty since Itoh et al. erroneously assumed that the same vesicle could shrink with two different kinetics at once (compare Section 4.1 below). Moreover, Itoh et al. did not reflect on the boundaries physical laws put on water transport through channels. We fill the void by providing theoretical estimates for unitary channel permeabilities based on the diffusional mobility of intraluminal water molecules and calculations of channel access resistance (compare Section 4.2 below).

4.1. Criticism of the Stopped-Flow Water Flux Measurements with Fluorous Nanochannels

Itoh et al. [10] reported different scattering traces for the experiments with ^F12NC₄ in DOPC vesicles (Figure 5B,C)—yet, neither of them supports fast channel-mediated water transport. The originally published trace does not show any fast deflation kinetics (Figure 5B), indicating sample homogeneity. Accordingly, a single permeability coefficient fits the trace well, whereby P_f = P_f,l, and thus, P_f,c = 0. The raw data, distributed several months later, do show both a fast and a slow kinetic (Figure 5C). The genesis of a channel-free vesicle population is unclear, as the provided data indicate an average of 1.9 channels per vesicle (see above) and contain no evidence for an inhomogeneous channel distribution.

However, in the case of 1.9 channels per vesicle, the slow component, which represents the major fraction (Figure 5), should not be observable because the allegedly gigantic channel permeability should have dwarfed the volume flow through the lipid matrix, rendering it negligibly small. Contrary to the approach of Itoh et al. [10], the unitary channel permeability to water and background bilayer permeability cannot both be found from the deflation kinetics of a vesicle ensemble in which each liposome contains superfast water channels (Figure 2) since P_f = P_f,l + P_f,c (Equation (7)) and only P_f is accessible experimentally. Benevolently, we could assume that the sample was inhomogeneous, allowing for the assignment of the fast component in Figure 5C to water transport facilitated by channels. Yet, this assumption is not warranted as (i) there is no evidence for an inhomogeneous vesicle ensemble and (ii) an increase in nanoring concentration, from which the channels are supposedly built, does not lead to an increase of the fraction of the fast component (Figure 7). Notably, the determination of the fraction of vesicles containing no channel, as well as the number of channels per vesicle in the other fraction, represents a critical challenge in accurate determinations of p_f and is typically completed in separate experiments [15]. Assumptions on both aforementioned parameters based on the fraction of added compound can be misleading. Finally, it may be added that for calculating water permeability from the time course of osmotically induced volume changes, Itoh et al. used an exponential equation that does not represent a solution of Equation (8).

On the other hand, the experiments with DPPC liposomes were ill-designed. The deflation of channel-containing DPPC vesicles should have occurred within the dead time of the stopped-flow instrument if the channels possessed the alleged water permeability. Inspection of the individual raw fluorescence traces reveals their stochastic behavior and raises the question of what physical process they might reflect—we are confident that it is not rapid water conduction through fluorous nanochannels.

4.2. Estimation of the Permeability Limit for Narrow Water-Conducting Channels

Itoh et al. [10] are not the first who claim to have found and successfully measured artificial nanochannels that surpass aquaporins. Narrow single-walled carbon nanotubes are another prominent example [7]. We refuted the claim based on the high activation energy measured for water transport across these tubes [8]. Given the repeated efforts to out-compete millions of years of evolution, it seems warranted to ask whether the water-channeling performance of aquaporins represents the theoretical limit to selective water channels—that is, water channels that are so narrow that hydrated ions cannot penetrate them.

We start by computing water flow, J_v, across a membrane channel from the transmembrane pressure difference:

$$J_{\mathrm{v}}=L_{\mathrm{p}}\left(\mathsf{\Delta }P-\mathsf{\Delta }\Pi \right)$$

(12)

where L_p is the hydraulic permeability coefficient, ΔP the hydraulic pressure difference, and ΔΠ the osmotic pressure difference across the membrane. We can set ΔP = 0 if the experiments are carried out in a liposome suspension, where the channels are located at the vesicular membrane.

At macroscopic scales, Hagen–Poiseuille’s formula successfully relates tube radius, r, and channel length, L, to L_p, given that r << L:

$$L_{\mathrm{p}}=\frac{\pi r^4}{8\eta L}$$

(13)

where η is water viscosity. It is difficult to imagine that Equation (13) works for very narrow channels—be it only because “one shudders to think what a parabolic velocity profile in a single-file pore could mean” [28]. The consensus is that the validity of a continuum description by conventional hydrodynamics (Navier–Stokes equation) breaks down at a length scale of 1 nm. A simple theoretical reasoning is that 1 nm represents the lower bound for defining fluid viscosity, η [29]. Experimental support comes, for example, from the breakdown of Poiseuille flow upon confinement of water between graphene oxide sheets spaced < 0.9 nm apart [30]. There is consensus that water transport through narrow channels with a diameter of less than 1 nm is diffusional [28,31,32]. Deviations from Equation (13) observed at the nanoscale are frequently accounted for by abandoning the no-slip condition. Upon introducing a nonzero slip length b [33] as r approaches tens of nanometer [34], L_p becomes [29]:

$$L_{\mathrm{p}}=\frac{\pi r^4}{8\eta L}\left(1+\frac{4b}{r}\right)$$

(14)

Yet, abandoning the continuum descriptions is not warranted as they are applicable outside the confined space of membrane channels, i.e., they provide information about the access resistance that may limit “ultrafast” water flow. The term access resistance is well known from the study of ion channels, where it signifies diffusional limitations in ion transport to the channel or away from it and the associated concentration changes [35]. The term acquires a new meaning for water channels, as it is not associated with alterations in water concentration close to the channel but may only be realized in terms of limited water mobility. Since b can easily reach several hundred nm [34], L_p could drastically increase if flux was not limited by the access resistance 1/L_e operating in series with the pore resistance 1/L_p (Figure 8).

L_e can be calculated using Sampson’s equation. It is valid for a nanopore of radius r and vanishing length [36]:

$$L_{\mathrm{e}}=\frac{r^3}{3\eta }$$

(15)

where L_e is the hydraulic entrance permeability coefficient. J_v must be the same through the entrance region and through the channel itself. Thus, we should add the hydrodynamic resistances of the entrance region and the channel itself (Equations (14) and (15)). In the limit of b >> r, the entrance-corrected hydraulic permeability becomes [29]:

$$L_{\mathrm{pe}}=\frac{r^3}{3\eta }\frac{1}{1+\frac{2L}{\begin{array}{c}3\pi b\end{array}}}$$

(16)

Considering that b >> L yields:

$$L_{\mathrm{pe}}=L_{\mathrm{e}}=\frac{r^3}{3\eta }$$

(17)

That is, the entrance resistance governs channel permeability [36]. Transforming L_pe (Equation (17)) into p_f yields:

$$p_{\mathrm{f}}=\frac{RT r^3}{3\eta V_{\mathrm{w}}}$$

(18)

Equation (18) only sets the upper p_f limit for a cylindrical channel that is as long as the membrane is thick. It specifies the maximum amount of water that can reach the inside of the channel. Besides defining an upper limit, Equation (18) does not allow predictions of the actual amount of water that may pass through such a channel.

Applying Equation (18), we find p_f = 1.5 × 10⁻¹³ cm³ s⁻¹ for a typical aquaporin with r = 0.15 nm [36]. Entrance effects, e.g., an hourglass shape of the vestibule, may augment p_f [36], so that the theoretical value comes close to the experimentally measured p_f for GlpF of 1.2 × 10⁻¹² cm³ s⁻¹ [14]. For the artificial nanotube ^F12NC₄ of Itoh et al. with r = 0.45 nm, we find p_f = 4.2 × 10⁻¹² cm³ s⁻¹. Thus, the theoretical limit falls short of the reported value of 5.5 × 10⁻¹⁰ cm³ s⁻¹ by a factor of 100.

A second approach at estimating the upper p_f limit, p_limit, of water movement through the 0.9-nm wide ^F12NC₄ nanochannel is based on assuming a superposition of N_f = 5 water files moving through the pore independently [37] (Figure 5A). This view suggests perfect slip at the channel wall [15,38] and between the water columns. p_f,file of every water file is intricately linked to the self-diffusion coefficient of liquid water, D_w. To ascertain the upper limit, we assume water retains bulk mobility, i.e., D_w = 2.57 × 10⁻⁵ cm² s⁻¹ [15,32,39]:

$$D_{\mathrm{w}}=\frac{p_{\mathrm{f},\mathrm{file}}}{v_{\mathrm{w}}}{z}^2$$

(19)

where v_w = 3 × 10⁻²³ cm³ is the volume of one water molecule and z = 0.275 nm is the single-file step distance. A thorough analysis of water transport through numerous biological single-file channels showed that intraluminal diffusivity only approaches but never significantly exceeds its bulk value [9]. The observation also holds in vitro [8] and in silico [40] for single-file carbon nanotubes whose hydrophobic pore walls do not form hydrogen bonds with permeating water molecules. Hence, we may write for p_limit:

$$p_{\mathrm{limit}}=N_{\mathrm{f}}\frac{D_{\mathrm{w}}{v}_{\mathrm{w}}}{z^2}=5.1\times {10}^{-12} {\mathrm{cm}}^3{\mathrm{s}}^{-1}$$

(20)

The value of p_limit is surprisingly close to the prediction, p_f = 4.2 × 10⁻¹² cm³ s⁻¹, made on the basis of Equation (18). Thus, both approaches agree on the fact that the reported p_f of 5.5 × 10⁻¹⁰ cm³ s⁻¹ for ^F12NC₄ cannot be valid.

5. Conclusions

Our re-evaluation of the underlying experimental stopped-flow water flux data in [10] demonstrates the failure of ^F12NC₄ to facilitate fast water transport. Both the experimental design and data analysis of the original report are flawed. Our analysis of the achievable water permeability limits in narrow channels indicates that aquaporins cannot be significantly out-competed. Limitations in feeding water to the channel and diffusion within the channel both hamper higher permeabilities of selective water channels, i.e., channels that are sufficiently narrow to prevent the flow of hydrated ions.