Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact
Abstract
:1. Introduction
2. Theoretical Approach
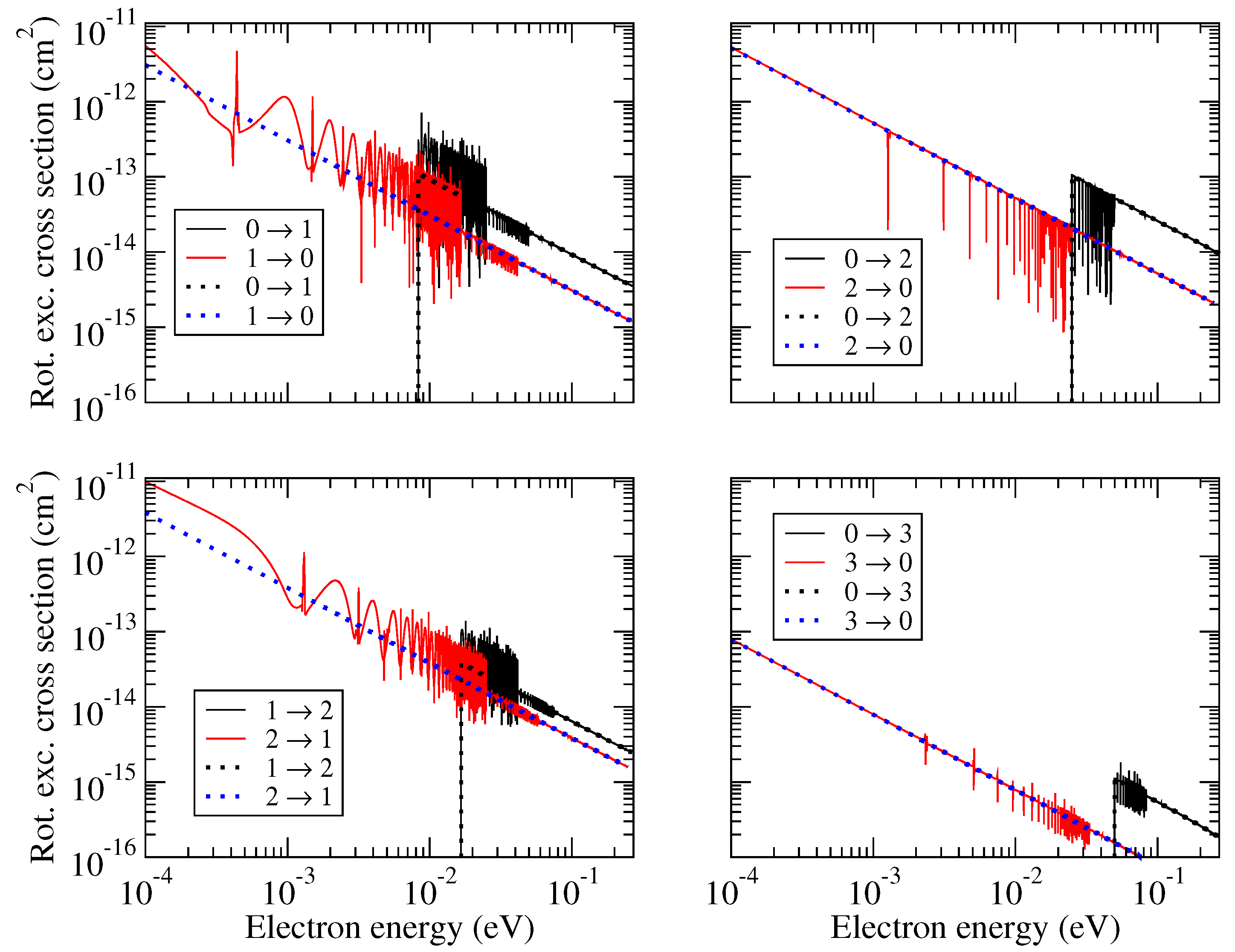
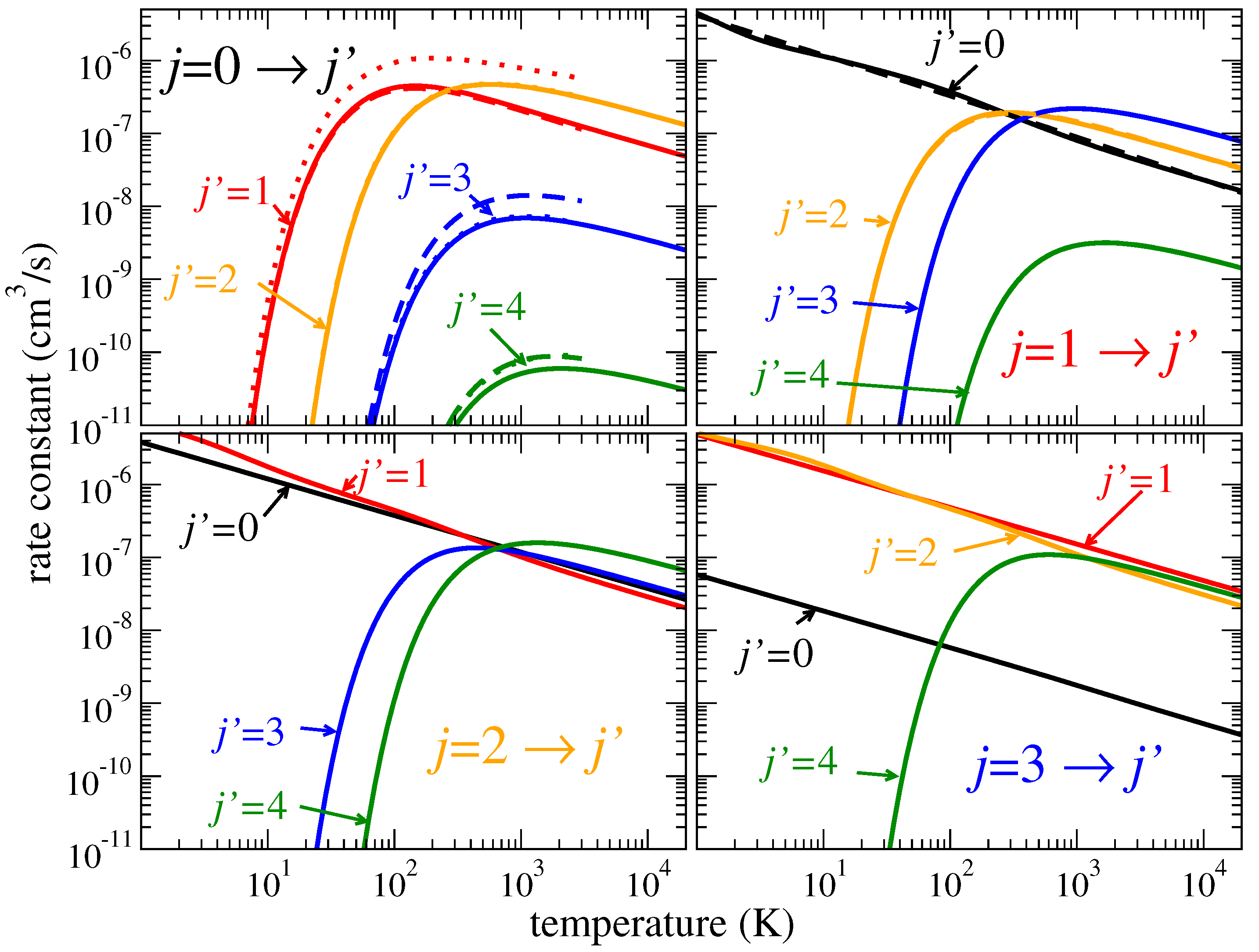
3. Cross Sections and Rate Coefficients
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Boikova, R.F.; Ob’edkov, V.D. Rotational and vibrational excitation of molecular ions by electrons. Sov. Phys. JETP 1968, 27, 772. [Google Scholar]
- Chu, S.I.; Dalgarno, A. Rotational excitation of CH+ by electron impact. Phys. Rev. A 1974, 10, 788. [Google Scholar] [CrossRef]
- Flower, D. Electron collisional excitation of rotational transitions in CH+ and HeH+. Astron. Astrophys. 1979, 73, 237–239. [Google Scholar]
- Rabadán, I.; Sarpal, B.K.; Tennyson, J. On the calculation of electron-impact rotational excitation cross sections for molecular ions. J. Phys. B At. Mol. Opt. Phys. 1998, 31, 2077. [Google Scholar] [CrossRef]
- Rabadán, I.; Sarpal, B.K.; Tennyson, J. Calculated rotational and vibrational excitation rates for electron-HeH+ collisions. Mon. Not. R. Astron. Soc. 1998, 299, 171–175. [Google Scholar] [CrossRef]
- Rabadán, I.; Tennyson, J. ROTIONS: A program for the calculation of rotational excitation cross sections in electron—Molecular ion collisions. Comput. Phys. Commun. 1998, 114, 129–141. [Google Scholar] [CrossRef]
- Tennyson, J. Electron—Molecule collision calculations using the R-matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- Faure, A.; Kokoouline, V.; Greene, C.H.; Tennyson, J. Near-threshold rotational excitation of molecular ions by electron impact. J. Phys. B At. Mol. Opt. Phys. 2006, 39, 4261. [Google Scholar] [CrossRef]
- Faure, A.; Tennyson, J.; Kokoouline, V.; Greene, C.H. Rotational excitation of interstellar molecular ions by electrons. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2009; Volume 192. [Google Scholar]
- Hamilton, J.R.; Faure, A.; Tennyson, J. Electron-impact excitation of diatomic hydride cations—I. HeH+, CH+, ArH+. Mon. Not. R. Astron. Soc. 2016, 455, 3281–3287. [Google Scholar] [CrossRef]
- Chang, E.S.; Fano, U. Theory of electron-molecule collisions by frame transformations. Phys. Rev. A 1972, 6, 173. [Google Scholar] [CrossRef]
- Greene, C.H.; Jungen, C. Molecular applications ofquantum defect theory. Adv. At. Mol. Phys. 1985, 21, 51–121. [Google Scholar]
- Jungen, C. Molecular Applications of Quantum Defect Theory; Institute of Physics Publishing: Bristol, UK, 1996. [Google Scholar]
- Motapon, O.; Pop, N.; Argoubi, F.; Mezei, J.Z.; Epée Epée, M.D.; Faure, A.; Telmini, M.; Tennyson, J.; Schneider, I.F. Rotational transitions induced by collisions of HD+ ions with low-energy electrons. Phys. Rev. A 2014, 90, 012706. [Google Scholar] [CrossRef]
- Epée Epée, M.D.; Mezei, J.Z.; Motapon, O.; Pop, N.; Schneider, I.F. Reactive collisions of very low-energy electrons with rotational transitions and dissociative recombination. Mon. Not. R. Astron. Soc. 2015, 455, 276–281. [Google Scholar] [CrossRef]
- Kokoouline, V.; Faure, A.; Tennyson, J.; Greene, C.H. Calculation of rate constants for vibrational and rotational excitation of the ion by electron impact. Mon. Not. R. Astron. Soc. 2010, 405, 1195–1202. [Google Scholar]
- Čurík, R.; Greene, C.H. Inelastic low-energy collisions of electrons with HeH+: Rovibrational excitation and dissociative recombination. J. Chem. Phys. 2017, 147, 054307. [Google Scholar] [CrossRef] [PubMed]
- Faure, A.; Halvick, P.; Stoecklin, T.; Honvault, P.; Epée Epée, M.; Mezei, J.; Motapon, O.; Schneider, I.; Tennyson, J.; Roncero, O.; et al. State-to-state chemistry and rotational excitation of CH+ in photon-dominated regions. Mon. Not. R. Astron. Soc. 2017, 469, 612–620. [Google Scholar] [CrossRef] [PubMed]
- Tanabe, T.; Katayama, I.; Ono, S.; Chida, K.; Watanabe, T.; Arakaki, Y.; Haruyama, Y.; Saito, M.; Odagiri, T.; Hosono, K.; et al. Dissociative recombination of HeH+ isotopes with an ultra-cold electron beam from a superconducting electron cooler in a storage ring. J. Phys. B At. Mol. Opt. Phys. 1998, 31, L297. [Google Scholar] [CrossRef]
- Takagi, H. Theoretical study of the dissociative recombination of HeH+. Phys. Rev. A 2004, 70, 022709. [Google Scholar] [CrossRef]
- Takagi, H. Cross sections of the processes induced by electron collisions with , HeH+, and their isotopes. Fusion Sci. Technol. 2013, 63, 406–412. [Google Scholar] [CrossRef]
- Takagi, H.; Tashiro, M. Study on the dissociative recombination of HeH+ by multi-channel quantum defect theory. In EPJ Web of Conferences; EDP Sciences: Les Ulis, France, 2015; Volume 84. [Google Scholar]
- Dabrowski, I.; Herzberg, G. The predicted infrared spectrum of HeH+ and its possible astrophysical importance. Trans. N. Y. Acad. Sci. 1977, 38, 14–25. [Google Scholar] [CrossRef]
- Black, J. Molecules in planetary nebulae. Astrophys. J. 1978, 222, 125–131. [Google Scholar] [CrossRef]
- Flower, D.; Roueff, E. On the formation and destruction of HeH+ in gaseous nebulae and the associated infra-red emission line spectrum. Astron. Astrophys. 1979, 72, 361–366. [Google Scholar]
- Roberge, W.; Dalgarno, A. The formation and destruction of HeH+ in astrophysical plasmas. Astrophys. J. 1982, 255, 489–496. [Google Scholar] [CrossRef]
- Harris, G.; Lynas-Gray, A.; Miller, S.; Tennyson, J. The role of HeH+ in cool helium-rich white dwarfs. Astrophys. J. Lett. 2004, 617, L143. [Google Scholar] [CrossRef]
- Guberman, S.L. Dissociative recombination without a curve crossing. Phys. Rev. A 1994, 49, R4277. [Google Scholar] [CrossRef] [PubMed]
- Tennyson, J.; Brown, D.B.; Munro, J.J.; Rozum, I.; Varambhia, H.N.; Vinci, N. Quantemol-N: An expert system for performing electron molecule collision calculations using the R-matrix method. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2007; Volume 86. [Google Scholar]
- Douguet, N.; Fonseca dos Santos, S.; Raoult, M.; Dulieu, O.; Orel, A.E.; Kokoouline, V. Theory of radiative electron attachment to molecules: Benchmark study of CN−. Phys. Rev. A 2013, 88, 052710. [Google Scholar] [CrossRef]
- Seaton, M.J. Quantum defect theory. Rep. Prog. Phys. 1983, 46, 167. [Google Scholar] [CrossRef]
- Aymar, M.; Greene, C.H.; Luc-Koenig, E. Multichannel Rydberg spectroscopy of complex atoms. Rev. Mod. Phys. 1996, 68, 1015. [Google Scholar] [CrossRef]
- Ayouz, M.; Kokoouline, V. Cross Sections and Rate Coefficients for Vibrational Excitation of HeH+ Molecule by Electron Impactukrm. Atoms 2016, 4, 30. [Google Scholar] [CrossRef]
- Friedrich, H. Scattering Theory; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]

| (K) | ||||
|---|---|---|---|---|
| 96 | ||||
| 289 | ||||
| 578 | ||||
| 964 | ||||
| 192 | ||||
| 482 | 0.38 | |||
| 868 | 0.75 | 0.35 | −0.98 | |
| 289 | 0.57 | −0.19 | −0.10 | |
| 675 | 0.53 | 0.61 | −0.39 | |
| 385 | 0.32 | 0.56 | −0.61 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khamesian, M.; Ayouz, M.; Singh, J.; Kokoouline, V. Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact. Atoms 2018, 6, 49. https://doi.org/10.3390/atoms6030049
Khamesian M, Ayouz M, Singh J, Kokoouline V. Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact. Atoms. 2018; 6(3):49. https://doi.org/10.3390/atoms6030049
Chicago/Turabian StyleKhamesian, Marjan, Mehdi Ayouz, Jasmeet Singh, and Viatcheslav Kokoouline. 2018. "Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact" Atoms 6, no. 3: 49. https://doi.org/10.3390/atoms6030049
APA StyleKhamesian, M., Ayouz, M., Singh, J., & Kokoouline, V. (2018). Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact. Atoms, 6(3), 49. https://doi.org/10.3390/atoms6030049





