Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser–Plasma Interactions
Abstract
1. Introduction
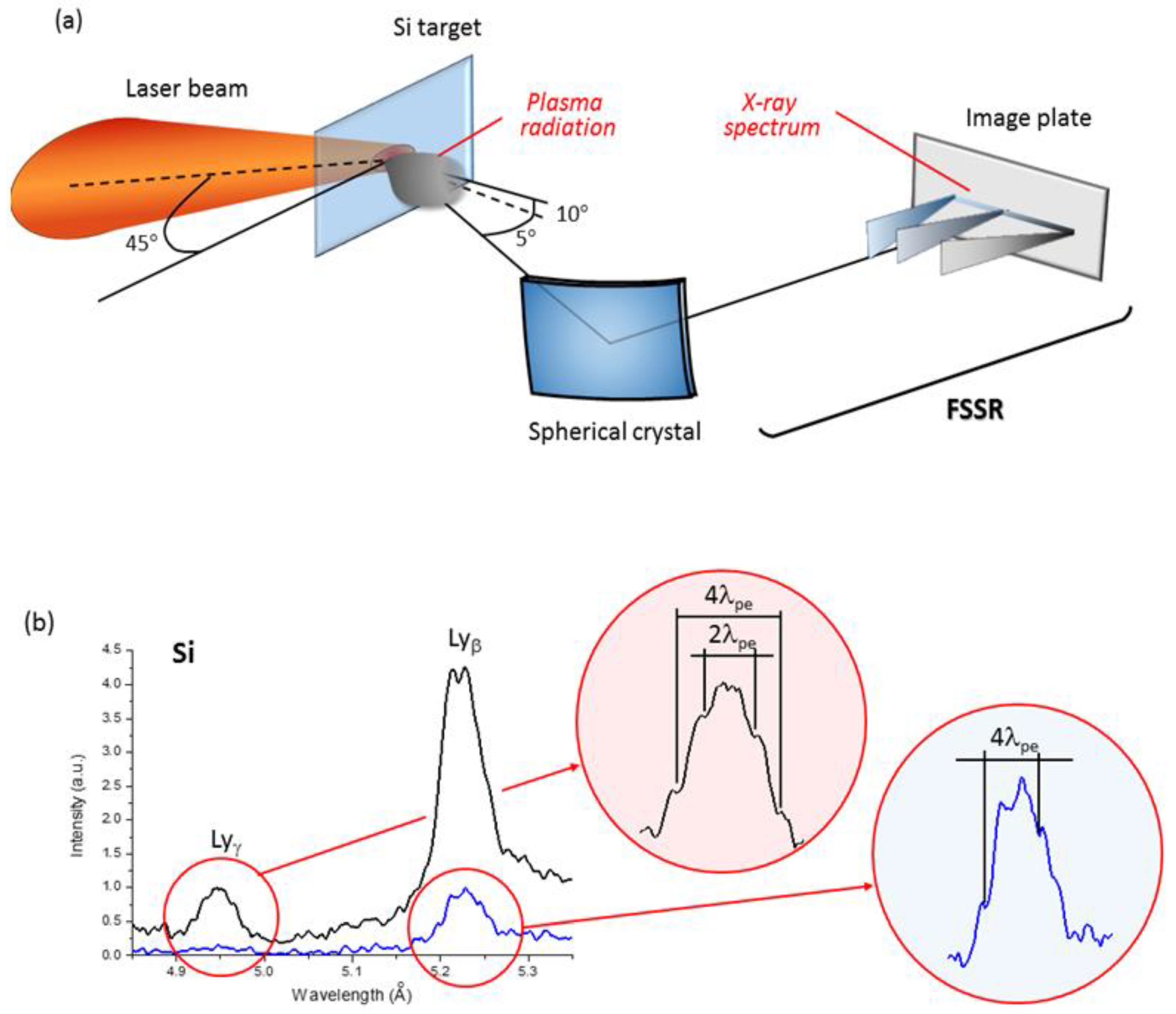
2. X-ray Spectra Measurements in Relativistic Laser–Plasma Interactions
3. Theory of Intra Stark Spectroscopy ISS for Relativistic Plasmas
3.1. The Langmuir-Wave-Caused Dips for Plasmas Dominated by a Low Frequency Electrostatic Turbulence LET
3.2. Rigorous Condition of the Dynamic Resonance
3.3. Robust Computation of Line Shapes Affected by the LET
4. Analysis of the Experimental Spectra and Comparison with Spectral Line Shapes Codes Simulations
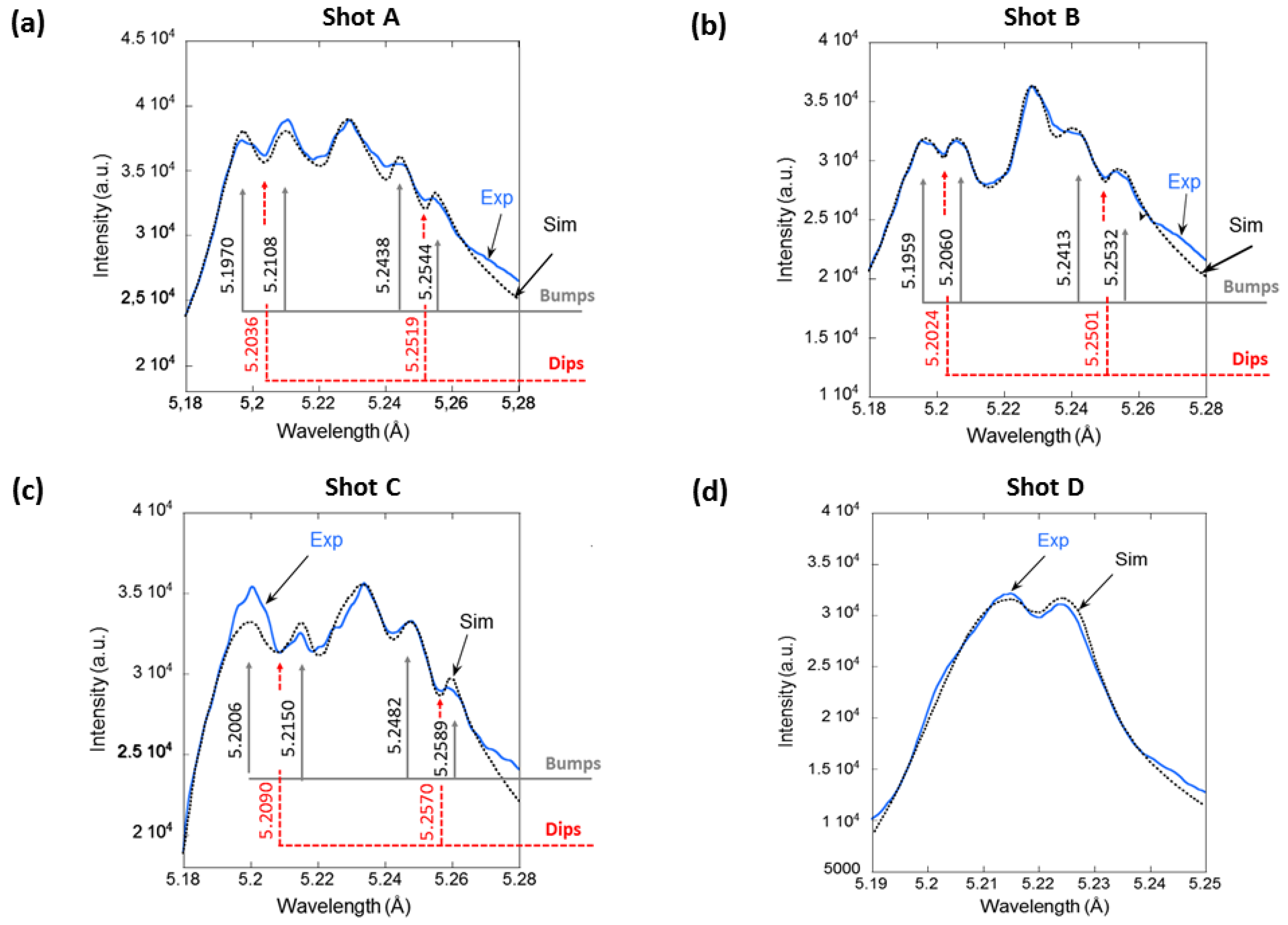
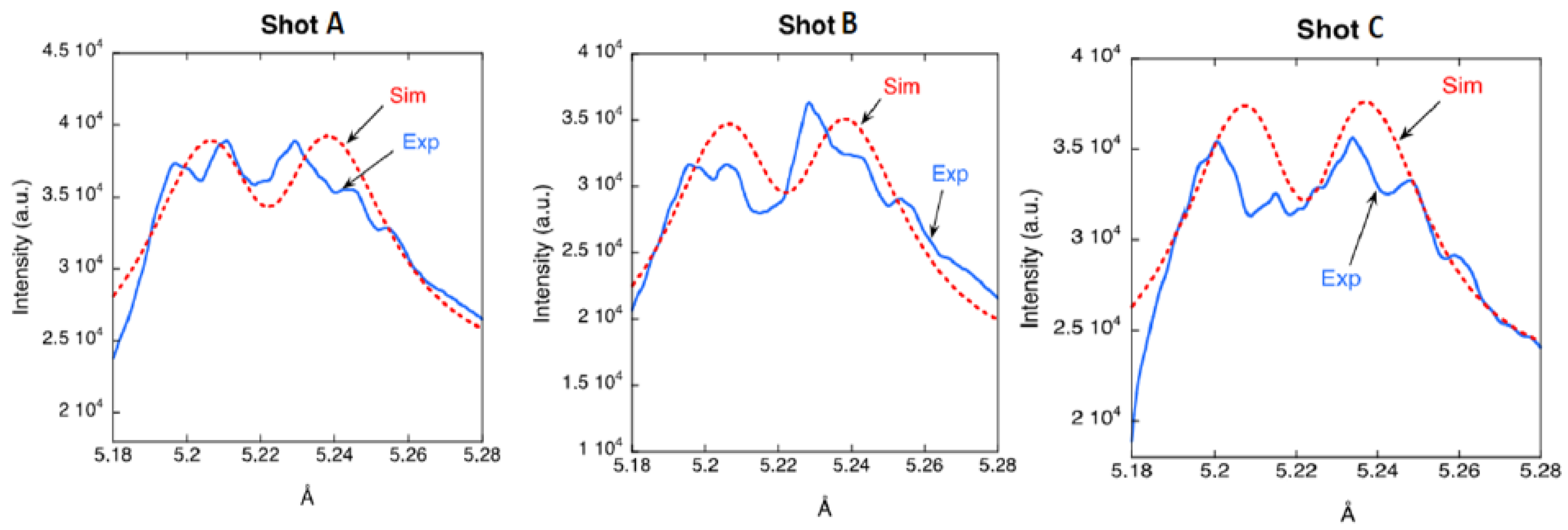
4.1. First Discovery of the Ion Acoustic Turbulence in Relativistic Laser–Plasmas Using X-ray Spectroscopy
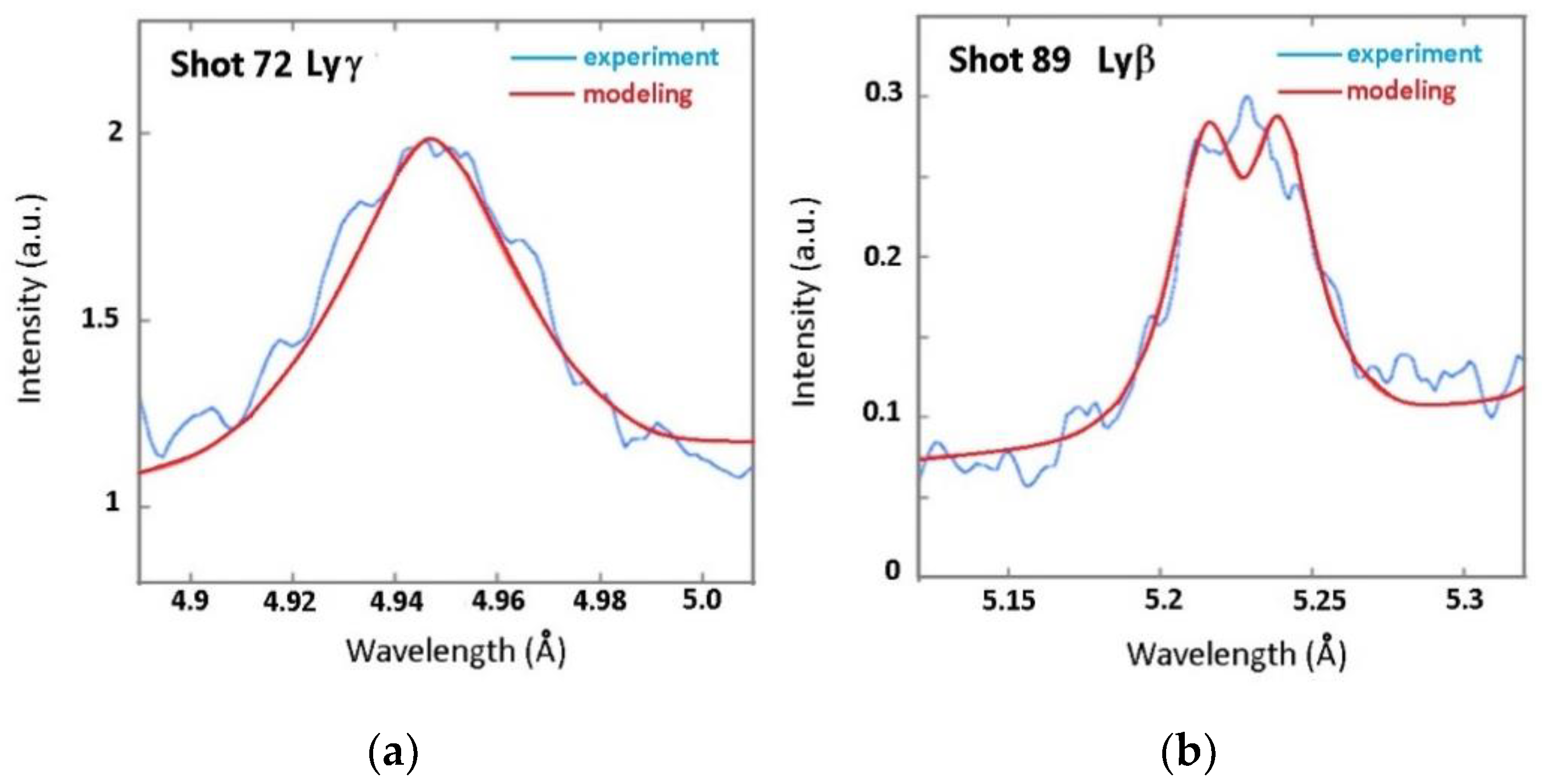
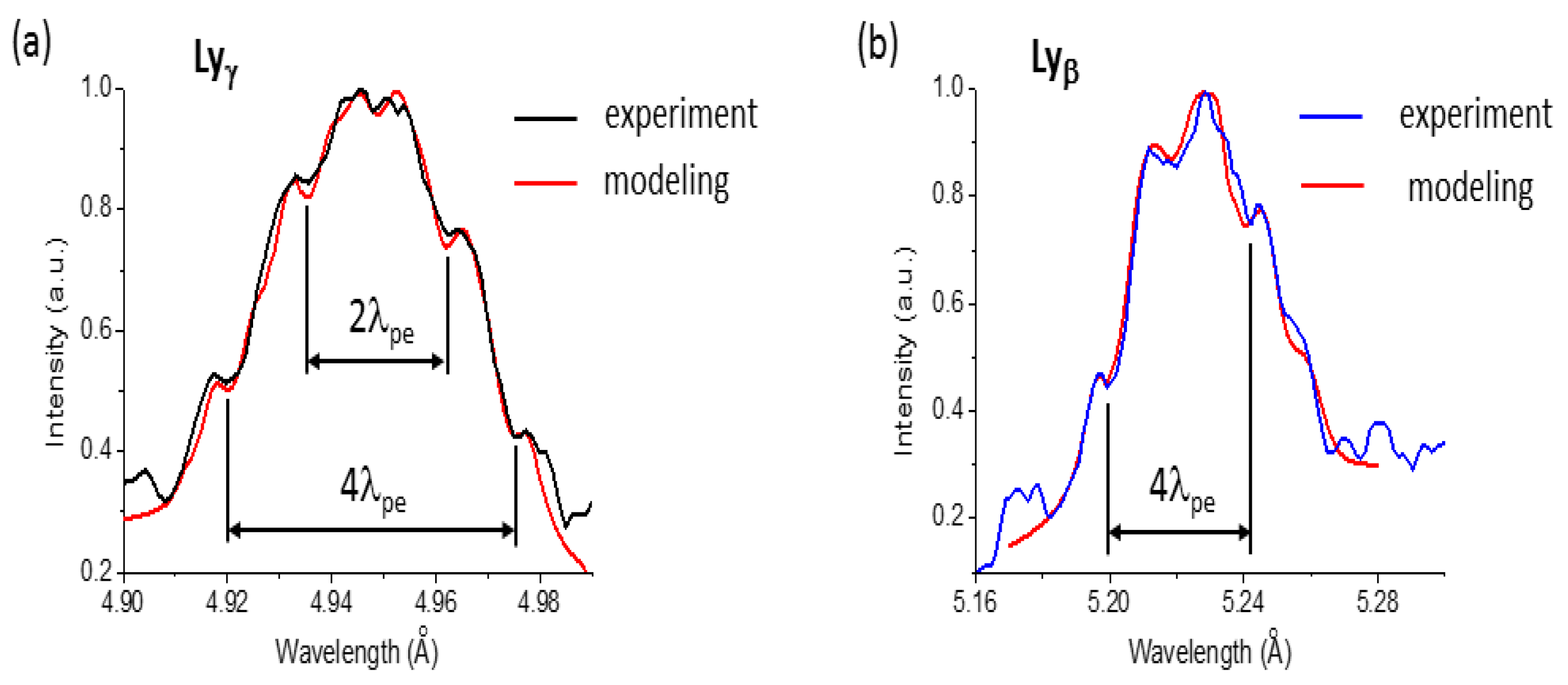
4.2. Robust Computations for the Analysis of the Experimental Si XIV Lyγ and Si XIV Lyβ Profiles
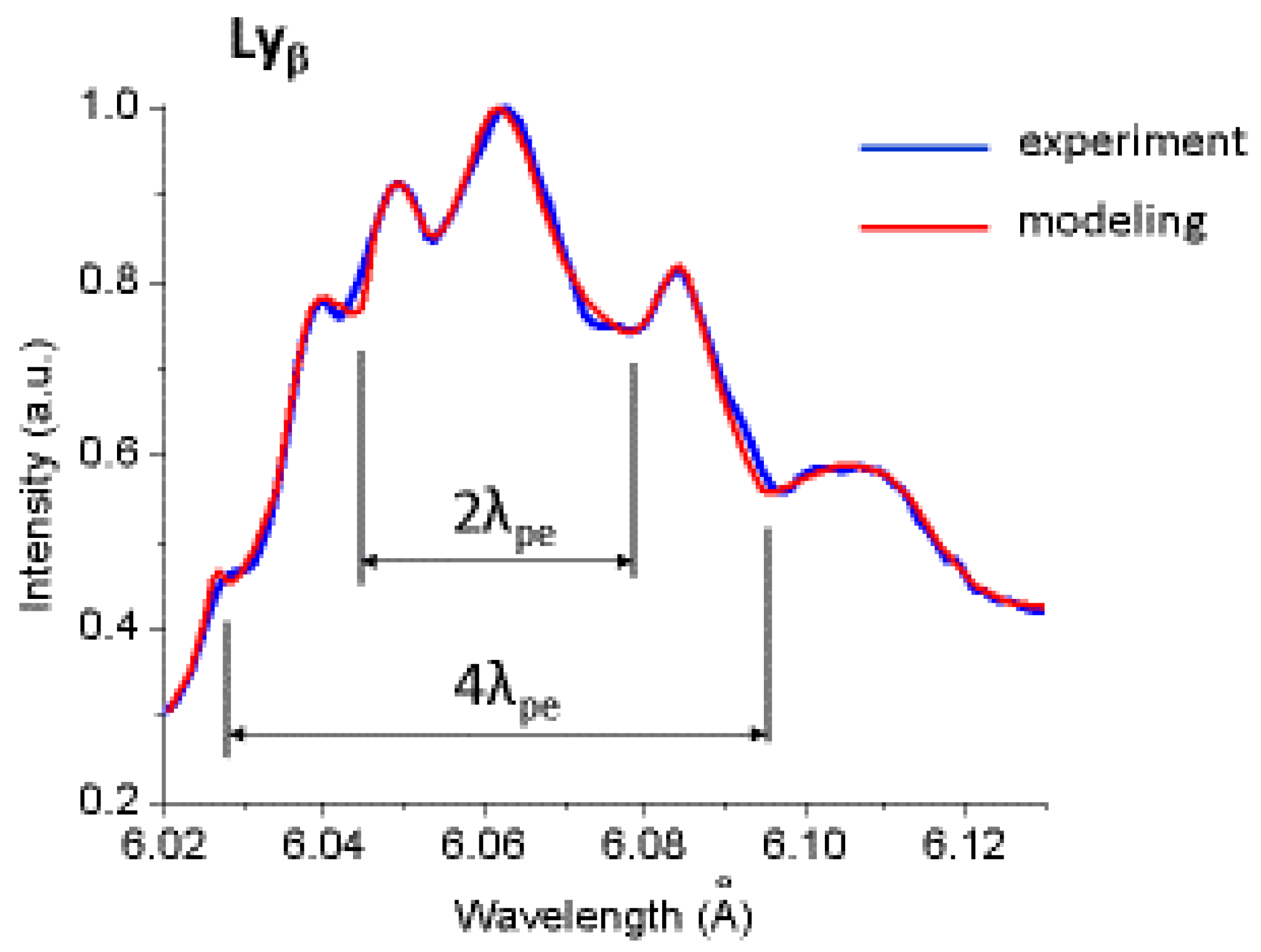
4.3. In-Depth Study of ISS in the X-ray Range Emission from Relativistic Laser–Plasmas
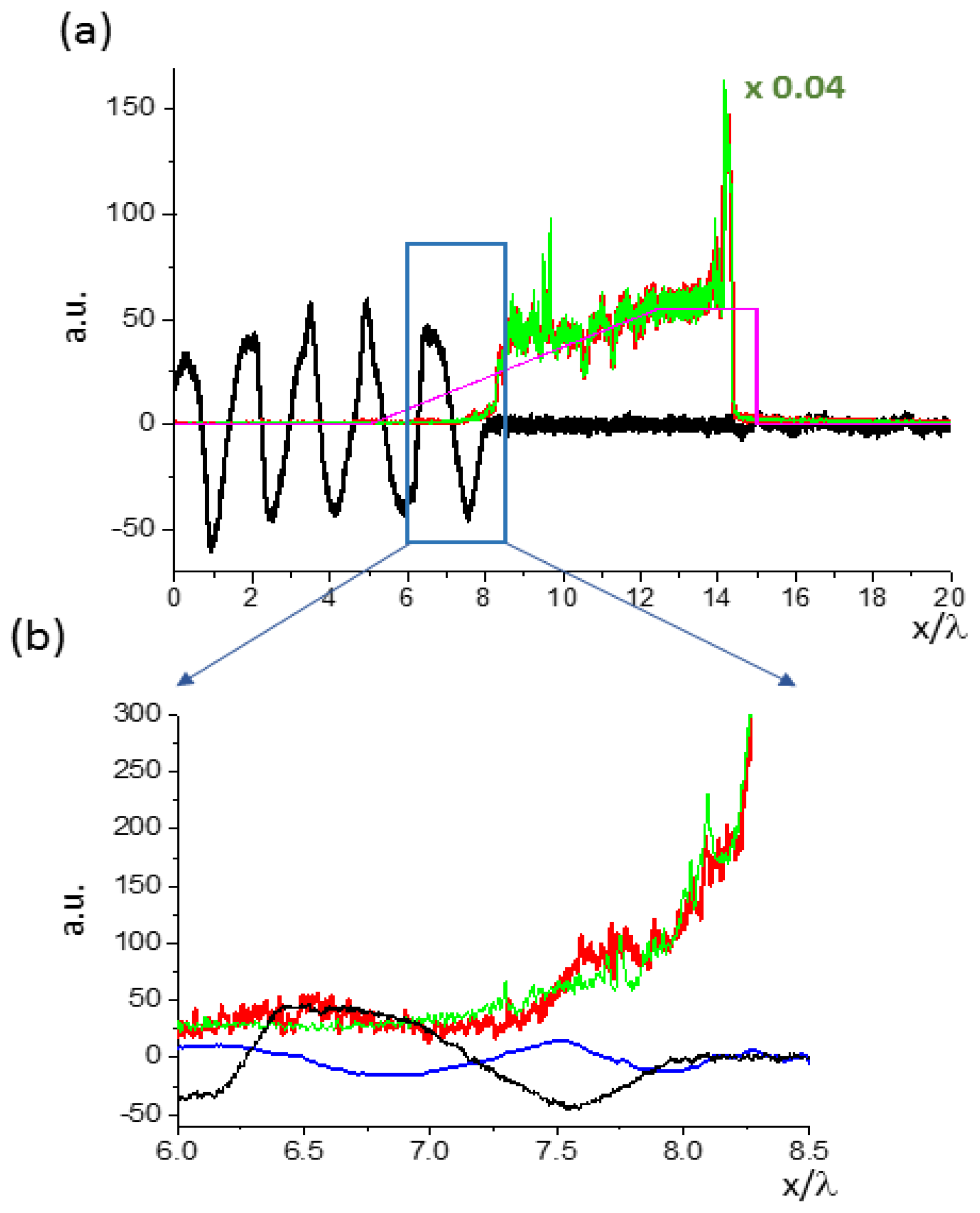
5. PIC Simulations for Relativistic Laser–Plasma Interactions
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Danson, C.N.; Brummitt, P.A.; Clarke, R.J.; Collier, J.L.; Fell, B.; Frackiewicz, A.J.; Hawkes, S.; Hernandez-Gomez, C.; Holligan, P.; Hutchinson, M.H.R.; et al. Vulcan petawatt: Design, operation and interactions at 5.1020 Wcm−2. Laser Part. Beams 2005, 23, 87. [Google Scholar] [CrossRef]
- Oks, E. Plasma Spectroscopy: The Influence of Microwave and Laser Fields; Ecker, G., Lambropoulos, P., Sobelman, I.I., Walther, H., Lotsch, H.K.V., Eds.; Springler-Verlag: Berlin/Heidelberg, Germany, 1995. [Google Scholar]
- Gavrilenko, V.P.; Oks, E. Intra-Stark spectroscopy of Coulomb radiators in a plasma with a quasi-monochromatic electric field. Sov. Phys. J. Plasma Phys. 1987, 13, 22. [Google Scholar]
- Oks, E.; Böddeker, S.; Kunze, H.J. Spectroscopy of atomic hydrogen in dense plasmas in the presence of dynamic fields: Intra-Stark Spectroscopy. Phys. Rev. A 1991, 44, 8338. [Google Scholar] [CrossRef] [PubMed]
- Gavrilenlo, V.P.; Oks, E. New effect in the Stark spectroscopy of atomic hydrogen: Dynamic resonance. Sov. Phys. JETP 1981, 53, 1122. [Google Scholar]
- Dalimier, E.; Oks, E.; Renner, O. Review of langmuir-wave-caused dips and charge-exchange caused dips in spectral lines from plasmas and their applications. Atoms 2014, 2, 178–194. [Google Scholar] [CrossRef]
- Dalimier, E.; Faenov, A.Y.; Oks, E.; Angelo, P.; Pikuz, T.A.; Fukuda, Y.; Andreev, A.; Koga, J.; Sakaki, H.; Kotaki, H.; et al. X-ray spectroscopy of super intense laser-produced plasmas for the study of non-linear processes. Comparison with PIC simulations. J. Phys. Conf. Ser. 2017, 810, 012004. [Google Scholar] [CrossRef]
- Dalimier, E.; Oks, E.; Renner, O. Dips in spectral line profiles and their applications in plasma physics and atomic physics. AIP Conf. Proc. 2017, 1811, 190003. [Google Scholar]
- Lu, J.; Xiao, S.; Yang, Q.; Liu, L.; Wu, Y. Spatially-resolved spectra from a new uniform dispersion crystal spectrometer for characterisation of Z-pinch plasmas. J. Quant. Spectrosc. Radiat. Transf. 2013, 116, 41. [Google Scholar]
- Renner, O.; Dalimier, E.; Oks, E.; Krasniqi, F.; Dufour, E.; Schott, R.; Foerster, E. Experimental evidence of Langmuir-wave caused features in spectral lines of laser-produced plasmas. J. Quant. Spectrosc. Radiat. Transf. 2006, 99, 439. [Google Scholar] [CrossRef]
- Oks, E.; Dalimier, E.; Faenov, A.Y.; Pikuz, T.; Fukuda, Y.; Jinno, S.; Sakaki, H.; Kotaki, H.; Pirozhkov, A.; Hayashi, Y.; et al. Two-plasmon decay instability’s signature in spectral lines and spectroscopic measurements of charge exchange rate in femtosecond laser-driven cluster-based plasma. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 221001. [Google Scholar] [CrossRef]
- Oks, E.; Dalimier, E.; Faenov, A.Y.; Angelo, P.; Pikuz, S.A.; Tubman, E.; Butler, N.M.H.; Dance, R.J.; Pikuz, T.A.; Skobelev, I.Y.; et al. Using X-ray spectroscopy of relativistic laser plasma interaction to reveal parametric decay instabilities: A modeling tool for astrophysics. Opt. Express 2017, 25, 1958. [Google Scholar] [CrossRef] [PubMed]
- Oks, E.; Dalimier, E.; Faenov, A.Y.; Angelo, P.; Pikuz, S.A.; Pikuz, T.A.; Skobelev, I.Y.; Ryazanzev, S.N.; Durey, P.; Doehm, L.; et al. In-depth study of intra-Stark spectroscopy in the X-ray range in relativistic laser-plasma interactions. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 245006. [Google Scholar] [CrossRef]
- Depierreux, S.; Fuchs, J.; Labaune, C.; Michard, A.; Baldis, H.A.; Pesme, D.; Hüller, S.; Laval, G. First observation of ion acoustic waves produced by the langmuir decay instability. Phys. Rev. Lett. 2000, 84, 2869. [Google Scholar] [CrossRef] [PubMed]
- Dromey, B.; Kar, S.; Bellei, C.; Carroll, D.C.; Clarke, R.J.; Green, J.S.; Kneip, S.; Markey, K.; Nagel, S.R.; Simpson, P.T.; et al. Bright multi-keV harmonic generation from relativistically oscillating plasma surfaces. Phys. Rev. Lett. 2007, 99, 085001. [Google Scholar] [CrossRef] [PubMed]
- Danson, C.N.; Brummitt, P.A.; Clarke, R.J.; Collier, J.L.; Fell, B.; Frackiewicz, A.J.; Hancock, S.; Hawkes, S.; Hernandez-Gomez, C.; Holligan, P.; et al. Vulcan Petawatt—An ultra-high-intensity interaction facility. Nucl. Fusion 2004, 44, 5239. [Google Scholar] [CrossRef]
- Dover, N.P.; Palmer, C.A.J.; Streeter, M.J.V.; Ahmed, H.; Albertazzi, B.; Borghesi, M.; Carroll, D.C.; Fuchs, J.; Heathcote, R.; Hilz, P.; et al. Buffered high charge spectrally-peaked proton beams in the relativistic-transparency regime. New J. Phys. 2016, 18, 013038. [Google Scholar] [CrossRef]
- Faenov, A.Y.; Pikuz, S.A.; Erko, A.I.; Bryunetkin, B.A.; Dyakin, V.M.; Ivanenkov, G.V.; Mingaleev, A.R.; Pikuz, T.A.; Romanova, V.M.; Shelkovenko, T.A. High-performance X-ray spectroscopic devices for plasma microsources investigations. Phys. Scr. 1994, 50, 333. [Google Scholar] [CrossRef]
- Lavrinenko, Y.S.; Morozov, I.V.; Pikuz, S.A.; Skobelev, I.Y. Reflectivity and imaging capabilities of spherically bent crystals studied by ray-tracing simulations. J. Phys. Conf. Ser. 2015, 653, 012027. [Google Scholar] [CrossRef]
- Alkhimova, M.A.; Pikuz, S.A.; Faenov, A.Y.; Skobelev, I.Y. Determination of spectral reflectivity of spherically bent mica crystals applied for diagnostics of relativistic laser plasmas. J. Phys. Conf. Ser. 2016, 774, 01215. [Google Scholar] [CrossRef]
- Böddeker, S.; Kunze, H.J.; Oks, E. Novel structure in the H alpha line profile of hydrogen in dense helium plasma. Phys. Rev. Lett. 1995, 75, 4740. [Google Scholar] [CrossRef] [PubMed]
- Maine, P.; Strickland, D.; Bado, P.; Pessot, M.; Mourou, G. Generation of ultrahigh peak power pulses by chirped pulse amplification. IEEE J. Quant. Electron 1988, QE-24, 398. [Google Scholar] [CrossRef]
- Akhiezer, A.I.; Polovin, R.V. Theory of wave motion of an electron plasma. Sov. Phys. JETP 1956, 3, 696. [Google Scholar]
- Lünow, W. On the relativistic non-linear interaction of cold plasma with electro-magnetic waves. Plasma Phys. 1968, 10, 879. [Google Scholar] [CrossRef]
- Guerin, S.; Mora, P.; Adam, J.C.; Heron, A.; Laval, G. Propagation of ultra-intense laser pulses through over-dense plasma layers. Phys. Plasmas 1996, 3, 2693. [Google Scholar] [CrossRef]
- Gray, R.J.; Carroll, D.C.; Yuan, X.H.; Brenner, C.M.; Burza, M.; Coury, M.; Lancaster, K.L.; Lin, X.X.; Li, Y.T.; Neely, D.; et al. Laser pulse propagation and enhanced energy coupling to fast electrons in dense plasma gradients. New J. Phys. 2014, 16, 113075. [Google Scholar] [CrossRef]
- Mourou, G.M.; Tajima, T.; Bulanov, S.V. Optics in the relativistic regime. Rev. Modern Phys. 2006, 78, 309. [Google Scholar] [CrossRef]
- Oks, E. Diagnostics of Laboratory and Astrophysical Plasmas Using Spectral Line-Shapes of One-, Two-, and Three-Electron Systems; Work Scientific Publishing: Singapore, 2017; ISBN 9814699071. [Google Scholar]
- Oks, E.; Sholin, G.V. On Stark profiles of hydrogen lines in a plasma with low-frequency turbulence. Sov. Phys. Tech. Phys. 1976, 21, 144. [Google Scholar]
- Iglesias, C.A.; Dewitt, H.E.; Lebowitz, J.L.; MacGowan, D.; Hubbard, W.B. Low-frequency electric microfield distributions in plasmas. Phys. Rev. A 1985, 31, 1698. [Google Scholar] [CrossRef]
- Dalimier, E.; Oks, E. Robust computational method for fast calculations of multi-charged ions line-shapes affected by a low-frequency electrostatic plasma turbulence. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 025701. [Google Scholar] [CrossRef]
- Djurovic, S.; Ćirišan, M.; Demura, A.V.; Demchenko, G.V.; Nikolić, D.; Gigosos, M.A.; González, M.A. Measurements of Hβ Stark central asymmetry and its analysis through standard theory and computer simulations. Phys. Rev. E 2009, 79, 046402. [Google Scholar] [CrossRef] [PubMed]
- Demura, A.V.; Demchenko, G.V.; Nikolic, D. Multiparametric dependence of hydrogen Stark profiles asymmetry. Eur. Phys. J. D 2008, 46, 111. [Google Scholar] [CrossRef]
- Chung, H.K.; Chen, M.H.; Morgan, W.L.; Ralchenko, Y.; Lee, R.W. Generalised population kinetics and spectral model for rapid spectroscopic analysis for all elements. High Energy Density Phys. 2005, 1, 3. [Google Scholar] [CrossRef]
- Lichters, R.; Meyer-ter-Vehn, J.; Pukhov, A. Short pulse laser harmonics from oscillating plasma surfaces driven at relativistic intensity. Phys. Plasmas 1996, 3, 3425–3437. [Google Scholar] [CrossRef]
- Kruer, W.L. The Physics of Laser Plasma Interactions; Edition Reprint, Collection Frontiers in Physics; Taylor and Francis, Ed.; Westview Press: Boulder, CO, USA, 2003. [Google Scholar]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dalimier, E.; Pikuz, T.A.; Angelo, P. Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser–Plasma Interactions. Atoms 2018, 6, 45. https://doi.org/10.3390/atoms6030045
Dalimier E, Pikuz TA, Angelo P. Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser–Plasma Interactions. Atoms. 2018; 6(3):45. https://doi.org/10.3390/atoms6030045
Chicago/Turabian StyleDalimier, Elisabeth, Tatiana A. Pikuz, and Paulo Angelo. 2018. "Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser–Plasma Interactions" Atoms 6, no. 3: 45. https://doi.org/10.3390/atoms6030045
APA StyleDalimier, E., Pikuz, T. A., & Angelo, P. (2018). Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser–Plasma Interactions. Atoms, 6(3), 45. https://doi.org/10.3390/atoms6030045



