General Aspects of Line Shapes in Plasmas in the Presence of External Electric Fields
Abstract
1. Introduction
2. The Lineshape Formula
3. Approximations on the Electron and Ion Treatments
4. Adding Randomness to the Oscillatory Field
- Different phases (e.g., plasma oscillations, such as Langmuir turbulence and, although the electric field that two different emitters experience as a function of time may have the same frequency and peak amplitude, their phases may be different, e.g., one emitter experiences an oscillatory field , the other , etc.
- Different field peak magnitudes (e.g., damping, i.e., the maximum field at an emiiter at point may be smaller than the maximum at an emitter at point ).
- Different frequencies (broadband frequency/dispersion).
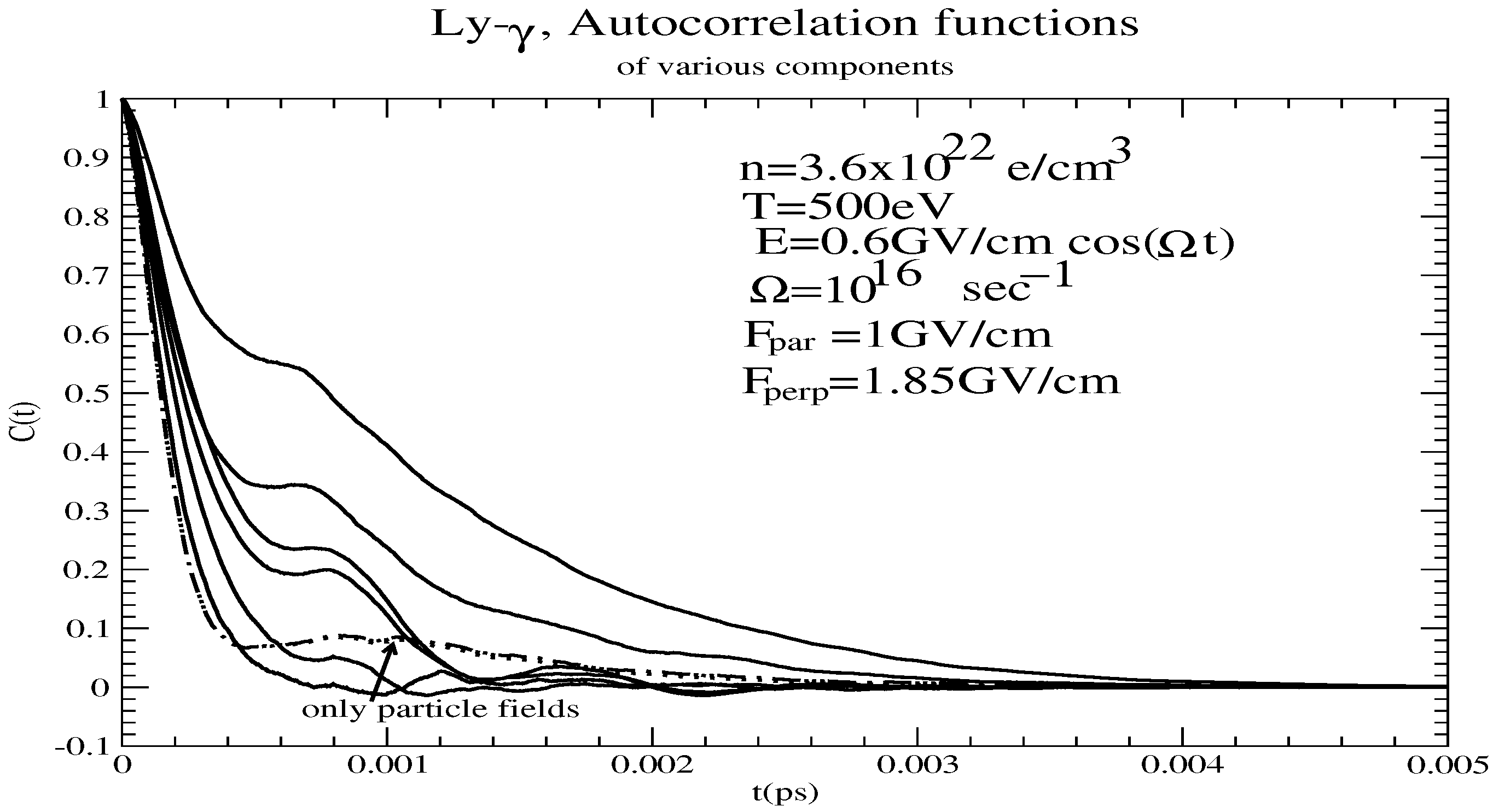
5. Numerical Solution: Stiffness Issues
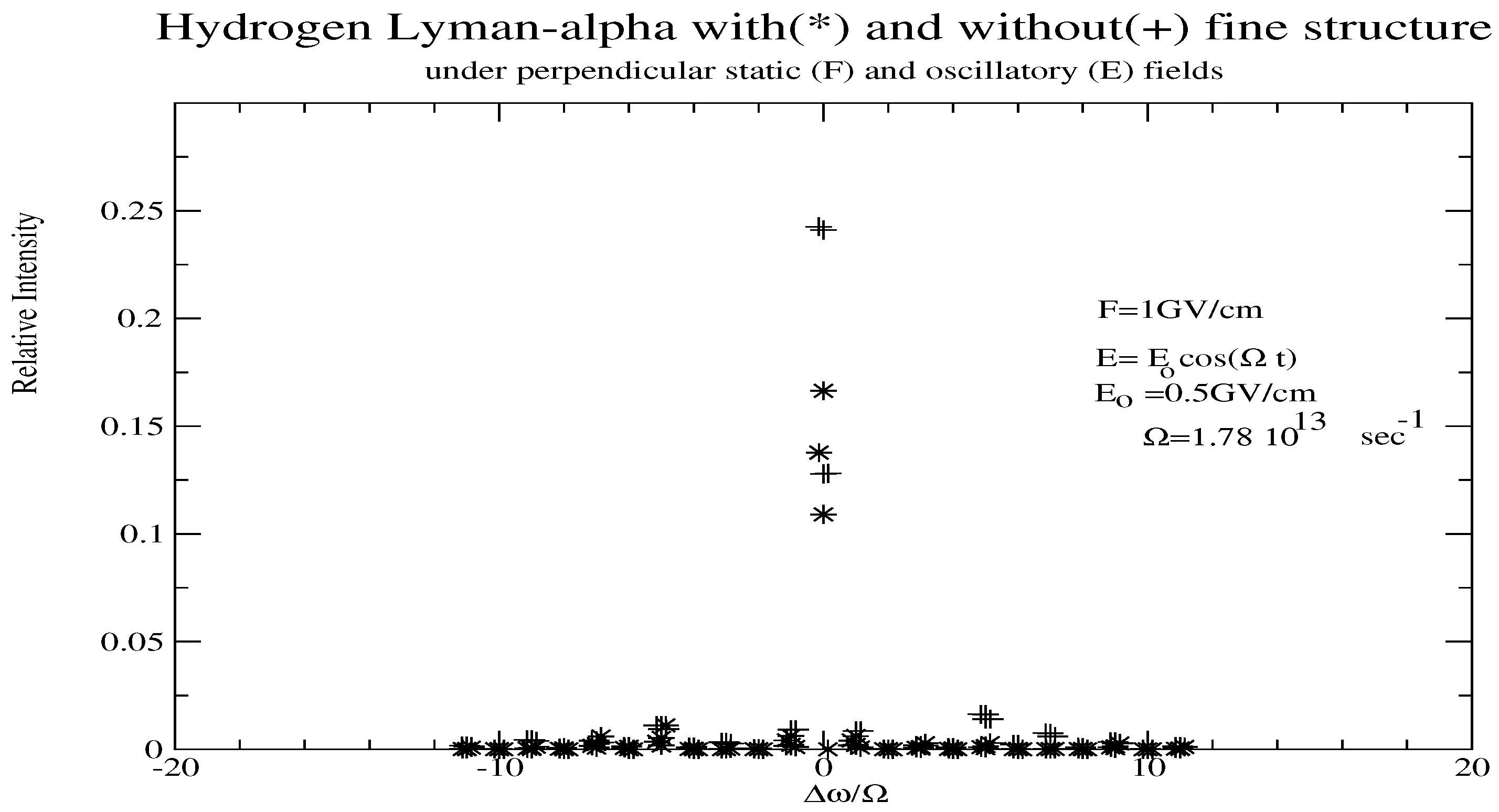
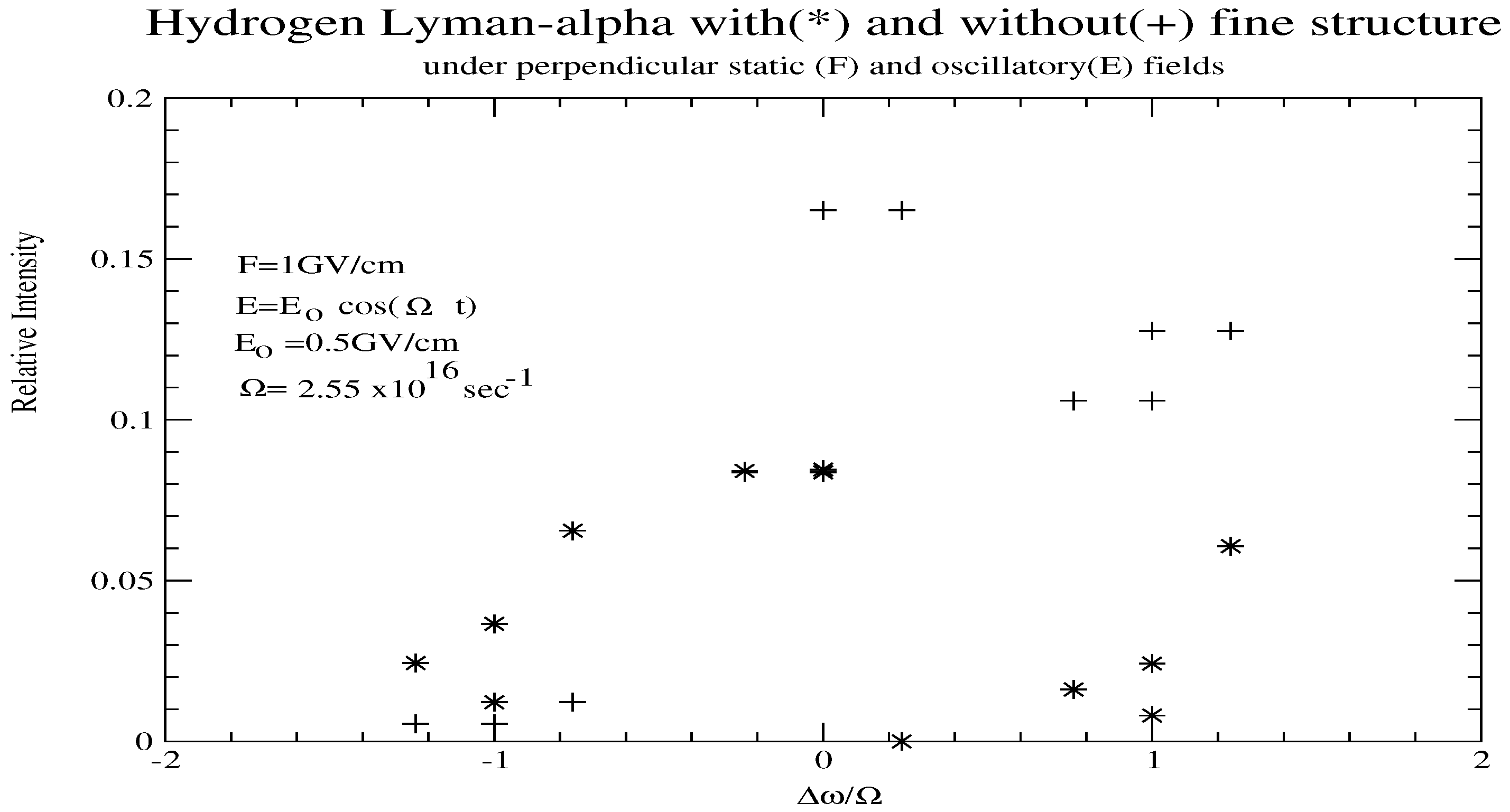
6. Role of Fine Structure
7. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Random Phases
References
- Alexiou, S. Overview of plasma line broadening. High Energy Density Phys. 2009, 5, 225–233. [Google Scholar] [CrossRef]
- Peyrusse, O. Stark-profile calculations for spectral lines of hydrogenic ions in plasmas submitted to a strong oscillating electric field. Phys. Scr. 1997, 56, 371–380. [Google Scholar] [CrossRef]
- Sauvan, P.; Dalimier, E. Floquet-Liouville approach for calculating Stark profiles in plasmas in the presence of a strong oscillating field. Phys. Rev. E 2009, 79, 036405. [Google Scholar] [CrossRef]
- Baranger, M.; Mozer, B. Light as a Plasma Probe. Phys. Rev. 1961, 1123, 25–28. [Google Scholar] [CrossRef]
- Lisitsa, V. Atoms in Plasma; Springer: Berlin/Heidelberg, Germany, 1994; ISBN 3-540-57580-4. [Google Scholar]
- Alexiou, S. Methods for Line Shapes in Plasmas in the Presence of External Electric Fields. Atoms 2021, 9, 30. [Google Scholar] [CrossRef]
- Oks, E.A. Plasma Spectroscopy: The Influence of Microwave and Laser Fields; Springer Series on Atoms and Plasmas; Springer: New York, NY, USA, 1995; Volume 9, ISBN 3-540-54100-4. [Google Scholar]
- Cohn, A.; Bakshi, P.; Kalman, G. Linear Stark Effect Due to Resonant Interactions of Static and Dynamic Fields. Phys. Rev. Lett. 1972, 29, 324–326, Erratum in Phys. Rev. Lett. 1973, 31, 620–620.. [Google Scholar] [CrossRef]
- Bakshi, P.; Kalman, G.; Cohn, A. Hydrogenic Stark-Zeeman Spectra for Combined Static and Dynamic Fields. Phys. Rev. Lett. 1973, 31, 1576–1579. [Google Scholar] [CrossRef]
- Deutsch, C.; Bekefi, G. Stark-broadening calculations of the Lyman-α line in a turbulent plasma. Phys. Rev. 1976, 14, 854–862. [Google Scholar] [CrossRef]
- Oks, E.; Böddeker, S.; Kunze, H.J. Spectroscopy of atomic hydrogen in dense plasmas in the presence of dynamic fields: Intra-Stark spectroscopy. Phys. Rev. A 1991, 44, 8338–8347. [Google Scholar] [CrossRef] [PubMed]
- Nee, T.-J.A.; Griem, H.R. Measurement of hydrogen n-α line Stark profiles in a turbulent plasma. Phys. Rev. A 1976, 14, 1853–1868. [Google Scholar] [CrossRef]
- Nee, T.-J. Calculation of helium plasma satellites in turbulent plasmas. J. Quant. Spectrosc. Radiat. Transf. 1987, 38, 213–224. [Google Scholar] [CrossRef]
- Griem, H.R.; Kunze, H.J. Stark Broadening of Two Ionized-Helium Lines by Collective Electric Fields in a Laboratory Plasma. Phys. Rev. Lett. 1969, 23, 1279–1281. [Google Scholar] [CrossRef]
- Sarid, E.; Maron, Y.; Troyanksi, L. Spectroscopic investigation of fluctuating anisotropic electric fields in a high-power diode plasma. Phys. Rev. E 1993, 48, 1364–1374. [Google Scholar] [CrossRef] [PubMed]
- Balakin, A.A. Operator of pair electron-ion collisions in alternating electromagnetic fields. Plasma Phys. Rep. 2008, 34, 1046–1053. [Google Scholar] [CrossRef]
- Rosato, J. Hydrogen Line Shapes in Plasmas with Large Magnetic Fields. Atoms 2020, 8, 74. [Google Scholar] [CrossRef]
- Alexiou, S. Line Shapes in a Magnetic Field: Trajectory Modifications I: Electrons. Atoms 2019, 7, 52. [Google Scholar] [CrossRef]
- Blokhintsev, O. Theory of the Stark Effect in a Time-Dependent Field. Phys. Z. Sowjet. 1933, 4, 501–515. [Google Scholar]
- Letunov, A.Y.; Lisitsa, V.S. Stark–Zeeman and Blokhintsev Spectra of Rydberg Atoms. JETP 2020, 131, 696–706. [Google Scholar] [CrossRef]
- Alexiou, S.; Weingarten, A.; Maron, Y.; Sarfaty, M.; Krasik, Y.E. Novel Spectroscopic Method for Analysis of Nonthermal Electric Fields in Plasmas. Phys. Rev. Lett. 1995, 75, 3126–3129. [Google Scholar] [CrossRef]
- Weingarten, A.; Alexiou, S.; Maron, Y.; Sarfaty, M.; Krasik, Y.E.; Kingsep, Y. Observation of nonthermal turbulent electric fields in a nanosecond plasma opening switch experiment. Phys. Rev. E 1999, 59, 1096–1110. [Google Scholar] [CrossRef]
- Alexiou, S. X-ray laser line narrowing: New developments. J. Quant. Spectrosc. Radiat. Transfer. 2001, 71, 139–146. [Google Scholar] [CrossRef]
- Rosato, J.; Marandet, Y.; Stamm, R. Quantifying the statistical noise in computer simulations of Stark broadening. J. Quant. Spectrosc. Radiat. Transfer. 2020, 249, 107002–107010. [Google Scholar] [CrossRef]
- Stambulchik, E.; Kroupp, E.; Maron, Y.; Malka, V. On the Stark Effect of the OI 777-nm Triplet in Plasma and Laser Fields. Atoms 2020, 8, 84. [Google Scholar] [CrossRef]
- Alexiou, S. Analysis of Plasma Emission Experiments and ‘Dips’. Atoms 2023, 11, 29. [Google Scholar] [CrossRef]
- Dalimier, E.; Pikuz, T.A.; Angelo, P. Mini-Review of Intra-Stark X-ray Spectroscopy of Relativistic Laser—Plasma Interactions. Atoms 2018, 6, 45. [Google Scholar] [CrossRef]
- Hairer, E.; Wanner, G. Solving ordinary differential equations II. In Stiff and Differential-Algebraic Problems, 2nd ed.; Springer Series in Computational Mathematics 14; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Hannachi, I.; Alexiou, S.; Stamm, R. Line shape code comparison of the effect of periodic fields on hydrogen lines. Atoms 2024. in print. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alexiou, S. General Aspects of Line Shapes in Plasmas in the Presence of External Electric Fields. Atoms 2024, 12, 17. https://doi.org/10.3390/atoms12030017
Alexiou S. General Aspects of Line Shapes in Plasmas in the Presence of External Electric Fields. Atoms. 2024; 12(3):17. https://doi.org/10.3390/atoms12030017
Chicago/Turabian StyleAlexiou, Spiros. 2024. "General Aspects of Line Shapes in Plasmas in the Presence of External Electric Fields" Atoms 12, no. 3: 17. https://doi.org/10.3390/atoms12030017
APA StyleAlexiou, S. (2024). General Aspects of Line Shapes in Plasmas in the Presence of External Electric Fields. Atoms, 12(3), 17. https://doi.org/10.3390/atoms12030017






