R-Matrix Calculation of Electron Collision with the BeO+ Molecular Ion
Abstract
1. Introduction
2. Theory
3. Calculations
3.1. Calculation of the BeO Target
| Target States | Model M1 | Model M2 | Model M3 | Ghalila et al. | Ghalila et al. VEE |
|---|---|---|---|---|---|
| X | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
| 1 | 1.58 | 1.48 | 1.71 | 1.16 | 1.28 |
| 1 | 3.94 | 3.71 | 3.71 | 2.92 | 3.77 |
| 1 | 4.14 | 3.88 | 3.92 | 3.15 | 4.11 |
| 2 | 5.80 | 5.44 | 5.68 | 4.92 | 5.51 |
| 1 | 6.00 | 5.88 | 5.78 | 5.04 | 5.93 |
| 2 | 7.27 | 7.13 | 7.08 | 6.11 | 6.93 |
| 2 | 7.92 | 7.24 | 7.74 | 6.12 | 7.12 |
| 3 | 7.74 | 7.45 | 7.68 | 6.21 | 7.39 |
| 4 | 8.64 | 8.15 | 8.46 | 6.91 | 8.14 |
| 1 | 9.28 | 8.47 | 9.21 | 7.34 | 8.19 |
| 1 | 9.47 | 8.72 | 9.40 | 7.51 | 8.51 |
| 2 | 9.62 | 8.92 | 9.54 | 7.57 | 8.74 |
| 2 | 9.73 | 9.07 | 9.64 | 7.79 | 9.09 |
| 2 | 11.77 | 11.12 | 11.75 | 7.83 | 9.18 |
3.2. Inner-Region Solutions
3.3. Outer-Region Solutions and Scattering Calculations
4. Results
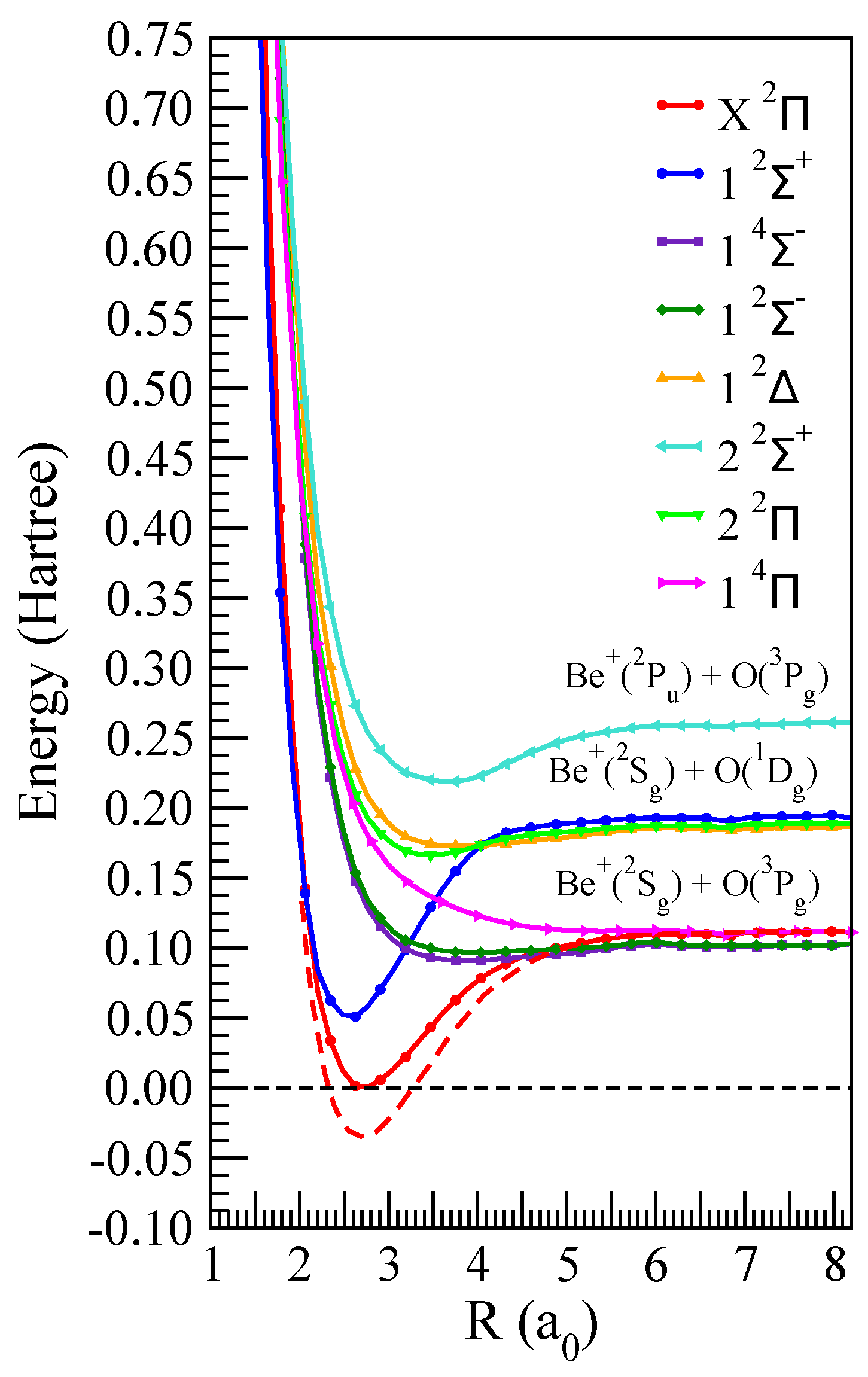
4.1. Bound States of BeO
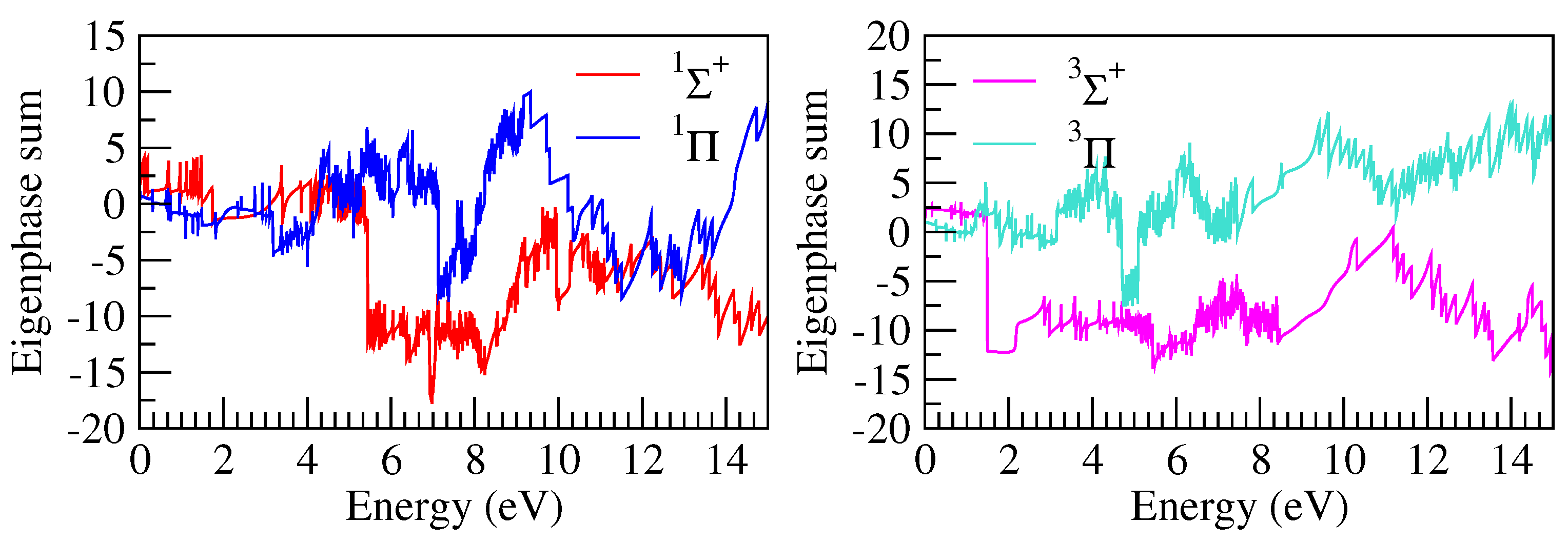
4.2. Resonances
4.3. Cross-Sections and Rate Coefficients
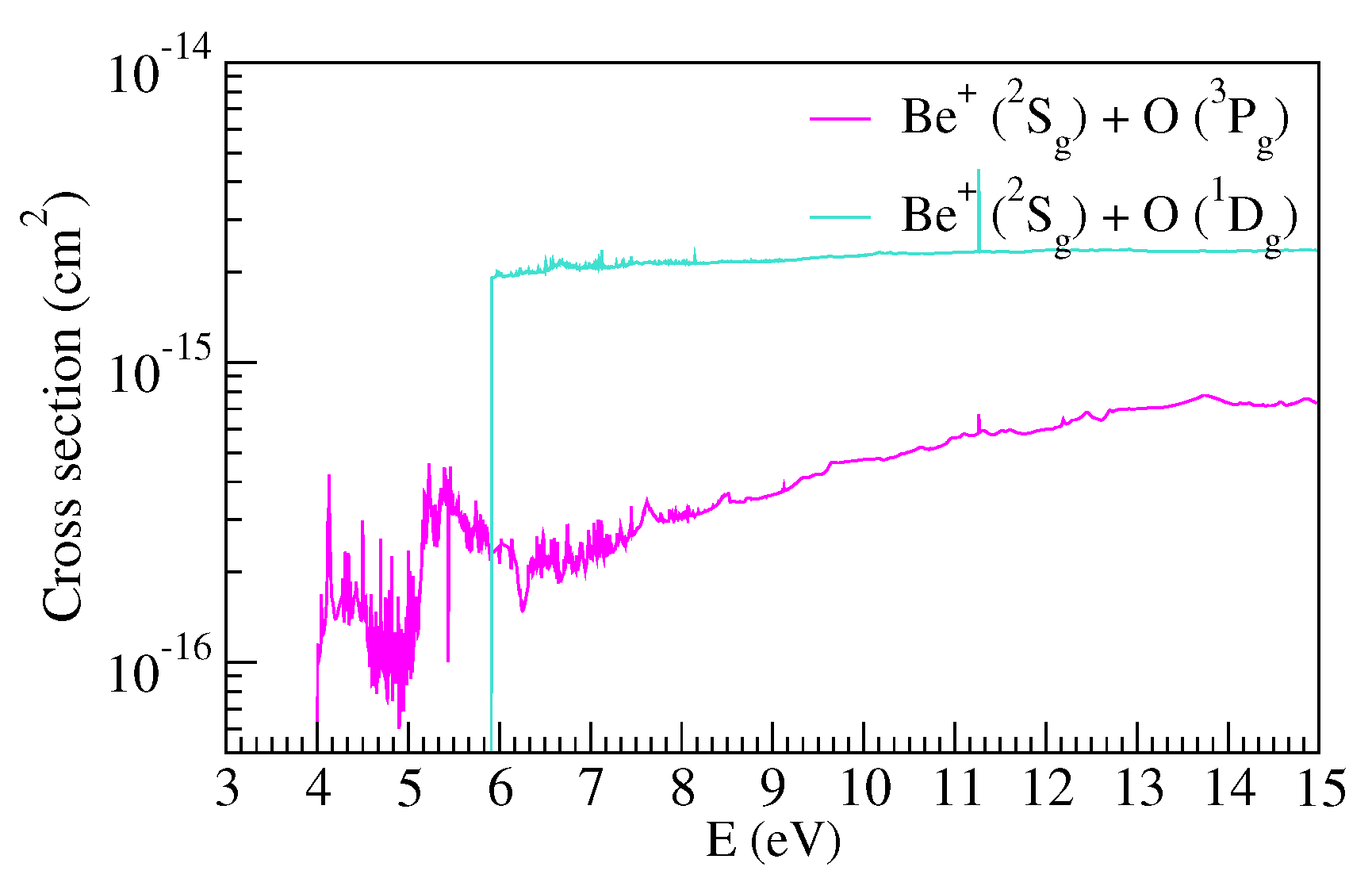
4.3.1. Electron Impact Excitation
4.3.2. Electron Impact Dissociation
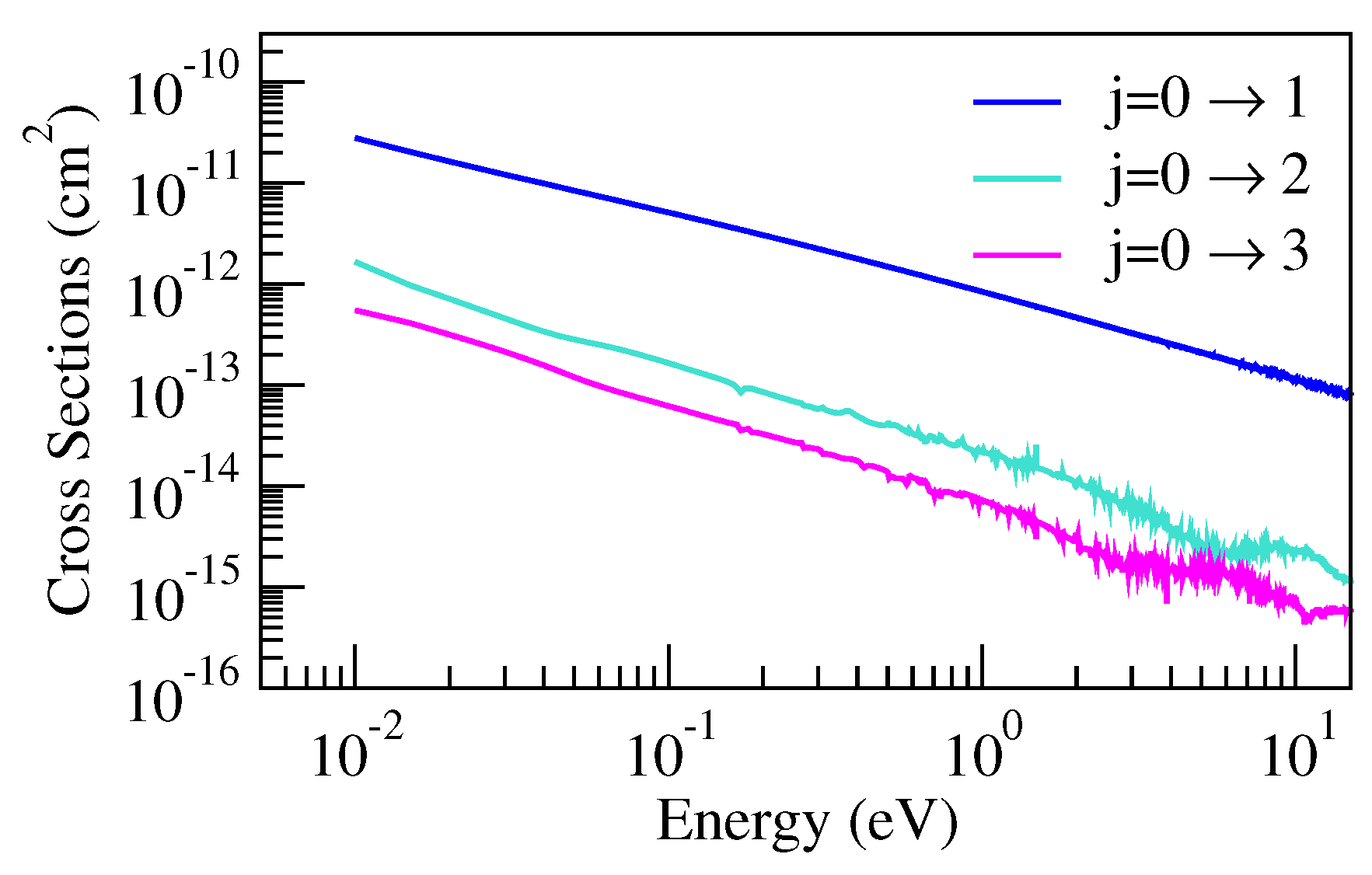
4.3.3. Rotational Excitation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Raffray, A.R.; Federici, G.; Barabash, V.; Pacher, H.D.; Bartels, H.W.; Cardella, A.; Jakeman, R.; Ioki, K.; Janeschitz, G.; Parker, R.; et al. Beryllium application in ITER plasma facing components. Fusion Eng. Des. 1997, 37, 261–286. [Google Scholar] [CrossRef]
- Pitts, R.A.; Carpentier, A.; Escourbiac, F.; Hirai, T.; Komarov, V.; Kukushkin, A.S.; Lisgo, S.; Loarte, A.; Merola, M.; Mitteau, R.; et al. Physics basis and design of the ITER plasma-facing components. J. Nucl. Mater. 2011, 415, S957–S964. [Google Scholar] [CrossRef]
- Makepeace, C.; Pardanaud, C.; Roubin, P.; Borodkina, I.; Ayres, C.; Coad, P.; Baron-Wiechec, A.; Jepu, I.; Heinola, K.; Widdowson, A.; et al. The effect of beryllium oxide on retention in JET ITER-like wall tiles. J. Nucl. Mater. 2019, 19, 346. [Google Scholar] [CrossRef]
- Hodille, E.A.; Byggmäster, J.; Safi, E.; Nordlund, K. Sputtering of beryllium oxide by deuterium at various temperatures simulated with molecular dynamics. Pys. Scr. 2020, T171, 014024. [Google Scholar] [CrossRef]
- Romazanov, J.; Kirschner, A.; Brezinsek, S.; Pitts, R.A.; Borodin, D.; Rode, S.; Navarro, M.X.; Schmid, K.; Veshchev, E.S.; Neverov, V.S.; et al. Beryllium erosion and redeposition in ITER H, He and D–T discharges. Nucl. Fusion 2022, 62, 036011. [Google Scholar] [CrossRef]
- Cardella, A.; Barabash, V.; Ioki, K.; Yamada, M.; Hatano, T.; Ohara, Y.; Lorenzetto, P.; Merola, M.; Mazul, I.; Strebkov, Y. Application of Beryllium as First Wall Armour for ITER Primary, Baffle, and Limiter Modules. Fusion Technol. 2020, 38, 326. [Google Scholar] [CrossRef]
- Partridge, H.; Langhoff, S.R.; Bauschlicher, W., Jr. Ab initio calculations on the positive ions of the alkalineearth oxides, fluorides, and hydroxides. J. Chem. Phys. 1986, 84, 4489. [Google Scholar] [CrossRef]
- Ghalila, H.; Lahmar, S.; Ben Lakhdar, Z.; Hochlaf, M. Spectroscopy and metastability of BeO+. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 205101. [Google Scholar] [CrossRef]
- Mukherjee, N.; Chakrabarti, K. Theoretical study of low energy electron collisions with the BeO molecule. J. Phys. B At. Mol. Opt. Phys. 2023, 56, 015202. [Google Scholar] [CrossRef]
- Tennyson, J. Electron–molecule collision calculations using the R-matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- Burke, P.G. R-Matrix Theory of Atomic Collisions; Springer: Berlin, Germany, 2011. [Google Scholar]
- Noble, C.J.; Nesbet, R.K. CFASYM, A Program for the Calculation of the Asymptotic Solutions of the Coupled Equations of Electron Collision Theory. Comput. Phys. Commun. 1984, 33, 399. [Google Scholar] [CrossRef]
- Carr, J.M.; Galiatsatos, P.G.; Gorfinkiel, J.D.; Harvey, A.G.; Lysaght, M.A.; Madden, D.; Mašín, Z.; Plummer, M.; Tennyson, J.; Varambhia, H.N. UKRmol: A low-energy electron- and positron-molecule scattering suite. Euro. Phys. J. D 2012, 66, 58. [Google Scholar] [CrossRef]
- Mašín, Z.; Benda, J.; Gorfinkiel, J.D.; Harvey, A.G.; Tennyson, J. UKRmol+: A suite for modelling electronic processes in molecules interacting with electrons, positrons and photons using the R-matrix method. Comput. Phys. Commun. 2020, 249, 107092. [Google Scholar] [CrossRef]
- Ghosh, R.; Chakrabarti, K.; Choudhury, B.S. Electron induced processes in CH: An R-matrix study of electronic excitation, dissociation and dissociative attachment. Plasma Sources Sci. Technol. 2020, 29, 095016. [Google Scholar] [CrossRef]
- Ghosh, R.; Chakrabarti, K.; Choudhury, B.S. Electron impact studies on the imidogen (NH+) molecular ion. Plasma Sources Sci. Technol. 2022, 31, 065005. [Google Scholar] [CrossRef]
- Bagus, P.S.; Moser, C.M.; Goethals, P.; Verhaegen, G. Accurate ab initio calculation of the BeH molecule. I. The X 2Π+ and A 2Π states. J. Chem. Phys. 1973, 58, 1886. [Google Scholar] [CrossRef]
- Cade, P.E.; Huo, H. Hartree-Fock-Roothaan Wavefunctions for Diatomic Molecules. Atomic Data Nucl. Data Tables 1973, 13, 415. [Google Scholar] [CrossRef]
- Buttle, P.J.A. Solution of Coupled Equations by R-Matrix Techniques. Phys. Rev. 1967, 160, 719. [Google Scholar] [CrossRef]
- Tennyson, J. A new algorithm for Hamiltonian matrix construction in electron–molecule collision calculations. J. Phys. B At. Mol. Opt. Phys. 1996, 29, 1817–1828. [Google Scholar] [CrossRef]
- Chakrabarti, K.; Dinda, S. Calculated cross sections for electron collisions with the BeN molecule. Plasma Phys. Control. Fusion 2023, 65, 085017. [Google Scholar] [CrossRef]
- Sarpal, B.K.; Branchett, S.E.; Tennyson, J.; Morgan, L.A. Bound states using the R-matrix method: Rydberg states of HeH. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 3685–3699. [Google Scholar] [CrossRef]
- Rabádan, I.; Tennyson’, J. R-Matrix calculation of the bound and continuum states of the e–NO+ system. J. Phys. B At. Mol. Opt. Phys. 1996, 29, 3747. [Google Scholar] [CrossRef]
- Buenker, R.J.; Leibermann, H.P.; Pichl, L.; Tachikawa, M.; Kimura, M. Role of the electric dipole moment in positron binding to the ground and excited states of the BeO molecule. J. Chem. Phys. 2007, 126, 104305. [Google Scholar] [CrossRef] [PubMed]
- Kalemos, A. The nature of the chemical bond in BeO0,-, BeOBe+,0,-, and in their hydrogenated products HBeO0,-, BeOH, HBeOH, BeOBeH+,0,-, and HBeOBeH. J. Chem. Phys. 2017, 29, 104307. [Google Scholar] [CrossRef]
- Tennyson, J.; Noble, C.J. RESON: For the automatic detection and fitting of Breit-Wigner resonances. Comput. Phys. Commun. 1984, 33, 421. [Google Scholar] [CrossRef]
- Rabadán, I.; Sarpal, B.K.; Tennyson, J. On the calculation of electron impact rotational excitation cross sections for molecular ions. J. Phys. B At. Mol. Opt. Phys. 1998, 31, 2077. [Google Scholar] [CrossRef]
- Rabadán, I.; Tennyson, J. ROTIONS: A program for the calculation of rotational excitation cross sections in electron molecular ion collisions. Comput. Phys. Commun. 1998, 114, 129. [Google Scholar] [CrossRef]


| States | Present Calculation | Previous Work Ref. [9] | Buenker et al. | Buenker et al. VEE | Kalemos |
|---|---|---|---|---|---|
| X | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
| 1 | 0.72 | 1.43 | 1.05 | 1.25 | 0.93 |
| 2 | 0.78 | 1.50 | 1.24 | 1.47 | 1.03 |
| 3 | 1.93 | 2.73 | 2.22 | 2.28 | 2.17 |
| 4 | 2.41 | 3.01 | 2.58 | 2.58 | 2.56 |
| 5 | 4.47 | 5.19 | 4.70 | 4.98 | 4.63 |
| 6 | 4.92 | 5.70 | – | 5.33 | 4.95 |
| 7 | 4.95 | 5.73 | 5.14 | 5.63 | 5.12 |
| 8 | 4.94 | 5.74 | – | – | 5.14 |
| 9 | 6.22 | 6.86 | – | – | 5.74 |
| Symmetry | |||
|---|---|---|---|
| Below state | |||
| 0.00328 | 3.3399(−03) | 3.0752 | |
| 0.01243 | 5.5263() | 3.2176 | |
| 0.04940 | 1.8623() | 4.0955 | |
| 0.05385 | 4.5688() | 4.2573 | |
| 0.07063 | 8.7957() | 5.1041 | |
| 0.02258 | 6.1004() | 3.4014 | |
| 0.04504 | 2.0100() | 3.9536 | |
| 0.04795 | 5.6762() | 4.0468 | |
| 0.05403 | 7.2960() | 4.2644 | |
| 0.06846 | 8.6172() | 4.9656 | |
| 0.01232 | 4.9394() | 3.2158 | |
| 0.05241 | 8.8364() | 4.2023 | |
| 0.06346 | 8.1198() | 4.6853 | |
| 0.07412 | 1.1718() | 5.3527 | |
| 0.02258 | 3.0147() | 3.3456 | |
| 0.04504 | 4.2167() | 3.7276 | |
| 0.04795 | 1.4491() | 3.9529 | |
| 0.05403 | 1.4659() | 4.1795 | |
| 0.06846 | 1.1719() | 4.5947 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mukherjee, N.; Bhattacharyya, A.; Chakrabarti, K. R-Matrix Calculation of Electron Collision with the BeO+ Molecular Ion. Atoms 2024, 12, 2. https://doi.org/10.3390/atoms12010002
Mukherjee N, Bhattacharyya A, Chakrabarti K. R-Matrix Calculation of Electron Collision with the BeO+ Molecular Ion. Atoms. 2024; 12(1):2. https://doi.org/10.3390/atoms12010002
Chicago/Turabian StyleMukherjee, Nilanjan, Abhijit Bhattacharyya, and Kalyan Chakrabarti. 2024. "R-Matrix Calculation of Electron Collision with the BeO+ Molecular Ion" Atoms 12, no. 1: 2. https://doi.org/10.3390/atoms12010002
APA StyleMukherjee, N., Bhattacharyya, A., & Chakrabarti, K. (2024). R-Matrix Calculation of Electron Collision with the BeO+ Molecular Ion. Atoms, 12(1), 2. https://doi.org/10.3390/atoms12010002





