1. Introduction
The Earth’s radiation belt (ERB), a region where energetic charged particles (with energy from tens of keV to hundreds of MeV) are stably trapped by the geomagnetic field, is located above an altitude ~1000 km, extending up to 7–8 Earth’s radii. However, in the vicinity of the South Atlantic Anomaly (SAA), it drops to heights of a few hundreds of km. The structure of ERB depends on the types of particles and their energy. The keV-energy electrons in the radiation belt form inner and outer zones interconnected by a slot region of very low fluxes.
The ERB particles can impact on the ionosphere causing additional ionization of atmospheric gases. Satellite studies show that energetic electrons (tens to hundreds of keV) of ERB play an important role in these processes at all latitudes. At high and middle latitudes, the impact is caused by electrons precipitating from the outer ERB during geomagnetic disturbances. Similarly, electron precipitations occur inside the area of SAA. The effects of particle precipitations in these regions have been studied in detail experimentally and theoretically for several decades [
1,
2,
3,
4,
5]. A relatively new result in this field is the phenomenon of penetration of energetic electrons from the inner ERB to low altitudes outside the SAA area, i.e., in low-latitude regions far beyond the SAA [
6,
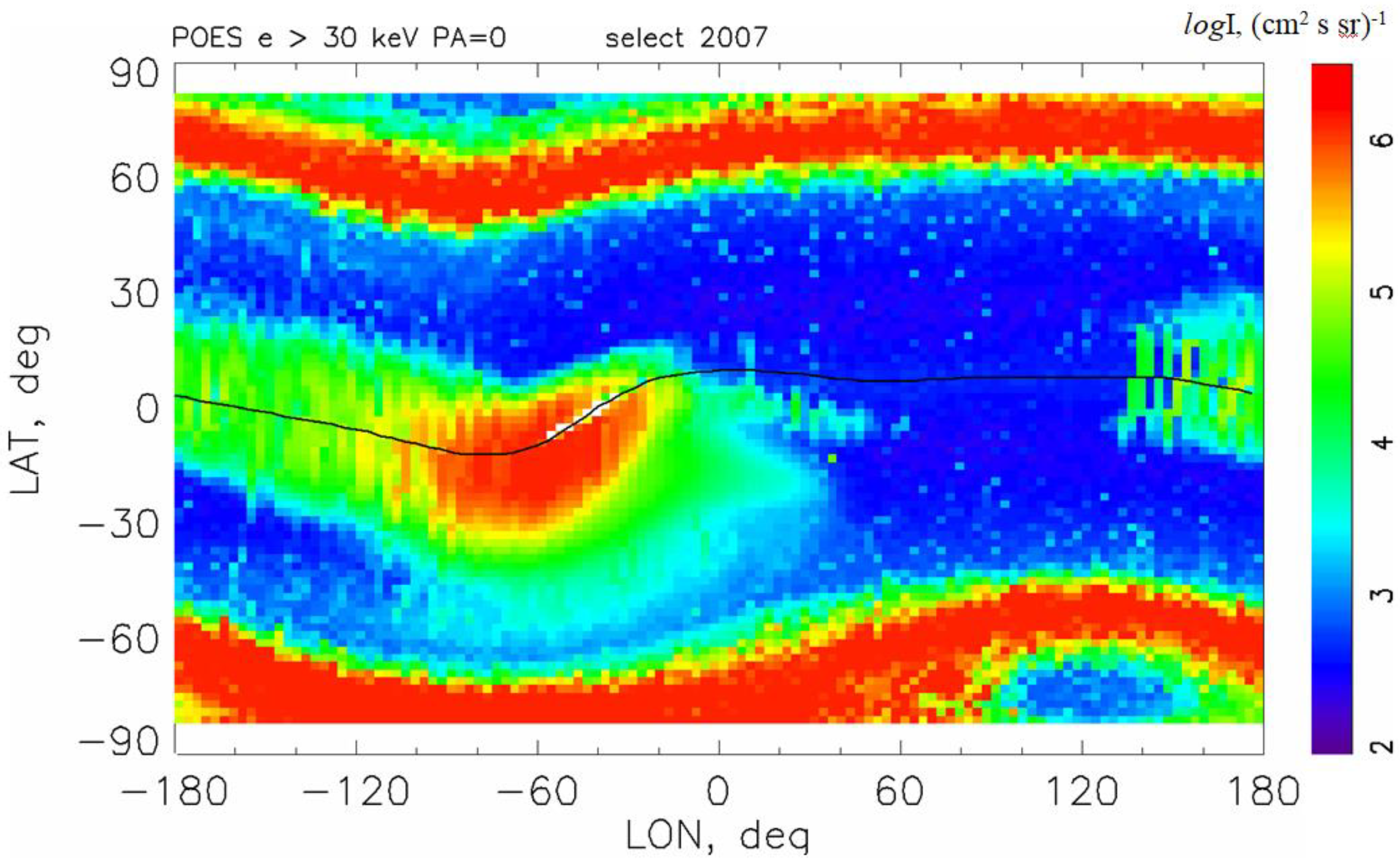
7]. This low-latitude region is known as a forbidden zone of quasi-trapped energetic particles, namely, the forbidden zone is an area of weak electron fluxes in the range of latitudes from 40° S to 40° N and longitudes from 0° to 180° E and from 100° W to 180° W (see
Figure 1). The experimental evidences of the ionization effect of >30 keV electrons injected into the forbidden zone were presented in [
7,
8,
9,
10,
11].
In the forbidden zone, the electrons are quasi-trapped because, drifting eastward across the geomagnetic field, they reach the SAA in less than 20 h, where they descend to altitudes below 100 km, which leads to their thermalization due to energy losses for ionization. The phenomenon of flux increase of quasi-trapped electrons in the forbidden zone is called forbidden energetic electrons (FEE) enhancement [
12]. It was proved that the source of these electrons is the inner ERB [
12]. However, the mechanism of their transport to low altitudes has not yet been fully studied. Analysis of the experimental data from NOAA/POES satellites showed that the mechanism is the rapid radial ExB transport toward the Earth, which can operate due to a strong westward electric field [
7]. The statistical study of FEE enhancements found unusual regularities in their occurrence on long and short time scales such as annual, seasonal, and local time variations [
13]. An explanation of these features can help in understanding how the electric field can penetrate to low latitudes at low altitudes.
Here, we present a comprehensive study of the solar-cycle variation of quasi-trapped electrons with energies >30 keV using continuous low-orbit satellite observations over a 25-year period from 1998 to 2022. The study extends and significantly enriches the statistical analyses of FEE events presented in previous works [
13,
14]. We use enhanced statistics by adding the data during the rising phase of the 25th solar cycle (2020–2022). The method of experimental data treatment and analysis is described in
Section 2. The results of analysis are presented in
Section 3 and discussed in
Section 4.
Section 5 includes the conclusions.
3. Results
Statistics of FEE enhancements were compared with solar activity variations. Solar activity is characterized by the number of sunspots provided by WDC-SILSO (Royal Belgian Observatory, Brussels). The time period from 1998 to 2023 (25 years) covers the 23rd solar cycle from 1998 to 2008, the 24th solar cycle from 2009 to 2019, and the rising phase of the 25th solar cycle from 2020 to 2022. Hence, the data set acquired from the POES and MetOp satellites includes two solar maxima and two solar minima.
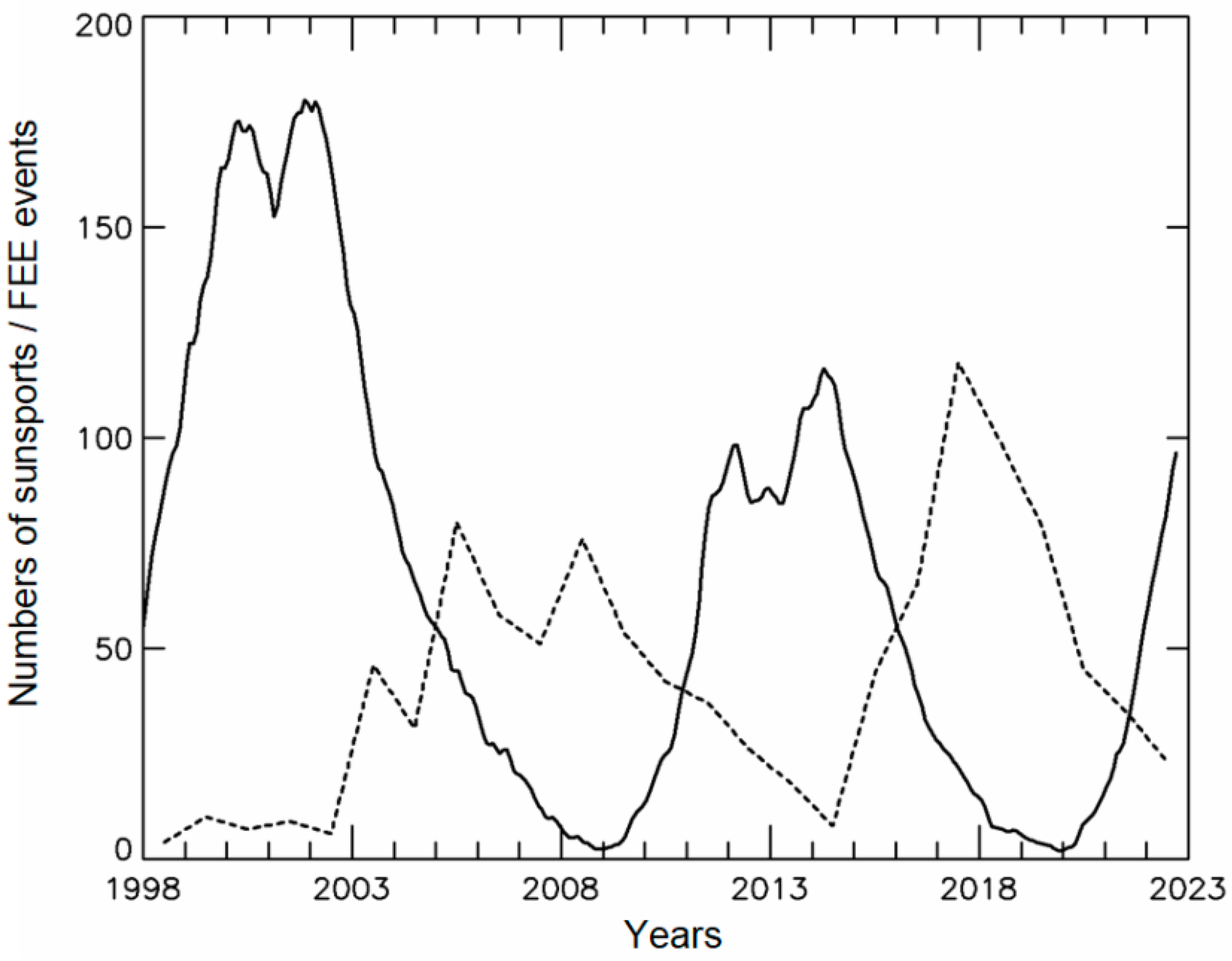
Figure 3 shows variations in the occurrence number of FEE events per year and the yearly-smoothed monthly sunspot number over 25 years. The power of the solar cycle is determined by the sunspot number in the solar maximum. The last two cycles of the 24th and 25th are weaker in power to the 23rd. The time period under study begins in the middle of the rising phase of the 23rd cycle (1998–1999), during which few events were detected (less than 10 per year). Taking into account the fact that only one satellite was operating at that time (see
Table 1), the number of events is most likely underestimated. For the rest of the interval (from 2000 to 2022), the reliability of the data is well supported statistically.
From a comparison of the electron flux dynamics in the rising phases of the last two weak cycles, 24 and 25, we see that the annual number of events decreases from ~50 to ~30–40. In the solar maxima (2000–2001, 2013–2014), the frequency of events continues to fall, reaching a minimum number of about 10 events; the same trend is clearly visible in 2022, near the maximum of the 25th solar cycle. In the beginning of the declining phase of cycles 23 and 24 (2002 and 2014), the occurrence number of FEE events again increases rapidly over 3 years, reaching its highest values approximately after the middle of the declining phase (2005–2006 and 2017), and then begins to decrease over an interval of 8 years, including phases of minimum (2008 and 2019), rising, and maximum of solar cycles. At the same time, in the weak 24th cycle in 2017, there is an absolute maximum in the number of events over the entire period of 25 years.
It was previously shown in [
14,
18] that electron fluxes reach a very high intensity of more than 10
7 (cm
2 s sr)
−1 during the declining phase of the 23rd and 24th cycles, where in the stronger 23rd cycle there were many more FEE events with extremely high fluxes—that is, the events in the declining phase of the 23rd cycle occurred, although less frequently, but in terms of intensity, on average, they exceeded the events in the weaker 24th cycle.
The result indicating a clear deficit in the number of electron injections during the maximum phase of solar cycles requires additional consideration. Previous results of the statistical analysis of the solar wind physical parameters during three cycles from 20 to 23 showed that two parameters, the Alfven Mach number (
MA) and the plasma beta
β, clearly anticorrelate with the sunspot number: at the solar maximum, the number of
MA and
β decrease, and at the solar minimum, they increase [
19]. The data on interplanetary parameters are available at
https://cdaweb.gsfc.nasa.gov (accessed on 15 August 2023). In the current work, we use these two parameters together with others such as the interplanetary magnetic field strength (IMF)
B and the solar wind density
D, velocity
V, and temperature
T, which vary with solar cycles in different ways [
19]. Another physical parameter of solar activity is 10.7 cm radio flux (the
F10.7 index), which is commonly accepted as an indicator of the solar far ultraviolet emission affecting the ionization of the Earth’s ionosphere. It is well known that
F10.7 strongly correlates with the sunspot number.
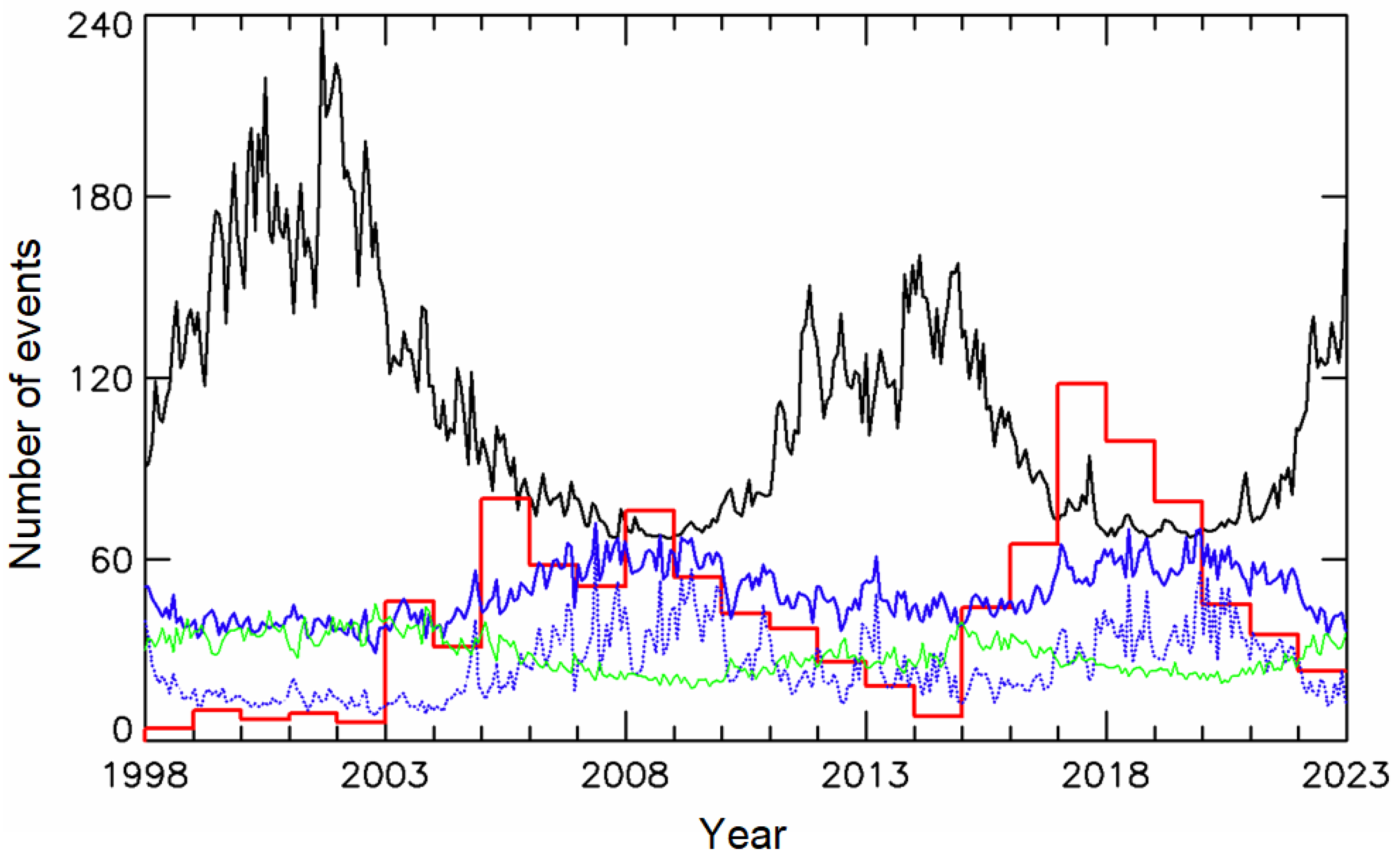
Figure 4 shows solar-cycle variations of the monthly averages of the
MA number, plasma
β, and
F10.7 index. The average annual and monthly parameters of solar activity and solar wind are taken from the OMNI2 database [
20].
We are also testing two more parameters that relate to the interaction between the solar wind and the magnetosphere. These parameters depend on the compression ratio between the densities of the solar wind and magnetosheath at the bow shock
C =
D/
Dms and the local reconnection rate
Rd at the dayside magnetopause. They are calculated as functions of the solar wind parameters [
21]. As shown in [
21], the compression ratio
C depends on
MA and is expressed as follows (Equation (7) in [
21]):
The dayside local reconnection rate
Rd has a dependence on the solar wind and magnetosheath parameters, and the clock angle between the magnetic field directions in the magnetosheath and the dayside magnetosphere at the nose magnetopause. For simplicity, we have considered the case of antiparallel magnetic fields, which gives a factor equal to 1. The dayside local reconnection rate
Rd is expressed as follows (cf. Equation (8) in [
21]):
In the study, a function of
MA is used:
As one can see in
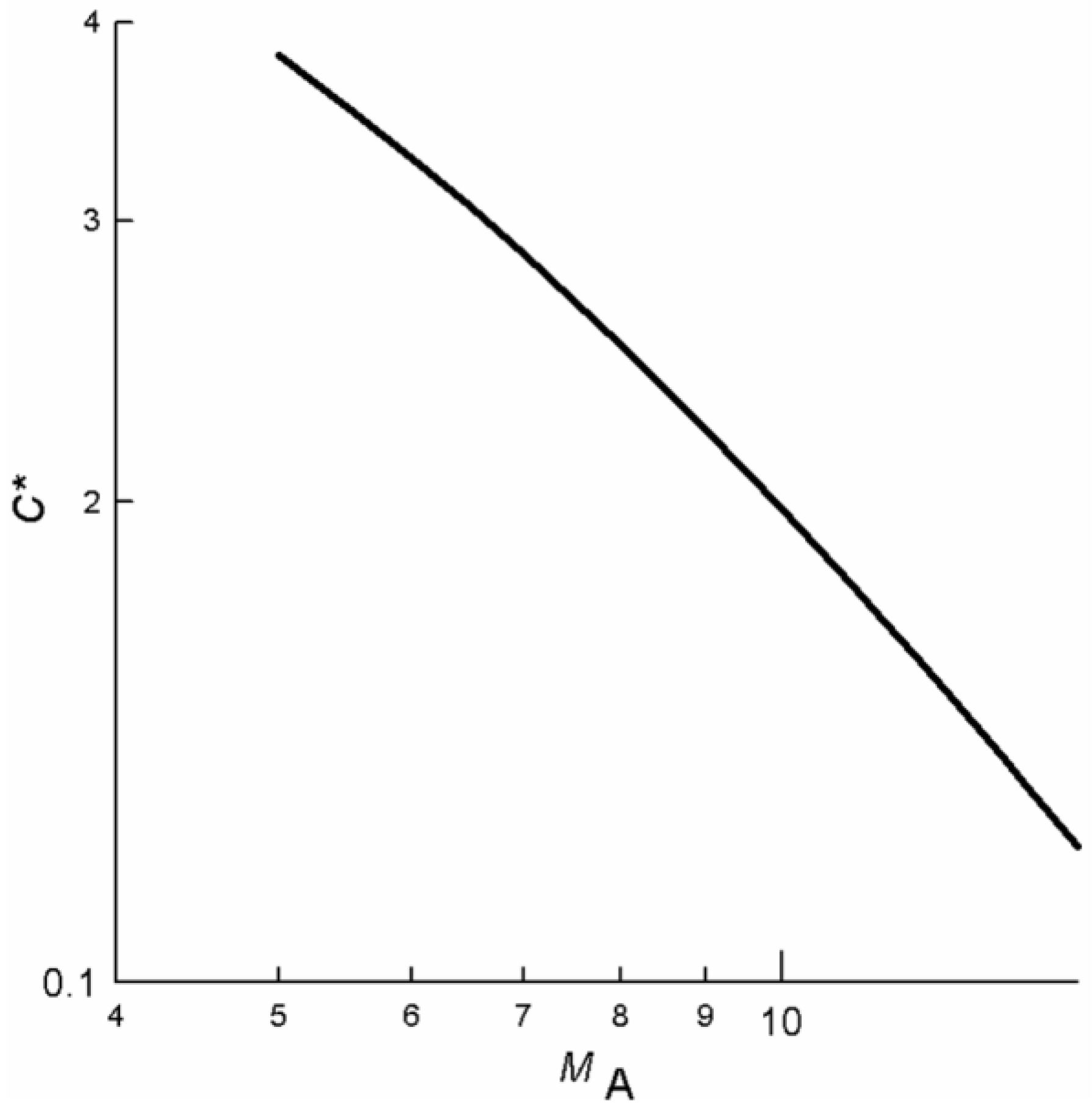
Figure 5, the function
C* ratio has complicated nonlinear dependence on
MA in the dynamic range of
MA from 7 to 12, which is typical for average solar wind conditions during the 23rd and 24th solar cycles.
The annual averages of solar activity and solar wind parameters
MA,
β,
D,
V,
T,
B, and
F10.7, together with the estimated driver functions
C* and
Rd, are used for correlation with the annual occurrence number of FEE events. Linear
Y =
A +
B·
X and power log
Y =
A +
B·log
X dependences between the FEE and the various parameters are applied in the time interval from 2000 to 2022.
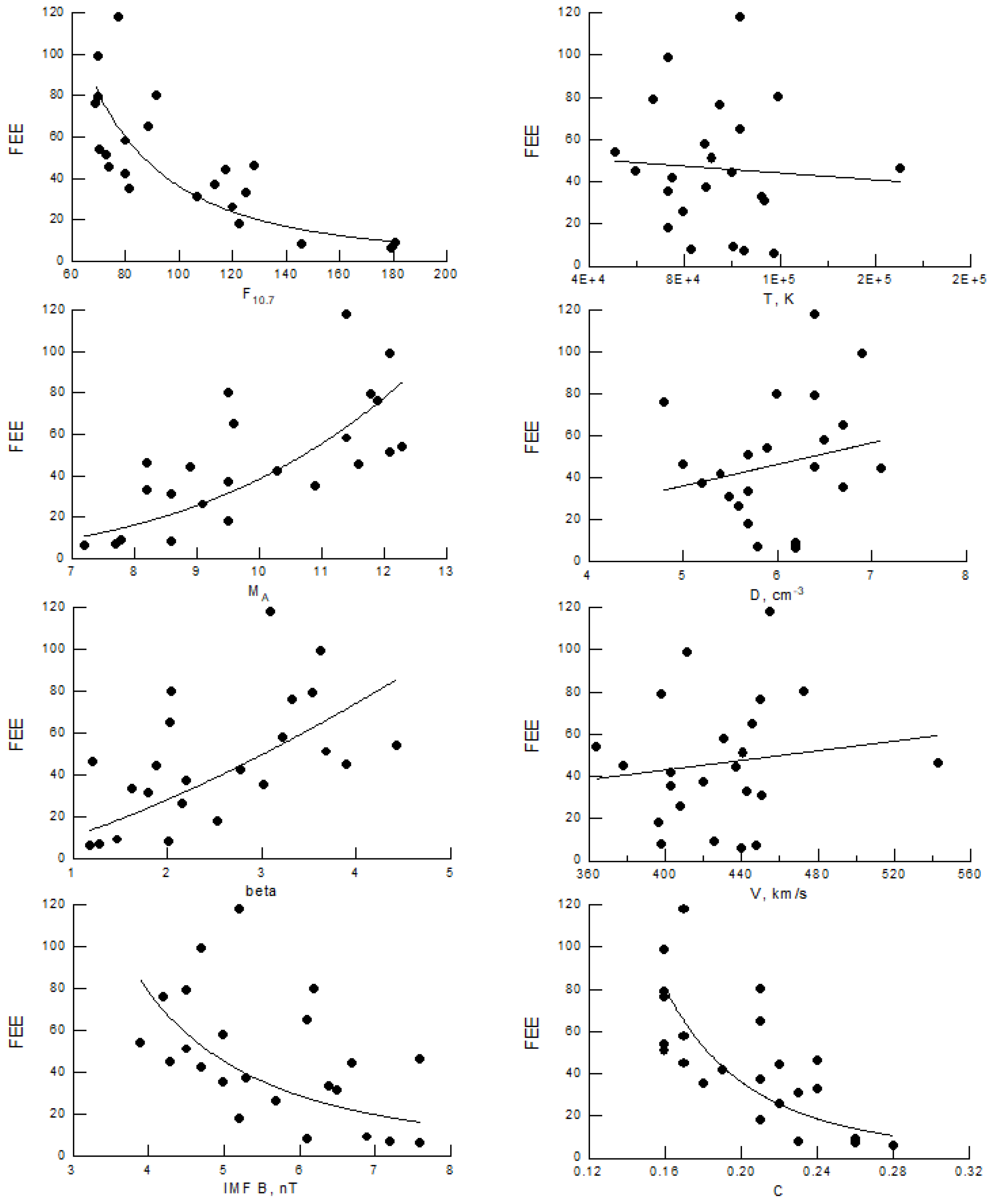
Figure 6 shows scatter plots with the best fits. The correlation coefficients are listed in
Table 2. One can see a very poor correlation between FEE and
D,
V, and
T. However, combinations of these parameters, when used in expressions for
MA,
β, and
C*, show clear power-law dependences. Linear regressions for five parameters
MA,
β,
B,
F10.7, and
C* give good correlation coefficients. The relation between the FEE occurrence and the reconnection rate
Rd is weak. Multi-parametric regression analysis for combinations (
F10.7,
MA,
β,
B), (
C*,
Rd), and (
B,
D,
V,
T) gives total correlation coefficients 0.94, 0.88, and 0.88, respectively. Hence, the best fit is found for the following dependence:
where
. It should be noted that the annual values of parameters in the regression (Equation (4)) are characterized by high pair correlations (see
Table 2) and their variance inflation factors (VIF) are higher than 10. The high correlations are explained on the one hand by the fact that physical parameters
MA and
β depend on
B:
MA~1/
B and
β~1/
B2. On the other hand, the solar parameter
F10.7 is absolutely independent from all other parameters in the physical sense. The large VIF for
F10.7 can be explained by the annual averaging such that the solar cycle variations dominate in the dynamics of solar and interplanetary parameters. The pair correlations between the parameters diminish for short-term daily or hourly variations.
In the present study, the technique of multi-parametric regression is used mainly for estimation of the qualitative dependencies (correlation/anticorrelation) on the driving parameters, which have a deep physical meaning. For instance, the strong anticorrelation between FEE and F10.7 can be associated with the fact that the F10.7 index controls the ionization and, hence, conductivity of the dayside ionosphere. The physical relations of the FEE with the driving parameters will be discussed in the next section. Due to the small statistics, we use the criterion of chi-square (χ2) for the validation of the results presented. For the regression in Equation (4), the parameter chi-square is equal to χ2 = 7.4, which corresponds to a 99% goodness of fit for the regression of four variables and 23 data points.
We obtained that the set of physical parameters including the F10.7 index, the MA number, the plasma β, and IMF B strongly influence the annual FEE occurrence number, while an influence of processes related to a dayside reconnection (Rd) during the southward IMF is weak. We emphasize an important role of the solar radio flux F10.7, which has a high anticorrelation with the FEE occurrence.
4. Discussion
The effect of solar activity on the penetration of >30 keV electrons into the forbidden zone was studied in [
13,
14]. The accumulation of statistics allows drawing more definite conclusions for the rising phase of solar cycles. In general, 25 years of satellite measurements made it possible to reveal the following pattern in the solar-cycle variation of FEE fluxes: the occurrence number of events increases during the first 3 years of the declining phase, then decreases over the next 8 years and reaches its minimum in the solar maximum.
Previous studies reported that the intensity of FEE fluxes decreased in the 24th solar cycle, perhaps due to noticeable weakening of the interplanetary and geomagnetic sources of ERB electrons [
14,
18]. At the same time, the peak occurrence number increased substantially in the weak 24th cycle. This fact can be explained by an increase in the number of operating satellites from 4 to 6 (see
Table 1) and by an increase in the number of single short-term (~15 min) FEE enhancements with a low intensity in the 24th solar cycle in comparison with the 23rd one—that is, injections of >30 keV electrons into the forbidden zone, although not so intense, continued even when solar wind drivers and, accordingly, geomagnetic activity weakened. The latter circumstance possibly indicates a change in the internal conditions associated with the Earth’s magnetic field at low latitudes, as discussed in detail in [
22,
23].
An analysis of the distribution of quasi-trapped electrons at low latitudes at an altitude of 850 km [
13] gave reliable confirmation of the mechanism of radial transport from the inner ERB. We believe that the main agent in this mechanism is the induced westward electric field in the post-midnight sector because it is in the sector of 2–6 LT that the highest probability of electron injections was found [
13]. According to a rough estimate [
7], this requires an electric field strength of at least 2 mV/m. Other researchers simulated the observed injection during a strong magnetic storm using a simplified model of the effect of an enhanced convection electric field on the inner ERB and obtained a field value of 5 mV/m [
24,
25,
26]. Note that the model calculations were limited by altitudes above 2000 km, i.e., within the inner ERB region. However, which electrodynamic process leads to the appearance of sufficiently strong (several mV/m) convection electric field at heights less than 2000 km is still an open question. Additionally, note an interesting fact that many FEE events observed in the absence of geomagnetic storms often occurred during the solar minimum [
27]. The authors found that the trigger for such events is local pressure pulses associated with the dynamics of the foreshock in front of the Earth’s bow shock.
On the other hand, the study by Ilie et al. [
28] presented a theoretical approach to calculate the inductive electric field, which is generated by a localized change in the magnetic field. It is important to note that the effect of the inductive electric field is global—that is, it extends over all space, even if changes in magnetic field are localized and occur at a distance of several Earth radii. Hence, in the near-Earth region, the radial transport of trapped particles into the forbidden zone can be produced by a strong azimuthal inductive electric field. A huge variety of disturbing parameters of solar wind can explain FEE enhancements occurring during both geomagnetic activity and quiet time. Disturbances in the geomagnetic filed can be generated by numerous different variations of solar wind parameters. As for experiments to measure the electric fields in the inner magnetosphere, Burke and Maynard [
29] showed that measurable strong electric fields can penetrate in the equatorial plane at least to the distance of two Earth’s radii; however, they noted that the penetration may be extended beyond the range of reliable measurements by the instruments. Thus, there is still no evidence of strong electric fields at altitudes below 2000 km due to limitations of experimental techniques.
The present study shows a clear anticorrelation of FEE occurrence with the solar cycle (
W &
F10.7 indices). This dynamic is similar to the solar cycle variation of galactic cosmic rays (GCR) as reported in [
30]. Previous studies showed that the interaction of GCR with the atmosphere produces high-energy (~200–800 keV) electrons via a β-decay of albedo neutrons [
31]. Those electrons can be trapped near the lower edge of the inner ERB. Hence, GCR might be considered as a potential source of FEE. However, the fluxes of albedo neutrons and secondary electrons at equatorial latitudes are much lower (orders of magnitudes) than the fluxes of FEE. Moreover, the latitudinal profile of particles generated in the decay should be positive, i.e., the flux of secondary particles increases with latitude due to a decrease of the cut-off rigidity of incident cosmic rays.
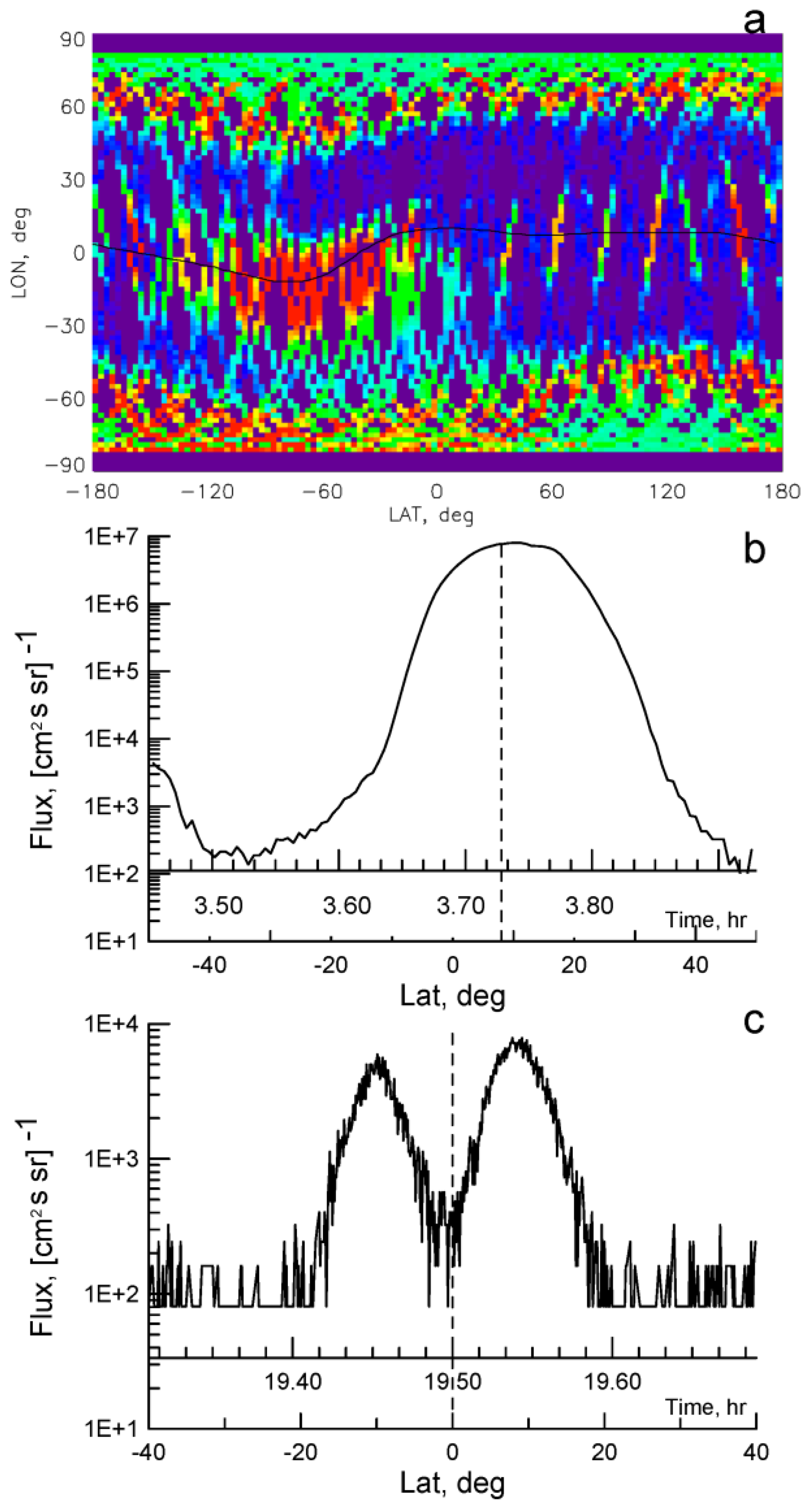
Figure 2 demonstrates totally different pattern for the FEE latitudinal profiles. Finally, the FEE events show clear seasonal and local time variations in the frequency of occurrence [
13] which is not observed in the GRC modulation. Hence, the FEE events cannot be related to the effect of GCR.
In order to explain the minimum occurrence number of FEE events in the solar maximum, we analyzed solar wind parameters, which anticorrelate with the solar cycle. We found two parameters
MA and
β, which demonstrate high correlation with the annual numbers of FEE events. The
MA number is known to be one of the key parameters controlling the effect of solar wind on the magnetosphere; so, geomagnetic activity at high latitudes anticorrelates with
MA [
32]. We believe that this fact can be an important argument in favor of the mechanism of electric field penetration from high latitudes to the equator. This can be understood in the following way. Substorms, as a manifestation of geomagnetic activity at high latitudes, result in enhanced precipitation of auroral particles at night. Note the substorms are characterized by intense precipitations of large scale and long duration, which change dramatically the ionization and conductivity in the high-latitude ionosphere on the night side. High-latitude precipitations also can occur on the day side, in the vicinity of the cusp for example [
33], but they are local in nature with a much lower intensity and short duration that result in a weak influence to the auroral ionosphere.
During the maximum of solar activity, when the
MA number is low and substorm recurrence period is short [
32], the ionization of nighttime ionosphere increases on average. Hence, the enhanced conductivity in the nightside auroral region restrains the penetration of the electric field from high latitudes to the equator. In the opposite case, when
MA is large (solar minimum) or increases (rising and declining phases), the substorm recurrence period is longer [
32]; hence, this condition (the smallest ionization at nighttime, especially in early morning) promotes the penetration of the electric field into low latitudes on the nightside. Thus, the mechanism of the radial transport of the inner ERB electrons into the forbidden zone due to the penetrated electric field effectively operates under the high-
MA-number solar wind and does not under the low-
MA-number. We emphasize the important role of the nighttime ionospheric conductivity in this mechanism.
Previously, the important role of the high-latitude ionospheric conductivity was also found in the seasonal variations of the FEE occurrence rate [
13]. In
Figure 7, one can clearly see a maximal FEE occurrence rate during northern summer periods, from May to September, and a secondary maximum corresponding to southern summer months, from December to February. Study [
13] clearly demonstrated that the seasonal feature in the FEE occurrence rate resulted from the changes of the auroral ionosphere conductivity due to a combination effect of the dipole tilt angle with solar illumination and to the fast solar wind velocity. Note that the higher the solar wind velocity, the greater the
MA number (for fixed values of
D and IMF
B)—that is, in seasonal variations, also a larger value of
MA contributes to the occurrence of FEE events.
Another physical parameter of the solar activity we tested is the 10.7 cm radio flux (the
F10.7 index). As is known, the
F10.7 index correlates with the solar cycle and influences the dayside ionospheric ionization [
34,
35]. We found that FEE occurrence strongly anticorrelates with
F10.7, which is the high occurrence of the annual number of FEE events associated with low annual values of the
F10.7 index. In terms of ionospheric conductivity, it means that during solar minimum the ionization of the ionosphere is significantly lower compared with that in solar maximum. The
F10.7 index controls the ionization and, hence, conductivity of the dayside ionosphere such that the ionization in the northern hemisphere has a maximum in May and June [
35]. The ionospheric ionization and conductivity decrease dramatically in the nighttime, especially in the early morning, and they have a poor correlation with the
F10.7 index [
34]. Apparently, the nightside ionization of the high-latitude ionosphere is controlled by auroral activity, which anticorrelates with
MA.
Thus, a combination of the following factors might create the condition triggering the FEE enhancements: (1) strong illumination and high conductivity of the auroral region, especially during summer months; (2) very low conductivity in the sub-auroral region at night and early morning local time. A greater difference between the day and night side conductivities promotes more effective penetration of perturbations in the electric field at high latitudes into the inner magnetosphere [
13].