Proposition of FSR Photon Suppression Employing a Two-Positron Decay Dark Matter Model to Explain Positron Anomaly in Cosmic Rays
Abstract
1. Introduction
2. Approaches to the Positron Anomaly Solution with Dark Matter
3. Models Used
- A model with a decay of a scalar DM particle into two positronsaccording to Lagrangianwith an accompanying decay of a DM particle into two positrons and an FSR photon
- and a more conventional model, to be compared with, with a decay of a scalar DM particle into an electron and a positronaccording to Lagrangianrespectively accompanied by a decay
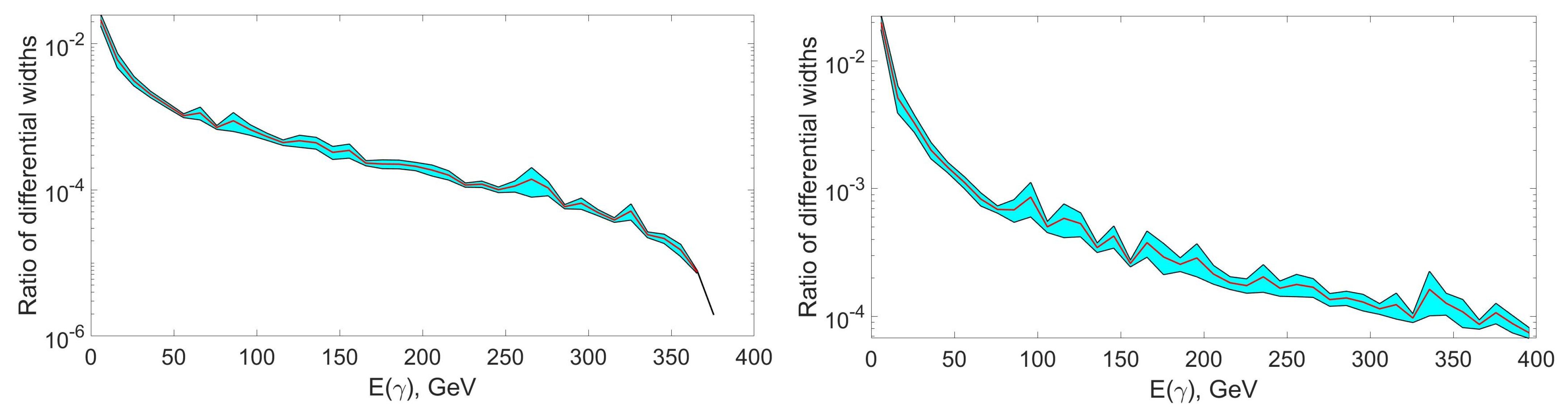
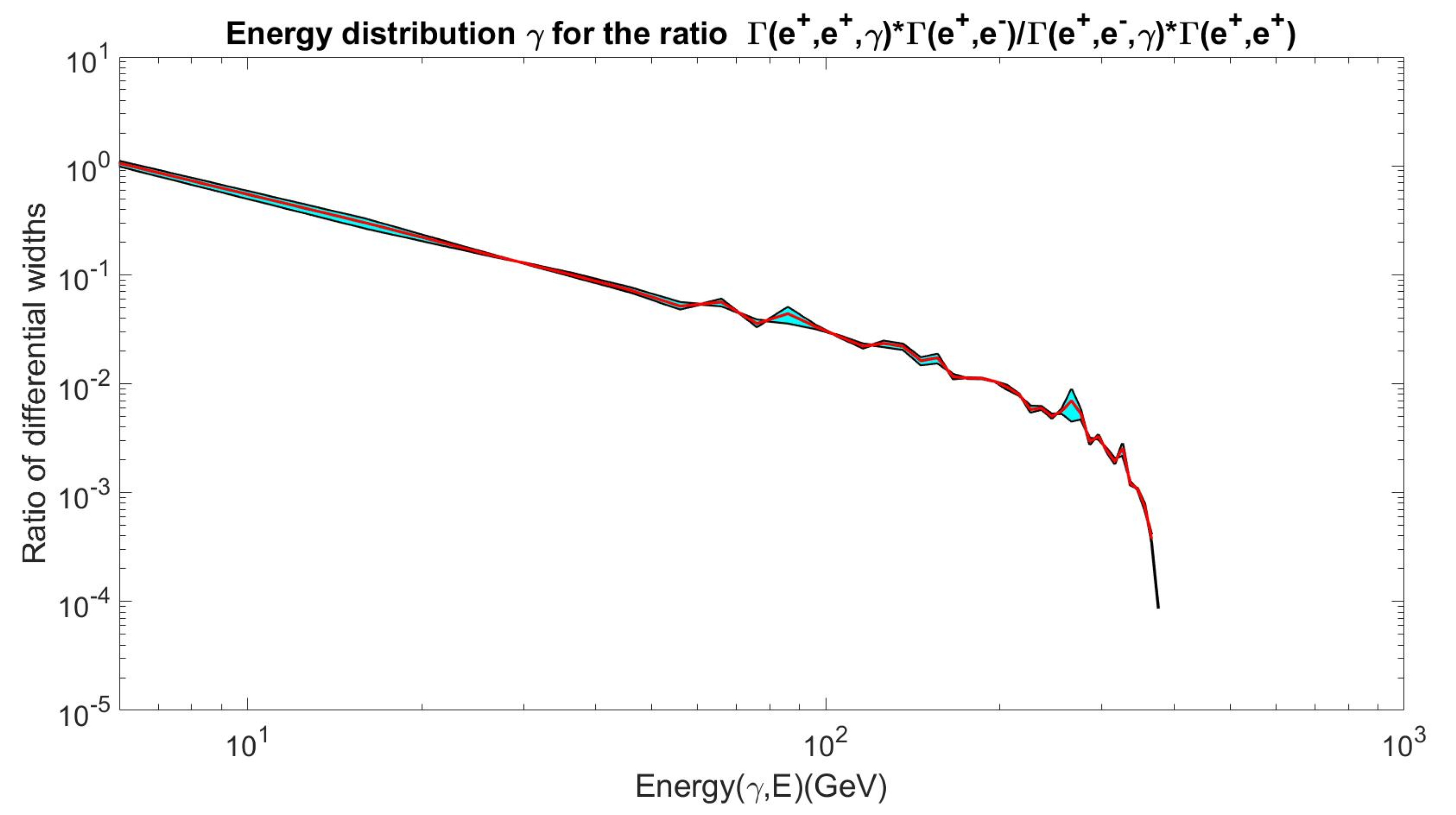
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| 1 | The size and binding energy of such an atom is defined by the mass value of each component, which is, as a rule, required to be TeV for PA explanation. |
References
- Adriani, O.; Barbarino, G.C.; Bazilevskaya, G.A.; Bellotti, R.; Boezio, M.; Bogomolov, E.A.; Bonechi, L.; Bongi, M.; Bonvicini, V.; Bottai, S.; et al. An anomalous positron abundance in cosmic rays with energies 1.5–100 GeV. Nature 2009, 458, 607–609. [Google Scholar] [CrossRef] [PubMed]
- Aguilar, M.; Alberti, G.; Alpat, B.; Alvino, A.; Ambrosi, G.; Andeen, K.; Anderhub, H.; Arruda, L.; Azzarello, P.; Bachlechner, A.; et al. First result from the Alpha Magnetic Spectrometer on the International Space Station: Precision measurement of the positron fraction in primary cosmic rays of 0.5–350 GeV. Phys. Rev. Lett. 2013, 110, 141102. [Google Scholar] [CrossRef]
- Ackermann, M.; Ajello, M.; Allafort, A.; Atwood, W.B.; Baldini, L.; Barbiellini, G.; Bastieri, D.; Bechtol, K.; Bellazzini, R.; Berenji, B.; et al. Measurement of separate cosmic-ray electron and positron spectra with the Fermi Large Area Telescope. Phys. Rev. Lett. 2012, 108, 011103. [Google Scholar] [CrossRef] [PubMed]
- Ambrosi, G.; An, Q.; Asfandiyarov, R.; Azzarello, P.; Bernardini, P.; Bertucci, B.; Cai, M.S.; Chang, J.; Chen, D.Y.; Chen, H.F.; et al. DAMPE collaboration, Direct detection of a break in the teraelectronvolt cosmic-ray spectrum of electrons and positrons. Nature 2017, 552, 63–66. [Google Scholar]
- Hooper, D.; Cholis, I.; Linden, T.; Fang, K. Hawc observations strongly favor pulsar interpretations of the cosmic-ray positron excess. Phys. Rev. D 2017, 96, 103013. [Google Scholar] [CrossRef]
- Profumo, S.; Reynoso-Cordova, J.; Kaaz, N.; Silverman, M. Lessons from HAWC pulsar wind nebulae observations: The diffusion constant is not a constant; pulsars remain the likeliest sources of the anomalous positron fraction; cosmic rays are trapped for long periods of time in pockets of inefficient diffusion. Phys. Rev. D 2018, 97, 123008. [Google Scholar] [CrossRef]
- Linares, M.; Kachelrieß, M. Cosmic ray positrons from compact binary millisecond pulsars. J. Cosmol. Astropart. Phys. 2021, 2021, 030030. [Google Scholar] [CrossRef]
- Orusa, L.; Manconi, S.; Donato, F.; Mauro, M.D. Constraining positron emission from pulsar populations with AMS-02 data. J. Phys. Conf. Ser. 2021, 2156, 012086. [Google Scholar] [CrossRef]
- Belotsky, K.M.; Kamaletdinov, A.K.; Shlepkina, E.S.; Solovyov, M.L. Cosmic Gamma Ray Constraints on the Indirect Effects of Dark Matter. Particles 2020, 3, 336–344. [Google Scholar] [CrossRef]
- Belotsky, K.M.; Esipova, E.A.; Kamaletdinov, A.K.; Shlepkina, E.S.; Solovyov, M.L. Indirect effects of dark matter. Int. J. Mod. Phys. D 2019, 28, 1941011. [Google Scholar] [CrossRef]
- Belotsky, K.; Budaev, R.; Kirillov, A.; Laletin, M. Fermi-LAT kills dark matter interpretations of AMS-02 data. Or not? J. Cosmol. Astropart. Phys. 2017, 2017, 021021. [Google Scholar] [CrossRef][Green Version]
- Belotsky, K.; Kamaletdinov, A.; Laletin, M.; Solovyov, M. The DAMPE excess and gamma-ray constraints. Phys. Dark Universe 2019, 26, 100333. [Google Scholar] [CrossRef]
- Huang, Z.-Q.; Liu, R.-Y.; Joshi, J.C.; Wang, X.-Y. Examining the Secondary Product Origin of Cosmic-Ray Positrons with the Latest AMS-02 Data. Astrophys. J. 2020, 895, 53. [Google Scholar] [CrossRef]
- Yang, F.; Su, M.; Zhao, Y. Dark Matter Annihilation from Nearby Ultra-compact Micro Halos to Explain the Tentative Excess at 1.4 TeV in DAMPE data. arXiv 2017, arXiv:1712.01724. [Google Scholar]
- Cheng, J.-G.; Li, S.; Gan, Y.-Y.; Liang, Y.-F.; Lu, R.-J.; Liang, E.-W. On the gamma-ray signals from UCMH/mini-spike accompanying the DAMPE 1.4 TeV e+e− excess. Mon. Not. R. Astron. Soc. 2020, 497, 2486–2492. [Google Scholar] [CrossRef]
- Ge, S.-F.; He, H.-J.; Wang, Y.-C.; Yuan, Q. Probing Flavor Structure of Cosmic Ray e∓ Spectrum and Implications for Dark Matter Indirect Searches. Nucl. Phys. B 2020, 959, 115140. [Google Scholar] [CrossRef]
- Chen, C.-H.; Chiang, C.-W.; Nomura, T. Dark matter for excess of AMS-02 positrons and antiprotons. Phys. Lett. B 2015, 747, 495–499. [Google Scholar] [CrossRef]
- Diamanti, R.; Lopez-Honorez, L.; Mena, O.; Palomares-Ruiz, S.; Vincent, A.C. Constraining dark matter late-time energy injection: Decays and p-wave annihilations. J. Cosmol. Astropart. Phys. 2014, 2014, 017017. [Google Scholar] [CrossRef]
- Xiang, Q.-F.; Bi, X.-J.; Lin, S.-J.; Yin, P.-F. A dark matter model that reconciles tensions between the cosmic-ray e± excess and the gamma-ray and CMB constraints. Phys. Lett. B 2017, 773, 448–454. [Google Scholar] [CrossRef]
- Fang, K.; Bi, X.-J.; Yin, P.-F. Explanation of the knee-like feature in the dampe cosmic e−+e+ energy spectrum. Astrophys. J. 2018, 854, 57. [Google Scholar] [CrossRef]
- Kachelrieß, M.; Neronov, A.; Semikoz, D.V. Cosmic ray signatures of a 2–3 myr old local supernova. Phys. Rev. D 2018, 97, 063011. [Google Scholar] [CrossRef]
- Blum, K.; Katz, B.; Waxman, E. AMS-02 Results Support the Secondary Origin of Cosmic Ray Positrons. Phys. Rev. Lett. 2013, 111, 211101. [Google Scholar] [CrossRef] [PubMed]
- Tomassetti, N. Cosmic-ray protons, nuclei, electrons, and antiparticles under a two-halo scenario of diffusive propagation. Phys. Rev. D 2015, 92, 081301. [Google Scholar] [CrossRef]
- Kappl, R.; Reinert, A. Secondary Cosmic Positrons in an Inhomogeneous Diffusion Model. Phys. Dark Universe 2017, 16, 71–80. [Google Scholar] [CrossRef][Green Version]
- Aguilar, M.; Cavasonza, L.A.; Ambrosi, G.; Arruda, L.; Attig, N.; Barao, F.; Barrin, L.; Bartoloni, A.; Başeğmez-du Pree, S.; Bates, J.; et al. AMS Collaboration, The Alpha Magnetic Spectrometer (AMS) on the international space station: Part II—Results from the first seven years. Phys. Rep. 2021, 894, 1–116. [Google Scholar] [CrossRef]
- Belotsky, K.M.; Budaev, R.I.; Kirillov, A.A.; Solovyov, M.L. Gamma-rays from possible disk component of dark matter. J. Phys. Conf. Ser. 2017, 798, 012084. [Google Scholar] [CrossRef]
- Alekseev, V.V.; Belotsky, K.M.; Bogomolov, Y.V.; Budaev, R.I.; Dunaeva, O.A.; Kirillov, A.A.; Kuznetsov, A.V.; Laletin, M.N.; Lukyanov, A.D.; Malakhov, V.V.; et al. High-energy cosmic antiparticle excess vs. isotropic gamma-ray background problem in decaying dark matter universe. J. Phys. Conf. Ser. 2016, 675, 012023. [Google Scholar] [CrossRef]
- Alekseev, V.V.; Belotsky, K.M.; Bogomolov, Y.V.; Budaev, R.I.; Dunaeva, O.A.; Kirillov, A.A.; Kuznetsov, A.V.; Laletin, M.N.; Lukyanov, A.D.; Malakhov, V.V.; et al. On a possible solution to gamma-ray overabundance arising in dark matter explanation of cosmic antiparticle excess. J. Phys. Conf. Ser. 2016, 675, 012026. [Google Scholar] [CrossRef]
- Alekseev, V.V.; Belotsky, K.M.; Bogomolov, Y.V.; Budaev, R.I.; Dunaeva, O.A.; Kirillov, A.A.; Kuznetsov, A.V.; Laletin, M.N.; Lukyanov, A.D.; Malakhov, V.V.; et al. Analysis of a possible explanation of the positron anomaly in terms of dark matter. Phys. At. Nucl. 2017, 80, 713–717. [Google Scholar] [CrossRef]
- Belotsky, K.M.; Kirillov, A.A.; Solovyov, M.L. Development of dark disk model of positron anomaly origin. Int. J. Mod. Phys. D 2018, 27, 1841010. [Google Scholar] [CrossRef]
- Solovyov, M.L.; Rakhimova, M.A.; Belotsky, K.M. The “Dark disk” model in the light of DAMPE experiment. In Proceedings of the 23rd Bled Workshop “What Comes Beyond Standard Models?”, Bled, Slovenia, 4–12 July 2020; Volume 21, pp. 156–161. [Google Scholar]
- Belotsky, K.; Khlopov, M.; Kouvaris, C.; Laletin, M. Decaying Dark Atom constituents and cosmic positron excess. Adv. High Energy Phys. 2014, 2014, 1–10. [Google Scholar] [CrossRef]
- Belotsky, K.; Khlopov, M.; Kouvaris, C.; Laletin, M. High-energy positrons and gamma radiation from decaying constituents of a two-component dark atom model. Int. J. Mod. Phys. D 2015, 24, 1545004. [Google Scholar] [CrossRef]
- Khlopov, M.Y. Physics of dark matter in the light of dark atoms. Mod. Phys. Lett. A 2006, 83, 1–4. [Google Scholar] [CrossRef]
- Khlopov, M.Y. Composite dark matter from 4th generation. JETP Lett. 2011, 26, 2823–2839. [Google Scholar]
- Khlopov, M.Y.; Kouvaris, C. Composite dark matter from a model with composite Higgs boson. Phys. Rev. D 2008, 78, 065040. [Google Scholar] [CrossRef]
- Khlopov, M.Y.; Stephan, C.A.; Fargion, D. Dark matter with invisible light from heavy double charged leptons of almost-commutative geometry? Class. Quantum Gravity 2006, 23, 7305. [Google Scholar] [CrossRef]
- Beylin, V.; Khlopov, M.; Kuksa, V.; Volchanskiy, N. Hadronic and Hadron-Like Physics of Dark Matter. Symmetry 2019, 11, 587. [Google Scholar] [CrossRef]
- Li, Y.; Liu, Z.; Xue, Y. XQC and CSR constraints on strongly interacting dark matter with spin and velocity dependent cross sections. J. Cosmol. Astropart. Phys. 2023, 2023, 060. [Google Scholar] [CrossRef]
- Fedderke, M.A.; Graham, P.W.; Rajendran, S. White dwarf bounds on charged massive particles. Phys. Rev. D 2020, 101, 115021. [Google Scholar] [CrossRef]
- Brown, R.W. Understanding something about nothing: Radiation zeros. AIP Conf. Proc. 1995, 350, 261–272. [Google Scholar]
- Boos, E. et al. [the CompHEP Collaboration] CompHEP 4.4—Automatic Computations from Lagrangians to Events. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2004, 534, 250–259. [Google Scholar] [CrossRef]
- Pukhov, A.; Boos, E.; Dubinin, M.; Edneral, V.; Ilyin, V.; Kovalenko, D.; Kryukov, A.; Savrin, V.; Shichanin, S.; Semenov, A. CompHEP—A package for evaluation of Feynman diagrams and integration over multi-particle phase space. User’s manual for version 33. arXiv 2000, arXiv:hep-ph/9908288. [Google Scholar]
- CompHEP Website. Available online: http://comphep.sinp.msu.ru (accessed on 5 September 2021).
- Alwall, J.; Frederix, R.; Frixione, S.; Hirschi, V.; Maltoni, F.; Mattelaer, O.; Shao, H.-S.; Stelzer, T.; Torrielli, P.; Zaro, M. The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations. J. High Energy Phys. 2014, 2014, 1–157. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barak, R.; Belotsky, K.; Shlepkina, E. Proposition of FSR Photon Suppression Employing a Two-Positron Decay Dark Matter Model to Explain Positron Anomaly in Cosmic Rays. Universe 2023, 9, 370. https://doi.org/10.3390/universe9080370
Barak R, Belotsky K, Shlepkina E. Proposition of FSR Photon Suppression Employing a Two-Positron Decay Dark Matter Model to Explain Positron Anomaly in Cosmic Rays. Universe. 2023; 9(8):370. https://doi.org/10.3390/universe9080370
Chicago/Turabian StyleBarak, Ramin, Konstantin Belotsky, and Ekaterina Shlepkina. 2023. "Proposition of FSR Photon Suppression Employing a Two-Positron Decay Dark Matter Model to Explain Positron Anomaly in Cosmic Rays" Universe 9, no. 8: 370. https://doi.org/10.3390/universe9080370
APA StyleBarak, R., Belotsky, K., & Shlepkina, E. (2023). Proposition of FSR Photon Suppression Employing a Two-Positron Decay Dark Matter Model to Explain Positron Anomaly in Cosmic Rays. Universe, 9(8), 370. https://doi.org/10.3390/universe9080370





