Equatorward Moving Auroral Arcs Associated with Impulse-Excited Field Line Resonance
Abstract
1. Introduction
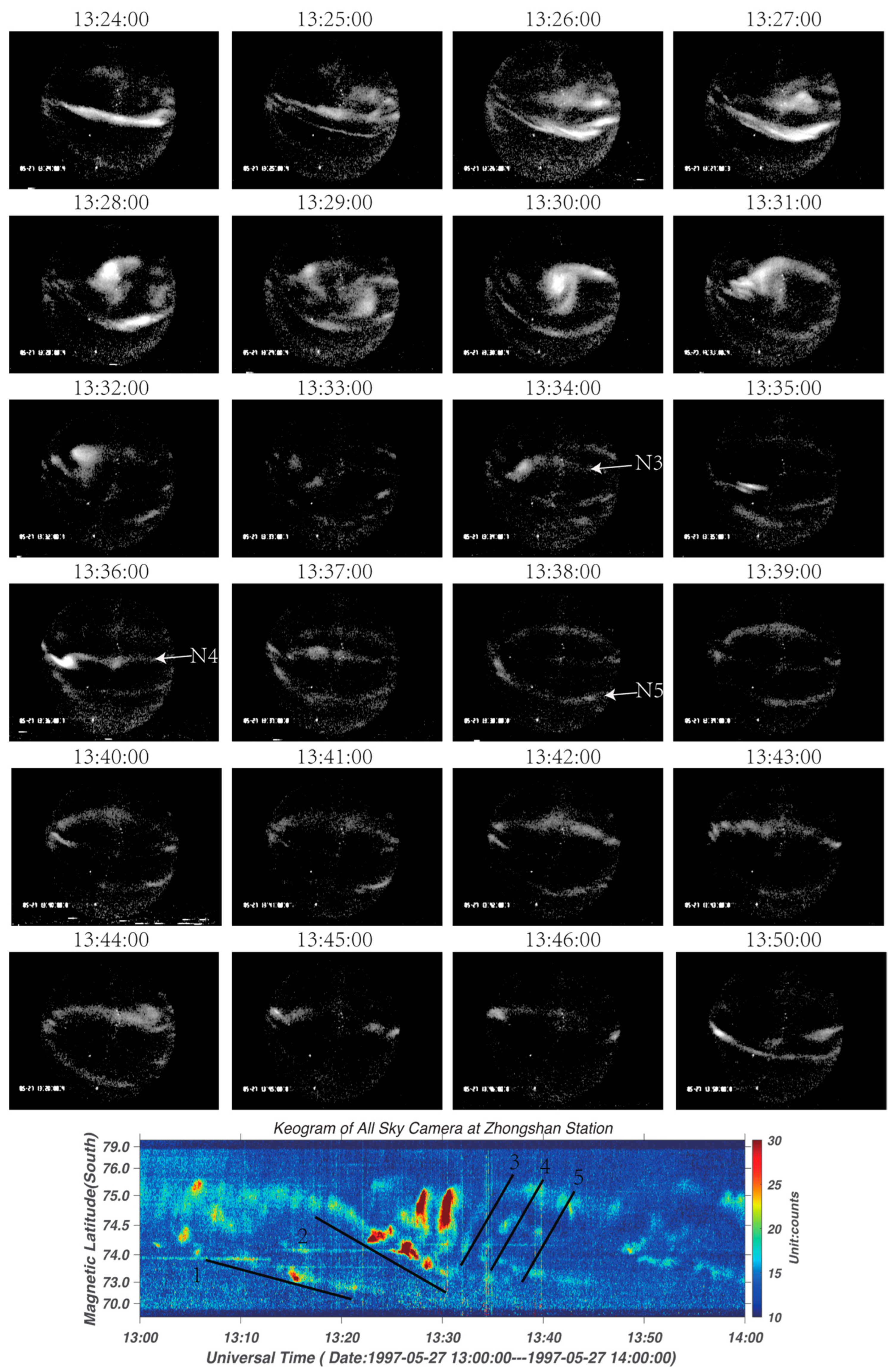
2. Data Presentation
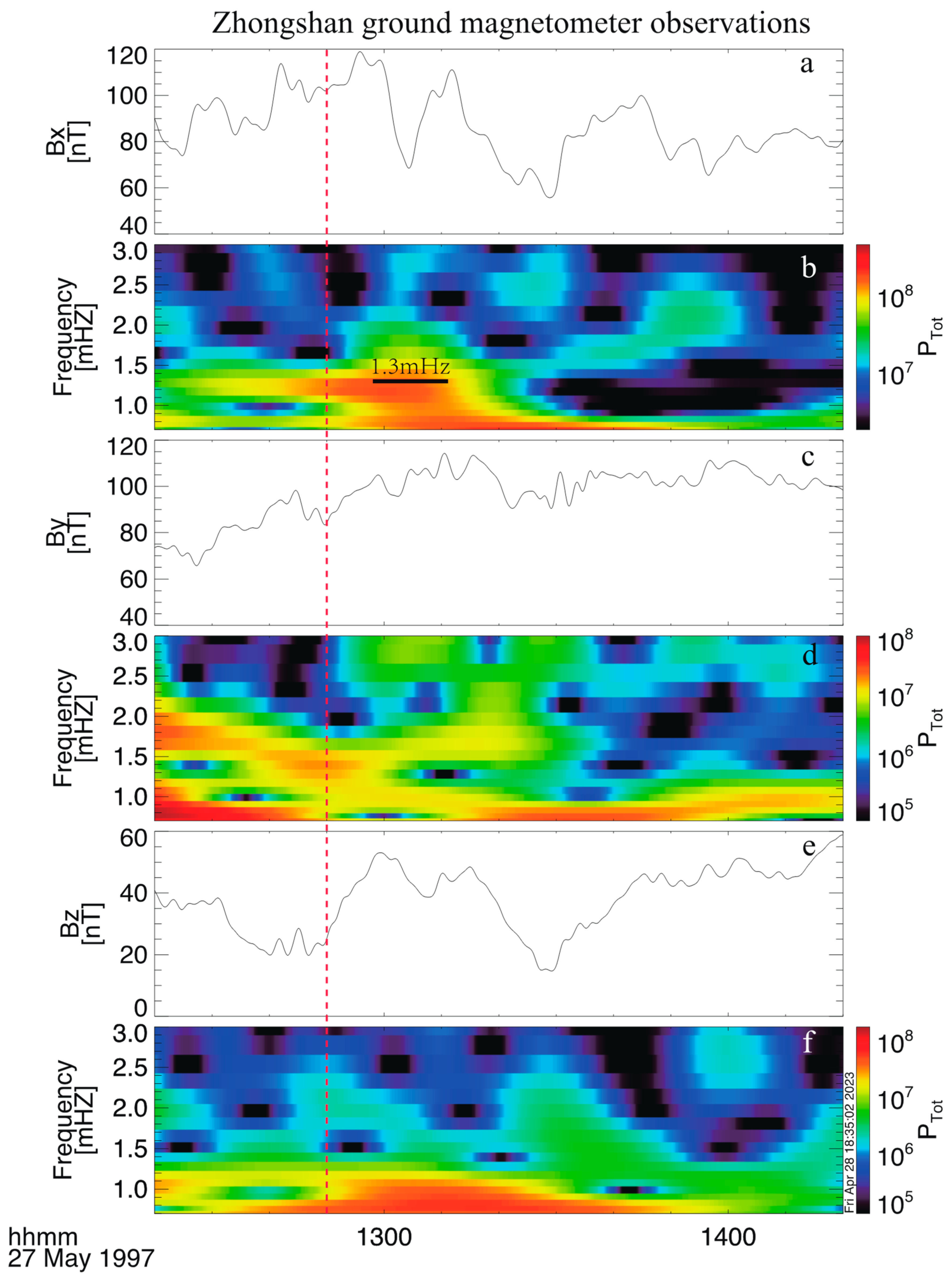
3. Observations
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mathews, J.T.; Mann, I.R.; Rae, I.J.; Moen, J. Multi-instrument observations of ULF wave-driven discrete auroral arcs propagating sunward and equatorward from the poleward boundary of the duskside auroral oval. Phys. Plasmas 2004, 11, 1250–1259. [Google Scholar] [CrossRef]
- Rae, I.J.; Murphy, K.R.; Watt, C.E.J.; Rostoker, G.; Rankin, R.; Mann, I.R.; Hodgson, C.R.; Frey, H.U.; Degeling, A.W.; Forsyth, C. Field line resonances as a trigger and a tracer for substorm onset. J. Geophys. Res. Space Phys. 2014, 119, 5343–5363. [Google Scholar] [CrossRef]
- Baddeley, L.J.; Lorentzen, D.; Partamies, N.; Denig, M.; Pilipenko, V.; Oksavik, K.; Chen, X.; Zhang, Y. Equatorward propagating auroral arcs driven by ULF wave activity: Multipoint ground- and space-based observations in the dusk sector auroral oval. J. Geophys. Res. Space Phys. 2017, 122, 5591–5605. [Google Scholar] [CrossRef]
- Mann, I.R.; Wright, A.N. Diagnosing the Excitation Mechanisms of Pc5 Magnetospheric Flank Waveguide Modes and FLRs. Geophys. Res. Lett. 1999, 26, 16. [Google Scholar] [CrossRef]
- Wright, A.N. Dispersion and wave coupling in inhomogeneous MHD waveguides. J. Geophys. Res. 1994, 99, 159–167. [Google Scholar] [CrossRef]
- Southwood, D.J. Some features of field line resonances in the magnetosphere. Planet. Space Sci. 1974, 22, 483–491. [Google Scholar] [CrossRef]
- Chen, L.; Hasegawa, A. A theory of long-period magnetic pulsations: 2. Impulse excitation of surface eigenmode. J. Geophys. Res. 1974, 79, 1033–1037. [Google Scholar] [CrossRef]
- Southwood, D.J.; Dungey, J.W.; Etherington, R.J. Bounce resonant interaction between pulsations and trapped particles. Planet. Space Sci. 1969, 17, 349–361. [Google Scholar] [CrossRef]
- Hughes, W.; McPherron, R.; Barfield, J. Geomagnetic pulsations observed simultaneously on three geostationary satellites. J. Geophys. Res. 1978, 83, 1109–1116. [Google Scholar] [CrossRef]
- Allan, W.; Poulter, E.M.; Glassmeier, K.-H.; Junginger, H. Spatial and temporal structure of a high-latitude transient ULF pulsation. Planet. Space Sci. 1985, 33, 159–173. [Google Scholar] [CrossRef]
- Zhao, H.Y.; Zhou, X.-Z.; Liu, Y.; Zong, Q.-G.; Rankin, R.; Wang, Y.F.; Shi, Q.Q.; Shen, X.-C.; Ren, J.; Liu, H.; et al. Poleward-moving recurrent auroral arcs associated with impulse-excited standing hydromagnetic waves. Earth Planet. Phys. 2019, 3, 305–313. [Google Scholar] [CrossRef]
- Mann, I.R.; Voronkov, I.; Dunlop, M.; Donovan, E.; Yeoman, T.K.; Milling, D.K.; Wild, J.; Kauristie, K.; Amm, O.; Bale, S.D.; et al. Co-ordinated ground-based and cluster observations of large amplitude global magnetospheric oscillations during a fast solar wind speed interval. Ann. Geophys. 2002, 20, 405. [Google Scholar] [CrossRef]
- Zheng, Y.; Lui, A.T.Y.; Mann, I.R.; Takahashi, K.; Watermann, J.; Chen, S.H.; Rae, I.J.; Mukai, T.; Russell, C.T.; Balogh, A. Coordinated observation of field line rsonance in the mid-tail. Ann. Geophys. 2006, 24, 707–723. [Google Scholar] [CrossRef]
- Newton, R.S.; Southwood, D.J.; Hughes, W.J. Damping of geomagnetic pulsations by the ionosphere. Planet. Space Sci. 1978, 26, 201–209. [Google Scholar] [CrossRef]
- Southwood, D.J.; Hughes, W.J. Theory of hydromagnetic waves in the magnetosphere. Space Sci. Rev. 1983, 35, 301. [Google Scholar] [CrossRef]
- Chen, L.; Hasegawa, A. Kinetic theory of geomagnetic pulsations, 1, Internal excitations by energetic particles. J. Geophys. Res. 1991, 96, 1503–1512. [Google Scholar] [CrossRef]
- Mager, P.N.; Klimushkin, D.Y. Alfvén ship waves: High-m ULF pulsations in the magnetosphere generated by a moving plasma inhomogeneity. Ann. Geophys. 2008, 26, 1653–1663. [Google Scholar] [CrossRef]
- Chisham, G.; Mann, I.R.; Orr, D. A statistical study of giant pulsation latitudinal polarization and amplitude variation. J. Geophys. Res. Atmos. 1997, 102, 9619–9629. [Google Scholar] [CrossRef]
- Rae, I.; Watt, C.; Fenrich, F.R.; Mann, I.R.; Ozeke, L.G.; Kale, A. Energy deposition in the ionosphere through a global field line resonance. Ann. Geophys. 2007, 25, 2529–2539. [Google Scholar] [CrossRef]
- Roldugin, V.C.; Roldugin, A.V. Pc5 pulsations on the ground, in the magnetosphere, and in the electron precipitation: Event of 19 January 2005. J. Geophys. Res. Atmos. 2008, 113, A04222. [Google Scholar] [CrossRef]
- Lotko, W.; Streltsov, A.V.; Carlson, C.W. Discrete auroral arc, electrostatic shock and suprathermal electrons powered by dispersive, anomalously resistive field line resonance. Geophys. Res. Lett. 1998, 25, 4449–4452. [Google Scholar] [CrossRef]
- Rankin, R.; Kabin, K.; Marchand, R. Alfvénic field line resonances in arbitrary magnetic field topology. Adv. Space Res. 2006, 38, 1720–1729. [Google Scholar] [CrossRef]
- Rankin, R.; Watt, C.E.J.; Samson, J.C. Self-consistent wave-particle interactions in dispersive scale long-period field-line-resonances. Geophys. Res. Lett. 2007, 34, L23103. [Google Scholar] [CrossRef]
- Wright, A.N.; Mills, K.J.; Ruderman, M.S.; Brevdo, L. The absolute and convective instability of the magnetospheric flanks. J. Geophys. Res. Atmos. 2000, 105, 385–393. [Google Scholar] [CrossRef]
- Russell, A.J.B.; Wright, A.N. Resonant absorption with 2D variation of field line eigenfrequencies. Astron. Astrophys. 2010, 511, A17. [Google Scholar] [CrossRef]
- Fenrich, F.R.; Samson, J.C.; Sofko, G.; Greenwald, R.A. ULF high- and low-mfield line resonances observed with the Super Dual Auroral Radar Network. J. Geophys. Res. Atmos. 1995, 100, 21535–21547. [Google Scholar] [CrossRef]
- Wright, A.N.; Allan, W. Structure, phase motion, and heating within Alfvén resonances. J. Geophys. Res. Atmos. 1996, 101, 17399–17408. [Google Scholar] [CrossRef]
- Wright, A.N.; Elphinstone, R.D.; Cogger, L.L.; Allan, W. Phase mixing and phase motion of Alfvén waves on tail-like and dipole-like magnetic field lines. J. Geophys. Res. Atmos. 1999, 104, 10159–10175. [Google Scholar] [CrossRef]
- Samson, J.C.; Harrold, B.G.; Ruohoniemi, J.M.; Greenwald, R.A.; Walker, A.D.M. Field line resonances associated with MHD waveguides in the magnetosphere. Geophys. Res. Lett. 1992, 19, 441–444. [Google Scholar] [CrossRef]
- Farrell, W.; Thompson, R.; Lepping, R.; Byrnes, J. A method of calibrating magnetometers on a spinning spacecraft. IEEE Trans. Magn. 1995, 31, 966–972. [Google Scholar] [CrossRef]
- Gloeckler, G.; Balsiger, H.; Bochsler, P.; Fisk, L.A.; Galvin, A.B.; Geiss, J.; Gliem, F.; Hamilton, D.C.; Holzer, T.E.; Hovestadt, D.; et al. The solar WIND and suprathermal ion composition investigation on the WIND spacecraft. Space Sci. Rev. 1995, 71, 79–124. [Google Scholar] [CrossRef]
- Yang, H.; Sato, N.; Makita, K.; Kikuchi, M.; Kadokura, A.; Ayukawa, M.; Hu, H.; Liu, R.; Häggström, I. Synoptic observations of auroras along the postnoon oval: A survey with all-sky TV observations at Zhongshan, Antarctica. J. Atmos. Solar-Terr. Phys. 2000, 62, 787–797. [Google Scholar] [CrossRef]
- Liu, R.-Y.; Liu, Y.-H.; Xu, Z.-H.; Hu, H.-Q.; Yang, H.-G.; Zhang, B.-C.; Xu, W.-Y.; Chen, G.-X.; Wu, J.; Zhen, W.-M.; et al. The Chinese ground-based instrumentation in support of the combined Cluster/Double Star satellite measurements. Ann. Geophys. 2005, 23, 2943–2951. [Google Scholar] [CrossRef]
- Tsyganenko, N.; Stern, D.P. Modeling the global magnetic field of the large-scale Birkeland current systems. J. Geophys. Res. Atmos. 1996, 101, 27187–27198. [Google Scholar] [CrossRef]
- Greenwald, R.A.; Walker, A.D.M. Energetics of long period resonant hydromagnetic waves. Geophys. Res. Lett. 1980, 7, 745–748. [Google Scholar] [CrossRef]
- Milan, S.E.; Sato, N.; Ejiri, M.; Moen, J. Auroral forms and the field-aligned current structure associated with field line resonances. J. Geophys. Res. Atmos. 2001, 106, 25825–25833. [Google Scholar] [CrossRef]
- Sarris, T.E.; Liu, W.; Kabin, K.; Li, X.; Elkington, S.R.; Ergun, R.; Rankin, R.; Angelopoulos, V.; Bonnell, J.; Glassmeier, K.H.; et al. Characterization of ULF pulsations by THEMIS. Geophys. Res. Lett. 2009, 36, L04104. [Google Scholar] [CrossRef]
- Sarris, T.E.; Liu, W.; Li, X.; Kabin, K.; Talaat, E.R.; Rankin, R.; Angelopoulos, V.; Bonnell, J.; Glassmeier, K.-H. THEMIS observations of the spatial extent and pressure-pulse excitation of field line resonances. Geophys. Res. Lett. 2010, 37, L15104. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Liu, Y.; Yang, H.; Zong, Q.; Hu, Z.; Zhou, X.; Wang, Y.; Sun, J.; Li, B. Equatorward Moving Auroral Arcs Associated with Impulse-Excited Field Line Resonance. Universe 2023, 9, 249. https://doi.org/10.3390/universe9060249
Zhao H, Liu Y, Yang H, Zong Q, Hu Z, Zhou X, Wang Y, Sun J, Li B. Equatorward Moving Auroral Arcs Associated with Impulse-Excited Field Line Resonance. Universe. 2023; 9(6):249. https://doi.org/10.3390/universe9060249
Chicago/Turabian StyleZhao, Huayu, Ying Liu, Huigen Yang, Qiugang Zong, Zejun Hu, Xuzhi Zhou, Yongfu Wang, Jicheng Sun, and Bin Li. 2023. "Equatorward Moving Auroral Arcs Associated with Impulse-Excited Field Line Resonance" Universe 9, no. 6: 249. https://doi.org/10.3390/universe9060249
APA StyleZhao, H., Liu, Y., Yang, H., Zong, Q., Hu, Z., Zhou, X., Wang, Y., Sun, J., & Li, B. (2023). Equatorward Moving Auroral Arcs Associated with Impulse-Excited Field Line Resonance. Universe, 9(6), 249. https://doi.org/10.3390/universe9060249







