On the Temperature Dependence of the String-Breaking Distance in QCD
Abstract
1. Introduction
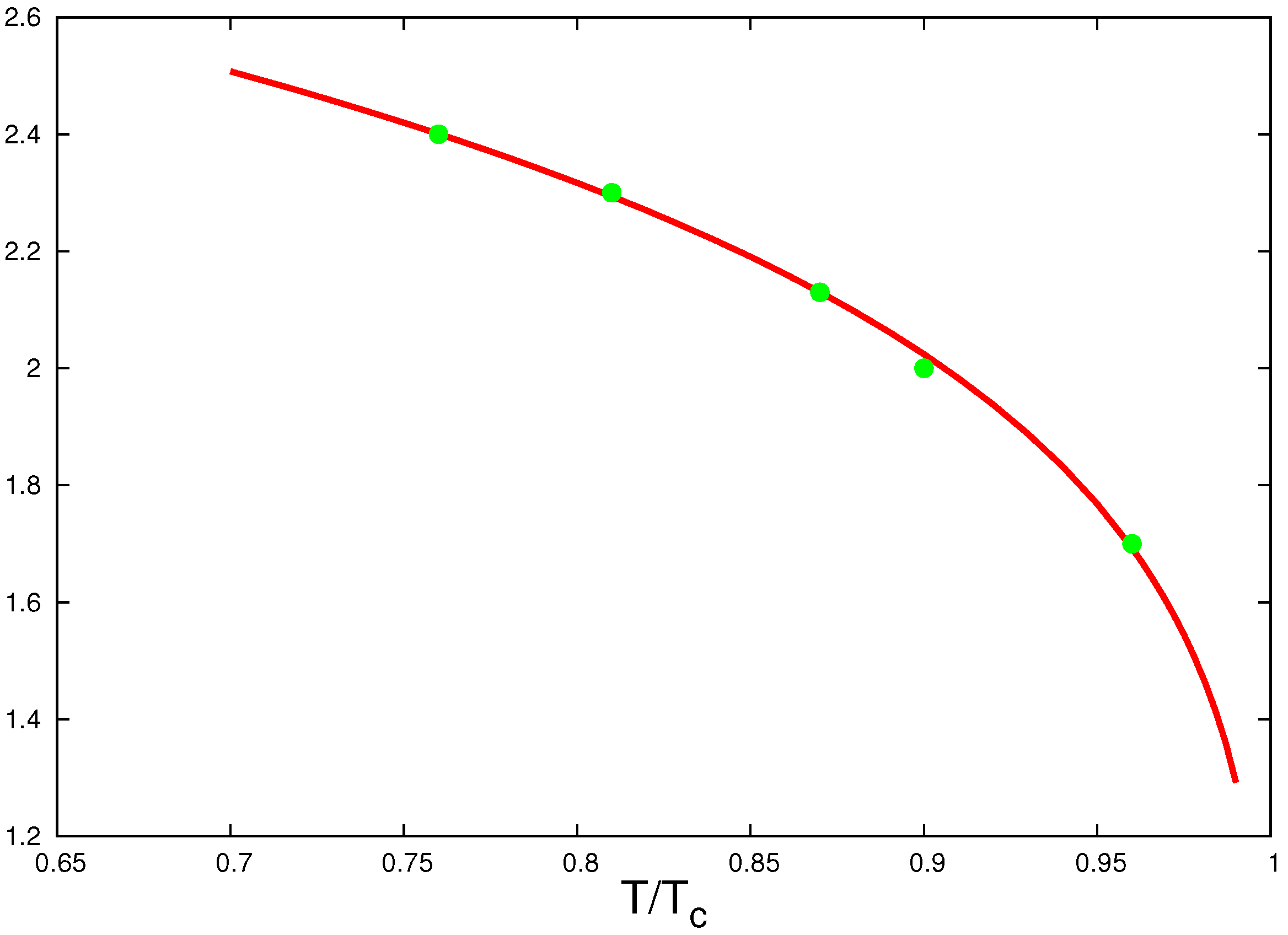
2. Evaluating the String-Breaking Distance and Its Temperature Dependence
3. Summary
Funding
Conflicts of Interest
References
- Bali, S.; Neff, H.; Duessel, T.; Lippert, T.; Schilling, K.; [SESAM Collaboration]. Observation of string breaking in QCD. Phys. Rev. D 2005, 71, 114513. [Google Scholar] [CrossRef]
- Bulava, J.; Hörz, B.; Knechtli, F.; Koch, V.; Moir, G.; Morningstar, C.; Peardon, M. String breaking by light and strange quarks in QCD. Phys. Lett. B 2019, 793, 493–498. [Google Scholar] [CrossRef]
- Satz, H. Charm and beauty in a hot environment. arXiv 2006, arXiv:hep-ph/0602245. [Google Scholar]
- Antonov, D. World-line formalism: Non-perturbative applications. Universe 2016, 2, 28. [Google Scholar] [CrossRef]
- Khokhlachev, S.B.; Makeenko, Y.M. Phase transition over the gauge group center and quark confinement in QCD. Phys. Lett. B 1981, 101, 403–406. [Google Scholar] [CrossRef]
- Greensite, J.; Halpern, M.B. Suppression of color screening at large N. Phys. Rev. D 1983, 27, 2545–2547. [Google Scholar] [CrossRef]
- Svetitsky, B.; Yaffe, L.G. Critical behavior at finite-temperature confinement transitions. Nucl. Phys. B 1982, 210, 423–447. [Google Scholar] [CrossRef]
- Antonov, D. Monopole-based scenarios of confinement and deconfinement in 3D and 4D. Universe 2017, 3, 50. [Google Scholar] [CrossRef]
- Montaña, G.; Ramos, À.; Tolos, L.; Torres-Rincon, J.M. Pseudoscalar and vector open-charm mesons at finite temperature. Phys. Rev. D 2020, 102, 096020. [Google Scholar] [CrossRef]
- Digal, S.; Kaczmarek, O.; Karsch, F.; Satz, H. Heavy-quark interactions in finite-temperature QCD. Eur. Phys. J. C 2005, 43, 71–75. [Google Scholar] [CrossRef]
- Mandelstam, S. Vortices and quark confinement in non-Abelian gauge theories. Phys. Lett. B 1975, 53, 476–478. [Google Scholar] [CrossRef]
- ’t Hooft, G. Topology of the gauge condition and new confinement phases in non-Abelian gauge theories. Nucl. Phys. B 1981, 190, 455–478. [Google Scholar] [CrossRef]
- Ripka, G. Dual Superconductor Models of Color Confinement; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Abrikosov, A.A. On the magnetic properties of superconductors of the second group. J. Exp. Theor. Phys. 1957, 5, 1174–1182. [Google Scholar]
- Nielsen, H.B.; Olesen, P. Vortex-line models for dual strings. Nucl. Phys. B 1973, 61, 45–61. [Google Scholar] [CrossRef]
- Di Giacomo, A.; Maggiore, M.; Olejnik, S. Evidence for flux tubes from cooled QCD configurations. Phys. Lett. B 1990, 236, 199–202. [Google Scholar] [CrossRef]
- Di Giacomo, A.; Maggiore, M.; Olejnik, S. Confinement and chromoelectric flux tubes in lattice QCD. Nucl. Phys. B 1990, 347, 441–460. [Google Scholar] [CrossRef]
- Cea, P.; Cosmai, L.; Papa, A. Chromoelectric flux tubes and coherence length in QCD. Phys. Rev. D 2012, 86, 054501. [Google Scholar] [CrossRef]
- Cea, P.; Cosmai, L.; Cuteri, F.; Papa, A. Flux tubes in the SU(3) vacuum: London penetration depth and coherence length. Phys. Rev. D 2014, 89, 094505. [Google Scholar] [CrossRef]
- Bogomolny, E.B. The stability of classical solutions. Sov. J. Nucl. Phys. 1976, 24, 449–454. [Google Scholar]
- Antonov, D. Calculating non-perturbative quantities through the world-line formalism. J. Phys. Conf. Ser. 2019, 1208, 012005. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics: Non-Relativistic Theory, 3rd ed.; Butterworth-Heinemann: Oxford, UK, 1981. [Google Scholar]
- Drummond, I.T.; Horgan, R.R. Lattice string breaking and heavy-meson decays. Phys. Lett. B 1999, 447, 298–307. [Google Scholar] [CrossRef]
- Pisarski, R.D.; Wilczek, F. Remarks on the chiral phase transition in chromodynamics. Phys. Rev. D 1984, 29, 338–341. [Google Scholar] [CrossRef]
- Rajagopal, K.; Wilczek, F. Static and dynamic critical phenomena at a second-order QCD phase transition. Nucl. Phys. B 1993, 399, 395–425. [Google Scholar] [CrossRef]
- Yagi, K.; Hatsuda, T.; Miake, Y. Quark-Gluon Plasma; Cambridge Univ. Press: Cambridge, UK, 2005; Chapter 6. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Antonov, D. On the Temperature Dependence of the String-Breaking Distance in QCD. Universe 2023, 9, 97. https://doi.org/10.3390/universe9020097
Antonov D. On the Temperature Dependence of the String-Breaking Distance in QCD. Universe. 2023; 9(2):97. https://doi.org/10.3390/universe9020097
Chicago/Turabian StyleAntonov, Dmitry. 2023. "On the Temperature Dependence of the String-Breaking Distance in QCD" Universe 9, no. 2: 97. https://doi.org/10.3390/universe9020097
APA StyleAntonov, D. (2023). On the Temperature Dependence of the String-Breaking Distance in QCD. Universe, 9(2), 97. https://doi.org/10.3390/universe9020097




