Einstein-AdS Gravity Coupled to Nonlinear Electrodynamics, Magnetic Black Holes, Thermodynamics in an Extended Phase Space and Joule–Thomson Expansion
Abstract
:1. Introduction
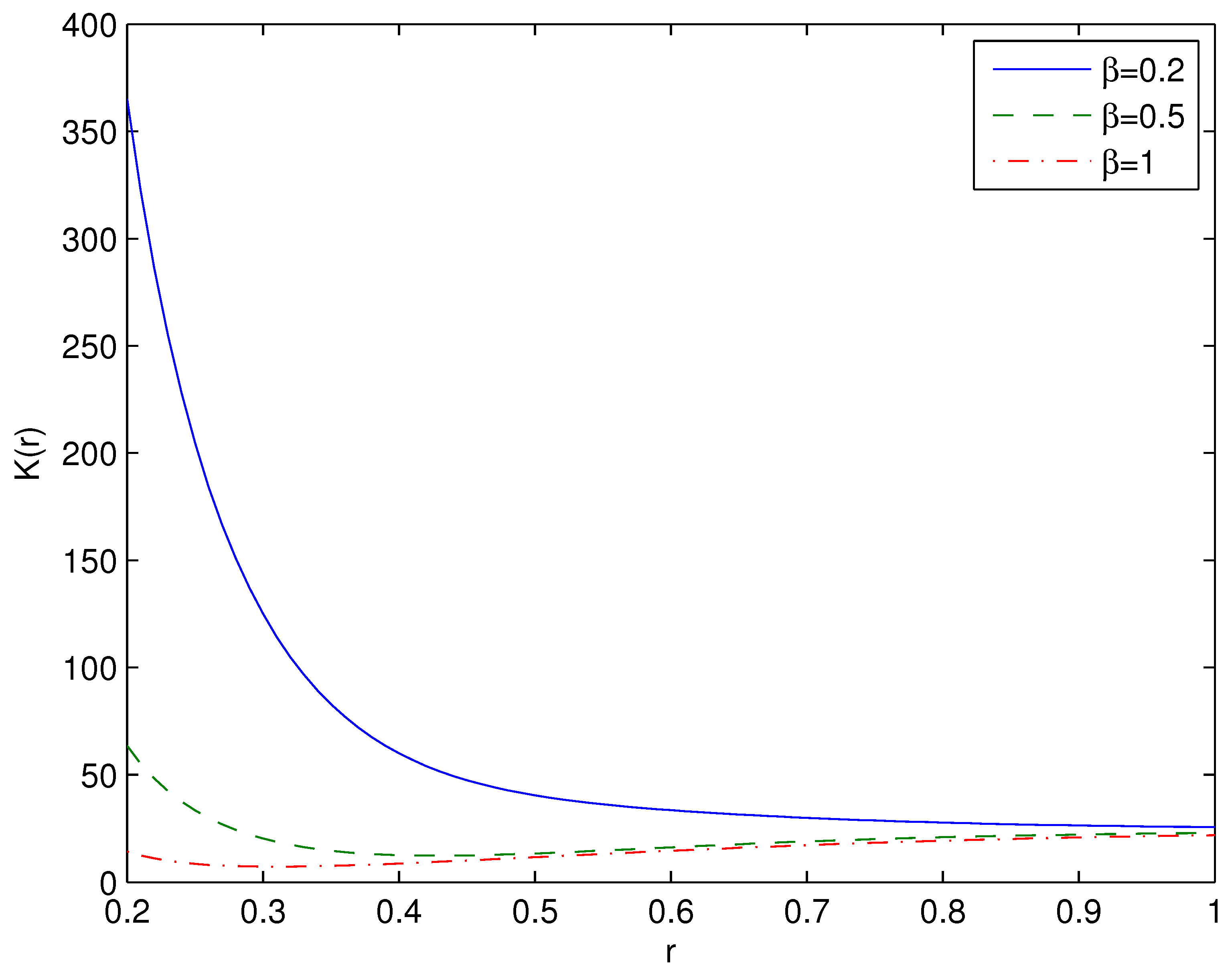
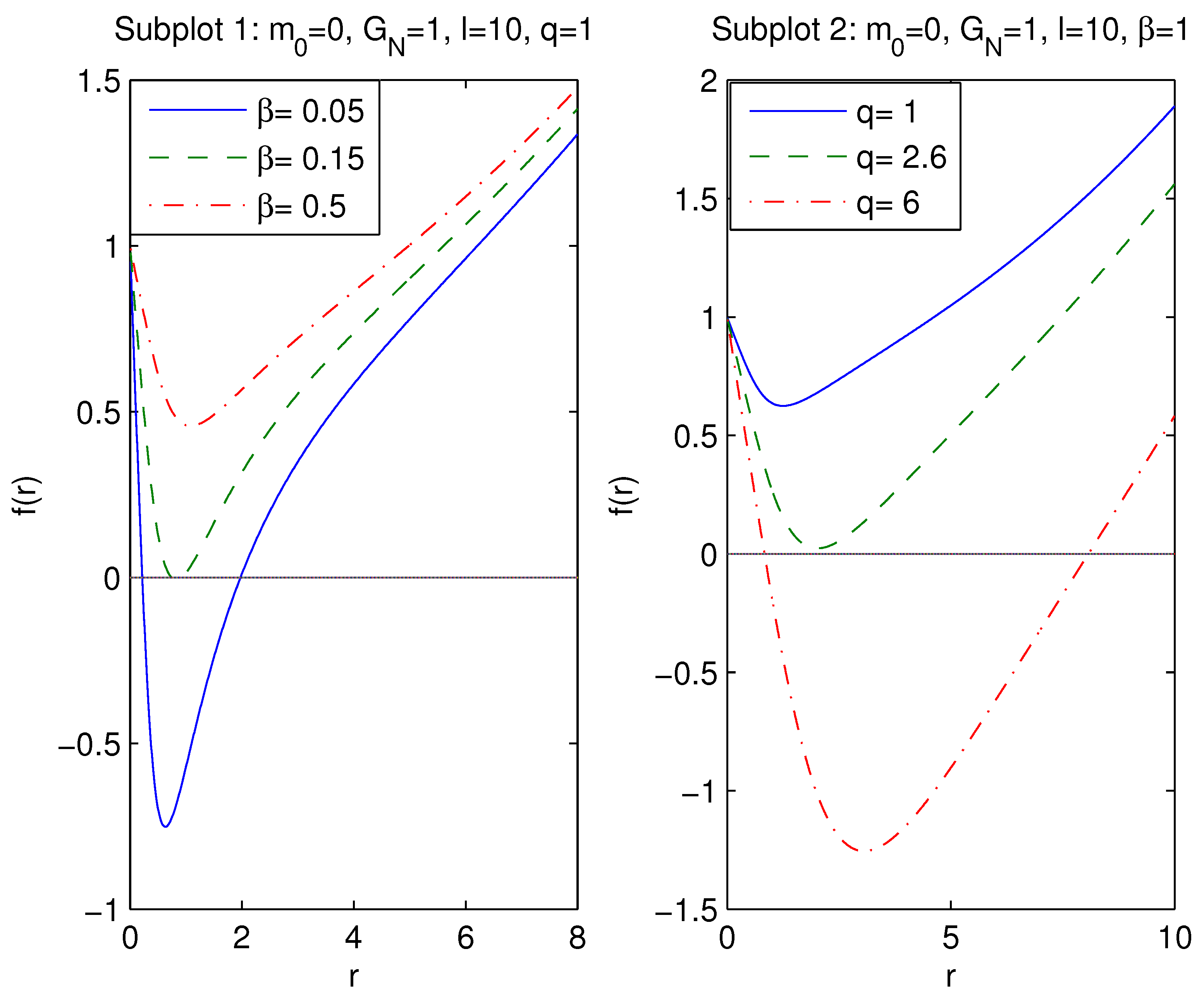
2. Einstein-AdS Black Hole Solution
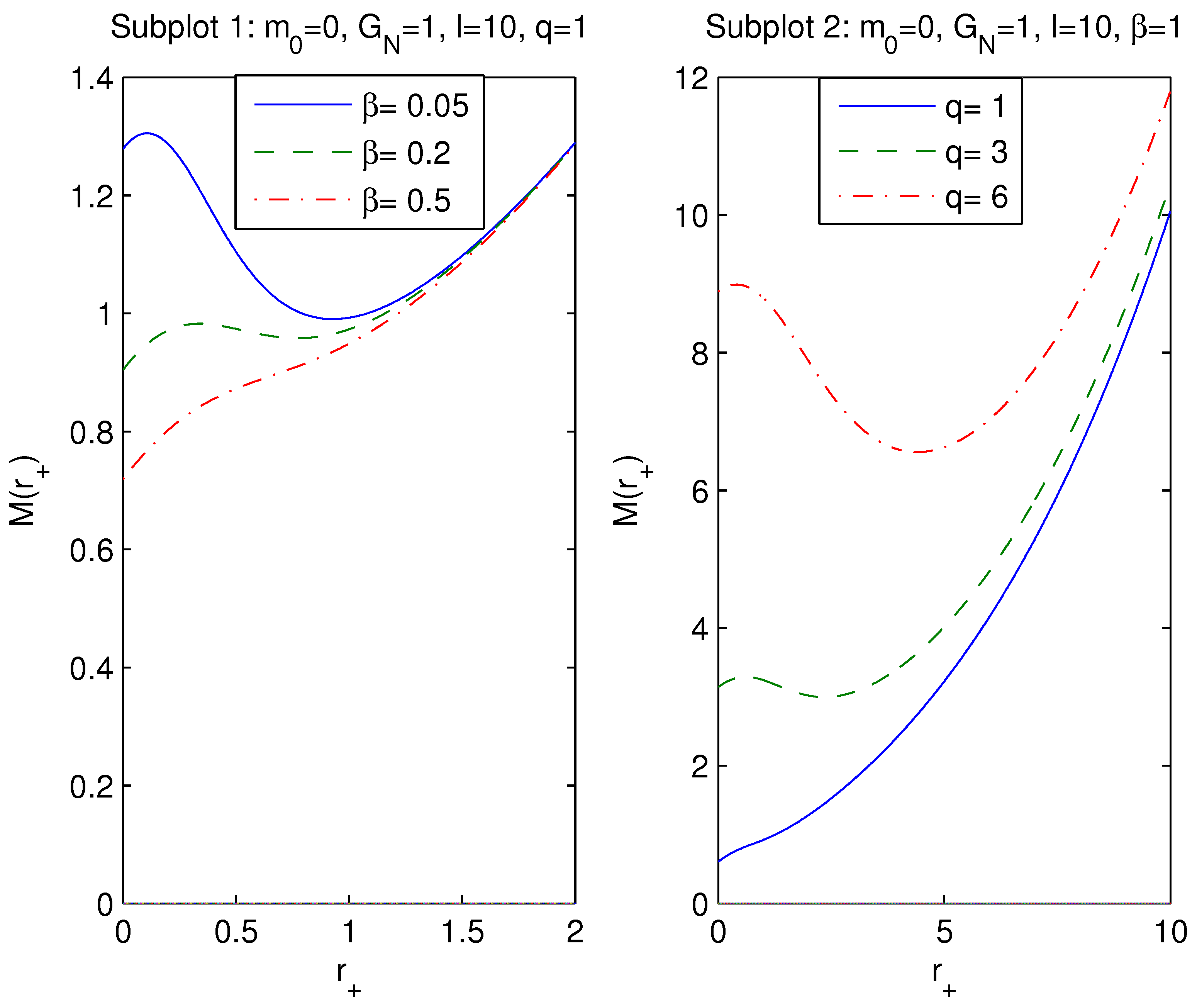
3. First Law of Black Hole Thermodynamics
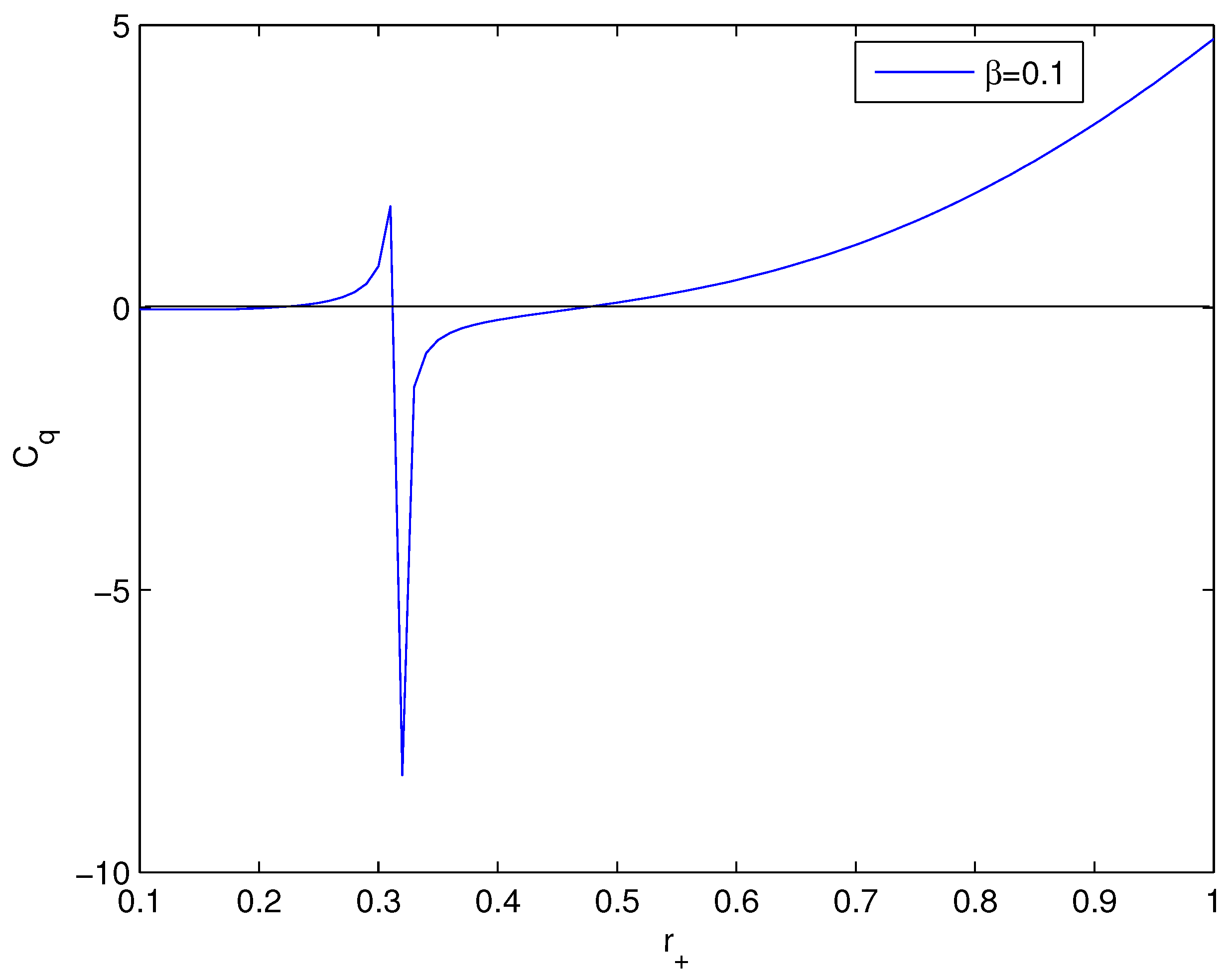
4. Thermodynamics of Black Hole
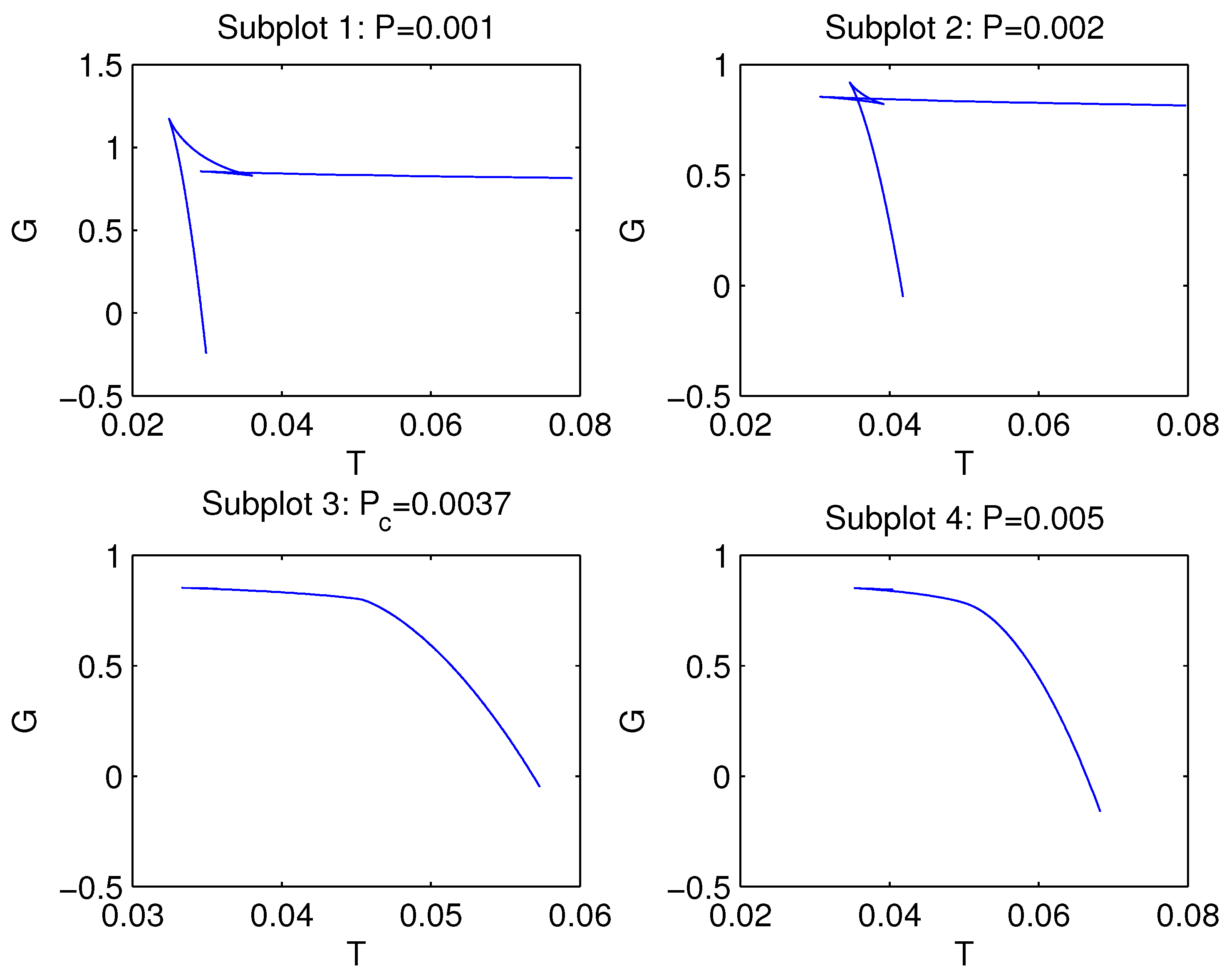
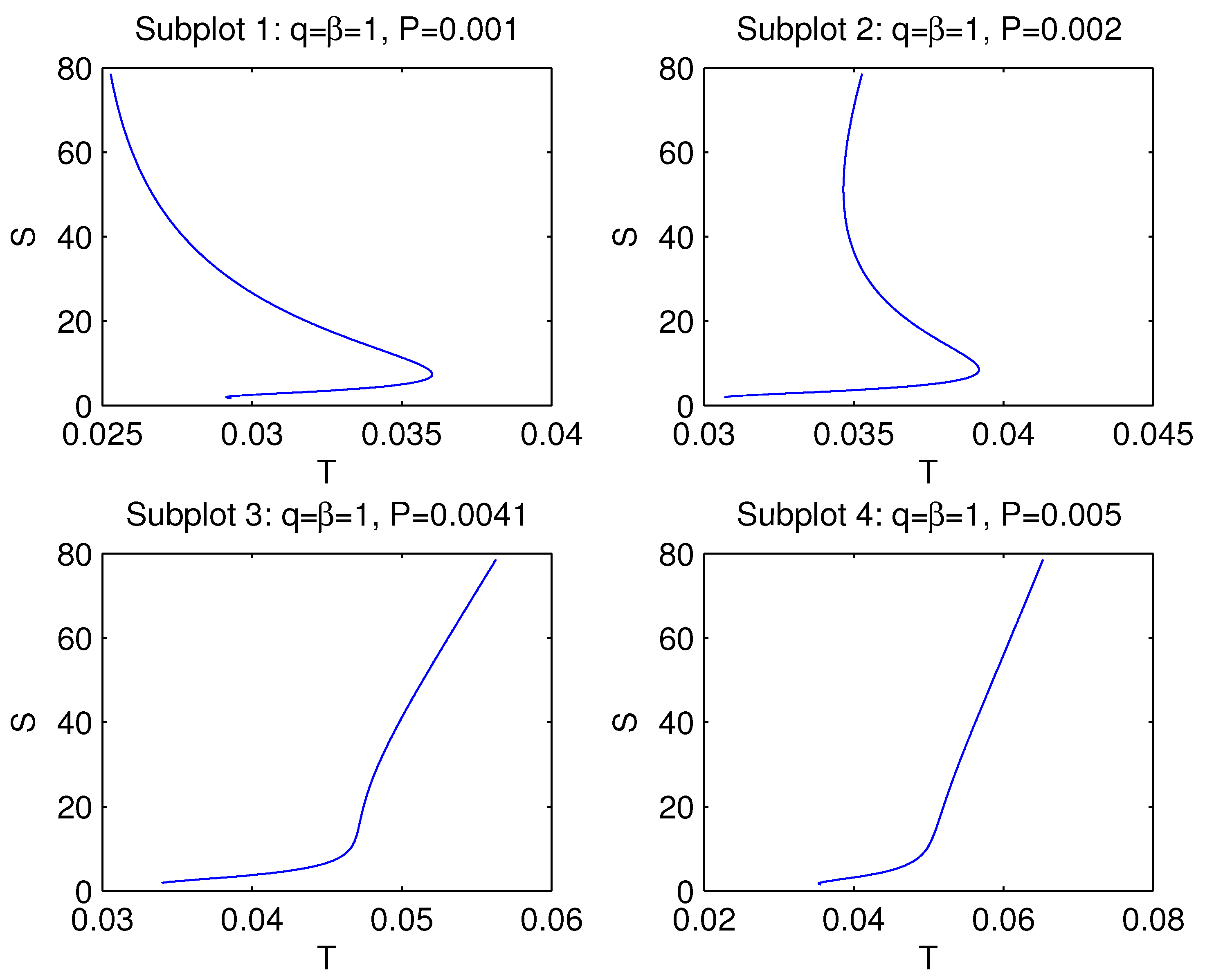
Reentrant Phase Transitions
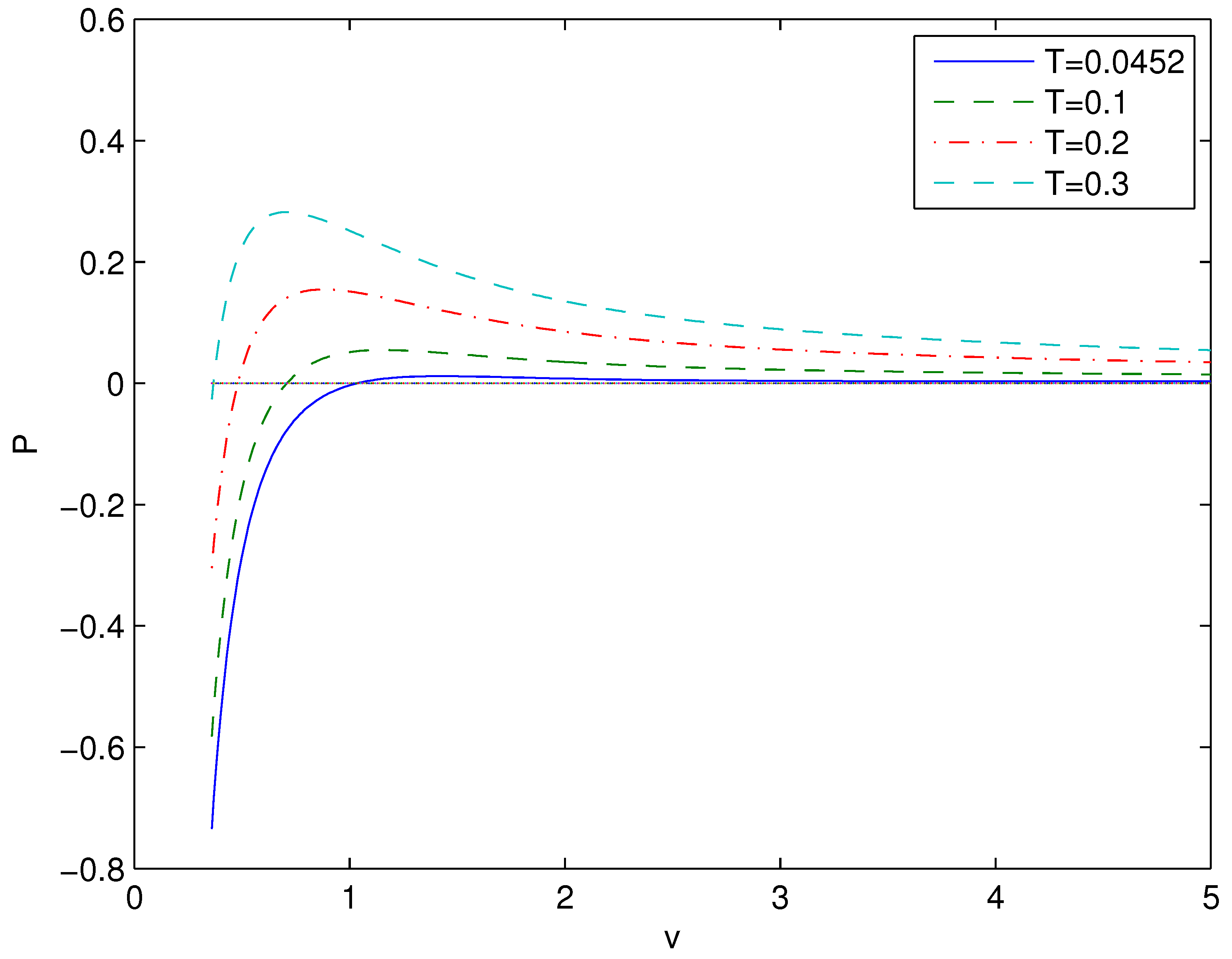
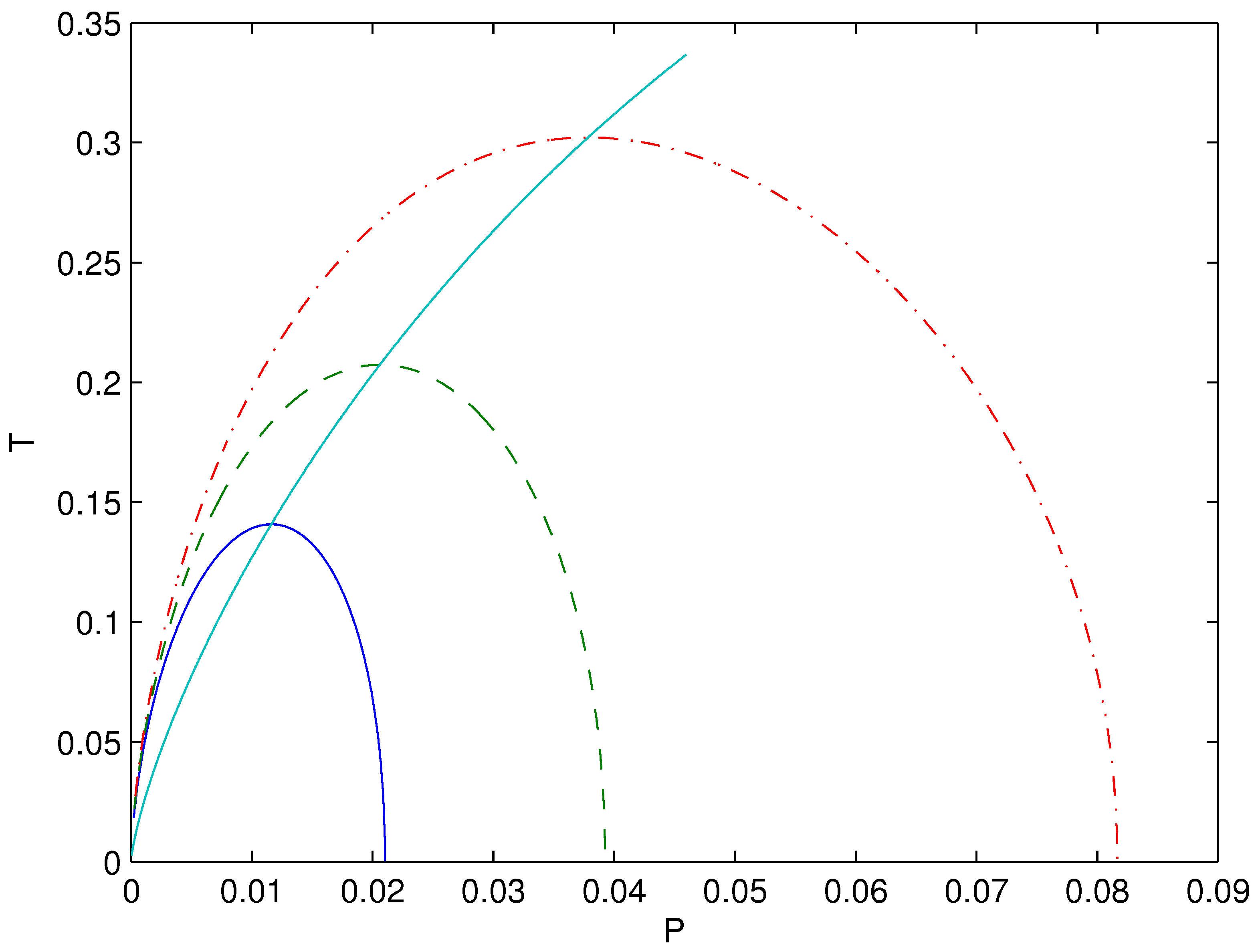
5. Joule–Thomson Expansion
6. Summary
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
| 1 |
References
- Bardeen, J.M.; Carter, B.; Hawking, S.W. The four laws of black hole mechanics. Commun. Math. Phys. 1973, 31, 161–170. [Google Scholar] [CrossRef]
- Jacobson, T. Thermodynamics of space-time: The Einstein equation of state. Phys. Rev. Lett. 1995, 75, 1260–1263. [Google Scholar] [CrossRef] [PubMed]
- Padmanabhan, T. Thermodynamical Aspects of Gravity: New insights. Rept. Prog. Phys. 2010, 73, 046901. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and entropy. Phys. Rev. D 1973, 7, 2333–2346. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle Creation by Black Holes. Commun. Math. Phys. 1975, 43, 199–220. [Google Scholar] [CrossRef]
- Hawking, S.W.; Page, D.N. Thermodynamics of Black Holes in anti-De Sitter Space. Commun. Math. Phys. 1983, 87, 577. [Google Scholar] [CrossRef]
- Maldacena, J.M. The Large N limit of superconformal field theories and supergravity. Int. J. Theor. Phys. 1999, 38, 1113–1133. [Google Scholar] [CrossRef]
- Dolan, B.P. Black holes and Boyle’s law? The thermodynamics of the cosmological constant. Mod. Phys. Lett. A 2015, 30, 1540002. [Google Scholar] [CrossRef]
- Kubiznak, D.; Mann, R.B. Black hole chemistry. Can. J. Phys. 2015, 93, 999–1002. [Google Scholar] [CrossRef]
- Mann, R.B. The Chemistry of Black Holes. In 1st Karl Schwarzschild Meeting on Gravitational Physics; Springer: Cham, Switzerland, 2016; Volume 170, pp. 197–205. [Google Scholar]
- Kubiznak, D.; Mann, R.B.; Teo, M. Black hole chemistry: Thermodynamics with Lambda. Class. Quant. Grav. 2017, 34, 063001. [Google Scholar] [CrossRef]
- Born, M.; Infeld, L. Foundations of the new field theory. Nature 1933, 132, 1004. [Google Scholar] [CrossRef]
- Heisenberg, W.; Euler, H. Folgerungen aus der Diracschen Theorie des Positrons. Z. Phys. 1936, 98, 714. [Google Scholar] [CrossRef]
- Sorokin, D.P. Introductory notes on nonlinear electrodynamics and its applications. Fortschritte Phys. 2022, 70, 2200092. [Google Scholar] [CrossRef]
- Bronnikov, K.A. Regular black holes sourced by nonlinear electrodynamics. In Regular Black Holes: Towards a New Paradigm of Gravitational Collapse; Springer Nature: Singapore, 2023; pp. 37–67. [Google Scholar]
- Fernando, S.; Krug, D. Charged black hole solutions in Einstein–Born–Infeld gravity with a cosmological constant. Gen. Rel. Grav. 2003, 35, 129–137. [Google Scholar] [CrossRef]
- Dey, T.K. Born–Infeld black holes in the presence of a cosmological constant. Phys. Lett. B 2004, 595, 484–490. [Google Scholar]
- Cai, R.-G.; Pang, D.-W.; Wang, A. Born–Infeld black holes in (A)dS spaces. Phys. Rev. D 2004, 70, 124034. [Google Scholar] [CrossRef]
- Fernando, S. Thermodynamics of Born–Infeld-anti-de Sitter black holes in the grand canonical ensemble. Phys. Rev. D 2006, 74, 104032. [Google Scholar] [CrossRef]
- Myung, Y.S.; Kim, Y.-W.; Park, Y.-J. Thermodynamics and phase transitions in the Born–Infeld-anti-de Sitter black holes. Phys. Rev. D 2008, 78, 084002. [Google Scholar] [CrossRef]
- Banerjee, R.; Roychowdhury, D. Critical phenomena in Born-Infeld AdS black holes. Phys. Rev. D 2012, 85, 044040. [Google Scholar] [CrossRef]
- Miskovic, O.; Olea, R. Thermodynamics of Einstein–Born–Infeld black holes with negative cosmological constant. Phys. Rev. D 2008, 77, 124048. [Google Scholar] [CrossRef]
- Pourhassan, B.; Dehghani, M.; Faizal, M.; Dey, S. Non-Pertubative Quantum Corrections to a Born–Infeld Black Hole and its Information Geometry. Class. Quantum Grav. 2021, 38, 105001. [Google Scholar] [CrossRef]
- Pourhassan, B.; Dehghani, M.; Upadhyay, S.; Sakalli, I.; Singh, D.V. Exponential corrected thermodynamics of quantum Born–Infeld BTZ black holes in massive gravity. Mod. Phys. Lett. A 2022, 37, 2250230. [Google Scholar] [CrossRef]
- Gunasekaran, S.; Mann, R.B.; Kubiznak, D. Extended phase space thermodynamics for charged and rotating black holes and Born–Infeld vacuum polarization. J. High Energy Phys. 2012, 1211, 110. [Google Scholar] [CrossRef]
- Zou, D.-C.; Zhang, S.-J.; Wang, B. Critical behavior of Born-Infeld AdS black holes in the extended phase space thermodynamics. Phys. Rev. D 2014, 89, 044002. [Google Scholar] [CrossRef]
- Hendi, S.H.; Vahidinia, M.H. Extended phase space thermodynamics and P-V criticality of black holes with a nonlinear source. Phys. Rev. D 2013, 88, 084045. [Google Scholar] [CrossRef]
- Hendi, S.H.; Panahiyan, S.; Panah, B.E. P-V criticality and geometrical thermodynamics of black holes with Born-Infeld type nonlinear electrodynamics. Int. J. Mod. Phys. D 2015, 25, 1650010. [Google Scholar] [CrossRef]
- Zeng, X.-X.; Liu, X.-M.; Li, L.-F. Phase structure of the Born–Infeld-anti-de Sitter black holes probed by non-local observables. Eur. Phys. J. C 2016, 76, 616. [Google Scholar] [CrossRef]
- Ökcü, Ö.; Aydiner, E. Joule–Thomson expansion of the charged AdS black holes. Eur. Phys. J. C 2017, 77, 24. [Google Scholar] [CrossRef]
- Ghaffarnejad, H.; Yaraie, E.; Farsam, M. Quintessence Reissner–Nordstrom anti de Sitter black holes and Joule–Thomson effect. Int. J. Theor. Phys. 2018, 57, 1671. [Google Scholar] [CrossRef]
- Rizwan, C.L.A.; Kumara, A.N.; Vaid, D.; Ajith, K.M. Joule–Thomson expansion in AdS black hole with a global monopole. Int. J. Mod. Phys. A 2018, 33, 1850210. [Google Scholar] [CrossRef]
- Chabab, M.; Moumni, H.E.; Iraoui, S.; Masmar, K.; Zhizeh, S. Joule-Thomson Expansion of RN-AdS Black Holes in f(R) gravity. Lett. High Energy Phys. 2018, 5, 2. [Google Scholar]
- Mirza, B.; Naeimipour, F.; Tavakoli, M. Joule-Thomson expansion of the quasitopological black holes. Front. Phys. 2021, 9, 628727. [Google Scholar] [CrossRef]
- Kruglov, S.I. NED-AdS black holes, extended phase space thermodynamics and Joule–Thomson expansion. Nucl. Phys. B 2022, 984, 115949. [Google Scholar] [CrossRef]
- Kruglov, S.I. Magnetic black holes in AdS space with nonlinear electrodynamics, extended phase space thermodynamics and Joule–Thomson expansion. Int. J. Geom. Meth. Mod. Phys. 2023, 20, 2350008. [Google Scholar] [CrossRef]
- Kruglov, S.I. AdS Black Holes in the Framework of Nonlinear Electrodynamics, Thermodynamics, and Joule–Thomson Expansion. Symmetry 2022, 14, 1597. [Google Scholar] [CrossRef]
- Kruglov, S.I. Nonlinearly charged AdS black holes, extended phase space thermodynamics and Joule–Thomson expansion. Ann. Phys. 2022, 441, 168894. [Google Scholar] [CrossRef]
- Kruglov, S.I. Magnetically Charged AdS Black Holes and Joule–Thomson Expansion. Grav. Cosm. 2023, 28, 57. [Google Scholar] [CrossRef]
- Bronnikov, K.A. Regular magnetic black holes and monopoles from nonlinear electrodynamics. Phys. Rev. D 2001, 63, 044005. [Google Scholar] [CrossRef]
- Kruglov, S.I. Nonlinear Electrodynamics and Magnetic Black Holes. Ann. Phys. 2017, 529, 1700073. [Google Scholar] [CrossRef]
- Kastor, D.; Ray, S.; Traschen, J. Enthalpy and the Mechanics of AdS Black Holes. Class. Quant. Grav. 2009, 26, 195011. [Google Scholar] [CrossRef]
- Dolan, B.P. The cosmological constant and the black hole equation of state. Class. Quant. Grav. 2011, 28, 125020. [Google Scholar] [CrossRef]
- Cvetic, M.; Gibbons, G.W.; Kubiznak, D.; Pope, C.N. Black Hole Enthalpy and an Entropy Inequality for the Thermodynamic Volume. Phys. Rev. D 2011, 84, 024037. [Google Scholar] [CrossRef]
- Kubiznak, D.; Mann, R.B. P-V criticality of charged AdS black holes. J. High Energy Phys. 2012, 7, 033. [Google Scholar] [CrossRef]
- Cong, W.; Kubiznak, D.; Mann, R.B.; Visser, M. Holographic CFT Phase Transitions and Criticality for Charged AdS Black Holes. J. High Energy Phys. 2022, 8, 174. [Google Scholar] [CrossRef]
- Smarr, L. Mass formula for Kerr black holes. Phys. Rev. Lett. 1973, 30, 71–73. [Google Scholar] [CrossRef]
- Hudson, C. Die gegenseitige löslichkeit von nikotin in wasser. Z. Phys. Chem. 1904, 47, 113. [Google Scholar] [CrossRef]
- Altamirano, N.; Kubiznak, D.; Mann, R.B.; Sherkatghanad, Z. Thermodynamics of rotating black holes and black rings: Phase transitions and thermodynamic volume. Galaxies 2014, 2, 89. [Google Scholar] [CrossRef]
- Altamirano, N.; Kubiznak, D.; Mann, R. Reentrant Phase Transitions in Rotating AdS Black Holes. Phys. Rev. D 2013, 88, 101502. [Google Scholar] [CrossRef]
- Narayanan, T.; Kumar, A. Reentrant phase transitions in multicomponent liquid mixtures. Phys. Rep. 1994, 249, 135–218. [Google Scholar] [CrossRef]
- Kruglov, S.I. Towards quantum gravity. J. Hologr. Appl. Phys. 2023, 3, 53–58. [Google Scholar]
- Shabad, A.E.; Usov, V.V. Effective Lagrangian in nonlinear electrodynamics and its properties of causality and unitarity. Phys. Rev. D 2011, 83, 105006. [Google Scholar] [CrossRef]





| 0.1 | 0.2 | 0.4 | 0.5 | 0.7 | 0.8 | 0.9 | 1 | |
|---|---|---|---|---|---|---|---|---|
| 4.790 | 4.708 | 4.552 | 4.472 | 4.297 | 4.196 | 4.080 | 3.936 | |
| 0.0438 | 0.0442 | 0.0448 | 0.0452 | 0.0459 | 0.0463 | 0.0467 | 0.0472 | |
| 0.0034 | 0.0035 | 0.0036 | 0.0037 | 0.0038 | 0.0039 | 0.0040 | 0.0041 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kruglov, S.I. Einstein-AdS Gravity Coupled to Nonlinear Electrodynamics, Magnetic Black Holes, Thermodynamics in an Extended Phase Space and Joule–Thomson Expansion. Universe 2023, 9, 456. https://doi.org/10.3390/universe9100456
Kruglov SI. Einstein-AdS Gravity Coupled to Nonlinear Electrodynamics, Magnetic Black Holes, Thermodynamics in an Extended Phase Space and Joule–Thomson Expansion. Universe. 2023; 9(10):456. https://doi.org/10.3390/universe9100456
Chicago/Turabian StyleKruglov, Sergey Il’ich. 2023. "Einstein-AdS Gravity Coupled to Nonlinear Electrodynamics, Magnetic Black Holes, Thermodynamics in an Extended Phase Space and Joule–Thomson Expansion" Universe 9, no. 10: 456. https://doi.org/10.3390/universe9100456
APA StyleKruglov, S. I. (2023). Einstein-AdS Gravity Coupled to Nonlinear Electrodynamics, Magnetic Black Holes, Thermodynamics in an Extended Phase Space and Joule–Thomson Expansion. Universe, 9(10), 456. https://doi.org/10.3390/universe9100456






