Cold Quark–Gluon Plasma EOS Applied to a Magnetically Deformed Quark Star with an Anomalous Magnetic Moment
Abstract
1. Introduction
2. QCD-Motivated EOS
3. TOV Framework
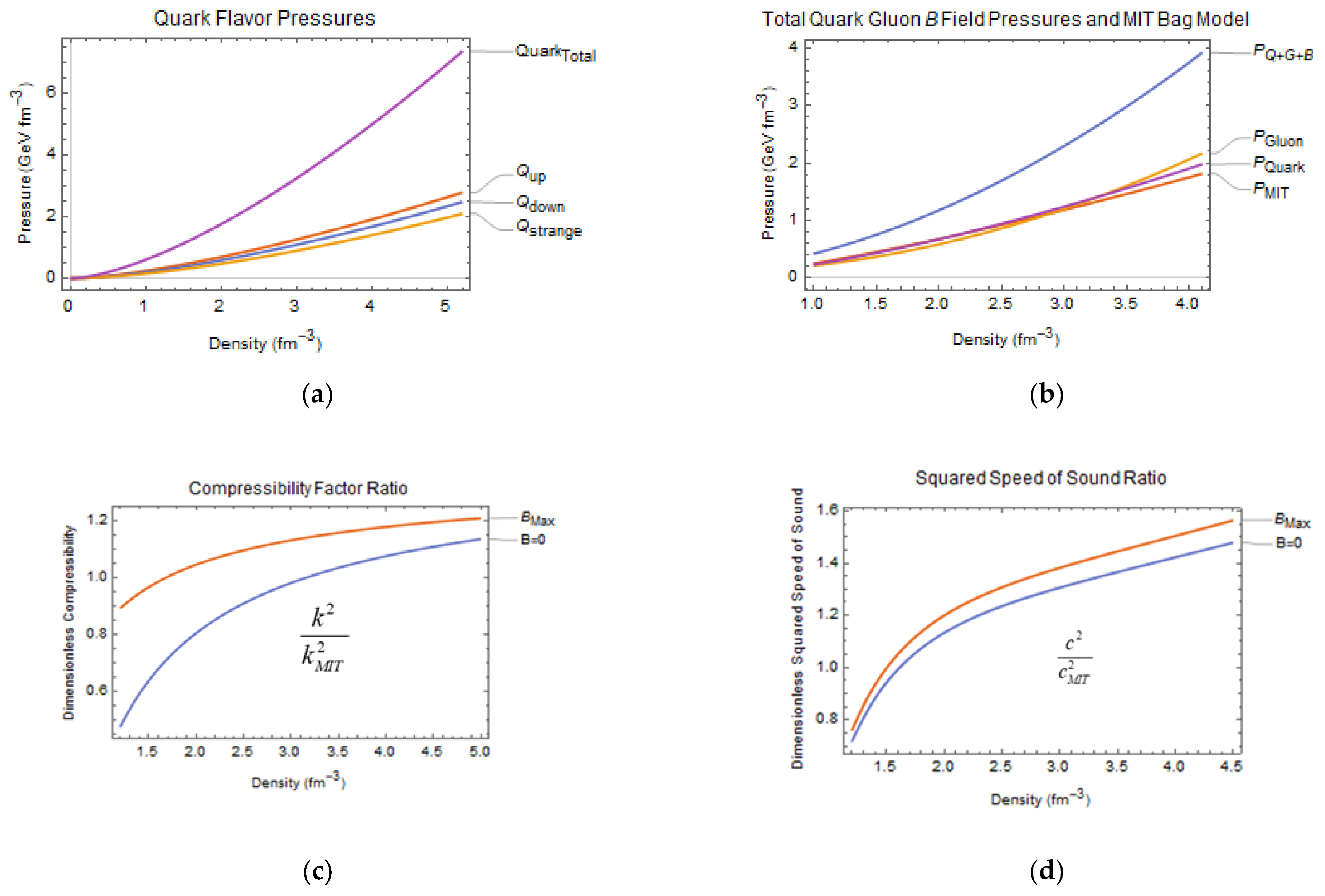
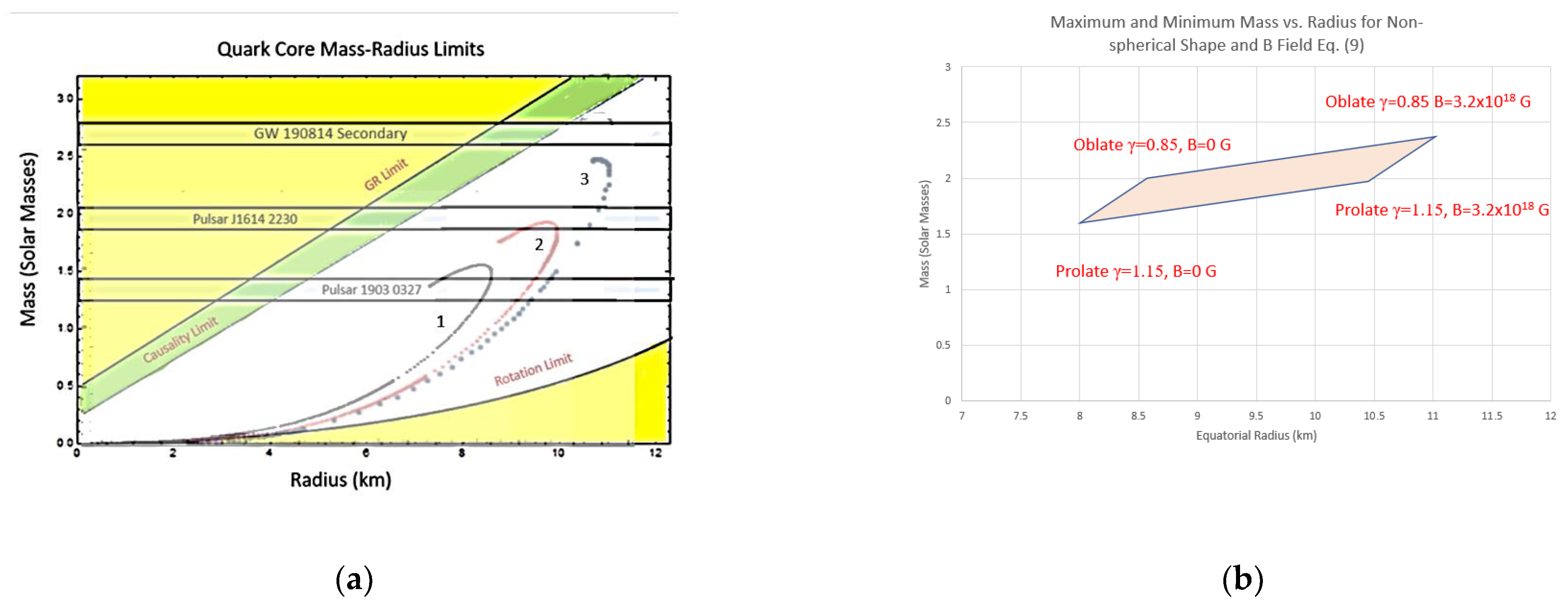
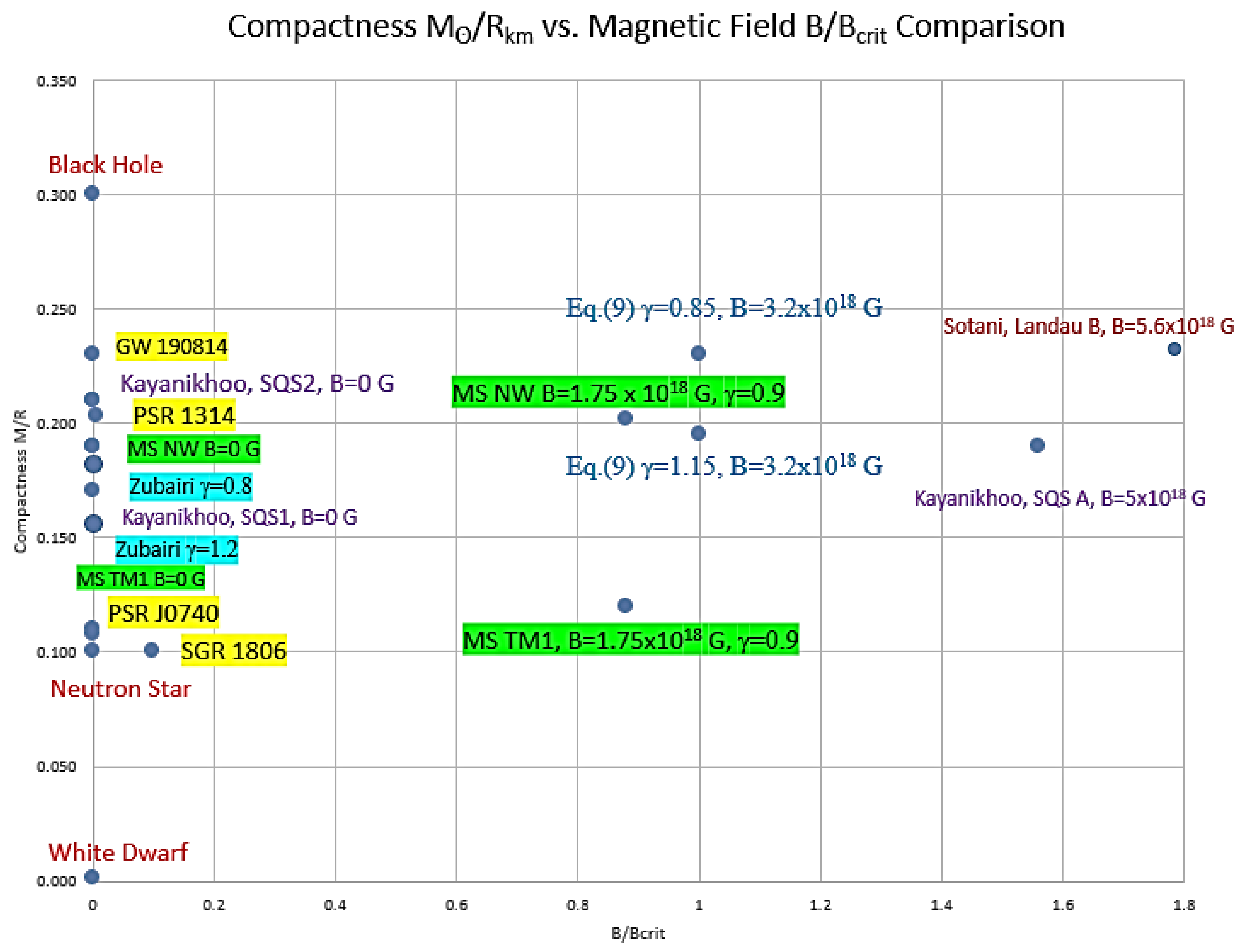
4. Numerical Solutions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Smith, N.; Li, W.; Foley, R.J.; Wheeler, J.C.; Pooley, D.; Chornock, R.; Filippenko, A.V.; Silverman, J.M.; Quimby, R.; Bloom, J.S.; et al. SN 2006gy: Discovery of the most luminous supernova ever recorded, powered by the death of an extremely massive star like η Carinae. Astrophys. J. 2007, 666, 1116. [Google Scholar] [CrossRef]
- Xu, R. Strange quark stars: Observations and speculations. J. Phys. G: Nucl. Part. Phys. 2009, 36, 064010. [Google Scholar] [CrossRef][Green Version]
- Burwitz, V.; Haberl, F.; Neuhäuser, R.; Predehl, P.; Trümper, J.; Zavlin, V.E. The thermal radiation of the isolated neutron star RX J1856. 5–3754 observed with Chandra and XMM-Newton. Astron. Astrophys. 2003, 399, 1109–1114. [Google Scholar] [CrossRef]
- Burwitz, V.; Zavlin, V.E.; Neuhäuser, R.; Predehl, P.; Trümper, J.; Brinkman, A.C. The Chandra LETGS high resolution X-ray spectrum of the isolated neutron star RX J1856. 5-3754. Astron. Astrophys. 2001, 379, L35–L38. [Google Scholar] [CrossRef]
- Xu, R.X.; Qiao, G.J.; Zhang, B. PSR 0943+ 10: A bare strange star? Astrophys. J. Lett. 1999, 522, L109. [Google Scholar] [CrossRef]
- Lyne, A.; Hobbs, G.; Kramer, M.; Stairs, I.; Stappers, B. Switched magnetospheric regulation of pulsar spin-down. Science 2010, 329, 408–412. [Google Scholar] [CrossRef]
- Alford, M.; Braby, M.; Paris, M.; Reddy, S. Hybrid stars that masquerade as neutron stars. Astrophys. J. 2005, 629, 969–978. Available online: https://iopscience.iop.org/article/10.1086/430902/pdf (accessed on 12 September 2017). [CrossRef]
- Olausen, S.A.; Kaspi, V.M. The McGill magnetar catalog. Astrophys. J. Suppl. Ser. 2014, 212, 6. [Google Scholar] [CrossRef]
- Burgio, G.F.; Vidaña, I. The Equation of State of Nuclear Matter: From Finite Nuclei to Neutron Stars. Universe 2020, 6, 119. [Google Scholar] [CrossRef]
- Shapiro, S.L.; Teukolsky, S.A.; Holes, B. White Dwarfs and Neutron Stars: The Physics of Compact Objects; John Wiley and Sons: New York, NY, USA, 1983. [Google Scholar]
- Baym, G.; Hatsuda, T.; Kojo, T.; Powell, P.D.; Song, Y.; Takatsuka, T. From hadrons to quarks in neutron stars: A review. Rep. Prog. Phys. 2018, 81, 056902. [Google Scholar] [CrossRef]
- Yagi, K.; Kyutoku, K.; Pappas, G.; Yunes, N.; Apostolatos, T.A. Effective no-hair relations for neutron stars and quark stars: Relativistic results. Phys. Rev. D 2014, 89, 124013. [Google Scholar] [CrossRef]
- Heinz, U. From SPS to RHIC: Breaking the barrier to the quark-gluon plasma. AIP Conf. Proc. 2001, 602, 281–292. [Google Scholar]
- Emerick, A.; Zhao, X.; Rapp, R. Bottomonia in the quark-gluon plasma and their production at RHIC and LHC. Eur. Phys. J. A 2012, 48, 72. [Google Scholar] [CrossRef]
- Teaney, D.; Lauret, J.; Shuryak, E.V. Flow at the SPS and RHIC as a quark-gluon plasma signature. Phys. Rev. Lett. 2001, 86, 4783–4786. [Google Scholar] [CrossRef]
- Arsene, I.; Bearden, I.; Beavis, D.; Besliu, C.; Budick, B.; Bøggild, H.; Chasman, C.; Christensen, C.; Christiansen, P.; Cibor, J.; et al. Quark–gluon plasma and color glass condensate at RHIC? The perspective from the BRAHMS experiment. Nucl. Phys. A 2005, 757, 1–27. [Google Scholar] [CrossRef]
- Florkowski, W.; Jaiswal, A.; Maksymiuk, E.; Ryblewski, R.; Strickland, M. Relativistic quantum transport coefficients for second-order viscous hydrodynamics. Phys. Rev. C 2015, 91, 054907. [Google Scholar] [CrossRef]
- Heinz, U.; Shen, C.; Song, H. The viscosity of quark-gluon plasma at RHIC and the LHC. AIP Conf. Proc. 2012, 1441, 766–770. [Google Scholar]
- Moore, G.; Saremi, O. Bulk viscosity and spectral functions in QCD. J. High Energy Phys. 2008, 2008, 015. [Google Scholar] [CrossRef]
- Miller, J.M.; Fabian, A.C.; Wijnands, R.; Reynolds, C.S.; Ehle, M.; Freyberg, M.J.; van der Klis, M.; Lewin, W.H.G.; Sanchez-Fernandez, C.; Castro-Tirado, A.J. Evidence of Spin and Energy Extraction in a Galactic Black Hole Candidate: The XMM-Newton/EPIC-pn Spectrum of XTE J1650–500. Astrophys. J. Lett. 2002, 570, L69. [Google Scholar] [CrossRef]
- Olive, K.A.; Pospelov, M. Environmental dependence of masses and coupling constants. Phys. Rev. D 2008, 77, 043524. [Google Scholar] [CrossRef]
- Dey, M.; Bombaci, I.; Dey, J.; Ray, S.; Samanta, B.C. Strange stars with realistic quark vector interaction and phenomenological density-dependent scalar potential. Phys. Lett. B 1998, 438, 123–128. Available online: https://arxiv.org/pdf/astro-ph/9810065.pdf (accessed on 12 May 2021). [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agathos, M.; et al. GW190814: Gravitational waves from the coalescence of a 23 solar mass black hole with a 2.6 solar mass compact object. Astrophys. J. Lett. 2020, 896, L44. [Google Scholar] [CrossRef]
- Most, E.R.; Papenfort, L.J.; Weih, L.R.; Rezzolla, L. A lower bound on the maximum mass if the secondary in GW190814 was once a rapidly spinning neutron star. Mon. Not. R. Astron. Soc. Lett. 2020, 499, L82–L86. [Google Scholar] [CrossRef]
- Lu, W.; Beniamini, P.; Bonnerot, C. On the formation of GW190814. Mon. Not. R. Astron. Soc. 2021, 500, 1817–1832. [Google Scholar] [CrossRef]
- Dolan, L.; Jackiw, R. Symmetry behavior at finite temperature. Phys. Rev. D 1974, 9, 3320–3341. [Google Scholar] [CrossRef]
- Ray, S.; Dey, J.; Dey, M. Density dependent strong coupling constant of QCD derived from compact star data. Mod. Phys. Lett. A 2000, 15, 1301–1306. [Google Scholar] [CrossRef]
- Weber, F. Strange quark matter and compact stars. Prog. Part. Nucl. Phys. 2005, 54, 193–288. [Google Scholar] [CrossRef]
- Alford, M.; Rajagopal, K.; Wilczek, F. Color-flavor locking and chiral symmetry breaking in high density QCD. Nucl. Phys. B 1999, 537, 443–458. [Google Scholar] [CrossRef]
- Andrew, K.; Steinfelds, E.; Andrew, K. QCD Color Vector Potential Effects on Color Flavor Locked Quark Stellar Cores. Bull. Am. Phys. Soc. 2018, 63, BAPS.2018.SES.D05.9. [Google Scholar]
- Rajagopal, K.; Wilczek, F. Enforced electrical neutrality of the color-flavor locked phase. Phys. Rev. Lett. 2001, 86, 3492–3495. [Google Scholar] [CrossRef]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455. [Google Scholar] [CrossRef]
- Alford, M.; Reddy, S. Compact stars with color superconducting quark matter. Phys. Rev. D 2003, 67, 074024. [Google Scholar] [CrossRef]
- Baldo, M.; Buballa, M.; Burgio, G.F.; Neumann, F.; Oertel, M.; Schulze, H.-J. Neutron stars and the transition to color superconducting quark matter. Phys. Lett. B 2003, 562, 153–160. [Google Scholar] [CrossRef]
- Lawley, S.; Bentz, W.; Thomas, A. Nucleons, nuclear matter and quark matter: A unified NJL approach. J. Phys. G Nucl. Part. Phys. 2006, 32, 667–679. [Google Scholar] [CrossRef]
- Baym, G.; Chin, S.A. Can a neutron star be a giant MIT bag? Phys. Lett. B 1976, 62, 241–244. [Google Scholar] [CrossRef]
- Källman, C.-G. Mean-field QCD model for hot/dense matter. Phys. Lett. B 1984, 134, 363–367. [Google Scholar] [CrossRef]
- Adler, S.L. Generalized bag models as mean-field approximations to QCD. Phys. Lett. B 1982, 110, 302–306. [Google Scholar] [CrossRef]
- Fodor, Z.; Sandor, D.K. A new method to study lattice QCD at finite temperature and chemical potential. Phys. Lett. B 2002, 534, 87–92. [Google Scholar] [CrossRef]
- Glendenning, N.K.; Weber, F. Nuclear solid crust on rotating strange quark stars. Astrophys. J. Lett. 1992, 400, 647–658. [Google Scholar] [CrossRef]
- Owen, B.J. Maximum elastic deformations of compact stars with exotic equations of state. Phys. Rev. Lett. 2005, 95, 211101. [Google Scholar] [CrossRef]
- Sharma, R.; Mukherjee, S. Compact stars: A core-envelope model. Mod. Phys. Lett. A 2002, 17, 2535–2544. [Google Scholar] [CrossRef]
- Ng, C.; Cheng, K.; Chu, M. Cooling properties of Cloudy Bag strange stars. Astropart. Phys. 2003, 19, 171–192. Available online: https://arxiv.org/pdf/astro-ph/0209016.pdf (accessed on 10 March 2018). [CrossRef]
- Bhattacharyya, A.; Ghosh, S.K.; Joarder, P.S.; Mallick, R.; Raha, S. Conversion of a neutron star to a strange star: A two-step process. Phys. Rev. C 2006, 74, 065804. [Google Scholar] [CrossRef]
- Kedia, A.; Kim, H.I.; Suh, I.S.; Mathews, G.J. Binary neutron star mergers as a probe of quark-hadron crossover equations of state. arXiv 2022, arXiv:2203.05461. [Google Scholar]
- Latifah, S.; Sulaksono, A.; Mart, T. Bosons star at Finite temperature. Phys. Rev. D 2014, 90, 127501. [Google Scholar] [CrossRef]
- Andrew, K.; Andrew, K.; Brown, R.; Thornberry, B.; Harper, S.; Steinfelds, E.; Roberts, T. A QCD Model of the Chemical Potential Kaon Boundary Formation for a Compact Quark Star. Bull. Am. Phys. Soc. 2016, 61, BAPS.2016.SES.K1.8. [Google Scholar]
- Thorsson, V.; Prakash, M.; Lattimer, J.M. Composition, structure and evolution of neutron stars with kaon condensates. Nucl. Phys. A 1994, 572, 693–731. [Google Scholar] [CrossRef]
- Alford, M. Color-superconducting quark matter. Annu. Rev. Nucl. Part. Sci. 2001, 51, 131–160. [Google Scholar] [CrossRef]
- Alford, M.; Bowers, J.A.; Rajagopal, K. Crystalline color superconductivity. Phys. Rev. D 2001, 63, 074016. [Google Scholar] [CrossRef]
- Casalbuoni, R.; Gatto, R.; Mannarelli, M.; Nardulli, G. Effective field theory for the crystalline colour superconductive phase of QCD. Phys. Lett. B 2001, 511, 218–228. [Google Scholar] [CrossRef]
- Andrew, K.; Brown, R.; Andrew, K.; Thornberry, B.; Harper, S.; Steinfelds, E.; Roberts, T. Analysis of Mass and Radius Sensitivity of a Crystalline Quark Star to a Strong Repulsive Equation of State. Bull. Am. Phys. Soc. 2016, 61, BAPS.2016.SES.K1.7. [Google Scholar]
- Paerels, F.B.; Mendez, M.; Agüeros, M.A.; Baring, M.; Barret, D.; Bhattacharyya, S.; Cackett, E.; Cottam, J.; Tringo, M.D.; Fox, D.; et al. The Behavior of Matter Under Extreme Conditions. arXiv 2009, arXiv:0904.0435. preprint. Available online: https://arxiv.org/pdf/0904.0435.pdf (accessed on 17 April 2019).
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+ 0451 mass and radius from NICER data and implications for the properties of neutron star matter. Astrophys. J. Lett. 2019, 887, L24. [Google Scholar] [CrossRef]
- Mak, M.K.; Harko, T. An exact anisotropic quark star model. Chin. J. Astron. Astrophys. 2002, 2, 248. [Google Scholar] [CrossRef]
- Zdunik, J.L.; Bejger, M.; Haensel, P. Crustal rigidity and rotational deformation of neutron stars. arXiv 2008, arXiv:0805.1814. preprint. Available online: https://www.aanda.org/articles/aa/pdf/2008/44/aa10183-08.pdf (accessed on 22 October 2018).
- Mallick, R.; Schramm, S. Deformation of a magnetized neutron star. Phys. Rev. C 2014, 89, 045805. [Google Scholar] [CrossRef]
- Chandrasekhar, S.; Fermi, E. Problems of gravitational stability in the presence of a magnetic field. Astrophys. J. 1953, 118, 116–141. [Google Scholar] [CrossRef]
- Sotani, H.; Tatsumi, T. Hybrid Quark Stars with Strong Magnetic Field. arXiv 2017, arXiv:1702.07843. preprint. Available online: https://arxiv.org/pdf/1702.07843.pdf (accessed on 12 May 2021). [CrossRef]
- Chatziioannou, K. Neutron-star tidal deformability and equation-of-state constraints. Gen. Relativ. Gravit. 2020, 52, 1–49. [Google Scholar] [CrossRef]
- Morsink, S.; Leahy, D.A.; Cadeau, C.; Braga, J. The oblate schwarzschild approximation for light curves of rapidly rotating neutron stars. Astrophys. J. 2007, 663, 1244–1251. [Google Scholar] [CrossRef]
- Zubairi, O.; Spinella, W.; Romero, A.; Mellinger, R.; Weber, F.; Orsaria, M.; Contrera, G. Non-spherical models of neutron stars. arXiv 2015, arXiv:1504.03006. preprint. Available online: https://arxiv.org/pdf/1504.03006.pdf (accessed on 11 July 2020).
- Chatterjee, D.; Novak, J.; Oertel, M. Structure of ultra-magnetised neutron stars. Eur. Phys. J. A 2021, 57, 249. [Google Scholar] [CrossRef]
- Bandyopadhyay, D.; Chakrabarty, S.; Pal, S. Quantizing magnetic field and quark-hadron phase transition in a neutron star. Phys. Rev. Lett. 1997, 79, 2176–2179. [Google Scholar] [CrossRef]
- Chakrabarty, S.; Bandyopadhyay, D.; Pal, S. Dense nuclear matter in a strong magnetic field. Phys. Rev. Lett. 1997, 78, 2898–2901. [Google Scholar] [CrossRef]
- Kayanikhoo, F.; Naficy, K.; Bordbar, G.H. Influence of strong magnetic field on the structure properties of strange quark stars. Eur. Phys. J. A 2020, 56, 2. [Google Scholar] [CrossRef]
- Rabhi, A.; Providência, C. Quark matter under strong magnetic fields in chiral models. Phys. Rev. C 2011, 83, 055801. [Google Scholar] [CrossRef]
- Chaudhuri, N.; Ghosh, S.; Sarkar, S.; Roy, P. Effect of anomalous magnetic moment of quarks on chiral and de-confinement transition in pNJL model. Proc. DAE Symp. Nucl. Phys. 2019, 64, 722. [Google Scholar]
- D’Elia, M.; Mukherjee, S.; Sanfilippo, F. QCD phase transition in a strong magnetic background. Phys. Rev. D 2010, 82, 051501. [Google Scholar] [CrossRef]
- Xu, K.; Chao, J.; Huang, M. Effect of the anomalous magnetic moment of quarks on magnetized QCD matter and meson spectra. Phys. Rev. D 2021, 103, 076015. [Google Scholar] [CrossRef]
- Ferrer, E.J.; De La Incera, V.; Wen, X.J. Quark antiscreening at strong magnetic field and inverse magnetic catalysis. Phys. Rev. D 2015, 91, 054006. [Google Scholar] [CrossRef]
- Martinez, A.; Perez, R.; Felipe, G.; Paret, D.M. Mass–Radius Relation for Magnetized Strange Quarks Stars. Int. J. Mod. Phys. D 2010, 19, 1511–1519. [Google Scholar] [CrossRef]
- Felipe, R.G.; Martínez, A.P.; Rojas, H.P.; Orsaria, M. Magnetized strange quark matter and magnetized strange quark stars. Phys. Rev. C 2008, 77, 015807. [Google Scholar] [CrossRef]
- Mueller, N.; Jan, M.P. Magnetic catalysis and inverse magnetic catalysis in QCD. Phys. Rev. D 2015, 91, 116010. [Google Scholar] [CrossRef]
- Chaudhuri, N.; Ghosh, S.; Sarkar, S.; Roy, P. Dilepton production from magnetized quark matter with an anomalous magnetic moment of the quarks using a three-flavor PNJL model. Phys. Rev. D 2021, 103, 096021. [Google Scholar] [CrossRef]
- Kawaguchi, M.; Huang, M. Restriction on the form of quark anomalous magnetic moment from lattice QCD results. arXiv 2022, arXiv:2205.08169. preprint. [Google Scholar]
- Fukushima, K.; Hatsuda, T. The phase diagram of dense QCD. Rep. Prog. Phys. 2010, 74, 014001. [Google Scholar] [CrossRef]
- Wen, X.-J.; He, R.; Liu, J.-B. Effect of the anomalous magnetic moment on the chiral transition in a strong magnetic field. Phys. Rev. D 2021, 103, 094020. [Google Scholar] [CrossRef]
- Ferrer, E.J.; De La Incera, V.; Portillo, I.; Quiroz, M. New look at the QCD ground state in a magnetic field. Phys. Rev. D 2014, 89, 085034. [Google Scholar] [CrossRef]
- Fayazbakhsh, S.; Sadooghi, N. Anomalous magnetic moment of hot quarks, inverse magnetic catalysis, and reentrance of the chiral symmetry broken phase. Phys. Rev. D 2014, 90, 105030. [Google Scholar] [CrossRef]
- Schwinger, J. On quantum-electrodynamics and the magnetic moment of the electron. Phys. Rev. 1948, 73, 416–417. [Google Scholar] [CrossRef]
- Ferrer, E.J.; De La Incera, V.; Paret, D.M.; Martínez, A.P.; Sanchez, A. Insignificance of the anomalous magnetic moment of charged fermions for the equation of state of a magnetized and dense medium. Phys. Rev. D 2015, 91, 085041. [Google Scholar] [CrossRef]
- Celenza, L.S.; Shakin, C.M. Description of the gluon condensate. Phys. Rev. D 1986, 34, 1591–1600. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Shakin, C.M. Description of gluon propagation in the presence of an A 2 condensate. Phys. Rev. D 2005, 71, 074007. [Google Scholar] [CrossRef]
- Fogaça, D.A.; Navarra, F.S. Gluon condensates in a cold quark–gluon plasma. Phys. Lett. B 2011, 700, 236–242. [Google Scholar] [CrossRef]
- Mao, G.-J.; Iwamoto, A.; Li, Z.-X. A study of neutron star structure in strong magnetic fields that includes anomalous magnetic moments. Chin. J. Astron. Astrophys. 2003, 3, 359–374. [Google Scholar] [CrossRef]
- Kettner, C.; Weber, F.; Weigel, M.K.; Glendenning, N.K. Structure and stability of strange and charm stars at finite temperatures. Phys. Rev. D 1995, 51, 1440–1457. [Google Scholar] [CrossRef]
- Jiménez, J.C.; Eduardo, S.F. Cold quark matter with heavy quarks and the stability of charm stars. Phys. Rev. D 2020, 102, 034015. [Google Scholar] [CrossRef]
- Zyla, P.A. Particle data group. Prog. Theor. Exp. Phys. 2020, 8, 144–146. Available online: https://pdg.lbl.gov/ (accessed on 12 May 2021).
- Dexheimer, V.; Torres, J.R.; Menezes, D.P. Stability windows for proto-quark stars. Eur. Phys. J. C 2013, 73, 2569. [Google Scholar] [CrossRef]
- Franzon, B.; Fogaça, D.; Navarra, F.S.; Horvath, J.E. Self-bound interacting QCD matter in compact stars. Phys. Rev. D 2012, 86, 065031. [Google Scholar] [CrossRef]
- Farhi, E.; Robert, L.J. Strange matter. Phys. Rev. D 1984, 30, 2379. [Google Scholar] [CrossRef]
- Yanis, A.; Sulaksono, A. Deformation and anisotropic magnetic field effects on neutron star. AIP Conf. Proc. 2018, 2023, 020009. [Google Scholar]
- Hartle, J.B.; Kip, S.T. Slowly rotating relativistic stars. II. Models for neutron stars and supermassive stars. Astrophys. J. 1968, 153, 807. [Google Scholar] [CrossRef]
- De Boer, S. Compact Stars as a Laboratory for Nuclear Matter. Bachelor Thesis, Faculty of Physics at University of Bielefeld, Bielefeld, Germany, 2011. [Google Scholar]
- Ganzha, V.G.; Evgenii, V.V. Numerical Solutions for Partial Differential Equations: Problem Solving Using Mathematica; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Koberlein, B.; Meisel, D. Astrophysics through Computation: With Mathematica® Support; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Mallik, R.; Schramm, S.; Dexheimer, V.; Bhattacharyya, A. Magnetic field and neutron stars: A comprehensive study. Proc. Sci. 2017, 242, 066. [Google Scholar]
- Zhang, S.N.; Feroci, M.A.R.C.O.; Santangelo, A.; Dong, Y.W.; Feng, H.; Lu, F.J.; Nandra, K.; Wang, Z.S.; Zhang, S.; Bozzo, E.; et al. eXTP: Enhanced X-ray Timing and Polarization mission. In Space Telescopes and Instrumentation 2016: Ultraviolet to Gamma Ray; International Society for Optics and Photonics: Bellingham, WA, USA, 2017; Volume 9905, p. 99051Q. [Google Scholar]
- Slane, P.O.; David, J.H.; Stephen, S.M. New constraints on neutron star cooling from Chandra observations of 3C 58. Astrophys. J. 2002, 571, L45. [Google Scholar] [CrossRef]
- Ho, W.C.G.; Zhao, Y.; O Heinke, C.; Kaplan, D.L.; Shternin, P.S.; Wijngaarden, M.J.P. X-ray bounds on cooling, composition, and magnetic field of the Cassiopeia A neutron star and young central compact objects. Mon. Not. R. Astron. Soc. 2021, 506, 5015–5029. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andrew, K.; Steinfelds, E.V.; Andrew, K.A. Cold Quark–Gluon Plasma EOS Applied to a Magnetically Deformed Quark Star with an Anomalous Magnetic Moment. Universe 2022, 8, 353. https://doi.org/10.3390/universe8070353
Andrew K, Steinfelds EV, Andrew KA. Cold Quark–Gluon Plasma EOS Applied to a Magnetically Deformed Quark Star with an Anomalous Magnetic Moment. Universe. 2022; 8(7):353. https://doi.org/10.3390/universe8070353
Chicago/Turabian StyleAndrew, Keith, Eric V. Steinfelds, and Kristopher A. Andrew. 2022. "Cold Quark–Gluon Plasma EOS Applied to a Magnetically Deformed Quark Star with an Anomalous Magnetic Moment" Universe 8, no. 7: 353. https://doi.org/10.3390/universe8070353
APA StyleAndrew, K., Steinfelds, E. V., & Andrew, K. A. (2022). Cold Quark–Gluon Plasma EOS Applied to a Magnetically Deformed Quark Star with an Anomalous Magnetic Moment. Universe, 8(7), 353. https://doi.org/10.3390/universe8070353





