RR Lyrae Variables as Tracers of the Galactic Bulge Kinematic Structure
Abstract
1. Introduction
2. Metallicities of Inner Galaxy RR Lyrae Stars
2.1. Spectroscopic Metallicities of Inner Galaxy RR Lyrae Stars
2.2. Photometric Metallicities of Inner Galaxy RR Lyrae Stars
2.3. Age–Metallicity Relation
3. Spatial Distribution of RR Lyrae Stars in the Inner Galaxy
3.1. Distances Derived from RR Lyrae Stars
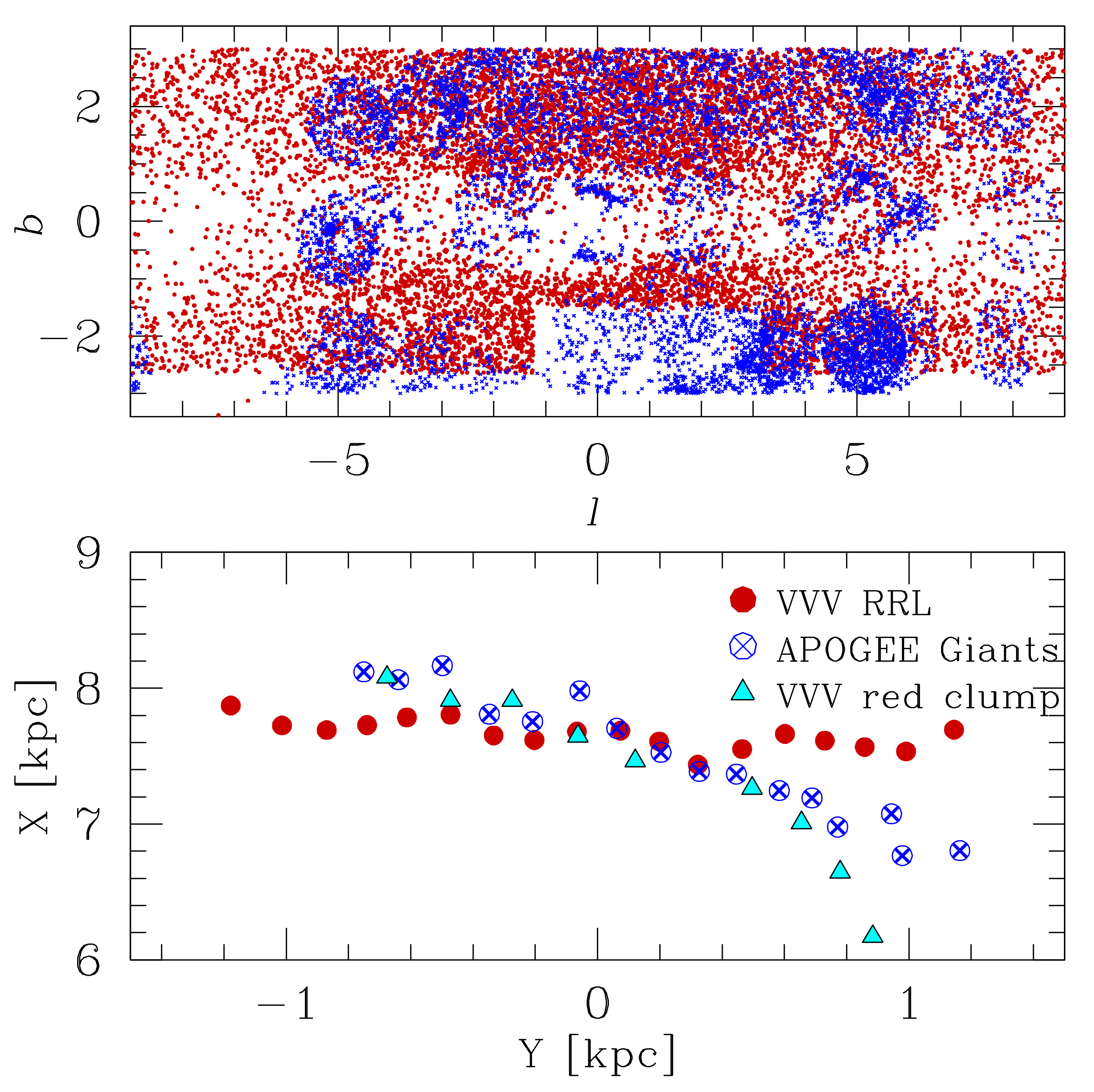
3.2. Inner Galaxy RR Lyrae Stars Spatial Distribution
4. The Inner Galaxy and Galactic Bulge/Bar Kinematics
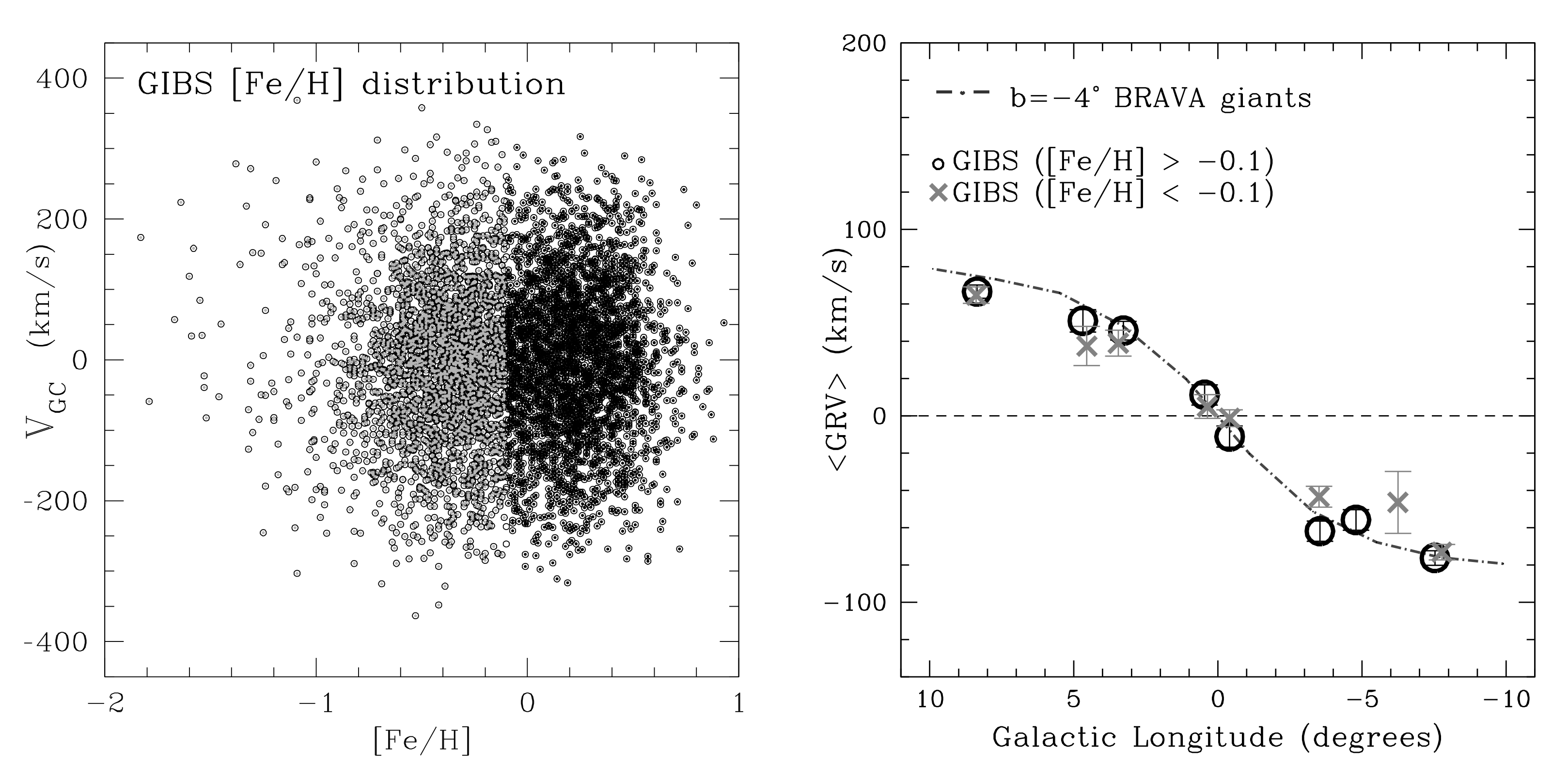
4.1. The First Inner Galaxy Kinematic Surveys
4.2. Kinematics of Stars with 1
4.3. Kinematics of Stars with 1
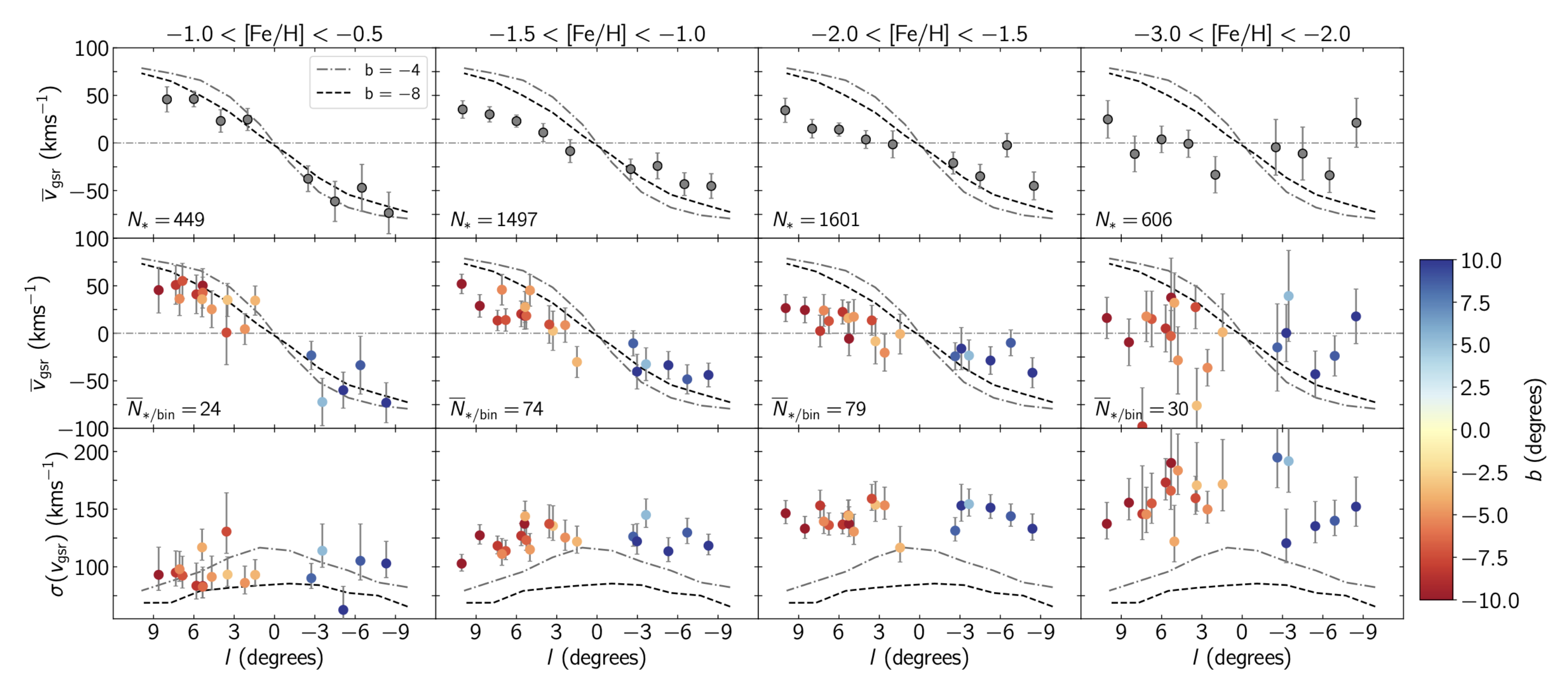
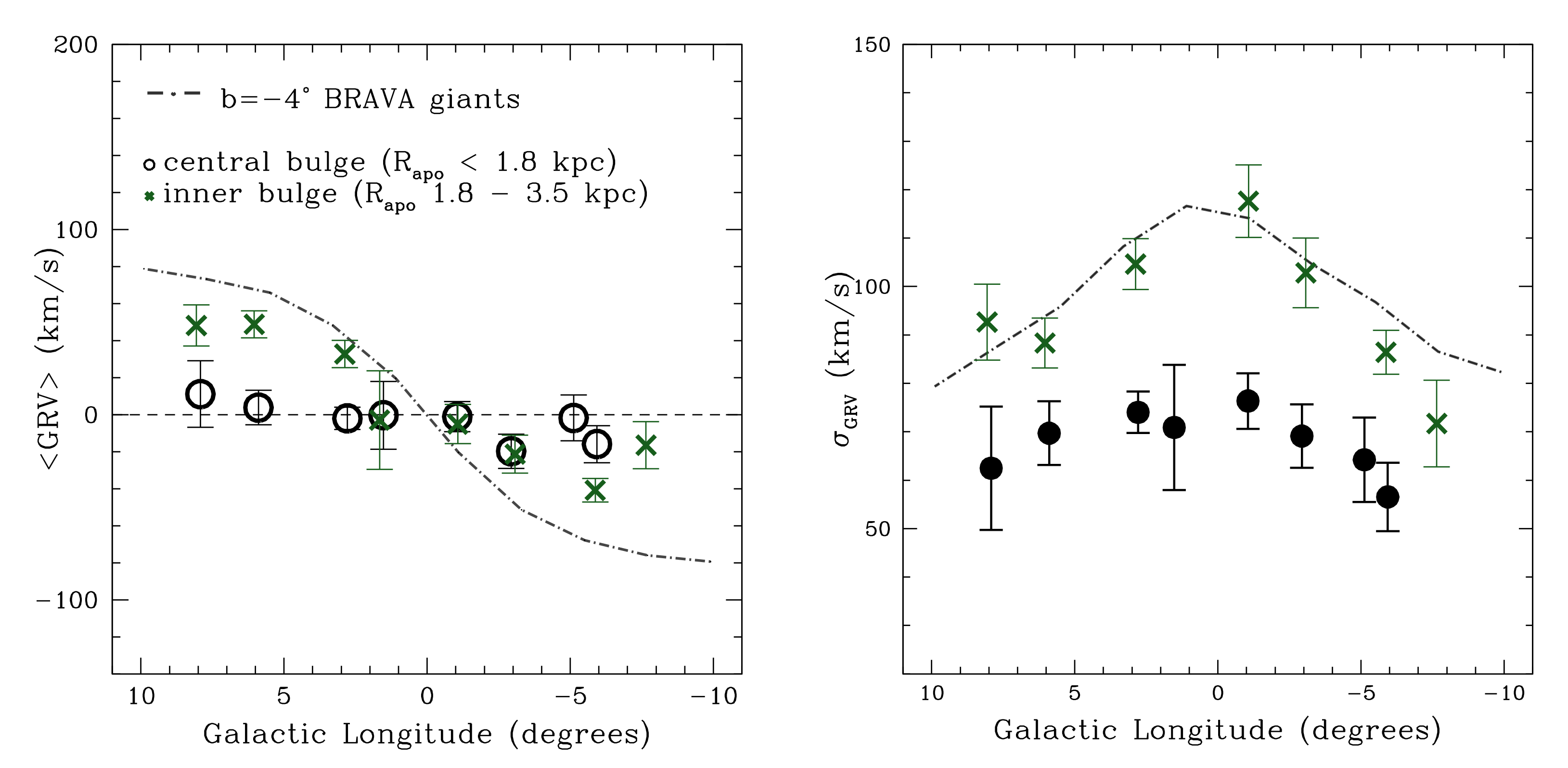
4.4. Kinematics of RR Lyrae Stars
4.5. Kinematics of RR Lyrae Stars in the Context of Milky Way Formation Scenarios
5. Conclusions
Funding
Conflicts of Interest
References
- Kinman, T.D.; Pier, J.R.; Suntzeff, N.B.; Harmer, D.L.; Valdes, F.; Hanson, R.B.; Klemola, A.R.; Kraft, R.P. An RR LYR star survey with the Lick 20-inch. astrograph. IV. A survey of 3 fields near North galactic pole. Astron. J. 1966, 111, 1164. [Google Scholar] [CrossRef]
- Oort, J.H.; Plaut, L. The distance to the galactic center derived from RR-Lyrae variables, the distribution of these variables in the Galaxy’s inner region and halo, and a rediscussion of the galactic rotation constants. Astron. Astrophys. 1975, 41, 71–86. [Google Scholar]
- Saha, A. RR Lyrae stars and the distant galactic halo: Distribution, chemical composition, kinematics and dynamics. Astrophys. J. 1985, 289, 310. [Google Scholar] [CrossRef]
- Layden, A.C. The Metallicities and Kinematics of RR Lyrae Variables. II. Galactic Structure and Formation from Local Stars. Astron. J. 1995, 110, 2288. [Google Scholar] [CrossRef]
- Catelan, M. Horizontal branch stars: The interplay between observations and theory, and insights into the formation of the Galaxy. Astrophys. Space Sci. 2009, 320, 261. [Google Scholar] [CrossRef]
- Catelan, M. Structure and Evolution of Low-Mass Stars: An Overview and Some Open Problems. In Proceedings of the GRADUATE SCHOOL IN ASTRONOMY: XI Special Courses at the National Observatory of Rio de Janeiro (XI CCE), Rio de Janeiro, Brazil, 16–20 October 2006; Alcaniz, J., Reza, R.d., Roig, F., Lopes, D.F., Eds.; AIP Conference Proceedings: Rio de Janeiro, Brazil, 2006; Volume 930, pp. 39–90. [Google Scholar]
- Catelan, M. The Evolutionary Status of M3 RR Lyrae Variable Stars: Breakdown of the Canonical Framework? Astrophys. J. 2004, 1, 409–418. [Google Scholar] [CrossRef]
- Marconi, M.; Nordgren, T.; Bono, G.; Schnider, G.; Caputo, F. Predicted and Empirical Radii of RR Lyrae Stars. Astrophys. J. 2005, 623, 133. [Google Scholar] [CrossRef]
- Smith, H.A. RR Lyrae Stars; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Lee, Y.-W. Evidence for an Old Galactic Bulge From RR Lyrae Stars in Baade’s Window: Implications for the Formation of the Galaxy and the Age of the Universe. Astron. J. 1992, 104, 1780. [Google Scholar] [CrossRef]
- Catelan, M.; Smith, H.A. Pulsating Stars; John Wiley & Sons, Incorporated: Hoboken, NJ, USA, 2015; pp. 157–182. [Google Scholar]
- Bailey, S.I. A discussion of variable stars in the cluster ω Centauri. Ann. Harv. Coll. Obs. 1902, 38, 1. [Google Scholar]
- Schwarzschild, M. Overtone Pulsations for the Standard Model. Astrophys. J. 1941, 94, 245. [Google Scholar] [CrossRef]
- Jerzykiewicz, M.; Wenzel, W. Analysis of the light variation of the RR Lyrae star AQ Leonis. Acta Astron. 1977, 27, 35. [Google Scholar]
- Cox, A.N.; Hodson, S.W.; Clancy, S.P. Double-mode RR Lyrae variables in M15. Astrophys. J. 1983, 266, 94–104. [Google Scholar] [CrossRef]
- Nemec, J.M. Double-mode RR Lyrae stars in M 15: Reanalysis and experiments with simulated photometry. Astron. J. 1985, 90, 240. [Google Scholar] [CrossRef]
- Clement, C.C.; Dickens, R.J.; Bingham, E.E. The RR Lyrae variable stars in the globular cluster IC 4499. Astron. J. 1979, 84, 217–230. [Google Scholar] [CrossRef]
- Walker, A.R.; Nemec, J.M. CCD Photometry of Galactic Globular Clusters. III. IC 4499 Astron. J. 1994, 112, 2026. [Google Scholar]
- Shapley, H. Studies based on the colors and magnitudes in stellar clusters. VI. On the determination of the distances of globular clusters. Astrophys. J. 1918, 48, 89. [Google Scholar] [CrossRef]
- Blanco, V.M.; Blanco, B.M. RR Lyraes in Baade’s Window and the value of Ro. Mem. Della Soc. Astron. Ital. 1985, 56, 15–22. [Google Scholar]
- Alcock, C.; Allsman, R.A.; Axelroc, T.S.; Bennett, D.P.; Cook, K.H.; Freeman, K.C.; Griest, K.; Guern, J.A.; Lehner, M.J.; Marshall, S.L.; et al. The MACHO Project First-Year Large Magellanic Cloud Results: The Microlensing Rate and the Nature of the Galactic Dark Halo. Astrophys. J. 1996, 461, 84. [Google Scholar] [CrossRef]
- Udalski, A.; Szymański, M.; Kaluzny, J.; Kubiak, M.; Mateo, M. The Optical Gravitational Lensing Experiment. Acta Astron. 1992, 42, 253–284. [Google Scholar]
- McWilliam, A.; Rich, R.M. The First Detailed Abundance Analysis of Galactic Bulge K Giants in Baade’s Window. Astrophys. J. Suppl. 1994, 91, 749. [Google Scholar] [CrossRef]
- Gonzalez, A.; Zoccali, M.; Vasquez, S.; Hill, V.; Rejkuba, M.; Valenti, E.; Rojas-Arriagada, A.; Renzini, A.; Babusiaux, C.; Minniti, D.; et al. The GIRAFFE Inner Bulge Survey (GIBS). II. Metallicity distributions and alpha element abundances at fixed Galactic latitude. Astron. Astrophys. 2015, 584, 46. [Google Scholar] [CrossRef]
- Johnson, C.I.; Rich, R.M.; Young, M.D.; Simion, I.T.; Clarkson, W.I.; Pilachowski, C.A.; Michael, S.; Kunder, A.; Koch, A.; Vivas, A.K. Blanco DECam Bulge Survey (BDBS) II: Project performance, data analysis, and early science results. Mon. Not. R. Astron. Soc. 2020, 499, 2357–2379. [Google Scholar] [CrossRef]
- Pancino, E.; Britavskiy, N.; Romano, D.; Cacciari, C.; Mucciarelli, A.; Clementini, G. Chemical abundances of solar neighbourhood RR Lyrae stars. Mon. Not. R. Astron. Soc. 2015, 447, 2404–2419. [Google Scholar] [CrossRef]
- Walker, A.R.; Terndrup, D.M. The Metallicity of RR Lyrae Stars in Baade’s Window. Astrophys. J. 1991, 378, 119. [Google Scholar] [CrossRef]
- Savino, A.; Koch, A.; Prudil, Z.; Kunder, A.; Smolec, R. The age of the Milky Way inner stellar spheroid from RR Lyrae population synthesis. Astron. Astrophys. 2020, 641, 96. [Google Scholar] [CrossRef]
- Zoccali, M.; Vasquez, S.; Gonzalez, O.A.; Valenti, E.; Rojas-Arriagada, A.; Minniti, J.; Rejkuba, M.; Minniti, D.; McWilliam, A.; Babusiaux, C.; et al. The GIRAFFE Inner Bulge Survey (GIBS). III. Metallicity distributions and kinematics of 26 Galactic bulge fields. Astron. Astrophys. 2017, 599, 12. [Google Scholar] [CrossRef]
- Rojas-Arriagada, A.; Zoccali, M.; Schultheis, M.; Recio-Blanco, A.; Zasowski, G.; Minniti, D.; Jönsson, H.; Cohen, R.E. The bimodal [Mg/Fe] versus [Fe/H] bulge sequence as revealed by APOGEE DR14. Astron. Astrophys. 2019, 626, 16. [Google Scholar] [CrossRef]
- Jurcsik, J.; Kovacs, G. Determination of [Fe/H] from the light curves of RR Lyrae stars. Astron. Astrophys. 1996, 312, 111–120. [Google Scholar]
- Sandate, A. The Metallicity Dependence of the Fourier Components of RR Lyrae Light Curves Is the Oosterhoff-Arp-Preston Period Ratio Effect in Disguise. Astron. J. 2004, 128, 858. [Google Scholar] [CrossRef]
- Szymański, M.; Udalski, A.; Soszyxnxski, I.; Kubiak, M.; Pietrzyxnxski, G.; Poleski, R.; Wyrzykowski, L.; Ulaczyk, K. Metallicity Dependence of the Blazhko Effect. Acta Astron. 2005, 55, 59–84. [Google Scholar]
- Nemec, J.M.; Cohen, J.G.; Ripepi, V.; Derekas, A.; Moskalik, P.; Sesar, B.; Chadid, M.; Bruntt, H. Metal Abundances, Radial Velocities, and Other Physical Characteristics for the RR Lyrae Stars in The Kepler Field. Astrophys. J. 2013, 773, 181. [Google Scholar] [CrossRef]
- Jurcsik, J. Revision of the [Fe/H] Scales Used for Globular Clusters and RR Lyrae Variables. Acta Astron. 1995, 45, 653–660. [Google Scholar]
- Salaris, M.; Chieffi, A.; Straniero, O. The alpha -enhanced Isochrones and Their Impact on the FITS to the Galactic Globular Cluster System. Astrophys. J. 1993, 414, 580. [Google Scholar] [CrossRef]
- Prudil, Z.; Dékány, I.; Grebel, E.K.; Catelan, M.; Skarka, M.; Smolec, R. Evidence for Galactic disc RR Lyrae stars in the solar neighbourhood. Mon. Not. R. Astron. Soc. 2019, 487, 3270–3278. [Google Scholar] [CrossRef]
- Zinn, R.; Chen, X.; Layden, A.C.; Casetti-Dinescu, D.I. Local RR Lyrae stars: Native and alien. Mon. Not. R. Astron. Soc. 2020, 492, 2161–2176. [Google Scholar] [CrossRef]
- Helmi, A.; Babusiaux, C.; Koppelman, H.H.; Massari, D.; Veljanoski, J.; Brown, A.G.A. The merger that led to the formation of the Milky Way’s inner stellar halo and thick disk. Nature 2018, 563, 85. [Google Scholar] [CrossRef]
- Mackereth, J.T.; Schiavon, R.P.; Pfeffer, J.; Hayes, C.R.; Bovy, J.; Anguiano, B.; Allende Prieto, C.; Hasselquist, S.; Holtzman, J.; Johnson, J.A.; et al. The origin of accreted stellar halo populations in the Milky Way using APOGEE, Gaia, and the EAGLE simulations. Mon. Not. R. Astron. Soc. 2019, 482, 3426. [Google Scholar] [CrossRef]
- Dékány, I.; Grebel, E.K.; Pojmański, G. Metallicity Estimation of RR Lyrae Stars From Their I-Band Light Curves. Astrophys. J. 2021, 920, 33. [Google Scholar] [CrossRef]
- Kunder, A.; Chaboyer, B. Metallicity Analysis of MACHO Galactic Bulge RR0 Lyrae Stars from their Light Curves. Astron. J. 2008, 136, 2441–2452. [Google Scholar] [CrossRef]
- Pietrukowicz, P.; Udalski, A.; Soszy’nski, I.; Nataf, D.M.; Wyrzykowski, L.; Poleski, R.; Kozlowski, S.; Szymański, M.K.; Kubiak, M.; Pietrzyński, G.; et al. The Optical Gravitational Lensing Experiment: Analysis of the Bulge RR Lyrae Population from the OGLE-III Data. Astrophys. J. 2012, 750, 169. [Google Scholar] [CrossRef]
- Pietrukowicz, P.; Kozlowski, S.; Skowron, J.; Soszyński, I.; Udalski, A.; Poleski, R.; Wyrzykowski, L.; Szymański, M.K.; Pietrzyński, G.; Ulaczyk, K.; et al. Deciphering the 3D Structure of the Old Galactic Bulge from the OGLE RR Lyrae Stars. Astrophys. J. 2015, 811, 113. [Google Scholar] [CrossRef]
- Zinn, R.; West, M.J. The globular cluster system of the Galaxy. III. Measurements of radial velocity and metallicity for 60 clusters and a compilation of metallicities for 121 clusters. Astrophys. J. Suppl. Ser. 1984, 55, 45–66. [Google Scholar] [CrossRef]
- Pietrukowicz, P.; Udalski, A.; Soszyński, I.; Skowron, D.M.; Wrona, M.; Szymański, M.K.; Poleski, R.; Ulaczyk, K.; Kozlowski, S.; Skowron, J.; et al. Properties of the Milky Way’s Old Populations Based on Photometric Metallicities of the OGLE RR Lyrae Stars. Acta Astron. 2020, 70, 121–139. [Google Scholar]
- Clarkson, W.; Sahu, K.; Anderson, J.; Smith, T.E.; Brown, T.M.; Rich, R.M.; Casertano, S.; Bond, H.E.; Livio, M.; Minniti, D.; et al. Stellar Proper Motions in the Galactic Bulge from Deep Hubble Space Telescope ACS WFC Photometry. Astrophys. J. 2008, 684, 1110–1142. [Google Scholar] [CrossRef]
- Renzini, A.; Gennaro, M.; Zoccali, M.; Brown, T.M.; Anderson, J.; Minniti, D.; Sahu, K.C.; Valenti, E.; VandenBerg, D.A. The WFC3 Galactic Bulge Treasury Program: Relative Ages of Bulge Stars of High and Low Metallicity. Astrophys. J. 2018, 863, 16. [Google Scholar] [CrossRef]
- Haywood, M.; Di Matteo, P.; Snaith, O.; Calamida, A. Hiding its age: The case for a younger bulge. Astron. Astrophys. 2016, 593, 82. [Google Scholar] [CrossRef]
- Bensby, T.; Feltzing, S.; Gould, A.; Yee, J.C.; Johnson, J.A.; Asplund, M.; Meléndez, J.; Lucatello, S.; Howes, L.M.; McWilliam, A.; et al. Chemical evolution of the Galactic bulge as traced by microlensed dwarf and subgiant stars. VI. Age and abundance structure of the stellar populations in the central sub-kpc of the Milky Way. Astron. Astrophys. 2017, 605, 89. [Google Scholar] [CrossRef]
- Pietrinferni, A.; Cassisi, S.; Salaris, M.; Castelli, F. A Large Stellar Evolution Database for Population Synthesis Studies. II. Stellar Models and Isochrones for an α-enhanced Metal Distribution. Astron. J. 2006, 642, 797. [Google Scholar] [CrossRef]
- Hasselquist, S.; Zasowski, G.; Feuillet, D.K.; Schultheis, M.; Nataf, D.M.; Anguiano, B.; Beaton, R.L.; Beers, T.C.; Cohen, R.E.; Cunha, K. Exploring the Stellar Age Distribution of the Milky Way Bulge Using APOGEE. Astrophys. J. 2020, 901, 109. [Google Scholar] [CrossRef]
- Zoccali, M.; Renzini, A.; Ortolani, S.; Greggio, L.; Saviane, I.; Cassisi, S.; Rejkuba, M.; Barbuy, B.; Rich, R.M.; Bica, E. Age and metallicity distribution of the Galactic bulge from extensive optical and near-IR stellar photometry. Astron. Astrophys. 2003, 399, 931–956. [Google Scholar] [CrossRef]
- Clarkson, W.; Sahu, K.; Anderson, J.; Rich, R.M.; Smith, T.E.; Brown, T.M.; Bond, H.E.; Livio, M.; Minniti, D.; Renzini, A.; et al. The First Detection of Blue Straggler Stars in the Milky Way Bulge. Astrophys. J. 2011, 735, 37. [Google Scholar] [CrossRef]
- Barbuy, B.; Chiappini, C.; Gerhard, O. Chemodynamical History of the Galactic Bulge. Annu. Rev. Astron. Astrophys. 2018, 56, 223–276. [Google Scholar] [CrossRef]
- Van Loon, J.T.; Gilmore, G.F.; Omont, A.; Blommaert, J.A.D.L.; Glass, I.S.; Messineo, M.; Schuller, F.; Schultheis, M.; Yamamura, I.; Zhao, H.S. Infrared stellar populations in the central parts of the Milky Way galaxy. Mon. Not. R. Astron. Soc. 2003, 338, 857–879. [Google Scholar] [CrossRef][Green Version]
- Bensby, T.; Yee, J.C.; Feltzing, S.; Johnson, J.A.; Gould, A.; Cohen, J.G.; Asplund, M.; Meléndez, J.; Lucatello, S.; Han, C.; et al. Chemical evolution of the Galactic bulge as traced by microlensed dwarf and subgiant stars. V. Evidence for a wide age distribution and a complex MDF. Astron. Astrophys. 2013, 549, 147. [Google Scholar] [CrossRef]
- Catchpole, R.M.; Whitelock, P.A.; Feast, M.W.; Hughes, S.M.G.; Irwin, M.; Alard, C. The age and structure of the Galactic bulge from Mira variables. Mon. Not. R. Astron. Soc. 2016, 455, 2216–2227. [Google Scholar] [CrossRef]
- Blitz, L.; Spergel, D.N. Direct Evidence for a Bar at the Galactic Center. Astrophys. J. 1991, 379, 631–638. [Google Scholar] [CrossRef]
- Dwek, E.; Arendt, R.G.; Hauser, M.G.; Kelsall, T.; Lisse, C.M.; Moseley, S.H.; Silverberg, R.F.; Sodroski, T.J.; Weil, J.L. Morphology, Near-Infrared Luminosity, and Mass of the Galactic Bulge from COBE DIRBE Observations. Astrophys. J. 1995, 445, 716. [Google Scholar] [CrossRef]
- Sandage, A. The Oosterhoff period groups and the age of globular clusters. II. Properties of RR Lyrae stars in six clusters: The P-L-A relation. Astrophys. J. 1981, 248, 161. [Google Scholar] [CrossRef]
- Sandage, A. Evidence for a period-luminosity-amplitude relation for RR Lyrae stars. Astrophys. J. 1981, 244, 23. [Google Scholar] [CrossRef]
- Caputo, F. The period-magnitude diagram of RR Lyrae stars—I. The controversy about the distance scale. Mon. Not. R. Astron. Soc. 1997, 284, 994. [Google Scholar] [CrossRef]
- Catelan, M.; Pritzl, B.J.; Smith, H.A. The RR Lyrae Period-Luminosity Relation. I. Theoretical Calibration. Astrophys. J. Suppl. Ser. 2004, 154, 633. [Google Scholar] [CrossRef]
- Demarque, P.; Zinn, R.; Lee, Y.-W.; Yi, S. The Metallicity Dependence of RR Lyrae Absolute Magnitudes from Synthetic Horizontal-Branch Models. Astron. J. 2000, 119, 1398. [Google Scholar] [CrossRef][Green Version]
- Caputo, F.; Castellani, V.; Marconi, M.; Ripepi, V. Pulsational MV versus [Fe/H] relation(s) for globular cluster RR Lyrae variables. Mon. Not. R. Astron. Soc. 2000, 316, 819–826. [Google Scholar] [CrossRef][Green Version]
- Gould, A.; Popowski, P. Systematics of RR Lyrae Statistical Parallax. III. Apparent Magnitudes and Extinctions. Astrophys. J. 1998, 508, 844–853. [Google Scholar] [CrossRef][Green Version]
- Fernley, J.; Carney, B.W.; Skillen, I.; Cacciari, C.; Janes, K. Radial velocities and iron abundances of field RR Lyraes. I. Mon. Not. R. Astron. Soc. 1998, 293, 61. [Google Scholar] [CrossRef]
- Sandage, A. The Oosterhoff Period-Metallicity Relation for RR Lyrae Stars at the Blue Fundamental Edge of the Instability Strip. I. Astrophys. J. 1993, 106, 687. [Google Scholar] [CrossRef]
- Feast, M.W. RR Lyraes, Galactic and extragalactic distances, and the age of the oldest globular clusters. Mon. Not. R. Astron. Soc. 1997, 284, 761–766. [Google Scholar] [CrossRef][Green Version]
- Fusi Pecci, F. The M(v)-HB Verses [Fe/H] Calibration. I. HST Color-Magnitude Diagrams of Eight Globular Clusters in M31. Astron. J. 1996, 112, 1461. [Google Scholar] [CrossRef][Green Version]
- Chaboyer, B.C. Globular Cluster Distance Determinations. In Post-Hipparcos Cosmic Candles; Heck, A., Caputo, F., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1999; Volume 137, p. 111. [Google Scholar]
- Clementini, G.; Gratton, R.; Bragaglia, A.; Carretta, E.; Di Fabrizio, L.; Maio, M. Distance to the Large Magellanic Cloud: The RR Lyrae Stars. Astron. J. 2003, 125, 1309–1329. [Google Scholar] [CrossRef]
- Gratton, R.G.; Bragaglia, A.; Clementini, G.; Carretta, E.; Di Fabrizio, L.; Maio, M.; Taribello, E. Metal abundances of RR Lyrae stars in the bar of the Large Magellanic Cloud. Astron. Astrophys. 2004, 421, 937. [Google Scholar] [CrossRef]
- Dambis, A.K.; Berdnikov, L.N.; Kniazev, A.Y.; Kravtsov, V.V.; Rastorguev, A.S.; Sefako, R.; Vozyakova, O.V. RR Lyrae variables: Visual and infrared luminosities, intrinsic colours and kinematics. Mon. Not. R. Astron. Soc. 2013, 435, 3206–3220. [Google Scholar] [CrossRef]
- Muraveva, T.; Delgado, H.E.; Clementini, G.; Sarro, L.M.; Garofalo, A. RR Lyrae stars as standard candles in the Gaia Data Release 2 Era. Mon. Not. R. Astron. Soc. 2018, 481, 1195–1211. [Google Scholar] [CrossRef]
- Clementini, G.; Ripepi, V.; Molinaro, R.; Garofalo, A.; Muraveva, T.; Rimoldini, L.; Guy, L.P.; Jevardat de Fombelle, G.; Nienartowicz, K.; Marchal, O.; et al. Gaia Data Release 2. Specific characterisation and validation of all-sky Cepheids and RR Lyrae stars. Astron. Astrophys. 2019, 622, 60. [Google Scholar] [CrossRef]
- Longmore, A.J.; Fernley, J.A.; Jameson, R.F. RR Lyrae stars in globular clusters: Better distances from infrared measurements? Mon. Not. R. Astron. Soc. 1986, 220, 279–287. [Google Scholar] [CrossRef]
- Dall’Ora, M.; Storm, J.; Bono, G.; Ripepi, V.; Monelli, M.; Testa, V.; Andreuzzi, G.; Buonanno, R.; Caputo, F.; Castellani, V.; et al. The Distance to the Large Magellanic Cloud Cluster Reticulum from the K-Band Period-Luminosity-Metallicity Relation of RR Lyrae Stars. Astrophys. J. 2004, 610, 269–274. [Google Scholar] [CrossRef]
- Bono, G.; Caputo, F.; Castellani, V.; Marconi, M.; Storm, J.; Degl’Innocenti, S. A pulsational approach to near-infrared and visual magnitudes of RR Lyr stars. Mon. Not. R. Astron. Soc. 2003, 344, 1097–1106. [Google Scholar] [CrossRef]
- Marconi, M.; Coppola, G.; Bono, G.; Braga, V.; Pietrinferni, A.; Buonanno, R.; Castellani, M.; Musella, I.; Ripepi, V.; Stellingwerf, R.F. On a New Theoretical Framework for RR Lyrae Stars. I. The Metallicity Dependence. Astrophys. J. 2015, 808, 50. [Google Scholar] [CrossRef]
- Madore, B.F.; Hoffman, D.; Freedman, W.L.; Kollmeier, J.A.; Monson, A.; Persson, S.E.; Rich, J.A., Jr.; Scowcroft, V.; Seibert, M. Predicted and Empirical Radii of RR Lyrae Stars. Astrophys. J. 1994, 776, 135. [Google Scholar] [CrossRef]
- Dambis, A.K.; Rastorguev, A.S.; Rastorguev, A.S.; Zabolotskikh, M.V. Mid-infrared period-luminosity relations for globular cluster RR Lyrae. Mon. Not. R. Astron. Soc. 2014, 439, 3765. [Google Scholar] [CrossRef]
- Braga, V.F.; Dall’Ora, M.; Bono, G.; Stetson, P.B.; Ferraro, I.; Iannicola, G.; Marengo, M.; Neeley, J.; Persson, S.E.; Buonanno, R.; et al. On the Distance of the Globular Cluster M4 (NGC 6121) Using RR Lyrae Stars. I. Optical and Near-infrared Period-Luminosity and Period-Wesenheit Relations. Astrophys. J. 2015, 799, 165. [Google Scholar] [CrossRef]
- Neeley, J.R.; Marengo, M.; Bono, G.; Braga, V.F.; Dall’Ora, M.; Stetson, P.B.; Buonanno, R.; Ferraro, I.; Freedman, W.L.; Iannicola, G.; et al. On the Distance of the Globular Cluster M4 (NGC 6121) Using RR Lyrae Stars. II. Mid-infrared Period-luminosity Relations. Astrophys. J. 2015, 808, 11. [Google Scholar] [CrossRef]
- Delgado, H.E.; Sarro, L.M.; Clementini, G.; Muraveva, T.; Garofalo, A. Hierarchical Bayesian model to infer PL(Z) relations using Gaia parallaxes. Astron. Astrophys. 2019, 623, 156. [Google Scholar] [CrossRef]
- Layden, A.C.; Tiede, G.P.; Chaboyer, B.; Bunner, C.; Smitka, M.T. Infrared K-band Photometry of Field RR Lyrae Variable Stars. Astron. J. 2019, 158, 105. [Google Scholar] [CrossRef]
- Cusano, F.; Moretti, M.I.; Clementini, G.; Ripepi, V.; Marconi, M.; Cioni, M.-R.L.; Rubele, S.; Garofalo, A.; de Grijs, R.; Groenewegen, M.A.T.; et al. The VMC Survey—XLII. Near-infrared period-luminosity relations for RR Lyrae stars and the structure of the Large Magellanic Cloud. Mon. Not. R. Astron. Soc. 2021, 504, 1–15. [Google Scholar] [CrossRef]
- Neeley, J.R.; Massimo, M.; Freedman, W.L.; Madore, B.F.; Beaton, R.L.; Hatt, D.; Hoyt, T.; Monson, A.J.; Rich, J.A.; Sarajedini, A.; et al. Standard Galactic field RR Lyrae II: A Gaia DR2 calibration of the period-Wesenheit-metallicity relation. Mon. Not. R. Astron. Soc. 2019, 490, 4254–4270. [Google Scholar] [CrossRef]
- Muhie, T.D.; Dambis, A.K.; Berdnikov, L.N.; Kniazev, A.Y.; Grebel, E.K. Kinematics and Multi Band Period-Luminosity-Metallicity Relation of RR Lyrae Stars via Statistical Parallax. Mon. Not. R. Astron. Soc. 2021, 502, 4074–4092. [Google Scholar] [CrossRef]
- Dékány, I.; Minniti, D.; Catelan, M.; Zoccali, M.; Saito, R.K.; Hempel, M.; Gonzalez, O.A. VVV Survey Near-infrared Photometry of Known Bulge RR Lyrae Stars: The Distance to the Galactic Center and Absence of a Barred Distribution of the Metal-poor Population. Astrophys. J. Lett. 2013, 776, 19. [Google Scholar] [CrossRef]
- Gran, F.; Minniti, D.; Saito, R.K.; Navarrete, C.; Dékány, I.; McDonald, I.; Ramos, R.C.; Catelan, M. Bulge RR Lyrae stars in the VVV tile b201. Astron. Astrophys. 2015, 575, 114–123. [Google Scholar] [CrossRef]
- Muraveva, T.; Palmer, M.; Clementini, G.; Luri, X.; Cioni, M.-R.L.; Moretti, M.I.; Marconi, M.; Ripepi, V.; Rubele, S. New Near-infrared Period-Luminosity-Metallicity Relations for RR Lyrae Stars and the Outlook for Gaia. Astrophys. J. 2015, 807, 127. [Google Scholar] [CrossRef]
- Minniti, D.; Dékany, I.; Majaess, D.; Palma, T.; Pullen, J.; Rejkuba, M.; Alonso-García, J.; Catelan, M.; Contreras Ramos, R. Characterization of the VVV Survey RR Lyrae Population across the Southern Galactic Plane. Astron. J. 2017, 153, 179. [Google Scholar] [CrossRef]
- Minniti, D.; Palma, T.; Dékany, I.; Hempel, M.; Rejkuba, M.; Pullen, J.; Alonso-García, J.; Barbá, R.; Barbuy, B.; Bica, E.; et al. FSR 1716: A New Milky Way Globular Cluster Confirmed Using VVV RR Lyrae Stars. Astrophys. J. Lett. 2017, 838, 14. [Google Scholar] [CrossRef]
- Oliveira, R.A.P.; Ortolani, S.; Barbuy, B.; Kerber, L.O.; Maia, F.F.S.; Bica, E.; Cassisi, S.; Souza, S.O.; Pérez-Villegas, A. Precise distances from OGLE-IV member RR Lyrae stars in six bulge globular clusters. Astron. Astrophys. 2022, 657, 123. [Google Scholar] [CrossRef]
- Alonso-García, J.; Smith, L.C.; Catelan, M.; Minniti, D.; Navarrete, C.; Borissova, J.; Carballo-Bello, J.A.; Contreras Ramos, R.; Fernández-Trincado, J.G.; Ferreira Lopes, C.E.; et al. Variable stars in the VVV globular clusters. II. NGC 6441, NGC 6569, NGC 6626 (M 28), NGC 6656 (M 22), 2MASS-GC 02, and Terzan 10. Astron. Astrophys. 2021, 651, 18. [Google Scholar] [CrossRef]
- Gonzalez, O.A.; Rejkuba, M.; Zoccali, M.; Valenti, E.; Minniti, D.; Schultheis, M.; Tobar, R.; Chen, B. Reddening and metallicity maps of the Milky Way bulge from VVV and 2MASS. II. The complete high resolution extinction map and implications for Galactic bulge studies. Astron. Astrophys. 2012, 543, 13. [Google Scholar] [CrossRef]
- Surot, F.; Valenti, E.; Gonzalez, O.A.; Zoccali, M.; Sökmen, E.; Hidalgo, S.L.; Minniti, D. Mapping the stellar age of the Milky Way bulge with the VVV. III. High-resolution reddening map. Astron. Astrophys. 2020, 644, 140. [Google Scholar] [CrossRef]
- Gosling, A.J.; Bandyopadhyay, R.M.; Blundell, K.M. The complex, variable near-infrared extinction towards the Nuclear Bulge. Mon. Not. R. Astron. Soc. 2009, 394, 2247–2254. [Google Scholar] [CrossRef]
- Nataf, D.M.; Gonzalez, O.A.; Casagrande, L.; Zasowski, G.; Wegg, C.; Wolf, C.; Kunder, A.; Alonso-Garcia, J.; Minniti, D.; Rejkuba, M.; et al. Interstellar extinction curve variations towards the inner Milky Way: A challenge to observational cosmology. Mon. Not. R. Astron. Soc. 2016, 456, 2692–2706. [Google Scholar] [CrossRef]
- Alcock, C.; Allsman, R.A.; Alves, D.R.; Axelrod, T.S.; Becker, A.C.; Basu, A.; Baskett, L.; Bennett, D.P.; Cook, K.H.; Freeman, K.C.; et al. The RR Lyrae Population of the Galactic Bulge from the MACHO Database: Mean Colors and Magnitudes. Astrophys. J. 1998, 492, 190–199. [Google Scholar] [CrossRef]
- Stanek, K.Z.; Mateo, M.; Udalski, A.; Szymanski, M.; Kaluzny, J.; Kubiak, M. Color-Magnitude Diagram Distribution of the Bulge Red Clump Stars: Evidence for the Galactic Bar. Astrophys. J. Lett. 1994, 429, 73. [Google Scholar] [CrossRef]
- Alcock, C.; Allsman, R.A.; Alves, D.R.; Axelrod, T.S.; Becker, A.C.; Bennett, D.P.; Cook, K.H.; Drake, A.J.; Freeman, K.C.; Geha, M.; et al. Calibration of the MACHO Photometry Database. Publ. Astron. Soc. Pac. 1999, 111, 1539–1558. [Google Scholar] [CrossRef]
- Szymański, M.; Udalski, A.; Soszyxnxski, I.; Kubiak, M.; Pietrzyxnxski, G.; Poleski, R.; Wyrzykowski, L.; Ulaczyk, K. The Optical Gravitational Lensing Experiment. OGLE-III Photometric Maps of the Galactic Bulge Fields. Acta Astron. 2011, 61, 83–102. [Google Scholar]
- Contreras Ramos, R.; Minniti, D.; Gran, F.; Zoccali, M.; Alonso-García, J.l.; Huijse, P.; Navarro, M.G.; Rojas-Arriagada, A.; Valenti, E. The VVV Survey RR Lyrae Population in the Galactic Center Region. Astrophys. J. 2018, 863, 79. [Google Scholar] [CrossRef]
- Soszyński, I.; Dziembowski, W.A.; Udalski, A.; Poleski, R.; Szymański, M.K.; Kubiak, M.; Pietrzyński, G.; Wyrzykowski, L.; Ulaczyk, K.; Kozłowski, S.; et al. The Optical Gravitational Lensing Experiment. The OGLE-III Catalog of Variable Stars. XI. RR Lyrae Stars in the Galactic Bulge. Acta Astron. 2011, 61, 1. [Google Scholar]
- Dékány, I.; Hajdu, G.; Grebel, E.K.; Catelan, M.; Elorrieta, F.; Eyheramendy, S.; Majaess, D.; Jordxaxn, A. A Near-infrared RR Lyrae Census along the Southern Galactic Plane: The Milky Way’s Stellar Fossil Brought to Light. Astrophys. J. 2018, 857, 54. [Google Scholar] [CrossRef]
- Dékány, I.; Grebel, E.K. Near-infrared Search for Fundamental-mode RR Lyrae Stars toward the Inner Bulge by Deep Learning. Astrophys. J. 2020, 898, 46. [Google Scholar] [CrossRef]
- Kunder, A.; Pérez-Villegas, A.; Rich, R.M.; Ogata, J.; Murari, E.; Boren, E.; Johnson, C.I.; Nataf, D.; Walker, A.; Bono, G.; et al. The Bulge Radial Velocity Assay for RR Lyrae Stars (BRAVA-RR) DR2: A Bimodal Bulge? Astron. J. 2020, 159, 270. [Google Scholar] [CrossRef]
- Alonso-García, J.; Dékány, I.; Catelan, M.; Ramos, R.C.; Gran, F.; Amigo, P.; Leyton, P.; Minniti, D. Variable Stars in the VVV Globular Clusters. I. 2MASS-GC 02 and Terzan 10. Astron. J. 2015, 149, 99. [Google Scholar] [CrossRef]
- Cardelli, J.A.; Clayton, G.C.; Mathis, J.S. The Relationship between Infrared, Optical, and Ultraviolet Extinction. Astrophys. J. 1989, 345, 245. [Google Scholar] [CrossRef]
- Queiroz, A.B.A.; Anders, F.; Chiappini, C.; Khalatyan, A.; Santiago, B.X.; Steinmetz, M.; Valentini, M.; Miglio, A.; Bossini, D.; Barbuy, B.; et al. From the bulge to the outer disc: StarHorse stellar parameters, distances, and extinctions for stars in APOGEE DR16 and other spectroscopic surveys. Astron. Astrophys. 2020, 638, 76. [Google Scholar] [CrossRef]
- Pérez-Villegas, A.; Portail, M.; Gerhard, O. The stellar halo in the inner Milky Way: Predicted shape and kinematics. Mon. Not. R. Astron. Soc. 2017, 464, 80–84. [Google Scholar] [CrossRef]
- Minkowski, R. The Sub-System of Planetary Nebulae. Publ. Astron. Soc. Pac. 1964, 76, 197. [Google Scholar] [CrossRef]
- Catchpole, R.M. Rotation of the galactic bulge In Bulges of galaxies, ESO Conference and Workshop Proceedings, La Serena, Chile, 1990; Jarvis, B.J., Terndrup, D.M., Eds. 111. Astrophys. J. 2004, 1, 409–418. [Google Scholar]
- Minniti, D.; White, S.D.M.; Olszewski, E.W.; Hill, J.M. Rotation of the Galactic Bulge. Astrophys. J. Lett. 1992, 393, 47. [Google Scholar] [CrossRef]
- Beaulieu, S.F.; Freeman, K.C.; Kalnajs, A.J.; Saha, P.; Zhao, H. Dynamics of the Galactic Bulge Using Planetary Nebulae. Astron. J. 2000, 120, 855–871. [Google Scholar] [CrossRef]
- Rich, R.M.; Reitzel, D.B.; Howard, C.D.; Zhao, H. The Bulge Radial Velocity Assay: Techniques and a Rotation Curve. Astrophys. J. 2007, 658, 29. [Google Scholar] [CrossRef]
- Kunder, A.; Koch, A.; Rich, R.M.; de Propris, R.; Howard, C.D.; Stubbs, S.A.; Johnson, C.I.; Shen, J.; Wang, Y.; Robin, A.C.; et al. The Bulge Radial Velocity Assay (BRAVA). II. Complete Sample and Data Release. Astron. J. 2012, 143, 57. [Google Scholar] [CrossRef]
- Howard, C.D.; Rich, R.M.; Clarkson, W.; Mallery, R.; Kormendy, J.; De Propris, R.; Robin, A.C.; Fux, R.; Reitzel, D.B.; Zhao, H.S.; et al. Kinematics at the Edge of the Galactic Bulge: Evidence for Cylindrical Rotation. Astrophys. J. Lett. 2009, 702, 153–157. [Google Scholar] [CrossRef]
- Molaeinezhad, A.; Falcón-Barroso, J.; Martínez-Valpuesta, I.; Khosroshahi, H.G.; Balcells, M.; Peletier, R.F. Establishing the level of cylindrical rotation in boxy/peanut bulges. Mon. Not. R. Astron. Soc. 2016, 456, 692. [Google Scholar] [CrossRef]
- Shen, J.; Rich, R.M.; Kormendy, J.l.; Howard, C.D.; De Propris, R.; Kunder, A. Our Milky Way as a Pure-disk Galaxy—A Challenge for Galaxy Formation. Astrophys. J. Lett. 2010, 720, 72–76. [Google Scholar] [CrossRef]
- Ness, M.; Freeman, K.; Athanassoula, E.; Wylie-de-Boer, E.; Bland-Hawthorn, J.; Asplund, M.; Lewis, G.F.; Yong, D.; Lane, R.R.; Kiss, L.L. ARGOS–III. Stellar populations in the Galactic bulge of the Milky Way. Mon. Not. R. Astron. Soc. 2013, 430, 836–857. [Google Scholar] [CrossRef]
- Ness, M.; Freeman, K.; Athanassoula, E.; Wylie-de-Boer, E.; Bland-Hawthorn, J.; Asplund, M.; Lewis, G.F.; Yong, D.; Lane, R.R.; Kiss, L.L.; et al. ARGOS—IV. The kinematics of the Milky Way bulge. Mon. Not. R. Astron. Soc. 2013, 432, 2092–2103. [Google Scholar] [CrossRef]
- Salaris, M.; Girardi, L. Population effects on the red giant clump absolute magnitude: The K band. Mon. Not. R. Astron. Soc. 2002, 337, 332–340. [Google Scholar] [CrossRef]
- Zoccali, M.; Gonzalez, O.A.; Vasquez, S.; Hill, V.; Rejkuba, M.; Valenti, E.; Renzini, A.; Rojas-Arriagada, A.; Martinez-Valpuesta, I.; Babusiaux, C.; et al. The GIRAFFE Inner Bulge Survey (GIBS). I. Survey description and a kinematical map of the Milky Way bulge. Astron. Astrophys. 2014, 562, 66. [Google Scholar] [CrossRef]
- Ness, M.; Zasowski, G.; Johnson, J.A.; Athanassoula, E.; Majewski, S.R.; García Pérez, A.E.; Bird, J.; Nidever, D.; Donald, P.S.; Sobeck, J.; et al. APOGEE Kinematics. I. Overview of the Kinematics of the Galactic Bulge as Mapped By APOGEE. Astrophys. J. 2016, 819, 2–18. [Google Scholar] [CrossRef]
- Zasowski, G.; Ness, M.K.; Pérez, A.E.G.; Martinez-Valpuesta, I.; Johnson, J.A.; Majewski, S.R. Kinematics in the Galactic Bulge with APOGEE. II. High-Order Kinematic Moments and Comparison to Extragalactic Bar Diagnostics. Astrophys. J. 2016, 832, 132–146. [Google Scholar] [CrossRef]
- Rojas-Arriagada, A.; Recio-Blanco, A.; Hill, V.; de Laverny, P.; Schultheis, M.; Babusiaux, C.; Zoccali, M.; Minniti, D.; Gonzalez, O.A.; Feltzing, S.; et al. The Gaia-ESO Survey: Metallicity and kinematic trends in the Milky Way bulge. Astron. Astrophys. 2014, 569, 103. [Google Scholar] [CrossRef]
- Rojas-Arriagada, A.; Recio-Blanco, A.; de Laverny, P.; Mikolaitis, S.; Matteucci, F.; Spitoni, E.; Schultheis, M.; Hayden, M.; Hill, V.; Zoccali, M.; et al. The Gaia-ESO Survey: Exploring the complex nature and origins of the Galactic bulge populations. Astron. Astrophys. 2017, 601, 140. [Google Scholar] [CrossRef]
- Zoccali, M.; Valenti, E.; Gonzalez, O.A. Weighing the two stellar components of the Galactic bulge. Astron. Astrophys. 2018, 618, 147–153. [Google Scholar] [CrossRef]
- Wegg, C.; Gerhard, O. Mapping the three-dimensional density of the Galactic bulge with VVV red clump stars. Mon. Not. R. Astron. Soc. 2013, 435, 1874–1887. [Google Scholar] [CrossRef]
- Kormendy, J.; Kennicutt, R.C. Secular Evolution and the Formation of Pseudobulges in Disk Galaxies. Annu. Rev. Astron. Astrophys. 2004, 42, 603–683. [Google Scholar] [CrossRef]
- Combes, F.; Debbasch, F.; Friedli, D.; Pfenniger, D. Box and peanut shapes generated by stellar bars. Astron. Astrophys. 1990, 233, 82. [Google Scholar]
- Zhao, H.; Rich, R.M.; Biello, J. Proper-Motion Anisotropy, Rotation, and the Shape of the Galactic Bulge. Astrophys. J. 1996, 470, 506. [Google Scholar] [CrossRef]
- Athanassoula, E. On the nature of bulges in general and of box/peanut bulges in particular: Input from N-body simulations. Mon. Not. R. Astron. Soc. 2005, 358, 1477–1488. [Google Scholar] [CrossRef]
- Raha, N.; Sellwood, J.A.; James, R.A.; Kahn, F.D. A dynamical instability of bars in disk galaxies. Nature 1991, 352, 411–412. [Google Scholar] [CrossRef]
- Martinez-Valpuesta, I.; Gerhard, O. Metallicity Gradients Through Disk Instability: A Simple Model for the Milky Way’s Boxy Bulge. Astrophys. J. 2013, 766, 3. [Google Scholar] [CrossRef]
- Horta, D.; Schiavon, R.P.; Mackereth, J.T.; Pfeffer, J.; Mason, A.C.; Kisku, S.; Fragkoudi, F.; Allende Prieto, C.; Cunha, K.; Hasselquist, S.; et al. Evidence from APOGEE for the presence of a major building block of the halo buried in the inner Galaxy. Mon. Not. R. Astron. Soc. 2021, 500, 1385–1403. [Google Scholar] [CrossRef]
- Kruijssen, D.J.M.; Pfeffer, J.L.; Reina-Campos, M.; Crain, R.A.; Bastian, N. The formation and assembly history of the Milky Way revealed by its globular cluster population. Mon. Not. R. Astron. Soc. 2019, 486, 3180. [Google Scholar] [CrossRef]
- Belokurov, V.; Erkal, D.; Evans, N.W.; Koposov, S.E.; Deason, A.J. Co-formation of the disc and the stellar halo. Mon. Not. R. Astron. Soc. 2018, 478, 611. [Google Scholar] [CrossRef]
- Ibata, R.A.; Gilmore, G.; Irwin, M.J. A dwarf satellite galaxy in Sagittarius. Nature 1994, 370, 194. [Google Scholar] [CrossRef]
- Schultheis, M.; Cunha, K.; Zasowski, G.; García Pérez, A.E.; Sellgren, K.; Smith, V.; García-Hernández, D.A.; Zamora, O.; Fritz, T.K.; Anders, F.; et al. Evidence for a metal-poor population in the inner Galactic bulge. Astron. Astrophys. 2015, 584, 45. [Google Scholar] [CrossRef]
- Rich, R.M.; Origlia, L.; Valenti, E. The First Detailed Abundances for M Giants in the Inner Bulge from Infrared Spectroscopy. Astrophys. J. 2007, 665, 119–122. [Google Scholar] [CrossRef]
- Cunha, K.; Sellgren, K.; Smith, V.V.; Ramirez, S.V.; Blum, R.D.; Terndrup, D.M. Chemical Abundances of Luminous Cool Stars in the Galactic Center from High-Resolution Infrared Spectroscopy. Astrophys. J. 2007, 669, 1011–1023. [Google Scholar] [CrossRef]
- Howes, L.M.; Asplund, M.; Casey, A.R. The Gaia-ESO Survey: The most metal-poor stars in the Galactic bulge. Mon. Not. R. Astron. Soc. 2014, 445, 4241. [Google Scholar] [CrossRef]
- Howes, L.M.; Casey, A.R.; Asplund, M.; Keller, S.C.; Yong, D.; Nataf, D.M.; Poleski, R.; Lind, K.; Kobayashi, C.; Owen, C.I.; et al. Extremely metal-poor stars from the cosmic dawn in the bulge of the Milky Way. Nature 2015, 527, 484. [Google Scholar] [CrossRef]
- Howes, L.M.; Asplund, M.; Keller, S.C.; Casey, A.R.; Yong, D.; Lind, K.; Frebel, A.; Hays, A.; Alves-Brito, A.; Bessell, M.S.; et al. The EMBLA survey—Metal-poor stars in the Galactic bulge. Mon. Not. R. Astron. Soc. 2016, 460, 884–901. [Google Scholar] [CrossRef]
- Arentsen, A.; Starkenburg, E.; Martin, N.F.; Hill, V.; Ibata, R.; Kunder, A.; Schultheis, M.; Venn, K.A.; Zucker, D.B.; Aguado, D.; et al. The Pristine Inner Galaxy Survey (PIGS) I: Tracing the kinematics of metal-poor stars in the Galactic bulge. Mon. Not. R. Astron. Soc. 2020, 491, 11–16. [Google Scholar] [CrossRef]
- Di Matteo, P. The Disc Origin of the Milky Way Bulge. Publ. Astron. Soc. Aust. 2016, 33, 27. [Google Scholar] [CrossRef]
- Debattista, B.P.; Ness, M.; Gonzalez, O.A.; Freeman, K.; Zoccali, M.; Minniti, D. Separation of stellar populations by an evolving bar: Implications for the bulge of the Milky Way. Mon. Not. R. Astron. Soc. 2017, 469, 1587–1611. [Google Scholar] [CrossRef]
- Arentsen, A.; Starkenburg, E.; Aguado, D.S.; Martin, N.F.; Placco, V.M.; Carlberg, R.; González Hernández, J.I.; Hill, V.; Jablonka, P.; Kordopatis, G.; et al. The Pristine Inner Galaxy Survey (PIGS) III: Carbon-enhanced metal-poor stars in the bulge. Mon. Not. R. Astron. Soc. 2021, 505, 1239–1253. [Google Scholar] [CrossRef]
- Kunder, A.; Rich, R.M.; Koch, A.; Storm, J.; Nataf, D.M.; De Propris, R.; Walker, A.R.; Bono, G.; Johnson, C.I.; Shen, J.; et al. Before the Bar: Kinematic Detection of a Spheroidal Metal-poor Bulge Component. Astrophys. J. Lett. 2016, 821, 25–31. [Google Scholar] [CrossRef]
- Du, H.; Mao, S.; Athanassoula, E.; Shen, J.; Pietrukowicz, P. Kinematics of RR Lyrae stars in the Galactic bulge with OGLE-IV and Gaia DR2. Mon. Not. R. Astron. Soc. 2020, 498, 5629–5642. [Google Scholar] [CrossRef]
- Kunder, A.; Rich, R.M.; Hawkins, K.; Poleski, R.; Storm, J.; Johnson, C.I.; Shen, J.; Li, Z.-Y.; Cordero, M.J.; Nataf, D.M.; et al. A High-velocity Bulge RR Lyrae Variable on a Halo-like Orbit. Astrophys. J. Lett. 2015, 808, 12–18. [Google Scholar] [CrossRef]
- Miyamoto, M.; Nagai, R. Three-dimensional models for the distribution of mass in galaxies. Publ. Astron. Soc. Jpn. 1975, 27, 533–543. [Google Scholar]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. A Universal Density Profile from Hierarchical Clustering. Astrophys. J. 1997, 490, 493–508. [Google Scholar] [CrossRef]
- Wegg, C.; Gerhard, O.; Portail, M. The structure of the Milky Way’s bar outside the bulge. Mon. Not. R. Astron. Soc. 2015, 450, 4050–4069. [Google Scholar] [CrossRef]
- Tumlinson, J. Chemical Evolution in Hierarchical Models of Cosmic Structure. II. The Formation of the Milky Way Stellar Halo and the Distribution of the Oldest Stars. Astrophys. J. 2010, 708, 1398–1418. [Google Scholar] [CrossRef]
- Martinez-Valpuesta, I.; Gerhard, O. Unifying A Boxy Bulge and Planar Long Bar in the Milky Way. Astrophys. J. 2011, 734, 20. [Google Scholar] [CrossRef]
- Buck, T.; Ness, M.K.; Macció, A.V.; Obreja, A.; Dutton, A.A. Stars Behind Bars. I. The Milky Way’s Central Stellar Populations. Astrophys. J. 2018, 861, 88. [Google Scholar] [CrossRef]
- Saha, K.; Gerhard, O.; Martinez-Valpuesta, I. Spin-up of massive classical bulges during secular evolution. Astron. Astrophys. 2016, 588, 42. [Google Scholar] [CrossRef]
- Saha, K.; Martinez-Valpuesta, I.; Gerhard, O. Spin-up of low-mass classical bulges in barred galaxies. Astron. Astrophys. 2012, 421, 333. [Google Scholar] [CrossRef]
- Prudil, Z.; Dékány, I.; Grebel, E.K.; Kunder, A. On the Oosterhoff dichotomy in the Galactic bulge—II. Kinematical distribution. Mon. Not. R. Astron. Soc. 2020, 492, 3408. [Google Scholar] [CrossRef]
- Schiavon, R.P.; Zamora, O.; Carrera, R.; Lucatello, S.; Robin, A.C.; Ness, M.; Martell, S.L.; Smith, V.V.; García-Hernández, D.A.; Manchado, A.; et al. Chemical tagging with APOGEE: Discovery of a large population of N-rich stars in the inner Galaxy. Mon. Not. R. Astron. Soc. 2017, 465, 501–524. [Google Scholar] [CrossRef]
- Fernández-Trincado, J.G.; Zamora, O.; García-Hernández, D.A.; Souto, D.; Dell’Agli, F.; Schiavon, R.P.; Geisler, D.; Tang, B.; Villanova, S.; Hasselquist, S.; et al. Atypical Mg-poor Milky Way Field Stars with Globular Cluster Second-generation-like Chemical Patterns. Astrophys. J. 2017, 846, 2. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kunder, A.M. RR Lyrae Variables as Tracers of the Galactic Bulge Kinematic Structure. Universe 2022, 8, 206. https://doi.org/10.3390/universe8040206
Kunder AM. RR Lyrae Variables as Tracers of the Galactic Bulge Kinematic Structure. Universe. 2022; 8(4):206. https://doi.org/10.3390/universe8040206
Chicago/Turabian StyleKunder, Andrea M. 2022. "RR Lyrae Variables as Tracers of the Galactic Bulge Kinematic Structure" Universe 8, no. 4: 206. https://doi.org/10.3390/universe8040206
APA StyleKunder, A. M. (2022). RR Lyrae Variables as Tracers of the Galactic Bulge Kinematic Structure. Universe, 8(4), 206. https://doi.org/10.3390/universe8040206





