Primordial Black Holes and a Common Origin of Baryons and Dark Matter
Abstract
:1. Introduction
2. The Quark-Hadron Transition
3. Electroweak Baryogenesis at the QCD Epoch
4. The Origin of the Large Curvature Fluctuations
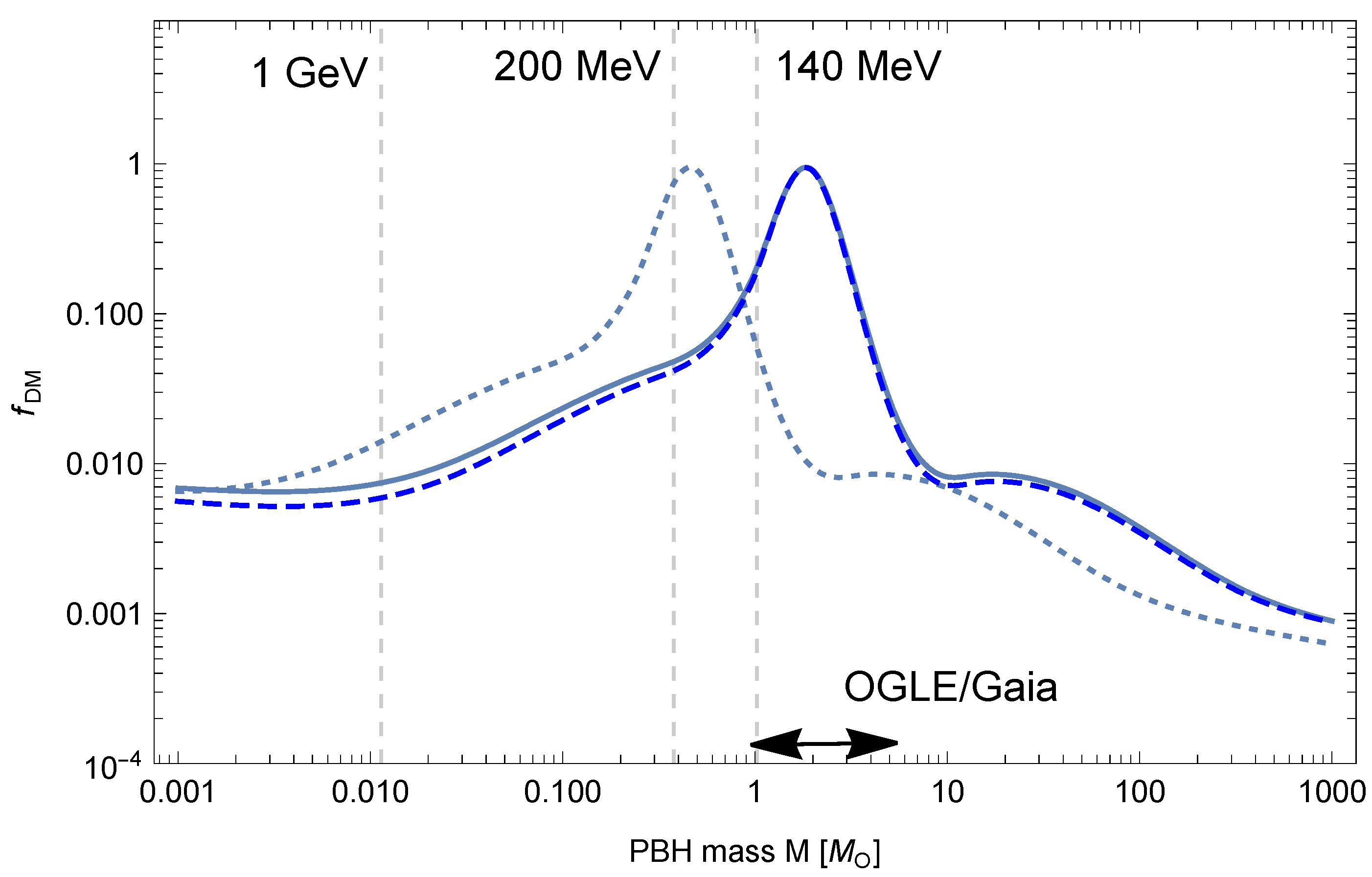
5. The PBH Mass Distribution
6. Addressing the Fine-Tunings
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Properties of the Binary Black Hole Merger GW150914. Phys. Rev. Lett. 2016, 116, 241102. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. Population Properties of Compact Objects from the Second LIGO-Virgo Gravitational-Wave Transient Catalog. Astrophys. J. Lett. 2021, 913, L7. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, N.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agarwal, D.; et al. GWTC-3: Compact Binary Coalescences Observed by LIGO and Virgo During the Second Part of the Third Observing Run. arXiv 2021, arXiv:2111.03606. [Google Scholar]
- Bird, S.; Cholis, I.; Muñoz, J.B.; Ali-Haïmoud, Y.; Kamionkowski, M.; Kovetz, E.D.; Raccanelli, A.; Riess, A.G. Did LIGO Detect Dark Matter? Phys. Rev. Lett. 2016, 116, 201301. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. The clustering of massive Primordial Black Holes as Dark Matter: Measuring their mass distribution with Advanced LIGO. Phys. Dark Univ. 2016, 10, 2. [Google Scholar] [CrossRef] [Green Version]
- Sasaki, M.; Suyama, T.; Tanaka, T.; Yokoyama, S. Primordial Black Hole Scenario for the Gravitational-Wave Event GW150914. Phys. Rev. Lett. 2016, 117, 061101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Carr, B.J.; Kühnel, F.; Sandstat, M. Constraints on primordial black holes from the Galactic gamma-ray background. Phys. Rev. D 2016, 94, 044029. [Google Scholar] [CrossRef] [Green Version]
- García-Bellido, J. Massive Primordial Black Holes as Dark Matter and their detection with Gravitational Waves. J. Phys. Conf. Ser. 2017, 840, 012032. [Google Scholar] [CrossRef]
- Carr, B.J. The primordial black hole mass spectrum. Astrophys. J. 1975, 201, 1. [Google Scholar] [CrossRef]
- Jedamzik, K. Primordial black hole formation during the QCD epoch. Phys. Rev. D 1997, 55, 5871. [Google Scholar] [CrossRef] [Green Version]
- Byrnes, C.T.; Hindmarsh, M.; Young, S.; Hawkins, M.R.S. Primordial black holes with an accurate QCD equation of state. JCAP 2018, 1808, 041. [Google Scholar] [CrossRef] [Green Version]
- Carr, B.; Clesse, S.; García-Bellido, J.; Kühnel, F. Cosmic Conundra Explained by Thermal History and Primordial Black Holes. Phys. Dark Univ. 2021, 31, 100755. [Google Scholar] [CrossRef]
- Asaka, T.; Grigoriev, D.; Kuzmin, V.; Shaposhnikov, M. Late Reheating, Hadronic Jets, and Baryogenesis. Phys. Rev. Lett. 2004, 92, 101303. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Carr, B.; Clesse, S.; García-Bellido, J. Primordial black holes from the QCD epoch: Linking dark matter, baryogenesis, and anthropic selection. Mon. Not. Roy. Astron. Soc. 2021, 501, 1426. [Google Scholar] [CrossRef]
- García-Bellido, J.; Ruiz Morales, E. Primordial black holes from single field models of inflation. Phys. Dark Univ. 2018, 18, 47. [Google Scholar] [CrossRef] [Green Version]
- Ezquiaga, J.M.; García-Bellido, J.; Ruiz Morales, E. Primordial Black Hole production in Critical Higgs Inflation. Phys. Lett. B 2018, 776, 345. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. Massive primordial black holes from hybrid inflation as dark matter and the seeds of galaxies. Phys. Rev. D 2015, 92, 023524. [Google Scholar] [CrossRef] [Green Version]
- Ezquiaga, J.M.; García-Bellido, J. Quantum diffusion beyond slow-roll: Implications for primordial black-hole production. JCAP 2018, 1808, 018. [Google Scholar] [CrossRef] [Green Version]
- Ezquiaga, J.M.; García-Bellido, J.; Vennin, V. The exponential tail of inflationary fluctuations: Consequences for primordial black holes. JCAP 2020, 2003, 029. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharya, T.; Buchoff, M.I.; Christ, N.H.; Ding, H.-T.; Gupta, R.; Jung, C.; Karsch, F.; Lin, Z.; Mawhinney, R.; McGlynn, G.; et al. QCD Phase Transition with Chiral Quarks and Physical Quark Masses. Phys. Rev. Lett. 2014, 113, 082001. [Google Scholar] [CrossRef] [PubMed]
- Musco, I.; Miller, J.C. Primordial black hole formation in the early universe: Critical behaviour and self-similarity. Class. Quant. Grav. 2013, 30, 145009. [Google Scholar] [CrossRef] [Green Version]
- Shaposhnikov, M. Baryogenesis. NATO Sci. Ser. C 2000, 555, 397. [Google Scholar]
- Sakharov, A.D. Violation of CP Invariance, C asymmetry, and baryon asymmetry of the universe. JETP Sov. Phys. Lett. 1967, 5, 24. [Google Scholar]
- Yao, W.M.; Amsler, C.D.; Asner, D.M.; Bamett, R.M.; Beringer, J.; Burchat, P.R.; Carone, C.D.; Caso, C.; Dahl, O.I.; D’Ambrosio, G.; et al. Review of Particle Physics. Phys. Rev. D 2018, 98, 030001. [Google Scholar]
- Kurkela, A.; Moore, G.D. Thermalization and non-equilibrium evolution in heavy-ion collisions. JHEP 2011, 1112, 044. [Google Scholar] [CrossRef] [Green Version]
- Reno, M.H.; Seckel, D. Primordial nucleosynthesis: The effects of injecting hadrons. Phys. Rev. D 1988, 37, 3441. [Google Scholar] [CrossRef]
- Wyrzykowski, L.; Mandel, I. Constraining the masses of microlensing black holes and the mass gap with Gaia DR2. Astron. Astrophys. 2020, 636, A20. [Google Scholar] [CrossRef] [Green Version]
- Hardwick, R.J.; Vennin, V.; Byrnes, C.T.; Torrado, J.; Wands, D. The stochastic spectator. JCAP 2017, 1710, 018. [Google Scholar] [CrossRef] [Green Version]
- Calcino, J.; García-Bellido, J.; Davis, T.M. Updating the MACHO fraction of the Milky Way dark halowith improved mass models. Mon. Not. Roy. Astron. Soc. 2018, 479, 2889. [Google Scholar] [CrossRef] [Green Version]
- García-Bellido, J.; Clesse, S. Constraints from microlensing experiments on clustered primordial black holes. Phys. Dark Univ. 2018, 19, 144. [Google Scholar] [CrossRef] [Green Version]
- Green, A.M. Astrophysical uncertainties on stellar microlensing constraints on multisolar mass primordial black hole dark matter. Phys. Rev. D 2017, 96, 043020. [Google Scholar] [CrossRef] [Green Version]
- Hawkins, M.R.S. The case for primordial black holes as dark matter. Mon. Not. Roy. Astron. Soc. 2011, 415, 2744. [Google Scholar] [CrossRef] [Green Version]
- Hawkins, M.R.S. A new look at microlensing limits on dark matter in the Galactic halo. Astron. Astrophys. 2015, 575, A107. [Google Scholar] [CrossRef] [Green Version]
- Belczynski, K.; Holz, D.E.; Bulik, T.; O’Shaughnessy, R. The first gravitational-wave source from the isolated evolution of two 40–100 Msun stars. Nature 2016, 534, 512. [Google Scholar] [CrossRef] [Green Version]
- Kashlinsky, A.; Ali-Haïmoud, Y.; Clesse, S.; Garcia-Bellido, J.; Wyrzykowski, L.; Achucarro, A.; Amendola, L.; Annis, J.; Arbey, A.; Arendt, R.G.; et al. Electromagnetic probes of primordial black holes as dark matter. Bull. Am. Astron. Soc. 2019, 51, 003. [Google Scholar]
- Ellis, J.; Sakurai, K.; Spannowsky, M. Search for Sphalerons: IceCube vs. LHC. JHEP 2016, 1605, 085. [Google Scholar] [CrossRef] [Green Version]
- Carr, B.J.; Turner, M.S. Does Explaining S Need More than Guts. Comm. Astrophys. 1981, 9, 63. [Google Scholar]
- Lacki, B.C.; Beacom, J.F. Primordial black holes as dark matter: Almost all or almost nothing. Astrophys. J. 2010, 720, L67. [Google Scholar] [CrossRef] [Green Version]
- Carr, B.J.; Kuhnel, F.; Visinelli, L. Black holes and WIMPs: All or nothing or something else. Mon. Not. Roy. Astron. Soc. 2021, 506, 3648. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
García-Bellido, J.; Carr, B.; Clesse, S. Primordial Black Holes and a Common Origin of Baryons and Dark Matter. Universe 2022, 8, 12. https://doi.org/10.3390/universe8010012
García-Bellido J, Carr B, Clesse S. Primordial Black Holes and a Common Origin of Baryons and Dark Matter. Universe. 2022; 8(1):12. https://doi.org/10.3390/universe8010012
Chicago/Turabian StyleGarcía-Bellido, Juan, Bernard Carr, and Sébastien Clesse. 2022. "Primordial Black Holes and a Common Origin of Baryons and Dark Matter" Universe 8, no. 1: 12. https://doi.org/10.3390/universe8010012
APA StyleGarcía-Bellido, J., Carr, B., & Clesse, S. (2022). Primordial Black Holes and a Common Origin of Baryons and Dark Matter. Universe, 8(1), 12. https://doi.org/10.3390/universe8010012






