The Evolution of Research on Abundances of Solar Energetic Particles
Abstract
1. Introduction
2. The First SEPs
3. Electrons
4. 3He-Rich Events
5. The Solar-Flare Myth
6. Jets
7. Gradual SEP Events
8. Conclusions and Questions
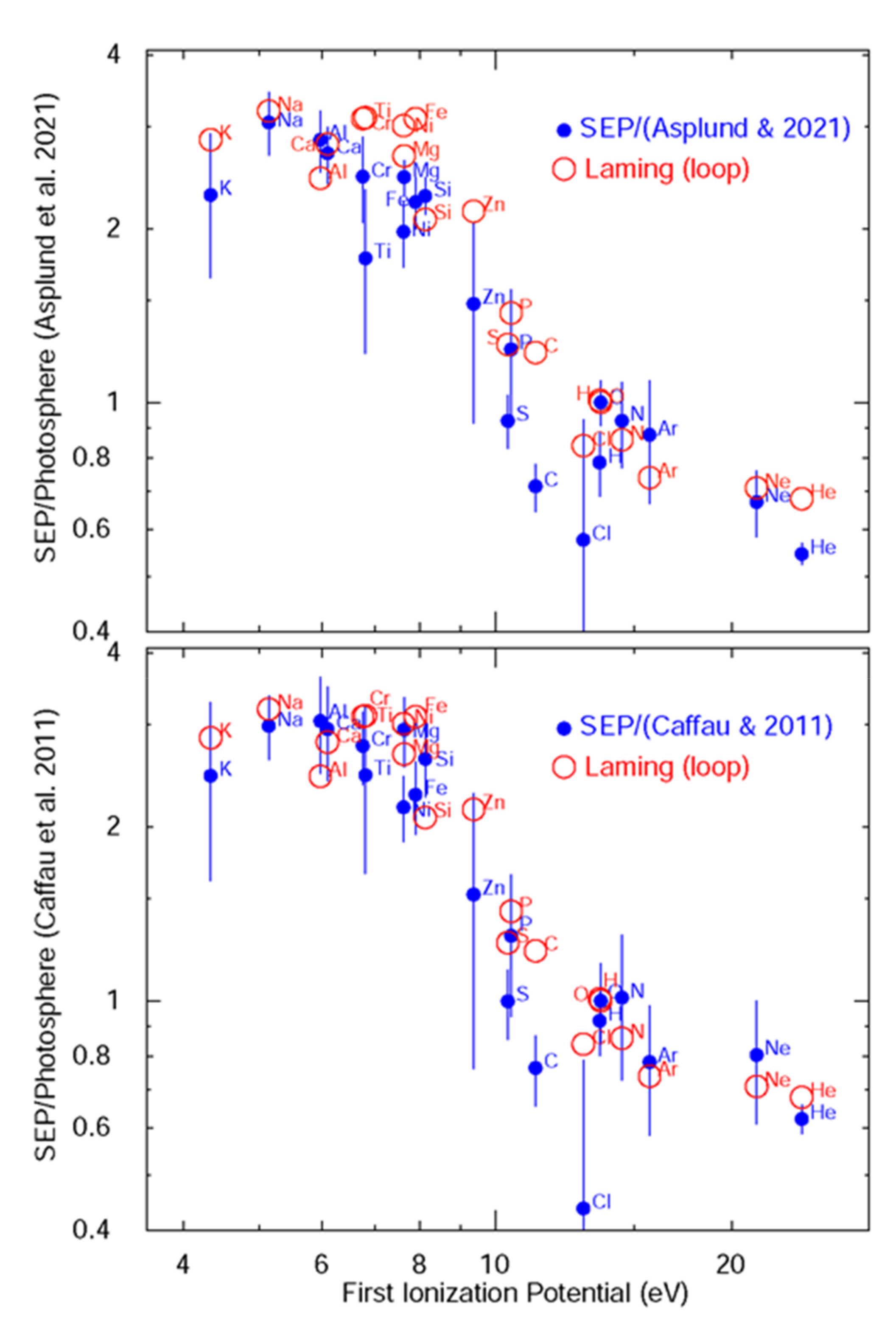
- Some variations in the abundance of He are not understood. SEP3 events have an average source He/O ≈ 91 (e.g., Figure 6), while SEP4 events tend to have He/O ≈ 55 [130]. This is usually attributed to the uniquely high FIP of He (24.6 eV), which can make He slow to ionize and enter the corona. Perhaps, but where is this low-He plasma located that is sampled for SEP4 events?
- About ~6% of impulsive SEP events are He-poor, where 4He/O is suppressed a factor of ~10 from the power-law fit line, even when H lies on that fit line [131]. Why?
- The overall C abundance, i.e., C/O = 0.420 ± 0.010, in SEPs is uniquely far below the theoretical value, as shown in Figure 1, and far below the latest photospheric value of C/O = 0.589 ± 0.054 [14]. Event-to-event variations are small. The photospheric value was C/O = 0.479 ± 0.055 in 1996 [132], but has been rising ever since, while the SEPs have changed little. What is wrong with carbon? Is C/O suppressed in SEPs (see Figure 1) or is the photospheric C/O too high? The FIP of C is not extreme, as with He.
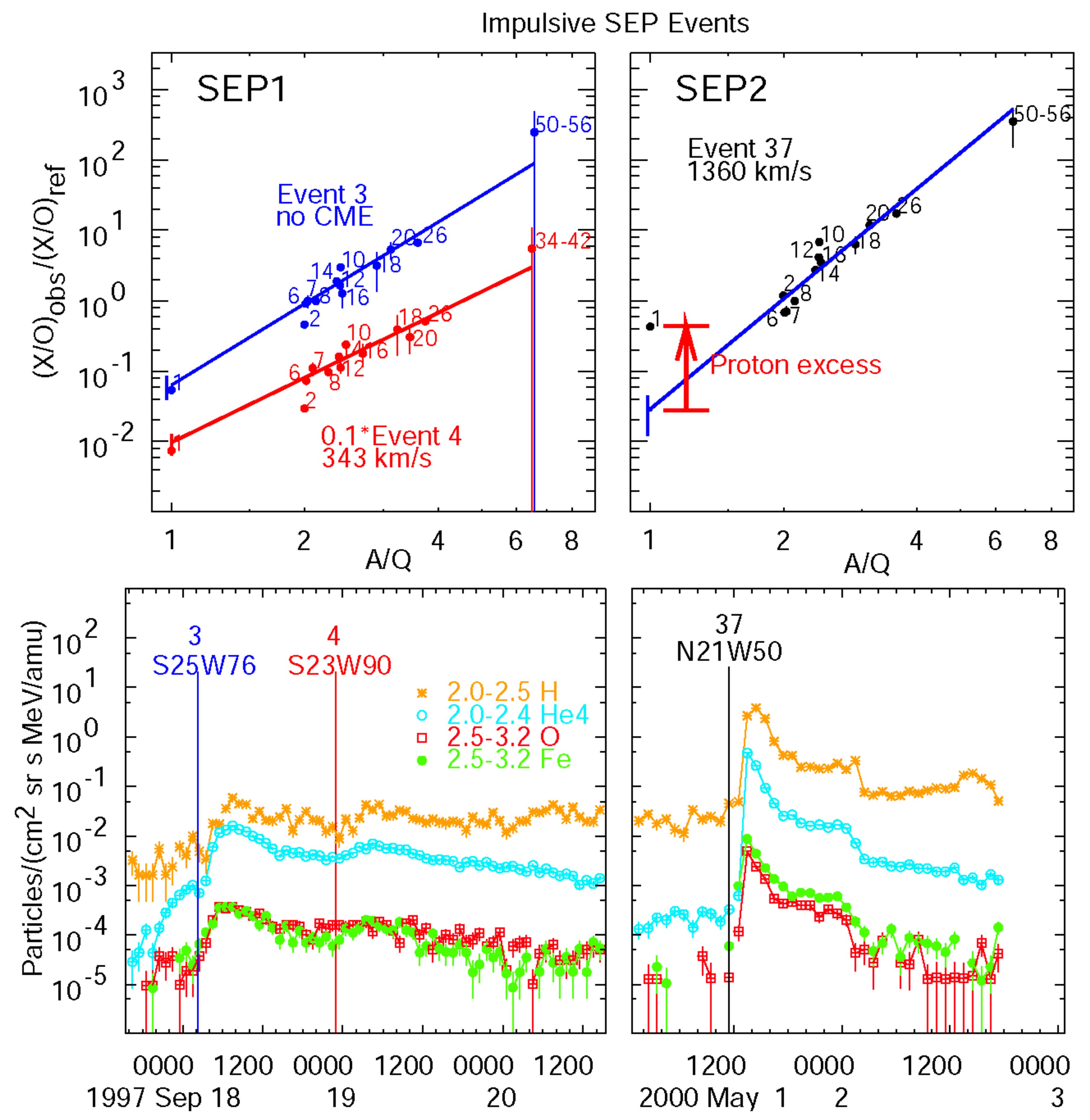
- Impulsive SEPs show enhancements of 3He and of heavy elements, each large and each with its own mechanism. How and where do these mechanisms fit together in a jet, and how do they merge with jet models that do not yet consider SEP acceleration at all?
- What is the origin of the relationship between power law element abundances and energy spectral indices in SEP4 shock events? Present SEP models treat source abundances as adjustable parameters, unrelated to the SEP reference abundances.
Funding
Conflicts of Interest
References
- Forbush, S.E. Three unusual cosmic ray increases possibly due to charged particles from the Sun. Phys. Rev. 1946, 70, 771. [Google Scholar] [CrossRef]
- Kahler, S.W.; Sheeley, N.R., Jr.; Howard, R.A.; Koomen, M.J.; Michels, D.J.; McGuire, R.E.; von Rosenvinge, T.T.; Reames, D.V. Associations between coronal mass ejections and solar energetic proton events. J. Geophys. Res. 1984, 89, 9683. [Google Scholar] [CrossRef]
- Fichtel, C.E.; Guss, D.E. Heavy nuclei in solar cosmic rays. Phys. Rev. Lett. 1961, 6, 495. [Google Scholar] [CrossRef]
- Meyer, J.P. The baseline composition of solar energetic particles. Astrophys. J. 1985, 57, 151. [Google Scholar] [CrossRef]
- Breneman, H.H.; Stone, E.C. Solar coronal and photospheric abundances from solar energetic particle measurements. Astrophys. J. Lett. 1985, 299, L57. [Google Scholar] [CrossRef]
- Reames, D.V. Coronal Abundances determined from energetic particles. Adv. Space Res. 1995, 15, 41. [Google Scholar] [CrossRef]
- Reames, D.V. Element abundances in solar energetic particles and the solar corona. Solar Phys. 2014, 289, 977. [Google Scholar] [CrossRef]
- Reames, D.V. Solar Energetic Particles, 2nd ed.; Open access; Springer Nature: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Laming, J.M. The FIP and inverse FIP effects in solar and stellar coronae. Living Rev. Sol. Phys. 2015, 12, 2. [Google Scholar] [CrossRef]
- Mewaldt, R.A.; Cohen, C.M.S.; Leske, R.A.; Christian, E.R.; Cummings, A.C.; Stone, E.C.; von Rosenvinge, T.T.; Wiedenbeck, M.E. Fractionation of solar energetic particles and solar wind according to first ionization potential. Advan. Space Res. 2002, 30, 79. [Google Scholar] [CrossRef]
- Reames, D.V. The "FIP effect" and the origins of solar energetic particles and of the solar wind. Solar Phys. 2018, 293, 47. [Google Scholar] [CrossRef]
- Laming, J.M.; Vourlidas, A.; Korendyke, C. Element abundances: A new diagnostic for the solar wind. Astrophys. J. 2019, 879, 124. [Google Scholar] [CrossRef]
- Caffau, E.; Ludwig, H.-G.; Steffen, M.; Freytag, B.; Bonofacio, P. Solar chemical abundances determined with a CO5BOLD 3D model atmosphere. Solar Phys. 2011, 268, 255. [Google Scholar] [CrossRef]
- Asplund, M.; Amarsi, A.M.; Grevesse, N. The chemical make-up of the Sun: A 2020 vision, Astron. arXiv 2021, arXiv:2105.01661. (in press)
- Wild, J.P.; Smerd, S.F.; Weiss, A.A. Solar Bursts. Annu. Rev. Astron. Astrophys. 1963, 1, 291. [Google Scholar] [CrossRef]
- Lin, R.P. The emission and propagation of 40 keV solar flare electrons. I: The relationship of 40 keV electron to energetic proton and relativistic electron emission by the sun. Sol. Phys. 1970, 12, 266. [Google Scholar] [CrossRef]
- Hsieh, K.C.; Simpson, J.A. Galactic 3He above 10 MeV per nucleon and the solar contributions of hydrogen and helium. Astrophys. J. Lett. 1970, 162, L197. [Google Scholar] [CrossRef]
- Serlemitsos, A.T.; Balasubrahmanyan, V.K. Solar particle events with anomalously large relative abundance of 3He. Astrophys. J. 1975, 198, 195. [Google Scholar] [CrossRef]
- McGuire, R.E.; von Rosenvinge, T.T.; McDonald, F.B. A survey of solar cosmic ray composition. Int. Cosm. Ray Conf. Tokyo 1979, 5, 61. [Google Scholar]
- Cook, W.R.; Stone, E.C.; Vogt, R.E. Elemental composition of solar energetic particles. Astrophys. J. 1984, 279, 827. [Google Scholar] [CrossRef]
- Mason, G.M.; Reames, D.V.; Klecker, B.; Hovestadt, D.; von Rosenvinge, T.T. The heavy-ion compositional signature in He-3-rich solar particle events. Astrophys. J. 1986, 303, 849. [Google Scholar] [CrossRef]
- Reames, D.V.; Meyer, J.P.; von Rosenvinge, T.T. Energetic-particle abundances in impulsive solar flare events. Astrophys. J. Suppl. 1994, 90, 649. [Google Scholar] [CrossRef]
- Reames, D.V. Abundances of trans-iron elements in solar energetic particle events. Astrophys. J. Lett. 2000, 540, L111. [Google Scholar] [CrossRef]
- Reames, D.V.; Ng, C.K. Heavy-element abundances in solar energetic particle events. Astrophys. J. 2004, 610, 510. [Google Scholar] [CrossRef]
- Mason, G.M.; Mazur, J.E.; Dwyer, J.R.; Jokippi, J.R.; Gold, R.E.; Krimigis, S.M. Abundances of heavy and ultraheavy ions in 3He-rich solar flares. Astrophys. J. 2004, 606, 555. [Google Scholar] [CrossRef]
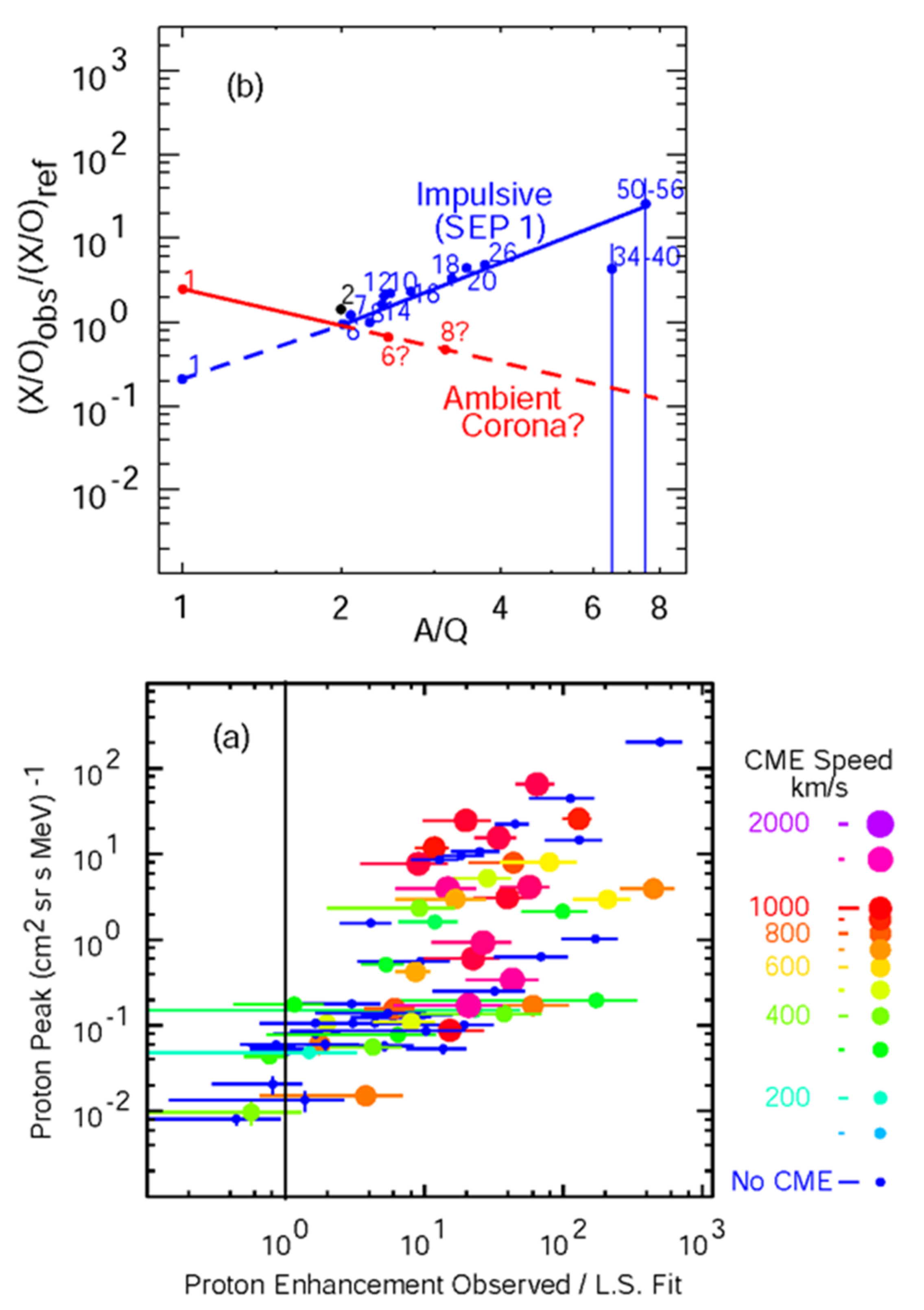
- Reames, D.V.; Cliver, E.W.; Kahler, S.W. Abundance enhancements in impulsive solar energetic-particle events with associated coronal mass ejections. Solar Phys. 2014, 289, 3817. [Google Scholar] [CrossRef]
- Reames, D.V. Particle acceleration at the Sun and in the heliosphere. Space Sci. Rev. 1999, 90, 413. [Google Scholar] [CrossRef]
- Ho, G.C.; Roelof, E.C.; Mason, G.M. The upper limit on 3He fluence in solar energetic particle events. Atrophys. J. Lett. 2005, 621, L141. [Google Scholar] [CrossRef]
- Mason, G.M. 3He-rich solar energetic particle events. Space Sci. Rev. 2007, 130, 231. [Google Scholar] [CrossRef]
- Reames, D.V.; von Rosenvinge, T.T.; Lin, R.P. Solar 3He-rich events and nonrelativistic electron events—A new association. Astrophys. J. 1985, 292, 716. [Google Scholar] [CrossRef]
- Reames, D.V.; Stone, R.G. The identification of solar 3He-rich events and the study of particle acceleration at the sun. Astrophys. J. 1986, 308, 902. [Google Scholar] [CrossRef]
- Ibragimov, I.A.; Kocharov, G.E. Possible mechanism for enrichment of solar cosmic rays by helium-three and heavy nuclei. Int. Conf. Cosm. Rays 1977, 11, 340. [Google Scholar]
- Kocharov, G.E.; Kocharov, L.G. Present state of experimental and theoretical investigations of solar events enriched by helium-3. Cosmophysics 1978, 37, 37–72. [Google Scholar]
- Kocharov, G.E.; Kocharov, L.G. 3He-rich solar flares. Space Science Rev. 1984, 38, 89. [Google Scholar] [CrossRef]
- Weatherall, J. Turbulent heating in solar cosmic-ray theory. Astrophys. J. 1984, 281, 468. [Google Scholar] [CrossRef]
- Fisk, L.A. 3He-rich flares—A possible explanation. Astrophys. J. 1978, 224, 1048. [Google Scholar] [CrossRef]
- Varvoglis, H.; Papadopoulis, K. Selective nonresonant acceleration of He-3(2+) and heavy ions by H(+) cyclotron waves. Astrophys. J. Lett. 1983, 270, L95. [Google Scholar] [CrossRef]
- Winglee, R.M. Heating and acceleration of heavy ions during solar flares. Astrophys. J. 1989, 343, 511. [Google Scholar] [CrossRef]
- Riyopoulos, S. Subthreshold stochastic diffusion with application to selective acceleration of 3He in solar flares. Astrophys. J. 1991, 381, 578. [Google Scholar] [CrossRef]
- Temerin, M.; Roth, I. The production of 3He and heavy ion enrichment in 3He-rich flares by electromagnetic hydrogen cyclotron waves. Astrophys. J. Lett. 1992, 391, L105. [Google Scholar] [CrossRef]
- Drake, J.F.; Cassak, P.A.; Shay, M.A.; Swisdak, M.; Quataert, E. A magnetic reconnection mechanism for ion acceleration and abundance enhancements in impulsive flares. Astrophys. J. Lett. 2009, 700, L16. [Google Scholar] [CrossRef]
- Arnold, H.; Drake, J.; Swisdak, M.; Guo, F.; Dahlin, J.; Chen, B.; Fleishman, G.; Glesener, L.; Kontar, E.; Phan, T.; et al. Electron acceleration during macroscale magnetic reconnection. arXiv 2011, arXiv:2011.01147. [Google Scholar]
- Luhn, A.; Klecker, B.; Hovestadt, D.; Gloeckler, G.; Ipavich, F.M.; Scholer, M.; Fan, C.Y.; Fisk, L.A. Ionic charge states of N, Ne, Mg, Si and S in solar energetic particle events. Adv. Space Res. 1984, 4, 161. [Google Scholar] [CrossRef]
- Luhn, A.; Klecker, B.; Hovestadt, D.; Möbius, E. The mean ionic charge of silicon in He-3-rich solar flares. Astrophys. J. 1987, 317, 951. [Google Scholar] [CrossRef]
- DiFabio, R.; Guo, Z.; Möbius, E.; Klecker, B.; Kucharek, H.; Mason, G.M.; Popecki, M. Energy-dependent charge states and their connection with ion abundances in impulsive solar energetic particle events. Astrophys. J. 2008, 687, 623. [Google Scholar] [CrossRef]
- Newkirk, G., Jr.; Wenzel, D.G. Rigidity-independent propagation of cosmic rays in the solar corona. J. Geophys. Res. 1978, 83, 2009. [Google Scholar] [CrossRef]
- Mason, G.M.; Gloeckler, G.; Hovestadt, D. Temporal variations of nucleonic abundances in solar flare energetic particle events. II—Evidence for large-scale shock acceleration. Astrophys. J. 1984, 280, 902. [Google Scholar] [CrossRef]
- Reames, D.V. Bimodal abundances in the energetic particles of solar and interplanetary origin. Astrophys. J. Lett. 1988, 330, L71. [Google Scholar] [CrossRef]
- Reames, D.V. Magnetic topology of impulsive and gradual solar energetic particle events. Astrophys. J. Lett. 2002, 571, L63. [Google Scholar] [CrossRef]
- Gosling, J.T. The solar flare myth. J. Geophys. Res. 1993, 98, 18937. [Google Scholar] [CrossRef]
- Gosling, J.T. Corrections to “The solar flare myth”. J. Geophys. Res. 1994, 99, 4259. [Google Scholar] [CrossRef]
- Zirin, H. Solar storminess, Sky and Telescope. 9 November 1994. [Google Scholar]
- Hudson, H.S. Solar flares: No “myth”. Eos Trans. 1995, AGU 76, 405. [Google Scholar] [CrossRef]
- Miller, J.A. Much ado about nothing. Eos Trans. 1995, AGU 76, 401. [Google Scholar] [CrossRef]
- Reames, D.V. The dark side of the solar flare myth. Eos Trans. 1995, AGU 76, 401. [Google Scholar] [CrossRef]
- Reames, D.V. Solar energetic particles: A paradigm shift. Revs. Geophys. Suppl. 1995, 33, 585. [Google Scholar] [CrossRef]
- Mason, G.M.; Mazur, J.E.; Dwyer, J.R. 3He enhancements in large solar energetic particle events. Astrophys. J. Lett. 1999, 525, L133. [Google Scholar] [CrossRef] [PubMed]
- Tylka, A.J.; Cohen, C.M.S.; Dietrich, W.F.; Maclennan, C.G.; McGuire, R.E.; Ng, C.K.; Reames, D.V. Evidence for remnant flare suprathermals in the source population of solar energetic particles in the 2000 bastille day event. Astrophys. J. Lett. 2001, 558, L59. [Google Scholar] [CrossRef][Green Version]
- Tylka, A.J.; Cohen, C.M.S.; Dietrich, W.F.; Lee, M.A.; Maclennan, C.G.; Mewaldt, R.A.; Ng, C.K.; Reames, D.V. Shock geometry, seed populations, and the origin of variable elemental composition at high energies in large gradual solar particle events. Astrophys. J. 2005, 625, 474. [Google Scholar] [CrossRef]
- Tylka, A.J.; Lee, M.A. Spectral and compositional characteristics of gradual and impulsive solar energetic particle events. Astrophys. J. 2006, 646, 1319. [Google Scholar] [CrossRef]
- Richardson, I.G.; Reames, D.V.; Wenzel, K.P.; Rodriguez-Pacheco, J. Quiet-time properties of <10 MeV/n interplanetary ions during solar maximum and minimum. Astrophys. J. Lett. 1990, 363, L9. [Google Scholar] [CrossRef]
- Desai, M.I.; Mason, G.M.; Dwyer, J.R.; Mazur, J.E.; Gold, R.E.; Krimigis, S.M.; Smith, C.W.; Skoug, R.M. Evidence for a suprathermal seed population of heavy ions accelerated by interplanetary shocks near 1 AU. Astrophys. J. 2003, 588, 1149. [Google Scholar] [CrossRef]
- Wiedenbeck, M.E.; Cohen, C.M.S.; Cummings, A.C.; de Nolfo, G.A.; Leske, R.A.; Mewaldt, R.A.; Stone, E.C.; von Rosenvinge, T.T. Persistent energetic 3He in the inner heliosphere. Int. Cosm. Ray Conf. 2008, 1, 91. [Google Scholar]
- Bučík, R.; Innes, D.E.; Mall, U.; Korth, A.; Mason, G.M.; Gómez-Herrero, R. Multi-spacecraft observations of recurrent 3He-rich solar energetic particles. Astrophys. J. 2014, 786, 71. [Google Scholar] [CrossRef]
- Bučík, R.; Innes, D.E.; Chen, N.H.; Mason, G.M.; Gómez-Herrero, R.; Wiedenbeck, M.E. Long-lived energetic particle source regions on the Sun. J. Phys. Conf. Ser. 2015, 642, 012002. [Google Scholar] [CrossRef]
- Chen, N.H.; Bučík, R.; Innes, D.E.; Mason, G.M. Case studies of multi-day 3He-rich solar energetic particle periods. Astron. Astrophys. 2015, 580, 16. [Google Scholar] [CrossRef][Green Version]
- Kahler, S.W.; Reames, D.V.; Sheeley, N.R., Jr. Coronal mass ejections associated with impulsive solar energetic particle events. Astrophys. J. 2001, 562, 558. [Google Scholar] [CrossRef]
- Shimojo, M.; Shibata, K. Physical parameters of solar X-ray jets. Astrophys. J. 2000, 542, 1100. [Google Scholar] [CrossRef]
- Ramaty, R.; Murphy, R.J. Nuclear processes and accelerated particles in solar flares. Space Sci. Rev. 1987, 45, 213. [Google Scholar] [CrossRef]
- Mandzhavidze, N.; Ramaty, R.; Kozlovsky, B. Determination of the abundances of subcoronal 4He and of solar flare-accelerated 3He and 4He from gamma-ray spectroscopy. Astrophys. J. 1999, 518, 918. [Google Scholar] [CrossRef]
- Murphy, R.J.; Kozlovsky, B.; Share, G.H. Evidence for enhanced 3He in flare-accelerated particles based on new calculations of the gamma-ray line spectrum. Astrophys. J. 2016, 833, 166. [Google Scholar] [CrossRef]
- Murphy, R.J.; Ramaty, R.; Kozlovsky, B.; Reames, D.V. Solar abundances from gamma-ray spectroscopy: Comparisons with energetic particle, photospheric, and coronal abundances. Astrophys. J. 1991, 371, 793. [Google Scholar] [CrossRef]
- Nitta, N.V.; Reames, D.V.; DeRosa, M.L.; Yashiro, S.; Gopalswamy, N. Solar sources of impulsive solar energetic particle events and their magnetic field connection to the earth. Astrophys. J. 2006, 650, 438. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Pick, M.; Mason, G.M. Coronal holes, jets, and the origin of 3He-rich particle events. Astrophys. J. 2006, 639, 495. [Google Scholar] [CrossRef]
- Ko, Y.-K.; Tylka, A.J.; Ng, C.K.; Wang, Y.-M.; Dietrich, W.F. Source regions of the interplanetary magnetic field and variability in heavy-ion elemental composition in gradual solar energetic particle events. Astrophys. J. 2013, 776, 92. [Google Scholar] [CrossRef][Green Version]
- Bučík, R.; Innes, D.E.; Mason, G.M.; Wiedenbeck, M.E.; Gómez-Herrero, R.; Nitta, N.V. 3He-rich solar energetic particles in helical jets on the Sun. Astrophys. J. 2018, 852, 76. [Google Scholar] [CrossRef]
- Bučík, R.; Wiedenbeck, M.E.; Mason, G.M.; Gómez-Herrero, R.; Nitta, N.V.; Wang, L. 3He-rich solar energetic particles from sunspot jets. Astrophys. J. Lett. 2018, 869, L21. [Google Scholar] [CrossRef]
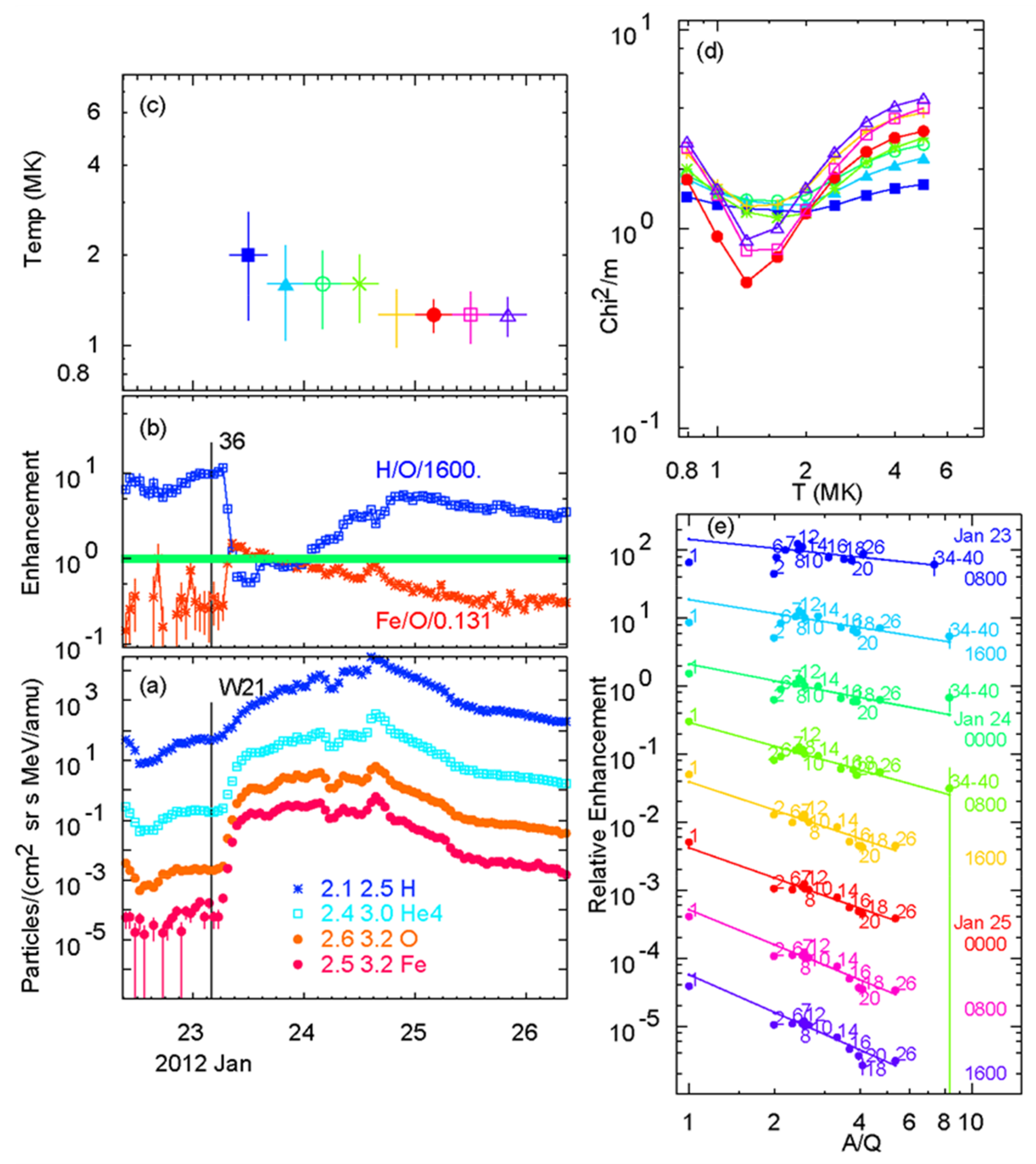
- Bučík, R.; Mulay, S.M.; Mason, G.M.; Nitta, N.V.; Desai, M.I.; Dayeh, M.A. Temperature in solar sources of 3He-rich solar energetic particles and relation to ion abundances. Astrophys. J. 2021, 908, 243. [Google Scholar] [CrossRef]
- Bučík, R. 3He-rich solar energetic particles: Solar sources. Space Sci. Rev. 2020, 216, 24. [Google Scholar] [CrossRef]
- Reames, D.V.; Cliver, E.W.; Kahler, S.W. Variations in abundance enhancements in impulsive solar energetic-particle events and related CMEs and flares. Solar Phys. 2014, 289, 4675. [Google Scholar] [CrossRef]
- Reames, D.V. Abundances, ionization states, temperatures, and FIP in solar energetic particles. Space Sci. Rev. 2018, 214, 61. [Google Scholar] [CrossRef]
- Reames, D.V. Four distinct pathways to the element abundances in solar energetic particles. Space Sci. Rev. 2020, 216, 20. [Google Scholar] [CrossRef]
- Reames, D.V. Hydrogen and the abundances of elements in impulsive solar energetic-particle events. Solar Phys. 2019, 294, 37. [Google Scholar] [CrossRef]
- Reames, D.V.; Cliver, E.W.; Kahler, S.W. Temperature of the source plasma for impulsive solar energetic particles. Sol. Phys. 2015, 290, 1761. [Google Scholar] [CrossRef]
- Lee, M.A. Coupled hydromagnetic wave excitation and ion acceleration at interplanetary traveling shocks. J. Geophys. Res. 1983, 88, 6109. [Google Scholar] [CrossRef]
- Lee, M.A. Coupled hydromagnetic wave excitation and ion acceleration at an evolving coronal/interplanetary shock. Astrophys. J. Suppl. 2005, 158, 38. [Google Scholar] [CrossRef]
- Jones, F.C.; Ellison, D.E. The plasma physics of shock acceleration. Space Sci. Rev. 1991, 58, 259. [Google Scholar] [CrossRef]
- Zank, G.P.; Rice, W.K.M.; Wu, C.C. Particle acceleration and coronal mass ejection driven shocks: A theoretical model. J. Geophys. Res. 2000, 105, 25079. [Google Scholar] [CrossRef]
- Zank, G.P.; Li, G.; Verkhoglyadova, O. Particle Acceleration at Interplanetary Shocks. Space Sci. Rev. 2007, 130, 255. [Google Scholar] [CrossRef]
- Cliver, E.W.; Kahler, S.W.; Reames, D.V. Coronal shocks and solar energetic proton events. Astrophys. J. 2004, 605, 902. [Google Scholar] [CrossRef]
- Sandroos, A.; Vainio, R. Simulation results for heavy ion spectral variability in large gradual solar energetic particle events. Astrophys. J. 2007, 662, L127. [Google Scholar] [CrossRef]
- Ng, C.K.; Reames, D.V. Shock acceleration of solar energetic protons: The first 10 minutes. Astrophys. J. Lett. 2008, 686, L123. [Google Scholar] [CrossRef]
- Reames, D.V. The two sources of solar energetic particles. Space Sci. Rev. 2013, 175, 53. [Google Scholar] [CrossRef]
- Lee, M.A.; Mewaldt, R.A.; Giacalone, J. Shock acceleration of ions in the heliosphere. Space Sci. Rev. 2012, 173, 247. [Google Scholar] [CrossRef]
- Desai, M.I.; Giacalone, J. Large gradual solar energetic particle events. Living Rev. Sol. Phys. 2016, 13, 1–32. [Google Scholar] [CrossRef]
- Reames, D.V.; Barbier, L.M.; Ng, C.K. The spatial distribution of particles accelerated by coronal mass ejection-driven shocks. Astrophys. J. 1996, 466, 473. [Google Scholar] [CrossRef]
- Reames, D.V.; Kahler, S.W.; Ng, C.K. Spatial and temporal invariance in the spectra of energetic particles in gradual solar events. Astrophys. J. 1997, 491, 414. [Google Scholar] [CrossRef]
- Rouillard, A.C.; Odstrčil, D.; Sheeley, N.R., Jr.; Tylka, A.J.; Vourlidas, A.; Mason, G.; Wu, C.-C.; Savani, N.P.; Wood, B.E.; Ng, C.K.; et al. Interpreting the properties of solar energetic particle events by using combined imaging and modeling of interplanetary shocks. Astrophys. J. 2011, 735, 7. [Google Scholar] [CrossRef]
- Rouillard, A.; Sheeley, N.R., Jr.; Tylka, A.; Vourlidas, A.; Ng, C.K.; Rakowski, C.; Cohen, C.M.S.; Mewaldt, R.A.; Mason, G.M.; Reames, D.; et al. The longitudinal properties of a solar energetic particle event investigated using modern solar imaging. Astrophys. J. 2012, 752, 44. [Google Scholar] [CrossRef]
- Rouillard, A.P.; Plotnikov, I.; Pinto, R.F.; Tirole, M.; Lavarra, M.; Zucca, P.; Vainio, R.; Tylka, A.J.; Vourlidas, A.; De Rosa, M.L.; et al. Deriving the properties of coronal pressure fronts in 3D: Application to the 2012 May 17 ground level enhancement. Astrophys. J. 2016, 833, 45. [Google Scholar] [CrossRef]
- Kahler, S.W. The correlation between solar energetic particle peak intensities and speeds of coronal mass ejections: Effects of ambient particle intensities and energy spectra. J. Geophys. Res. 2001, 106, 20947. [Google Scholar] [CrossRef]
- Kouloumvakos, A.; Rouillard, A.P.; Wu, Y.; Vainio, R.; Vourlidas, A.; Plotnikov, I.; Afanasiev, A.; Önel, H. Connecting the properties of coronal shock waves with those of solar energetic particles. Astrophys. J. 2019, 876, 80. [Google Scholar] [CrossRef]
- Kahler, S.W. Injection profiles of solar energetic particles as functions of coronal mass ejection heights. Astrophys. J. 1994, 428, 837. [Google Scholar] [CrossRef]
- Tylka, A.J.; Cohen, C.M.S.; Dietrich, W.F.; Krucker, S.; McGuire, R.E.; Mewaldt, R.A.; Ng, C.K.; Reames, D.V.; Share, G.H. Contributed papers v. 6 (SH sessions 1.1-2.3). In Proceedings of the 28th International Cosmic Ray Conference (Tsukuba) 6 3305 (2003), Tsukuba, Japan, 31 July–7 August 2003. [Google Scholar]
- Reames, D.V. Solar release times of energetic particles in ground-level events. Astrophys. J. 2009, 693, 812. [Google Scholar] [CrossRef]
- Reames, D.V. Solar energetic-particle release times in historic ground-level events. Astrophys. J. 2009, 706, 844. [Google Scholar] [CrossRef]
- Cliver, E.W.; Ling, A.G. Electrons and protons in solar energetic particle events. Astrophys. J. 2007, 658, 1349. [Google Scholar] [CrossRef]
- Cliver, E.W. Flare vs. shock acceleration of high-energy protons in solar energetic particle events. Astrophys. J. 2016, 832, 128. [Google Scholar] [CrossRef]
- Tylka, A.J.; Dietrich, W.F. A New and Comprehensive Analysis of Proton Spectra in Ground-Level Enhanced (GLE) Solar Particle Events. In Proceedings of the 31st International Cos. Ray Conference Lódz, Lódz, Poland, 8 July 2009; Available online: http://icrc2009.uni.lodz.pl/proc/pdf/icrc0273.pdf (accessed on 7 August 2021).
- Gopalswamy, N.; Xie, H.; Yashiro, S.; Akiyama, S.; Mäkelä, P.; Usoskin, I.G. Properties of Ground level enhancement events and the associated solar eruptions during solar cycle 23. Space Sci. Rev. 2012, 171, 23. [Google Scholar] [CrossRef]
- Mewaldt, R.A.; Looper, M.D.; Cohen, C.M.S.; Haggerty, D.K.; Labrador, A.W.; Leske, R.A.; Mason, G.M.; Mazur, J.E.; von Rosenvinge, T.T. Energy spectra, composition, other properties of ground-level events during solar cycle 23. Space Sci. Rev. 2012, 171, 97. [Google Scholar] [CrossRef]
- Reames, D.V. Hydrogen and the abundances of elements in gradual solar energetic-particle events. Solar Phys. 2019, 294, 69. [Google Scholar] [CrossRef]
- Reames, D.V. Temperature of the source plasma in gradual solar energetic particle events. Solar Phys. 2016, 291, 911. [Google Scholar] [CrossRef]
- Reames, D.V. Distinguishing the rigidity dependences of acceleration and transport in solar energetic particles. Solar Phys. 2020, 295, 113. [Google Scholar] [CrossRef]
- Mason, G.M.; Ng, C.K.; Klecker, B.; Green, G. Impulsive acceleration and scatter-free transport of about 1 MeV per nucleon ions in 3He-rich solar particle events. Astrophys. J. 1989, 339, 529. [Google Scholar] [CrossRef]
- Stix, T.H. Waves in Plasmas; AIP: New York, NY, USA, 1992. [Google Scholar]
- Reames, D.V. Acceleration of energetic particles by shock waves from large solar flares. Astrophys. J. Lett. 1990, 358, L63. [Google Scholar] [CrossRef]
- Reames, D.V.; Ng, C.K.; Tylka, A.J. Initial time dependence of abundances in solar particle events. Astrophys. J. Lett. 2000, 531, L83. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Ng, C.K.; Reames, D.V. Focused interplanetary transport of approximately 1 MeV solar energetic protons through self-generated Alfven waves. Astrophys. J. 1994, 424, 1032. [Google Scholar] [CrossRef]
- Ng, C.K.; Reames, D.V.; Tylka, A.J. Effect of proton-amplified waves on the evolution of solar energetic particle composition in gradual events. Geophys. Res. Lett. 1999, 26, 2145. [Google Scholar] [CrossRef]
- Ng, C.K.; Reames, D.V.; Tylka, A.J. Modeling shock-accelerated solar energetic particles coupled to interplanetary Alfvén waves. Astrophys. J. 2003, 591, 461. [Google Scholar] [CrossRef]
- Ng, C.K.; Reames, D.V.; Tylka, A.J. Solar energetic particles: Shock acceleration and transport through self-amplified waves. AIP Conf. Proc. 2012, 1436, 212. [Google Scholar] [CrossRef]
- Reames, D.V.; Ng, C.K. Streaming-limited intensities of solar energetic particles. Astrophys. J. 1998, 504, 1002. [Google Scholar] [CrossRef]
- Reames, D.V.; Ng, C.K. Streaming-limited intensities of solar energetic particles on the intensity plateau. Astrophys. J. 2010, 723, 1286. [Google Scholar] [CrossRef]
- Reames, D.V. The correlation between energy spectra and element abundances in solar energetic particles. Sol. Phys. 2021, 296, 24. [Google Scholar] [CrossRef]
- Zank, G.P.; Rice, W.K.M.; le Roux, J.A.; Cairns, I.H.; Webb, G.M. The “injection problem” for quasiparallel shocks. Phys. Plasmas 2001, 8, 4560. [Google Scholar] [CrossRef]
- Li, G.; Zank, G.P.; Rice, W.K.M. Acceleration and transport of heavy ions at coronal mass ejection-driven shocks. J. Geophys. Res. 2005, 110, A06104. [Google Scholar] [CrossRef]
- Li, G.; Zank, G.P.; Verkhoglyadova, O.; Mewaldt, R.A.; Cohen, C.M.S.; Mason, G.M.; Desai, M.I. Shock geometry and spectral breaks in large SEP events. Astrophys. J. 2009, 702, 998. [Google Scholar] [CrossRef]
- Zhao, L.; Zhang, M.; Rassoul, H. Double power laws in the event-integrated solar energetic particle spectrum. Astrophys. J. 2016, 821, 62. [Google Scholar] [CrossRef]
- Reames, D.V. The abundance of helium in the source plasma of solar energetic particles. Solar Phys. 2017, 292, 156. [Google Scholar] [CrossRef][Green Version]
- Reames, D.V. Helium suppression in impulsive solar energetic-particle events. Sol. Phys. 2019, 294, 32. [Google Scholar] [CrossRef]
- Grevesse, N.; Noels, A.; Sauval, A.J. Standard Abundances. Astron. Soc. Pacif. Conf. Ser. 1996, 99, 117. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reames, D.V. The Evolution of Research on Abundances of Solar Energetic Particles. Universe 2021, 7, 292. https://doi.org/10.3390/universe7080292
Reames DV. The Evolution of Research on Abundances of Solar Energetic Particles. Universe. 2021; 7(8):292. https://doi.org/10.3390/universe7080292
Chicago/Turabian StyleReames, Donald V. 2021. "The Evolution of Research on Abundances of Solar Energetic Particles" Universe 7, no. 8: 292. https://doi.org/10.3390/universe7080292
APA StyleReames, D. V. (2021). The Evolution of Research on Abundances of Solar Energetic Particles. Universe, 7(8), 292. https://doi.org/10.3390/universe7080292





