Mechanisms and Evolution of Geoeffective Large-Scale Plasma Jets in the Magnetosheath
Abstract
1. Introduction
- collapsing foreshock (CFS) [19]
2. Methods
2.1. Data
2.2. Identification of Geoeffective Plasma Jets in the Magnetosheath
2.3. Identification of Interplanetary Sources
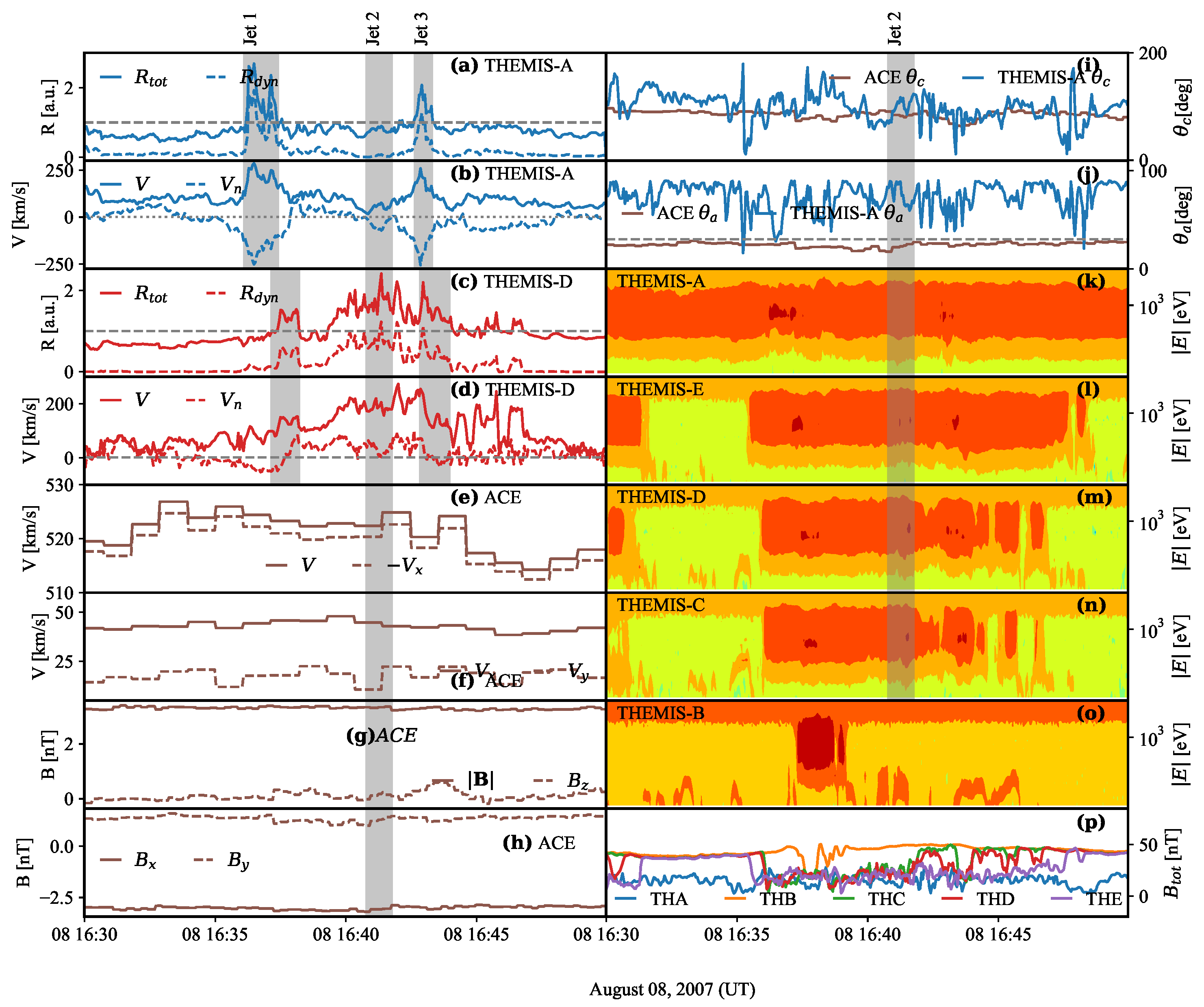
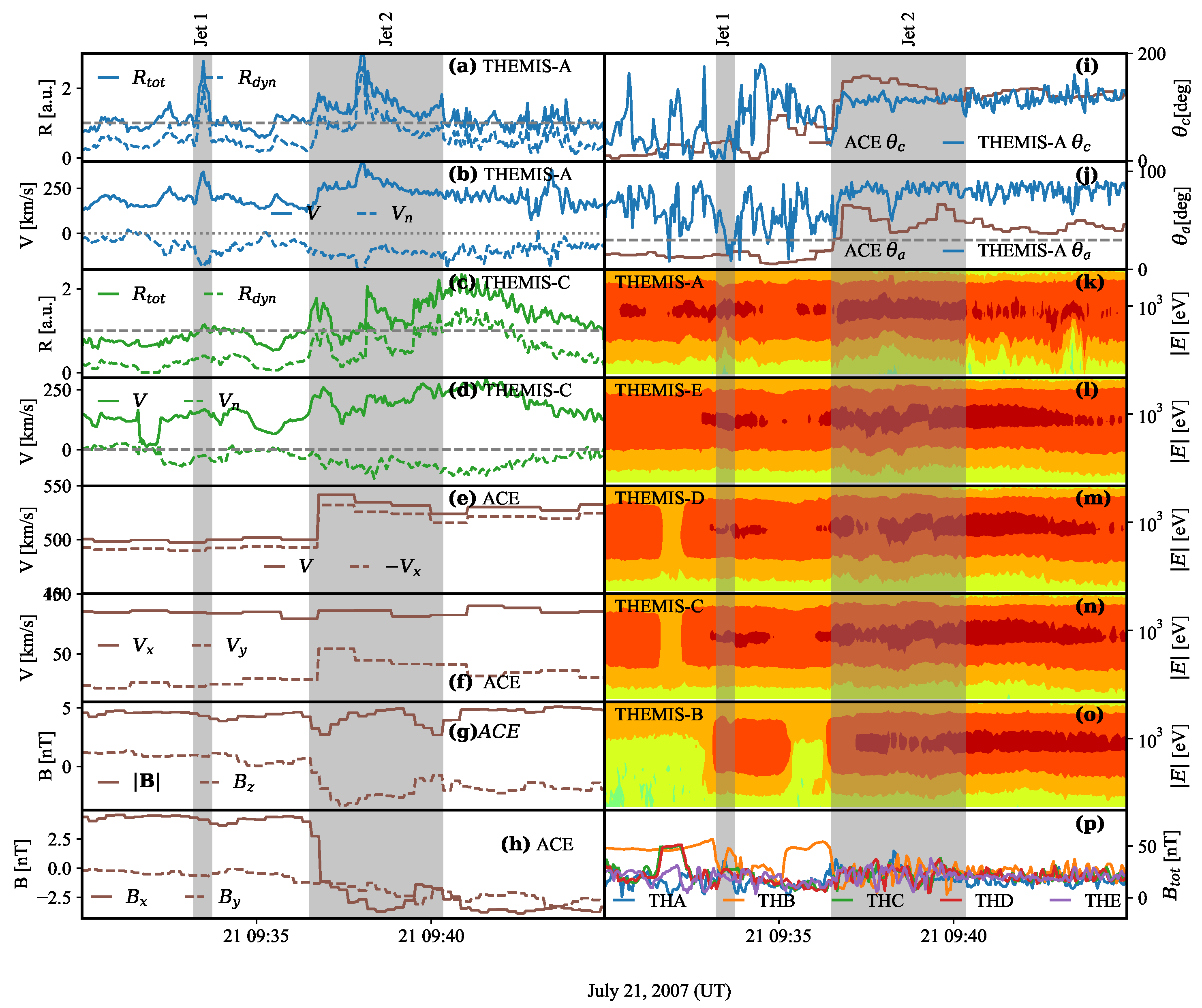
3. Results
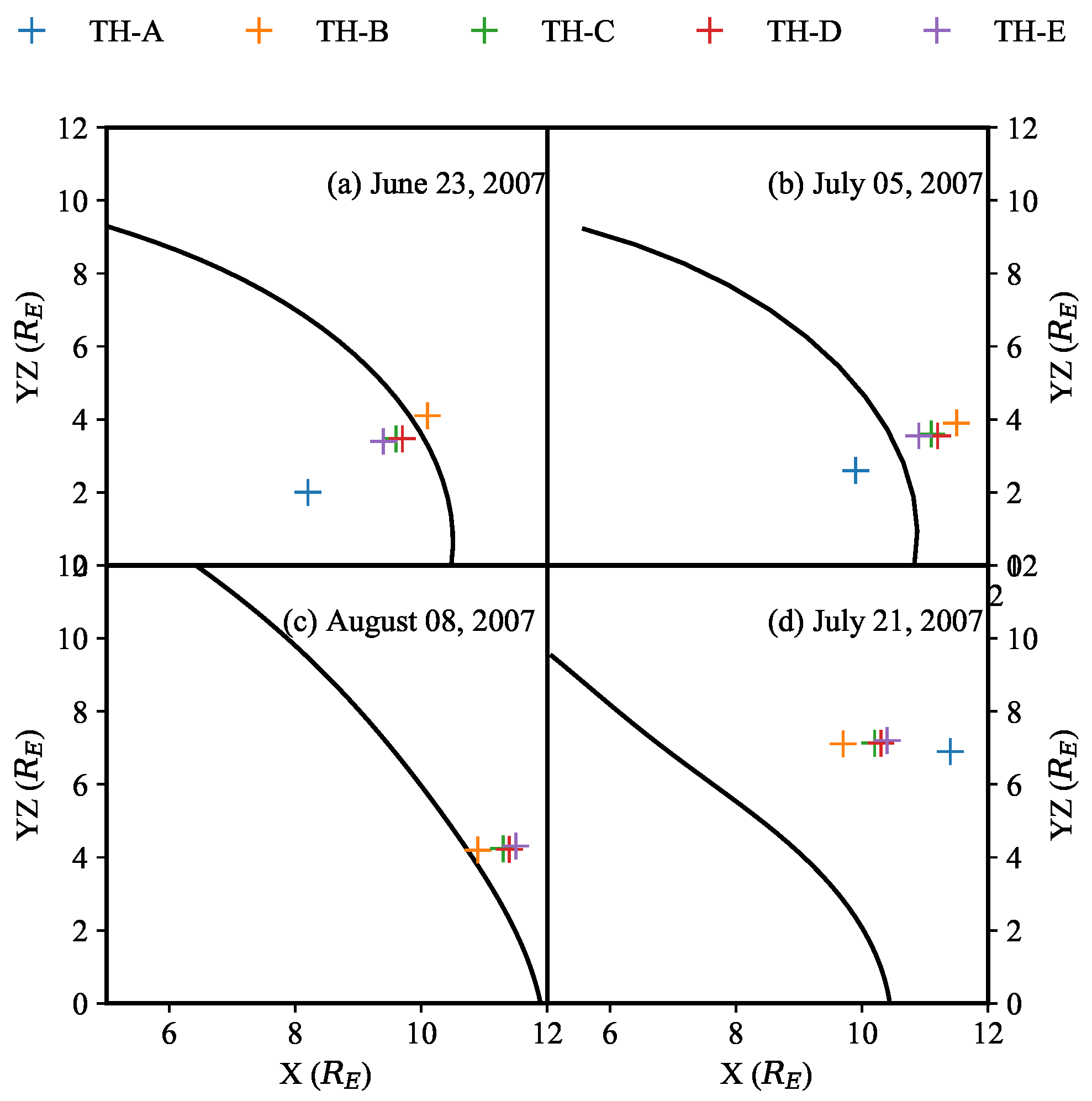
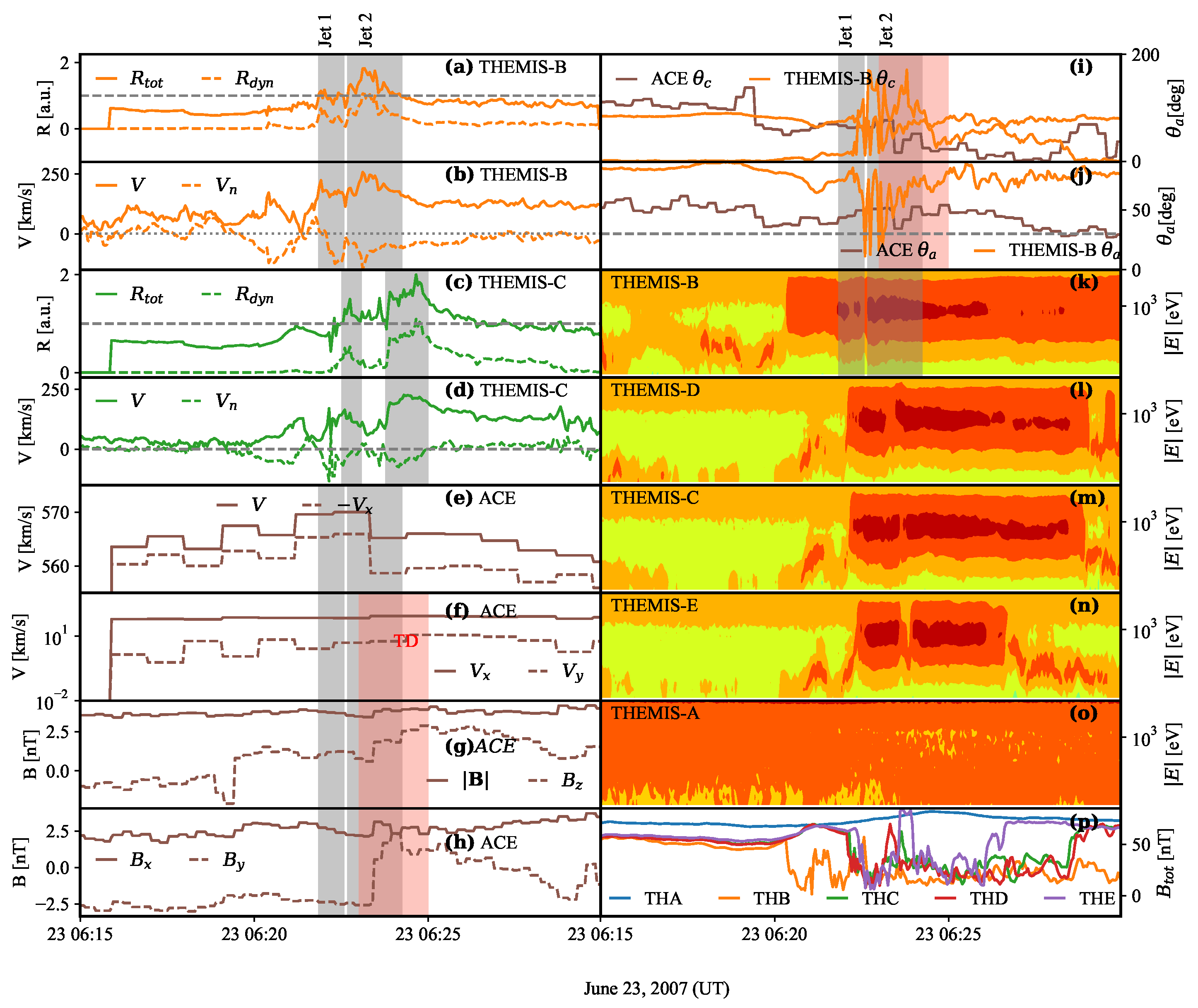
3.1. Tangential Discontinuity
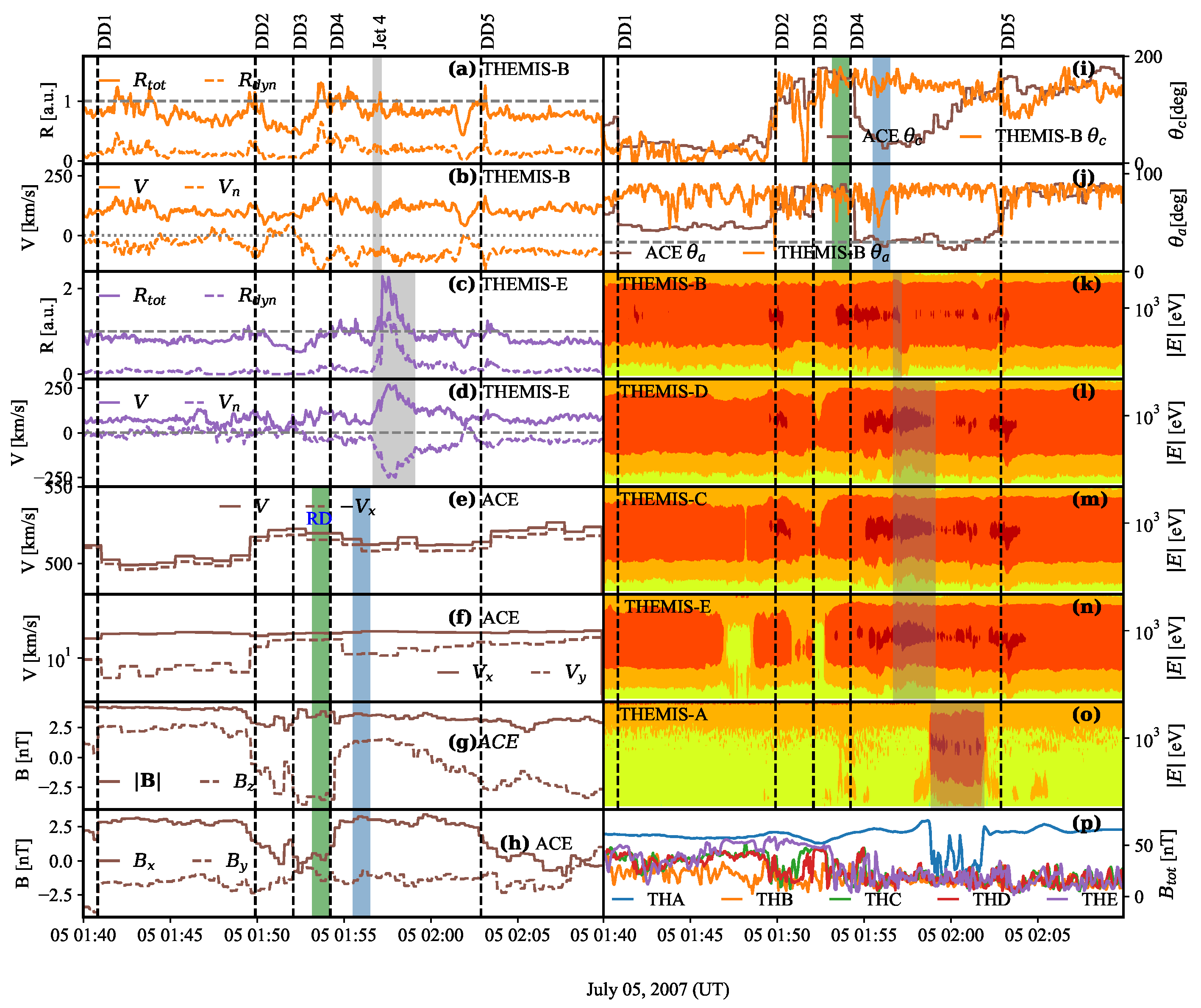
3.2. Rotational Discontinuity
3.3. Quasi-Radial IMF
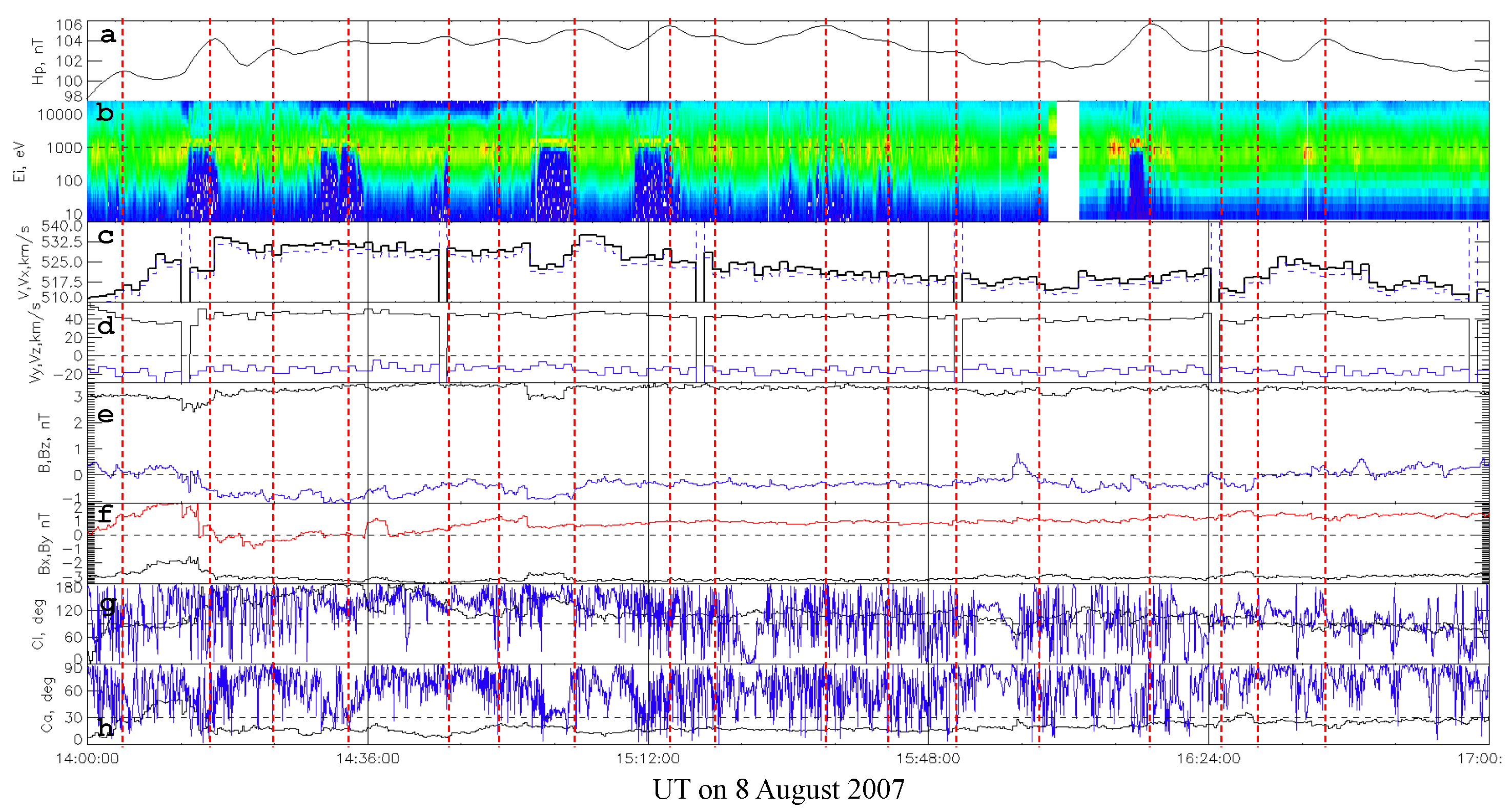
3.4. Collapsing Foreshock
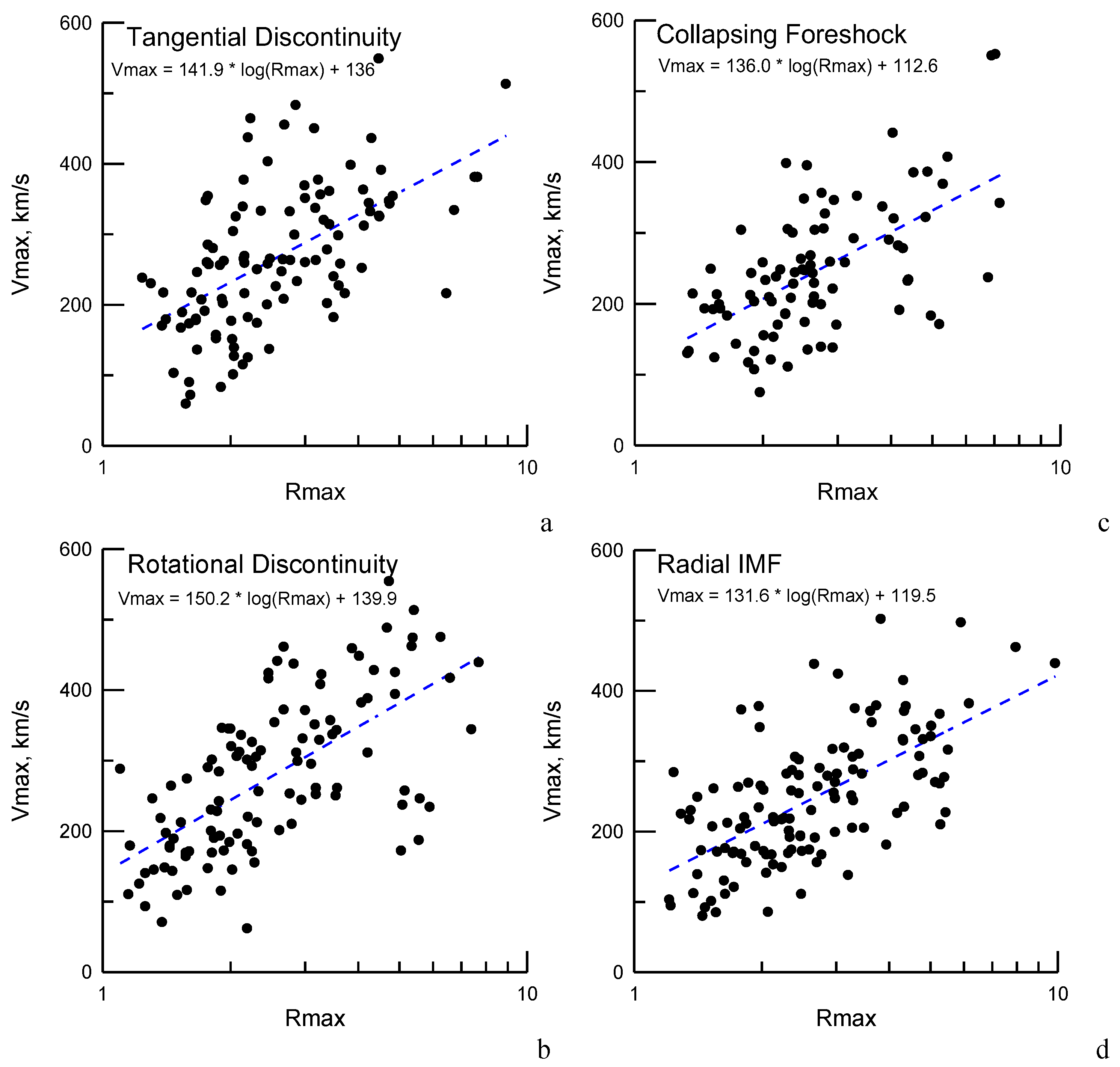
3.5. Statistical Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Borovsky, J.E.; Valdivia, J.A. The Earth’s Magnetosphere: A Systems Science Overview and Assessment. Surv. Geophys. 2018, 39, 817–859. [Google Scholar] [CrossRef]
- Plaschke, F.; Hietala, H.; Archer, M.; Blanco-Cano, X.; Kajdič, P.; Karlsson, T.; Lee, S.H.; Omidi, N.; Palmroth, M.; Roytershteyn, V.; et al. Jets Downstream of Collisionless Shocks. Space Sci. Rev. 2018, 214, 81. [Google Scholar] [CrossRef]
- Escoubet, C.P.; Hwang, K.J.; Toledo-Redondo, S.; Turc, L.; Haaland, S.E.; Aunai, N.; Dargent, J.; Eastwood, J.P.; Fear, R.C.; Fu, H.; et al. Cluster and MMS Simultaneous Observations of Magnetosheath High Speed Jets and Their Impact on the Magnetopause. Front. Astron. Sp. Sci. 2020, 6, 78. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Lindahl, S.; Viljanen, A.; Pirjola, R. Geomagnetic storm of 29–31 October 2003: Geomagnetically induced currents and their relation to problems in the Swedish high-voltage power transmission system. Sp. Weather 2005, 3. [Google Scholar] [CrossRef]
- Horne, R.B.; Phillips, M.W.; Glauert, S.A.; Meredith, N.P.; Hands, A.D.P.; Ryden, K.A.; Li, W. Realistic Worst Case for a Severe Space Weather Event Driven by a Fast Solar Wind Stream. Sp. Weather 2018, 16, 1202–1215. [Google Scholar] [CrossRef] [PubMed]
- Tsurutani, B.; Lakhina, G.; Verkhoglyadova, O.; Gonzalez, W.; Echer, E.; Guarnieri, F. A review of interplanetary discontinuities and their geomagnetic effects. J. Atmos. Solar-Terrestrial Phys. 2011, 73, 5–19. [Google Scholar] [CrossRef]
- Archer, M.O.; Horbury, T.S.; Eastwood, J.P. Magnetosheath pressure pulses: Generation downstream of the bow shock from solar wind discontinuities. J. Geophys. Res. Sp. Phys. 2012, 117, 1–13. [Google Scholar] [CrossRef]
- Sibeck, D.G.; Kudela, K.; Lepping, R.P.; Lin, R.; Nemecek, Z.; Nozdrachev, M.N.; Phan, T.D.; Prech, L.; Safrankova, J.; Singer, H.; et al. Magnetopause motion driven by interplanetary magnetic field variations. J. Geophys. Res. Sp. Phys. 2000, 105, 25155–25169. [Google Scholar] [CrossRef]
- Savin, S.; Amata, E.; Zelenyi, L.; Lutsenko, V.; Safrankova, J.; Nemecek, Z.; Borodkova, N.; Buechner, J.; Daly, P.W.; Kronberg, E.A.; et al. Super fast plasma streams as drivers of transient and anomalous magnetospheric dynamics. Ann. Geophys. 2012, 30, 1–7. [Google Scholar] [CrossRef]
- Lin, Y.; Swift, D.W.; Lee, L.C. Simulation of pressure pulses in the bow shock and magnetosheath driven by variations in interplanetary magnetic field direction. J. Geophys. Res. Sp. Phys. 1996, 101, 27251–27269. [Google Scholar] [CrossRef]
- Tsubouchi, K.; Matsumoto, H. Effect of upstream rotational field on the formation of magnetic depressions in a quasi- Perpendicular shock downstream. J. Geophys. Res. Sp. Phys. 2005, 110, 1–11. [Google Scholar] [CrossRef]
- Dmitriev, A.V.; Suvorova, A.V. Traveling magnetopause distortion related to a large-scale magnetosheath plasma jet: THEMIS and ground-based observations. J. Geophys. Res. Sp. Phys. 2012, 117, 1–16. [Google Scholar] [CrossRef]
- Neugebauer, M.; Clay, D.R.; Tsurutani, B.T.; Zwickl, R.D.; Goldstein, B.E. Reexamination of Rotational and Tangential Discontinuities in the Solar Wind. J. Geophys. Res. 1984, 89, 5395–5408. [Google Scholar] [CrossRef]
- Artemyev, A.V.; Angelopoulos, V.; Vasko, I.Y. Kinetic Properties of Solar Wind Discontinuities at 1 AU Observed by ARTEMIS. J. Geophys. Res. Sp. Phys. 2019, 124, 3858–3870. [Google Scholar] [CrossRef]
- Neukirch, T.; Vasko, I.Y.; Artemyev, A.V.; Allanson, O. Kinetic models of tangential discontinuities in the solar wind. Astrophys. J. 2020, 891, 86. [Google Scholar] [CrossRef]
- Neugebauer, M. The structure of rotational discontinuities. Geophys. Res. Lett. 1989, 16, 1261–1264. [Google Scholar] [CrossRef]
- Suvorova, A.V.; Shue, J.H.; Dmitriev, A.V.; Sibeck, D.G.; McFadden, J.P.; Hasegawa, H.; Ackerson, K.; Jelínek, K.; Šafráková, J.; Němeček, Z. Magnetopause expansions for quasi-radial interplanetary magnetic field: THEMIS and Geotail observations. J. Geophys. Res. Sp. Phys. 2010, 115, 1–16. [Google Scholar] [CrossRef]
- Dmitriev, A.V.; Suvorova, A.V. Large-scale jets in the magnetosheath and plasma penetration across the magnetopause: THEMIS observations. J. Geophys. Res. Sp. Phys. 2015, 120, 4423–4437. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Nakamura, R.; Turc, L.; Mejnertsen, L.; Hesse, M. The Scientific Foundations of Forecasting Magnetospheric Space Weather. Space Sci. Rev. 2017, 212, 1221–1252. [Google Scholar] [CrossRef]
- Sibeck, D.G.; Trivedi, N.B.; Zesta, E.; Decker, R.B.; Singer, H.J.; Szabo, A.; Tachihara, H.; Watermann, J. Pressure-pulse interaction with the magnetosphere and ionosphere. J. Geophys. Res. Sp. Phys. 2003, 108, 1–12. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Sibeck, D.G.; Angelopoulos, V.; Phan, T.D.; Bale, S.D.; McFadden, J.P.; Cully, C.M.; Mende, S.B.; Larson, D.; Frey, S.; et al. THEMIS observations of a hot flow anomaly: Solar wind, magnetosheath, and ground-based measurements. Geophys. Res. Lett. 2008, 35, 1–5. [Google Scholar] [CrossRef]
- Jacobsen, K.S.; Phan, T.D.; Eastwood, J.P.; Sibeck, D.G.; Moen, J.I.; Angelopoulos, V.; McFadden, J.P.; Engebretson, M.J.; Provan, G.; Larson, D.; et al. THEMIS observations of extreme magnetopause motion caused by a hot flow anomaly. J. Geophys. Res. Sp. Phys. 2009, 114. [Google Scholar] [CrossRef]
- Archer, M.O.; Horbury, T.S.; Eastwood, J.P.; Weygand, J.M.; Yeoman, T.K. Magnetospheric response to magnetosheath pressure pulses: A low-pass filter effect. J. Geophys. Res. Sp. Phys. 2013, 118, 5454–5466. [Google Scholar] [CrossRef]
- Plaschke, F.; Hietala, H.; Angelopoulos, V. Anti-sunward high-speed jets in the subsolar magnetosheath. Ann. Geophys. 2013, 31, 1877–1889. [Google Scholar] [CrossRef]
- Savin, S.; Amata, E.; Zelenyi, L.; Budaev, V.; Consolini, G.; Treumann, R.; Lucek, E.; Safrankova, J.; Nemecek, Z.; Khotyaintsev, Y.; et al. High energy jets in the Earth’s magnetosheath: Implications for plasma dynamics and anomalous transport. JETP Lett. 2008, 87, 593–599. [Google Scholar] [CrossRef]
- Hietala, H.; Plaschke, F. On the generation of magnetosheath high-speed jets by bow shock ripples. J. Geophys. Res. Sp. Phys. 2013, 118, 7237–7245. [Google Scholar] [CrossRef]
- Lucek, E.A.; Horbury, T.S.; Dandouras, I.; Réme, H. Cluster observations of the Earth’s quasi-parallel bow shock. J. Geophys. Res. Sp. Phys. 2008, 113, 1–11. [Google Scholar] [CrossRef]
- Archer, M.O.; Horbury, T.S. Magnetosheath dynamic pressure enhancements: Occurrence and typical properties. Ann. Geophys. 2013, 31, 319–331. [Google Scholar] [CrossRef]
- Pi, G.; Shue, J.H.; Chao, J.K.; Němeček, Z.; Šafránková, J.; Lin, C.H. A reexamination of long-duration radial IMF events. J. Geophys. Res. Sp. Phys. 2014, 119, 7005–7011. [Google Scholar] [CrossRef]
- Angelopoulos, V. The THEMIS Mission. Space Sci. Rev. 2008, 141, 5–34. [Google Scholar] [CrossRef]
- Stone, E.C.; Frandsen, A.M.; Mewaldt, R.A.; Christian, E.R.; Margolies, D.; Ormes, J.F.; Snow, F. The Advanced Composition Explorer. Space Sci. Rev. 1998, 86, 1–22. [Google Scholar] [CrossRef]
- Lepping, R.P.; Acũna, M.H.; Burlaga, L.F.; Farrell, W.M.; Slavin, J.A.; Schatten, K.H.; Mariani, F.; Ness, N.F.; Neubauer, F.M.; Whang, Y.C.; et al. The WIND magnetic field investigation. Space Sci. Rev. 1995, 71, 207–229. [Google Scholar] [CrossRef]
- Auster, H.U.; Glassmeier, K.H.; Magnes, W.; Aydogar, O.; Baumjohann, W.; Constantinescu, D.; Fischer, D.; Fornacon, K.H.; Georgescu, E.; Harvey, P.; et al. The THEMIS fluxgate magnetometer. Space Sci. Rev. 2008, 141, 235–264. [Google Scholar] [CrossRef]
- McFadden, J.P.; Carlson, C.W.; Larson, D.; Ludlam, M.; Abiad, R.; Elliott, B.; Turin, P.; Marckwordt, M.; Angelopoulos, V. The THEMIS ESA plasma instrument and in-flight calibration. Space Sci. Rev. 2008, 141, 277–302. [Google Scholar] [CrossRef]
- Chao, J.K.; Wu, D.J.; Lin, C.H.; Yang, Y.H.; Wang, X.Y.; Kessel, M.; Chen, S.H.; Lepping, R.P. Models for the size and shape of the earth’s magnetopause and bow shock. COSPAR Colloq. Ser. 2002, 12, 127–135. [Google Scholar] [CrossRef]
- Lin, R.L.; Zhang, X.X.; Liu, S.Q.; Wang, Y.L.; Gong, J.C. A three-dimensional asymmetric magnetopause model. J. Geophys. Res. Sp. Phys. 2010, 115. [Google Scholar] [CrossRef]
- Dmitriev, A.V.; Chao, J.K.; Wu, D.J. Comparative study of bow shock models using Wind and Geotail observations. J. Geophys. Res. Sp. Phys. 2003, 108, 1–19. [Google Scholar] [CrossRef]
- Escoubet, C.P.; Schmidt, R.; Goldstein, M.L. Cluster—Science and Mission Overview. In The Cluster and Phoenix Missions; Springer: Dordrecht, The Netherlands, 1997; Volume 79, pp. 11–32. [Google Scholar] [CrossRef]
- Burch, J.L.; Moore, T.E.; Torbert, R.B.; Giles, B.L. Magnetospheric Multiscale Overview and Science Objectives. Space Sci. Rev. 2016, 199, 5–21. [Google Scholar] [CrossRef]
- Lu, S.W.; Wang, C.; Li, W.Y.; Tang, B.B.; Torbert, R.B.; Giles, B.L.; Russell, C.T.; Burch, J.L.; McFadden, J.P.; Auster, H.U.; et al. Prolonged Kelvin–Helmholtz Waves at Dawn and Dusk Flank Magnetopause: Simultaneous Observations by MMS and THEMIS. Astrophys. J. 2019, 875, 57. [Google Scholar] [CrossRef]
- Dmitriev, A.V.; Lin, R.L.; Liu, S.Q.; Suvorova, A.V. Model prediction of geosynchronous magnetopause crossings. Sp. Weather 2016, 14, 530–543. [Google Scholar] [CrossRef]
- Plaschke, F.; Hietala, H.; Angelopoulos, V.; Nakamura, R. Geoeffective jets impacting the magnetopause are very common. J. Geophys. Res. Sp. Phys. 2016, 121, 3240–3253. [Google Scholar] [CrossRef]
- Burton, R.K.; McPherron, R.L.; Russell, C.T. An empirical relationship between interplanetary conditions and Dst. J. Geophys. Res. 1975, 80, 4204–4214. [Google Scholar] [CrossRef]
- Shue, J.H.; Chao, J.K.; Song, P.; McFadden, J.P.; Suvorova, A.; Angelopoulos, V.; Glassmeier, K.H.; Plaschke, F. Anomalous magnetosheath flows and distorted subsolar magnetopause for radial interplanetary magnetic fields. Geophys. Res. Lett. 2009, 36, 3–7. [Google Scholar] [CrossRef]
- Voitcu, G.; Echim, M. Tangential deflection and formation of counterstreaming flows at the impact of a plasma jet on a tangential discontinuity. Geophys. Res. Lett. 2017, 44, 5920–5927. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, L.; He, J.; Tu, C.; Wang, L.; Marsch, E.; Wang, X.; Zhang, S.; Feng, X. The formation of rotational discontinuities in compressive three-dimensional mhd turbulence. Astrophys. J. 2015, 809, 155. [Google Scholar] [CrossRef]
- Plaschke, F.; Karlsson, T.; Hietala, H.; Archer, M.; Vörös, Z.; Nakamura, R.; Magnes, W.; Baumjohann, W.; Torbert, R.B.; Russell, C.T.; et al. Magnetosheath High-Speed Jets: Internal Structure and Interaction with Ambient Plasma. J. Geophys. Res. Sp. Phys. 2017, 122, 10,157–10,175. [Google Scholar] [CrossRef]
- Vuorinen, L.; Hietala, H.; Plaschke, F. Jets in the magnetosheath: IMF control of where they occur. Ann. Geophys. 2019, 37, 689–697. [Google Scholar] [CrossRef]
- Walén, C. On the Theory of Sunspots. Arkiv för Matematik Astronomi och Fysik 1944, 30A, 1–87. [Google Scholar]
- Crooker, N.U.; Eastman, T.E.; Frank, L.A.; Smith, E.J.; Russell, C.T. Energetic magnetosheath ions and the interplanetary magnetic field orientation. J. Geophys. Res. Sp. Phys. 1981, 86, 4455–4460. [Google Scholar] [CrossRef]
- Terasawa, T. Energy spectrum of ions accelerated through Fermi process at the terrestrial bow shock. J. Geophys. Res. Sp. Phys. 1981, 86, 7595–7606. [Google Scholar] [CrossRef]
- Fuselier, S.A.; Lennartsson, O.W.; Thomsen, M.F.; Russell, C.T. He2+ heating at a quasi-parallel shock. J. Geophys. Res. 1991, 96, 9805. [Google Scholar] [CrossRef]
- Suvorova, A.V.; Dmitriev, A.V. On magnetopause inflation under radial IMF. Adv. Sp. Res. 2016, 58, 249–256. [Google Scholar] [CrossRef]
- Jelínek, K.; Němeček, Z.; Šafránková, J.; Shue, J.H.; Suvorova, A.V.; Sibeck, D.G. Thin magnetosheath as a consequence of the magnetopause deformation: THEMIS observations. J. Geophys. Res. Sp. Phys. 2010, 115. [Google Scholar] [CrossRef]
- Kajdič, P.; Blanco-Cano, X.; Omidi, N.; Rojas-Castillo, D.; Sibeck, D.G.; Billingham, L. Traveling Foreshocks and Transient Foreshock Phenomena. J. Geophys. Res. Sp. Phys. 2017, 122, 9148–9168. [Google Scholar] [CrossRef]
- Sonnerup, B.; Scheible, M. Minimum and maximum variance analysis. Anal. Methods Multi-Spacecr. Data 1998, 1, 185–220. [Google Scholar]
- Weimer, D.R.; Ober, D.M.; Maynard, N.C.; Burke, W.J.; Collier, M.R.; McComas, D.J.; Ness, N.F.; Smith, C.W. Variable time delays in the propagation of the interplanetary magnetic field. J. Geophys. Res. Sp. Phys. 2002, 107. [Google Scholar] [CrossRef]
- Knetter, T.; Neubauer, F.M.; Horbury, T.; Balogh, A. Four-point discontinuity observations using Cluster magnetic field data: A statistical survey. J. Geophys. Res. Sp. Phys. 2004, 109, 1–12. [Google Scholar] [CrossRef]
- Sergeev, V.A.; Sormakov, D.A.; Apatenkov, S.V.; Baumjohann, W.; Nakamura, R.; Runov, A.V.; Mukai, T.; Nagai, T. Survey of large-amplitude flapping motions in the midtail current sheet. Ann. Geophys. 2006, 24, 2015–2024. [Google Scholar] [CrossRef]
- Sonnerup, B.U.Ö. Orientation and motion of two-dimensional structures in a space plasma. J. Geophys. Res. 2005, 110, A06208. [Google Scholar] [CrossRef]
- Gosling, J.T.; Asbridge, J.R.; Bame, S.J.; Paschmann, G.; Sckopke, N. Observations of two distinct populations of bow shock ions in the upstream solar wind. Geophys. Res. Lett. 1978, 5, 957–960. [Google Scholar] [CrossRef]
- Riazantseva, M.O.; Dalin, P.A.; Dmitriev, A.V.; Orlov, Y.V.; Paularena, K.I.; Richardson, J.D.; Zastenker, G.N. A multifactor analysis of parameters controlling solar wind ion flux correlations using an artificial neural network technique. J. Atmos. Sol.-Terr. Phys. 2002, 64, 657–660. [Google Scholar] [CrossRef]

| Structure | Type | Number | Percentage (Structure) | Percentage |
|---|---|---|---|---|
| Discontinuities | RD | 131 | 24% | 49% |
| TD | 139 | 25% | ||
| Quasi-radial IMF | CFS | 110 | 20% | 51% |
| rIMF | 174 | 31% |
| Structure | Slope | Vmax | Rmax | Rmax > 5 |
|---|---|---|---|---|
| RD | 150 ± 17 | 275 ± 110 | 2.3 ± 1.6 | 13% |
| TD | 142 ± 20 | 265 ± 102 | 2.4 ± 1.5 | 6% |
| CFS | 136 ± 20 | 245 ± 92 | 2.4 ± 1.5 | 9% |
| rIMF | 132 ± 15 | 246 ± 91 | 2.3 ± 1.6 | 7% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dmitriev, A.V.; Lalchand, B.; Ghosh, S. Mechanisms and Evolution of Geoeffective Large-Scale Plasma Jets in the Magnetosheath. Universe 2021, 7, 152. https://doi.org/10.3390/universe7050152
Dmitriev AV, Lalchand B, Ghosh S. Mechanisms and Evolution of Geoeffective Large-Scale Plasma Jets in the Magnetosheath. Universe. 2021; 7(5):152. https://doi.org/10.3390/universe7050152
Chicago/Turabian StyleDmitriev, Alexei V., Bhavana Lalchand, and Sayantan Ghosh. 2021. "Mechanisms and Evolution of Geoeffective Large-Scale Plasma Jets in the Magnetosheath" Universe 7, no. 5: 152. https://doi.org/10.3390/universe7050152
APA StyleDmitriev, A. V., Lalchand, B., & Ghosh, S. (2021). Mechanisms and Evolution of Geoeffective Large-Scale Plasma Jets in the Magnetosheath. Universe, 7(5), 152. https://doi.org/10.3390/universe7050152







