De Sitter Solutions in Einstein–Gauss–Bonnet Gravity †
Abstract
1. Introduction
2. Models the Gauss–Bonnet Term
3. Stability of de Sitter Solutions
4. Different Models with the Same Structure of de Sitter Solutions
5. Examples of Models
5.1. Evolution Equations
5.2. The Function F in a Role of the Effective Potential
5.3. The Case of a Power Function of the Gauss–Bonnet Term
- 1.
- At , we get de Sitter solution
- 2.
- At we get
- 3.
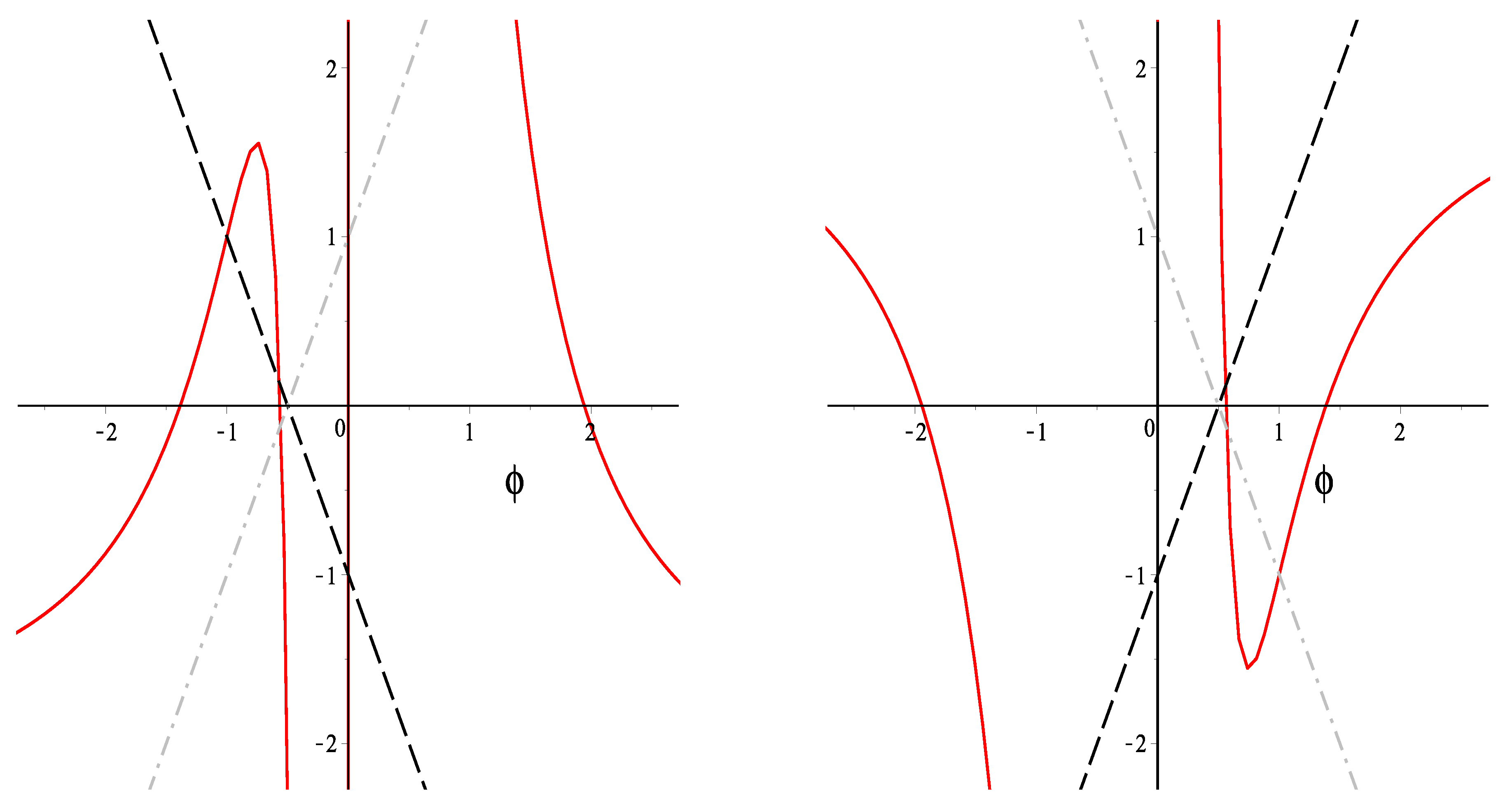
5.4. The Case of a Quadratic Polynomial
5.4.1. Equation for
5.4.2. The Case Gravity
5.4.3. The Case Gravity
5.4.4. The Case of the Absence of the Cosmological Constant
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Capozziello, S.; Faraoni, V. Beyond Einstein Gravity: A Survey of Gravitational Theories for Cosmology and Astrophysics; Springer: New York, NY, USA, 2011. [Google Scholar]
- Capozziello, S.; De Laurentis, M. Extended Theories of Gravity. Phys. Rep. 2011, 509, 167–321. [Google Scholar] [CrossRef]
- Fujii, Y.; Maeda, K. The Scalar—Tensor Theory of Gravitation; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Faraoni, V. Cosmology in Scalar—Tensor Gravity; Kluwer Academic: Dordrecht, The Netherlands, 2004. [Google Scholar]
- Chernikov, E.A.; Tagirov, E.A. Quantum theory of scalar fields in de Sitter space-time. Ann. Poincare Phys. Theor. A 1968, 9, 109. [Google Scholar]
- Tagirov, E.A. Consequences of field quantization in de Sitter type cosmological models. Ann. Phys. 1973, 76, 561. [Google Scholar] [CrossRef]
- Callan, C.G.; Coleman, S.R.; Jackiw, R. A New improved energy—Momentum tensor. Ann. Phys. 1970, 59, 42. [Google Scholar] [CrossRef]
- Barvinsky, A.O.; Kamenshchik, A.Y. Quantum scale of inflation and particle physics of the early universe. Phys. Lett. B 1994, 332, 270. [Google Scholar] [CrossRef]
- Bezrukov, F.L.; Shaposhnikov, M. The Standard Model Higgs boson as the inflaton. Phys. Lett. B 2008, 659, 703. [Google Scholar] [CrossRef]
- Barvinsky, A.O.; Kamenshchik, A.Y.; Starobinsky, A.A. Inflation scenario via the Standard Model Higgs boson and LHC. J. Cosmol. Asropart. Phys. 2008, 2008, 021. [Google Scholar] [CrossRef]
- De Simone, A.; Hertzberg, M.P.; Wilczek, F. Running Inflation in the Standard Model. Phys. Lett. B 2009, 678, 1. [Google Scholar] [CrossRef]
- Barvinsky, A.O.; Kamenshchik, A.Y.; Kiefer, C.; Starobinsky, A.A.; Steinwachs, C.F. Higgs boson, renormalization group, and cosmology. Eur. Phys. J. C 2012, 72, 2219. [Google Scholar] [CrossRef]
- Lerner, R.N.; McDonald, J. Higgs Inflation and Naturalness. J. Cosmol. Astropart. Phys. 2010, 1004, 015. [Google Scholar] [CrossRef]
- Bezrukov, F.L.; Magnin, A.; Shaposhnikov, M.; Sibiryakov, S. Higgs inflation: Consistency and generalisations. J. High Energy Phys. 2011, 1101, 016. [Google Scholar] [CrossRef]
- Greenwood, R.N.; Kaiser, D.I.; Sfakianakis, E.I. Multifield Dynamics of Higgs Inflation. Phys. Rev. D 2013, 87, 064021. [Google Scholar] [CrossRef]
- Bezrukov, F.L. The Higgs field as an inflaton. Class. Quant. Grav. 2013, 30, 214001. [Google Scholar] [CrossRef]
- Cerioni, A.; Finelli, F.; Tronconi, A.; Venturi, G. Inflation and Reheating in Induced Gravity. Phys. Lett. B 2009, 681, 383. [Google Scholar] [CrossRef]
- Kallosh, R.; Linde, A.; Roest, D. The double attractor behavior of induced inflation. J. High Energy Phys. 2014, 1409, 062. [Google Scholar] [CrossRef]
- Rinaldi, M.; Vanzo, L.; Zerbini, S.; Venturi, G. Inflationary quasi-scale invariant attractors. Phys. Rev. D 2016, 93, 024040. [Google Scholar] [CrossRef]
- Elizalde, E.; Odintsov, S.D.; Pozdeeva, E.O.; Vernov, S.Y. Renormalization-group inflationary scalar electrodynamics and SU(5) scenarios confronted with Planck2013 and BICEP2 results. Phys. Rev. D 2014, 90, 084001. [Google Scholar] [CrossRef]
- Elizalde, E.; Odintsov, S.D.; Pozdeeva, E.O.; Vernov, S.Y. Cosmological attractor inflation from the RG-improved Higgs sector of finite gauge theory. J. Cosmol. Astropart. Phys. 2016, 1602, 025. [Google Scholar] [CrossRef]
- Pozdeeva, E.O.; Vernov, S.Y. Renormalization-group improved inflationary scenarios. Phys. Part. Nucl. Lett. 2017, 14, 386. [Google Scholar] [CrossRef][Green Version]
- Dubinin, M.N.; Petrova, E.Y.; Pozdeeva, E.O.; Sumin, M.V.; Vernov, S.Y. MSSM-inspired multifield inflation. J. High Energy Phys. 2017, 1712, 036. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Tronconi, A.; Venturi, G. Quantum cosmology and the inflationary spectra from a nonminimally coupled inflaton. Phys. Rev. D 2020, 101, 023534. [Google Scholar] [CrossRef]
- van de Bruck, C.; Longden, C. Higgs Inflation with a Gauss–Bonnet term in the Jordan Frame. Phys. Rev. D 2016, 93, 063519. [Google Scholar] [CrossRef]
- Mathew, J.; Shankaranarayanan, S. Low scale Higgs inflation with Gauss–Bonnet coupling. Astropart. Phys. 2016, 84, 1. [Google Scholar] [CrossRef]
- Pozdeeva, E.O.; Vernov, S.Y. Construction of inflationary scenarios with the Gauss–Bonnet term and nonminimal coupling. arXiv 2021, arXiv:2104.04995. [Google Scholar]
- Nojiri, S.; Odintsov, S.D.; Sasaki, M. Gauss–Bonnet dark energy. Phys. Rev. D 2005, 71, 123509. [Google Scholar] [CrossRef]
- Cognola, G.; Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Zerbini, S. Dark energy in modified Gauss–Bonnet gravity: Late-time acceleration and the hierarchy problem. Phys. Rev. D 2006, 73, 084007. [Google Scholar] [CrossRef]
- Cognola, G.; Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Zerbini, S. String-inspired Gauss–Bonnet gravity reconstructed from the universe expansion history and yielding the transition from matter dominance to dark energy. Phys. Rev. D 2007, 75, 086002. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Oikonomou, V.K. Modified Gravity Theories on a Nutshell: Inflation, Bounce and Late-time Evolution. Phys. Rept. 2017, 692, 1–104. [Google Scholar] [CrossRef]
- Antoniadis, I.; Rizos, J.; Tamvakis, K. Singularity-free cosmological solutions of the superstring effective action. Nucl. Phys. B 1994, 415, 497. [Google Scholar] [CrossRef]
- Kawai, S.; Soda, J. Evolution of fluctuations during graceful exit in string cosmology. Phys. Lett. B 1999, 460, 41. [Google Scholar] [CrossRef]
- Cartier, C.; Hwang, J.C.; Copeland, E.J. Evolution of cosmological perturbations in nonsingular string cosmologies. Phys. Rev. D 2001, 64, 103504. [Google Scholar] [CrossRef]
- Hwang, J.C.; Noh, H. Classical evolution and quantum generation in generalized gravity theories including string corrections and tachyon: Unified analyses. Phys. Rev. D 2005, 71, 063536. [Google Scholar] [CrossRef]
- Calcagni, G.; Tsujikawa, S.; Sami, M. Dark energy and cosmological solutions in second-order string gravity. Class. Quant. Grav. 2005, 22, 3977. [Google Scholar] [CrossRef]
- Tsujikawa, S.; Sami, M. String-inspired cosmology: Late time transition from scaling matter era to dark energy universe caused by a Gauss–Bonnet coupling. J. Cosmol. Astropart. Phys. 2007, 0701, 006. [Google Scholar] [CrossRef]
- Guo, Z.; Schwarz, D.J. Slow-roll inflation with a Gauss–Bonnet correction. Phys. Rev. D 2010, 81, 123520. [Google Scholar] [CrossRef]
- Koh, S.; Lee, B.H.; Lee, W.; Tumurtushaa, G. Observational constraints on slow-roll inflation coupled to a Gauss–Bonnet term. Phys. Rev. D 2014, 90, 063527. [Google Scholar] [CrossRef]
- Jiang, P.X.; Hu, J.W.; Guo, Z.K. Inflation coupled to a Gauss–Bonnet term. Phys. Rev. D 2013, 88, 123508. [Google Scholar] [CrossRef]
- De Laurentis, M.; Paolella, M.; Capozziello, S. Cosmological inflation in F(R, ) gravity. Phys. Rev. D 2015, 91, 083531. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Autonomous dynamical system approach for inflationary Gauss–Bonnet modified gravity. Int. J. Mod. Phys. D 2018, 27, 1850059. [Google Scholar] [CrossRef]
- Wu, Q.; Zhu, T.; Wang, A. Primordial Spectra of slow-roll inflation at second-order with the Gauss–Bonnet correction. Phys. Rev. D 2018, 97, 103502. [Google Scholar] [CrossRef]
- Nozari, K.; Rashidi, N. Perturbation, nonGaussianity, and reheating in a Gauss–Bonnet α-attractor model. Phys. Rev. D 2017, 95, 123518. [Google Scholar] [CrossRef]
- Koh, S.; Lee, B.H.; Tumurtushaa, G. Reconstruction of the Scalar Field Potential in Inflationary Models with a Gauss–Bonnet term. Phys. Rev. D 2017, 95, 123509. [Google Scholar] [CrossRef]
- Chakraborty, S.; Paul, T.; SenGupta, S. Inflation driven by Einstein–Gauss–Bonnet gravity. Phys. Rev. D 2018, 98, 083539. [Google Scholar] [CrossRef]
- Yi, Z.; Gong, Y.; Sabir, M. Inflation with Gauss–Bonnet coupling. Phys. Rev. D 2018, 98, 083521. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Viable Inflation in Scalar–Gauss–Bonnet Gravity and Reconstruction from Observational Indices. Phys. Rev. D 2018, 98, 044039. [Google Scholar] [CrossRef]
- Yi, Z.; Gong, Y. Gauss–Bonnet Inflation and the String Swampland. Universe 2019, 5, 200. [Google Scholar] [CrossRef]
- Fomin, I.V.; Chervon, S.V. Reconstruction of GR cosmological solutions in modified gravity theories. Phys. Rev. D 2019, 100, 023511. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Fronimos, F.P. Rectifying Einstein–Gauss–Bonnet Inflation in View of GW170817. Nucl. Phys. B 2020, 958, 115135. [Google Scholar] [CrossRef]
- Odintsov, S.; Oikonomou, V.K. Swampland Implications of GW170817-compatible Einstein–Gauss–Bonnet Gravity. Phys. Lett. B 2020, 805, 135437. [Google Scholar] [CrossRef]
- Fomin, I. Gauss–Bonnet term corrections in scalar field cosmology. Eur. Phys. J. C 2020, 80, 1145. [Google Scholar] [CrossRef]
- Pozdeeva, E.O. Generalization of cosmological attractor approach to Einstein–Gauss–Bonnet gravity. Eur. Phys. J. C 2020, 80, 612. [Google Scholar] [CrossRef]
- Pozdeeva, E.O.; Gangopadhyay, M.R.; Sami, M.; Toporensky, A.V.; Vernov, S.Y. Inflation with a quartic potential in the framework of Einstein–Gauss–Bonnet gravity. Phys. Rev. D 2020, 102, 043525. [Google Scholar] [CrossRef]
- Oikonomou, V.K.; Fronimos, F.P. Non-minimally coupled Einstein–Gauss–Bonnet gravity with massless gravitons: The constant-roll case. Eur. Phys. J. Plus 2020, 135, 917. [Google Scholar] [CrossRef]
- Pozdeeva, E.O. Violation of the slow-roll regime in the EGB inflationary models with . arXiv 2021, arXiv:2105.02772. [Google Scholar]
- Sami, M.; Toporensky, A.V.; Tretjakov, P.V.; Tsujikawa, S. The Fate of (phantom) dark energy universe with string curvature corrections. Phys. Lett. B 2005, 619, 193. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Toporensky, A.V.; Tretjakov, P.V. Reconstruction and deceleration-acceleration transitions in modified gravity. Gen. Relativ. Grav. 2010, 42, 1997–2008. [Google Scholar] [CrossRef]
- Elizalde, E.; Myrzakulov, R.; Obukhov, V.V.; Saez-Gomez, D. LambdaCDM epoch reconstruction from F(R,G) and modified Gauss–Bonnet gravities. Class. Quant. Grav. 2010, 27, 095007. [Google Scholar] [CrossRef]
- Myrzakulov, R.; Saez-Gomez, D.; Tureanu, A. On the ΛCDM Universe in f(G) gravity. Gen. Relativ. Grav. 2011, 43, 1671–1684. [Google Scholar] [CrossRef]
- de la Cruz-Dombriz, A.; Saez-Gomez, D. On the stability of the cosmological solutions in f(R,G) gravity. Class. Quant. Grav. 2012, 29, 245014. [Google Scholar] [CrossRef]
- Benetti, M.; Santos da Costa, S.; Capozziello, S.; Alcaniz, J.S.; De Laurentis, M. Observational constraints on Gauss–Bonnet cosmology. Int. J. Mod. Phys. D 2018, 27, 1850084. [Google Scholar] [CrossRef]
- Navó, G.; Elizalde, E. Stability of hyperbolic and matter-dominated bounce cosmologies from F(R,) modified gravity at late evolution stages. Int. J. Geom. Meth. Mod. Phys. 2020, 17, 2050162. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Fronimos, F.P.; Fasoulakos, K.V. Unification of a Bounce with a Viable Dark Energy Era in Gauss–Bonnet Gravity. Phys. Rev. D 2020, 102, 104042. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Fronimos, F.P. Late-time cosmology of scalar-coupled f(R,) gravity. Class. Quant. Grav. 2021, 38, 075009. [Google Scholar] [CrossRef]
- Pozdeeva, E.O.; Sami, M.; Toporensky, A.V.; Vernov, S.Y. Stability analysis of de Sitter solutions in models with the Gauss–Bonnet term. Phys. Rev. D 2019, 100, 083527. [Google Scholar] [CrossRef]
- Skugoreva, M.A.; Toporensky, A.V.; Vernov, S.Y. Global stability analysis for cosmological models with nonminimally coupled scalar fields. Phys. Rev. D 2014, 90, 064044. [Google Scholar] [CrossRef]
- Pozdeeva, E.O.; Skugoreva, M.A.; Toporensky, A.V.; Vernov, S.Y. Possible evolution of a bouncing universe in cosmological models with nonminimally coupled scalar fields. J. Cosmol. Astropart. Phys. 2016, 1612, 006. [Google Scholar] [CrossRef]
- Järv, L.; Toporensky, A. Global portraits of nonminimal inflation. arXiv 2021, arXiv:2104.10183. [Google Scholar]
- Starobinsky, A.A. A New Type of Isotropic Cosmological Models without Singularity. Phys. Lett. B 1980, 91, 99. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Dynamics of phase transition in the new inflationary universe scenario and generation of perturbations. Phys. Lett. B 1982, 117, 175. [Google Scholar] [CrossRef]
- Starobinsky, A.A. The Perturbation Spectrum Evolving from a Nonsingular Initially De-Sitter Cosmology and the Microwave Background Anisotropy. Sov. Astron. Lett. 1983, 9, 302. [Google Scholar]
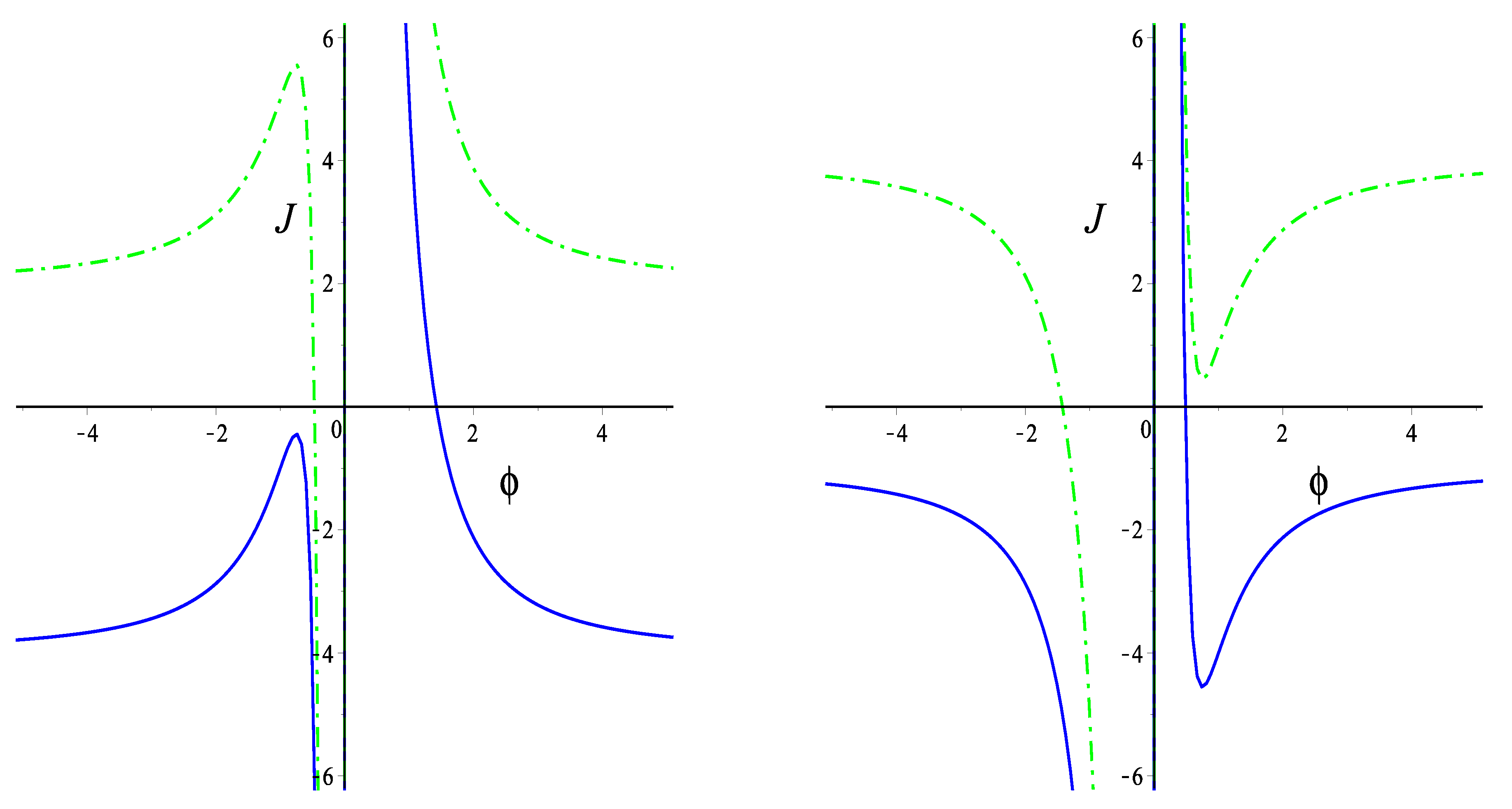
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vernov, S.; Pozdeeva, E. De Sitter Solutions in Einstein–Gauss–Bonnet Gravity. Universe 2021, 7, 149. https://doi.org/10.3390/universe7050149
Vernov S, Pozdeeva E. De Sitter Solutions in Einstein–Gauss–Bonnet Gravity. Universe. 2021; 7(5):149. https://doi.org/10.3390/universe7050149
Chicago/Turabian StyleVernov, Sergey, and Ekaterina Pozdeeva. 2021. "De Sitter Solutions in Einstein–Gauss–Bonnet Gravity" Universe 7, no. 5: 149. https://doi.org/10.3390/universe7050149
APA StyleVernov, S., & Pozdeeva, E. (2021). De Sitter Solutions in Einstein–Gauss–Bonnet Gravity. Universe, 7(5), 149. https://doi.org/10.3390/universe7050149





