Clock Time in Quantum Cosmology
Abstract
:1. Introduction
2. Mini-Superspace Model
3. Emergence of Time
3.1. WKB Time
3.2. Scalar Field as a Clock
3.3. Evolution Equation
4. Solution of the WD Equation with Clock
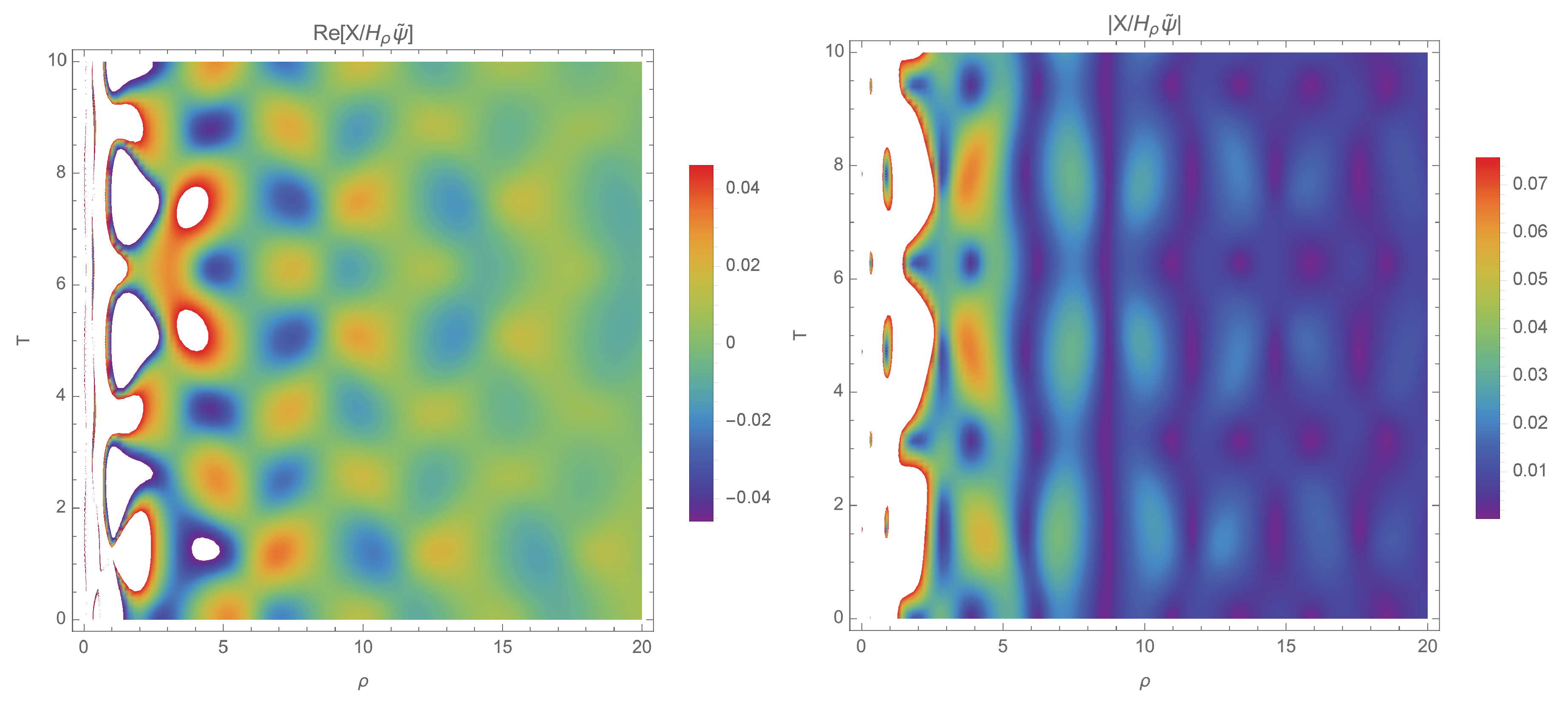
4.1. Clock with a Single Eigenstate
4.2. Clock with Two Eigenstates
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- DeWitt, B.S. Quantum theory of gravity. I. The canonical theory. Phys. Rev. 1967, 160, 1113–1148. [Google Scholar] [CrossRef]
- Banks, T. TCP, quantum gravity, the cosmological constant and all that... Nucl. Phys. B 1985, 249, 332–360. [Google Scholar] [CrossRef]
- Zeh, H.D. Emergence of classical time from a universal wavefunction. Phys. Lett. A 1986, 116, 9–12. [Google Scholar] [CrossRef]
- Unruh, W.G.; Wald, R.M. Time and the interpretation of canonical quantum gravity. Phys. Rev. D 1989, 40, 2598–2614. [Google Scholar] [CrossRef]
- Halliwell, J.J.; Pérez-Mercader, J.; Zurek, W.H. Physical Origins of Time Asymmetry; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Anderson, E. Problem of time in quantum gravity. Annalen Phys. 2012, 524, 757–786. [Google Scholar] [CrossRef] [Green Version]
- Kuchař, K.V. Time and interpretations of quantum gravity. Int. J. Mod. Phys. D 2011, 20, 3–86. [Google Scholar] [CrossRef]
- Isham, C.J. Canonical quantum gravity and the problem of time. In Integrable Systems, Quantum Groups, and Quantum Field Theories; Ibort, L.A., Rodríguez, M.A., Eds.; NATO ASI Series; Springer: Dordrecht, The Netherlands, 1993; pp. 157–287. [Google Scholar] [CrossRef]
- Kiefer, C. Conceptual issues in quantum cosmology. In Towards Quantum Gravity; Kowalski-Glikman, J., Ed.; Lecture notes in physics; Springer: Berlin/Heidelberg, Germany, 2000; pp. 158–187. [Google Scholar]
- Page, D.N.; Wootters, W.K. Evolution without evolution: Dynamics described by stationary observables. Phys. Rev. D 1983, 27, 2885–2892. [Google Scholar] [CrossRef]
- Wootters, W.K. “Time” replaced by quantum correlations. Int. J. Theor. Phys. 1984, 23, 701–711. [Google Scholar] [CrossRef]
- Dolby, C.E. The conditional probability interpretation of the Hamiltonian Constraint. arXiv, 2004; arXiv:gr-qc/0406034. [Google Scholar]
- Gambini, R.; Porto, R.A.; Pullin, J.; Torterolo, S. Conditional probabilities with Dirac observables and the problem of time in quantum gravity. Phys. Rev. D 2009, 79, 041501. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum time. Phys. Rev. D 2015, 92, 045033. [Google Scholar] [CrossRef] [Green Version]
- Marletto, C.; Vedral, V. Evolution without evolution and without ambiguities. Phys. Rev. D 2017, 95, 043510. [Google Scholar] [CrossRef] [Green Version]
- Moreva, E.; Brida, G.; Gramegna, M.; Giovannetti, V.; Maccone, L.; Genovese, M. Time from quantum entanglement: An experimental illustration. Phys. Rev. A 2014, 89, 052122. [Google Scholar] [CrossRef] [Green Version]
- Gambini, R.; Porto, R.A.; Pullin, J. A relational solution to the problem of time in quantum mechanics and quantum gravity: A fundamental mechanism for quantum decoherence. New J. Phys. 2004, 6, 45. [Google Scholar] [CrossRef]
- Bryan, K.L.H.; Medved, A.J.M. Realistic clocks for a Universe without time. Found. Phys. 2018, 48, 48–59. [Google Scholar] [CrossRef]
- Bryan, K.L.H.; Medved, A.J.M. Requiem for an ideal clock. arXiv, 2018; arXiv:1803.02045. [Google Scholar]
- Bryan, K.L.H.; Medved, A.J.M. The problem with “The problem of Time”. arXiv, 2018; arXiv:1811.09660. [Google Scholar]
- Smith, A.R.H.; Ahmadi, M. Quantizing time: Interacting clocks and systems. arXiv, 2017; arXiv:1712.00081. [Google Scholar]
- Berry, M.V. Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. Math. Phys. Sci. 1984, 392, 45–57. [Google Scholar] [CrossRef]
- Berry, M.V. Classical adiabatic angles and quantal adiabatic phase. J. Phys. A Math. Gen. 1985, 18, 15. [Google Scholar] [CrossRef]
- Kiefer, C. The semiclassical approximation to quantum gravity. In Canonical Gravity: From Classical to Quantum; Ehlers, J., Friedrich, H., Eds.; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1994; pp. 170–212. [Google Scholar]
- Halliwell, J.J.; Hawking, S.W. Origin of structure in the Universe. Phys. Rev. D 1985, 31, 1777–1791. [Google Scholar] [CrossRef]
- Balbinot, R.; Barletta, A.; Venturi, G. Matter, quantum gravity, and adiabatic phase. Phys. Rev. D 1990, 41, 1848–1854. [Google Scholar] [CrossRef]
- Datta, D. Semiclassical backreaction and berry’s phase. Mod. Phys. Lett. A 1993, 8, 191–196. [Google Scholar] [CrossRef]
- Kiefer, C. Quantum Gravity; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Brout, R.; Venturi, G. Time in semiclassical gravity. Phys. Rev. D 1989, 39, 2436–2439. [Google Scholar] [CrossRef]
- Bertoni, C.; Finelli, F.; Venturi, G. The Born-Oppenheimer approach to the matter—Gravity system and unitarity. Class. Quant. Grav. 1996, 13, 2375–2384. [Google Scholar] [CrossRef]
- Venturi, G. Minisuperspace, matter and time. Class. Quant. Grav. 1990, 7, 1075–1087. [Google Scholar] [CrossRef]
- Joos, E.; Zeh, H.D.; Kiefer, C.; Giulini, D.J.W.; Kupsch, J.; Stamatescu, I.O. Decoherence and the Appearance of a Classical World in Quantum Theory, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
| 1 | |
| 2 | For a function , for . The solution of this condition is . |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rotondo, M.; Nambu, Y. Clock Time in Quantum Cosmology. Universe 2019, 5, 66. https://doi.org/10.3390/universe5020066
Rotondo M, Nambu Y. Clock Time in Quantum Cosmology. Universe. 2019; 5(2):66. https://doi.org/10.3390/universe5020066
Chicago/Turabian StyleRotondo, Marcello, and Yasusada Nambu. 2019. "Clock Time in Quantum Cosmology" Universe 5, no. 2: 66. https://doi.org/10.3390/universe5020066
APA StyleRotondo, M., & Nambu, Y. (2019). Clock Time in Quantum Cosmology. Universe, 5(2), 66. https://doi.org/10.3390/universe5020066




