On Manifestation of In-Medium Effects in Neutron Stars and Heavy-Ion Collisions
Abstract
1. Introduction
2. EoS of Baryon-Rich Dense Not Too Hot Matter
2.1. Existing Constraints on EoS
- satisfy experimental information on properties of dilute nuclear matter and not contradict the results of microscopical approaches like the Brueckner–Hartree–Fock (BHF) one;
- place empirical constraints on global characteristics of atomic nuclei [1];
- allow for the heaviest known compact stars [4];
- allow for an adequate description of the compact star cooling in the absence of the direct Urca (DU) neutrino processes in the majority of the known pulsars detected in soft X rays [1];
- and yield a mass–radius relation comparable with the empirical constraints including recent gravitation wave LIGO–Virgo detection [5];
2.2. Cut-Mechanism for Stiffening of EoS
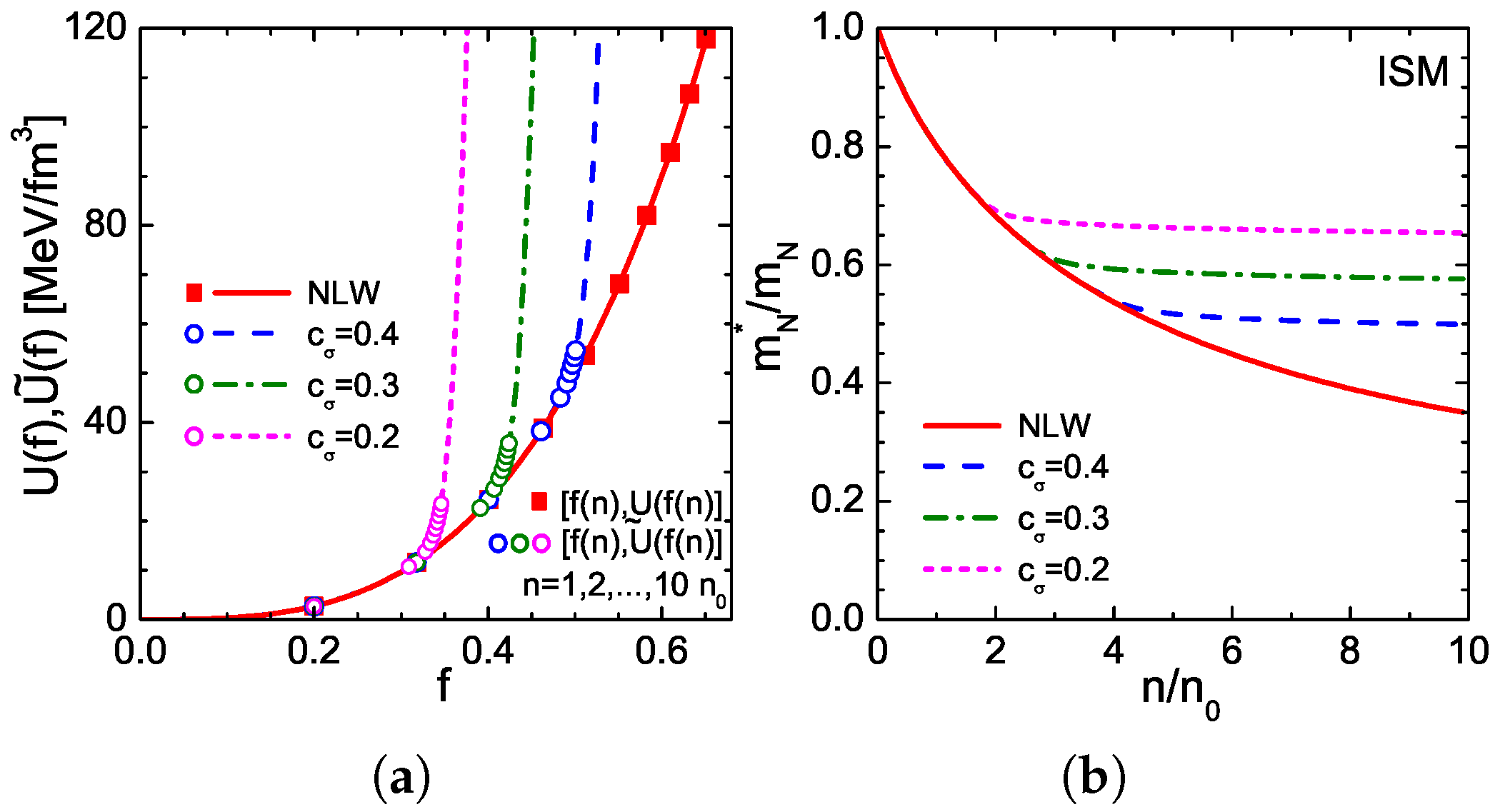
2.3. Hyperon and -Isobar Puzzles and Their Resolution in RMF Models with Scaled Hadron Masses and Couplings
2.4. Charged Condensation
3. Viscosity and Thermal Conductivity Effects at First-Order Phase Transitions in Heavy-Ion Collisions and Neutron Stars
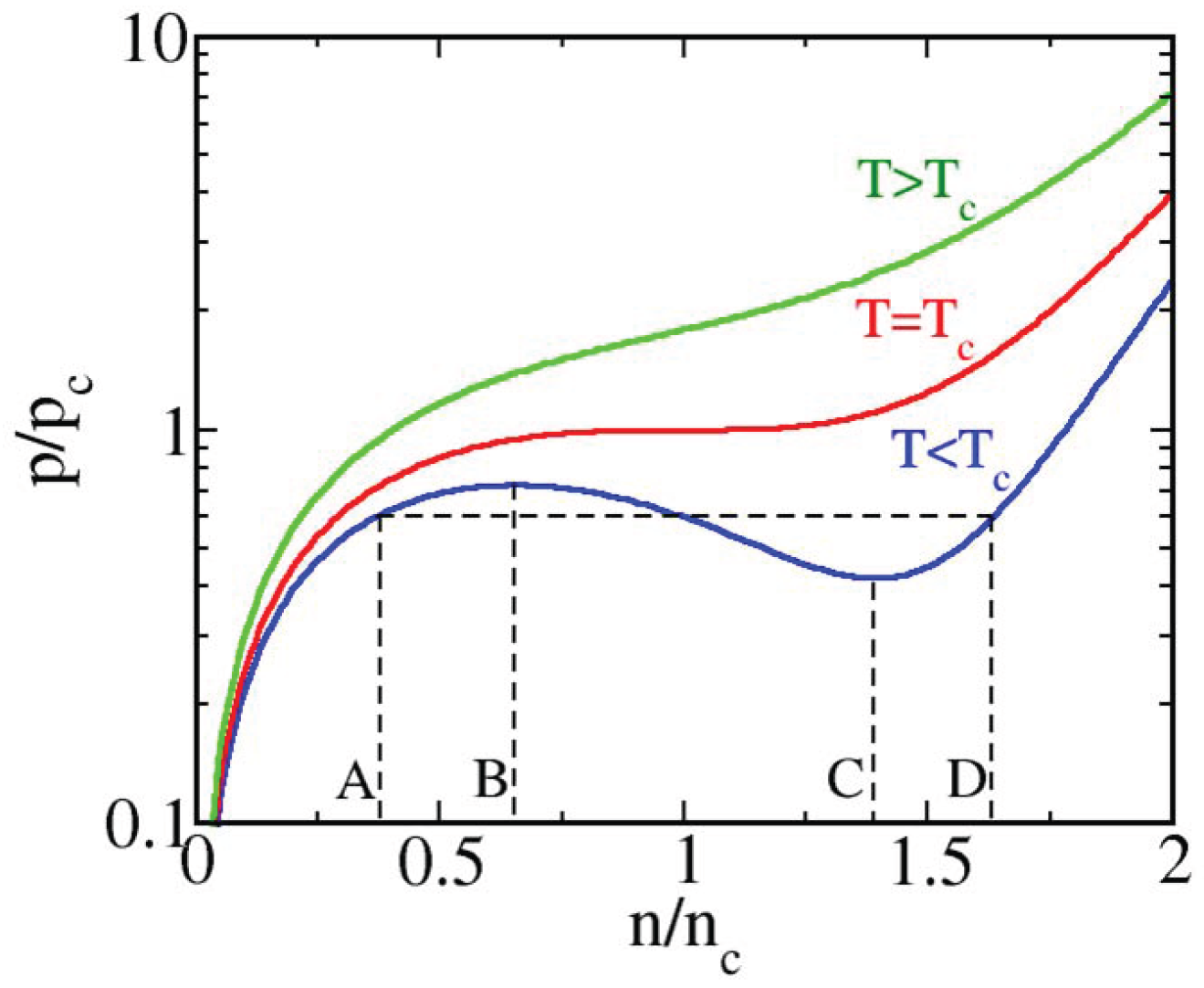
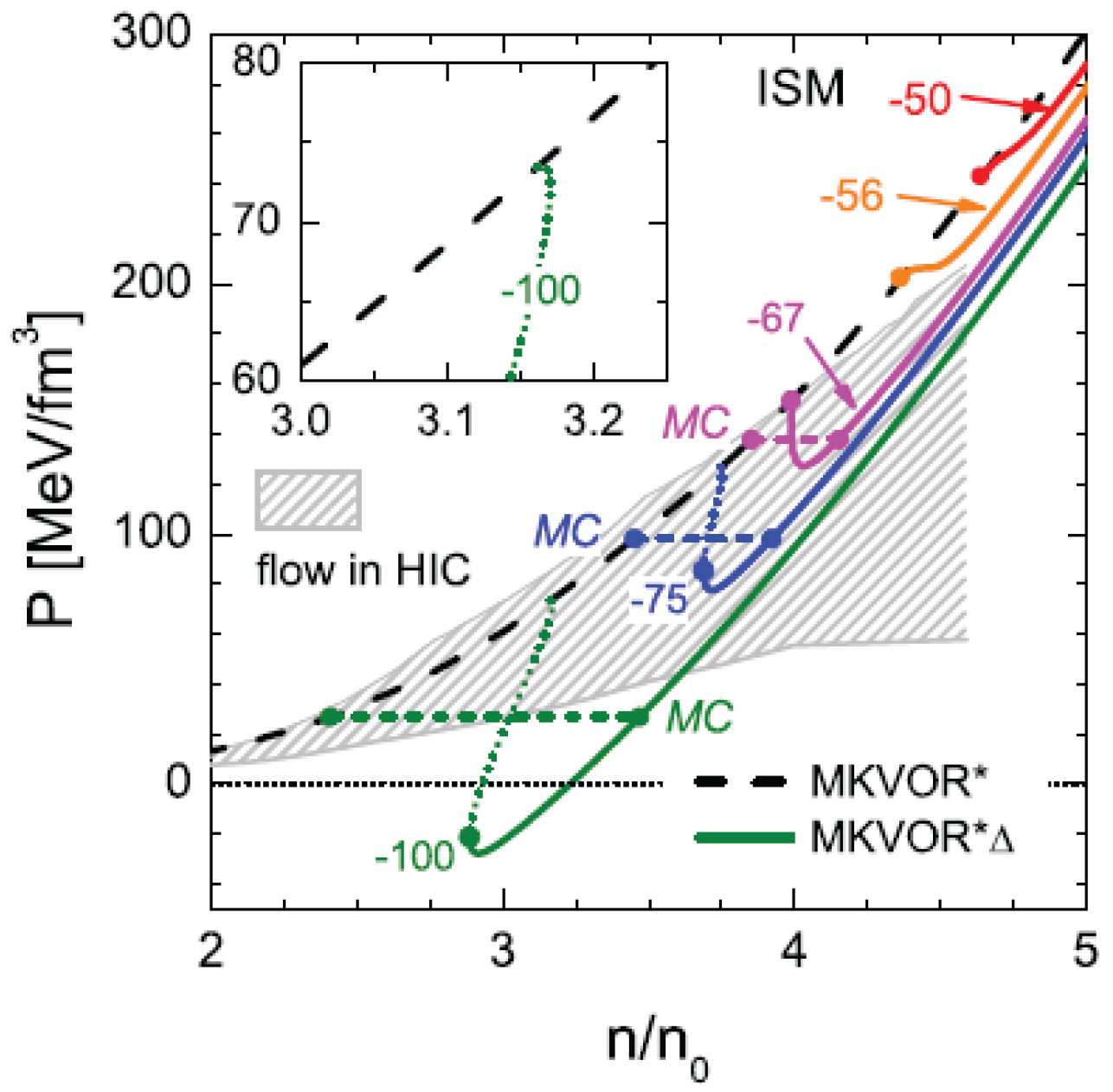
3.1. Pressure–Baryon Density Behavior at First-Order Phase Transitions
3.2. Hydrodynamical Description
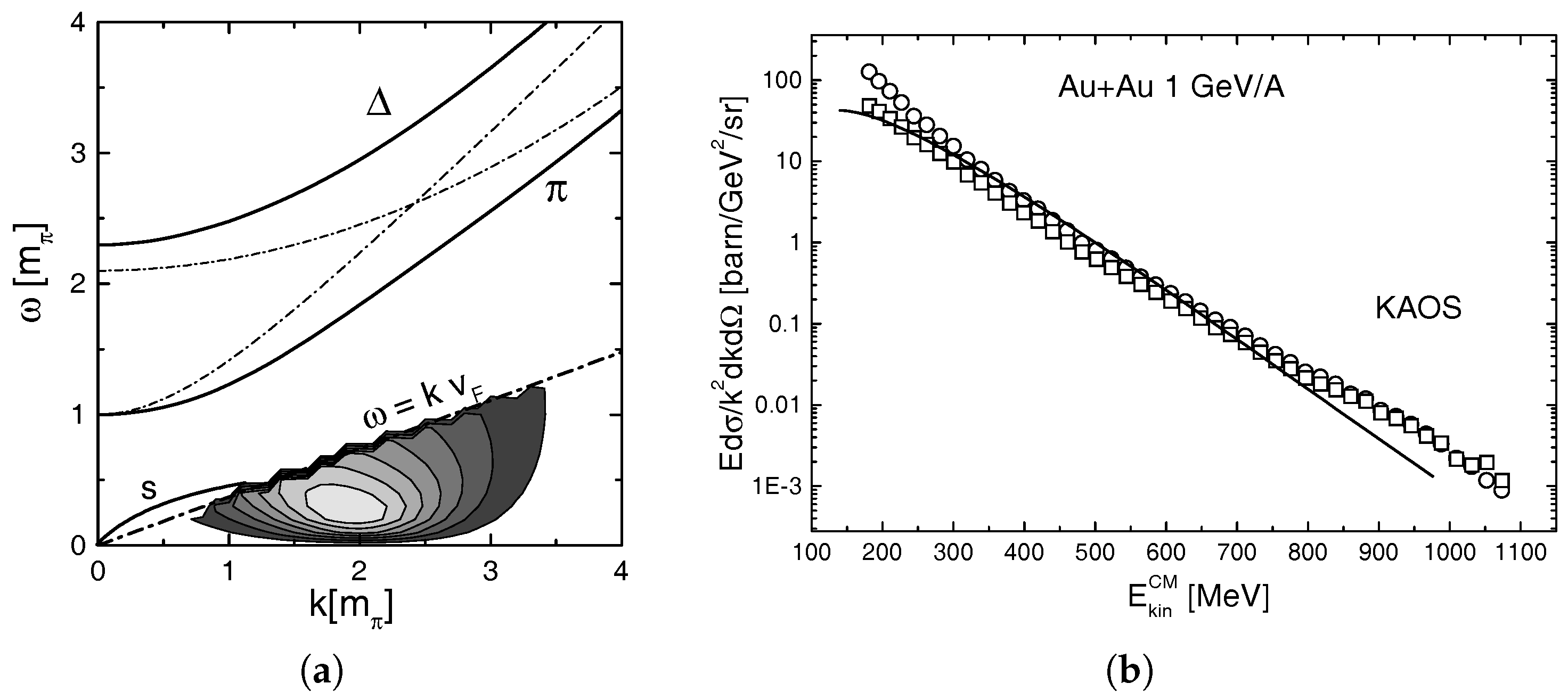
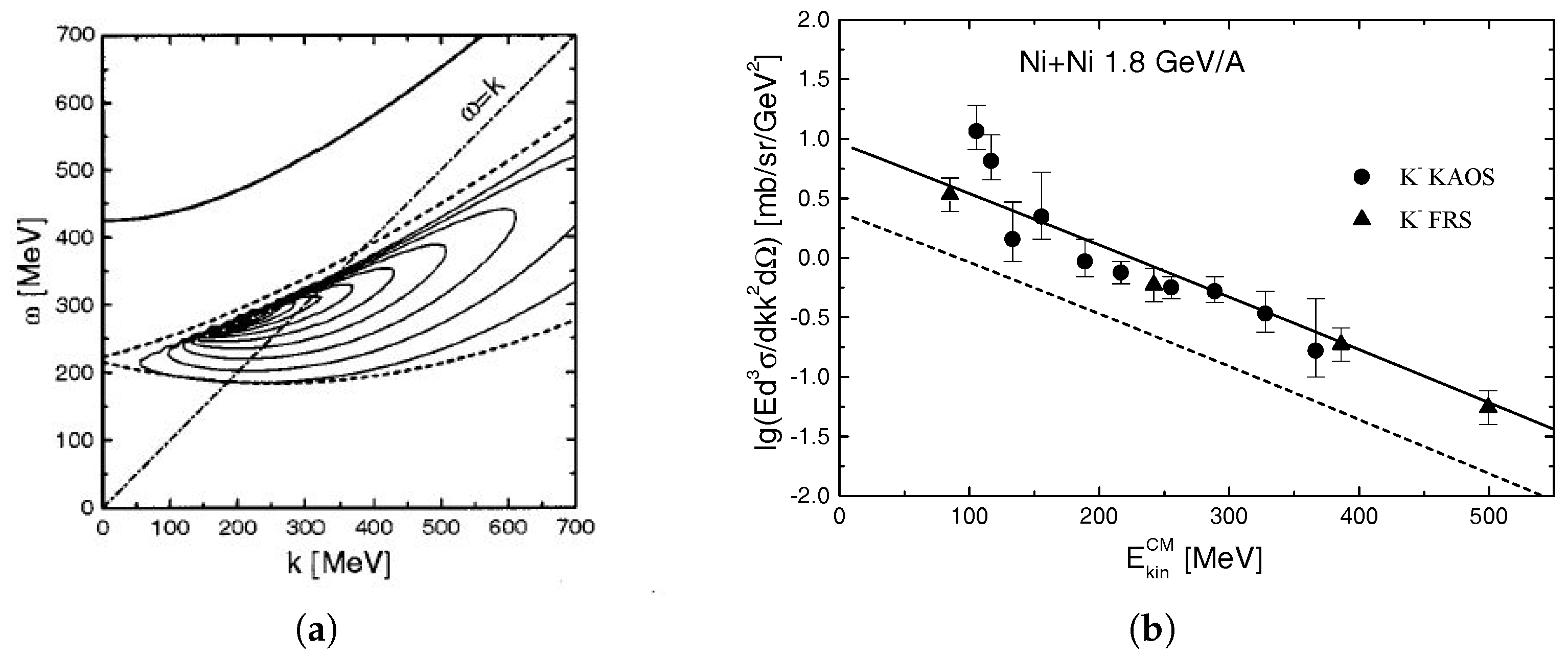
4. Pions and Kaons in Dense Baryon Matter
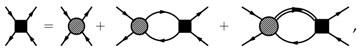
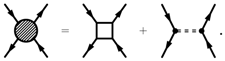




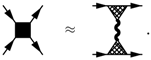
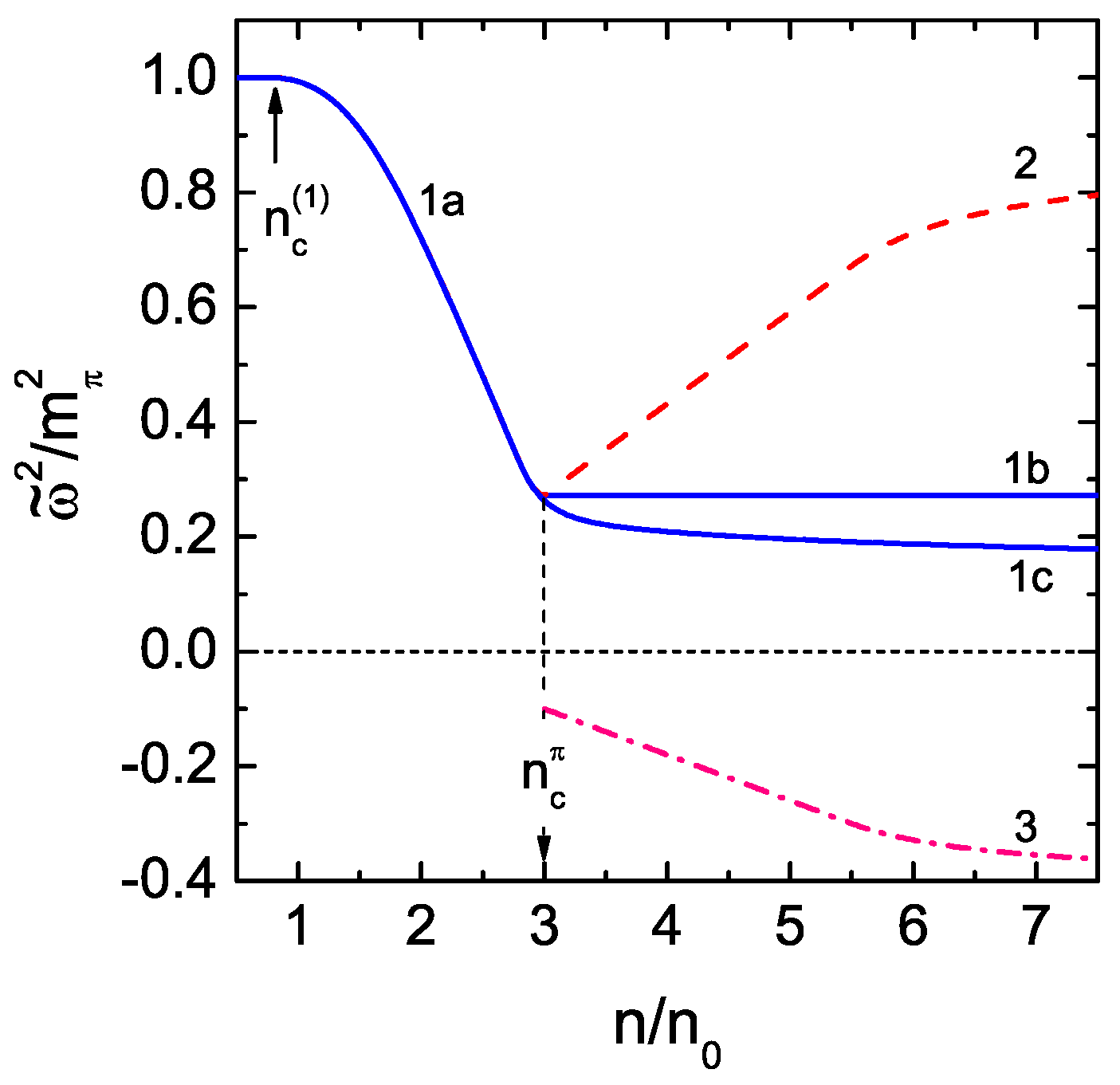
5. Pion Softening and Neutrino Emission from Neutron Stars
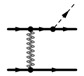

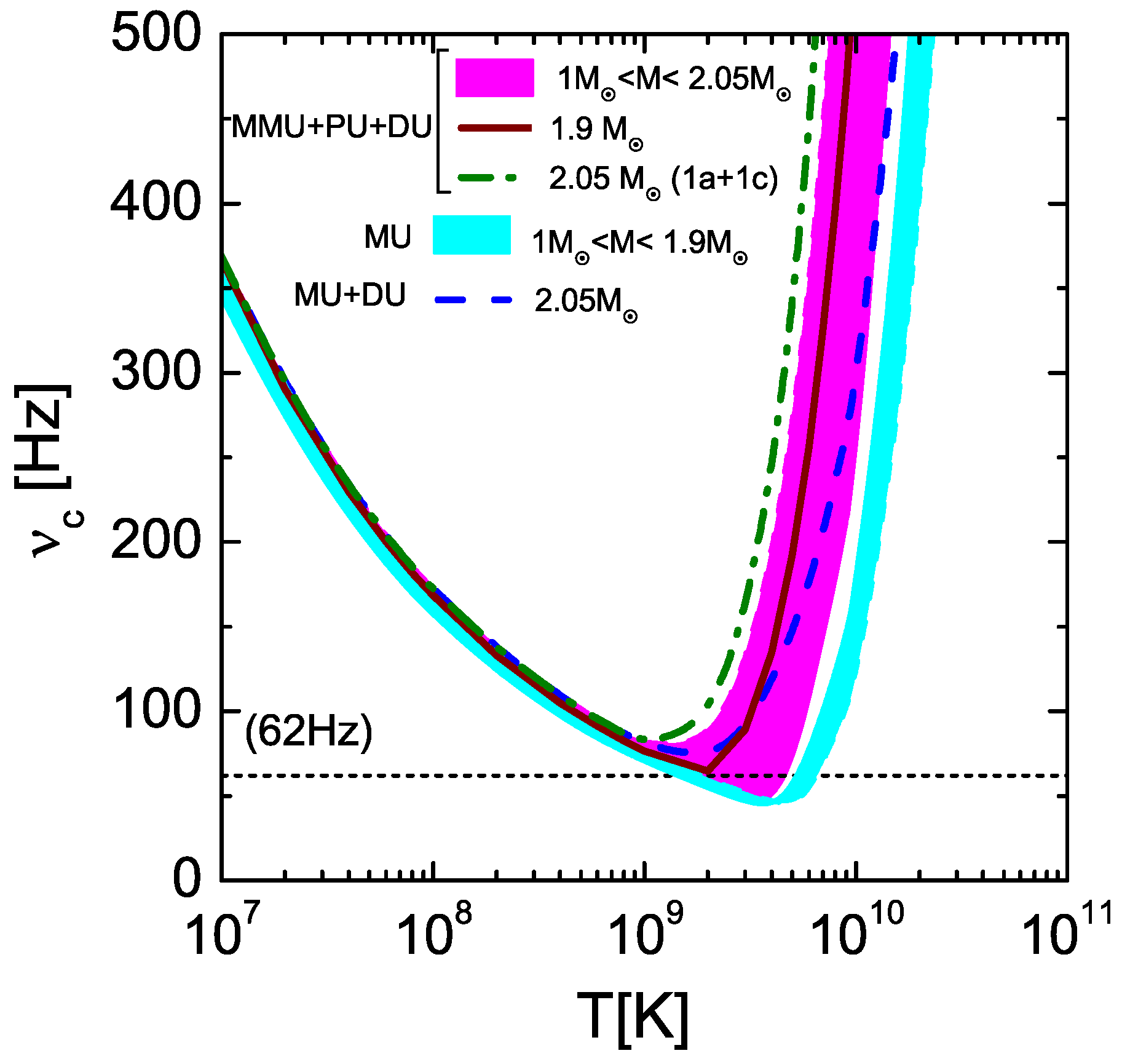
6. Viscosity of Neutron-Star Matter and r-Modes in Rotating Pulsars
7. Conclusions
Acknowledgments
Conflicts of Interest
Abbreviations
| EoS | Equation of state |
| BEM | Beta-equilibrium matter |
| ISM | Isospin-symmetric matter |
| RMF model | Relativistic mean-field model |
| NLW model | Nonlinear Walecka model |
| DU process | Direct Urca process |
| MU | modified Urca |
| MMU | medium modified Urca |
| PU | pion (condensate) Urca |
| NB | nucleon bremstrahlung |
| MNB | medium-modified nucleon bremstrahlung |
| PBF | Pair-breaking-formation |
| FOPE | Free one-pion exchange |
| MOPE | Medium one-pion exchange |
References
- Klähn, T.; Blaschke, D.; Typel, S.; Van Dalen, E.N.; Faessler, A.; Fuchs, C.; Gaitanos, T.; Grigorian, H.; Ho, A.; Kolomeitsev, E.E.; et al. Constraints on the high-density nuclear equation of state from the phenomenology of compact stars and heavy-ion collisions. Phys. Rev. C 2006, 74, 035802. [Google Scholar] [CrossRef]
- Danielewicz, P.; Lacey, R.; Lynch, W.G. Determination of the equation of state of dense matter. Science 2002, 298, 1592–1596. [Google Scholar] [CrossRef] [PubMed]
- Lynch, W.G.; Tsang, M.B.; Zhang, Y.; Danielewicz, P.; Famiano, M.; Li, Z.; Steiner, A.W. Probing the Symmetry Energy with Heavy Ions. Prog. Part. Nucl. Phys. 2009, 62, 427–432. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 1233232. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [PubMed]
- Khvorostukhin, A.S.; Toneev, V.D.; Voskresensky, D.N. Equation of State for Hot and Dense Matter: σ-ω-ρ Model with Scaled Hadron Masses and Couplings. Nucl. Phys. A 2007, 791, 180–221. [Google Scholar] [CrossRef]
- Khvorostukhin, A.S.; Toneev, V.D.; Voskresensky, D.N. Relativistic Mean-Field Model with Scaled Hadron Masses and Couplings. Nucl. Phys. A 2008, 813, 313–346. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Relativistic mean-field models with effective hadron masses and coupling constants, and rho- condensation. Nucl. Phys. A 2005, 759, 373–413. [Google Scholar] [CrossRef]
- Maslov, K.A.; Kolomeitsev, E.E.; Voskresensky, D.N. Solution of the Hyperon Puzzle within a Relativistic Mean-Field Model. Phys. Lett. B 2015, 748, 369–375. [Google Scholar] [CrossRef]
- Maslov, K.A.; Kolomeitsev, E.E.; Voskresensky, D.N. Relativistic Mean-Field Models with Scaled Hadron Masses and Couplings: Hyperons and Maximum Neutron Star Mass. Nucl. Phys. A 2016, 950, 64–109. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Maslov, K.A.; Voskresensky, D.N. Delta isobars in relativistic mean-field models with σ-scaled hadron masses and couplings. Nucl. Phys. A 2016, 961, 106–141. [Google Scholar] [CrossRef]
- Maslov, K.A.; Kolomeitsev, E.E.; Voskresensky, D.N. Making a soft relativistic mean-field equation of state stiffer at high density. Phys. Rev. C 2016, 92, 052801. [Google Scholar] [CrossRef]
- Voskresensky, D.N. Comments on manifestation of in-medium effects in heavy-ion collisions. Eur. Phys. J. A 2016, 52, 223. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Maslov, K.A.; Voskresensky, D.N. Charged ρ-meson condensation in neutron stars. Nucl. Phys. A 2018, 970, 291–315. [Google Scholar] [CrossRef]
- Schaffner-Bielich, J. Hypernuclear Physics for Neutron Stars. Nucl. Phys. A 2008, 804, 309–321. [Google Scholar] [CrossRef]
- Djapo, H.; Schaefer, B.J.; Wambach, J. On the appearance of hyperons in neutron stars. Phys. Rev. C 2010, 81, 035803. [Google Scholar]
- Drago, A.; Lavagno, A.; Pagliara, G.; Pigato, D. Early appearance of Δ isobars in neutron stars. Phys. Rev. C 2014, 90, 065809. [Google Scholar] [CrossRef]
- Drago, A.; Lavagno, A.; Pagliara, G.; Pigato, D. The scenario of two families of compact stars: Part 1. Equations of state, mass–radius relations and binary systems. Eur. Phys. J. A 2016, 52, 41. [Google Scholar] [CrossRef]
- Riek, F.; Lutz, M.F.M.; Korpa, C.L. Photoabsorption off nuclei with self consistent vertex corrections. Phys. Rev. C 2008, 80, 024902. [Google Scholar] [CrossRef]
- Voskresensky, D.N. On the possibility of the condensation of the charged rho meson field in dense isospin asymmetric baryon matter. Phys. Lett. B 1997, 392, 262–266. [Google Scholar] [CrossRef]
- Chernodub, M.N. Electromagnetic superconductivity of vacuum induced by strong magnetic field. Lect. Notes Phys. 2013, 871, 143–180. [Google Scholar]
- Mallick, R.; Schramm, S.; Dexheimer, V.; Bhattacharyya, A. On the possibility of rho-meson condensation in neutron stars. Mon. Not. R. Astron. Soc. 2015, 449, 1347–1351. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Senatorov, A.V.; Kampfer, B.; Haubold, H.J. A possible explanation of the second neutrino burst in SN1987A. Astrophys. Space Sci. 1987, 138, 421–424. [Google Scholar] [CrossRef]
- Haubold, H.J.; Kampfer, B.; Senatorov, A.V.; Voskresensky, D.N. A Tentative approach to the second neutrino burst in SN1987A. Astron. Astrophys. 1988, 191, L22–L24. [Google Scholar]
- Migdal, A.B.; Saperstein, E.E.; Troitsky, M.A.; Voskresensky, D.N. Pion degrees of freedom in nuclear matter. Phys. Rept. 1990, 192, 179–437. [Google Scholar] [CrossRef]
- Galeotti, P.; Pizzella, G. New analysis for the correlation between gravitational wave and neutrino detectors during SN1987A. Eur. Phys. J. C 2016, 76, 426. [Google Scholar] [CrossRef]
- Prakash, M.; Bombaci, I.; Prakash, M.; Ellis, P.J.; Lattimer, J.M.; Knorren, R. Composition and structure of protoneutron stars. Phys. Rept. 1997, 280, 1–77. [Google Scholar] [CrossRef]
- Skokov, V.V.; Voskresensky, D.N. Hydrodynamical description of a hadron–quark first-order phase transition. JETP Lett. 2009, 90, 223–227. [Google Scholar] [CrossRef]
- Skokov, V.V.; Voskresensky, D.N. Hydrodynamical description of first-order phase transitions: Analytical treatment and numerical modeling. Nucl. Phys. A 2009, 828, 401–438. [Google Scholar] [CrossRef]
- Skokov, V.V.; Voskresensky, D.N. Thermal conductivity in dynamics of first-order phase transition. Nucl. Phys. A 2010, 847, 253–267. [Google Scholar] [CrossRef]
- Skokov, V.V.; Voskresensky, D.N. Viscosity and thermal conductivity effects at first-order phase transitions in heavy-ion collisions. Phys. Atom. Nucl. 2012, 75, 770–775. [Google Scholar]
- Randrup, J. Phase transition dynamics for baryon-dense matter. Phys. Rev. C 2009, 79, 054911. [Google Scholar] [CrossRef]
- Steinheimer, J.; Randrup, J. Spinodal density enhancements in simulations of relativistic nuclear collisions. Phys. Rev. C 2013, 87, 054903. [Google Scholar] [CrossRef]
- Romatschke, P.; Romatschke, U. Viscosity Information from Relativistic Nuclear Collisions: How Perfect is the Fluid Observed at RHIC? Phys. Rev. Lett. 2007, 99, 172301. [Google Scholar] [CrossRef] [PubMed]
- Voskresensky, D.N. Quasiclassical description of condensed systems by a complex order parameter. Phys. Scr. 1993, 47, 333–354. [Google Scholar] [CrossRef]
- Glendenning, N.K. First order phase transitions with more than one conserved charge: Consequences for neutron stars. Phys. Rev. D 1992, 46, 1274–1287. [Google Scholar] [CrossRef]
- Heiselberg, H.; Pethick, C.J.; Staubo, E.F. Quark matter droplets in neutron stars. Phys. Rev. Lett. 1993, 70, 1355–1359. [Google Scholar] [CrossRef] [PubMed]
- Voskresensky, D.N.; Yasuhira, M.; Tatsumi, T. Charge screening at first order phase transitions. Phys. Lett. B 2002, 541, 93–100. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Yasuhira, M.; Tatsumi, T. Charge screening at first order phase transitions and hadron quark mixed phase. Nucl. Phys. A 2003, 723, 291–339. [Google Scholar] [CrossRef]
- Maruyama, T.; Tatsumi, T.; Voskresensky, D.N.; Tanigawa, T.; Endo, T.; Chiba, S. Finite size effects on kaonic pasta structures. Phys. Rev. C 2006, 73, 035802. [Google Scholar] [CrossRef]
- Maruyama, T.; Tatsumi, T.; Voskresensky, D.N.; Tanigawa, T.; Chiba, S. Nuclear pasta structures and the charge screening effect. Phys. Rev. C 2005, 72, 015802. [Google Scholar] [CrossRef]
- Voskresensky, D.N. Many particle effects in nucleus-nucleus collisions. Nucl. Phys. A 1993, 555, 293–328. [Google Scholar] [CrossRef]
- Migdal, A.B. Pion Fields in Nuclear Matter. Rev. Mod. Phys. 1978, 50, 107–172. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Mishustin, I.N. Polarization operator of pions at finite temperatures. Yad. Fiz. 1982, 35, 1139–1156. [Google Scholar]
- Kolomeitsev, E.E.; Voskresensky, D.N. Superfluid nucleon matter in and out of equilibrium and weak interactions. Phys. Atom. Nucl. 2011, 74, 1316–1363. [Google Scholar] [CrossRef]
- Roepke, G.; Voskresensky, D.; Kryukov, I.; Blaschke, D. Fermi liquid, clustering, and structure factor in dilute warm nuclear matter. Nucl. Phys. A 2018, 970, 224–258. [Google Scholar] [CrossRef]
- Migdal, A.B. Theory of Finite Fermi Systems and Properties of Atomic Nuclei, Wiley and Sons: New York, NY, USA, 1967, 2nd ed.; Nauka: Moscow, Russia, 1983. [Google Scholar]
- Dyugaev, A.M. Crystalline And Liquid Phases Of A Pion Condensate. Pisma Zh. Eksp. Teor. Fiz. 1982, 35, 341–344. [Google Scholar]
- Kolomeitsev, E.E.; Voskresensky, D.N. Meson particle hole dynamics. arXiv, 2000; arXiv:nucl-th/0001062. [Google Scholar]
- Wagner, A.; Muntz, C.; Oeschler, H.; Sturm, C.; Barth, R.; Cieslak, M.; Debowski, M.; Grosse, E.; Koczon, P.; Mang, M.; et al. Evidence for different freezeout radii of high-energy and low-energy pions emitted in Au + Au collisions at 1-A/GeV. Phys. Lett. B 1998, 420, 20–24. [Google Scholar] [CrossRef]
- Akmal, A.; Pandharipande, V.R.; Ravenhall, D.G. The Equation of state of nucleon matter and neutron star structure. Phys. Rev. C 1998, 58, 1804–1828. [Google Scholar] [CrossRef]
- Senatorov, A.V.; Voskresensky, D.N. Pion Dynamics in Heavy Ion Collisions. Phys. Lett. B 1989, 219, 31–34. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Blaschke, D.; Roepke, G.; Schulz, H. Nonequilibrium approach to dense hadronic matter. Int. J. Mod. Phys. E 1995, 4, 1–45. [Google Scholar] [CrossRef]
- Voskresensky, D.N. Hadron liquid with a small baryon chemical potential at finite temperature. Nucl. Phys. A 2004, 744, 378–444. [Google Scholar] [CrossRef]
- Voskresensky, D.N. Thermodynamics of resonances and blurred particles. Nucl. Phys. A 2008, 812, 158–185. [Google Scholar] [CrossRef]
- Dyugaev, A.M. Nature of Phase Transition in Case of pi- Condensation. Pisma Zh. Eksp. Teor. Fiz. 1975, 22, 181–185. [Google Scholar]
- Kolomeitsev, E.E.; Kampfer, B.; Voskresensky, D.N. Kaon polarization in nuclear matter. Nucl. Phys. A 1995, 588, 889–917. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Negative kaons in dense baryonic matter. Phys. Rev. C 2003, 68, 015803. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Kampfer, B.; Voskresensky, D.N. The impact of kaon polarization in nuclear matter on the K- production in heavy ion collisions. Int. J. Mod. Phys. E 1996, 5, 313–328. [Google Scholar] [CrossRef]
- Barth, R.; Senger, P.; Ahner, W.; Beckerle, P.; Bormann, C.; Brill, D.; Cieślak, M.; Grosse, E.; Koczoń, P.; Kohlmeyer, B.; et al. Subthreshold production of kaons and anti-kaons in nucleus-nucleus collisions at equivalent beam energies. Phys. Rev. Lett. 1997, 78, 4007–4010. [Google Scholar] [CrossRef]
- Schröter, A.; Berdermann, E.; Geissel, H.; Gillitzer, A.; Homolka, J.; Kienle, P.; Koenig, W.; Povh, B.; Schumacher, F.; Ströher, H. Subthreshold anti-proton and K- production in heavy ion collisions. Z. Phys. A Hadron. Nucl. 1994, 350, 101–113. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Senatorov, A.V. Pion Excitations In A Nucleonic Medium May Be Pertinent To The Luminosity Of Neutron Stars. Pisma Zh. Eksp. Teor. Fiz. 1984, 40, 395–398. [Google Scholar]
- Voskresensky, D.N.; Senatorov, A.V. Emission of Neutrinos by Neutron Stars. Zh. Eksp. Teor. Fiz. 1986, 90, 1505–1526. [Google Scholar]
- Senatorov, A.V.; Voskresensky, D.N. Collective Excitations in Nucleonic Matter and the Problem of Cooling of Neutron Stars. Phys. Lett. B 1987, 184, 119–124. [Google Scholar] [CrossRef]
- Voskresensky, D.N.; Senatorov, A.V. Description of Nuclear Interaction in Keldysh’s Diagram Technique and Neutrino Luminosity of Neutron Stars. Yad. Fiz. 1987, 45, 657–669. (In Russian) [Google Scholar]
- Voskresensky, D.N. Neutrino cooling of neutron stars: Medium effects. In Physics of Neutron Star Interiors; Springer: Berlin/Heidelberg, Germany, 2001; pp. 467–503. [Google Scholar]
- Blaschke, D.; Grigorian, H.; Voskresensky, D.N. Cooling of neutron stars: Hadronic model. Astron. Astrophys. 2004, 424, 979–992. [Google Scholar] [CrossRef]
- Tsuruta, S. Thermal properties and detectability of neutron stars. I. Cooling and heating of neutron stars. Phys. Rep. 1979, 56, 237–277. [Google Scholar] [CrossRef]
- Shapiro, S.; Teukolsky, S.A. Black Holes, White Dwarfs and Neutron Stars: The Physics of Compact Objects; Wiley: New York, NY, USA, 1983; Chapter 11. [Google Scholar]
- Page, D.; Lattimer, J.M.; Prakash, M.; Steiner, A.W. Minimal cooling of neutron stars: A New paradigm. Astrophys. J. Suppl. Ser. 2004, 155, 623–650. [Google Scholar] [CrossRef]
- Friman, B.L.; Maxwell, O.V. Neutron Star Neutrino Emissivities. Astrophys. J. 1979, 232, 541–557. [Google Scholar] [CrossRef]
- Demorest, P.; Pennucci, T.; Ransom, S.; Roberts, M.; Hessels, J. Shapiro Delay Measurement of A Two Solar Mass Neutron Star. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef] [PubMed]
- Fonseca, E.; Pennucci, T.T.; Ellis, J.A.; Stairs, I.H.; Nice, D.J.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Crowter, K.; Dolch, T.; et al. The NANOGrav Nine-year Data Set: Mass and Geometric Measurements of Binary Millisecond Pulsars. Astrophys. J. 2016, 832, 167. [Google Scholar] [CrossRef]
- Kramer, M.; Stairs, I.H.; Manchester, R.N.; McLaughlin, M.A.; Lyne, A.G.; Ferdman, R.D.; Burgay, M.; Lorimer, D.R.; Possenti, A.; D’amico, N.; et al. Tests of general relativity from timing the double pulsar. Science 2006, 314, 97–102. [Google Scholar] [CrossRef] [PubMed]
- Faulkner, A.J.; Kramer, M.; Lyne, A.G.; Manchester, R.N.; McLaughlin, M.A.; Stairs, I.H.; Hobbs, G.; Possenti, A.; Lorimer, D.R.; D’Amico, N.; et al. PSR J1756-2251: A New relativistic double neutron star system. Astrophys. J. 2004, 618, L119–L122. [Google Scholar] [CrossRef]
- Ferdman, R.D.; Stairs, I.H.; Kramer, M.; Janssen, G.H.; Bassa, C.G.; Stappers, B.W.; Demorest, P.B.; Cognard, I.; Desvignes, G.; Theureau, G.; et al. PSR J1756-2251: A pulsar with a low-mass neutron star companion. Mon. Not. R. Astron. Soc. 2014, 443, 2183–2196. [Google Scholar] [CrossRef]
- Flowers, E.; Ruderman, M.; Sutherland, P. Neutrino pair emission from finite-temperature neutron superfluid and the cooling of young neutron stars. Astrophys. J. 1976, 205, 541–544. [Google Scholar] [CrossRef]
- Yakovlev, D.G.; Kaminker, A.D.; Gnedin, O.Y.; Haensel, P. Neutrino emission from neutron stars. Phys. Rep. 2001, 354, 1–55. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Neutrino emission due to Cooper-pair recombination in neutron stars revisited. Phys. Rev. C 2008, 77, 065808. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Neutral weak currents in nucleon superfluid Fermi liquids: Larkin-Migdal and Leggett approaches. Phys. Rev. C 2010, 81, 065801. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Spin excitonic and diffusive modes in superfluid Fermi liquids. Phys. Rev. C 2011, 84, 068801. [Google Scholar] [CrossRef]
- Schaab, C.; Voskresensky, D.; Sedrakian, A.D.; Weber, F.; Weigel, M.K. Impact of medium effects on the cooling of nonsuperfluid and superfluid neutron stars. Astron. Astrophys. 1997, 321, 591–604. [Google Scholar]
- Grigorian, H.; Voskresensky, D.N. Medium effects in cooling of neutron stars and 3P(2) neutron gap. Astron. Astrophys. 2005, 444, 913–929. [Google Scholar] [CrossRef]
- Blaschke, D.; Grigorian, H.; Voskresensky, D.N.; Weber, F. On the Cooling of the Neutron Star in Cassiopeia A. Phys. Rev. C 2012, 85, 022802. [Google Scholar] [CrossRef]
- Blaschke, D.; Grigorian, H.; Voskresensky, D.N. Nuclear medium cooling scenario in the light of new Cas A cooling data and the 2M⊙ pulsar mass measurements. Phys. Rev. C 2013, 88, 065805. [Google Scholar]
- Grigorian, H.; Voskresensky, D.N.; Blaschke, D. Influence of the stiffness of the equation of state and in-medium effects on the cooling of compact stars. Eur. Phys. J. A 2016, 52, 1–19. [Google Scholar] [CrossRef]
- Grigorian, H.; Kolomeitsev, E.E.; Maslov, K.A.; Voskresensky, D.N. On cooling of neutron stars with stiff equation of state including hyperons. arXiv, 2017; arXiv:1801.00040. [Google Scholar]
- Andersson, N. A New class of unstable modes of rotating relativistic stars. Astrophys. J. 1998, 502, 708–713. [Google Scholar] [CrossRef]
- Friedman, J.L.; Morsink, S.M. Axial instability of rotating relativistic stars. Astrophys. J. 1998, 502, 714–720. [Google Scholar] [CrossRef]
- Lindblom, L.; Owen, B.J.; Morsink, S.M. Gravitational radiation instability in hot young neutron stars. Phys. Rev. Lett. 1998, 80, 4843–4846. [Google Scholar] [CrossRef]
- Andersson, N.; Kokkotas, K.D. The R mode instability in rotating neutron stars. Int. J. Mod. Phys. D 2001, 10, 381–441. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Mechanism of r-mode stability in young rapidly rotating pulsars. Eur. Phys. J. A 2014, 50, 180. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Voskresensky, D.N. Viscosity of neutron star matter and r-modes in rotating pulsars. Phys. Rev. C 2015, 91, 025805. [Google Scholar] [CrossRef]
- Shternin, P.S.; Yakovlev, D.G. Electron-muon heat conduction in neutron star cores via the exchange of transverse plasmons. Phys. Rev. D 2007, 75, 103004. [Google Scholar] [CrossRef]
| 1. | In order to avoid misunderstanding, by the pion condensation we understand the liquid-crystal-like or solid-like phases (of an inhomogeneous condensate) rather than a liquid phase mentioned above. |





© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Voskresensky, D.N. On Manifestation of In-Medium Effects in Neutron Stars and Heavy-Ion Collisions. Universe 2018, 4, 28. https://doi.org/10.3390/universe4020028
Voskresensky DN. On Manifestation of In-Medium Effects in Neutron Stars and Heavy-Ion Collisions. Universe. 2018; 4(2):28. https://doi.org/10.3390/universe4020028
Chicago/Turabian StyleVoskresensky, Dmitry N. 2018. "On Manifestation of In-Medium Effects in Neutron Stars and Heavy-Ion Collisions" Universe 4, no. 2: 28. https://doi.org/10.3390/universe4020028
APA StyleVoskresensky, D. N. (2018). On Manifestation of In-Medium Effects in Neutron Stars and Heavy-Ion Collisions. Universe, 4(2), 28. https://doi.org/10.3390/universe4020028



