Period Variation Rates of Four Radial Single-Mode High-Amplitude Delta Scuti Stars
Abstract
1. Introduction
2. Data Sources and Data Reduction
3. Analysis
3.1. Analysis of XX Cyg
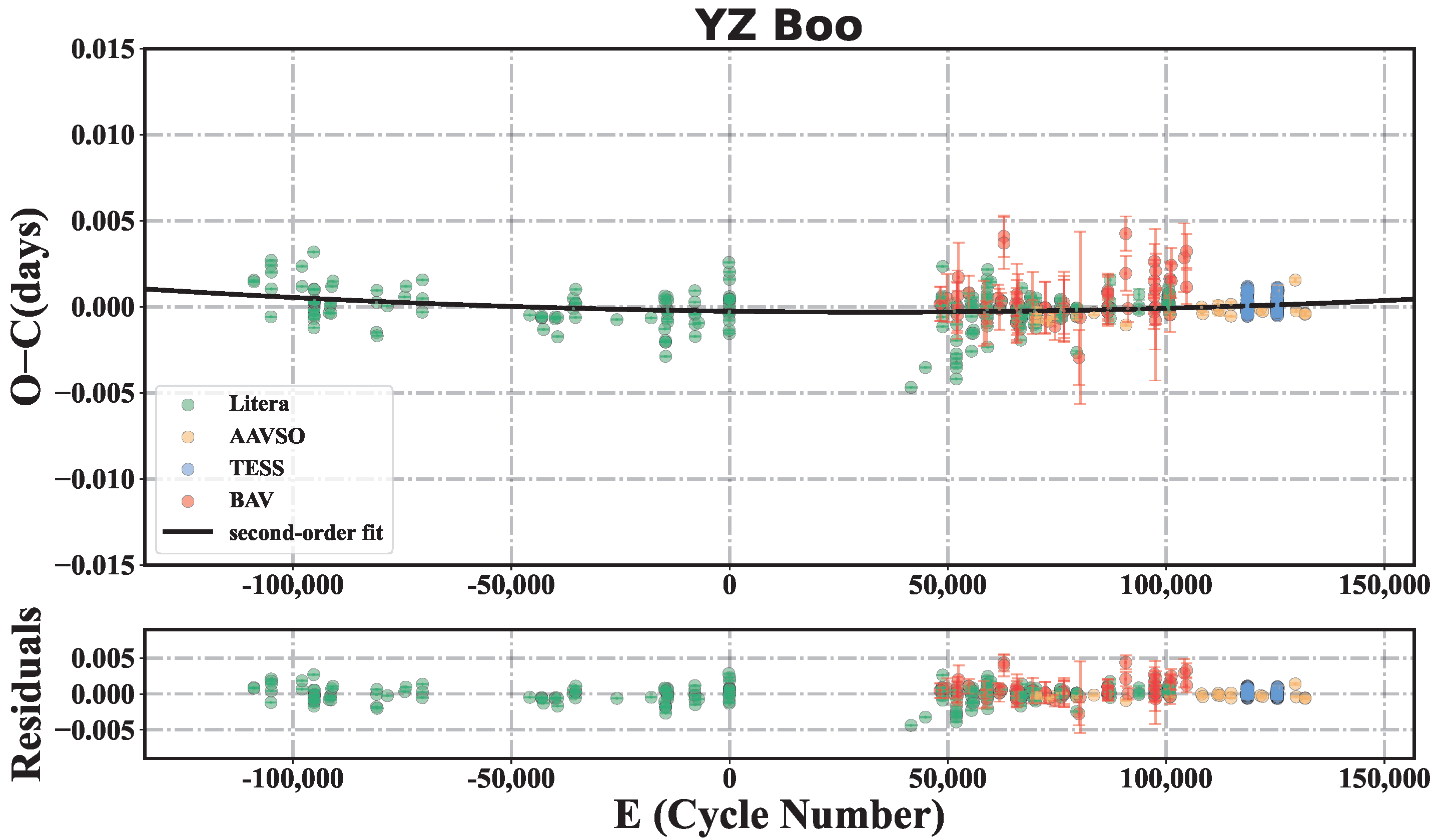
3.2. Analysis of YZ Boo
3.3. Analysis of GP And
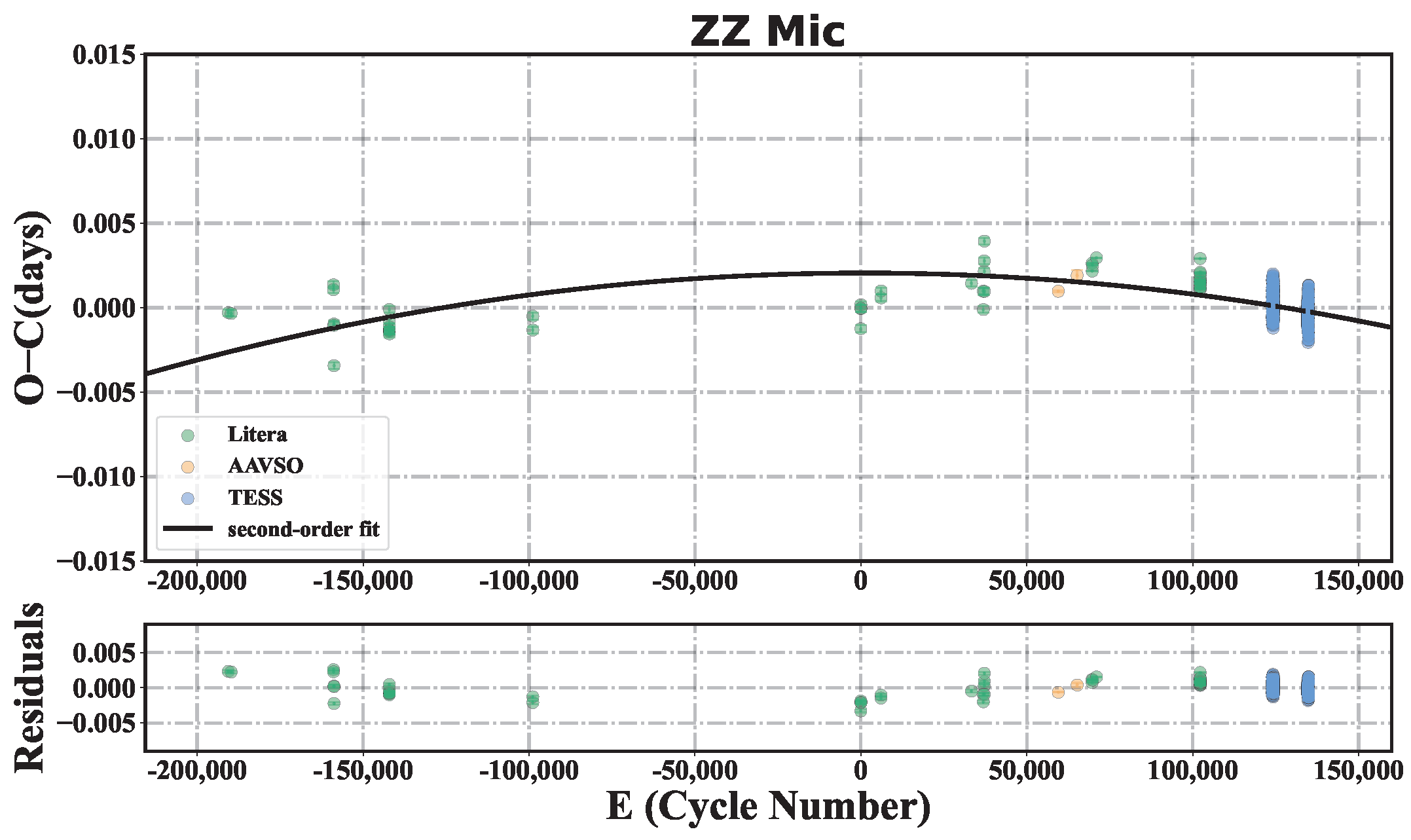
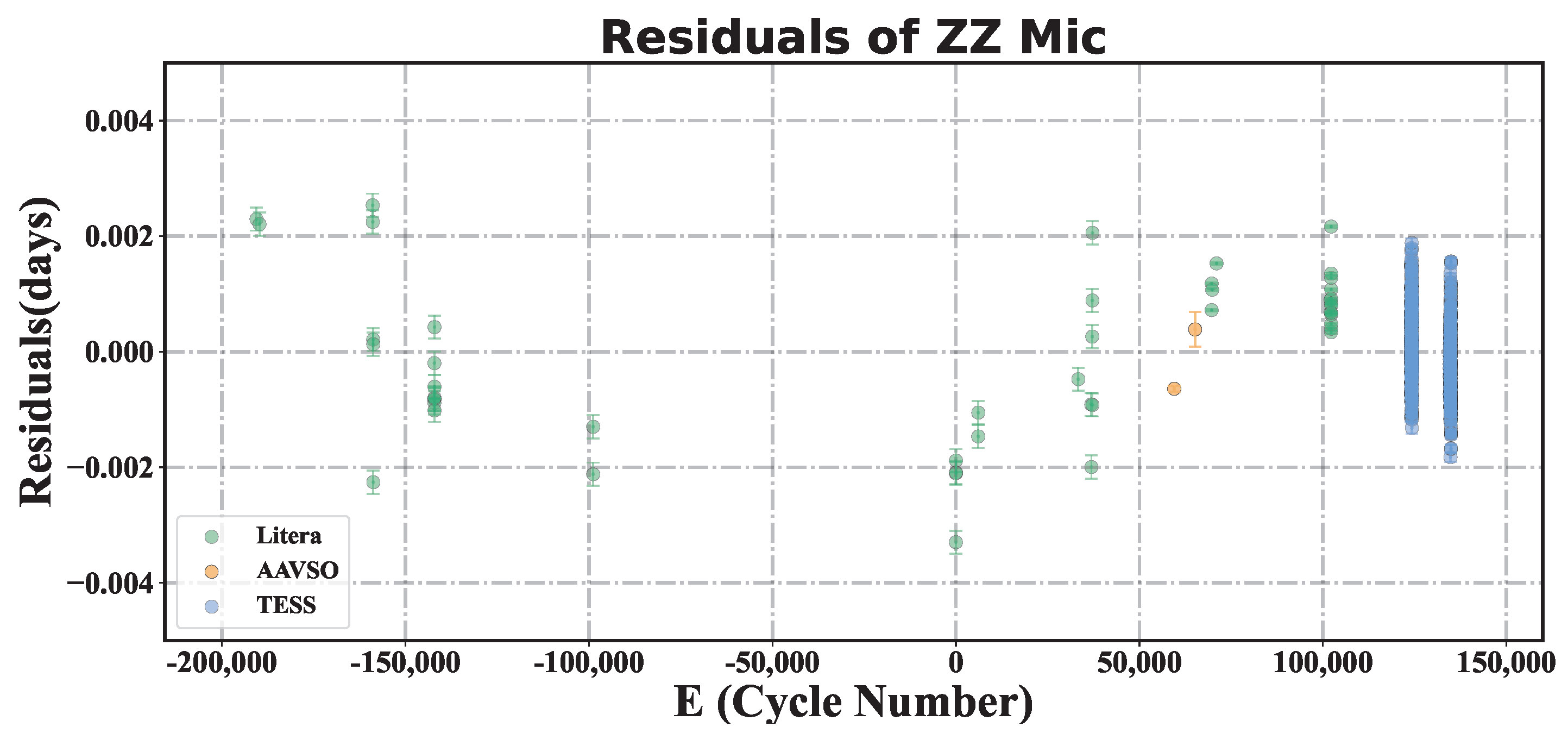
3.4. Analysis of ZZ Mic
4. Discussion
4.1. Discussion of XX Cyg
4.2. Discussion of YZ Boo
4.3. Discussion of GP And
4.4. Discussion of ZZ Mic
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Times of Maximum Light Used in This Work
| 1 | https://mast.stsci.edu/portal/Mashup/Clients/Mast/Portal.html, accessed on 1 May 2024 |
| 2 | https://www.aavso.org/, accessed on 1 June 2024 |
| 3 | https://www.bav-astro.eu/index.php, accessed on 1 July 2024 |
| 4 | https://doncarona.tamu.edu/apps/jd/, accessed on 15 June 2024 |
| 5 | https://astroutils.astronomy.osu.edu/time/hjd2bjd.html, accessed on 15 July 2024 |
| 6 | https://www.statology.org/f-test-python/, accessed on 15 September 2024 |
References
- Netzel, H.; Smolec, R. Modelling of multimode radially pulsating high-amplitude δ Scuti stars from the OGLE Galactic bulge sample. Mon. Not. R. Astron. Soc. 2022, 515, 4574–4586. [Google Scholar] [CrossRef]
- Breger, M. δ Scuti stars (Review). In Astronomical Society of the Pacific Conference Series, Proceedings of the Delta Scuti and Related Stars, Vienna, Austria, 4–7 August 1999; Breger, M., Montgomery, M., Eds.; BiblioGov: Ottawa, ON, Canada, 2000; Volume 210, p. 3. [Google Scholar]
- Wils, P.; Rozakis, I.; Kleidis, S.; Hambsch, F.J.; Bernhard, K. Photometry of GSC 762-110, a new triple-mode radially pulsating star. Astron. Astrophys. 2008, 478, 865–868. [Google Scholar] [CrossRef]
- Niu, J.S.; Fu, J.N.; Zong, W.K. Pulsation analysis of the high amplitude δ Scuti star CW Serpentis. Res. Astron. Astrophys. 2013, 13, 1181–1188. [Google Scholar] [CrossRef][Green Version]
- Niu, J.S.; Fu, J.N.; Li, Y.; Yang, X.H.; Zong, W.; Xue, H.F.; Zhang, Y.P.; Liu, N.; Du, B.; Zuo, F. AE Ursae Majoris—A δ Scuti star in the Hertzsprung Gap. Mon. Not. R. Astron. Soc. 2017, 467, 3122–3139. [Google Scholar] [CrossRef]
- Xue, H.F.; Fu, J.N.; Fox-Machado, L.; Shi, J.R.; Zhou, Y.T.; Zhang, J.B.; Michel, R.; Yan, H.L.; Niu, J.S.; Zong, W.K.; et al. Asteroseismology of the Double-mode High-amplitude δ Scuti Star VX Hydrae. Astrophys. J. 2018, 861, 96. [Google Scholar] [CrossRef]
- Xue, H.F.; Niu, J.S. DY Pegasi: An SX Phoenicis Star in a Binary System with an Evolved Companion. Astrophys. J. 2020, 904, 5. [Google Scholar] [CrossRef]
- Daszyńska-Daszkiewicz, J.; Walczak, P.; Pamyatnykh, A.A.; Szewczuk, W. Asteroseismology of the double-radial mode δ Scuti star BP Pegasi. Mon. Not. R. Astron. Soc. 2022, 512, 3551–3565. [Google Scholar] [CrossRef]
- Xue, W.; Niu, J.S.; Xue, H.F.; Yin, S. Pulsation Analysis of High-Amplitude δ Scuti Stars with TESS. Res. Astron. Astrophys. 2023, 23, 075002. [Google Scholar] [CrossRef]
- Breger, M.; Pamyatnykh, A.A. Period changes of delta Scuti stars and stellar evolution. Astron. Astrophys. 1998, 332, 958–968. [Google Scholar] [CrossRef]
- Bowman, D.M.; Kurtz, D.W.; Breger, M.; Murphy, S.J.; Holdsworth, D.L. Amplitude modulation in δ Sct stars: Statistics from an ensemble study of Kepler targets. Mon. Not. R. Astron. Soc. 2016, 460, 1970–1989. [Google Scholar] [CrossRef]
- Bowman, D.M.; Hermans, J.; Daszyńska-Daszkiewicz, J.; Holdsworth, D.L.; Tkachenko, A.; Murphy, S.J.; Smalley, B.; Kurtz, D.W. KIC 5950759: A high-amplitude δ Sct star with amplitude and frequency modulation near the terminal age main sequence. Mon. Not. R. Astron. Soc. 2021, 504, 4039–4053. [Google Scholar] [CrossRef]
- Niu, J.S.; Xue, H.F. A Rapidly Evolving High-amplitude δ Scuti Star Crossing the Hertzsprung Gap. Astrophys. J. Lett. 2022, 938, L20. [Google Scholar] [CrossRef]
- Xue, H.F.; Niu, J.S.; Fu, J.N. Precise Evolutionary Asteroseismology of High-Amplitude δ Scuti Star AE Ursae Majoris. Res. Astron. Astrophys. 2022, 22, 105006. [Google Scholar] [CrossRef]
- Yang, X.H.; Fu, J.N.; Zha, Q. Stellar Pulsations and Period Changes in the SX Phoenicis Star XX Cygni. Astron. J. 2012, 144, 92. [Google Scholar] [CrossRef]
- Ceraski, W. Une nouvelle variable 14.1904 Cygni. Astron. Nachrichten 1904, 165, 61. [Google Scholar] [CrossRef]
- Joner, M.D. The nature of the DWARF cepheid XX CYG. Publ. Astron. Soc. Pac. 1982, 94, 289. [Google Scholar] [CrossRef]
- Hintz, E.G.; Joner, M.D. Fourier decomposition of the light curves of three DWARF CEPHEIDS: CY AQUARII, XX CYGNI, AND V798 CYGNI. Publ. Astron. Soc. Pac. 1997, 109, 639. [Google Scholar] [CrossRef][Green Version]
- Withers, S.; Kantorowitz-Sapers, A.; Parsons, J.; Wu, J.Y.; Delaney, P.A. Photometric Monitoring and Period Changes in SX Phoenicis Star XX Cygni. IV. J. R. Astron. Soc. Can. 2021, 115, 238. [Google Scholar]
- Yang, T.Z.; Esamdin, A.; Fu, J.N.; Niu, H.B.; Feng, G.J.; Song, F.F.; Liu, J.Z.; Ma, L. Pulsations of the High-Amplitude δ Scuti star YZ Bootis. Res. Astron. Astrophys. 2018, 18, 002. [Google Scholar] [CrossRef]
- Joner, M.D.; McNamara, D.H. A photometric and spectrographic study of YZ Bootis. Publ. Astron. Soc. Pac. 1983, 95, 433–439. [Google Scholar] [CrossRef]
- Zhou, A.Y.; Jiang, S.Y. Period and Amplitude Variability of the High-amplitude δ Scuti Star GP Andromedae. Astron. J. 2011, 142, 100. [Google Scholar] [CrossRef]
- Szeidl, B.; Schnell, A.; Pocs, M.D. The high-amplitude delta Scuti star GP Andromedae. Inf. Bull. Var. Stars 2006, 5718, 1. [Google Scholar]
- Pop, A.; Liteanu, V.; Moldovan, D. On the period variability of GP Andromedae. Astrophys. Space Sci. 2003, 284, 1207–1222. [Google Scholar] [CrossRef]
- Rodriguez, E.; Rolland, A.; Lopez de Coca, P. Simultaneous uvby-beta photometry of GP Andromedae. Astron. Astrophys. Suppl. 1993, 101, 421. [Google Scholar]
- Churms, J.; Evans, D.S. HD 199757: A variable stars of extremely short period. Observatory 1961, 81, 25–29. [Google Scholar]
- Kim, C.; Moon, B.K. A Photometric Study of ZZ Microscopium. Publ. Astron. Soc. Pac. 2009, 121, 478. [Google Scholar] [CrossRef]
- Axelsen, R.A.; Napier-Munn, T. Recently Determined Light Elements for the delta Scuti Star ZZ Microscopii. J. Am. Assoc. Var. Star Obs. 2015, 43, 50. [Google Scholar]
- Ricker, G.R.; Winn, J.N.; Vanderspek, R.; Latham, D.W.; Bakos, G.Á.; Bean, J.L.; Berta-Thompson, Z.K.; Brown, T.M.; Buchhave, L.; Butler, N.R.; et al. Transiting Exoplanet Survey Satellite (TESS). J. Astron. Telesc. Instrum. Syst. 2015, 1, 014003. [Google Scholar] [CrossRef]
- Jenkins, J.M.; Twicken, J.D.; McCauliff, S.; Campbell, J.; Sanderfer, D.; Lung, D.; Mansouri-Samani, M.; Girouard, F.; Tenenbaum, P.; Klaus, T.; et al. The TESS science processing operations center. In Society of Photo-Optical Instrumentation Engineers (SPIE) Conference Series, Proceedings of the Software and Cyberinfrastructure for Astronomy IV, Edinburgh, UK, 26–30 June 2016; Chiozzi, G., Guzman, J.C., Eds.; SPI: Bellingham, WA, USA, 2016; Volume 9913, p. 99133E. [Google Scholar] [CrossRef]
- Caldwell, D.A.; Tenenbaum, P.; Twicken, J.D.; Jenkins, J.M.; Ting, E.; Smith, J.C.; Hedges, C.; Fausnaugh, M.M.; Rose, M.; Burke, C. TESS Science Processing Operations Center FFI Target List Products. Res. Notes Am. Astron. Soc. 2020, 4, 201. [Google Scholar] [CrossRef]
- Cardoso, J.V.d.M. et al. [Lightkurve Collaboration] Lightkurve: Kepler and TESS time series analysis in Python. Astrophysics Source Code Library, record ascl:1812.013, 2018. Available online: http://ascl.net/1812.013 (accessed on 1 February 2024).
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental algorithms for scientific computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef]
- Kiss, L.L.; Derekas, A. Period Variation of XX Cygni Revisited. Inf. Bull. Var. Stars 2000, 4950, 1. [Google Scholar]
- Breger, M. Mode and period changes in pulsating stars near the main sequence: δ Scuti stars. New Astron. Rev. 1999, 43, 441–447. [Google Scholar] [CrossRef]
- Powell, J.M.; Joner, M.D.; McNamara, D.H. The Long-Term Period Variations of the SX Phoenicis Star CY Aquarii. Publ. Astron. Soc. Pac. 1995, 107, 225. [Google Scholar] [CrossRef]
- Fang, W.J.; Luo, Z.Q.; Zhang, X.B.; Deng, L.C.; Wang, K.; Luo, Y.P.; Pan, Y.; Peng, Y.J. The high-amplitude δ Scuti variable CY Aqr is probably a triple system. Res. Astron. Astrophys. 2016, 16, 96. [Google Scholar] [CrossRef]
- Rodriguez, E.; Rolland, A.; Lopez de Coca, P. Period change in GP And. Delta Scuti Star Newsl. 1993, 6, 10. [Google Scholar]
- Boonyarak, C.; Fu, J.N.; Khokhuntod, P.; Jiang, S.Y. On the period variations of several low declination high amplitude delta Scuti variables. Astrophys. Space Sci. 2011, 333, 125–131. [Google Scholar] [CrossRef]
- Li, G.; Fu, J.; Su, J.; Fox-Machado, L.; Michel, R.; Guo, Z.; Liu, J.; Feng, G. Pulsations and period variations of the δ Scuti star AN Lyncis in a possible three-body system. Mon. Not. R. Astron. Soc. 2018, 473, 398–411. [Google Scholar] [CrossRef]
- Breger, M. Pre-Main Stars. I. Light Variability, Shells, and Pulsation in NGC 2264. Astrophys. J. 1972, 171, 539. [Google Scholar] [CrossRef]
- Díaz-Fraile, D.; Rodríguez, E.; Amado, P.J. Searching for δ Scuti-type pulsation and characterising northern pre-main-sequence field stars. Astron. Astrophys. 2014, 568, A32. [Google Scholar] [CrossRef]
- Zwintz, K.; Fossati, L.; Ryabchikova, T.; Guenther, D.; Aerts, C.; Barnes, T.G.; Themeßl, N.; Lorenz, D.; Cameron, C.; Kuschnig, R.; et al. Echography of young stars reveals their evolution. Science 2014, 345, 550–553. [Google Scholar] [CrossRef]
- Zong, P.; Esamdin, A.; Fu, J.N.; Biao Niu, H.; Jie Feng, G.; Zhi Yang, T.; Hai Bai, C.; Zhang, Y.; Liu, J.Z. Pulsations of the SX Phe Star BL Camelopardalis. Publ. Astron. Soc. Pac. 2019, 131, 064202. [Google Scholar] [CrossRef]
- Deng, Z.L.; Gao, Z.F.; Li, X.D.; Shao, Y. On the Formation of PSR J1640+2224: A Neutron Star Born Massive? Astrophys. J. 2020, 892, 4. [Google Scholar] [CrossRef]
- Deng, Z.L.; Li, X.D.; Gao, Z.F.; Shao, Y. Evolution of LMXBs under Different Magnetic Braking Prescriptions. Astrophys. J. 2021, 909, 174. [Google Scholar] [CrossRef]
- Parkhurst, J.A.; Jordan, F.C. Photographic photometry of short-period variable stars. Astrophys. J. 1906, 23, 79–91. [Google Scholar] [CrossRef]
- Shapley, H.; Shapley, M.B. A study of the lightcurve of XX Cygni. Astrophys. J. 1915, 42, 148–162. [Google Scholar] [CrossRef]
- Jordan, F.C. Photographic photometry with the thirty-inch Thaw refractor: The light curves of twenty nine Cepheid variables. Publ. Allegh. Obs. Univ. Pittsburgh 1929, 7, 1–124. [Google Scholar]
- Kleissen, E. Untersuchungen über Gesetzmäßigkeiten des Lichtwechsels der RR Lyrae-Sterne. Astron. Nachrichten 1938, 267, 137. [Google Scholar] [CrossRef]
- Detre, L. XX Cygni. Astron. Nachrichten 1936, 258, 329. [Google Scholar] [CrossRef]
- Szeidl, B.; Mahdy, H.A. Period Changes in Dwarf Cepheids, II. YZ Bootis, XX Cygni and DY Herculis. Commun. Konkoly Obs. Hung. 1981, 75, 1–35. [Google Scholar]
- Fitch, W.S.; Wisniewski, W.Z.; Johnson, H.L. Photoelectric UBV observations of RR Lyr variable stars. Commun. Lunar Planet. Lab. 1966, 5, 71. [Google Scholar]
- Romano, G.; Perissinotto, M. Observations of Some Variable Stars. Inf. Bull. Var. Stars 1982, 2161, 1. [Google Scholar]
- McNamara, D.H.; Feltz, K.A. A photometric and spectrographic study of XX Cyg. Publ. Astron. Soc. Pac. 1980, 92, 195–201. [Google Scholar] [CrossRef]
- Sadun, A.C.; Ressler, M. Photometric observations of the dwarf cepheid XX Cygni. Publ. Astron. Soc. Pac. 1986, 98, 504–506. [Google Scholar] [CrossRef]
- Kim, C.; Joner, M.D. VRc Photometry of Dwarf Cepheids (I). Observation. Astrophys. Space Sci. 1994, 218, 113–135. [Google Scholar] [CrossRef]
- Acerbi, F.; Barani, C. CCD observations of the variable star of SX Phe type, XX Cyg. In Proceedings of the 27th Conference on Variable Star Research, Brno, Czech Republic, 10–12 November 1995; Zejda, M., Ed.; Astronomical Institute, Slovak Academy of Science: Tatranská Lomnica, The Slovak Republic, 1996; pp. 18–22. [Google Scholar]
- Blake, R.M.; Delaney, P.; Khosravani, H.; Tome, J.; Lightman, M. Period Changes in SX Phoenicis Stars. II. XX Cygni. Publ. Astron. Soc. Pac. 2003, 115, 212–217. [Google Scholar] [CrossRef]
- Agerer, F.; Hubscher, J. Photoelectric Minima of Selected Eclipsing Binaries and Maxima of Pulsating Stars. Inf. Bull. Var. Stars 2000, 4912, 1. [Google Scholar]
- Zhou, A.Y.; Jiang, S.Y.; Chayan, B.; Du, B.T. Amplitude and period changes in the SX Phoenicis star XX Cygni. Astrophys. Space Sci. 2002, 281, 699–714. [Google Scholar] [CrossRef]
- Hubscher, J. Photoelectric Minima of Selected Eclipsing Binaries and Maxima of Pulsating Stars. Inf. Bull. Var. Stars 2005, 5643, 1. [Google Scholar]
- Hubscher, J.; Paschke, A.; Walter, F. Photoelectric Minima of Selected Eclipsing Binaries and Maxima of Pulsating Stars. Inf. Bull. Var. Stars 2005, 5657, 1. [Google Scholar]
- Klingenberg, G.; Dvorak, S.W.; Robertson, C.W. Times of Maxima for Selected Delta Scuti Stars. Inf. Bull. Var. Stars 2006, 5701, 1. [Google Scholar]
- Hubscher, J.; Paschke, A.; Walter, F. Photoelectric Minima of Selected Eclipsing Binaries and Maxima of Pulsating Stars. Inf. Bull. Var. Stars 2006, 5731, 1. [Google Scholar]
- Biro, I.B.; Borkovits, T.; Csizmadia, S.; Hegedus, T.; Klagyivik, P.; Kiss, Z.T.; Kovacs, T.; Lampens, P.; Patkos, L.; Pal, A.; et al. New Times of Minima of Eclipsing Binary Systems and of Maximum of SXPHE Type Stars. Inf. Bull. Var. Stars 2006, 5684, 1. [Google Scholar]
- Derekas, A.; Kiss, L.L.; Bedding, T.R.; Ashley, M.C.B.; Csák, B.; Danos, A.; Fernandez, J.M.; Fűrész, G.; Mészáros, S.; Szabó, G.M.; et al. Binarity and multiperiodicity in high-amplitude δ Scuti stars. Mon. Not. R. Astron. Soc. 2009, 394, 995–1008. [Google Scholar] [CrossRef]
- Fiacconi, D.; Tinelli, L. Light curve analysis of XX Cygni from data taken using DSLR. Open Eur. J. Var. Stars 2009, 114, 1. [Google Scholar]
- Conidis, G.J.; Sadavoy, S.I.; Maxwell, A.J.; Delaney, P.A.; Manzer, L.H. Period Changes in SX Phoenicis Stars. III. XX Cygni. Publ. Astron. Soc. Pac. 2011, 123, 26. [Google Scholar] [CrossRef]
- Eggen, O.J. A New Short-Period Variable Star. Publ. Astron. Soc. Pac. 1955, 67, 354. [Google Scholar] [CrossRef]
- Broglia, P.; Masani, A. Osservazioni fotoelettriche in due colori della variabile BD + 37°2635. Mem. Soc. Astron. Ital. 1957, 28, 13. [Google Scholar]
- Spinrad, H. Photoelectric Observations of RR Lyrae Stars. Astrophys. J. 1959, 130, 539. [Google Scholar] [CrossRef]
- Broglia, P. Sui periodi di alcune variabiliti di tipo RR Lyr. Mem. Soc. Astron. Ital. 1961, 32, 7. [Google Scholar]
- Heiser, A.M.; Hardie, R.H. Three-Color Photometry of YZ Boötis. Astrophys. J. 1964, 140, 694. [Google Scholar] [CrossRef]
- Gieren, W.; Gieseking, F.; Hoffmann, M. Three-colour Photometry of the RR Lyrae-Star YZ Bootis. Astron. Astrophys. 1974, 37, 443. [Google Scholar]
- Langford, W.R. Intermediate-Band Photometry of Short Period Dwarf Cepheid Variables; Provided by the SAO/NASA Astrophysics Data System. Ph.D. Thesis, Brigham Young University, Provo, UT, USA, 1976. [Google Scholar]
- Ward, R.; Delaney, P.; Sadavoy, S.; Maxwell, A.; Senthilnathan, S.; Hsu, S. Period Changes for the β Scuti Star YZ Boo. J. R. Astron. Soc. Can. 2008, 102, 134. [Google Scholar]
- Jiang, S.Y. The period variation of YZ Boo. Acta Astron. Sin. 1985, 26, 297–300. [Google Scholar]
- Peniche, R.; Gonzalez, S.F.; Pena, J.H. Photometry and period determination of the delta Scuti star YZ Bootis. Publ. Astron. Soc. Pac. 1985, 97, 1172–1177. [Google Scholar] [CrossRef]
- Hamdy, M.A.; Mahdy, H.A.; Soliman, M.A. On the Period Variation of YZ Boo. Inf. Bull. Var. Stars 1986, 2963, 1. [Google Scholar]
- Agerer, F.; Dahm, M.; Hubscher, J. Photoelectric Minima of Selected Eclipsing Binaries and Maxima of Pulsating Stars. Inf. Bull. Var. Stars 1999, 4712, 1. [Google Scholar]
- Derekas, A.; Kiss, L.L.; Székely, P.; Alfaro, E.; Csák, B.; Mészáros, S.; Rodriguez, E.; Rolland, A.; Sárneczky, K.; Szabó, G.M.; et al. A photometric monitoring of bright high-amplitude δ Scuti stars-II. Period updates for seven stars. Astron. Astrophys. 2003, 402, 733–743. [Google Scholar] [CrossRef][Green Version]
- Agerer, F.; Hubscher, J. Photoelectric Maxima of Selected Pulsating Stars. Inf. Bull. Var. Stars 2003, 5485, 1. [Google Scholar]
- Jin, H.; Kim, S.L.; Kwon, S.G.; Youn, J.H.; Lee, C.U.; Lee, D.J.; Kim, K.S. Multiband photometric re-classification of ROTSE-I δ Scuti type stars. Astron. Astrophys. 2003, 404, 621–629. [Google Scholar] [CrossRef]
- Zhou, A.Y. Stability in period and amplitude: The High-amplitude δ Scuti Star YZ Boötis. Astrophys. Space Sci. 2006, 301, 11–20. [Google Scholar] [CrossRef]
- Splittgerber, E. 18 Maxima von GP Andromedae. Zentralinstitut Astrophys. Sternwarte Sonneb. Mitteilungen Veraenderliche Sterne 1976, 7, 137–138. [Google Scholar]
- Gieseking, F.; Hoffmann, M.; Nelles, B. Photoelectric photometry of the RRs-variable GP And. Astron. Astrophys. Suppl. 1979, 36, 457–463. [Google Scholar]
- Eggen, O.J. Intermediate Band and Hbeta Photometry of Ultrashort Period Cepheids. Inf. Bull. Var. Stars 1978, 1517, 1. [Google Scholar]
- Burchi, R.; de Santis, R.; di Paolantonio, A.; Piersimoni, A.M. Photoelectric photometry of field variables. I. Astron. Astrophys. Suppl. 1993, 97, 827–834. [Google Scholar]
- Schmidt, E.G.; Chab, J.R.; Reiswig, D.E. The Behlen Observatory Variable Star Survey. Paper III. Astron. J. 1995, 109, 1239. [Google Scholar] [CrossRef]
- Leung, K.C. Dwarf cepheid HD 199757. Astron. J. 1968, 73, 500–503. [Google Scholar] [CrossRef]
- Chambliss, C.R. Photoelectric Photometry of the Dwarf Cepheid HD 199757. Astrophys. J. 1971, 165, 365. [Google Scholar] [CrossRef]
- Balona, L.A.; Martin, W.L. The radius of the dwarf cepheid ZZ Mic. Mon. Not. R. Astron. Soc. 1978, 184, 11–15. [Google Scholar] [CrossRef][Green Version]


| ID | TESS Sector | Time Spans of AAVSO | Time Span of BAV |
|---|---|---|---|
| XX Cyg | 14 (2019), 41 (2021) | 2008–2023 | 2001–2022 |
| YZ Boo | 24 (2020), 50 (2022) | 2006–2024 | 2000–2016 |
| GP And | 17 (2019) | 2008–2023 | 1998–2014 |
| ZZ Mic | 1 (2018), 27 (2020) | 2006–2007 | — |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, T.-F.; Niu, J.-S.; Xue, H.-F. Period Variation Rates of Four Radial Single-Mode High-Amplitude Delta Scuti Stars. Universe 2025, 11, 86. https://doi.org/10.3390/universe11030086
Ma T-F, Niu J-S, Xue H-F. Period Variation Rates of Four Radial Single-Mode High-Amplitude Delta Scuti Stars. Universe. 2025; 11(3):86. https://doi.org/10.3390/universe11030086
Chicago/Turabian StyleMa, Tian-Fang, Jia-Shu Niu, and Hui-Fang Xue. 2025. "Period Variation Rates of Four Radial Single-Mode High-Amplitude Delta Scuti Stars" Universe 11, no. 3: 86. https://doi.org/10.3390/universe11030086
APA StyleMa, T.-F., Niu, J.-S., & Xue, H.-F. (2025). Period Variation Rates of Four Radial Single-Mode High-Amplitude Delta Scuti Stars. Universe, 11(3), 86. https://doi.org/10.3390/universe11030086







