Dark Energy and Cosmological Bounce Supported by an Unconventional Spinor Field
Abstract
1. Introduction
2. A Spinor–Scalar–Tensor (SST) Model with a Gauge Scalar Singlet
2.1. Connection and Curvature
2.2. Covariant Derivative of the Dirac Spinor Field
2.3. Action, Gauge Transformations and Field Equations
Eliminating the Scalar Field
3. Cosmological Evolution
3.1. Integrable Examples
3.1.1. Subcase I (Dust): , and
3.1.2. Subcase II (Cosmological Constant): , and
3.1.3. Subcase III (Bounce): , and
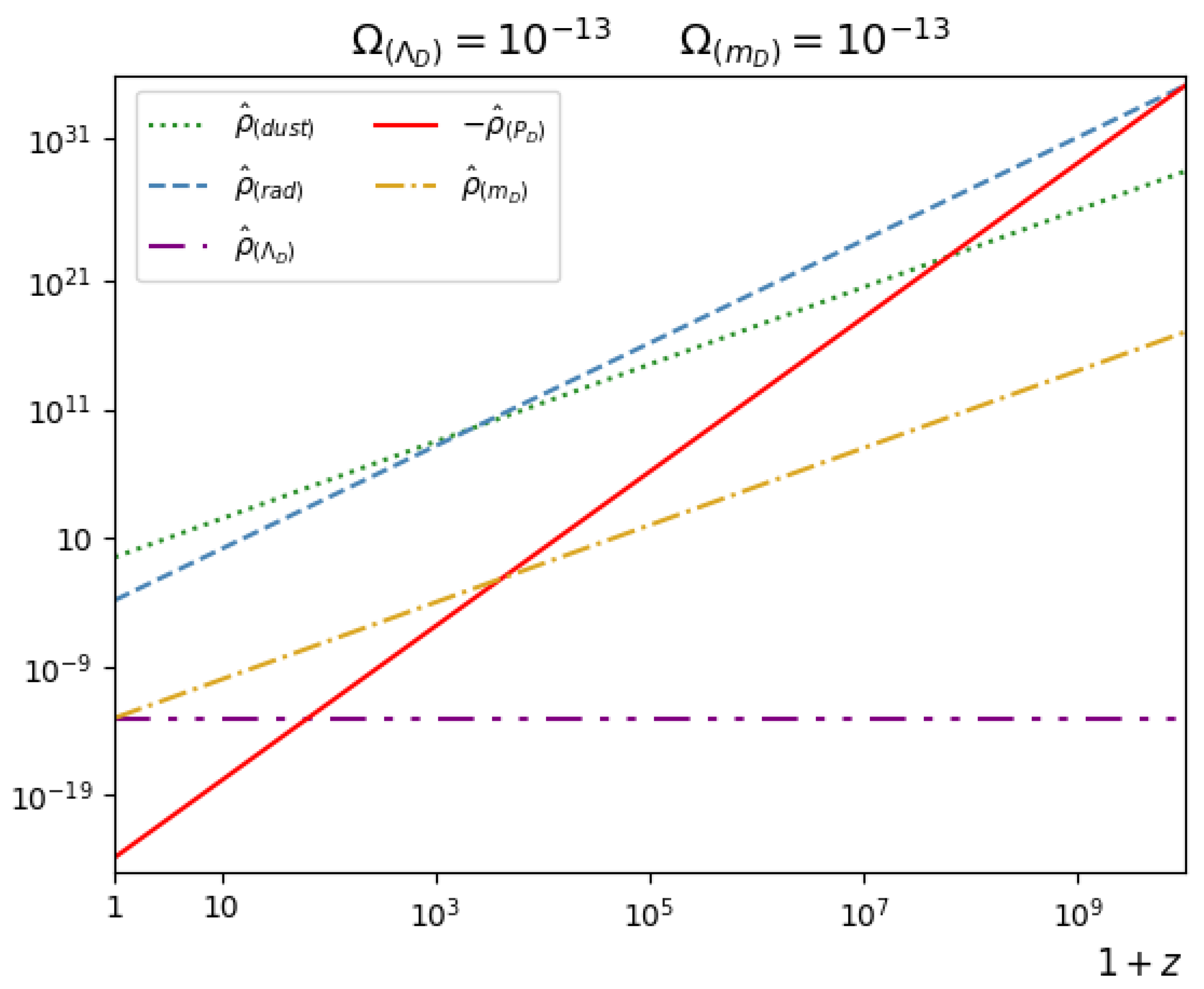
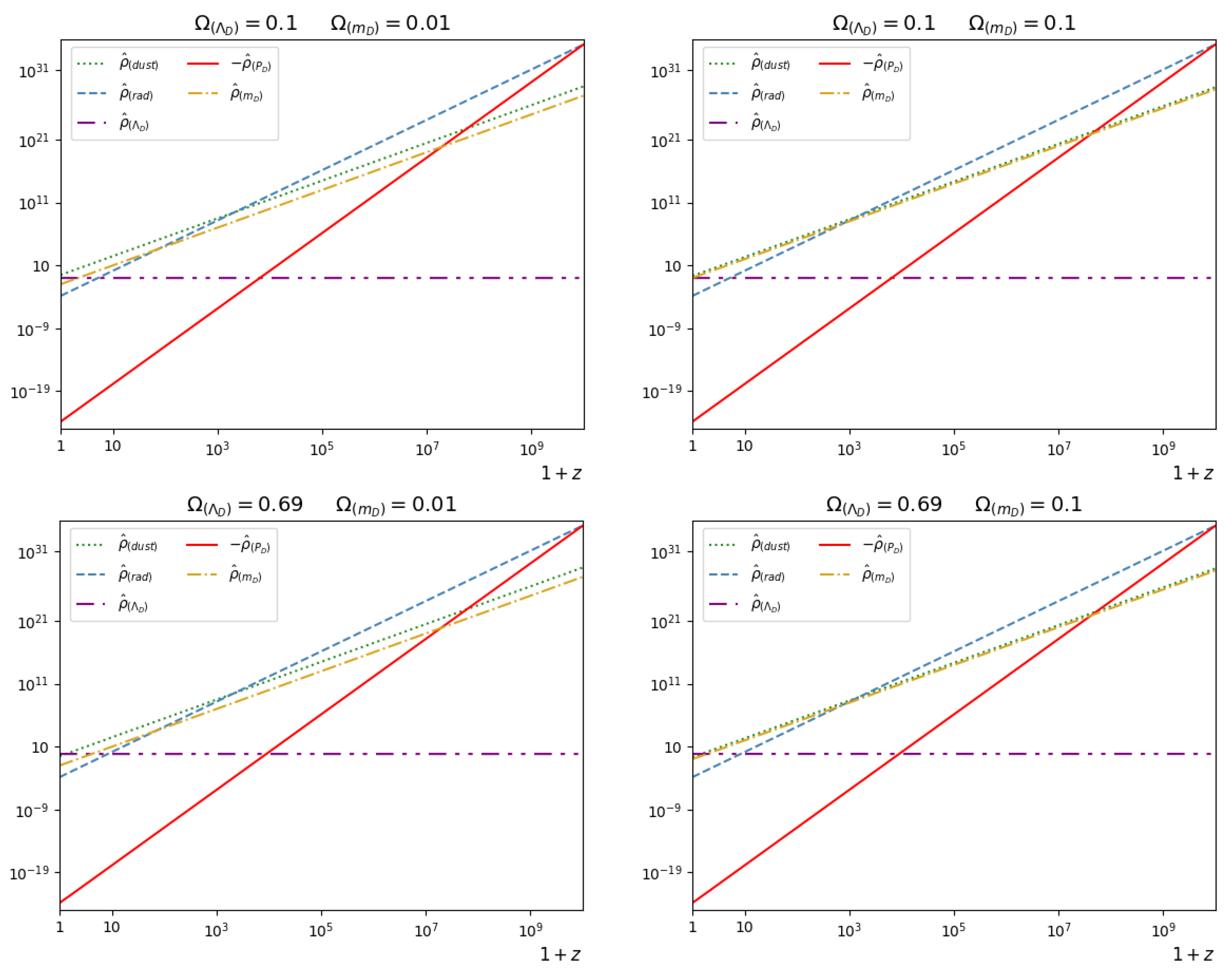
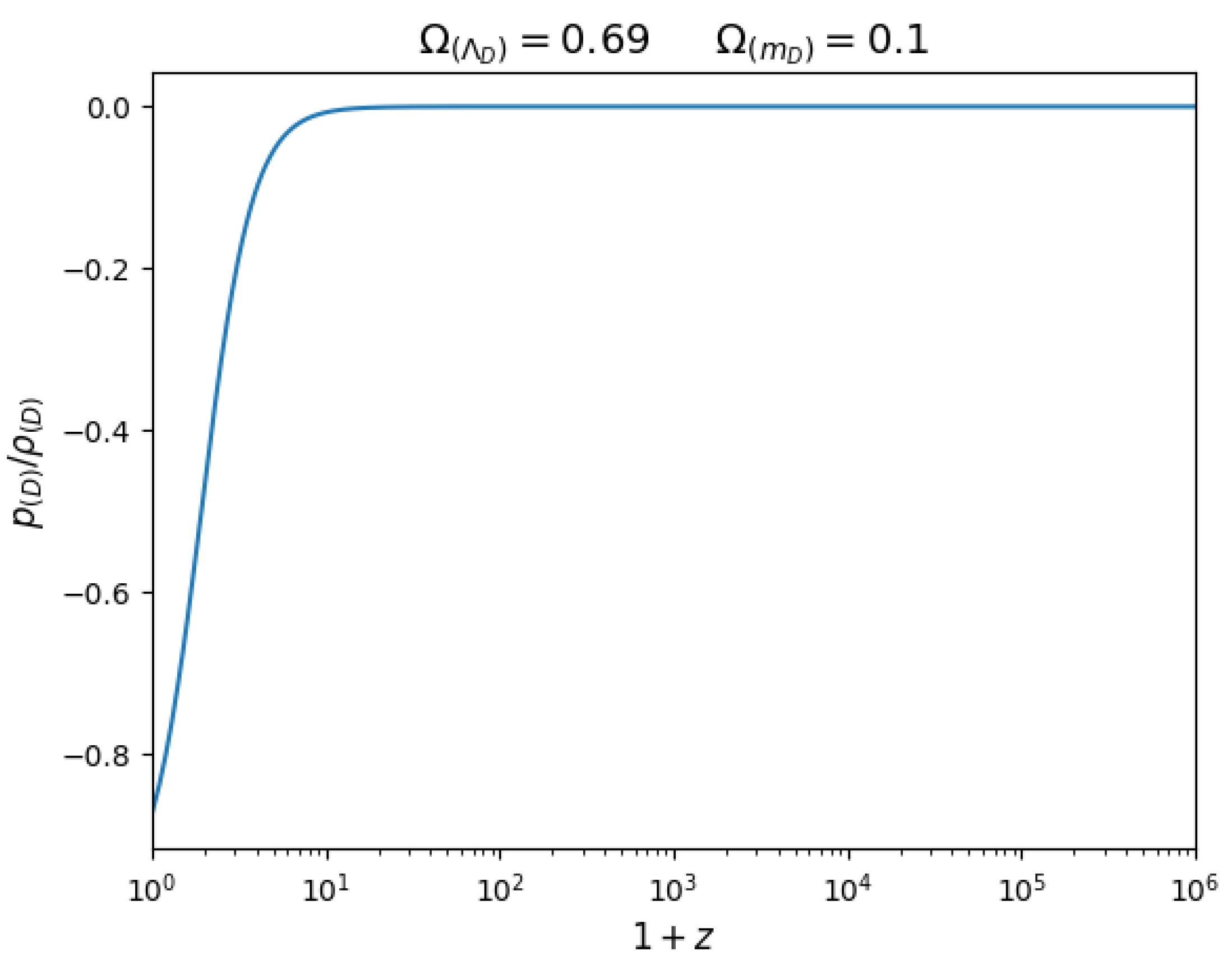
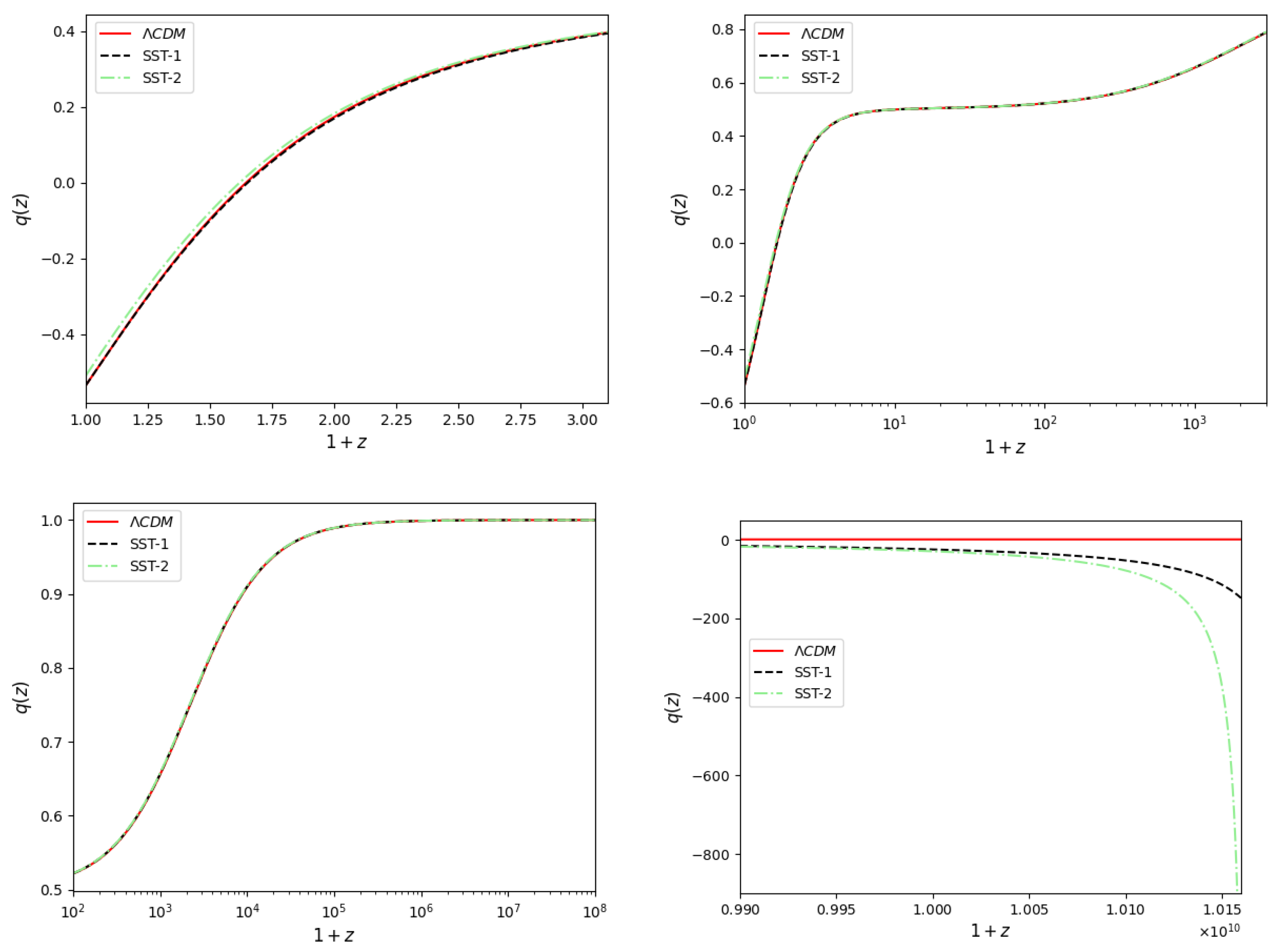
3.2. Numerical Examples
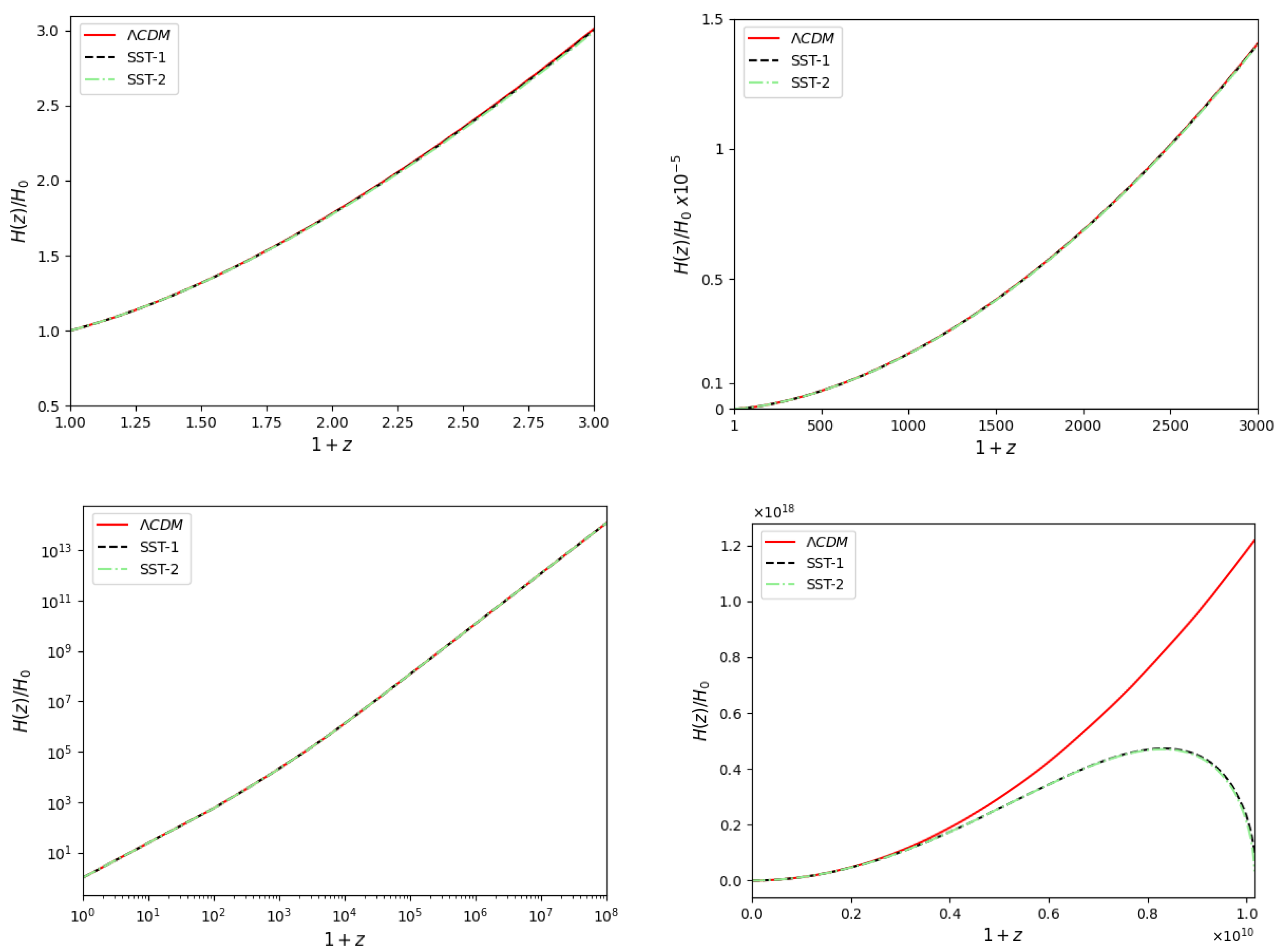
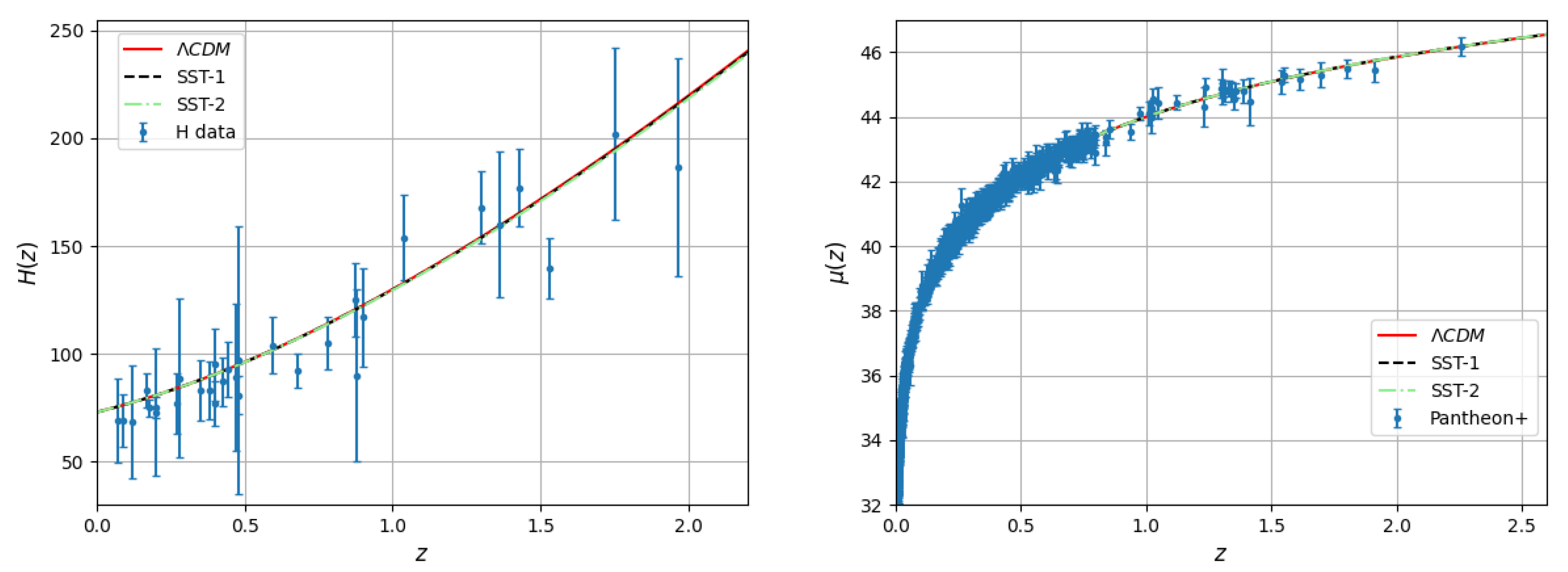
3.3. Comparison with a Flat CDM Model and Observations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Equation of System Derived from Modified Dirac Equation
| 1 | |
| 2 | The representation for the Dirac gamma matrices is given [63] by |
| 3 | |
| 4 | Note that is introduced with an extra minus factor in Ref. [63]. |
| 5 | The global phase invariance of the Dirac field action implies a conserved, real 4-current . The integration of the perpendicular component of this current to a three-dimensional spacelike hypersurface over gives a conserved charge Q. Upon quantization Q is interpreted as the fermion number inside . Here, we have defined the conserved 4-current including an extra factor , which must be taken into account when relating to the particle number. |
| 6 | We have dropped the boundary term resulting from in the expression of the Ricci scalar. |
References
- Hinshaw, G.; Larson, D.; Komatsu, E.; Spergel, D.N.; Bennett, C.; Dunkley, J.; Nolta, M.R.; Halpern, M.; Hill, R.S.; Odegard, N.; et al. [WMAP Collaboration]. Nine-year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Cosmological Parameter Results. Astrophys. J. Suppl. Ser. 2013, 208, 19. [Google Scholar] [CrossRef]
- Collaboration, P.; Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; et al. [Planck Collaboration]. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar]
- Borde, A.; Vilenkin, A. Eternal inflation and the initial singularity. Phys. Rev. Lett. 1994, 72, 3305. [Google Scholar] [CrossRef]
- Brandenberger, R.H.; Vafa, C. Superstrings in the early universe. Nucl. Phys. B 1989, 316, 391. [Google Scholar] [CrossRef]
- Ellis, G.F.R.; Maartens, R. The Emergent Universe: Inflationary cosmology with no singularity. Class. Quantum Grav. 2004, 21, 223. [Google Scholar] [CrossRef]
- Ellis, G.F.R.; Murugan, J.; Tsagas, C.G. The emergent universe: An explicit construction. Class. Quantum Grav. 2004, 21, 233. [Google Scholar] [CrossRef]
- Khodadi, M.; Heydarzade, Y.; Darabi, F.; Saridakis, E.N. Emergent universe in Horava-Lifshitz-like F(R) gravity. Phys. Rev. D 2016, 93, 124019. [Google Scholar] [CrossRef]
- Huang, Q.; Wu, P.; Yu, H. Stability of Einstein static universe in gravity theory with a non-minimal derivative coupling. Eur. Phys. J. C 2018, 78, 51. [Google Scholar] [CrossRef]
- Huang, Q.; Huang, H.; Chen, J.; Kang, S. On the stability of Einstein static universe in general scalar–tensor theory with non-minimal derivative coupling. Ann. Phys. 2018, 399, 124. [Google Scholar] [CrossRef]
- Paul, B.C.; Majumdar, A.S. Emergent universe with wormholes in massive gravity. Class. Quantum Grav. 2018, 35, 065001. [Google Scholar] [CrossRef]
- Li, S.-L.; Lü, H.; Wei, H.; Wu, P.; Yu, H. Emergent universe scenario, bouncing universes, and cyclic universes in degenerate massive gravity. Phys. Rev. D 2019, 99, 104057. [Google Scholar] [CrossRef]
- Ilyas, A.; Zhu, M.; Zheng, Y.; Cai, Y.-F. Emergent Universe and Genesis from the DHOST Cosmology. J. High Energy Phys. 2021, 2101, 141. [Google Scholar] [CrossRef]
- Huang, Q.; Xu, B.; Huang, H.; Tu, F.; Zhang, R. Emergent scenario in mimetic gravity. Class. Quantum Grav. 2020, 37, 195002. [Google Scholar] [CrossRef]
- Labraña, P.; Cossio, H. Emergent universe by tunneling in a Jordan-Brans-Dicke theory. Eur. Phys. J. C 2019, 79, 303. [Google Scholar]
- Sarkar, P.; Das, P.K. Emergent cosmology in models of nonlinear electrodynamics. New Astron. 2023, 101, 102003. [Google Scholar] [CrossRef]
- Cai, Y.-F.; Li, M.; Zhang, X. Emergent Universe Scenario via Quintom Matter. Phys. Lett. B 2012, 718, 248. [Google Scholar] [CrossRef]
- Cai, Y.-F.; Wan, Y.; Zhang, X. Cosmology of the Spinor Emergent Universe and Scale-invariant Perturbations. Phys. Lett. B 2014, 731, 217. [Google Scholar] [CrossRef]
- Huang, Q.; Wu, P.; Yu, H. Emergent scenario in the Einstein-Cartan theory. Phys. Rev. D 2015, 91, 103502. [Google Scholar] [CrossRef]
- Shabani, H.; Ziaie, A.H. Stability of the Einstein static Universe in Einstein–Cartan–Brans–Dicke gravity. Eur. Phys. J. C 2019, 79, 270. [Google Scholar] [CrossRef]
- Mukhanov, V.; Brandenberger, R. A nonsingular universe. Phys. Rev. Lett. 1992, 68, 1969. [Google Scholar] [CrossRef]
- Brandenberger, R.; Mukhanov, V.; Sornborger, A. Cosmological theory without singularities. Phys. Rev. D 1993, 48, 1629. [Google Scholar] [CrossRef] [PubMed]
- Abramo, L.R.; Yasuda, I.; Peter, P. onsingular bounce in modified gravity. Phys. Rev. D 2010, 81, 023511. [Google Scholar] [CrossRef]
- Chamseddine, A.H.; Mukhanov, V. Resolving cosmological singularities. J. Cosmol. Astropart. Phys. 2017, 2017, 009. [Google Scholar] [CrossRef]
- Chamseddine, A.H.; Mukhanov, V. Mimetic Dark Matter. J. High Energy Phys. 2013, 1311, 131. [Google Scholar] [CrossRef]
- Chaichian, M.; Kluson, J.; Oksanen, M.; Tureanu, A. Mimetic dark matter, ghost instability and a mimetic tensor-vector-scalar gravity. J. High Energy Phys. 2014, 1412, 102. [Google Scholar] [CrossRef]
- Firouzjahi, H.; Gorji, M.A.; Mansoori, S.A.H. Instabilities in mimetic matter perturbations. J. Cosmol. Astropart. Phys. 2017, 1707, 031. [Google Scholar] [CrossRef]
- Yoshida, D.; Quintin, J.; Yamaguchi, M.; Brandenberger, R. Cosmological perturbations and stability of nonsingular cosmologies with limiting curvature. Phys. Rev. D 2017, 96, 043502. [Google Scholar] [CrossRef]
- Chamseddine, A.H.; Mukhanov, V.; Vikman, A. Cosmology with Mimetic Matter. J. Cosmol. Astropart. Phys. 2014, 1406, 017. [Google Scholar] [CrossRef]
- Brandenberger, R.H. Alternatives to the inflationary paradigm of structure formation. Int. J. Mod. Phys. Conf. Ser. 2011, 1, 67. [Google Scholar] [CrossRef]
- Cartier, C.; Durrer, R.; Copeland, E.J. Cosmological perturbations and the transition from contraction to expansion. Phys. Rev. D 2003, 67, 103517. [Google Scholar] [CrossRef]
- Cai, Y.F.; Qiu, T.; Zhang, X.; Piao, Y.S.; Li, M. Bouncing universe with Quintom matter. J. High Energy Phys. 2007, 0710, 071. [Google Scholar] [CrossRef]
- Cai, Y.F.; Qiu, T.; Brandenberger, R.; Piao, Y.S.; Zhang, X. On Perturbations of Quintom Bounce. J. Cosmol. Astropart. Phys. 2008, 0803, 013. [Google Scholar] [CrossRef]
- Cline, J.M.; Jeon, S.; Moore, G.D. The phantom menaced: Constraints on low-energy effective ghosts. Phys. Rev. D 2004, 70, 043543. [Google Scholar] [CrossRef]
- Lin, C.; Brandenberger, R.H.; Levasseur, L.P. A Matter Bounce By Means of Ghost Condensation. J. Cosmol. Astropart. Phys. 2011, 1104, 019. [Google Scholar] [CrossRef]
- Khoury, J.; Ovrut, B.A.; Steinhardt, P.J.; Turok, N. Ekpyrotic universe: Colliding branes and the origin of the hot big bang. Phys. Rev. D 2001, 64, 123522. [Google Scholar] [CrossRef]
- Brandenberger, R.; Finelli, F. On the Spectrum of Fluctuations in an Effective Field Theory of the Ekpyrotic Universe. J. High Energy Phys. 2001, 0111, 056. [Google Scholar] [CrossRef][Green Version]
- Lyth, D.H. The primordial curvature perturbation in the ekpyrotic Universe. Phys. Lett. B 2002, 524, 1. [Google Scholar] [CrossRef]
- Cai, Y.-F.; Easson, D.A.; Brandenberger, R. Towards a nonsingular bouncing cosmology. J. Cosmol. Astropart. Phys. 2012, 1208, 020. [Google Scholar] [CrossRef]
- Brandenberger, R.; Wang, Z. Ekpyrotic cosmology with a zero-shear S-brane. Phys. Rev. D 2020, 101, 063522. [Google Scholar] [CrossRef]
- Houndjo, M.J.S. Unimodular f(G) gravity. Eur. Phys. J. C 2017, 77, 607. [Google Scholar] [CrossRef][Green Version]
- Casalino, A.; Sebastiani, L.; Zerbini, S. Note on nonsingular Einstein-Aether cosmologies. Phys. Rev. D 2020, 101, 104059. [Google Scholar] [CrossRef]
- Caruana, M.; Farrugia, G.; Said, J.L. Cosmological bouncing solutions in f(T, B) gravity. Eur. Phys. J. C 2020, 80, 640. [Google Scholar] [CrossRef]
- Ilyas, A.; Zhu, M.; Zheng, Y.; Cai, Y.-F.; Saridakis, E.N. DHOST bounce. J. Cosmol. Astropart. Phys. 2020, 2009, 002. [Google Scholar] [CrossRef]
- Casalino, A.; Sanna, B.; Sebastiani, L.; Zerbini, S. Bounce models within teleparallel modified gravity. Phys. Rev. D 2021, 103, 023514. [Google Scholar] [CrossRef]
- Ilyas, M.; Rahman, W.U. Bounce cosmology in f(R) gravity. Eur. Phys. J. C 2021, 81, 160. [Google Scholar] [CrossRef]
- Chakraborty, S.; Gregoris, D. Cosmological evolution with quadratic gravity and nonideal fluids. Eur. Phys. J. C 2021, 81, 944. [Google Scholar] [CrossRef]
- Polarski, D.; Starobinsky, A.A.; Verbin, Y. Bouncing Cosmological Isotropic Solutions in Scalar-Tensor Gravity. J. Cosmol. Astropart. Phys. 2022, 2201, 052. [Google Scholar] [CrossRef]
- Ganiou, M.G.; Houndjo, M.J.S.; Aïnamon, C.; Ayivi, L.; Kanfon, A. Reconstruction method applied to bounce cosmology and inflationary scenarios in cosmological f(G) gravity. Eur. Phys. J. P 2022, 137, 208. [Google Scholar] [CrossRef]
- Kolář, I.; Torralba, F.J.M.; Mazumdar, A. New nonsingular cosmological solution of nonlocal gravity. Phys. Rev. D 2022, 105, 044045. [Google Scholar] [CrossRef]
- Poplawski, N. Nonsingular, big-bounce cosmology from spinor-torsion coupling. Phys. Rev. D 2012, 85, 107502. [Google Scholar] [CrossRef]
- Bambi, C.; Malafarina, D.; Marciano, A.; Modesto, L. Singularity avoidance in classical gravity from four-fermion interaction. Phys. Lett. B 2014, 734, 27. [Google Scholar] [CrossRef]
- Alexander, S.; Bambi, C.; Marciano, A.; Modesto, L. Fermi-bounce cosmology and scale-invariant power spectrum. Phys. Rev. D 2014, 90, 123510. [Google Scholar] [CrossRef]
- Molinari, P.A.P.; Devecchi, F.P.; Ribas, M.O. Brans-Dicke accelerated cosmologies with fermionic sources. Phys. Rev. D 2022, 105, 043527. [Google Scholar] [CrossRef]
- Battefeld, D.; Peter, P. A critical review of classical bouncing cosmologies. Phys. Rept. 2015, 571, 1. [Google Scholar] [CrossRef]
- Brandenberger, R.; Peter, P. Bouncing Cosmologies: Progress and Problems. Found. Phys. 2017, 47, 797. [Google Scholar] [CrossRef]
- Yoo, J.; Watanabe, Y. Theoretical Models of Dark Energy. Int. J. Mod. Phys. D 2012, 21, 1230002. [Google Scholar] [CrossRef]
- Arun, K.; Gudennavar, S.B.; Sivaram, C. Dark matter, dark energy, and alternate models: A review. Adv. Space Res. 2017, 60, 166. [Google Scholar] [CrossRef]
- Motta, V.; García-Aspeitia, M.A.; Hernández-Almada, A.; Magaña, J. Verdugo, Tomás Taxonomy of Dark Energy Models. Universe 2021, 7, 163. [Google Scholar] [CrossRef]
- Hehl, F.W.; von der Heyde, P.; Kerlick, G.D.; Nester, J.M. General relativity with spin and torsion: Foundations and prospects. Rev. Mod. Phys. 1976, 48, 393. [Google Scholar] [CrossRef]
- Belyaev, A.S.; Shapiro, I.L. Torsion action and its possible observables. Nucl. Phys. B 1999, 543, 20. [Google Scholar] [CrossRef]
- Shapiro, I.L. Physical Aspects of the Space-Time Torsion. Phys. Rept. 2002, 357, 113. [Google Scholar] [CrossRef]
- Aldrovandi, R.; Pereira, J.G. An Introduction to Geometrical Physics, Chapter 36: Einstein–Cartan Theory, 2nd ed.; World Scientific: Singapore, 2016. [Google Scholar]
- Dolan, S.R.; Dempsey, D. Bound states of the Dirac equation on Kerr spacetime. Class. Quantum Grav. 2015, 32, 184001. [Google Scholar] [CrossRef]
- Herdeiro, C.A.R.; Pombo, A.M.; Radu, E. Asymptotically flat scalar, Dirac and Proca stars: Discrete vs. continuous families of solutions. Phys. Lett. B 2017, 773, 654. [Google Scholar] [CrossRef]
- Cabral, F.; Lobo, F.S.N.; Rubiera-Garcia, D. Cosmological bounces, cyclic universes, and effective cosmological constant in Einstein-Cartan-Dirac-Maxwell theory. Phys. Rev. D 2020, 102, 083509. [Google Scholar] [CrossRef]
- Magueijo, J.; Zlosnik, T.G.; Kibble, T.W.B. Cosmology with a spin. Phys. Rev. D 2013, 87, 063504. [Google Scholar] [CrossRef]
- Farnsworth, S.; Lehners, J.-L.; Qiu, T. Spinor driven cosmic bounces and their cosmological perturbations. Phys. Rev. D 2017, 96, 083530. [Google Scholar] [CrossRef]
- Myrzakulov, N.; Koussour, M.; Gogoi, D.J. A New Om(z) Diagnostic of Dark Energy in General Relativity Theory. Eur. Phys. J. C 2023, 83, 594. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astrophys. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Long range forces and broken symmetries. Proc. R. Soc. Lond. A 1973, 33, 403. [Google Scholar]
- Rosen, N. Weyl’s geometry and physics. Found. Phys. 1982, 12, 213. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fekecs, B.; Keresztes, Z. Dark Energy and Cosmological Bounce Supported by an Unconventional Spinor Field. Universe 2025, 11, 59. https://doi.org/10.3390/universe11020059
Fekecs B, Keresztes Z. Dark Energy and Cosmological Bounce Supported by an Unconventional Spinor Field. Universe. 2025; 11(2):59. https://doi.org/10.3390/universe11020059
Chicago/Turabian StyleFekecs, Barna, and Zoltán Keresztes. 2025. "Dark Energy and Cosmological Bounce Supported by an Unconventional Spinor Field" Universe 11, no. 2: 59. https://doi.org/10.3390/universe11020059
APA StyleFekecs, B., & Keresztes, Z. (2025). Dark Energy and Cosmological Bounce Supported by an Unconventional Spinor Field. Universe, 11(2), 59. https://doi.org/10.3390/universe11020059





