From Be X-Ray Binaries to Double Neutron Stars: Exploring the Spin and Orbital Evolution

Abstract

We explore the evolutionary link between Galactic Be X-ray binaries (BeXBs) and Galactic field double neutron stars (DNSs) based on the properties of the NS spin period—P and binary orbital period—$P_{\mathrm{orb}}$. First, both BeXB and DNS sources show positive correlation trends in P versus $P_{\mathrm{orb}}$ relation ($P-P_{\mathrm{orb}}$ diagram), which may relate to the influence of the accretion evolution. Secondly, the two types of sources exhibit similar bi-modal P/$P_{\mathrm{orb}}$ distributions with the gaps of $P\sim 40$ s/$P_{\mathrm{orb}}\sim 60$ days for BeXBs and $P\sim 50$ ms/$P_{\mathrm{orb}}\sim 1$ day for DNSs, respectively. We propose a possibility that Galactic BeXBs may transfer the bi-modal P/$P_{\mathrm{orb}}$ classifications to Galactic field DNSs during the evolution process. Furthermore, based on the bi-modal gaps, we infer the contraction factors of P (by $\sim {\displaystyle \frac{1}{800}}$) and $P_{\mathrm{orb}}$ (by $\sim {\displaystyle \frac{1}{60}}$) valuesin the evolution from Galactic BeXBs to Galactic field DNSs. We find that the scaled P or $P_{\mathrm{orb}}$ values of Galactic BeXBs share the similar bi-modal distributions to that of Galactic field DNSs, and these results can offer a reference to trace the classification and evolution between the two types of sources.

1. Introduction

Be X-ray binaries (BeXBs) are composed of a neutron star (NS) and a massive B-type star with emission lines [1,2,3,4,5], which are the young populations of high-mass X-ray binaries (HMXBs) and originate from the binaries of two massive stars [6,7,8,9]. These objects usually show the wide ranges of a binary orbital period—$P_{\mathrm{orb}}$ and eccentricity—$Ecc$ [3,10], and are mostly transient sources with several types of X-ray outbursts [11]: (I). Type I outburst, which occurs when NS of the system crosses the circum-stellar disk of its companion at periastron [11,12]. (II). Type II outburst or giant X-ray outburst, which exhibits the luminosity up to the Eddington limit, and is not clearly related to the orbital phase [5].

BeXBs show a positive correlation trend in the relation of NS spin period—P versus binary orbital period—$P_{\mathrm{orb}}$ (i.e., $P-P_{\mathrm{orb}}$ diagram or Corbet diagram [13,14]), which is explained by the accretion effect, i.e., the shorter orbit can cause the more efficient angular momentum transfer from the companion matter to NS by accretion [15]. Knigge, Coe, and Podsiadlowski (2011) found the bi-modal P and $P_{\mathrm{orb}}$ distributions of BeXBs [16], and attributed them to the two types of supernovas, where electron-capture supernovas preferentially produce BeXBs with short orbital periods and low eccentricities, while iron-core collapse supernovas produce BeXBs with long orbital periods and high eccentricities [16]. However, some authors indicated that the bi-modal classification of BeXBs may be related to the different accretion effects, which is based on the fact that the BeXB group with $P<40 \mathrm{s}$/$P_{\mathrm{orb}}<60 \mathrm{days}$ share a higher X-ray luminosity and more Type II outbursts than the other group sources with $P>40 \mathrm{s}$/$P_{\mathrm{orb}}>60 \mathrm{days}$ [17,18].

Double-neutron stars (DNSs) are the binaries containing two NSs, i.e., a recycled one and a non-recycled one [19,20]. This kind of system was firstly discovered in 1974 [21], and there are about ∼25 DNSs at present that have been detected in the Galactic field [22]. In theory, DNSs are suggested to originate from NS-HMXBs [8,20,23,24,25,26], where NS in the system accretes the companion matter to be accelerated to the faster spin period (i.e., the recycled NS), while its companion can become an NS with the relatively slower spin period (i.e., the non-recycled NS) through the supernova explosion. Therefore, the formation of DNSs is proposed to relate to the processes of supernova explosion [20,27,28,29,30,31], stellar evolution, binary mass transfer, common envelope [32,33,34,35,36], and the accretion-induced spin-up and magnetic field decay of NSs, etc. [18,37]. Moreover, it is also proposed in theory that some DNSs may finally merge into single stars by emitting gravitational wave radiation, making them the particular objects to test the general relativity [38,39,40,41,42,43,44]. The previous investigations of DNSs usually focus on the physical property analysis such as the distributions of $P_{\mathrm{orb}}$, $Ecc$ and mass, etc., as well as the theoretical study such as the birth, evolution, and merging of systems [22,45,46,47,48,49]. For example, Andrews and Mandel (2019) divided DNSs into the different types based on Ecc-$P_{\mathrm{orb}}$ distribution [47].

The evolutionary link between BeXBs and DNSs may induce the similar physical properties or classifications between the two types of sources [50]. For example, BeXBs show bi-modal features in $P/P_{\mathrm{orb}}$ distributions [16,17,18], and DNSs can be divided into different subgroups according to $P_{\mathrm{orb}}$ distribution [47]. In fact, Zhou et al. [50] proposed that the similar bi-modal classifications may be the evidence of an evolutionary link between the two group sources. In this paper, we try to explore the evolution correlation between BeXBs and DNSs by analyzing their $P/P_{\mathrm{orb}}$ properties, and the contents of the paper are arranged as follows: in Section 2, we show BeXB and DNS samples, as well as P/$P_{\mathrm{orb}}$ values. Then, in Section 3, we analyze and compare the $P/P_{\mathrm{orb}}$ properties between BeXBs and DNSs. In Section 4, we probe the spin and orbital evolution from BeXBs to DNSs, and further infer the corresponding contraction factors of P/$P_{\mathrm{orb}}$ values. Last, in Section 5, we summary the discussions and conclusions.

2. Samples of BeXBs and DNSs

We search the published literature for BeXBs in the Milky Way and DNSs in the Galactic field, and then collect their parameter values of the NS spin period—P and binary orbital period—$P_{\mathrm{orb}}$. First, we refer to the catalogues of [3,6,10,51,52,53] and collect 94 Galactic BeXBs, which include 63 P and 60 $P_{\mathrm{orb}}$ data. In addition, 48 BeXBs among the previous 94 samples have both P and $P_{\mathrm{orb}}$ values. Secondly, we collect 25 field DNSs in the Galactic field from the published paper (see the reference in

Table 1. NS spin period—P and binary orbital period—$P_{\mathrm{orb}}$ of Galactic field DNSs.

DNS (25)	${\mathit{P} }^{\mathit{a}}$	${\mathit{P}}_{\mathbf{orb}}$ b	Refs
	(s)	(day)
$P_{\mathrm{orb}}<1$ day (10)
J1946+2052	0.0170	0.079	[59]
J0737-3039A c	0.0227	0.102	[54]
J1757-1854	0.0215	0.184	[60]
J1913+1102	0.0273	0.206	[61,62]
J1756-2251	0.0285	0.320	[63]
B1913+16	0.0590	0.323	[64,65]
J0509+3801	0.0765	0.380	[66]
B1534+12	0.0379	0.421	[67]
J1846-0513	0.0234	0.613	[68]
J1208-5936	0.0287	0.632	[69]
$P_{\mathrm{orb}}>1$ day (13)
J1829+2456	0.0410	1.176	[70]
J1325-6253	0.0290	1.816	[71]
J1759+5036	0.1760	2.043	[72]
J1411+2551	0.0625	2.616	[73]
J1155-6529	0.0789	3.670	[74]
J0453+1559	0.0458	4.072	[63]
J1518+4904	0.0410	8.634	[75]
J1018-1523	0.0832	8.839	[76]
J2150+3427	0.0653	10.592	[77]
J1753-2240	0.0951	13.638	[78]
J1901+0658	0.0757	14.455	[79]
J1811-1736	0.1042	18.779	[80]
J1930-1852	0.1855	45.060	[81]
Non-recycled pulsar detected in DNS (2)
J1906+0746	0.1441	0.166	[55,56]
J1755-2550	0.3152	9.696	[57,58]

^a $P$–NS spin period. ^b $P_{\mathrm{orb}}$—Binary orbital period. ^c—PSR J0737-3039 is observed as a double pulsars, where J0737-3039A is the recycled one and J0737-3039B is the non-recycled one.

), where PSR J0737-3039 is a double neutron star system with PSR J0737-3039A/PSR J0737-3039B being the recycled/non-recycled NS [54]. Then, we also collect P and $P_{\mathrm{orb}}$ values of these DNS sources, as shown in Table 1. It should be noticed from Table 1 that PSR J1755-2550 ($P\sim 0.315$ s) and PSR J1906+0746 ($P\sim 0.144$ s) are suggested as the non-recycled DNSs in the binary systems [55,56,57,58]. As we only consider the recycled NSs in DNS systems, we can trace the influence of the accretion-induced spin-up process. Therefore, the two DNS systems detected with the non-recycled NSs are removed from the samples in the following analysis.

3. P and P_orb Distributions

We try to trace the evolutionary links between Galactic BeXBs and Galactic field DNSs based on the relation between P and $P_{\mathrm{orb}}$.

3.1. $P-P_{\mathrm{orb}}$ Diagram

Figure 1 shows the $P-P_{\mathrm{orb}}$ relation of 48 Galactic BeXBs and 23 Galactic field DNSs. It can be seen that both the two types of sources exhibit a positive correlation trend between P and $P_{\mathrm{orb}}$. In fact, the correlation coefficient of the $P-P_{\mathrm{orb}}$ relation for BeXBs in the elliptical region of Figure 1 is ∼0.58, while the corresponding correlation coefficient for 23 DNSs is ∼0.72. Moreover, it should also be noticed from Figure 1 that Galactic BeXBs show potential gaps of $P\sim 40$ s and $P_{\mathrm{orb}}\sim 60$ day in $P-P_{\mathrm{orb}}$ relation, which are also noticed by Knigge, Coe, and Podsiadlowski (2011) [16]. Similarly, Galactic field DNSs show the potential gaps of $P\sim 50$ ms and $P_{\mathrm{orb}}\sim 1$ day in Figure 1, as also being noticed by and Andrews and Mandel (2019) [46].

3.2. P Distribution

Figure 2a,b show the P distributions of Galactic BeXBs and Galactic field DNSs, respectively. It can be seen that Galactic BeXBs show a bi-modal feature in P distribution divided by $P\sim 40$ s. We apply double-Gaussian function to fit this P distribution, and obtain the fitted bi-modal peaks as $\mu \pm \sigma \sim 15.8\pm 2.5$ s and $\mu \pm \sigma \sim 199.5\pm 3.0$ s, respectively, (see

Table 2. Double-Gaussian function fittings for bi-modal BeXBs and DNSs.

BeXB a	P < 40 s	P > 40 s	Porb < 60 day	Porb > 60 day
$\mu \pm \sigma$	$\mathbf{15.8}\pm \mathbf{2.5}$ s	$\mathbf{199.5}\pm \mathbf{3.0}$ s	$\mathbf{28.2}\pm \mathbf{2.3}$ day	$\mathbf{151.4}\pm \mathbf{1.9}$ day
DNS b	$P<50$ ms	$P>50$ ms	$P_{\mathrm{orb}}<1$ day	$P_{\mathrm{orb}}>1$ day
$\mu \pm \sigma$	$\mathbf{31.6}\pm \mathbf{1.4}$ ms	$\mathbf{89.1}\pm \mathbf{1.9}$ ms	$\mathbf{0.3}\pm \mathbf{2.0}$ day	$\mathbf{5.6}\pm \mathbf{2.9}$ day
Scaled BeXB c	$P/800<50$ ms	$P/800>50$ ms	$P_{\mathrm{orb}}/60<1$ day	$P_{\mathrm{orb}}/60>1$ day
$\mu \pm \sigma$	$\mathbf{20.0}\pm \mathbf{2.5}$ ms	$\mathbf{251.2}\pm \mathbf{3.0}$ ms	$\mathbf{0.5}\pm \mathbf{2.3}$ day	$\mathbf{2.5}\pm \mathbf{1.9}$ day

^a The fitting data are from Figure 2a,c. ^b The fitting data are from Figure 2b,d. ^c The fitting data are from Figure 4a,c.

). Similarly, Galactic field DNSs also show a bi-modal feature in P distribution divided by $P\sim 50$ ms, and the double-Gaussian function fitting gives the bi-modal peaks as $\mu \pm \sigma \sim 31.6\pm 1.4$ ms and $\mu \pm \sigma \sim 89.1\pm 1.9$ ms, respectively, (see Table 2).

3.3. $P_{\mathrm{orb}}$ Distribution

Figure 2c,d show $P_{\mathrm{orb}}$ distributions of Galactic BeXBs and Galactic field DNSs. It can be seen that Galactic BeXBs show a bi-modal feature of $P_{\mathrm{orb}}$ distribution divided by $P_{\mathrm{orb}}\sim 60$ day. We apply double-Gaussian function to fit this $P_{\mathrm{orb}}$ distribution, and obtain the fitted bi-modal peaks as $\mu \pm \sigma \sim 28.2\pm 2.3$ days and $\mu \pm \sigma \sim 151.4\pm 1.9$ days, respectively, (see Table 2). Similarity, Galactic field DNSs also show a bi-modal feature of $P_{\mathrm{orb}}$ distribution divided by $P_{\mathrm{orb}}\sim 1$ day, and the double-Gaussian function fitting gives the bi-modal peaks as $\mu \pm \sigma \sim 0.3\pm 2.0$ days and $\mu \pm \sigma \sim 5.6\pm 2.9$ days, respectively, (see Table 2).

4. Spin and Orbital Evolution from BeXBs to DNSs

Theoretically, BeXBs are suggested as the progenitors of DNSs [8,20,24,25]. Then, the similar bi-modal classifications of the two types of sources may be the evidence for this evolutionary link, i.e., Galactic BeXBs transfer the bi-modal classification to Galactic field DNSs. Moreover, this evolution scenario also predicts the contraction of $P/P_{\mathrm{orb}}$ values from BeXBs to DNSs. Then, we try to study the evolutionary links between Galactic BeXBs and Galactic field DNSs by the P/$P_{\mathrm{orb}}$ relations.

4.1. $P-P_{\mathrm{orb}}$ Diagram

Figure 3 shows the $P-P_{\mathrm{orb}}$ diagram of Galactic BeXBs and Galactic field DNSs. Then, we infer the contraction factors of $P/P_{\mathrm{orb}}$ values between the two types of sources by the gaps of the bi-modal classifications: as the evolution from Galactic BeXBs to Galactic field DNSs, the gaps of $P\sim 40$ s/$P_{\mathrm{orb}}\sim 60$ days for bi-modal BeXBs transfer to the gaps of $P\sim 50 \mathrm{ms}$/$P_{\mathrm{orb}}\sim 1 \mathrm{day}$ for bi-modal DNSs, which infer the contraction factor of P values as $\alpha \sim {\displaystyle \frac{P\sim 50 \mathrm{ms}}{P\sim 40 \mathrm{s}}}\sim {\displaystyle \frac{1}{800}}$, and the contraction factor of $P_{\mathrm{orb}}$ values as $\beta \sim {\displaystyle \frac{P_{\mathrm{orb}}\sim 1 \mathrm{day}}{P_{\mathrm{orb}}\sim 60 \mathrm{days}}}\sim {\displaystyle \frac{1}{60}}$. We show the scaled P values (by $\sim {\displaystyle \frac{1}{800}}$) and scaled $P_{\mathrm{orb}}$ values (by $\sim {\displaystyle \frac{1}{60}}$) of Galactic BeXBs in Figure 3. It can be seen that the scaled $P-P_{\mathrm{orb}}$ relation of Galactic BeXBs share a similar range to Galactic field DNSs. Moreover, both scaled Galactic BeXBs and Galactic field DNSs show positive correlation trends in $P-P_{\mathrm{orb}}$ relation.

4.2. P Distribution

Figure 4a,b show the P distributions of Galactic BeXBs and Galactic field DNSs, respectively, in which the P values of Galactic BeXBs are scaled to ${\displaystyle \frac{1}{800}}$. Moreover, the double-Gaussian function fitting gives the bi-modal peaks of scaled P distributions for Galactic BeXBs as $\mu \pm \sigma \sim 20.0\pm 2.5$ ms and $\mu \pm \sigma \sim 251.2\pm 3.0$ ms, respectively, (see Table 2). It can be seen that scaled Galactic BeXBs and Galactic field DNSs exhibit the P distributions with the similar gaps of the bi-modal features.

4.3. $P_{\mathrm{orb}}$ Distribution

Figure 4c,d show the $P_{\mathrm{orb}}$ distribution of Galactic BeXBs and Galactic field DNSs, respectively, in which the $P_{\mathrm{orb}}$ values of Galactic BeXBs are scaled to ${\displaystyle \frac{1}{60}}$. Moreover, the double-Gaussian function fitting gives the bi-modal peaks of scaled $P_{\mathrm{orb}}$ distributions for Galactic BeXBs as $\mu \pm \sigma \sim 0.5\pm 2.3$ days and $\mu \pm \sigma \sim 2.5\pm 1.9$ days, respectively, (as can be seen in Table 2). It can be seen that scaled Galactic BeXBs and Galactic field DNSs exhibit the $P_{\mathrm{orb}}$ distributions with similar gaps and peaks in the bi-modal features.

5. Discussions and Conclusions

We the analyze P and $P_{\mathrm{orb}}$ distributions of Galactic BeXBs and Galactic field DNSs, and find similar bi-modal classifications between the two types of sources. These results may suggest an evolutionary link between Galactic BeXBs and Galactic field DNSs, which can further infer the spin and binary orbital evolution between them. The detailed discussions and conclusions are summarized as follows.

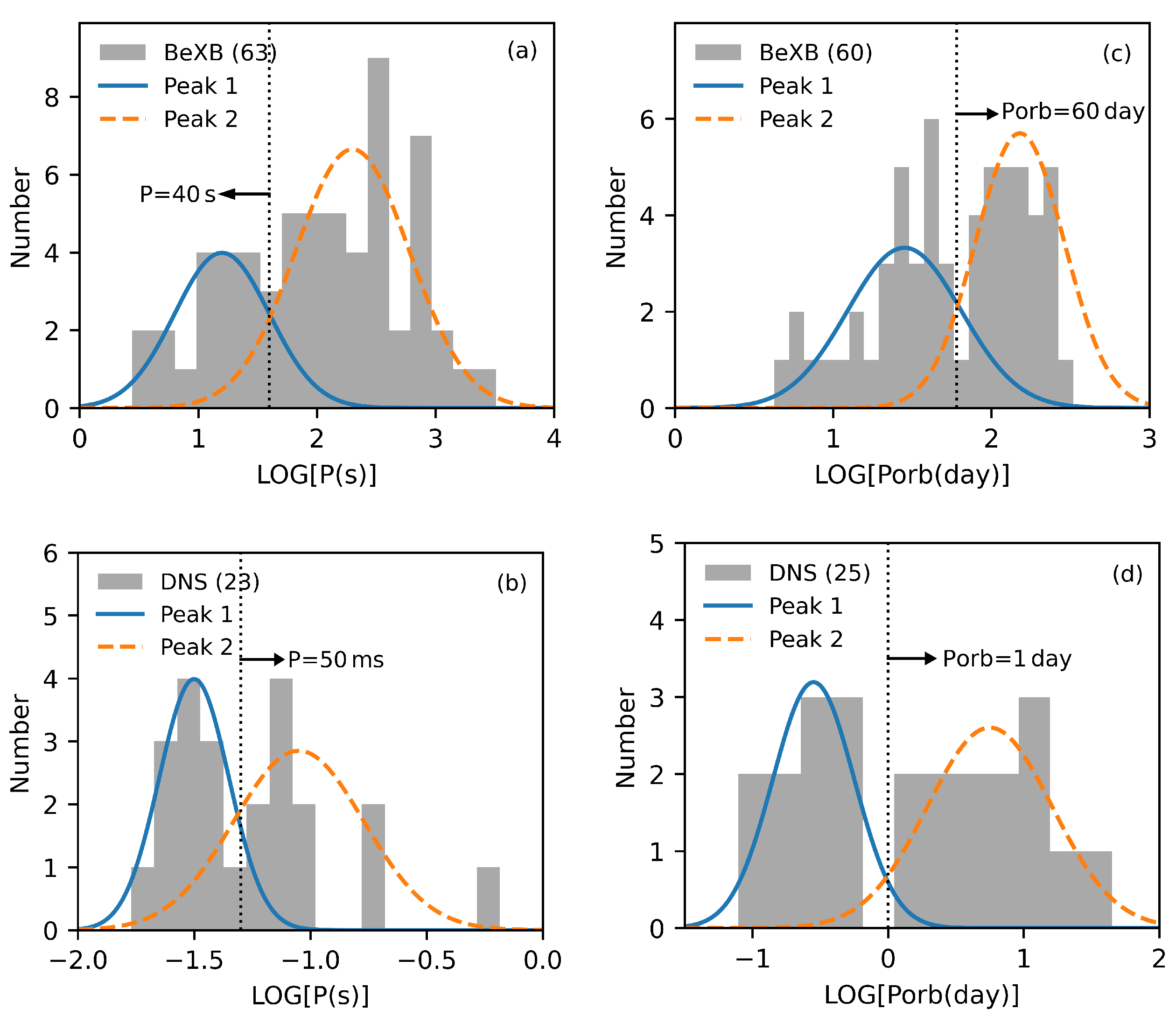
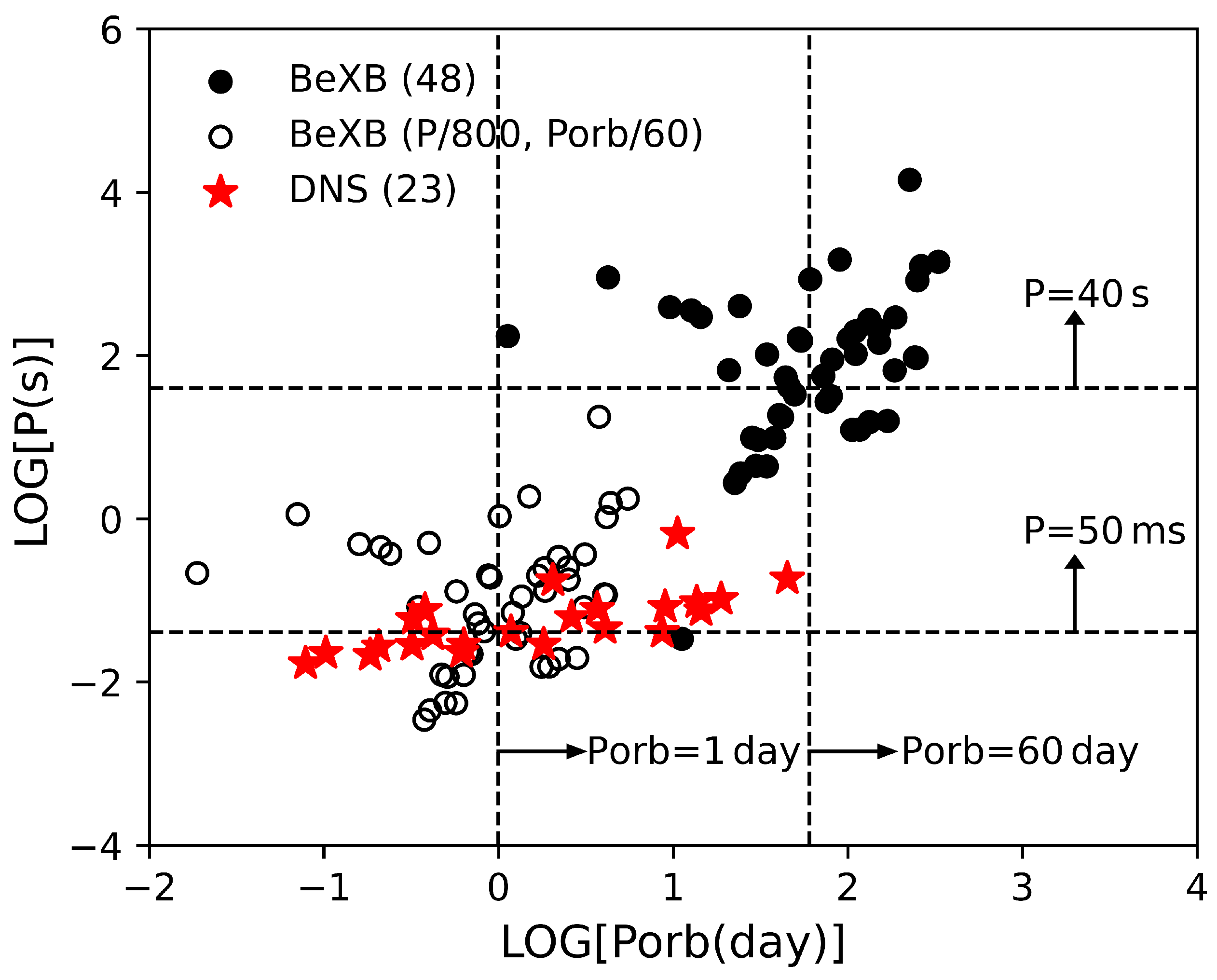
• Both Galactic BeXBs and Galactic field DNSs show the positive correlation trends in $P-P_{\mathrm{orb}}$ relation (see Figure 1), which may relate to the influence of accretion evolution, i.e., the shorter binary orbit may result in the more efficient angular momentum transfer as NS accretes the companion matter [15]. Moreover, the two types of sources also show similar bi-modal classifications in P or $P_{\mathrm{orb}}$ distributions (see Figure 2), which has also been noticed by Zhou et al. [50]. In theory, BeXBs are the young populations of NS-HMXBs with orbits which have not obviously evolved, and it is suggested that DNSs are the evolutionary products of NS-HMXBs [8,20,24,25]. Therefore, the similar $P-P_{\mathrm{orb}}$ relations and bi-modal $P/P_{\mathrm{orb}}$ classifications of the two types of sources support the evolution scenario from BeXBs to DNS. In fact, Zhou et al. [50] suggested the possibility that Galactic BeXBs may transfer the bi-modal $P/P_{\mathrm{orb}}$ classifications to DNSs during the accretion evolution process. Moreover, Zhou et al. [50] also indicated that, as the accretion effects can cause the spin-up of NS and the contraction of binary orbit [82,83,84,85], then BeXBs with shorter orbital periods will preferentially evolve into DNSs with faster spin periods and shorter orbits than those with longer orbits.
• It is noticed that the similar bi-modal P/$P_{\mathrm{orb}}$ distributions in BeXBs and DNSs may not only arise from an evolutionary relationship, but may also be affected by the influence from other processes, such as variations in initial conditions or differences in supernova mechanisms. For example, the supernova explosion of the donor stars may significantly affect the evolution from NS-HMXBs to DNSs [31]. First, a binary like NS-HMXBs remains bound only if less than half of the total mass of the system is explosively ejected in the supernova [27]. Secondly, a NS-HMXB will disrupt its orbit if the critical angle—${\theta }_{\mathrm{crit}}$ during the supernova satisfies $\theta <{\theta }_{\mathrm{crit}}$ [29]. Thirdly, the high-velocity kick of the donor star supernova in a NS-HMXB can lengthen the binary orbit and cause high eccentricity, or even disrupt the system [28]. However, it is noticed that a considerable fraction of DNSs is detected with quite low orbital eccentricities, implying that the second-born NSs seem to receive on average smaller kicks at birth [20]. In fact, it is theoretically suggested that these low-velocity kick NSs may either have been formed by electron-capture collapse, or by the collapse of ultra-stripped iron cores with a small kick formed though the Case BB Roche-lobe overflow process [20,30]. Therefore, compared to the accretion evolution process, these low-energy supernova with low-velocity kicks of the progenitors of the second-born NSs may be not the dominated factors for the formation of the bi-modal DNSs.
• The common envelope phase is another process that may significantly affect the evolution from BeXBs to DNSs [86]. Theoretically, in the latter evolution of a NS-HMXB, the unstable Roche lobe overflow of the companion can engulf NS and form a common envelope, and it is suggested that the formation of DNSs may have experienced at least one common envelope episode during their progenitor evolution [5,9,34]. The models of a common envelope usually consider the interaction between the binary and envelope, and predict several possible phases, e.g., the plunge-in, based on the multiple physical processes on various of timescales [35,36]. However, the predicted common envelope phase has not been observed until now, which may be due to the short duration time of the envelope phase (∼${10}^3$ y, see, e.g., Meurs and van den Heuvel (1989) [87]) compared to the lifetime of a massive star (∼${10}^6–{10}^7$ y, see, e.g., Chaty (2013) [5]). Moreover, the current simulations of the common envelope phase cannot give the consensus on a thorough understanding of this evolution on all the relevant spatial and timescales [26,34,35,36]. As for the evolution from NS-HMXBs to DNSs, Taam (1996) [88] and Taam and Sandquist (2000) [32] suggested that only a NS-HMXB starting out with the long orbit can survive the common envelope evolution and may then form a DNS. On the contrary, a NS-HMXB with the short initial orbit will finally lead to the merge of the binary into a single Thorne–Zytkow star [32]. However, the predicted Thorne–Zytkow star has not been observed until now [8]. Meanwhile, it is indicated by Taam and Sandquist (2000) [32] that, if the envelope matter is ejected out from the system during the common envelope stage due to the binary interaction, the system can also form a DNS with a short binary orbit. Therefore, both NS-HMXBs with short or long orbits have the opportunity to survive and form a DNS if the envelope matter is effectively ejected out from the system.
• It should be noted that potential uncertainties introduced from the sample selections may affect our conclusions. First, we only analyze BeXBs in the Milky Way, and do not consider the sources in the large Magellanic cloud or small Magellanic cloud, which are based on the following considerations: (I). Knigge et al. [16] and Zhou et al. [50] indicated that all three BeXB groups, i.e., those detected in the Milky Way, large Magellanic clouds, and small Magellanic clouds, exhibit positive correlations in the $P-P_{\mathrm{orb}}$ relation, as well as the similar bi-modal classifications in P and $P_{\mathrm{orb}}$ distributions. (II). The detected DNSs at present are all discovered in the Milky Way, and then the evolution analysis between BeXBs and DNSs should be constrained in the same host galaxy to maintain consistency. Secondly, as for DNSs, we only retain the sources in the Galactic field of the Milky Way, and discard those in the global clusters. The reason is that cluster DNSs constitute most candidates, and are suggested to have experienced more complicated formation and evolution processes than those in the Galactic field. Finally, it is also noticed that there are selection biases in the detections of binary orbital periods of BeXBs, since, for the longer orbit sources, the X-ray outbursts are less frequent, and the pulse timing is not easy to implement [89].
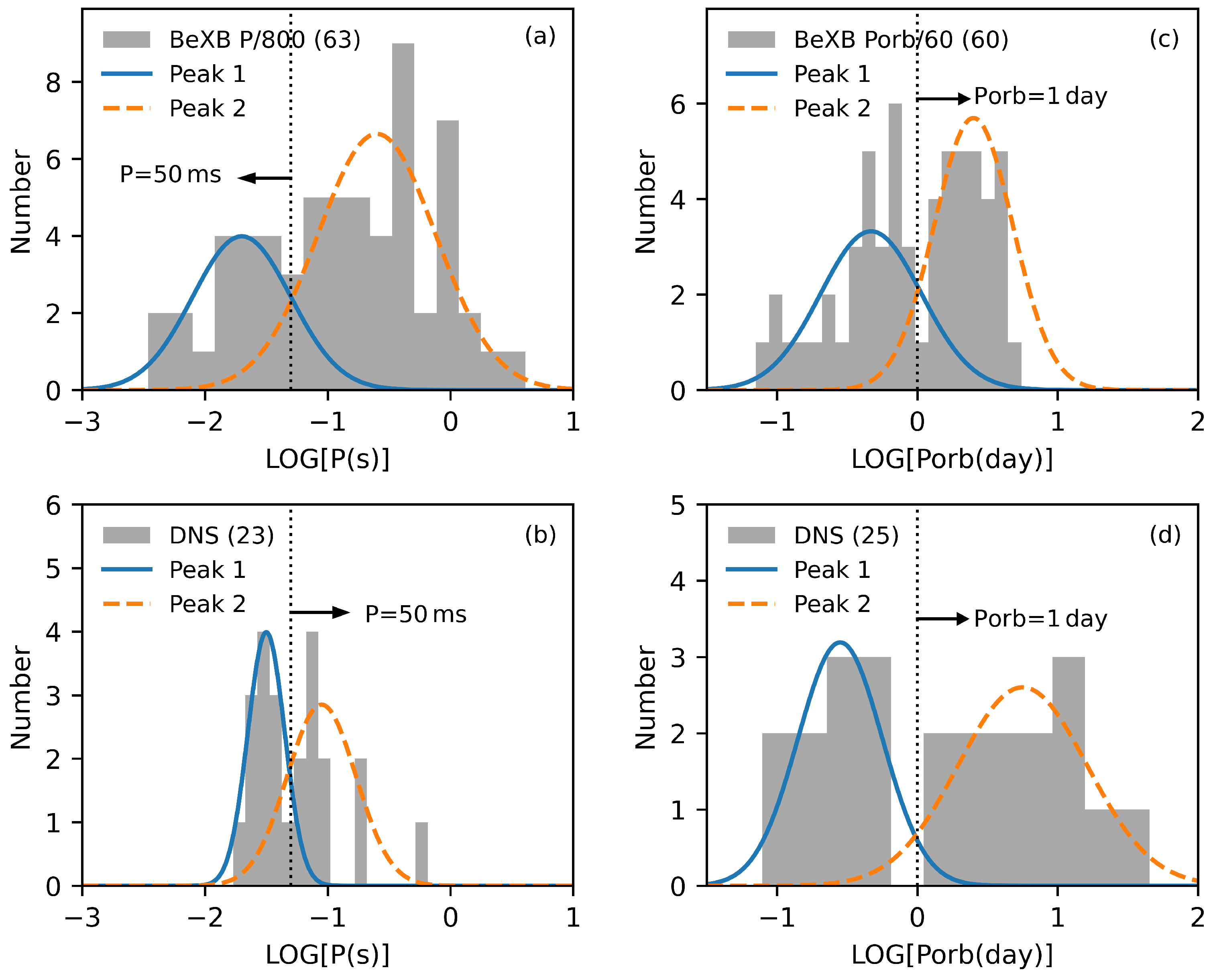
• In order to explore the evolutionary link between BeXBs and DNSs, we assume that Galactic BeXBs transfer the bi-modal P/$P_{\mathrm{orb}}$ classifications to Galactic field DNSs through the accretion process. Moreover, we also assume that the Galactic BeXB group that corresponds to the short orbit sources ($P_{\mathrm{orb}}<60$ days) of bi-modal classifications will preferentially evolve into the Galactic field DNS group that corresponds to the sources with a fast spin ($P<50$ ms) and short orbit ($P_{\mathrm{orb}}<1$ day) in the bi-modal classifications. Furthermore, we infer the spin (P values are scaled by ${\displaystyle \frac{1}{800}}$) and orbital ($P_{\mathrm{orb}}$ values are scaled by ${\displaystyle \frac{1}{60}}$) contraction factors, which are inferred by the gaps ($P\sim 40$ s and $P_{\mathrm{orb}}\sim 60$ days for Galactic BeXBs, and $P\sim 50$ ms and $P_{\mathrm{orb}}\sim 1$ day for Galactic field DNSs, as can be seen in Figure 2) showing the bi-modal classifications of the two types of sources. It can be seen from Figure 3 that the scaled P and $P_{\mathrm{orb}}$ values of Galactic BeXBs and Galactic field DNSs show similar positive correlation trends in the $P-P_{\mathrm{orb}}$ relation. In addition, it can be also noticed from Figure 4 and Table 2 that the scaled P and $P_{\mathrm{orb}}$ values of Galactic BeXBs share the similar bi-modal distributions to those of Galactic field DNSs. It is noticed that these contraction factors of P/$P_{\mathrm{orb}}$ values are derived in a statistical way, which may cause uncertainties due to the limit of the sample numbers. In fact, it can be seen from Figure 4a,b and Table 2 that the two peaks of bi-modal P distributions of scaled Galactic BeXBs and Galactic field DNSs are not quite symmetric, which may be due to influence of the binary accretion-induced spin-up evolution. Moreover, the contraction of NS spin and binary orbit from Galactic BeXBs to Galactic field DNSs may be also affected by other physical factors. In fact, it is indicated by Zhou et al. [50] that BeXBs with short orbits of $P_{\mathrm{orb}}<60$ days on average share faster NS spin periods, lower NS magnetic field strengths [18], lower binary orbital eccentricities, and more high energy outbursts (Type II outbursts) with higher average peak X-ray luminosities than those sources with longer orbits of $P_{\mathrm{orb}}>60$ days. Moreover, Zhou et al. [50] also indicated that DNSs with short orbits of $P_{\mathrm{orb}}<1$ day on average share faster NS spin periods, higher spin-down powers, and higher binary accretion rates than those sources with longer orbits of $P_{\mathrm{orb}}>1$ day. Therefore, the potential evolution relation and channels from BeXBs to DNSs may need further observation confirmation and theoretical analysis, e.g., the larger samples, and the detailed modeling of binary evolution. However, the inferred contraction factors of P and $P_{\mathrm{orb}}$ can offer a reference for the binary evolution from BeXBs and DNSs, which can also offer a tool to trace the classification of the two types of sources.