Imaging Analyses for Pion and Kaon Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model
Abstract
1. Introduction
2. Imaging Technique
3. Imaging Results for Heavy-Ion Collisions Using the Ampt Model at the LHC
3.1. Calculations of Correlation Functions Using the Ampt Model
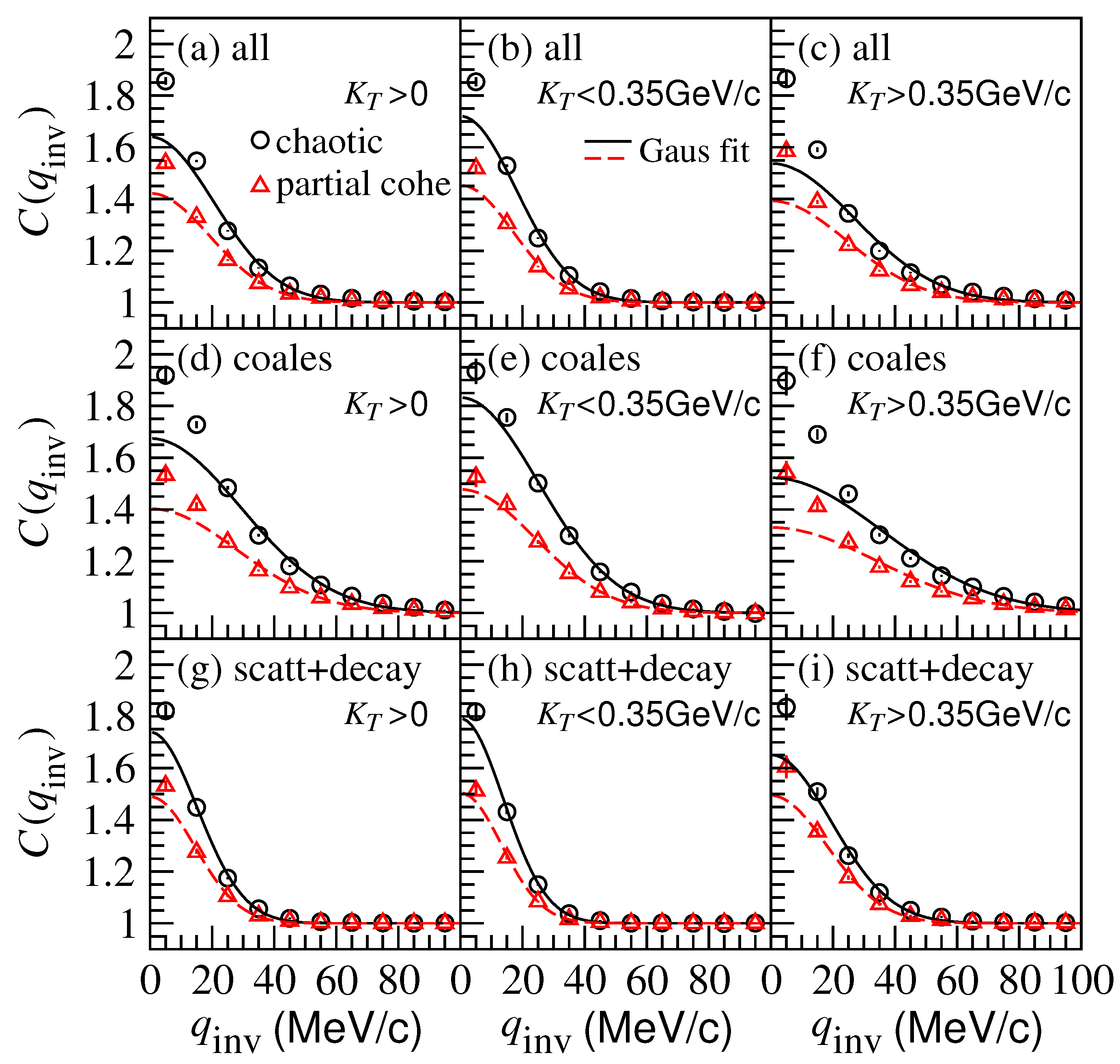
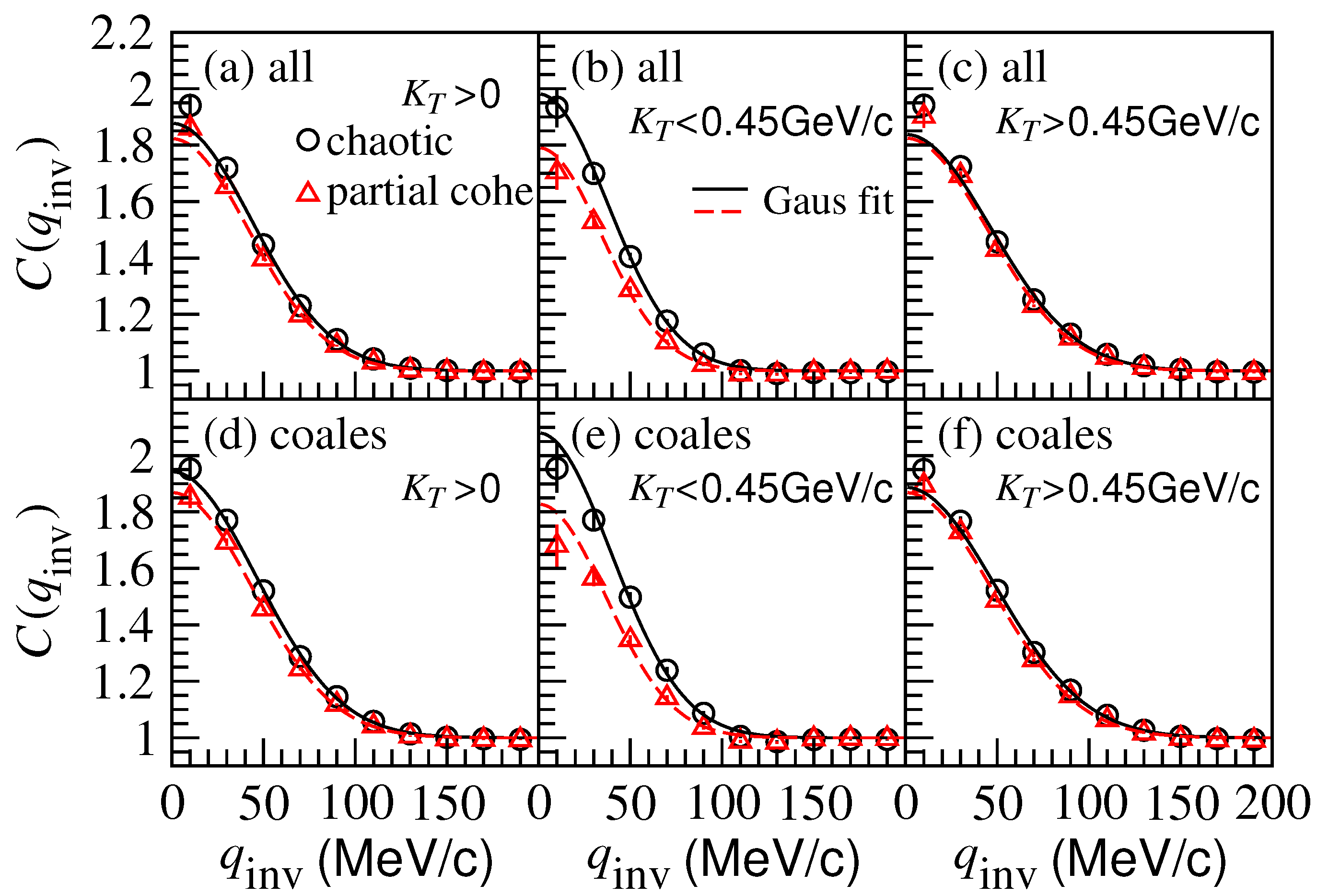
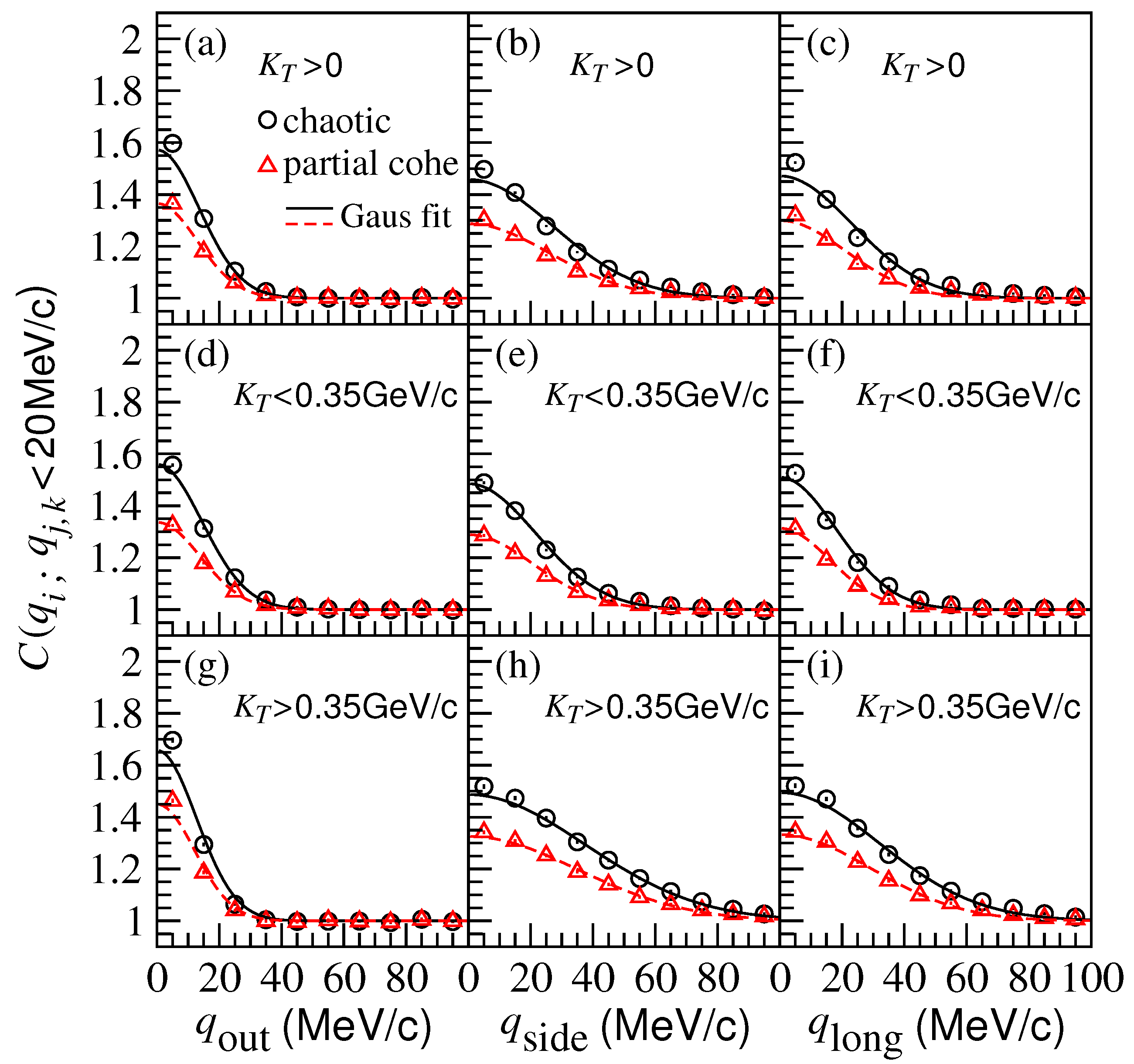
3.2. One-Dimension Results
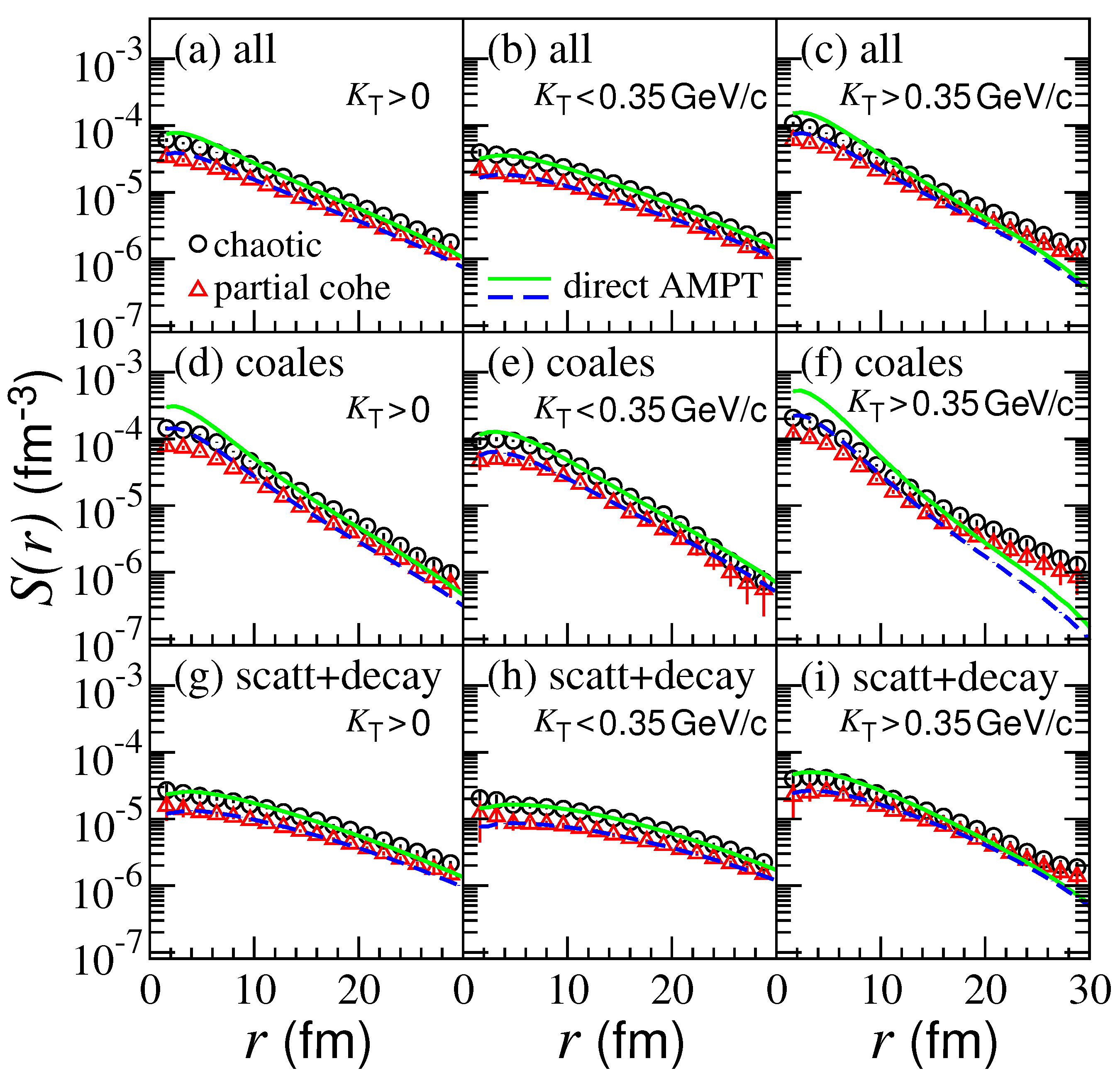
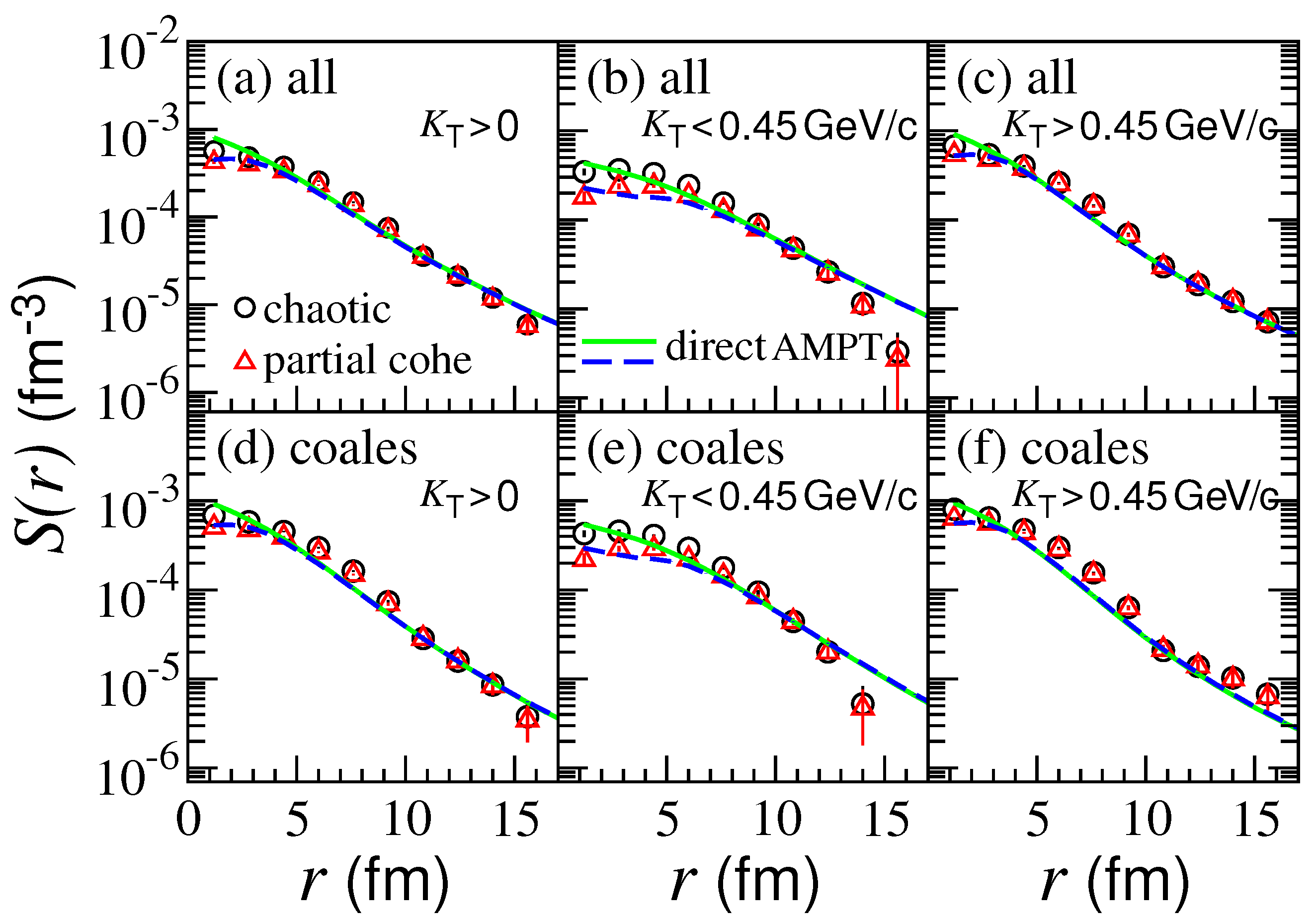
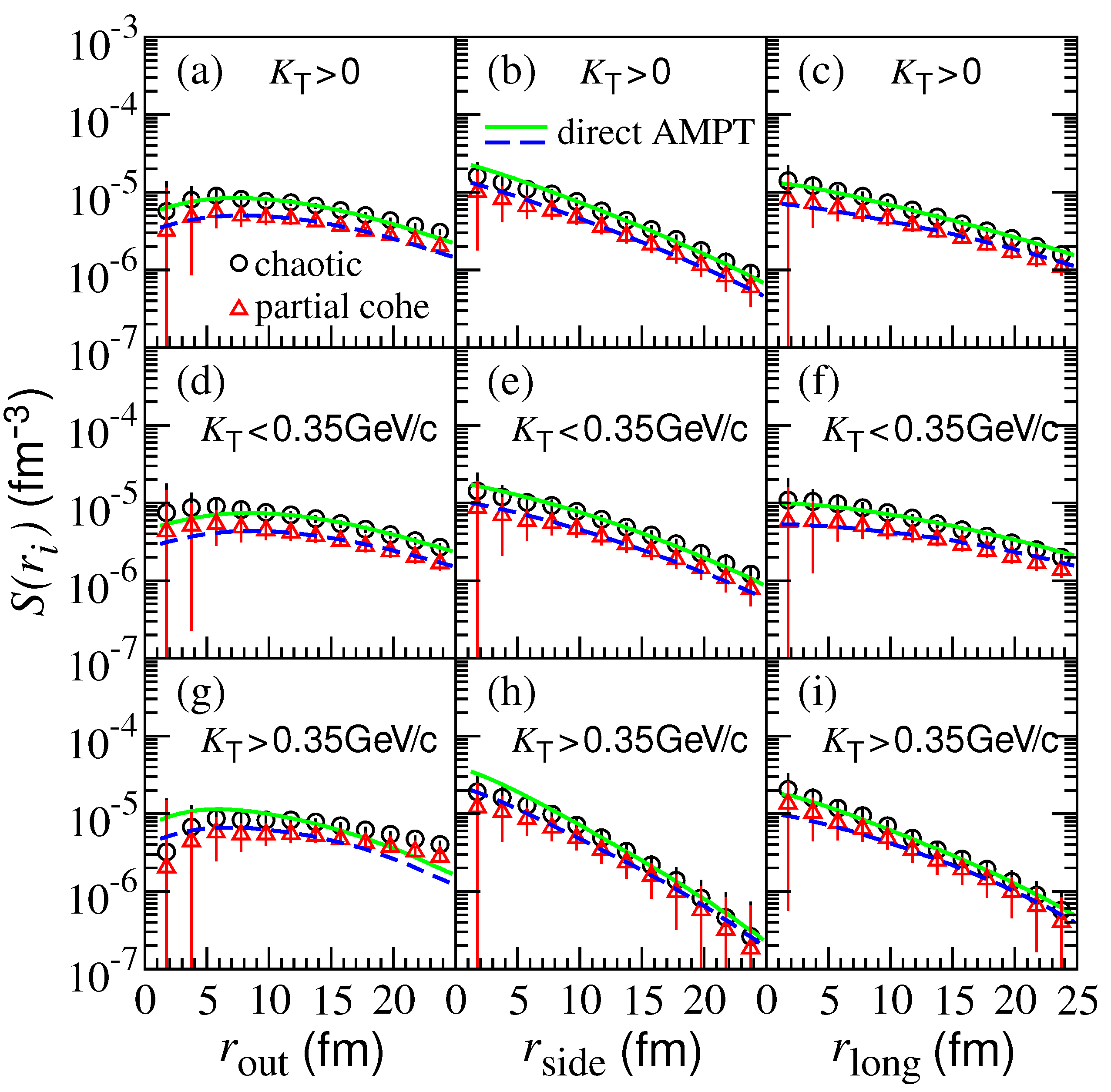
3.3. Three-Dimension Results
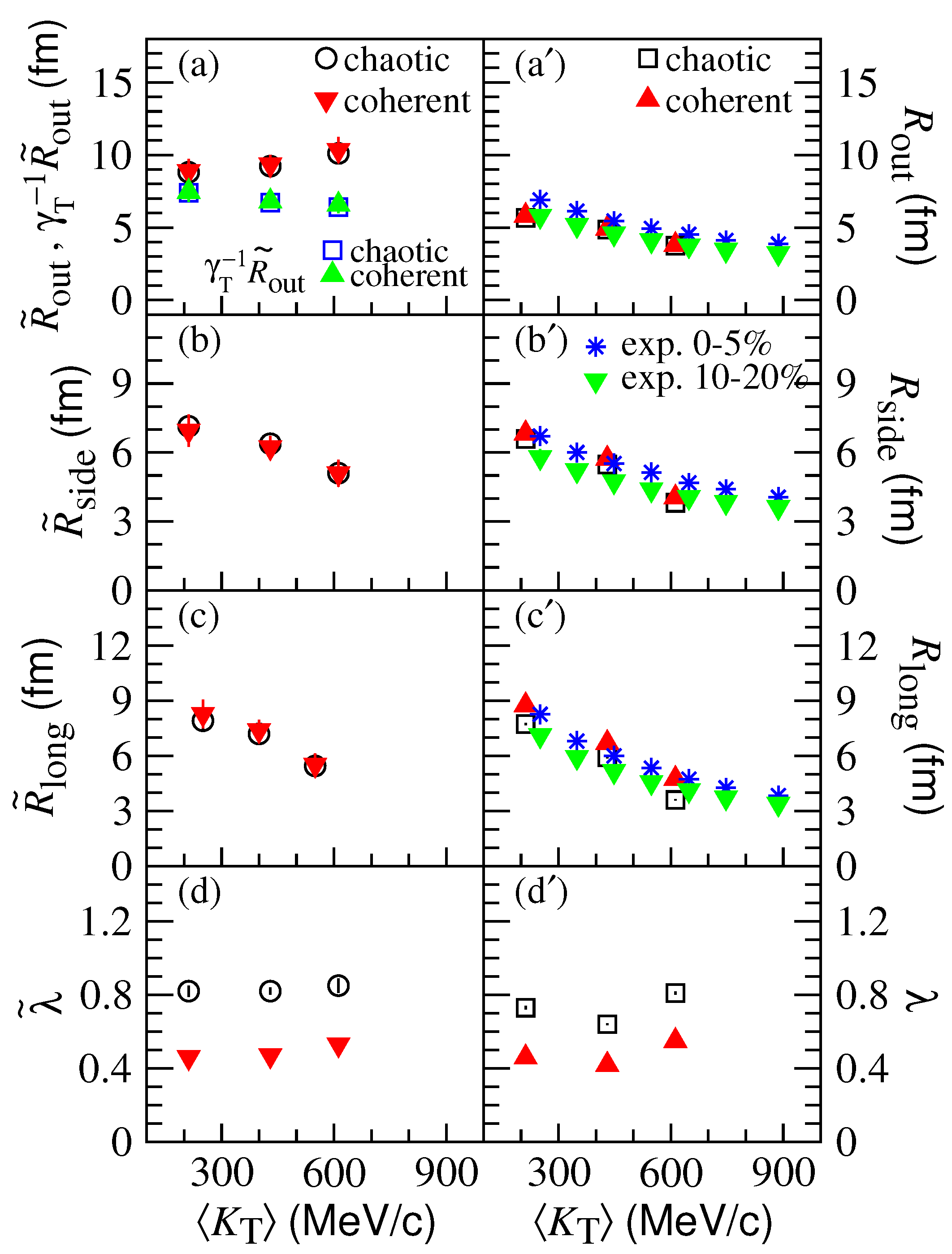
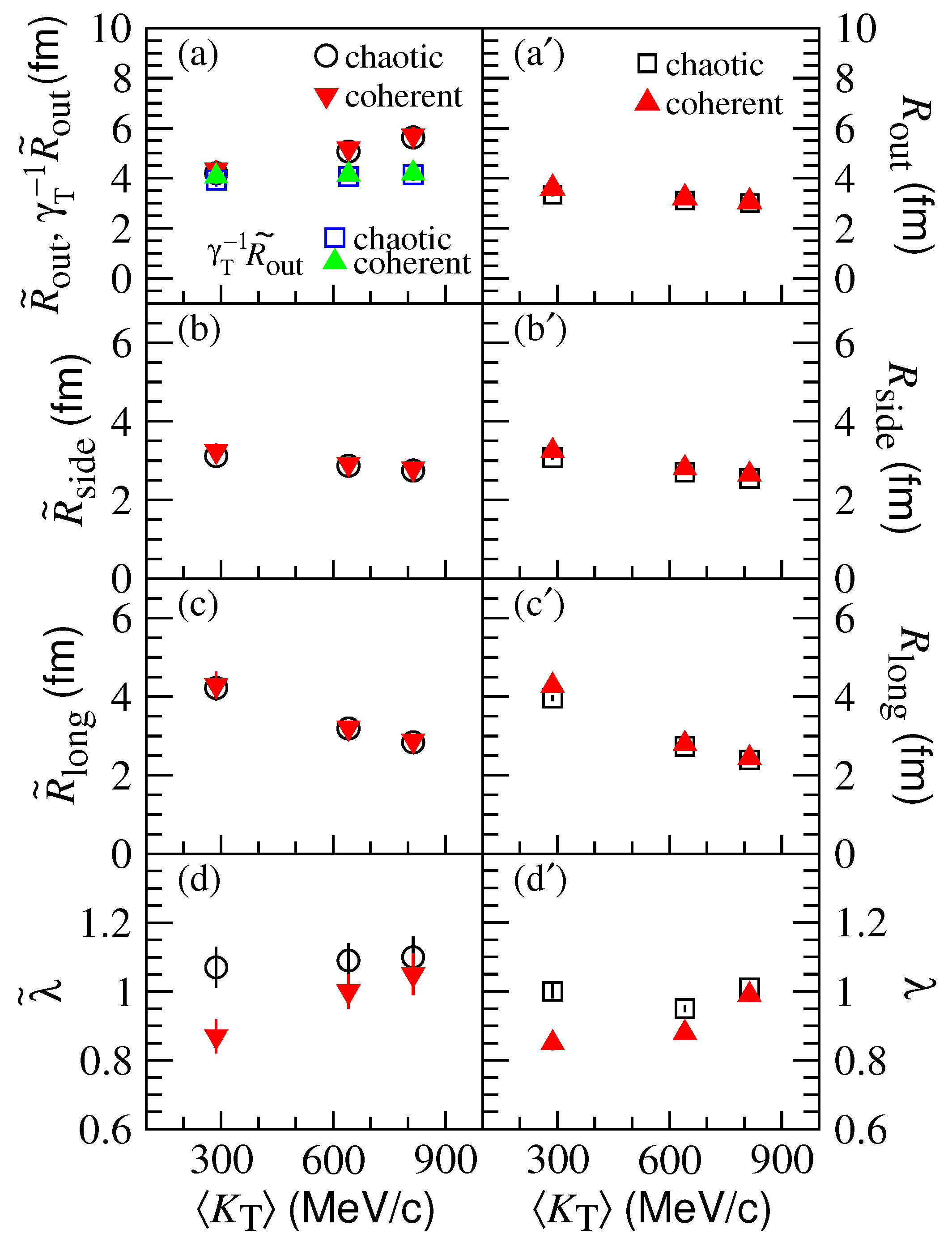
3.4. Characteristic Source Function Quantities
4. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gyulassy, M.; Kauffmann, S.K.; Wilson, L.W. Pion Interferometry of Nuclear Collisions. 1. Theory. Phys. Rev. C 1979, 20, 2267. [Google Scholar] [CrossRef]
- Wong, C.Y. Introduction to High-Energy Heavy-Ion Collisions; World Scientific: Singapore, 1994; Chapter 17. [Google Scholar]
- Wiedemann, U.A.; Heinz, U. Particle Interferometry for Relativistic Heavy-Ion Collisions. Phys. Rep. 1999, 319, 145. [Google Scholar] [CrossRef]
- Weiner, R.M. Boson Interferometry in High-Energy Physics. Phys. Rep. 2000, 327, 249. [Google Scholar] [CrossRef]
- Csörgő, T. Particle Interferometry from 40 MeV to 40 TeV. Heavy Ion Phys. 2002, 15, 1, arXiv:hep-ph/0001233. [Google Scholar] [CrossRef]
- Lisa, M.A.; Pratt, S.; Soltz, R.; Wiedemann, U. Femtoscopy in Relativistic Heavy Ion Collisions: Two Decades of Progress. Annu. Rev. Nucl. Part. Sci. 2005, 55, 357. [Google Scholar] [CrossRef]
- Adler, C. et al. [STAR Collaboration] Pion Interferometry of = 130 GeV Au+Au Collisions at RHIC. Phys. Rev. Lett. 2001, 87, 082301. [Google Scholar] [CrossRef] [PubMed]
- Adler, S.S. et al. [PHENIX Collaboration] Bose-Einstein Correlations of Charged Pion Pairs in Au+Au Collisions at = 200 GeV. Phys. Rev. Lett. 2004, 93, 152302. [Google Scholar] [CrossRef] [PubMed]
- Adams, J. et al. [STAR Collaboration] Pion Interferometry in Au+Au Collisions at = 200 GeV. Phys. Rev. C 2005, 71, 044906. [Google Scholar] [CrossRef]
- Aamodt, K. et al. [ALICE Collaboration] Two-Pion Bose-Einstein Correlations in Central Pb-Pb Collisions at = 2.76 TeV. Phys. Lett. B 2011, 696, 328. [Google Scholar] [CrossRef]
- Abelev, B. et al. [ALICE Collaboration] Two- and Three-Pion Quantum Statistics Correlations in Pb-Pb Collisions at = 2.76 TeV at the CERN Large Hadron Collider. Phys. Rev. C 2014, 89, 024911. [Google Scholar] [CrossRef]
- Adamczky, L. et al. [STAR Collaboration] Beam-Energy-Dependent Two-Pion Interferometry and the Freeze-out Eccentricity of Pions Measured in Heavy Ion Collisions at the STAR Detector. Phys. Rev. C 2015, 92, 014904. [Google Scholar] [CrossRef]
- Adam, J. et al. [ALICE Collaboration] One-Dimensional Pion, Kaon, and Proton Femtoscopy in Pb-Pb Collisions at = 2.76 TeV. Phys. Rev. C 2015, 92, 054908. [Google Scholar] [CrossRef]
- Adam, J. et al. [ALICE Collaboration] Centrality Dependence of Pion Freeze-out Radii in Pb-Pb Collisions at = 2.76 TeV. Phys. Rev. C 2016, 93, 024905. [Google Scholar] [CrossRef]
- Brown, D.A.; Danielewicz, P. Imaging of Sources in Heavy-Ion Reactions. Phys. Lett. B 1997, 398, 252. [Google Scholar] [CrossRef]
- Brown, D.A.; Danielewicz, P. Optimized Discretization of Sources Imaged in Heavy-Ion Reactions. Phys. Rev. C 1998, 57, 2474. [Google Scholar] [CrossRef]
- Brown, D.A.; Danielewicz, P. Observing Non-Gaussian Sources in Heavy-Ion Reactions. Phys. Rev. C 2001, 64, 014902. [Google Scholar] [CrossRef]
- Panitkin, S.Y. et al. [E895 Collaboration] Model-Independent Source Imaging Using Two-Pion Correlations in (2 to 8)A GeV Au+Au Collisions. Phys. Rev. Lett. 2001, 87, 112304. [Google Scholar] [CrossRef] [PubMed]
- Chung, P. et al. [E895 Collaboration] Comparison of Source Images for Protons, π−’s, and Λ’s in 6A GeV Au+Au Collisions. Phys. Rev. Lett. 2003, 91, 162301. [Google Scholar] [CrossRef] [PubMed]
- Chung, P.; [for PHENIX Collaboration]. Evidence for a Long Range Structure in the Pion Emission Source in Au+Au Collisions at RHIC. Nucl. Phys. A 2006, 774, 607. [Google Scholar] [CrossRef][Green Version]
- Adler, S.S. et al. [PHENIX Collabortion] Evidence for a Long-Range Component in the Pion Emission Source in Au+Au Collisions at = 200 GeV. Phys. Rev. Lett. 2007, 98, 132301. [Google Scholar] [CrossRef]
- Chung, P.; [for PHENIX Collaboration]. Three-Dimensional Two-Pion Emission Source at RHIC-PHENIX. J. Phys. G 2008, 35, 044034. [Google Scholar] [CrossRef]
- Lacey, R.A.; [for PHENIX Collaboration]. PHENIX Measurements of 3D Emission Source Functions in Au+Au Collisions at = 200 GeV. J. Phys. G 2008, 35, 104139. [Google Scholar] [CrossRef][Green Version]
- Afanasiev, S. et al. [PHENIX Collaboration] Source Breakup Dynamics in Au+Au Collisions at = 200 GeV via Three-Dimensional Two-Pion Source Imaging. Phys. Rev. Lett. 2008, 100, 232301. [Google Scholar] [CrossRef]
- Yang, Z.T.; Zhang, W.N.; Huo, L.; Zhang, J.B. Imaging of Granular Sources in High Energy Heavy Ion Collisions. J. Phys. G 2009, 36, 015113. [Google Scholar] [CrossRef]
- Zhang, W.N.; Yang, Z.T.; Ren, Y.Y. Characteristic Quantities of Pion-Emitting Sources Extracted by Model-Independent Analysis in Relativistic Heavy Ion Collisions. Phys. Rev. C 2009, 80, 044908. [Google Scholar] [CrossRef]
- Wang, S.Y.; Ye, J.T.; Zhang, W.N. Two-Pion Interferometry for Partially Coherent Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model. Phys. Rev. C 2024, 109, 014912. [Google Scholar] [CrossRef]
- Lin, Z.W.; Ko, C.M. Partonic Effects on the Elliptic Flow at Relativistic Heavy Ion Collisions. Phys. Rev. C 2002, 65, 034904. [Google Scholar] [CrossRef]
- Lin, Z.W.; Ko, C.M.; Li, B.A.; Zhang, B.; Pal, S. Multiphase Transport Model for Relativistic Heavy Ion Collisions. Phys. Rev. C 2005, 72, 064901. [Google Scholar] [CrossRef]
- Wang, S.Y.; Ye, J.T.; Zhang, W.N. Multiboson Hanbury-Brown-Twiss Correlations for Partially Coherent Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Mode. Phys. Rev. C 2024, 110, 054907. [Google Scholar] [CrossRef]
- Koonin, S.E. Proton Pictures of High-energy Nuclear Collisions. Phys. Lett. B 1977, 70, 43. [Google Scholar] [CrossRef]
- Pratt, S.; Csörgő, T.; Zimányi, J. Detailed Predictions for Two-Pion Correlations in Ultrarelativistic Heavy-Ion Collisions. Phys. Rev. C 1990, 42, 2646. [Google Scholar] [CrossRef]
- Brown, D.A.; Panitkin, S.Y.; Bertsch, G.F. Extracting Particle Freeze-out Phase-Space Densities and Entropies from Sources Imaged in Heavy-Ion Reactions. Phys. Rev. C 2000, 62, 014904. [Google Scholar] [CrossRef]
- Brown, D.A.; Enokizono, A.; Heffner, M.; Soltz, R.; Danielewicz, P.; Pratt, S. Imaging Three Dimensional Two-Particle Correlations for Heavy-Ion Reaction Studies. Phys. Rev. C 2005, 72, 054902. [Google Scholar] [CrossRef]
- Nasim, M.; Kumar, L.; Kumar, P.; Mohanty, B. Energy Dependence of Elliptic Flow from Heavy-Ion Collision Models. Phys. Rev. C 2010, 82, 054908. [Google Scholar] [CrossRef]
- Xu, J.; Ko, C.M. Effects of Triangular Flow on Di-Hadron Azimuthal Correlations in Relativistic Heavy Ion Collisions. Phys. Rev. C 2011, 83, 021903(R). [Google Scholar] [CrossRef]
- Solanki, D.; Sorensen, P.; Basu, S.; Raniwala, R.; Nayak, T.K. Beam Energy Dependence of Elliptic and Triangular Flow with the AMPT Model. Phys. Lett. B 2013, 720, 352. [Google Scholar] [CrossRef]
- Bzdak, A.; Ma, G.L. Elliptic and Triangular Flow in p-Pb and Peripheral Pb-Pb Collisions from Parton Scatterings. Phys. Rev. Lett. 2014, 113, 252301. [Google Scholar] [CrossRef] [PubMed]
- Ma, G.L.; Lin, Z.W. Predictions for = 5.02 TeV Pb+Pb Collisions from a Multiphase Transport Model. Phys. Rev. C 2016, 93, 054911. [Google Scholar] [CrossRef]
- Li, H.; He, L.; Lin, Z.W.; Molnar, D.; Wang, F.; Xie, W. Origin of the Mass Splitting of Azimuthal Anisotropies in a Multiphase Transport Model. Phys. Rev. C 2017, 96, 014901. [Google Scholar] [CrossRef]
- Haque, M.R.; Nasim, M.; Mohanty, B. Systematic Investigation of Azimuthal Anisotropy in Au+Au and U+U Collisions at = 200 GeV. J. Phys. G 2019, 46, 085104. [Google Scholar] [CrossRef]
- Dordevic, M.; Milosevic, J.; Nadderd, L.; Stojanovic, M.; Wang, F.; Zhu, X. Correlations between Azimuthal Anisotropy Fourier Harmonics in Pb-Pb Collisions at = 2.76 TeV in the HYDJET++ Model and in the Multiphase Transport Model. Phys. Rev. C 2020, 101, 014908. [Google Scholar] [CrossRef]
- Shao, T.; Chen, J.; Ko, C.M.; Lin, Z.W. Enhanced Production of Strange Baryons in High-Energy Nuclear Collisions from a Multiphase Transport Model. Phys. Rev. C 2020, 102, 014906. [Google Scholar] [CrossRef]
- Sun, K.J.; Ko, C.M. Light Nuclei Production in a Multiphase Transport Model for Relativistic Heavy Ion Collisions. Phys. Rev. C 2021, 103, 064909. [Google Scholar] [CrossRef]
- Magdy, N.; Lacey, R.A. Model Investigation of the Longitudinal Broadening of the Transverse Momentum Two-Particle Correlator. Phys. Rev. C 2021, 104, 014907. [Google Scholar] [CrossRef]
- Basu, S.; Gonzalez, V.; Pan, J.; Knospe, A.; Marin, A.; Markert, C.; Pruneau, C. Differential Two-Particle Number and Momentum Correlations with the AMPT, UrQMD, and EPOS Models in Pb-Pb Collisions at = 2.76 TeV. Phys. Rev. C 2021, 104, 064902. [Google Scholar] [CrossRef]
- Magdy, N.; Evdokimov, O.; Lacey, R.A. A Method to Test the Coupling Strength of the Linear and Nonlinear Contributions to Higher-Order Flow Harmonics via Event Shape Engineering. J. Phys. G 2021, 48, 025101. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, N.; Xu, H. Neutron Density Fluctuation and Neutron-Proton Correlation from AMPT Model. Eur. Phys. J. A 2022, 58, 240. [Google Scholar] [CrossRef]
- Wang, X.N.; Gyulassy, M. Hijing: A Monte Carlo Model for Multiple Jet Production in pp, pA, and AA Collisions. Phys. Rev. D 1991, 44, 3501. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B. ZPC 1.0.1: A Parton Cascade for Ultrarelativistic Heavy Ion Collisions. Comput. Phys. Commun. 1998, 109, 193. [Google Scholar] [CrossRef]
- Li, B.A.; Ko, C.M. Formation of Superdense Hadronic Matter in High Energy Heavy-Ion Collisions. Phys. Rev. C 1995, 52, 2037. [Google Scholar] [CrossRef]


| Chaotic Source | Partially Coherent Source | |||||
|---|---|---|---|---|---|---|
| (fm) | (fm) | (fm) | (fm) | |||
| pion, GeV | 0.82 ± 0.03 | 8.21 ± 0.62 | 8.18 ± 0.34 | 0.46 ± 0.02 | 7.98 ± 0.87 | 7.88 ± 0.45 |
| pion, GeV | 0.85 ± 0.04 | 7.04 ± 0.74 | 7.21 ± 0.43 | 0.53 ± 0.03 | 6.82 ± 0.88 | 6.89 ± 0.49 |
| pion, | 0.82 ± 0.02 | 7.80 ± 0.48 | 7.84 ± 0.26 | 0.47 ± 0.02 | 7.53 ± 0.63 | 7.50 ± 0.33 |
| kaon, GeV | 1.07 ± 0.06 | 3.61 ± 0.46 | 3.56 ± 0.26 | 0.87 ± 0.05 | 3.73 ± 0.49 | 3.66 ± 0.26 |
| kaon, GeV | 1.10 ± 0.06 | 3.49 ± 0.45 | 3.57 ± 0.29 | 1.05 ± 0.06 | 3.55 ± 0.46 | 3.61 ± 0.29 |
| kaon, | 1.09 ± 0.05 | 3.55 ± 0.37 | 3.58 ± 0.23 | 1.00 ± 0.05 | 3.62 ± 0.40 | 3.65 ± 0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.-Y.; Ren, Y.-Y.; Zhang, W.-N. Imaging Analyses for Pion and Kaon Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model. Universe 2025, 11, 31. https://doi.org/10.3390/universe11020031
Wang S-Y, Ren Y-Y, Zhang W-N. Imaging Analyses for Pion and Kaon Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model. Universe. 2025; 11(2):31. https://doi.org/10.3390/universe11020031
Chicago/Turabian StyleWang, Shi-Yao, Yan-Yu Ren, and Wei-Ning Zhang. 2025. "Imaging Analyses for Pion and Kaon Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model" Universe 11, no. 2: 31. https://doi.org/10.3390/universe11020031
APA StyleWang, S.-Y., Ren, Y.-Y., & Zhang, W.-N. (2025). Imaging Analyses for Pion and Kaon Sources in Relativistic Heavy-Ion Collisions in a Multiphase Transport Model. Universe, 11(2), 31. https://doi.org/10.3390/universe11020031





