Dynamical Analysis of the Redshift Drift in FLRW Universes
Abstract
1. Introduction
2. Dynamics of the RD in Terms of
- (i)
- The second-order Raychaudhuri equation (or second Friedmann equation):where and . Here, and p are the energy density and the pressure of the matter content of the universe, respectively, described by an isotropic perfect fluid. Note that we have adopted the simplification of absorbing the cosmological constant, if present, into the stress-energy tensor.
- (ii)
- The first Friedmann equation:The latter equation acts as a first integral of Equation (6) and constrains the solutions in connection with the possible spatial curvature cases set by .
2.1. Choosing a Specific Cosmological Model
2.1.1. Generic Model
2.1.2. -CDM
2.1.3. -CDM (Simplified Two-Component Version)
2.1.4. The Relation between , , and
2.1.5. Clarifying a Potential Mis-Application to the CMB
2.1.6. Higher-Order RD
2.2. Summary
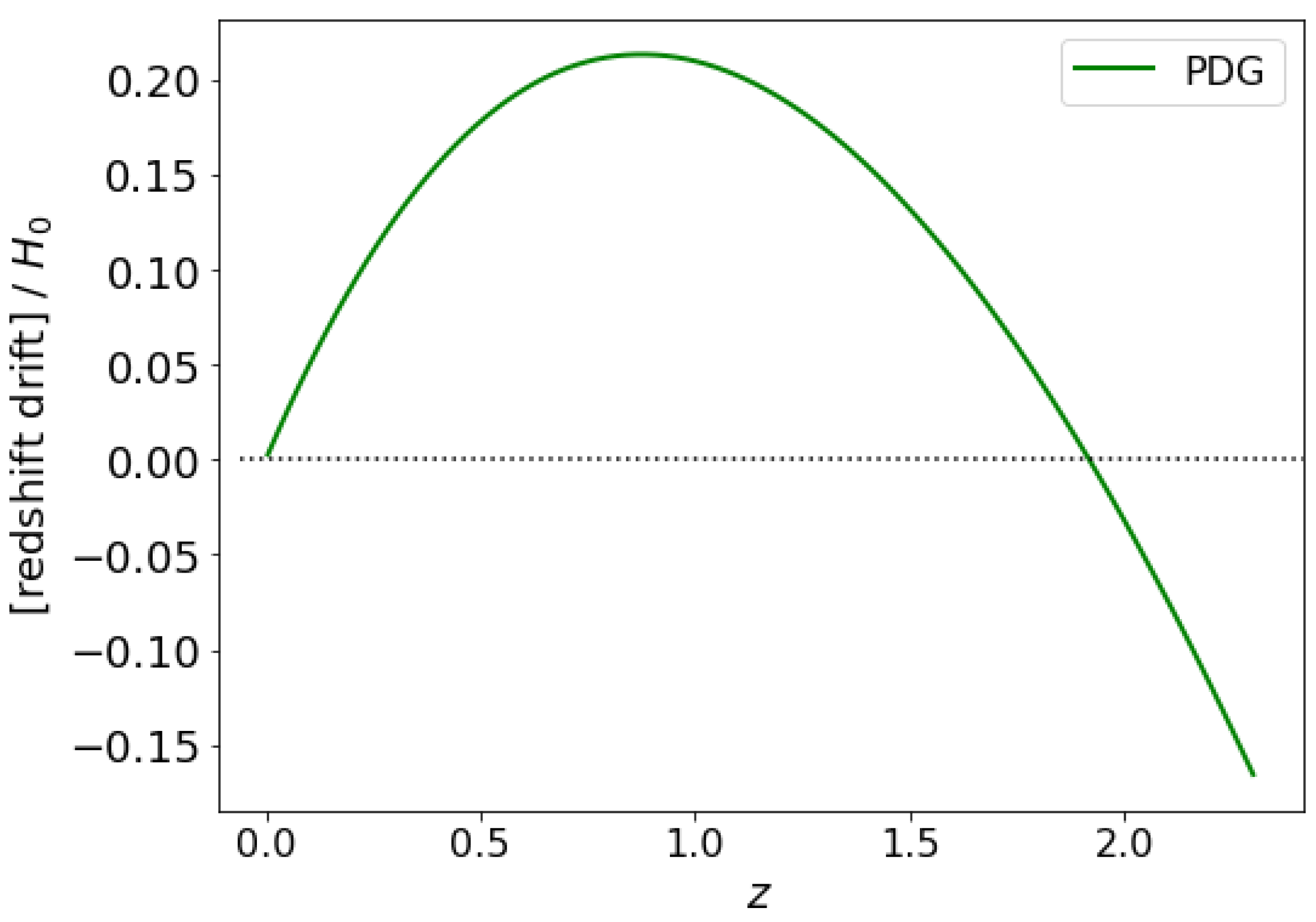
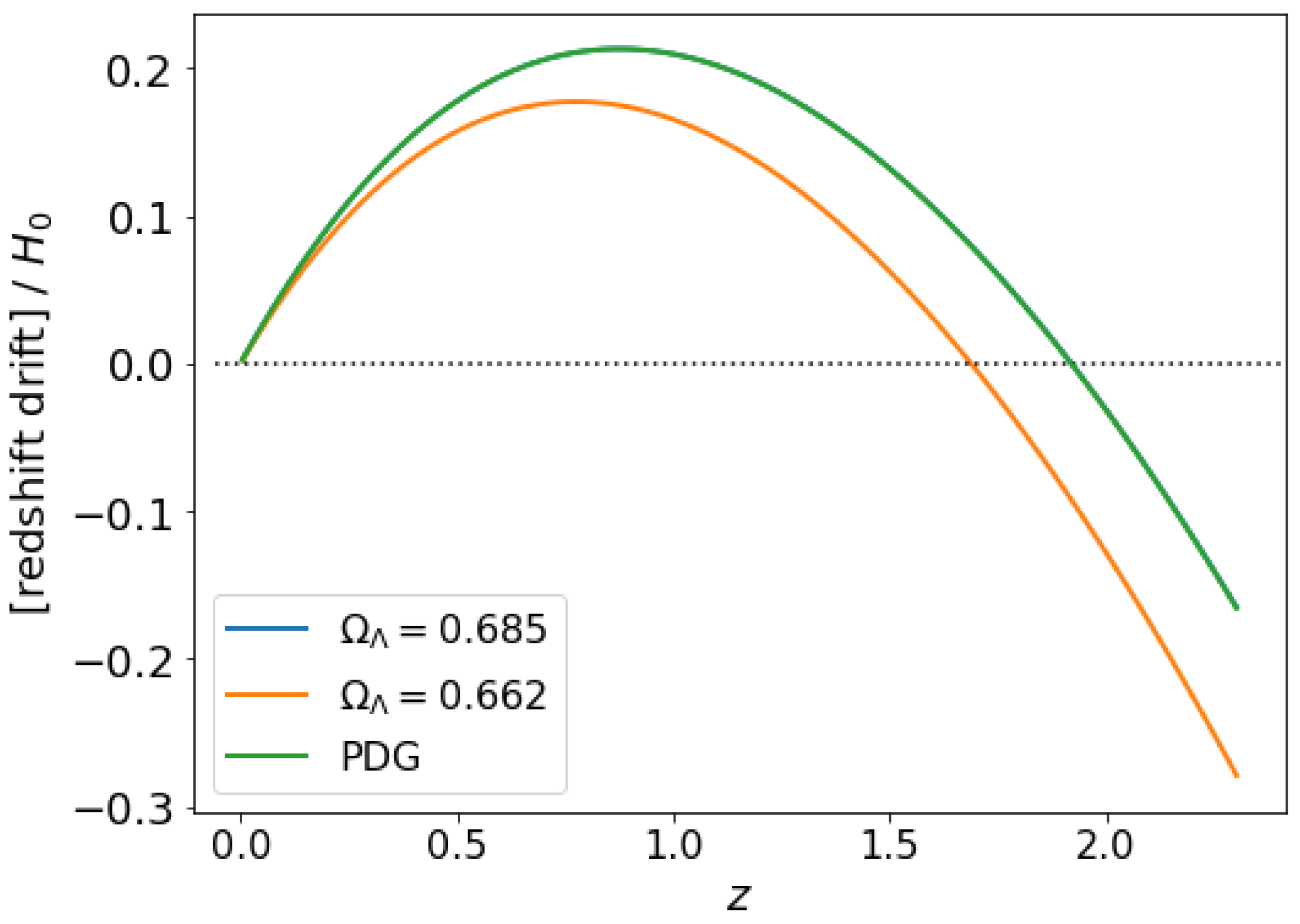
3. Redshift Drift for Different Dark Energy Models
- CDM: (or simply wCDM): It is the simplest dark energy model, where the barotropic equation of state is given by , with being a constant. In this case, as presented above, the Hubble parameter evolves as
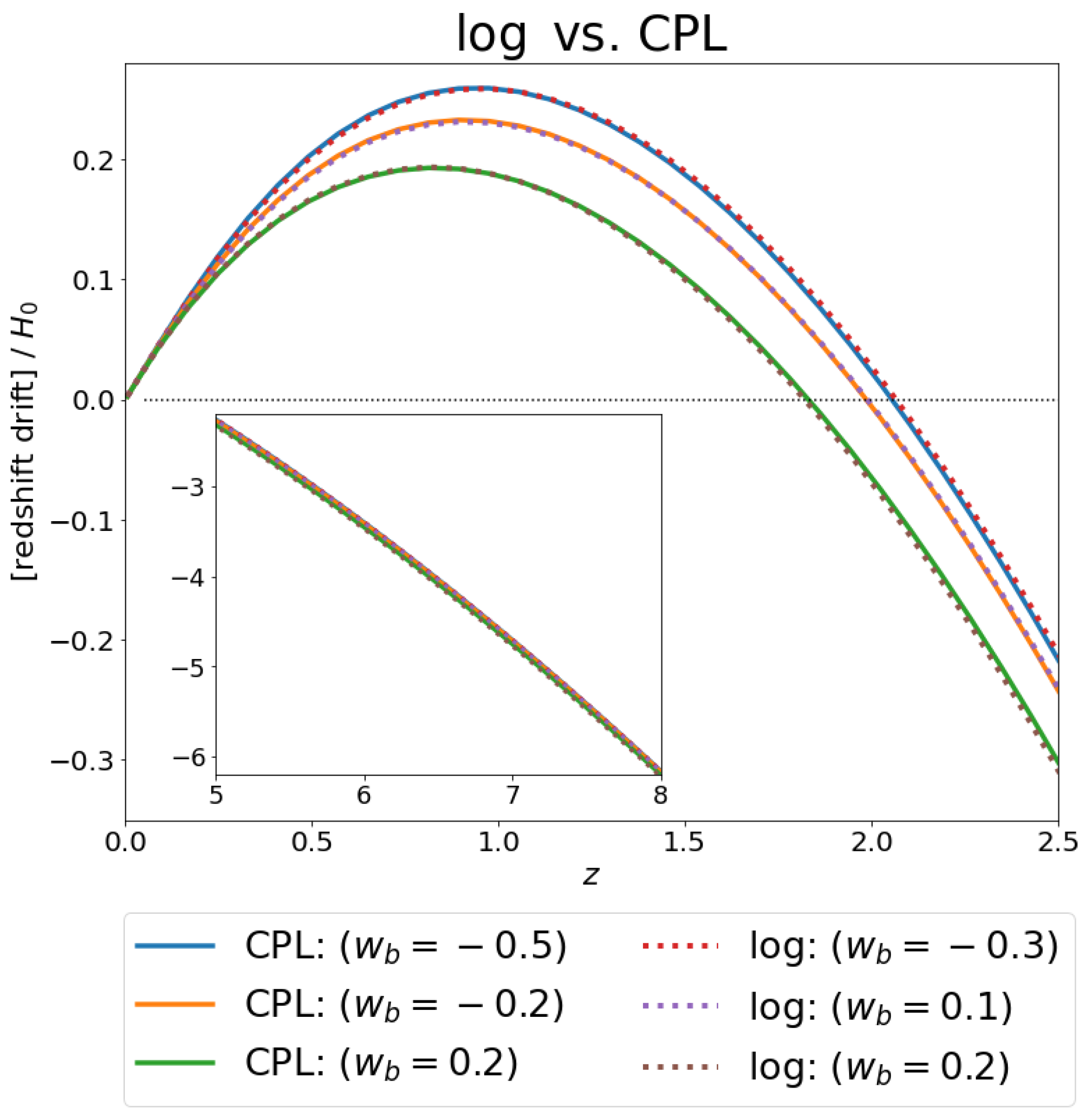
- BAZS EoS: The Barboza–Alcaniz–Zhu–Silva [60] equation of state is a three-parameter model for dark energy given byThis model includes the linear model as the limit of and CPL as the limit of . Furthermore, taking the limit of gives us the logarithmic model. The Hubble parameter evolution, assuming , is then given by [60]The results for a fixed and for different values of the parameter b are presented in Figure 4b.
- Linear model: In this model, the barotropic equation of state is allowed to vary along the evolution of the universe in a linear way:The two free variables and are constants, and the Hubble parameter evolution is given byFigure 4c,d show how the RD data changes when keeping, respectively, and fixed while varying the other variable.
- CPL: Named after Chevallier, Polarski, and Linder [61,62], this two-parameter equation of state guarantees, when compared to the linear model, a bound on how much w can grow as we go back in time.Here, represents the value of w at the present moment, while represents its value at the asymptotic past. In this case, we haveThe results for the expected RD signal for distinct values of and can be found in Figure 4e,f, respectively.Figure 4. Redshift drift for different dark energy models.
- Logarithmic evolution: This was first introduced by Efstathiou [63], who suggested a logarithmic evolution of into the asymptotic past:The results for different values of and are presented in Figure 5a,b, and the Hubble parameter evolution is given byFigure 5. Redshift drift for the logarithmic model.
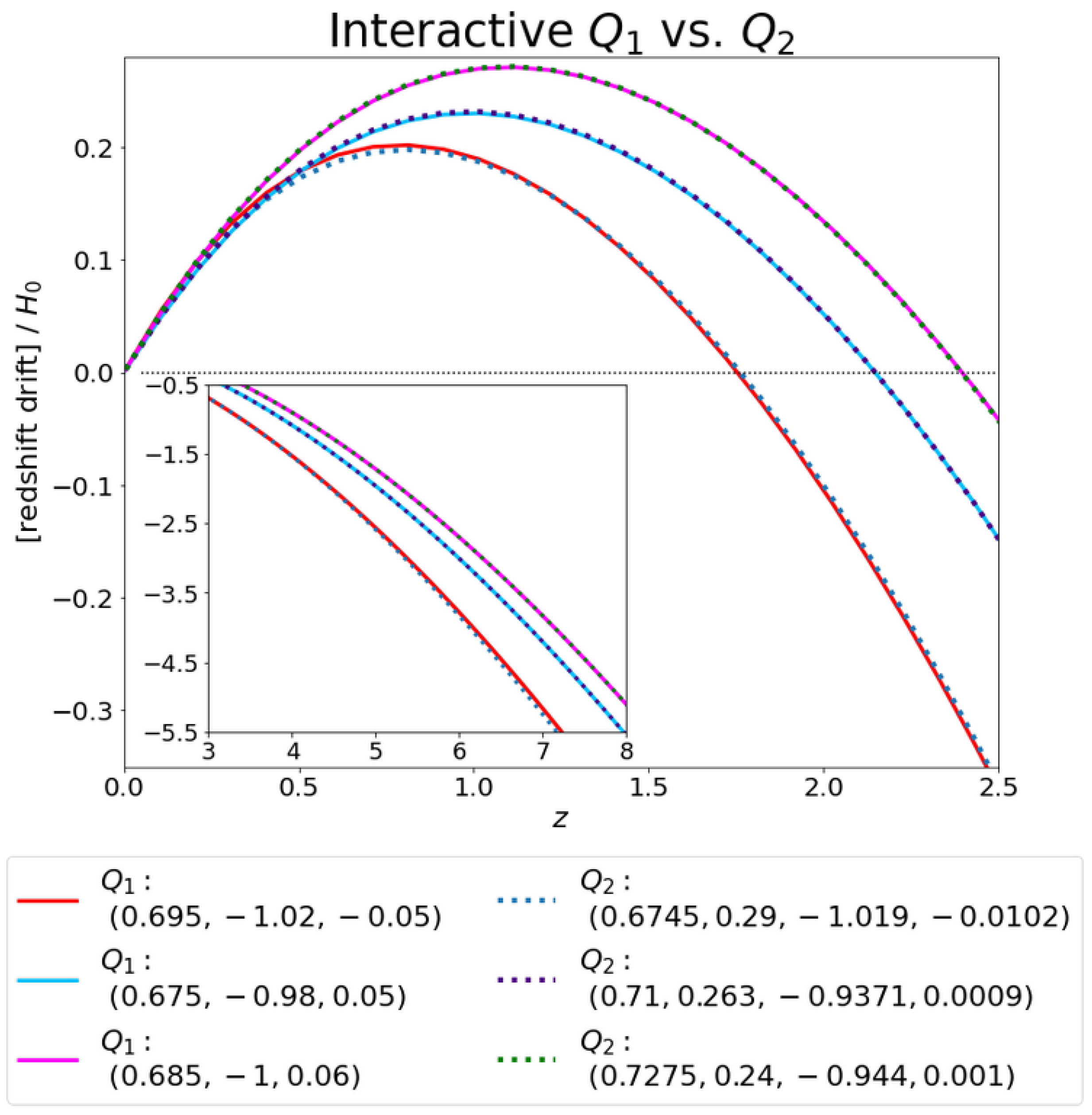
- Interactive dark energy models: Another possible scenario is to consider interactive models, where dark energy and dark matter exchange energy via an interaction term, Q. In this case, the energy conservation equations in FLRW give usHere, and stand for the energy density of cold dark matter and dark energy, respectively.
Can RD Data Distinguish Different Models?
| log: | , , |
| BAZS: | , , |
| : | , , , |
| : | , , , |
4. Redshift Drift for Other Auxiliary Variables
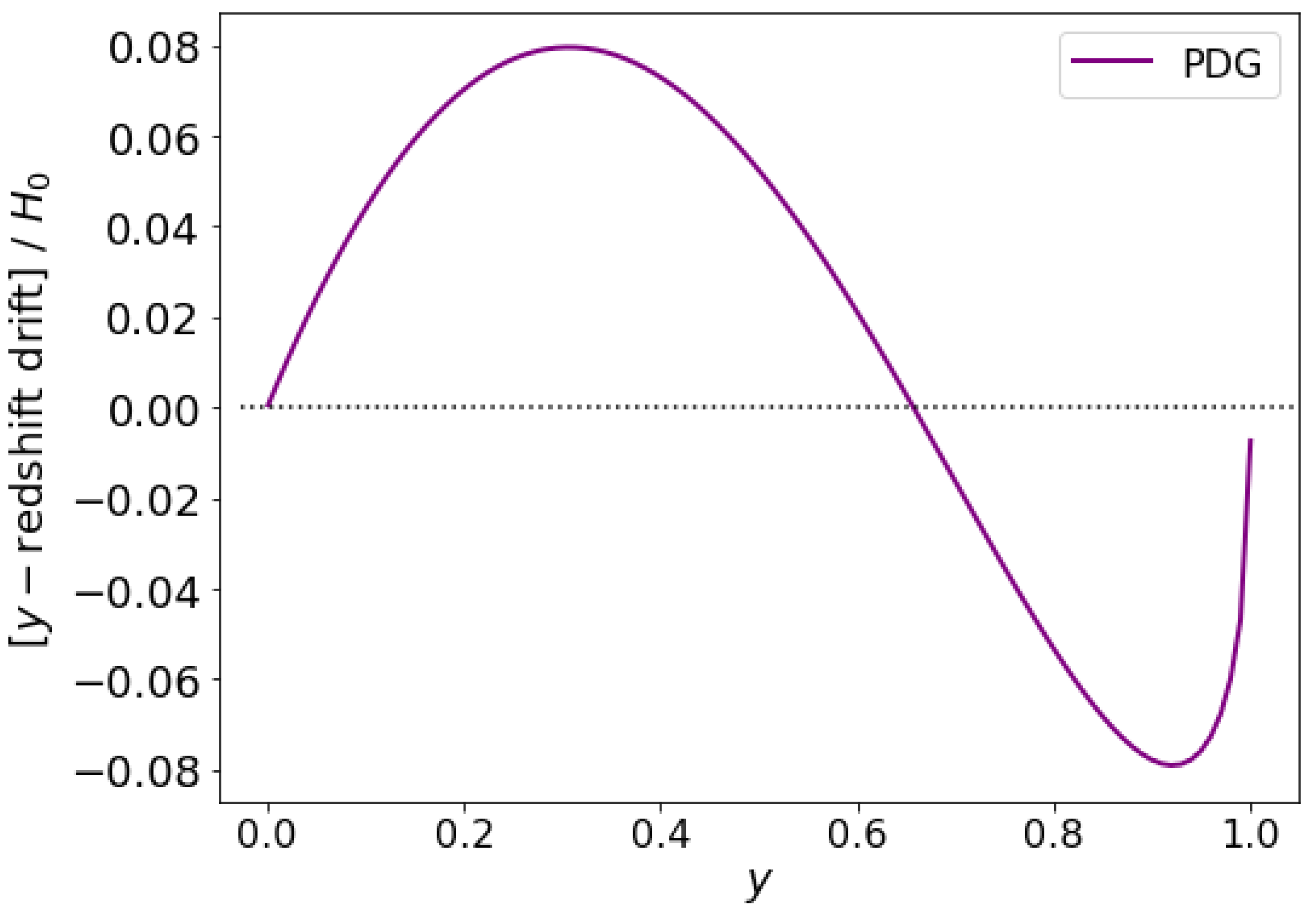
4.1. Redshift Drift in Terms of y
- One-component model:
- CDM (general four-component version):
- CDM (two-component version):
4.2. Table of Expressions for Other Auxiliary Variables
| Variable: | |
| Convergence radius: | |
| Variable: | |
| Convergence radius: | |
| Variable: | |
| Convergence radius: | |
| Variable: | |
| Convergence radius: | |
4.3. Summary
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sandage, A. The change of redshift and apparent luminosity of galaxies due to the deceleration of selected expanding universes. Astrophys. J. 1962, 136, 319. [Google Scholar] [CrossRef]
- McVittie, G.C. Appendix to the change of redshift and apparent luminosity of galaxies due to the deceleration of selected expanding universes. Astrophys. J. 1962, 136, 334. [Google Scholar]
- Loeb, A. Direct measurement of cosmological parameters from the cosmic deceleration of extragalactic objects. Astrophys. J. 1998, 499, L111. [Google Scholar] [CrossRef]
- Linder, E.V. Mapping the Cosmological Expansion. Rept. Prog. Phys. 2008, 71, 56901. [Google Scholar] [CrossRef]
- Quercellini, C.; Amendola, L.; Balbi, A.; Cabella, P.; Quartin, M. Real-time Cosmology. Phys. Rept. 2012, 521, 95. [Google Scholar] [CrossRef]
- Alves, C.S.; Leite, A.C.O.; Martins, C.J.A.P.; Matos, J.G.B.; Silva, T.A. Forecasts of redshift drift constraints on cosmological parameters. Mon. Not. R. Astron. Soc. 2019, 488, 3607. [Google Scholar] [CrossRef]
- Liske, J.; Grazian, A.; Vanzella, E.; Dessauges, M.; Viel, M.; Pasquini, L.; Haehnelt, M.; Cristiani, S.; Pepe, F.; Avila, G.; et al. Cosmic dynamics in the era of Extremely Large Telescopes. Mon. Not. R. Astron. Soc. 2008, 386, 1192. [Google Scholar] [CrossRef]
- Steinmetz, T.; Wilken, T.; Araujo-Hauck, C.; Holzwarth, R.; Hansch, T.W.; Pasquini, L.; Manescau, A.; D’odorico, S.; Murphy, M.T.; Kentischer, T.; et al. Laser frequency combs for astronomical observations. Science 2008, 321, 1335. [Google Scholar] [CrossRef]
- Kim, A.G.; Linder, E.V.; Edelstein, J.; Erskine, D. Giving Cosmic Redshift Drift a Whirl. Astropart. Phys. 2015, 62, 195. [Google Scholar] [CrossRef]
- Killedar, M.; Lewis, G.F. Lyman alpha absorbers in motion: Consequences of gravitational lensing for the cosmological redshift drift experiment. Mon. Not. R. Astron. Soc. 2010, 402, 650. [Google Scholar] [CrossRef]
- Lazkoz, R.; Leanizbarrutia, I.; Salzano, V. Forecast and analysis of the cosmological redshift drift. Eur. Phys. J. C 2018, 78, 11. [Google Scholar] [CrossRef] [PubMed]
- Marcori, O.H.; Pitrou, C.; Uzan, J.P.; Pereira, T.S. Direction and redshift drifts for general observers and their applications in cosmology. Phys. Rev. D 2018, 98, 023517. [Google Scholar] [CrossRef]
- Lu, C.Z.; Zhang, T.; Zhang, T.J. Statistical distribution of HI 21 cm absorbers as potential cosmic acceleration probes. Mon. Not. R. Astron. Soc. 2023, 521, 3150–3161. [Google Scholar] [CrossRef]
- Dong, C.; Gonzalez, A.; Eikenberry, S.; Jeram, S.; Likamonsavad, M.; Liske, J.; Stelter, D.; Townsend, A. Forecasting cosmic acceleration measurements using the Lyman-α forest. Mon. Not. R. Astron. Soc. 2022, 514, 5493–5505. [Google Scholar] [CrossRef]
- Covone, G.; Sereno, M. Lensing cosmic drift. Mon. Not. R. Astron. Soc. 2022, 513, 5198–5203. [Google Scholar] [CrossRef]
- Chakrabarti, S.; Gonzalez, A.H.; Eikenberry, S.; Erskine, D.; Ishak, M.; Kim, A.; Linder, E.; Nomerotski, A.; Pierce, M.; Slosar, A.; et al. Real-time Cosmology with High Precision Spectroscopy and Astrometry. arXiv 2023, arXiv:2203.05924. [Google Scholar]
- Lu, C.Z.; Jiao, K.; Zhang, T.; Zhang, T.J.; Zhu, M. Toward a direct measurement of the cosmic acceleration: The first preparation with FAST. Phys. Dark Univ. 2022, 37, 101088. [Google Scholar] [CrossRef]
- Martins, C.J.A.P.; Alves, C.S.; Esteves, J.; Lapel, A.; Pereira, B.G. Closing the cosmological loop with the redshift drift. In Proceedings of the Sixteenth Marcel Grossmann Meeting on Recent Developments in Theoretical and Experimental General Relativity, Astrophysics and Relativistic Field Theories, Online, 5–10 July 2021. [Google Scholar]
- Eikenberry, S.S.; Gonzalez, A.; Darling, J.; Liske, J.; Slepian, Z.; Mueller, G.; Conklin, J.; Fulda, P.; de Oliveira, C.M.; Bentz, M.; et al. Astro2020 Project White Paper: The Cosmic Accelerometer. arXiv 2019, arXiv:1907.08271. [Google Scholar]
- Esteves, J.; Martins, C.J.A.P.; Pereira, B.G.; Alves, C.S. Cosmological impact of redshift drift measurements. Mon. Not. R. Astron. Soc. 2021, 508, L53–L57. [Google Scholar] [CrossRef]
- Uzan, J.P.; Clarkson, C.; Ellis, G.F.R. Time drift of cosmological redshifts as a test of the Copernican principle. Phys. Rev. Lett. 2008, 100, 191303. [Google Scholar] [CrossRef]
- Koksbang, S.M.; Heinesen, A. Redshift drift in a universe with structure: Lemaître-Tolman-Bondi structures with arbitrary angle of entry of light. Phys. Rev. D 2022, 106, 043501. [Google Scholar] [CrossRef]
- Koksbang, S.M.; Hannestad, S. Redshift drift in an inhomogeneous universe: Averaging and the backreaction conjecture. J. Cosmol. Astropart. Phys. 2016, 1601, 9. [Google Scholar] [CrossRef]
- Quartin, M.; Amendola, L. Distinguishing Between Void Models and Dark Energy with Cosmic Parallax and Redshift Drift. Phys. Rev. D 2010, 81, 043522. [Google Scholar] [CrossRef]
- Yoo, C.M.; Kai, T.; Nakao, K.i. Redshift Drift in LTB Void Universes. Phys. Rev. D 2011, 83, 43527. [Google Scholar] [CrossRef]
- Geng, J.J.; Li, Y.H.; Zhang, J.F.; Zhang, X. Redshift drift exploration for interacting dark energy. Eur. Phys. J. C 2015, 75, 356. [Google Scholar] [CrossRef][Green Version]
- Calabrese, E.; Martinelli, M.; Pandolfi, S.; Cardone, V.F.; Martins, C.J.A.P.; Spiro, S.; Vielzeuf, P.E. Dark Energy coupling with electromagnetism as seen from future low-medium redshift probes. Phys. Rev. D 2014, 89, 83509. [Google Scholar] [CrossRef]
- Mishra, P.; Celerier, M.N.; Singh, T.P. Redshift drift as a test for discriminating between different cosmological models. Phys. Rev. D 2012, 86, 83520. [Google Scholar] [CrossRef]
- Li, Z.; Liao, K.; Wu, P.; Yu, H.; Zhu, Z.-H. Probing modified gravity theories with the Sandage-Loeb test. Phys. Rev. D 2021, 88, 23003. [Google Scholar] [CrossRef]
- Scherrer, R.J.; Loeb, A. Ultra Long-Term Cosmology and Astrophysics. New Astron. 2023, 99, 101940. [Google Scholar] [CrossRef]
- Lobo, F.S.; Mimoso, J.P.; Visser, M. Cosmographic analysis of redshift drift. J. Cosmol. Astropart. Phys. 2020, 4, 43. [Google Scholar] [CrossRef]
- Dunsby, P.K.S.; Luongo, O. On the theory and applications of modern cosmography. Int. J. Geom. Meth. Mod. Phys. 2016, 13, 1630002. [Google Scholar] [CrossRef]
- Visser, M. Jerk and the cosmological equation of state. Class. Quant. Grav. 2004, 21, 2603. [Google Scholar] [CrossRef]
- Dunajski, M.; Gibbons, G. Cosmic Jerk, Snap and Beyond. Class. Quant. Grav. 2008, 25, 235012. [Google Scholar] [CrossRef]
- Visser, M. Cosmography: Cosmology without the Einstein equations. Gen. Rel. Grav. 2005, 37, 1541. [Google Scholar] [CrossRef]
- Aviles, A.; Gruber, C.; Luongo, O.; Quevedo, H. Cosmography and constraints on the equation of state of the Universe in various parametrizations. Phys. Rev. D 2012, 86, 123516. [Google Scholar] [CrossRef]
- Cattöen, C.; Visser, M. The Hubble series: Convergence properties and redshift variables. Class. Quant. Grav. 2007, 24, 5985. [Google Scholar] [CrossRef]
- Cattöen, C.; Visser, M. Cosmographic Hubble fits to the supernova data. Phys. Rev. D 2008, 78, 063501. [Google Scholar] [CrossRef]
- Eager, D.; Pendrill, A.M.; Reistad, N. Beyond velocity and acceleration: Jerk, snap and higher derivatives. Eur. J. Phys. 2016, 37, 065008. [Google Scholar] [CrossRef]
- Busti, V.C.; Cruz-Dombriz, Á.; Dunsby, P.K.S.; Sáez-Gómez, D. Is cosmography a useful tool for testing cosmology? Phys. Rev. D 2015, 92, 123512. [Google Scholar] [CrossRef]
- Risaliti, G.; Lusso, E. Cosmological constraints from the Hubble diagram of quasars at high redshifts. Nat. Astron. 2019, 3, 272–277. [Google Scholar] [CrossRef]
- Hu, J.P.; Wang, F.Y. High-redshift cosmography: Application and comparison with different methods. Astron. Astrophys. 2022, 661, A71. [Google Scholar] [CrossRef]
- Yang, T.; Banerjee, A.; Colgáin, E.Ó. Cosmography and flat ΛCDM tensions at high redshift. Phys. Rev. D 2020, 102, 123532. [Google Scholar] [CrossRef]
- Capozziello, S.; D’Agostino, R.; Luongo, O. High-redshift cosmography: Auxiliary variables versus Padé polynomials. Mon. Not. R. Astron. Soc. 2020, 494, 2576–2590. [Google Scholar] [CrossRef]
- Madsen, M.S.; Ellis, G.F.R.; Mimoso, J.P.; Butcher, J.A. Evolution of the density parameter in inflationary cosmology reexamined. Phys. Rev. D 1992, 46, 1399–1415. [Google Scholar] [CrossRef] [PubMed]
- Particle Data Group. Available online: https://pdg.lbl.gov/2020/reviews/rpp2020-rev-astrophysical-constants.pdf (accessed on 21 March 2024).
- Particle Data Group; Workman, R.L.; Burkert, V.D.; Crede, V.; Klempt, E.; Thoma, U.; Tiator, L.; Agashe, K.; Aielli, G.; Allanach, B.C.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar]
- Brout, D.; Scolnic, D.; Popovic, B.; Riess, A.G.; Carr, A.; Zuntz, J.; Kessler, R.; Davis, T.M.; Hinton, S.; Jones, D.; et al. The Pantheon+ Analysis: Cosmological Constraints. Astrophys. J. 2022, 938, 110. [Google Scholar] [CrossRef]
- Recombination (Cosmology). Available online: https://en.wikipedia.org/wiki/Recombination_(cosmology) (accessed on 21 March 2024).
- Corless, R.; Gonnet, G.; Hare, D.; Jeffrey, D.; Knuth, D. On the Lambert W function. Adv. Comput. Math. 1996, 5, 329–359. [Google Scholar] [CrossRef]
- Valluri, S.R.; Jeffrey, D.J.; Corless, R.M. Some applications of the Lambert W function to physics. Can. J. Phys. 2000, 78, 823. [Google Scholar] [CrossRef]
- Valluri, S.R.; Gil, M.; Jeffrey, D.J.; Basu, S. The Lambert W function and quantum statistics. J. Math. Phys. 2009, 50, 102103. [Google Scholar] [CrossRef]
- Boonserm, P.; Ngampitipan, T.; Visser, M. Regge-Wheeler equation, linear stability, and greybody factors for dirty black holes. Phys. Rev. D 2013, 88, 041502. [Google Scholar] [CrossRef]
- Sonoda, H. Solving renormalization group equations with the Lambert W function. Phys. Rev. D 2013, 87, 85023. [Google Scholar] [CrossRef]
- Visser, M. Primes and the Lambert W function. Mathematics 2018, 6, 56. [Google Scholar] [CrossRef]
- Charters, T.; Mimoso, J. Self-interacting scalar field cosmologies: Unified exact solutions and symmetries. J. Cosmol. Astropart. Phys. 2010, 8, 22. [Google Scholar] [CrossRef]
- Balbi, A.; Quercellini, C. The time evolution of cosmological redshift as a test of dark energy. Mon. Not. R. Astron. Soc. 2007, 382, 1623. [Google Scholar] [CrossRef]
- Corasaniti, P.S.; Huterer, D.; Melchiorri, A. Exploring the dark energy redshift desert with the Sandage-Loeb test. Phys. Rev. D 2007, 75, 062001. [Google Scholar] [CrossRef]
- Barboza, E.M., Jr.; Alcaniz, J.S.; Zhu, Z.-H.; Silva, R. Generalized equation of state for dark energy. Phys. Rev. D 2009, 80, 043521. [Google Scholar] [CrossRef]
- Chevallier, M.; Polarski, D. Accelerating Universes with Scaling Dark Matter. Int. J. Mod. Phys. D 2001, 10, 213. [Google Scholar] [CrossRef]
- Linder, E.V. Exploring the Expansion History of the Universe. Phys. Rev. Lett. 2003, 90, 091301. [Google Scholar] [CrossRef]
- Efstathiou, G. Constraining the equation of state of the Universe from distant Type Ia supernovae and cosmic microwave background anisotropies. Mon. Not. R. Astron. Soc. 1999, 310, 842–850. [Google Scholar] [CrossRef]
- Cao, S.; Liang, N. Interaction between dark energy and dark matter: Observational constraints from OHD, BAO, CMB and SNe Ia. Int. J. Mod. Phys. D 2013, 22, 1350082. [Google Scholar] [CrossRef]
- Xia, D.-M.; Wang, S. Constraining interacting dark energy models with latest cosmological observations. Mont. Not. R. Astron. Soc. 2016, 463, 952–956. [Google Scholar] [CrossRef][Green Version]
- Zhang, J.F.; Zhang, M.; Jin, S.J.; Qi, J.Z.; Zhang, X. Cosmological parameter estimation with future gravitational wave standard siren observation from the Einstein Telescope. J. Cosmol. Astropart. Phys. 2019, 9, 68. [Google Scholar] [CrossRef]
- Qi, J.Z.; Jin, S.J.; Fan, X.L.; Zhang, J.F.; Zhang, X. Using a multi-messenger and multi-wavelength observational strategy to probe the nature of dark energy through direct measurements of cosmic expansion history. J. Cosmol. Astropart. Phys. 2019, 12, 42. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, B.; Wu, P.; Qi, J.; Xu, Y.; Zhang, J.; Zhang, X. Prospects for Constraining Interacting Dark Energy Models with 21 cm Intensity Mapping Experiments. Astrophys. J. 2021, 918, 56. [Google Scholar] [CrossRef]
- Jin, S.J.; Zhu, R.Q.; Wang, L.F.; Li, H.L.; Zhang, J.F.; Zhang, X. Impacts of gravitational-wave standard siren observations from Einstein Telescope and Cosmic Explorer on weighing neutrinos in interacting dark energy models. Commun. Theor. Phys. 2022, 74, 105404. [Google Scholar] [CrossRef]
- Hou, W.T.; Qi, J.Z.; Han, T.; Zhang, J.F.; Cao, S.; Zhang, X. Prospects for constraining interacting dark energy models from gravitational wave and gamma ray burst joint observation. J. Cosmol. Astropart. Phys. 2023, 5, 17. [Google Scholar] [CrossRef]
- CODEX Phase A, Science Case, Document E-TRE-IOA-573-0001 Issue 1 (2010). The Science Case for CODEX, an Ultra-Stable High-Resolution Spectrograph for the E-ELT. Available online: http://research.iac.es/proyecto/codex//media/codex_files/CO01_Science_Case.pdf (accessed on 21 March 2024).




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lobo, F.S.N.; Mimoso, J.P.; Santiago, J.; Visser, M. Dynamical Analysis of the Redshift Drift in FLRW Universes. Universe 2024, 10, 162. https://doi.org/10.3390/universe10040162
Lobo FSN, Mimoso JP, Santiago J, Visser M. Dynamical Analysis of the Redshift Drift in FLRW Universes. Universe. 2024; 10(4):162. https://doi.org/10.3390/universe10040162
Chicago/Turabian StyleLobo, Francisco S. N., José Pedro Mimoso, Jessica Santiago, and Matt Visser. 2024. "Dynamical Analysis of the Redshift Drift in FLRW Universes" Universe 10, no. 4: 162. https://doi.org/10.3390/universe10040162
APA StyleLobo, F. S. N., Mimoso, J. P., Santiago, J., & Visser, M. (2024). Dynamical Analysis of the Redshift Drift in FLRW Universes. Universe, 10(4), 162. https://doi.org/10.3390/universe10040162









