Analysis of the Geodesic Motions of Massive Particles in Kerr–Sen–AdS4 Spacetime
Abstract
1. Introduction
2. The Kerr–Sen–AdS4 Black Hole Solution
3. The Geodesic Equations
4. Analysis of the Geodesic Equations
Types of Latitudinal Motion
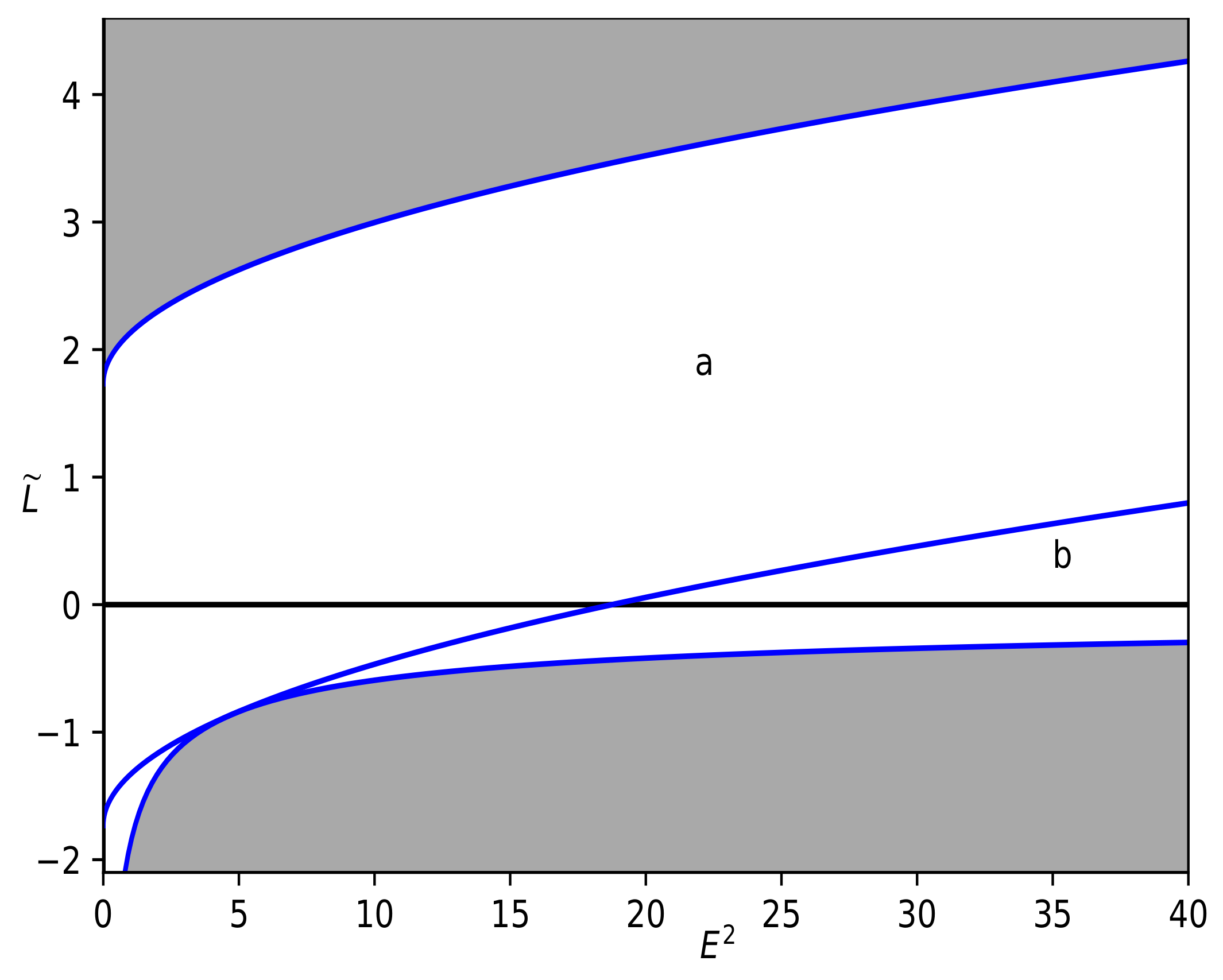
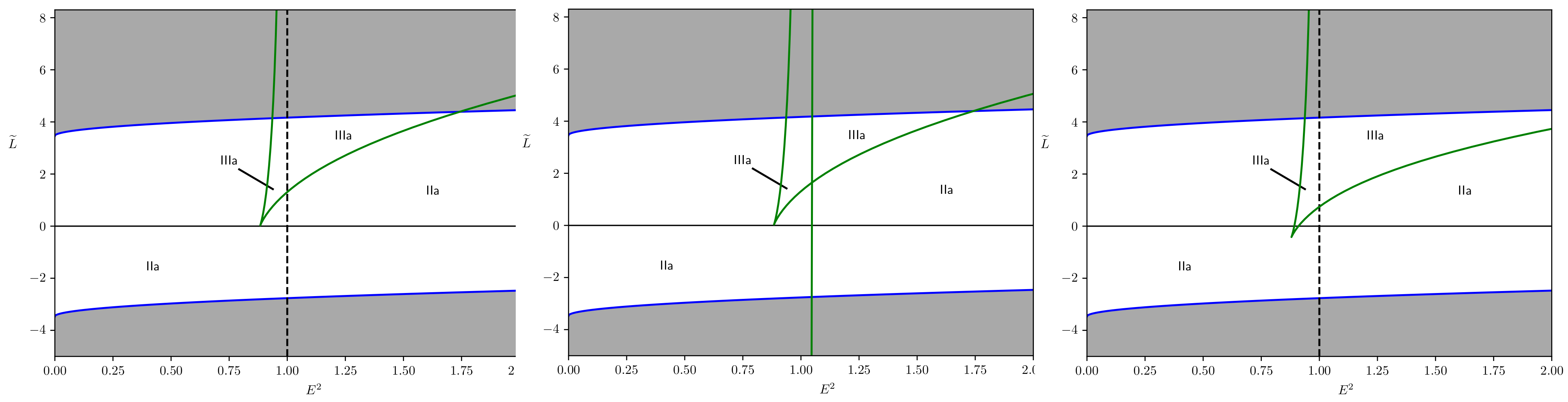
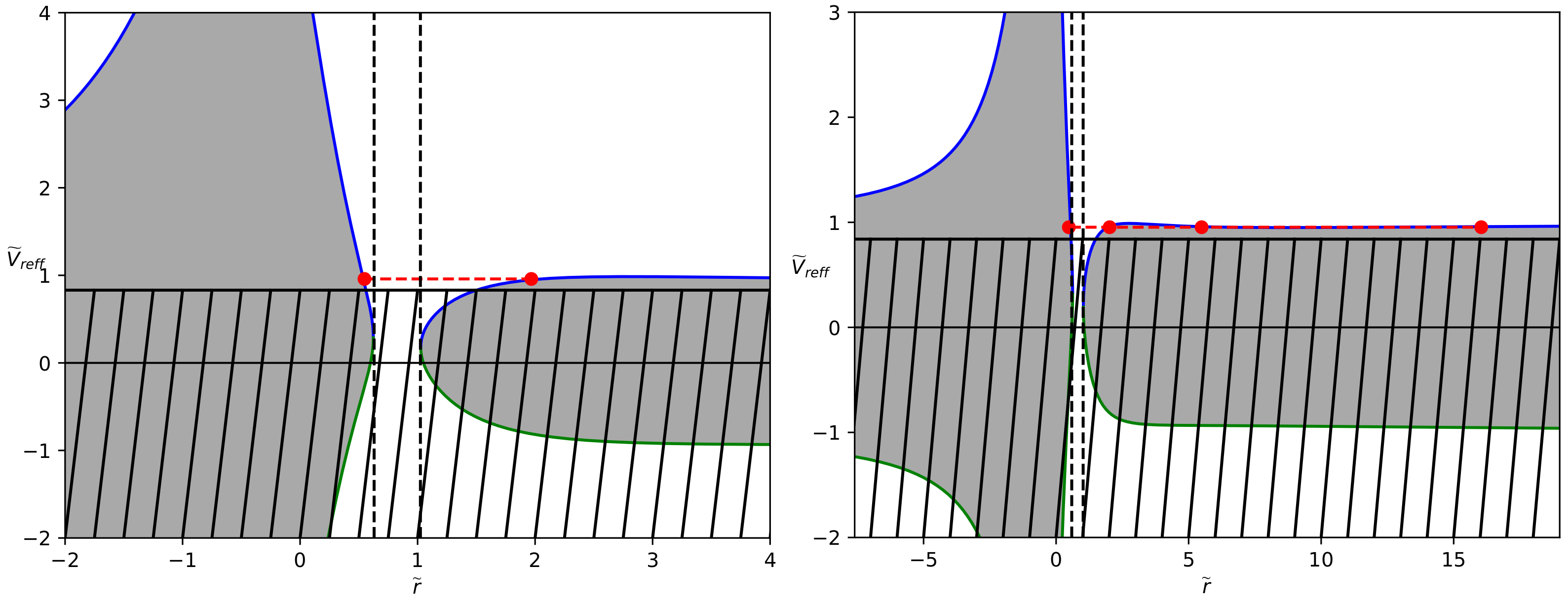
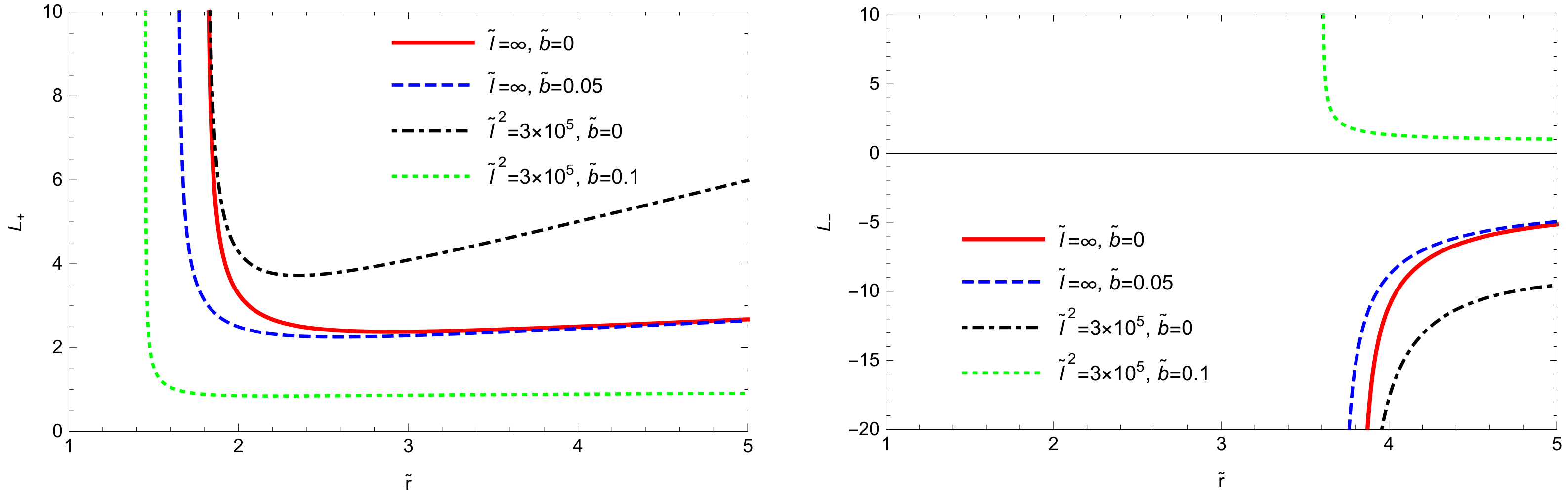
5. Analysis of the Radial Geodesic Equations
5.1. Types of Radial Motion
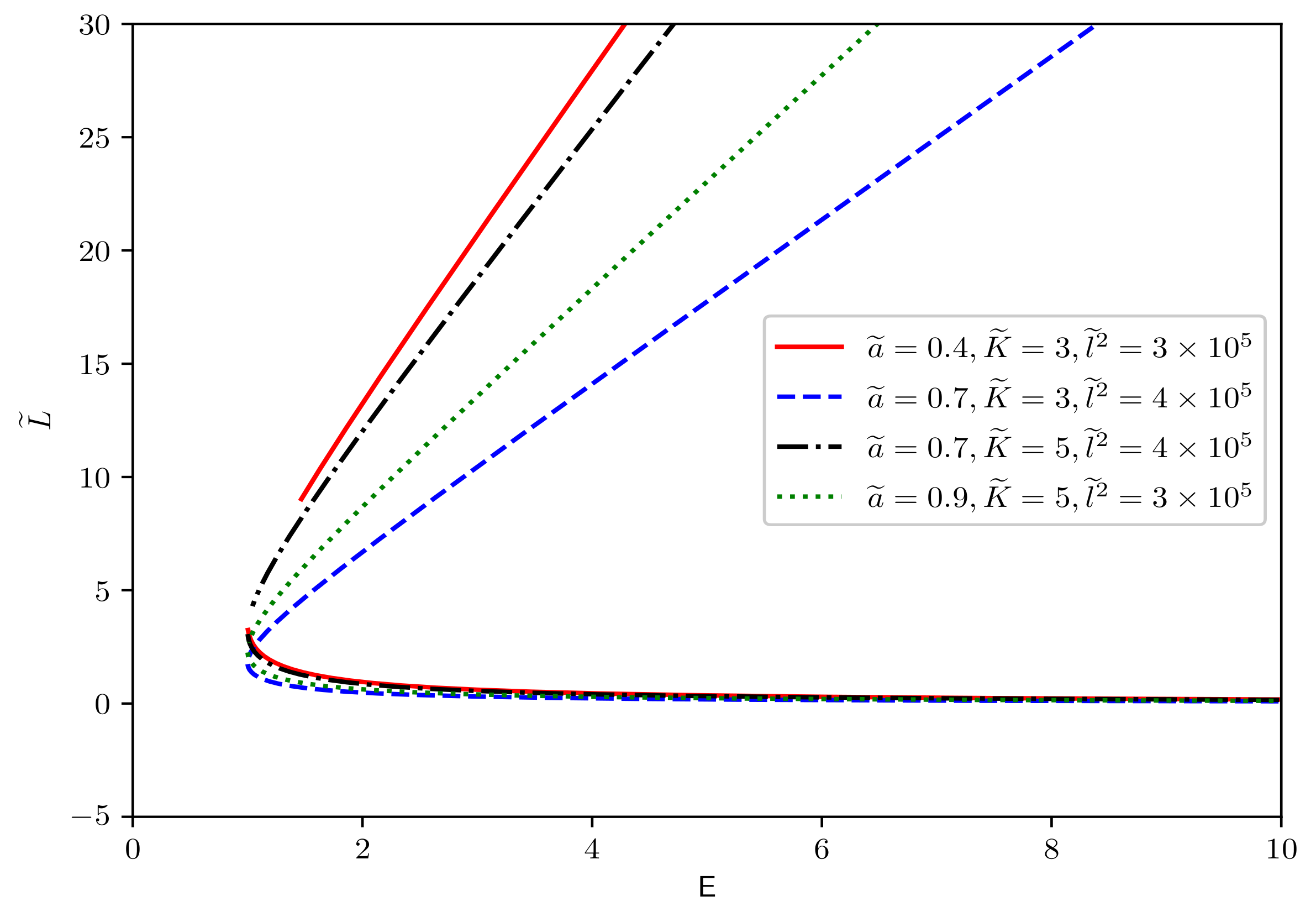
5.2. Circular Orbits and Equatorial Circular Orbits
5.3. Innermost Stable Circular Orbit
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hagihara, Y. Theory of the relativistic trajeetories in a gravitational field of schwarzschild. Jpn. J. Astron. Geophys. 1930, 8, 67. [Google Scholar]
- Chandrasekhar, S. The Mathematical Theory of Black Holes; Oxford University Press: Oxford, UK, 1983. [Google Scholar]
- Hoseini, B.; Saffari, R.; Soroushfar, S. Study of the geodesic equations of a spherical symmetric spacetime in conformal Weyl gravity. Class. Quant. Grav. 2017, 34, 055004. [Google Scholar] [CrossRef]
- Soroushfar, S.; Saffari, R.; Kazempour, S.; Grunau, S.; Kunz, J. Detailed study of geodesics in the Kerr-Newman-(A)dS spacetime and the rotating charged black hole spacetime in f(R) gravity. Phys. Rev. D 2016, 94, 024052. [Google Scholar] [CrossRef]
- Hackmann, E.; Lammerzahl, C. Complete Analytic Solution of the Geodesic Equation in Schwarzschild-(Anti-)de Sitter Spacetimes. Phys. Rev. Lett. 2008, 100, 171101. [Google Scholar] [CrossRef] [PubMed]
- Hackmann, E.; Lammerzahl, C.; Kagramanova, V.; Kunz, J. Analytical solution of the geodesic equation in Kerr-(anti) de Sitter space-times. Phys. Rev. D 2010, 81, 044020. [Google Scholar] [CrossRef]
- Soroushfar, S.; Saffari, R.; Kunz, J.; Lämmerzahl, C. Analytical solutions of the geodesic equation in the spacetime of a black hole in f(R) gravity. Phys. Rev. D 2015, 92, 044010. [Google Scholar] [CrossRef]
- Flathmann, K.; Grunau, S. Analytic solutions of the geodesic equation for Einstein-Maxwell-dilaton-axion black holes. Phys. Rev. D 2015, 92, 104027. [Google Scholar] [CrossRef]
- Soroushfar, S.; Saffari, R.; Sahami, E. Geodesic equations in the static and rotating dilaton black holes: Analytical solutions and applications. Phys. Rev. D 2016, 94, 024010. [Google Scholar] [CrossRef]
- Flathmann, K.; Grunau, S. Analytic solutions of the geodesic equation for U(1)2 dyonic rotating black holes. Phys. Rev. D 2016, 94, 124013. [Google Scholar] [CrossRef]
- Hackmann, E.; Kagramanova, V.; Kunz, J.; Lammerzahl, C. Analytic solutions of the geodesic equation in higher dimensional static spherically symmetric space-times. Phys. Rev. D 2008, 78, 124018. [Google Scholar] [CrossRef]
- Kagramanova, V.; Reimers, S. Analytic treatment of geodesics in five-dimensional Myers-Perry space–times. Phys. Rev. D 2012, 86, 084029. [Google Scholar] [CrossRef]
- Diemer, V.; Kunz, J. Supersymmetric rotating black hole spacetime tested by geodesics. Phys. Rev. D 2014, 89, 084001. [Google Scholar] [CrossRef]
- Diemer, V.; Kunz, J.; Lämmerzahl, C.; Reimers, S. Dynamics of test particles in the general five-dimensional Myers-Perry spacetime. Phys. Rev. D 2014, 89, 124026. [Google Scholar] [CrossRef]
- Kagramanova, V.; Kunz, J.; Hackmann, E.; Lammerzahl, C. Analytic treatment of complete and incomplete geodesics in Taub-NUT space-times. Phys. Rev. D 2010, 81, 124044. [Google Scholar] [CrossRef]
- Diemer, V.; Smolarek, E. Dynamics of test particles in thin-shell wormhole spacetimes. Class. Quant. Grav. 2013, 30, 175014. [Google Scholar] [CrossRef]
- Hackmann, E.; Lammerzahl, C. Geodesic equation in Schwarzschild- (anti-) de Sitter space-times: Analytical solutions and applications. Phys. Rev. D 2008, 78, 024035. [Google Scholar] [CrossRef]
- Hackmann, E.; Kagramanova, V.; Kunz, J.; Lammerzahl, C. Analytic solutions of the geodesic equation in axially symmetric space-times. Europhys. Lett. 2009, 88, 30008. [Google Scholar] [CrossRef]
- Grunau, S.; Kagramanova, V. Geodesics of electrically and magnetically charged test particles in the Reissner-Nordström space-time: Analytical solutions. Phys. Rev. D 2011, 83, 044009. [Google Scholar] [CrossRef]
- Enolski, V.Z.; Hackmann, E.; Kagramanova, V.; Kunz, J.; Lammerzahl, C. Inversion of hyperelliptic integrals of arbitrary genus with application to particle motion in General Relativity. J. Geom. Phys. 2011, 61, 899–921. [Google Scholar] [CrossRef]
- Hackmann, E.; Hartmann, B.; Lammerzahl, C.; Sirimachan, P. Test particle motion in the space-time of a Kerr black hole pierced by a cosmic string. Phys. Rev. D 2010, 82, 044024. [Google Scholar] [CrossRef]
- Hackmann, E.; Hartmann, B.; Laemmerzahl, C.; Sirimachan, P. The Complete set of solutions of the geodesic equations in the space-time of a Schwarzschild black hole pierced by a cosmic string. Phys. Rev. D 2010, 81, 064016. [Google Scholar] [CrossRef]
- Grunau, S.; Khamesra, B. Geodesic motion in the (rotating) black string spacetime. Phys. Rev. D 2013, 87, 124019. [Google Scholar] [CrossRef]
- Ozdemir, F.; Ozdemir, N.; Kaynak, B.T. Multi-black holes solution with cosmic strings. Int. J. Mod. Phys. A 2004, 19, 1549–1557. [Google Scholar] [CrossRef]
- Aliev, A.N.; Galtsov, D.V. Gravitational Effects in the Field of a Central Body Threaded by a Cosmic String. Sov. Astron. Lett. 1988, 14, 48. [Google Scholar]
- Galtsov, D.V.; Masar, E. Geodesics in Space-times Containing Cosmic Strings. Class. Quant. Grav. 1989, 6, 1313–1341. [Google Scholar] [CrossRef]
- Chakraborty, S.; Biswas, L. Motion of test particles in the gravitational field of cosmic strings in different situations. Class. Quant. Grav. 1996, 13, 2153–2161. [Google Scholar] [CrossRef]
- Compère, G.; Liu, Y.; Long, J. Classification of radial Kerr geodesic motion. Phys. Rev. D 2022, 105, 024075. [Google Scholar] [CrossRef]
- Cieślik, A.; Hackmann, E.; Mach, P. Kerr geodesics in terms of Weierstrass elliptic functions. Phys. Rev. D 2023, 108, 024056. [Google Scholar] [CrossRef]
- Kraniotis, G.V. Precise relativistic orbits in Kerr space-time with a cosmological constant. Class. Quant. Grav. 2004, 21, 4743–4769. [Google Scholar] [CrossRef]
- Kraniotis, G.V. Gravitational redshift/blueshift of light emitted by geodesic test particles, frame-dragging and pericentre-shift effects, in the Kerr–Newman–de Sitter and Kerr–Newman black hole geometries. Eur. Phys. J. C 2021, 81, 147. [Google Scholar] [CrossRef]
- García, A.; Hackmann, E.; Kunz, J.; Lämmerzahl, C.; Macías, A. Motion of test particles in a regular black hole space–time. J. Math. Phys. 2015, 56, 032501. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, X. Geodesics on metrics of Eguchi–Hanson type. Eur. Phys. J. C 2023, 83, 574. [Google Scholar] [CrossRef]
- Deng, X.M. Geodesics and periodic orbits around quantum-corrected black holes. Phys. Dark Univ. 2020, 30, 100629. [Google Scholar] [CrossRef]
- Wei, S.W.; Yang, J.; Liu, Y.X. Geodesics and periodic orbits in Kehagias-Sfetsos black holes in deformed Horava-Lifshitz gravity. Phys. Rev. D 2019, 99, 104016. [Google Scholar] [CrossRef]
- Fathi, M.; Olivares, M.; Villanueva, J.R. Study of null and time-like geodesics in the exterior of a Schwarzschild black hole with quintessence and cloud of strings. Eur. Phys. J. C 2022, 82, 629. [Google Scholar] [CrossRef]
- Ospino, J.; Hernandez-Pastora, J.L.; Nunez, L.A. All analytic solutions for geodesic motion in axially symmetric space-times. Eur. Phys. J. C 2022, 82, 591, Erratum in Eur. Phys. J. C 2022, 82, 627. [Google Scholar] [CrossRef]
- Liu, Y.; Sun, B. The Analytical Solutions of Equatorial Geodesic Motion in Kerr Spacetime. arXiv 2023, arXiv:2305.11045. [Google Scholar] [CrossRef]
- Wang, C.Y.; Lee, D.S.; Lin, C.Y. Null and timelike geodesics in the Kerr-Newman black hole exterior. Phys. Rev. D 2022, 106, 084048. [Google Scholar] [CrossRef]
- Mummery, A.; Balbus, S. Complete characterization of the orbital shapes of the noncircular Kerr geodesic solutions with circular orbit constants of motion. Phys. Rev. D 2023, 107, 124058. [Google Scholar] [CrossRef]
- Frolov, V.P.; Krtous, P.; Kubiznak, D. Black holes, hidden symmetries, and complete integrability. Living Rev. Rel. 2017, 20, 6. [Google Scholar] [CrossRef]
- Okai, T. Global structure and thermodynamic property of the four-dimensional twisted Kerr solution. Prog. Theor. Phys. 1994, 92, 47–66. [Google Scholar] [CrossRef][Green Version]
- Wu, S.Q.; Cai, X. Massive complex scalar field in the Kerr-Sen geometry: Exact solution of wave equation and Hawking radiation. J. Math. Phys. 2003, 44, 1084–1088. [Google Scholar] [CrossRef]
- Hioki, K.; Miyamoto, U. Hidden symmetries, null geodesics, and photon capture in the Sen black hole. Phys. Rev. D 2008, 78, 044007. [Google Scholar] [CrossRef]
- Blaga, P.A.; Blaga, C. Bounded radial geodesics around a Kerr-Sen black hole. Class. Quant. Grav. 2001, 18, 3893–3905. [Google Scholar] [CrossRef]
- Houri, T.; Kubiznak, D.; Warnick, C.M.; Yasui, Y. Generalized hidden symmetries and the Kerr-Sen black hole. J. High Energy Phys. 2010, 07, 055. [Google Scholar] [CrossRef]
- Wu, D.; Wu, P.; Yu, H.; Wu, S.Q. Are ultraspinning Kerr-Sen- AdS4 black holes always superentropic? Phys. Rev. D 2020, 102, 044007. [Google Scholar] [CrossRef]
- Plebanski, J.F.; Demianski, M. Rotating, charged, and uniformly accelerating mass in general relativity. Annals Phys. 1976, 98, 98–127. [Google Scholar] [CrossRef]
- Carter, B. Hamilton-Jacobi and Schrodinger separable solutions of Einstein’s equations. Commun. Math. Phys. 1968, 10, 280–310. [Google Scholar] [CrossRef]
- Sen, A. Rotating charged black hole solution in heterotic string theory. Phys. Rev. Lett. 1992, 69, 1006–1009. [Google Scholar] [CrossRef]
- Carter, B. Global structure of the Kerr family of gravitational fields. Phys. Rev. 1968, 174, 1559–1571. [Google Scholar] [CrossRef]
- Wilkins, D.C. Bound Geodesics in the Kerr Metric. Phys. Rev. D 1972, 5, 814–822. [Google Scholar] [CrossRef]
- Mino, Y. Perturbative approach to an orbital evolution around a supermassive black hole. Phys. Rev. D 2003, 67, 084027. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, Z.; Liu, M.; Wang, W.-Q.; He, T.-Y.; Han, Z.-W.; Yang, R.-J. Analysis of the Geodesic Motions of Massive Particles in Kerr–Sen–AdS4 Spacetime. Universe 2024, 10, 133. https://doi.org/10.3390/universe10030133
Cai Z, Liu M, Wang W-Q, He T-Y, Han Z-W, Yang R-J. Analysis of the Geodesic Motions of Massive Particles in Kerr–Sen–AdS4 Spacetime. Universe. 2024; 10(3):133. https://doi.org/10.3390/universe10030133
Chicago/Turabian StyleCai, Ziqiang, Ming Liu, Wen-Qian Wang, Tong-Yu He, Zhan-Wen Han, and Rong-Jia Yang. 2024. "Analysis of the Geodesic Motions of Massive Particles in Kerr–Sen–AdS4 Spacetime" Universe 10, no. 3: 133. https://doi.org/10.3390/universe10030133
APA StyleCai, Z., Liu, M., Wang, W.-Q., He, T.-Y., Han, Z.-W., & Yang, R.-J. (2024). Analysis of the Geodesic Motions of Massive Particles in Kerr–Sen–AdS4 Spacetime. Universe, 10(3), 133. https://doi.org/10.3390/universe10030133





