A Tutorial on the Strong Gravity Effects in Black Hole X-Ray Spectra
Abstract
1. Introduction
1.1. Basic Concepts
- The specific intensity is independent of the distance of the source , while the spectral flux is proportional to the inverse of the square of the distance .
- The specific intensity can be seen as the energy flowing out of the source as well as the energy flowing into the detector and, in general, as the energy flowing along any photon trajectory.
- If a source is unresolved (i.e., it appears as a point-like source because its angular size is smaller than the angular resolution of the instrument used for its observation), we can measure the spectral flux , but we cannot measure the specific intensity .
1.2. Relativistic Effects
2. Thin Accretion Disk
2.1. Motion in a Stationary and Axisymmetric Spacetime
2.2. Infinitesimally Thin Disk
2.3. Motion in the Disk Region ()
2.4. Motion in the Plunging Region ()
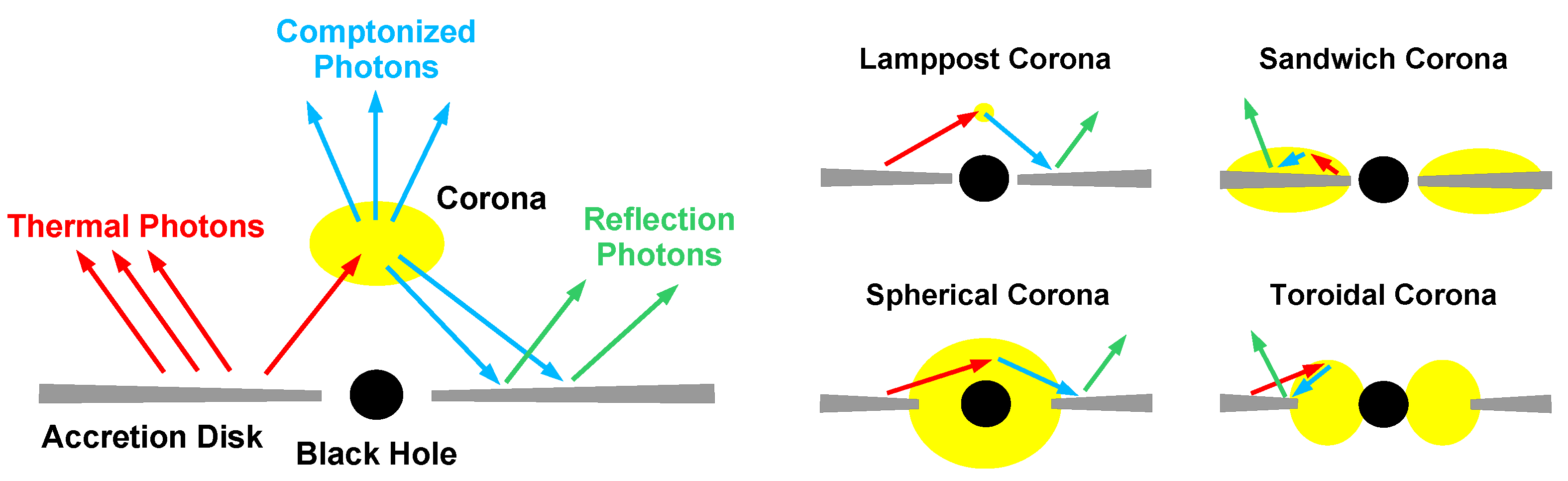
3. Corona
3.1. Coronal Spectrum
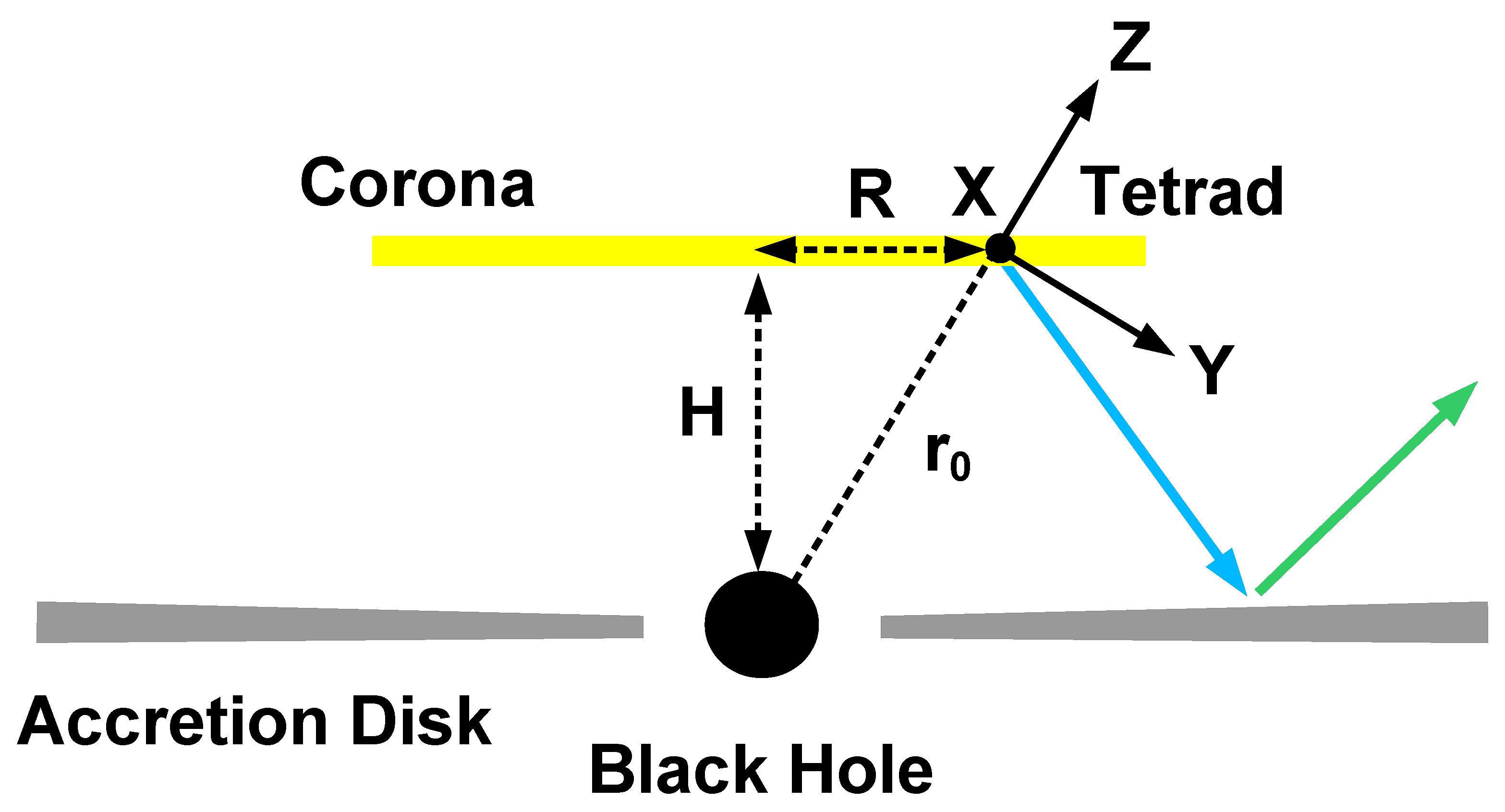
3.2. Illumination of the Accretion Disk
3.3. Spectral Flux Illuminating the Disk
4. Non-Relativistic Reflection Spectrum
5. Returning Radiation
6. Relativistic Reflection Spectrum
6.1. Photon Initial Conditions
6.2. Redshift Factor and Emission Angle
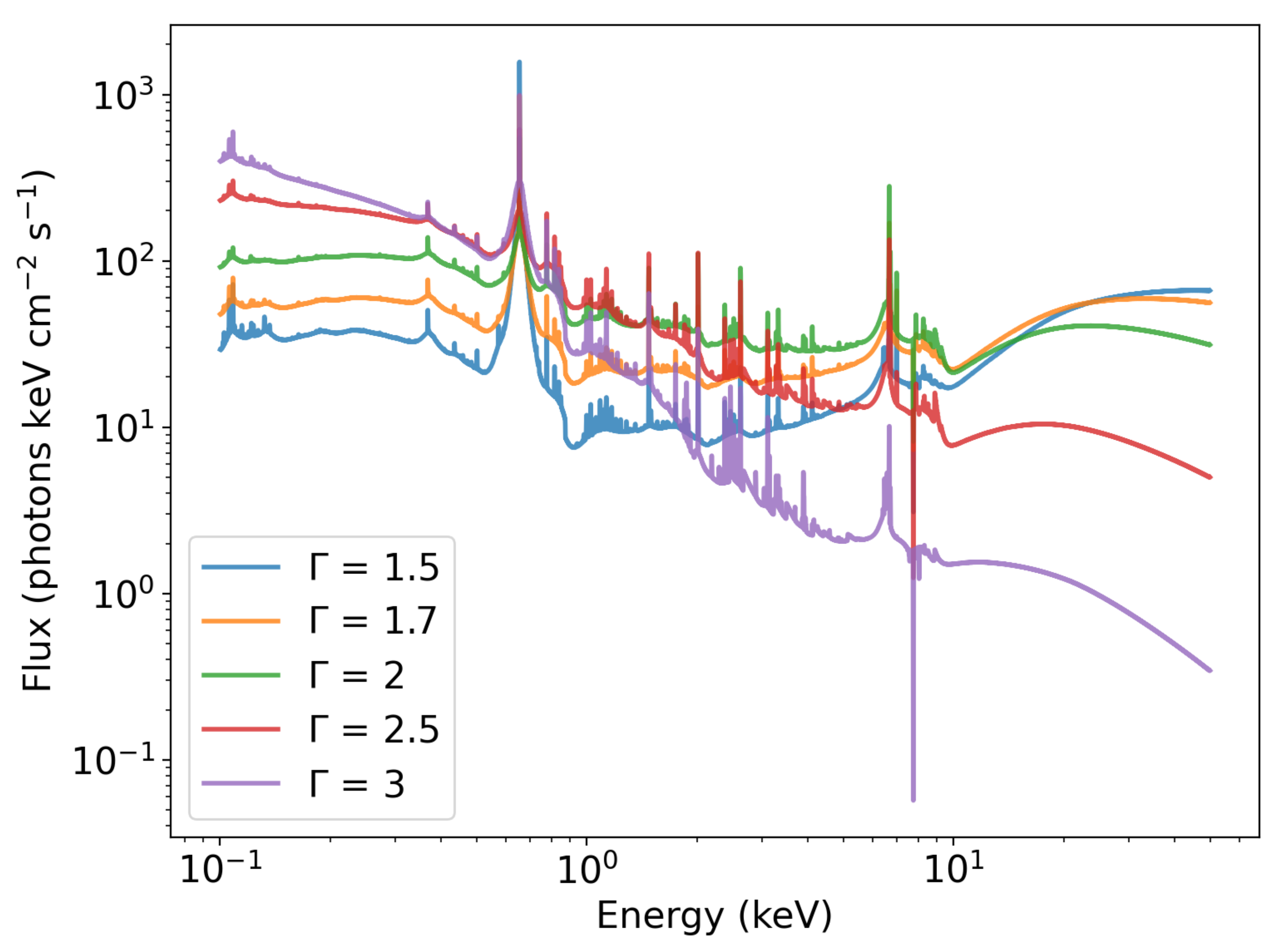
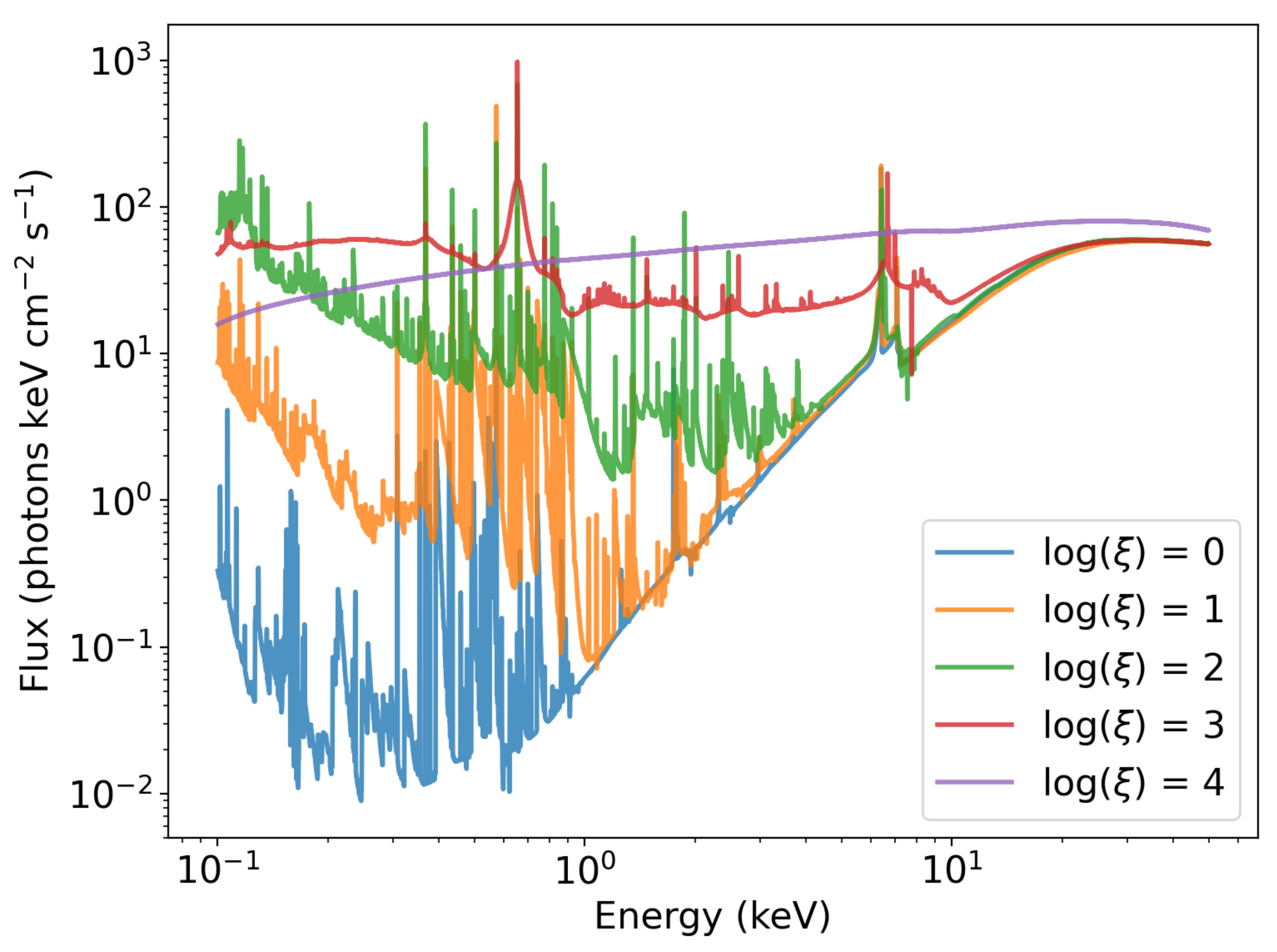
6.3. Examples of Relativistic Reflection Spectra
7. Novikov–Thorne Disk
7.1. Near Equatorial Metric
7.2. Radial Structure of the Disk
7.3. Plunging Region
8. Relativistic Thermal Spectrum
9. Cunningham’s Transfer Function
10. Comparison Between Theoretical Predictions and Observational Data
10.1. Requirements in the Analysis of Reflection Features with Current Reflection Models
10.2. Accuracy of the Theoretical Models
11. Concluding Remarks
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Locally Minkowskian Reference Frames
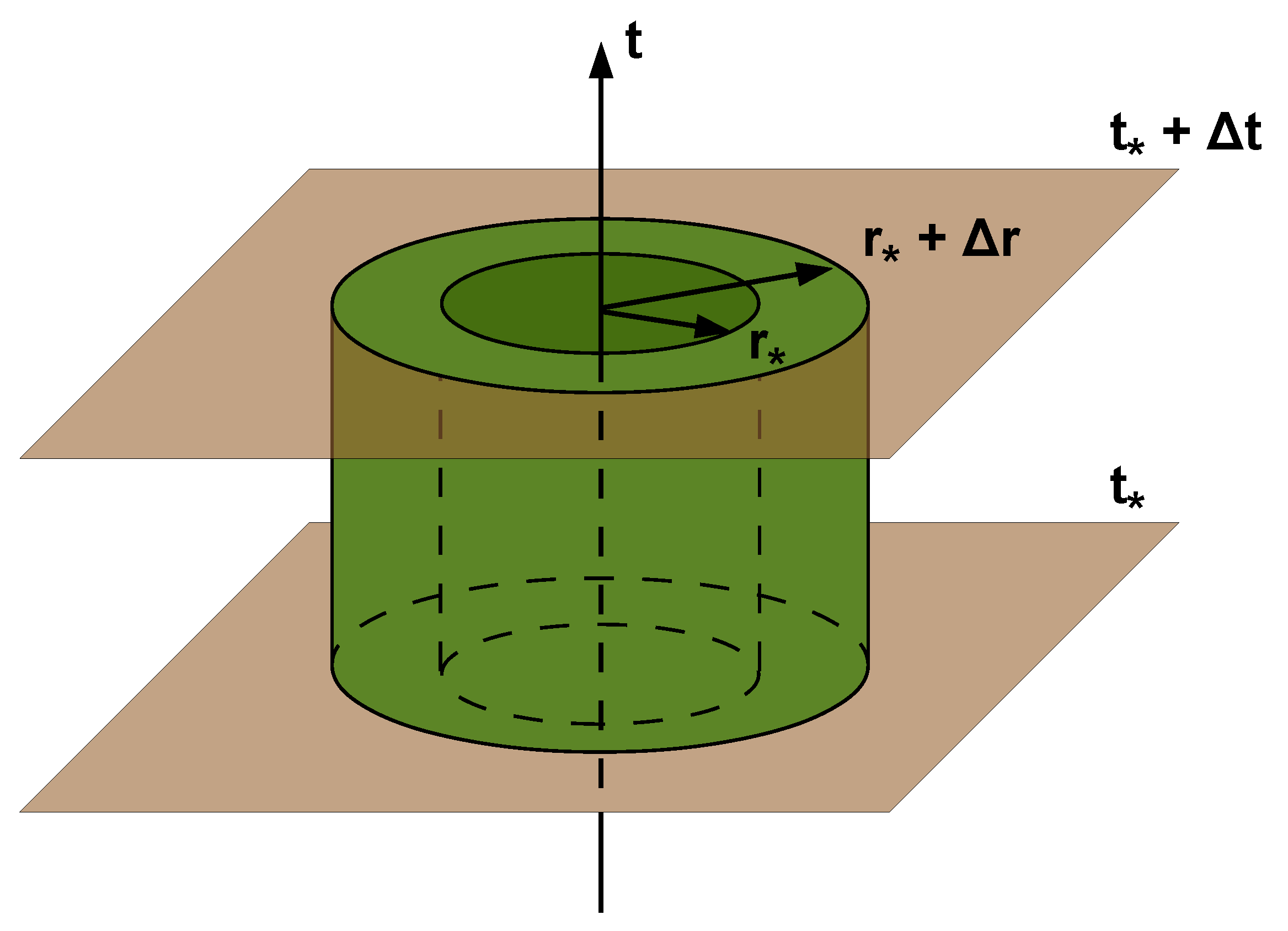
Appendix B. Proper Areas of the Annuli of an Accretion Disk
| 1 | Generally speaking, an accretion disk is geometrically thin if , where h is the thickness of the disk at the radial coordinate r, and is optically thick is , where is the photon mean free path in the disk. The accretion disk is instead geometrically thick if . The accretion disk is optically thin if . |
| 2 | This is normally a very acceptable approximation. Let us consider, for example, a 10 black hole in an X-ray binary. Its Eddington luminosity is erg . Its Eddington mass accretion rate can be found from the relation , where is the radiative efficiency, and we have /yr. In a typical outburst of a black hole binary, the luminosity of the source is around 10% of its Eddington limit and the outburst lasts for about a month, so the total mass in the accretion disk is roughly and the ratio between the mass of the disk and the mass of the black hole is of order . Even if the mass of the disk were confined in a relatively small space region (which is not the case), it could produce only a very small perturbation on the background metric. We can thus conclude that the gravitational field of the disk can be ignored. See, for instance, Ref. [32] for more details. |
| 3 | We note that we are not considering the motion of the single particles in the accretion disk (ions and electrons) but the motion of a “parcel” of particles. |
| 4 | We use the term “specific” because the Lagrangian of a point-like free particle is , and we set in Equation (12). |
| 5 | Here we ignore the “quasi” and we assume that the material in the disk follows geodesic equatorial circular orbits. However, the “quasi” is important to have accretion onto the black hole. It is indeed necessary a mechanism to transport energy and angular momentum outward, so the material of the disk can slowly inspiral onto the black hole. In reality, the magnetorotational instability is the mechanism responsible to transport energy and angular momentum outward and to permit the accretion process [33]. |
| 6 | The subindex is used to indicate that this is the Keplerian angular velocity of the material in the accretion disk and to avoid confusion in the formulas between such a quantity and the infinitesimal solid angle . |
| 7 | If the emission is isotropic, we can divide the sky of every emission point in small solid angles and fire a photon from each of these directions. Since , we need a grid of constant and . |
| 8 | For the proper area of an annulus in the plunging region, we can consider a locally non-rotating observer as described in Appendix B and evaluate the Lorentz factor with Equation (A18) by using the 4-velocity of the material in the plunging region for . |
| 9 | In models in which gravity does not universally couple to matter, we can have, for example, the phenomenon of variation of “fundamental” constants, namely constants like the fine structure constant , the electron mass , etc., may not be actual constants and may change in space and/or time [42]. For example, their value in the strong gravitational field of a black hole may be different from their value in our laboratories on Earth. In such a context, the atomic physics may depend on the gravitational field. |
| 10 | The public version of xillver has several “flavors”. One can choose among three types of incident spectra: a power law with a high-energy cutoff (2 parameters: photon index and high-energy cutoff ); a Comptonized spectrum (2 parameters: photon index and electron temperature of the corona , while the temperature of the seed photons is eV); a blackbody spectrum (1 parameter: blackbody temperature ). The cold material is characterized by the ionization parameter , the iron abundance (while all other elements have Solar abundances), and in some flavors there is also the electron density N. The emission angle is another parameter of the model, while the incident angle is and cannot be changed. |
| 11 | While current X-ray observatories do not have the necessary angular resolution to resolve the accretion disk of a black hole and the system appears as a point-like source, we have to consider an ideal observer with an excellent angular resolution in order to calculate the theoretical spectrum of an accretion disk within our model. |
| 12 | Starting from Ref. [45], ray-tracing codes to calculate images of accretion disks around black holes have been significantly developed and today there are a number of public codes that can do these calculations. |
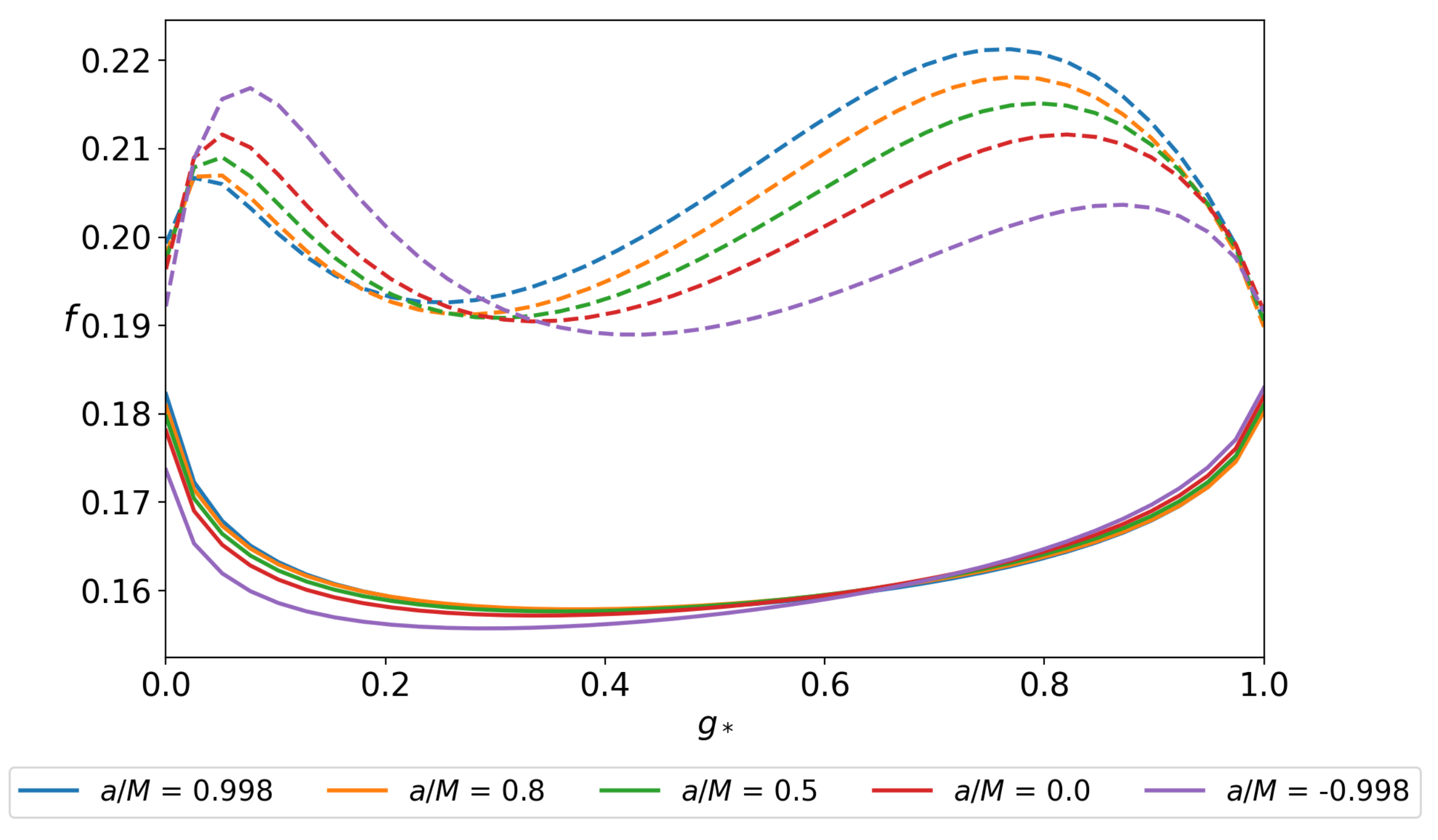
| 13 | In the soft state, when the X-ray spectrum of black hole X-ray binaries is dominated by the thermal spectrum of the disk, powerful disk winds are common [52]. While current calculations of the color factor ignore disk winds, the latter may affect the color temperature. |
| 14 | These results, strictly speaking, are valid in the case of infinitesimally thin Keplerian accretion disk in Kerr spacetimes. They are normally valid even in the case of infinitesimally thin Keplerian accretion disk in non-Kerr spacetimes, but exceptions are possible. In the case of accretion disks of a finite thickness, some parts of the disk may not be visible to a distant observer, so the image of the points on the accretion disk at the same emission radius may not be a closed loop [54]. |
| 15 | FITS (Flexible Image Transport System) is a common format for astronomical data files that can store multidimensional arrays and tables. |
| 16 | This approach is motivated by the need to be able to calculate quickly a relativistic reflection spectrum. Note that the ionization parameter is , where F is the total flux illuminating the disk at the radial coordinate r and n is the electron density of the disk at the same radial coordinate. A model in which the ionization parameter and the disk electron density n are constant over the disk would require that F is constant too. This is not how most of the current models work: they employ , which is calculated for certain values of and n, and model the emissivity profile with . |
| 17 | In Model 1, we have the inclination angle of the disk i in relconv, while reflionx does not have the emission angle as a parameter of the model. In Model 2, the value of the inclination angle of the disk i in relconv is tied to that of the emission angle in xillver, even if the two angles are different, in general, and should change value over the disk. In Model 3, there is only the inclination angle of the disk i in relxill: the model calculates the averaged emission angle and extracts the corresponding spectrum from the xillver table. |
| 18 | The transformation (A1) reduces the metric tensor to the Minkowski metric at a point of the spacetime, which is always possible because it is equivalent to make diagonal a symmetric matrix with constant coefficients and then rescale the coordinates to reduce the diagonal elements to . See, for example, Ref. [41] for more details. |
| 19 | Please note the notation adopted in this tutorial. is used to indicate a generic angular velocity, while the Keplerian angular velocity of the material in the disk is indicated by and the angular velocity of a locally non-rotating reference frame is indicated by . |
| 20 | From Equation (14) we see that a particle with has a non-vanishing angular velocity in the coordinate system : . A locally non-rotating observer is thus an observer with vanishing and its non-vanishing angular momentum is due to the frame dragging of the spacetime. |
References
- Page, D.N.; Thorne, K.S. Disk-Accretion onto a Black Hole. Time-Averaged Structure of Accretion Disk. Astrophys. J. 1974, 191, 499–506. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Bisnovatyi-Kogan, G.S.; Blinnikov, S.I. Disk accretion onto a black hole at subcritical luminosity. Astron. Astrophys. 1977, 59, 111–125. [Google Scholar]
- Dove, J.B.; Wilms, J.; Maisack, M.; Begelman, M.C. Self-consistent thermal accretion disk corona models for compact objects. 2. Application to cygnus x-1. Astrophys. J. 1997, 487, 759. [Google Scholar] [CrossRef]
- Haardt, F.; Maraschi, L.A.M.U. A two-phase model for the X-ray emission from Seyfert galaxies. Astrophys. J. Lett. 1991, 380, L51–L54. [Google Scholar] [CrossRef]
- Liu, B.F.; Mineshige, S.; Ohsuga, K. Spectra from a magnetic reconnection-heated corona in agn. Astrophys. J. 2003, 587, 571–579. [Google Scholar] [CrossRef]
- Markoff, S.; Nowak, M.A.; Wilms, J. Going with the flow: Can the base of jets subsume the role of compact accretion disk coronae? Astrophys. J. 2005, 635, 1203–1216. [Google Scholar] [CrossRef]
- Petrucci, P.O.; Paltani, S.; Malzac, J.; Kaastra, J.S.; Cappi, M.; Ponti, G.; De Marco, B.; Kriss, G.A.; Steenbrugge, K.C.; Bianchi, S.; et al. Multiwavelength campaign on Mrk 509 XII. Broad band spectral analysis. Astron. Astrophys. 2013, 549, A73. [Google Scholar] [CrossRef]
- Sironi, L.; Beloborodov, A.M. Kinetic Simulations of Radiative Magnetic Reconnection in the Coronae of Accreting Black Holes. Astrophys. J. 2020, 899, 52. [Google Scholar] [CrossRef]
- Titarchuk, L. Generalized Comptonization models and application to the recent high-energy observations. Astrophys. J. 1994, 434, 570–586. [Google Scholar] [CrossRef]
- Kara, E.; Steiner, J.F.; Fabian, A.C.; Cackett, E.M.; Uttley, P.; Remillard, R.A.; Gendreau, K.C.; Arzoumanian, Z.; Altamirano, D.; Eikenberry, S.; et al. The corona contracts in a black-hole transient. Nature 2019, 565, 198. [Google Scholar] [CrossRef] [PubMed]
- Dai, X.; Kochanek, C.S.; Chartas, G.; Kozlowski, S.; Morgan, C.W.; Garmire, G.; Agol, E. The Sizes of the X-ray and Optical Emission Regions of RXJ1131-1231. Astrophys. J. 2010, 709, 278–285. [Google Scholar] [CrossRef]
- Wilkins, D.R.; Gallo, L.C. Driving extreme variability: The evolving corona and evidence for jet launching in Markarian 335. Mon. Not. R. Astron. Soc. 2015, 449, 129–146. [Google Scholar] [CrossRef]
- Zdziarski, A.A.; Johnson, W.N.; Magdziarz, P. Broad-band gamma-ray and x-ray spectra of ngc 4151 and their implications for physical processes and geometry. Mon. Not. R. Astron. Soc. 1996, 283, 193. [Google Scholar] [CrossRef]
- Zdziarski, A.A.; Szanecki, M.; Poutanen, J.; Gierlinski, M.; Biernacki, P. Spectral and temporal properties of Compton scattering by mildly relativistic thermal electrons. Mon. Not. R. Astron. Soc. 2020, 492, 5234–5246. [Google Scholar] [CrossRef]
- Bambi, C. Black hole X-ray spectra: Notes on the relativistic calculations. arXiv 2024, arXiv:2408.12262. [Google Scholar]
- Garcia, J.; Kallman, T. X-ray reflected spectra from accretion disk models. I. Constant density atmospheres. Astrophys. J. 2010, 718, 695. [Google Scholar] [CrossRef]
- Ross, R.R.; Fabian, A.C. A Comprehensive range of x-ray ionized reflection models. Mon. Not. R. Astron. Soc. 2005, 358, 211–216. [Google Scholar] [CrossRef]
- Bambi, C. Black Holes: A Laboratory for Testing Strong Gravity; Springer: Singapore, 2017; ISBN 978-981-10-4524-0. [Google Scholar] [CrossRef]
- Fabian, A.C.; Rees, M.J.; Stella, L.; White, N.E. X-ray fluorescence from the inner disc in Cygnus X-1. Mon. Not. R. Astron. Soc. 1989, 238, 729–736. [Google Scholar] [CrossRef]
- Kojima, Y. The effects of black hole rotation on line profiles from accretion discs. Mon. Not. R. Astron. Soc. 1991, 250, 629. [Google Scholar] [CrossRef][Green Version]
- Laor, A. Line profiles from a disk around a rotating black hole. Astrophys. J. 1991, 376, 90. [Google Scholar] [CrossRef]
- Bambi, C.; Brenneman, L.W.; Dauser, T.; Garcia, J.A.; Grinberg, V.; Ingram, A.; Jiang, J.; Liu, H.; Lohfink, A.M.; Marinucci, A.; et al. Towards Precision Measurements of Accreting Black Holes Using X-Ray Reflection Spectroscopy. Space Sci. Rev. 2021, 217, 65. [Google Scholar] [CrossRef]
- Brenneman, L.W.; Reynolds, C.S. Constraining Black Hole Spin Via X-ray Spectroscopy. Astrophys. J. 2006, 652, 1028–1043. [Google Scholar] [CrossRef]
- Draghis, P.A.; Miller, J.M.; Costantini, E.; Gallo, L.C.; Reynolds, M.; Tomsick, J.A.; Zoghbi, A. Systematically Revisiting All NuSTAR Spins of Black Holes in X-Ray Binaries. Astrophys. J. 2024, 969, 40. [Google Scholar] [CrossRef]
- Cao, Z.; Nampalliwar, S.; Bambi, C.; Dauser, T.; Garcia, J.A. Testing general relativity with the reflection spectrum of the supermassive black hole in 1H0707-495. Phys. Rev. Lett. 2018, 120, 51101. [Google Scholar] [CrossRef]
- Tripathi, A.; Nampalliwar, S.; Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Dauser, T.; Garcia, J.A.; Marinucci, A. Toward Precision Tests of General Relativity with Black Hole X-Ray Reflection Spectroscopy. Astrophys. J. 2019, 875, 56. [Google Scholar] [CrossRef]
- Tripathi, A.; Zhang, Y.; Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Jiang, J.; Liu, H.; Zhou, M. Testing General Relativity with NuSTAR data of Galactic Black Holes. Astrophys. J. 2021, 913, 79. [Google Scholar] [CrossRef]
- Papapetrou, A. Champs gravitationnels stationnaires a symetrie axiale. Ann. Inst. H. Poincare Phys. Theor. 1966, 4, 83–105. [Google Scholar]
- Wald, R.M. General Relativity; Chicago University Press: Chicago, IL, USA, 1984. [Google Scholar] [CrossRef]
- Lindquist, R.W. Relativistic transport theory. Ann. Phys. 1966, 37, 487–518. [Google Scholar] [CrossRef]
- Bambi, C.; Malafarina, D.; Tsukamoto, N. Note on the effect of a massive accretion disk in the measurements of black hole spins. Phys. Rev. D 2014, 89, 127302. [Google Scholar] [CrossRef]
- Balbus, S.A.; Hawley, J.F. A powerful local shear instability in weakly magnetized disks. 1. Linear analysis. 2. Nonlinear evolution. Astrophys. J. 1991, 376, 214–233. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Press, W.H.; Teukolsky, S.A. Rotating black holes: Locally nonrotating frames, energy extraction, and scalar synchrotron radiation. Astrophys. J. 1972, 178, 347. [Google Scholar] [CrossRef]
- Bambi, C.; Barausse, E. The Final stages of accretion onto non-Kerr compact objects. Phys. Rev. D 2011, 84, 84034. [Google Scholar] [CrossRef]
- Bambi, C.; Lukes-Gerakopoulos, G. Testing the existence of regions of stable orbits at small radii around black hole candidates. Phys. Rev. D 2013, 87, 83006. [Google Scholar] [CrossRef]
- Shashank, S.; Riaz, S.; Abdikamalov, A.B.; Bambi, C. Testing Relativistic Reflection Models with GRMHD Simulations of Accreting Black Holes. Astrophys. J. 2022, 938, 53. [Google Scholar] [CrossRef]
- Riaz, S.; Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Wang, H.; Yu, Z. Reflection Spectra of Accretion Disks Illuminated by Disk-like Coronae. Astrophys. J. 2022, 925, 51. [Google Scholar] [CrossRef]
- Dauser, T.; Garcia, J.; Wilms, J.; Bock, M.; Brenneman, L.W.; Falanga, M.; Fukumura, K.; Reynolds, C.S. Irradiation of an Accretion Disc by a Jet: General Properties and Implications for Spin Measurements of Black Holes. Mon. Not. R. Astron. Soc. 2013, 430, 1694. [Google Scholar] [CrossRef]
- Fukumura, K.; Kazanas, D. Accretion Disk Illumination in Schwarzschild and Kerr Geometries: Fitting Formulae. Astrophys. J. 2007, 664, 14–25. [Google Scholar] [CrossRef]
- Bambi, C. Introduction to General Relativity; Springer: Singapore, 2018; ISBN 978-981-13-1089-8/978-981-13-1090-4. [Google Scholar] [CrossRef]
- Bambi, C. Search for Variations of Fundamental Constants in the book. In Recent Progress on Gravity Tests: Challenges and Future Perspectives; Bambi, C., Cardenas-Avendano, A., Eds.; Springer: Singapore, 2024; pp. 417–432. [Google Scholar] [CrossRef]
- Mirzaev, T.; Riaz, S.; Abdikamalov, A.B.; Bambi, C.; Dauser, T.; Garcia, J.A.; Jiang, J.; Liu, H.; Shashank, S. Toward More Accurate Synthetic Reflection Spectra: Improving the Calculations of Returning Radiation. Astrophys. J. 2024, 965, 66. [Google Scholar] [CrossRef]
- Mirzaev, T.; Bambi, C.; Abdikamalov, A.B.; Jiang, J.; Liu, H.; Riaz, S.; Shashank, S. X-Ray Spectra of Black Hole X-Ray Binaries with Returning Radiation. Astrophys. J. 2024, 976, 229. [Google Scholar] [CrossRef]
- Luminet, J.P. Image of a spherical black hole with thin accretion disk. Astron. Astrophys. 1979, 75, 228–235. [Google Scholar]
- Reynolds, C.S.; Begelman, M.C. Iron fluorescence from within the innermost stable orbit of black hole accretion disks. Astrophys. J. 1997, 488, 109. [Google Scholar] [CrossRef]
- García, J.; Dauser, T.; Lohfink, A.; Kallman, T.R.; Steiner, J.; McClintock, J.E.; Brenneman, L.; Wilms, J.; Eikmann, W.; Reynolds, C.S.; et al. Improved Reflection Models of Black-Hole Accretion Disks: Treating the Angular Distribution of X-rays. Astrophys. J. 2014, 782, 76. [Google Scholar] [CrossRef]
- Novikov, I.D.; Thorne, K.S. Astrophysics of Black Holes. In Black Holes; De Witt, C., De Witt, B., Eds.; Gordon and Breach: New York, NY, USA, 1973; pp. 343–450. [Google Scholar]
- Davis, S.W.; Blaes, O.M.; Hubeny, I.; Turner, N.J. Relativistic accretion disk models of high state black hole x-ray binary spectra. Astrophys. J. 2005, 621, 372–387. [Google Scholar] [CrossRef]
- Davis, S.W.; Hubeny, I. A grid of relativistic, non-lte accretion disk models for spectral fitting of black hole binaries. Astrophys. J. Suppl. 2006, 164, 530. [Google Scholar] [CrossRef]
- Shimura, T.; Takahara, F. On the spectral hardening factor of the X-ray emission from accretion disks in black hole candidates. Astrophys. J. 1995, 445, 780–788. [Google Scholar] [CrossRef]
- Miller, J.M.; Fabian, A.C.; Kaastra, J.; Kallman, T.; King, A.L.; Proga, D.; Raymond, J.; Reynolds, C.S. Powerful, Rotating Disk Winds from Stellar-mass Black Holes. Astrophys. J. 2015, 814, 87. [Google Scholar] [CrossRef]
- Mummery, A.; Ingram, A.; Davis, S.; Fabian, A. Continuum emission from within the plunging region of black hole discs. Mon. Not. R. Astron. Soc. 2024, 531, 366–386. [Google Scholar] [CrossRef]
- Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Dauser, T.; Garcia, J.A.; Nampalliwar, S.; Tripathi, A.; Zhou, M. Testing the Kerr black hole hypothesis using X-ray reflection spectroscopy and a thin disk model with finite thickness. Astrophys. J. 2020, 899, 80. [Google Scholar] [CrossRef]
- Bambi, C.; Cardenas-Avendano, A.; Dauser, T.; Garcia, J.A.; Nampalliwar, S. Testing the Kerr black hole hypothesis using X-ray reflection spectroscopy. Astrophys. J. 2017, 842, 76. [Google Scholar] [CrossRef]
- Cunningham, C.T. The effects of redshifts and focusing on the spectrum of an accretion disk around a Kerr black hole. Astrophys. J. 1975, 202, 788–802. [Google Scholar] [CrossRef]
- Speith, R.; Riffert, H.; Ruder, H. The photon transfer function for accretion disks around a Kerr black hole. Comput. Phys. Comm. 1995, 88, 109–120. [Google Scholar] [CrossRef]
- Zhou, M.; Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Liu, H.; Nampalliwar, S. XSPEC model for testing the Kerr black hole hypothesis using the continuum-fitting method. Phys. Rev. D 2019, 99, 104031. [Google Scholar] [CrossRef]
- Gonzalez, A.G.; Wilkins, D.R.; Gallo, L.C. Probing the geometry and motion of AGN coronae through accretion disc emissivity profiles. Mon. Not. R. Astron. Soc. 2017, 472, 1932–1945. [Google Scholar] [CrossRef]
- Tripathi, A.; Yan, J.; Yang, Y.; Yan, Y.; Garnham, M.; Yao, Y.; Li, S.; Ding, Z.; Abdikamalov, A.B.; Ayzenberg, D.; et al. Constraints on the Spacetime Metric around Seven “Bare” AGNs Using X-Ray Reflection Spectroscopy. Astrophys. J. 2019, 874, 135. [Google Scholar] [CrossRef]
- Fabian, A.C.; Vaughan, S.; Nandra, K.; Iwasawa, K.; Ballantyne, D.R.; Lee, J.C.; De Rosa, A.; Turner, A.; Young, A.J. A Long hard look at MCG-6-30-15 with XMM-Newton. Mon. Not. R. Astron. Soc. 2002, 335, L1. [Google Scholar] [CrossRef]
- Iwasawa, K.; Fabian, A.C.; Reynolds, C.S.; Nandra, K.; Otani, C.; Inoue, H.; Hayashida, K.; Brandt, W.N.; Dotani, T.; Kunieda, H.; et al. The Variable iron k emission line in MCG-6-30-15. Mon. Not. R. Astron. Soc. 1996, 282, 1038–1048. [Google Scholar] [CrossRef]
- Guainazzi, M.; Matt, G.; Molendi, S.; Orr, A.; Fiore, F.; Grandi, P.; Matteuzzi, A.; Mineo, T.; Perola, G.C.; Parmar, A.N.; et al. BeppoSAX confirms extreme relativistic effects in the X-ray spectrum of MCG-6-30-15. Astron. Astrophys. 1999, 341, L27. [Google Scholar]
- Marinucci, A.; Matt, G.; Miniutti, G.; Guainazzi, M.; Parker, M.L.; Brenneman, L.; Fabian, A.C.; Kara, E.; Arevalo, P.; Ballantyne, D.R.; et al. The Broadband Spectral Variability of MCG–6-30-15 Observed by NuSTAR and XMM-Newton. Astrophys. J. 2014, 787, 83. [Google Scholar] [CrossRef]
- Miniutti, G.; Fabian, A.C.; Anabuki, N.; Crummy, J.; Fukazawa, Y.; Gallo, L.; Haba, Y.; Hayashida, K.; Holt, S.; Kunieda, H.; et al. Suzaku observations of the hard X-ray variability of MCG-6-30-15: The effects of strong gravity around a Kerr black hole. Publ. Astron. Soc. Jap. 2007, 59, 315. [Google Scholar] [CrossRef]
- Tanaka, Y.; Nandra, K.; Fabian, A.C.; Inoue, H.; Otani, C.; Dotani, T.; Hayashida, K.; Iwasawa, K.; Kii, T.; Kunieda, H.; et al. Gravitationally Redshifted Emission Implying an Accretion Disk and Massive Black Hole in the Active Galaxy MCG:-6-30-15. Nature 1995, 375, 659. [Google Scholar] [CrossRef]
- Tripathi, A.; Liu, H.; Bambi, C. Impact of the reflection model on the estimate of the properties of accreting black holes. Mon. Not. R. Astron. Soc. 2020, 498, 3565–3577. [Google Scholar] [CrossRef]
- Kammoun, E.S.; Nardini, E.; Risaliti, G. Testing the accuracy of reflection-based supermassive black hole spin measurements in AGN. Astron. Astrophys. 2018, 614, A44. [Google Scholar] [CrossRef]
- Fabian, A.C.; Parker, M.L.; Wilkins, D.R.; Miller, J.M.; Kara, E.; Reynolds, C.S.; Dauser, T. On the determination of the spin and disc truncation of accreting black holes using X-ray reflection. Mon. Not. R. Astron. Soc. 2014, 439, 2307–2313. [Google Scholar] [CrossRef]
- Steiner, J.F.; McClintock, J.E.; Remillard, R.A.; Gou, L.; Yamada, S.; Narayan, R. The Constant Inner-Disk Radius of LMC X-3: A Basis for Measuring Black Hole Spin. Astrophys. J. Lett. 2010, 718, L117–L121. [Google Scholar] [CrossRef]
- Tripathi, A.; Abdikamalov, A.B.; Ayzenberg, D.; Bambi, C.; Liu, H. Impact of the Disk Thickness on X-Ray Reflection Spectroscopy Measurements. Astrophys. J. 2021, 913, 129. [Google Scholar] [CrossRef]
- Riaz, S.; Ayzenberg, D.; Bambi, C.; Nampalliwar, S. Reflection spectra of thick accretion discs. Mon. Not. R. Astron. Soc. 2020, 491, 417–426. [Google Scholar] [CrossRef]
- Riaz, S.; Ayzenberg, D.; Bambi, C.; Nampalliwar, S. Modeling bias in supermassive black hole spin measurements. Astrophys. J. 2020, 895, 61. [Google Scholar] [CrossRef]
- Wilkins, D.R.; Fabian, A.C. Determination of the X-ray reflection emissivity profile of 1H 0707-495. Mon. Not. R. Astron. Soc. 2011, 414, 1269–1277. [Google Scholar] [CrossRef]
- Reynolds, C.S.; Fabian, A.C. Broad iron K-alpha emission lines as a diagnostic of black hole spin. Astrophys. J. 2008, 675, 1048. [Google Scholar] [CrossRef]
- Cardenas-Avendano, A.; Zhou, M.; Bambi, C. Modeling uncertainties in X-ray reflection spectroscopy measurements II: Impact of the radiation from the plunging region. Phys. Rev. D 2020, 101, 123014. [Google Scholar] [CrossRef]
- Li, L.X.; Zimmerman, E.R.; Narayan, R.; McClintock, J.E. Multi-temperature blackbody spectrum of a thin accretion disk around a Kerr black hole: Model computations and comparison with observations. Astrophys. J. Suppl. 2005, 157, 335–370. [Google Scholar] [CrossRef]
- Dauser, T.; Garc, J.A.; Joyce, A.; Licklederer, S.; Connors, R.M.T.; Ingram, A.; Reynolds, C.S.; Wilms, J. The effect of returning radiation on relativistic reflection. Mon. Not. R. Astron. Soc. 2022, 514, 3965–3983. [Google Scholar] [CrossRef]
- Tashiro, M.; Maejima, H.; Toda, K.; Kelley, R.; Reichenthal, L.; Hartz, L.; Petre, R.; Williams, B.; Guainazzi, M.; Costantini, E.; et al. Status of x-ray imaging and spectroscopy mission (XRISM). Proc. SPIE Int. Soc. Opt. Eng. 2020, 11444, 1144422. [Google Scholar] [CrossRef]
- Barret, D.; Trong, T.L.; Herder, J.W.D.; Piro, L.; Barcons, X.; Huovelin, J.; Kelley, R.; Mas-Hesse, J.M.; Mitsuda, K.; Paltani, S.; et al. The Athena X-ray Integral Field Unit (X-IFU). Proc. SPIE Int. Soc. Opt. Eng. 2016, 9905, 99052F. [Google Scholar] [CrossRef]







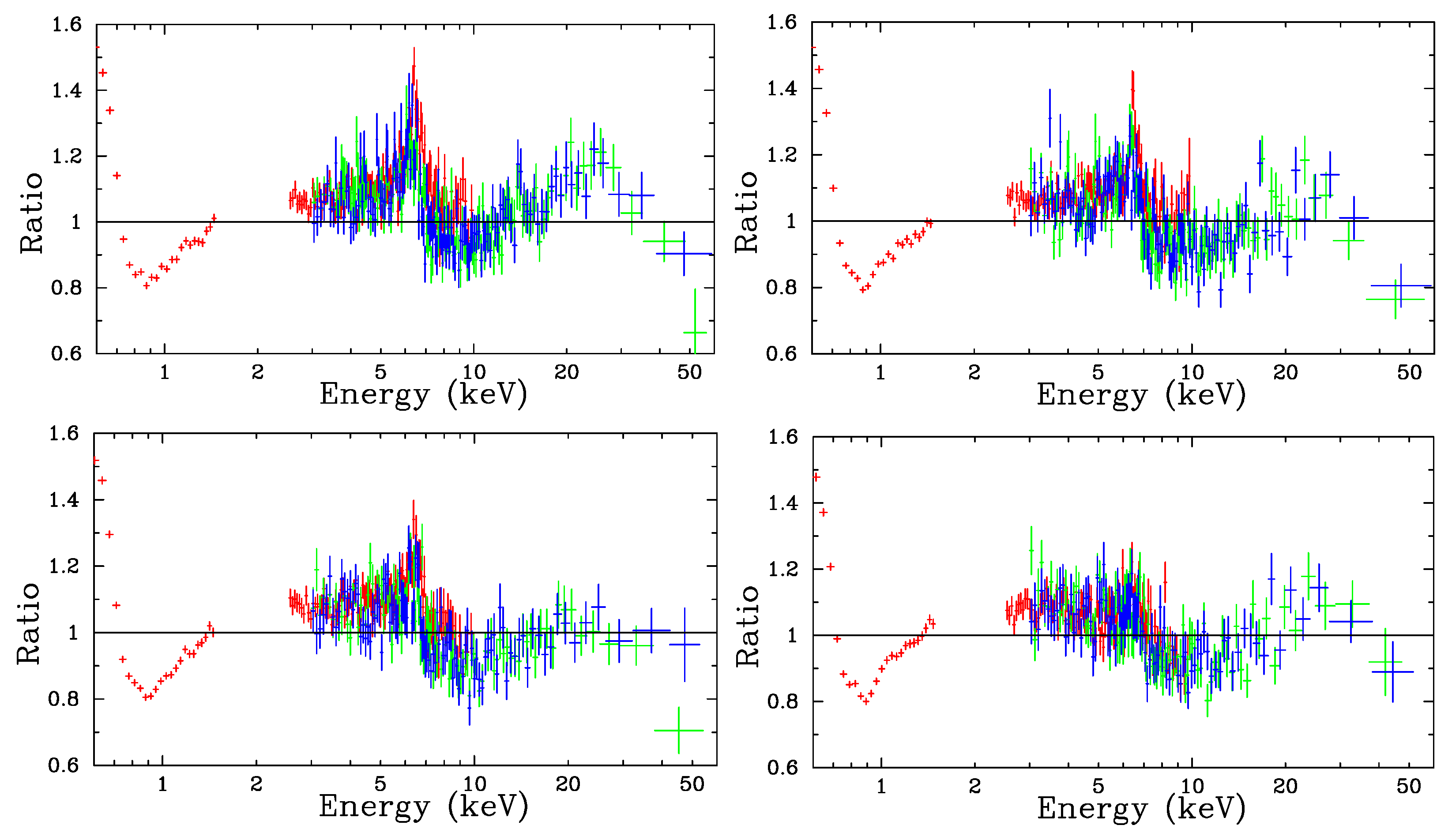
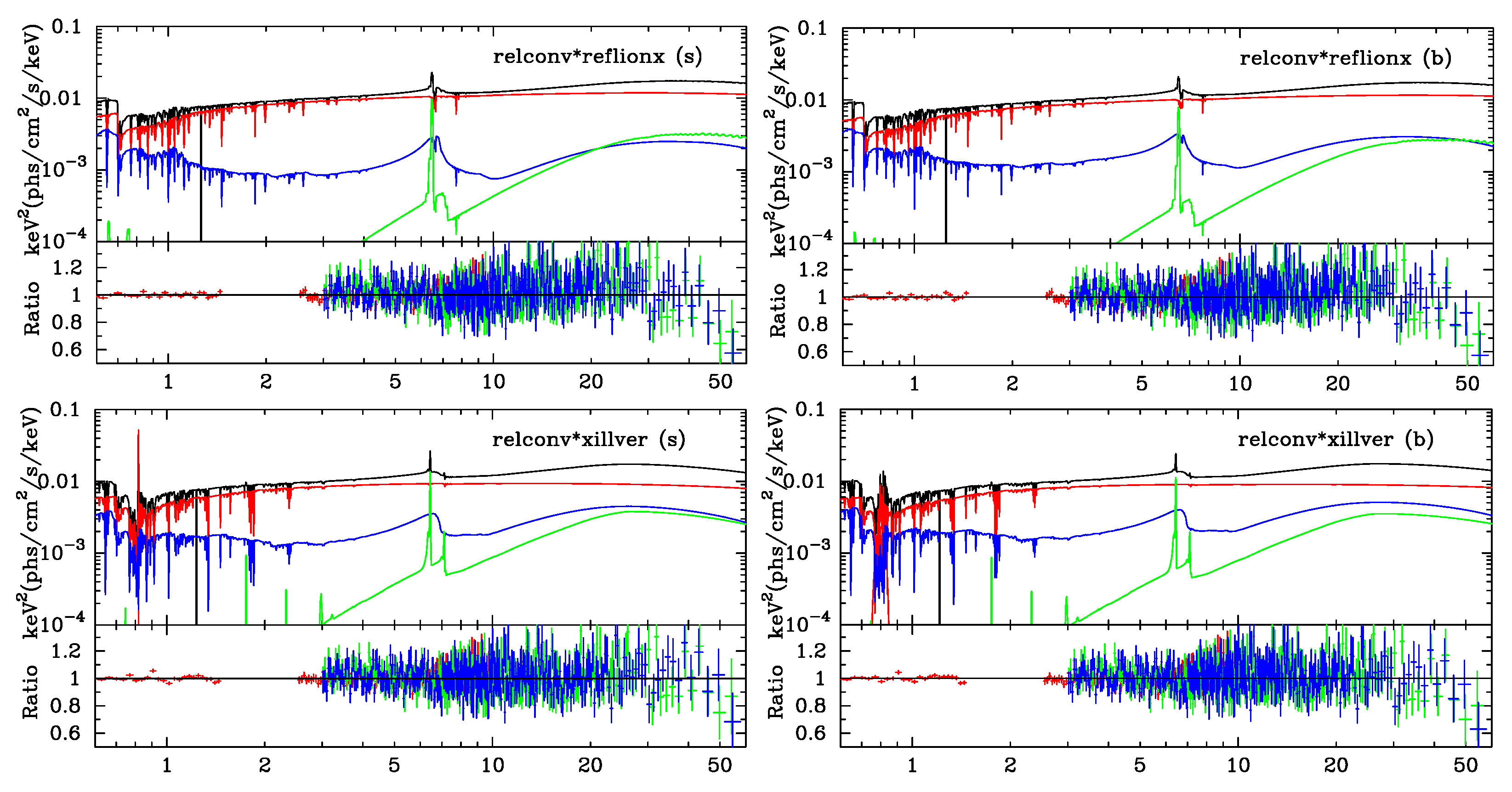
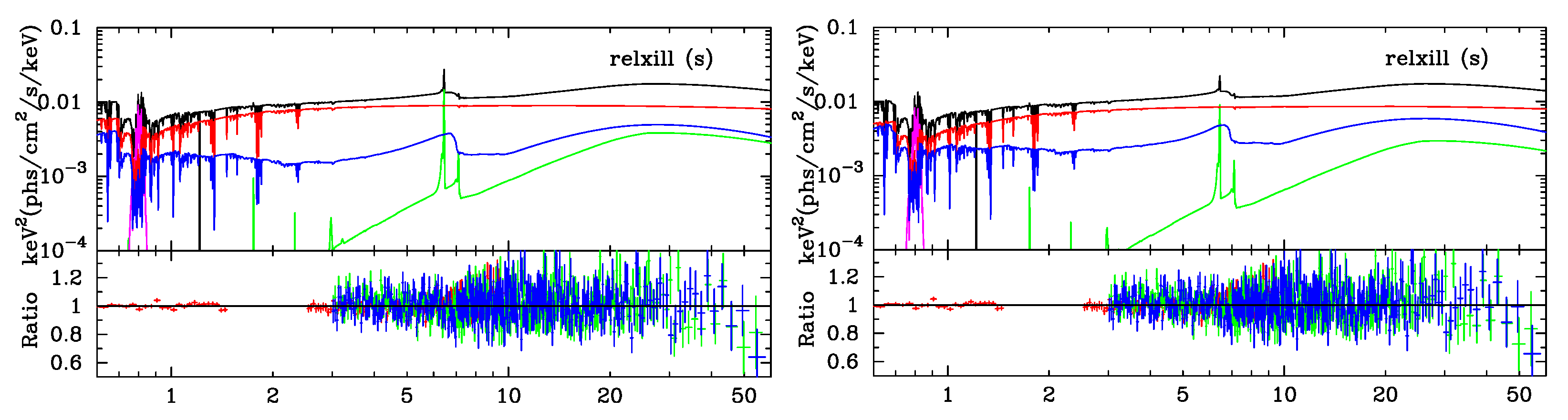
| Model 1 | Model 2 | Model 3 | ||||
|---|---|---|---|---|---|---|
| s | b | s | b | s | b | |
| i [deg] | ||||||
| 3171.36 | 3130.26 | 3094.68 | 3079.54 | 3081.74 | 3024.46 | |
| dof | 2691 | 2683 | 2689 | 2681 | 2691 | 2683 |
| /dof | 1.17850 | 1.16670 | 1.15087 | 1.14865 | 1.14520 | 1.12727 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bambi, C. A Tutorial on the Strong Gravity Effects in Black Hole X-Ray Spectra. Universe 2024, 10, 451. https://doi.org/10.3390/universe10120451
Bambi C. A Tutorial on the Strong Gravity Effects in Black Hole X-Ray Spectra. Universe. 2024; 10(12):451. https://doi.org/10.3390/universe10120451
Chicago/Turabian StyleBambi, Cosimo. 2024. "A Tutorial on the Strong Gravity Effects in Black Hole X-Ray Spectra" Universe 10, no. 12: 451. https://doi.org/10.3390/universe10120451
APA StyleBambi, C. (2024). A Tutorial on the Strong Gravity Effects in Black Hole X-Ray Spectra. Universe, 10(12), 451. https://doi.org/10.3390/universe10120451








