Multistability Emergence through Fractional-Order-Derivatives in a PWL Multi-Scroll System
Abstract
1. Introduction
2. Material and Methods
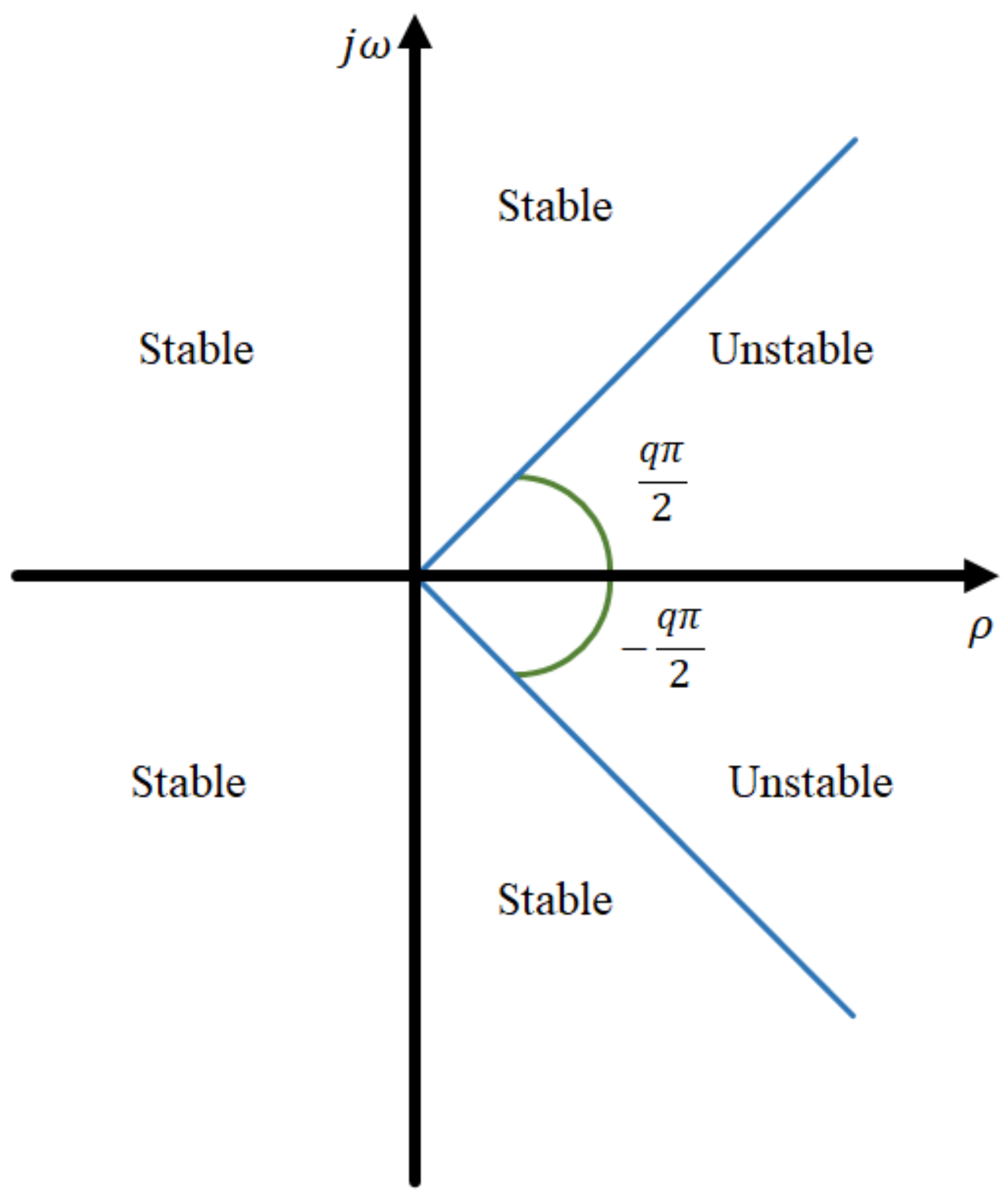
2.1. Fractional Calculus Preliminaries
- Stable, if and only if .
- Asymptotically stable, if and only if . In this case, the solution as .
- Unstable, if and only if , for at least one eigenvalue.
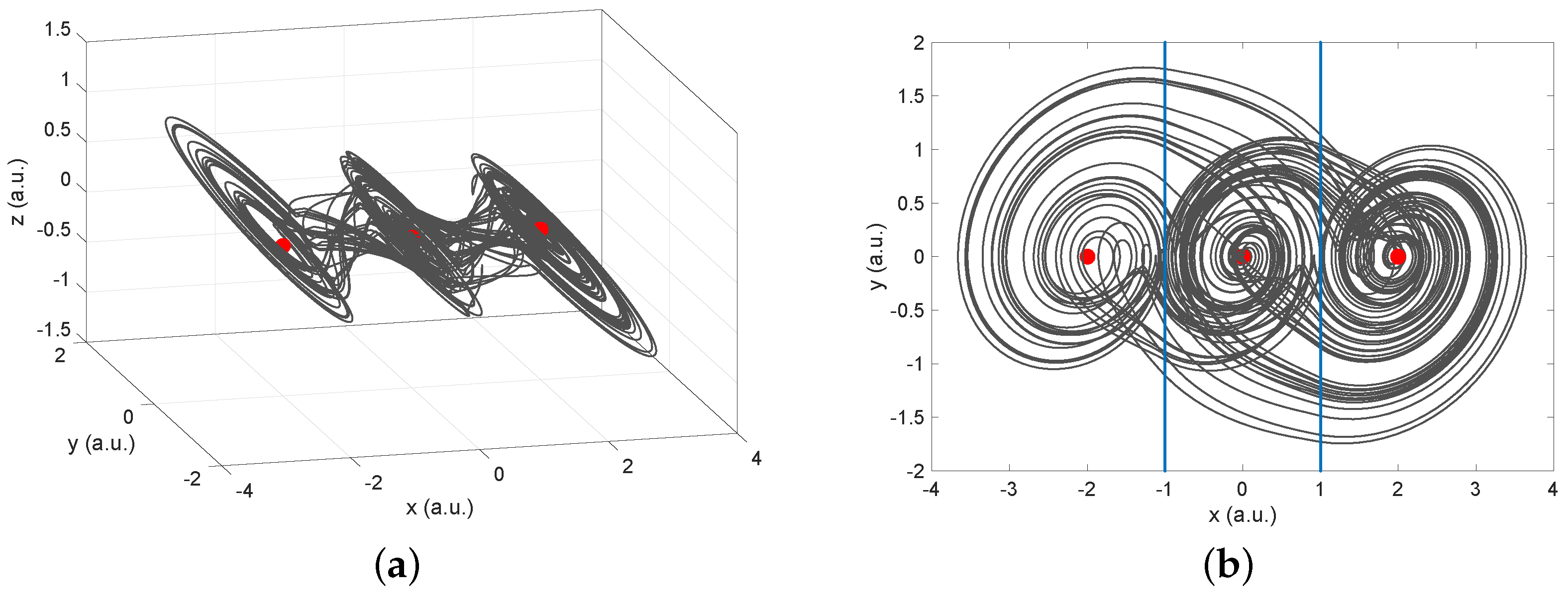
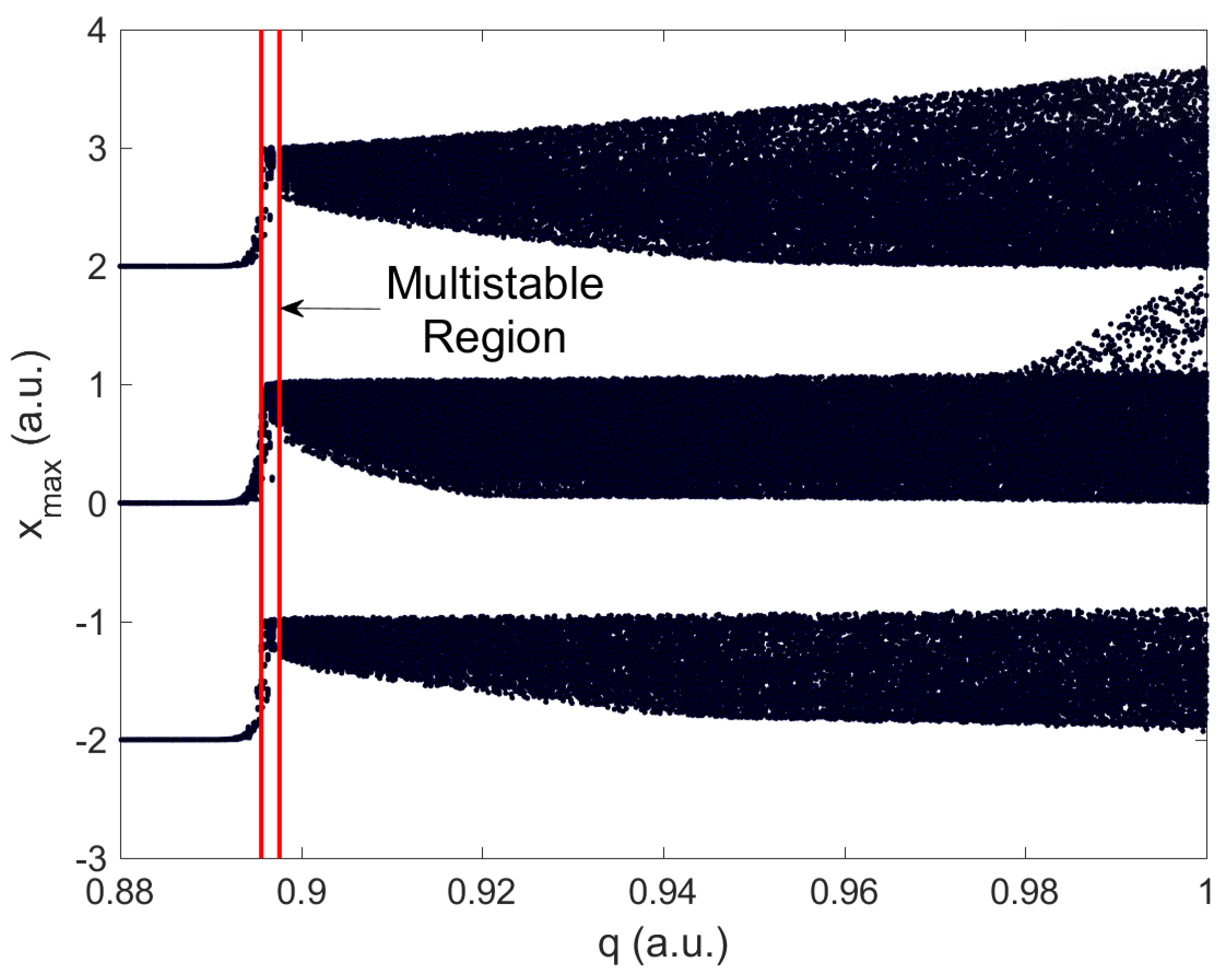
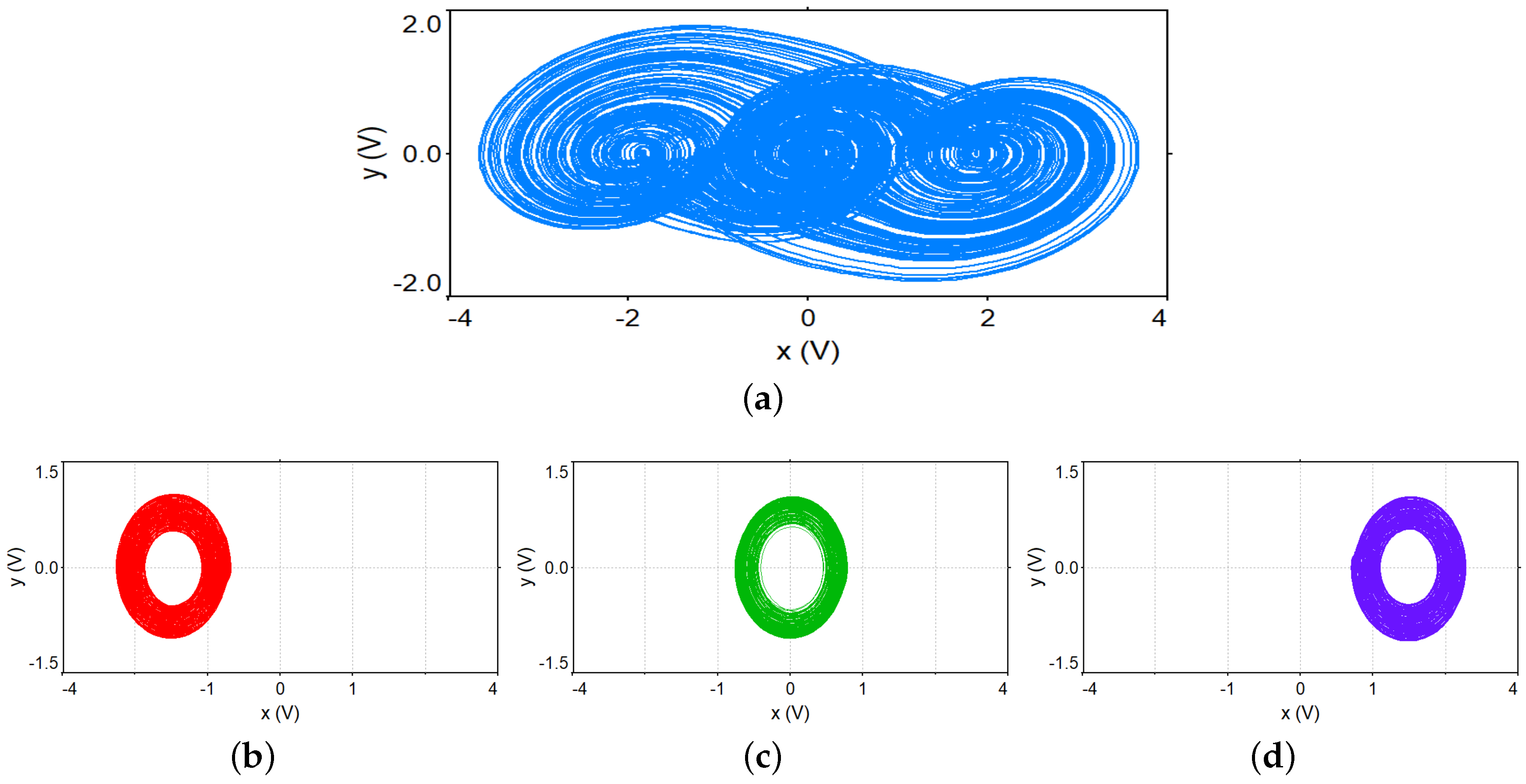
2.2. Multiscroll Chaotic System
- Fixed point behavior, MLE = 0.
- Multistable single scroll, , MLE = 0.0144.
- Monostable multi-scroll, , MLE = 0.4347.
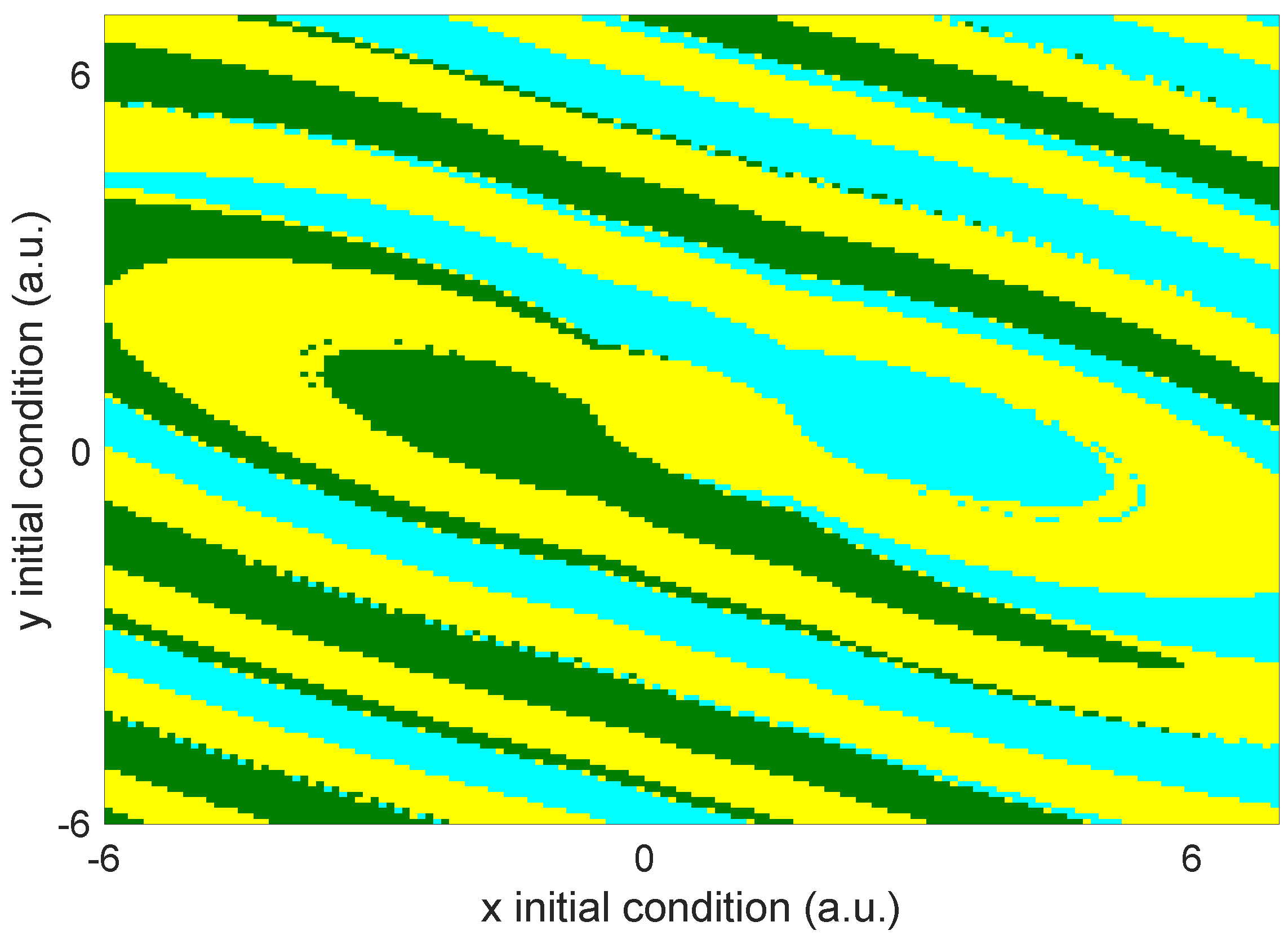
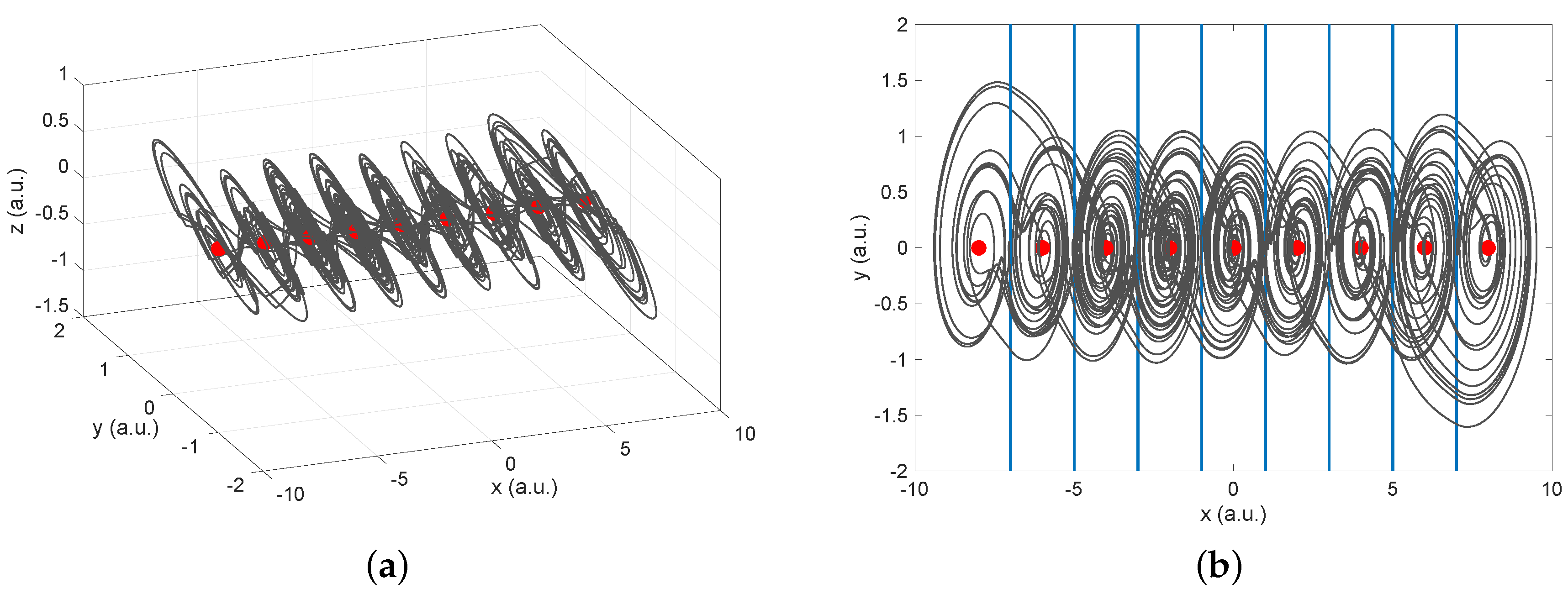
3. Results
Electronic Validation
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Maurer, J.; Libchaber, A. Effect of the Prandtl number on the onset of turbulence in liquid 4He. J. Phys. Lett. 1980, 41, 515–518. [Google Scholar] [CrossRef]
- Huerta-Cuellar, G.; Pisarchik, A.N.; Barmenkov, Y.O. Experimental characterization of hopping dynamics in a multistable fiber laser. Phys. Rev. E 2008, 78, 035202. [Google Scholar] [CrossRef] [PubMed]
- Sevilla-Escoboza, R.; Huerta-Cuéllar, G.; Jaimes-Reátegui, R.; García-López, J.; Medel-Ruiz, C.; Castañeda, C.E.; López-Mancilla, D.; Pisarchik, A.N. Error-feedback control of multistability. J. Frankl. Inst. 2017, 354, 7346–7358. [Google Scholar] [CrossRef]
- Thompson, J.M.T.; Stewart, H.B. Nonlinear Dynamics and Chaos; John Wiley & Sons: Chichester, West Sussex, UK, 2002. [Google Scholar]
- Pisarchik, A.N.; Feudel, U. Control of multistability. Phys. Rep. 2014, 540, 167–218. [Google Scholar] [CrossRef]
- Escalante-González, R.; Gilardi-Velázquez, H.; Campos-Cantón, E. A class of Chua-like systems with only two saddle-foci of different type. IFAC-PapersOnLine 2018, 51, 156–161. [Google Scholar] [CrossRef]
- Suykens, J.A.K.; Huang, A. A family of n-scroll attractors from a generalized Chua’s circuit. Arch. Elektron. Ubertragungstechnik 1997, 51, 131–137. [Google Scholar]
- Campos-Cantón, E.; Campos-Cantón, I.; González Salas, J.; Cruz Ordaz, F. A parameterized family of single-double-triple-scroll chaotic oscillations. Rev. Mex. De Física 2008, 54, 411–415. [Google Scholar]
- Li, G.; Chen, X. Constructing piecewise linear chaotic system based on the heteroclinic Shil’nikov theorem. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 194–203. [Google Scholar] [CrossRef]
- Wu, T.; Wang, L.; Yang, X.S. Chaos generator design with piecewise affine systems. Nonlinear Dyn. 2016, 84, 817–832. [Google Scholar] [CrossRef]
- Wang, L.; Yang, X.S. Heteroclinic cycles in a class of 3-dimensional piecewise affine systems. Nonlinear Anal. Hybrid Syst. 2017, 23, 44–60. [Google Scholar] [CrossRef]
- Goebel, R.; Sanfelice, R.G.; Teel, A.R. Hybrid dynamical systems. IEEE Control Syst. Mag. 2009, 29, 28–93. [Google Scholar] [CrossRef]
- Ontañón-García, L.; Campos-Cantón, E.; Femat, R. Analog electronic implementation of a class of hybrid dissipative dynamical system. Int. J. Bifurc. Chaos 2016, 26, 1650018. [Google Scholar] [CrossRef]
- Echenausía-Monroy, J.L.; Huerta-Cuéllar, G. A novel approach to generate attractors with a high number of scrolls. Nonlinear Anal. Hybrid Syst. 2020, 35, 100822. [Google Scholar] [CrossRef]
- Petráš, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar] [CrossRef]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Measurement of supercapacitor fractional-order model parameters from voltage-excited step response. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 367–376. [Google Scholar] [CrossRef]
- Elwakil, A.S. Fractional-order circuits and systems: An emerging interdisciplinary research area. IEEE Circuits Syst. Mag. 2010, 10, 40–50. [Google Scholar] [CrossRef]
- Gilardi-Velázquez, H.E.; Ontañón-García, L.J.; Hurtado-Rodriguez, D.G.; Campos-Cantón, E. Multistability in piecewise linear systems versus eigenspectra variation and round function. Int. J. Bifurc. Chaos 2017, 27, 1730031. [Google Scholar] [CrossRef]
- Ontañón-García, L.; Campos-Cantón, E. Widening of the basins of attraction of a multistable switching dynamical system with the location of symmetric equilibria. Nonlinear Anal. Hybrid Syst. 2017, 26, 38–47. [Google Scholar] [CrossRef]
- Pham, V.T.; Kingni, S.T.; Volos, C.; Jafari, S.; Kapitaniak, T. A simple three-dimensional fractional-order chaotic system without equilibrium: Dynamics, circuitry implementation, chaos control and synchronization. AEU-Int. J. Electron. Commun. 2017, 78, 220–227. [Google Scholar] [CrossRef]
- Xu, Y.; Sun, K.; He, S.; Zhang, L. Dynamics of a fractional-order simplified unified system based on the Adomian decomposition method. Eur. Phys. J. Plus 2016, 131, 186. [Google Scholar] [CrossRef]
- Munoz-Pacheco, J.M.; Zambrano-Serrano, E.; Volos, C.; Jafari, S.; Kengne, J.; Rajagopal, K. A new fractional-order chaotic system with different families of hidden and self-excited attractors. Entropy 2018, 20, 564. [Google Scholar] [CrossRef]
- Zhou, C.; Li, Z.; Xie, F. Coexisting attractors, crisis route to chaos in a novel 4D fractional-order system and variable-order circuit implementation. Eur. Phys. J. Plus 2019, 134, 73. [Google Scholar] [CrossRef]
- Bhalekar, S.; Patil, M. Singular points in the solution trajectories of fractional order dynamical systems. Chaos: Interdiscip. J. Nonlinear Sci. 2018, 28, 113123. [Google Scholar] [CrossRef] [PubMed]
- Anzo-Hernández, A.; Gilardi-Velázquez, H.E.; Campos-Cantón, E. On multistability behavior of unstable dissipative systems. Chaos: Interdiscip. J. Nonlinear Sci. 2018, 28, 033613. [Google Scholar] [CrossRef] [PubMed]
- Diethelm, K. The Analysis of Fractional Differential Equations: An Application-Oriented Exposition Using Differential Operators of Caputo Type; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: San Diego, CA, USA, 1998; Volume 198. [Google Scholar]
- Zambrano-Serrano, E.; Campos-Cantón, E.; Muñoz-Pacheco, J.M. Strange attractors generated by a fractional order switching system and its topological horseshoe. Nonlinear Dyn. 2016, 83, 1629–1641. [Google Scholar] [CrossRef]
- Sprott, J.C. Some simple chaotic flows. Phys. Rev. E 1994, 50, R647. [Google Scholar] [CrossRef] [PubMed]
- Chiu, R.; López-Mancilla, D.; Castañeda, C.E.; Orozco-López, O.; Villafaña-Rauda, E.; Sevilla-Escoboza, R. Design and implementation of a jerk circuit using a hybrid analog–digital system. Chaos Solitons Fractals 2019, 119, 255–262. [Google Scholar] [CrossRef]
- Glover, K.; Willems, J. Parametrizations of linear dynamical systems: Canonical forms and identifiability. IEEE Trans. Autom. Control 1974, 19, 640–646. [Google Scholar] [CrossRef]
- Denham, M. Canonical forms for the identification of multivariable linear systems. IEEE Trans. Autom. Control 1974, 19, 646–656. [Google Scholar] [CrossRef]
- Echenausía-Monroy, J.L.; García-López, J.H.; Jaimes-Reátegui, R.; López-Mancilla, D.; Huerta-Cuellar, G. Family of bistable attractors contained in an unstable dissipative switching system associated to a SNLF. Complexity 2018, 2018. [Google Scholar] [CrossRef]
- Campos-Cantón, E.; Barajas-Ramírez, J.G.; Solis-Perales, G.; Femat, R. Multiscroll attractors by switching systems. Chaos: Interdiscip. J. Nonlinear Sci. 2010, 20, 013116. [Google Scholar] [CrossRef]
- Campos-Cantón, E.; Femat, R.; Chen, G. Attractors generated from switching unstable dissipative systems. Chaos: Interdiscip. J. Nonlinear Sci. 2012, 22, 033121. [Google Scholar] [CrossRef] [PubMed]
- Campos-Cantón, E. Chaotic attractors based on unstable dissipative systems via third-order differential equation. Int. J. Mod. Phys. C 2016, 27, 1650008. [Google Scholar] [CrossRef]
- Diethelm, K.; Ford, N.J.; Freed, A.D. A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn. 2002, 29, 3–22. [Google Scholar] [CrossRef]
- Gilardi-Velázquez, H.; Campos-Cantón, E. Nonclassical point of view of the Brownian motion generation via fractional deterministic model. Int. J. Mod. Phys. C 2018, 29, 1850020. [Google Scholar] [CrossRef]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Phys. D: Nonlinear Phenom. 1985, 16, 285–317. [Google Scholar] [CrossRef]
- Hammouch, Z.; Mekkaoui, T. Circuit design and simulation for the fractional-order chaotic behavior in a new dynamical system. Complex Intell. Syst. 2018, 4, 251–260. [Google Scholar] [CrossRef]
- Chen, D.; Liu, C.; Wu, C.; Liu, Y.; Ma, X.; You, Y. A new fractional-order chaotic system and its synchronization with circuit simulation. Circuits Syst. Signal Process. 2012, 31, 1599–1613. [Google Scholar] [CrossRef]
- Lu, J.; Yu, S.; Leung, H.; Chen, G. Experimental verification of multidirectional multiscroll chaotic attractors. IEEE Trans. Circuits Syst. I Regul. Pap. 2006, 53, 149–165. [Google Scholar] [CrossRef]
- Zambrano-Serrano, E.; Muñoz-Pacheco, J.M.; Campos-Cantón, E. Circuit synthesis of an incommensurate fractional order multi-scroll PWL chaotic system. In Proceedings of the 2017 6th International Conference on Modern Circuits and Systems Technologies (MOCAST), Thessaloniki, Greece, 4–6 May 2017; pp. 1–4. [Google Scholar]


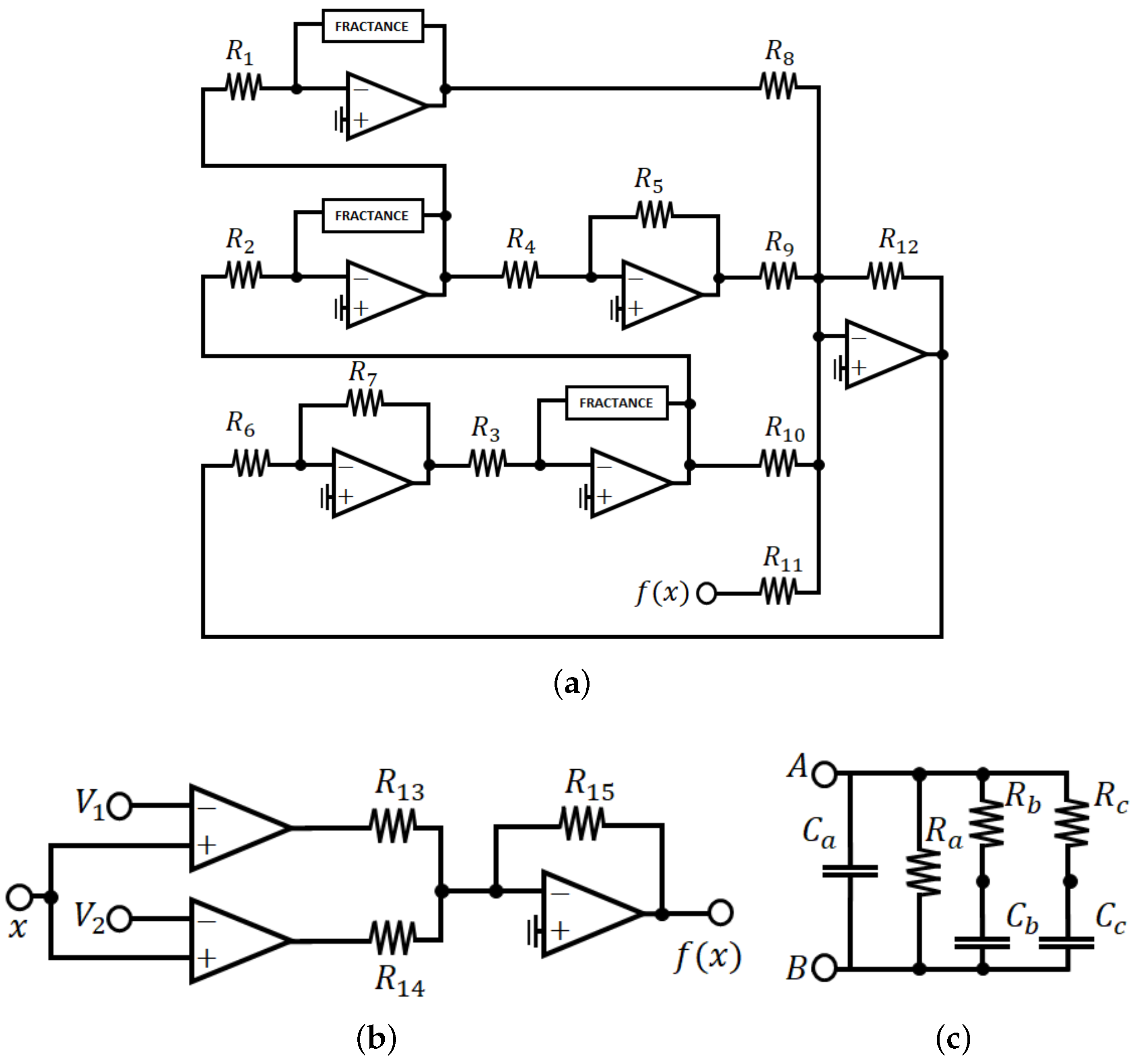

| Component | Value | Component | Value |
| k | 1 k | ||
| 10 k | k | ||
| 107 k | k | ||
| V | V | ||
| Voltage | Value | Component | Value |
| M | F | ||
| M | F | ||
| M | F |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Echenausía-Monroy, J.L.; Huerta-Cuellar, G.; Jaimes-Reátegui, R.; García-López, J.H.; Aboites, V.; Cassal-Quiroga, B.B.; Gilardi-Velázquez, H.E. Multistability Emergence through Fractional-Order-Derivatives in a PWL Multi-Scroll System. Electronics 2020, 9, 880. https://doi.org/10.3390/electronics9060880
Echenausía-Monroy JL, Huerta-Cuellar G, Jaimes-Reátegui R, García-López JH, Aboites V, Cassal-Quiroga BB, Gilardi-Velázquez HE. Multistability Emergence through Fractional-Order-Derivatives in a PWL Multi-Scroll System. Electronics. 2020; 9(6):880. https://doi.org/10.3390/electronics9060880
Chicago/Turabian StyleEchenausía-Monroy, José Luis, Guillermo Huerta-Cuellar, Rider Jaimes-Reátegui, Juan Hugo García-López, Vicente Aboites, Bahia Betzavet Cassal-Quiroga, and Héctor Eduardo Gilardi-Velázquez. 2020. "Multistability Emergence through Fractional-Order-Derivatives in a PWL Multi-Scroll System" Electronics 9, no. 6: 880. https://doi.org/10.3390/electronics9060880
APA StyleEchenausía-Monroy, J. L., Huerta-Cuellar, G., Jaimes-Reátegui, R., García-López, J. H., Aboites, V., Cassal-Quiroga, B. B., & Gilardi-Velázquez, H. E. (2020). Multistability Emergence through Fractional-Order-Derivatives in a PWL Multi-Scroll System. Electronics, 9(6), 880. https://doi.org/10.3390/electronics9060880











