Predictive Mobility Model for β-Ga2O3 at Cryogenic Temperature
Abstract
1. Introduction
2. Methodology
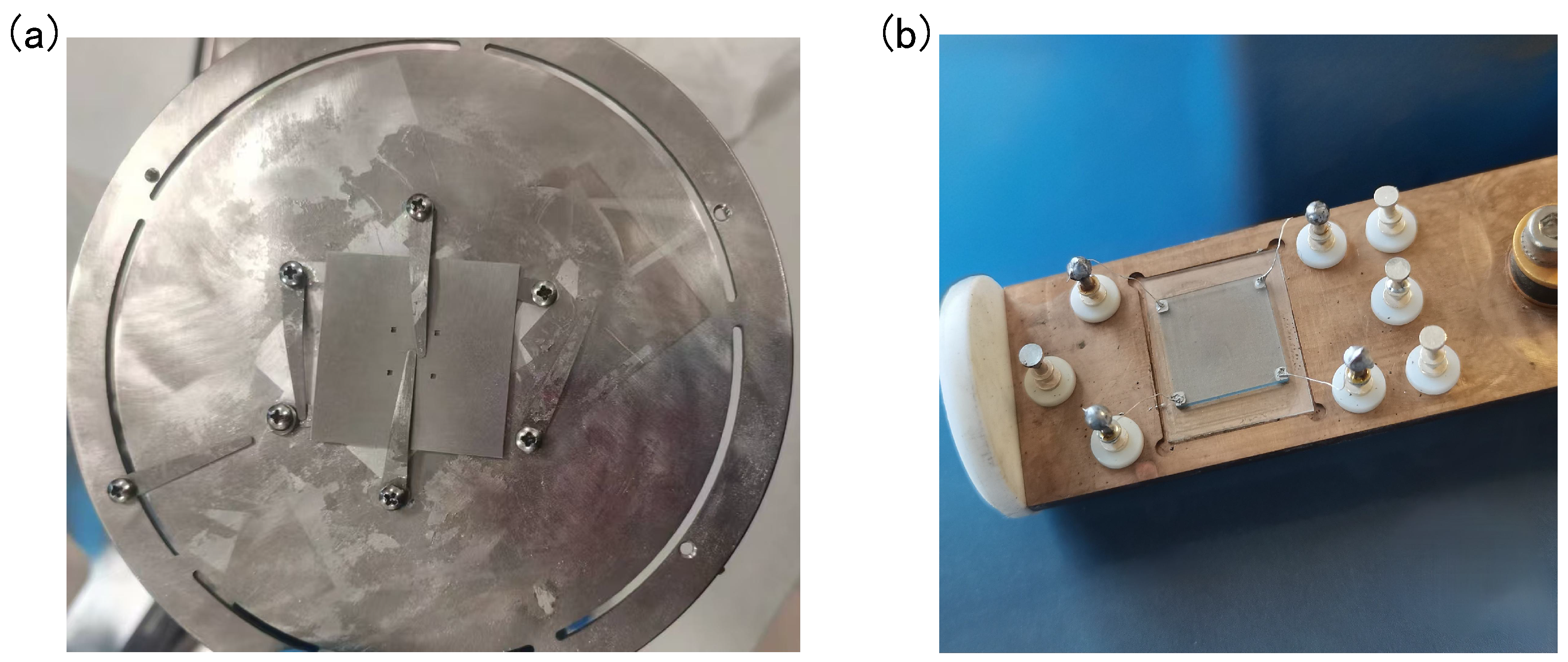
2.1. Experimental Details
2.2. Theoretical Model
3. Predictive Model
3.1. Effects of Doping Concentration on Mobility
3.2. Unified Mobility Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, H.; Yuan, L.; Tang, X.; Hu, J.; Sun, J.; Zhang, Y.; Zhang, Y.; Jia, R. Progress of ultra-wide bandgap Ga2O3 semiconductor materials in power MOSFETs. IEEE Trans. Power Electron. 2020, 35, 5157–5179. [Google Scholar] [CrossRef]
- Lei, D.; Han, K.; Wu, Y.; Liu, Z.; Gong, X. High performance Ga2O3 metal-oxide-semiconductor field-effect transistors on an AlN/Si substrate. IEEE J. Electron Devices Soc. 2019, 7, 596–600. [Google Scholar] [CrossRef]
- Antonov, V.; Harmon, B.; Yaresko, A. Electronic Structure and Magneto-Optical Properties of Solids, 1st ed.; Kluwer Academic Publishers: New York, NY, USA, 2006; pp. 268–487. [Google Scholar]
- Wang, X.; Faizan, M.; Na, G.; He, X.; Fu, Y.H.; Zhang, L. Discovery of new polymorphs of Ga2O3 with particle swarm optimization-based structure searches. Adv. Electron. Mater. 2020, 6, 2000119. [Google Scholar] [CrossRef]
- Snow, J.M.; Nirmal, D.; Samuel, G.; Khausik, S.; Du John, H.V. Investigation of Gd2O3/α-Ga2O3/Y2O3 Based MOS-HEMT for Drain Current Improvement. In Proceedings of the 2022 6th International Conference on Devices, Circuits and Systems (ICDCS), Coimbatore, India, 21–22 April 2022; pp. 489–493. [Google Scholar]
- Li, Y.; Yang, C.; Wu, L.; Zhang, R. Electrical and optical properties of Si-doped Ga2O3. Mod. Phys. Lett. B 2017, 31, 1750172. [Google Scholar] [CrossRef]
- Zhang, H.; Tang, H.; He, N.; Zhu, Z.; Chen, J.; Liu, B.; Xu, J. Growth and physical characterization of high resistivity Fe: β-Ga2O3 crystals. Chinese Phys. B 2020, 29, 087201. [Google Scholar] [CrossRef]
- Zhang, C.; Wu, X.; Xing, Y.; Zhou, L.; Zhou, H.; Li, S.; Xu, N. A first-principles study of hydrostatic strain engineering on the electronic properties of β-Ga2O3. Phys. B Condens. Matter. 2023, 660, 414851. [Google Scholar] [CrossRef]
- Cheng, T.; Sun, J.; Lin, N.; Jia, Z.; Mu, W.; Tao, X.; Zhao, X. Electronic structure and optical property of metal-doped Ga2O3: A first principles study. RSC Adv. 2016, 6, 78322–78334. [Google Scholar] [CrossRef]
- Huang, X.; Liao, F.; Li, L.; Liang, X.; Liu, Q.; Zhang, C.; Hu, X. 3.4 kV breakdown voltage Ga2O3 trench Schottky diode with optimized trench corner radius. ECS J. Solid State Sci. Technol. 2020, 9, 045012. [Google Scholar] [CrossRef]
- Jacoboni, C.; Canali, C.; Ottaviani, G.; Quaranta, A.A. A review of some charge transport properties of silicon. Solid-State Electron. 1977, 20, 77–89. [Google Scholar] [CrossRef]
- Arora, N.D.; Hauser, J.R.; Roulston, D.J. Electron and hole mobilities in silicon as a function of concentration and temperature. IEEE Trans. Electron. Dev. 1982, 29, 292–295. [Google Scholar] [CrossRef]
- Masetti, G.; Severi, M.; Solmi, S. Modeling of carrier mobility against carrier concentration in arsenic-, phosphorus-, and boron-doped silicon. IEEE Trans. Electron. Dev. 1983, 30, 764–769. [Google Scholar] [CrossRef]
- Suzuki, N.; Ohira, S.; Tanaka, M.; Sugawara, T.; Nakajima, K.; Shishido, T. Fabrication and characterization of transparent conductive Sn-doped β-Ga2O3 single crystal. Phys. Stat. Sol. 2007, 4, 2310–2313. [Google Scholar] [CrossRef]
- Hoshikawa, K.; Kobayashi, T.; Ohba, E.; Kobayashi, T. 50 mm diameter Sn-doped (001) β-Ga2O3 crystal growth using the vertical Bridgeman technique in ambient air. J. Cryst. Growth 2020, 546, 125778. [Google Scholar] [CrossRef]
- Ohira, S.; Suzuki, N.; Arai, N.; Tanaka, M.; Sugawara, T.; Nakajima, K.; Shishido, T. Characterization of transparent and conducting Sn-doped β-Ga2O3 single crystal after annealing. Thin Solid Films 2008, 516, 5763–5767. [Google Scholar] [CrossRef]
- Oishi, T.; Harada, K.; Koga, Y.; Kasu, M. Conduction mechanism in highly doped β-Ga2O3 single crystals grown by edge-defined film-fed growth method and their Schottky barrier diodes. Jpn. J. Appl. Phys. 2016, 55, 030305. [Google Scholar] [CrossRef]
- Galazka, Z.; Irmscher, K.; Uecker, R.; Bertram, R.; Pietsch, M.; Kwasniewski, A.; Naumann, M.; Schulz, T.; Schewski, R.; Klimm, D.; et al. On the bulk β-Ga2O3 single crystals grown by the Czochralski method. J. Cryst. Growth 2014, 404, 184–191. [Google Scholar] [CrossRef]
- Yoshioka, S.; Hayashi, H.; Kuwabara, A.; Oba, F.; Matsunaga, K.; Tanaka, I. Structures and energetics of Ga2O3 polymorphs. J. Phys. Condens. Matter. 2007, 19, 346211. [Google Scholar] [CrossRef]
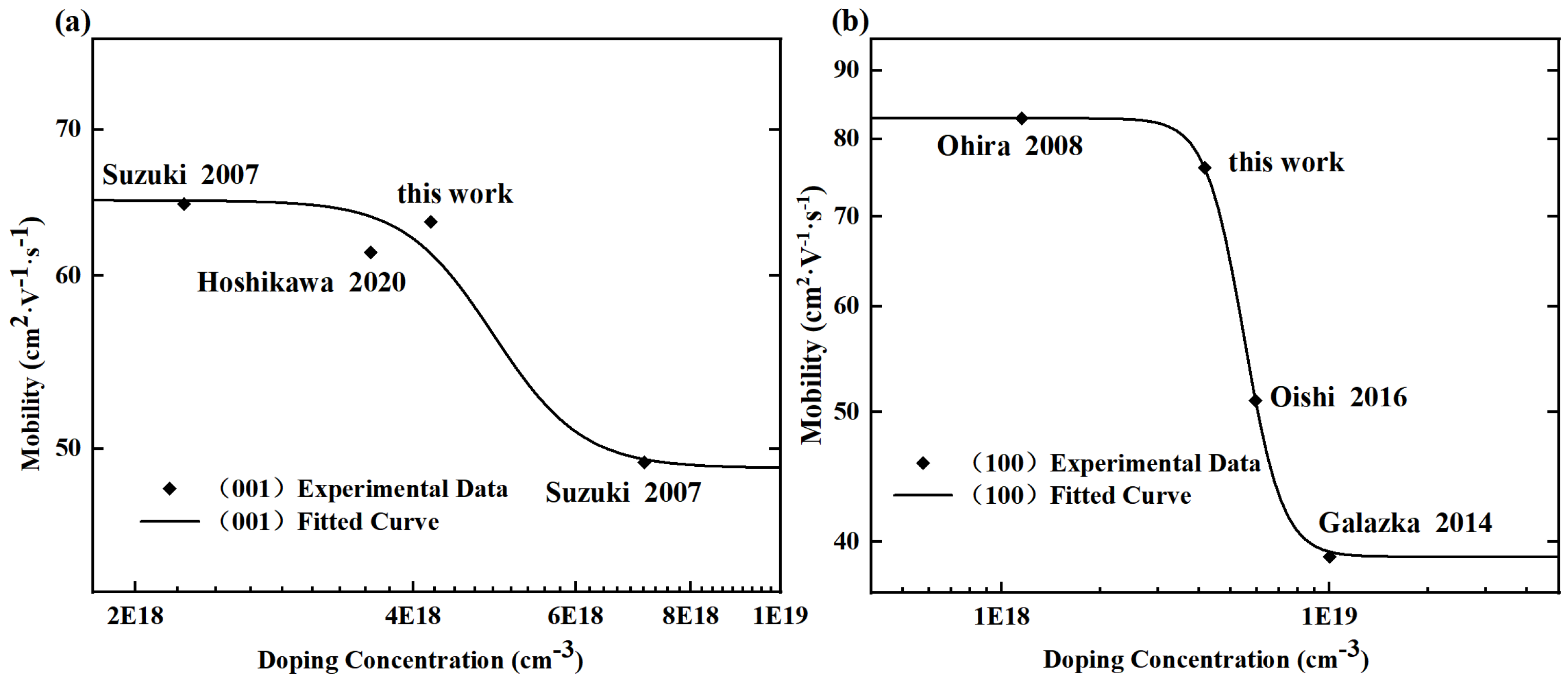

| Crystal Planes | ||||||
|---|---|---|---|---|---|---|
| [cm Vs] | [cm Vs] | [cm] | ||||
| (100) | 64.7 | 46.5 | 5.48 × 1018 | 6.14 | 0.49 | 0.29 |
| (001) | 82.9 | 39.1 | 5.22 × 1018 | 7.50 | 0.86 | 0.40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, C.; Chen, S.; Wang, D.; Liu, Y.; Wang, G. Predictive Mobility Model for β-Ga2O3 at Cryogenic Temperature. Electronics 2025, 14, 2120. https://doi.org/10.3390/electronics14112120
Zhou C, Chen S, Wang D, Liu Y, Wang G. Predictive Mobility Model for β-Ga2O3 at Cryogenic Temperature. Electronics. 2025; 14(11):2120. https://doi.org/10.3390/electronics14112120
Chicago/Turabian StyleZhou, Chunyu, Shuai Chen, Danying Wang, Yong Liu, and Guanyu Wang. 2025. "Predictive Mobility Model for β-Ga2O3 at Cryogenic Temperature" Electronics 14, no. 11: 2120. https://doi.org/10.3390/electronics14112120
APA StyleZhou, C., Chen, S., Wang, D., Liu, Y., & Wang, G. (2025). Predictive Mobility Model for β-Ga2O3 at Cryogenic Temperature. Electronics, 14(11), 2120. https://doi.org/10.3390/electronics14112120






