Constructing a Microstrip Phase Shifter with Low Phase Error Using a Y-Resonator
Abstract
1. Introduction
2. Proposed Structure
3. Analysis and Design
Design Steps
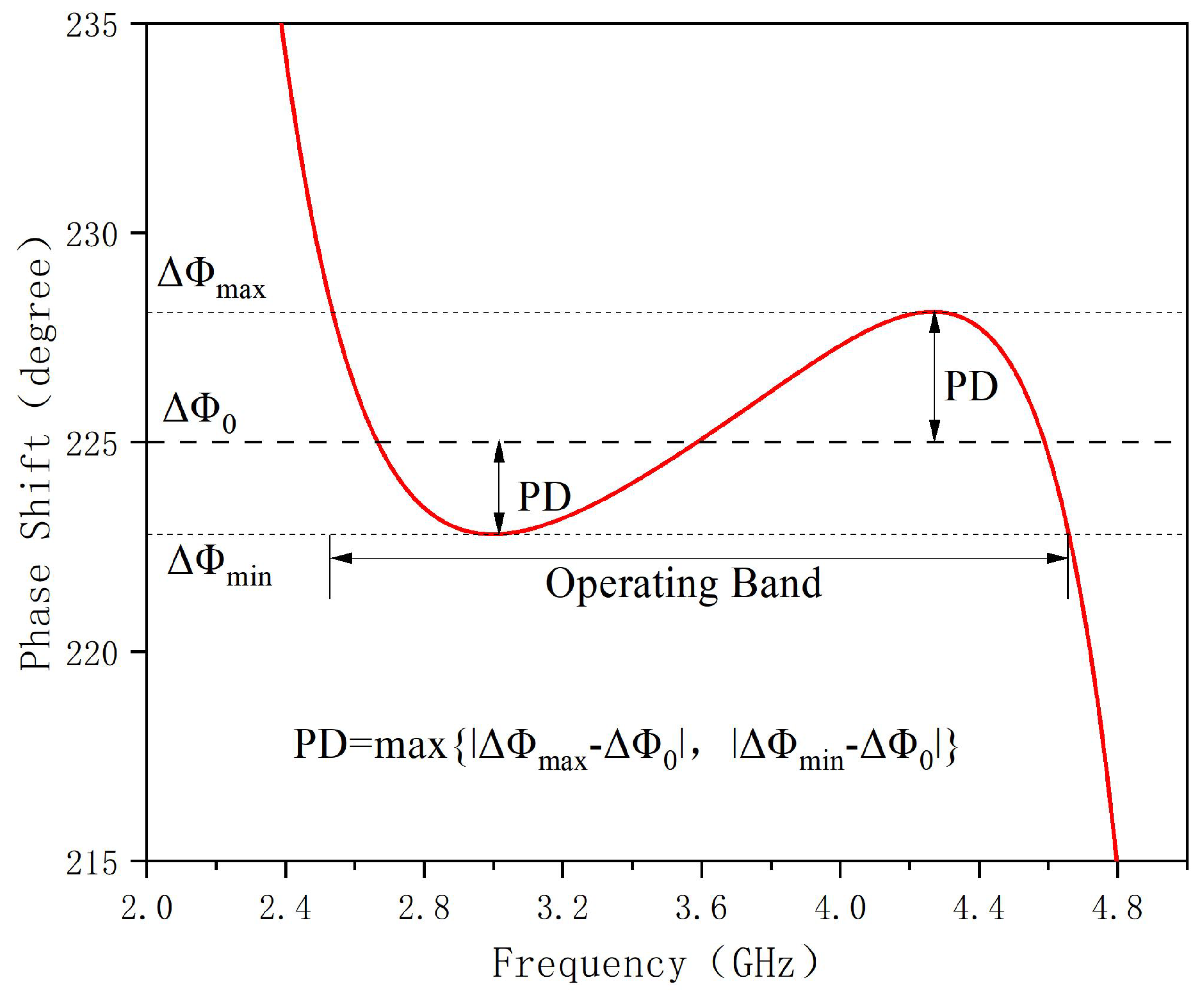
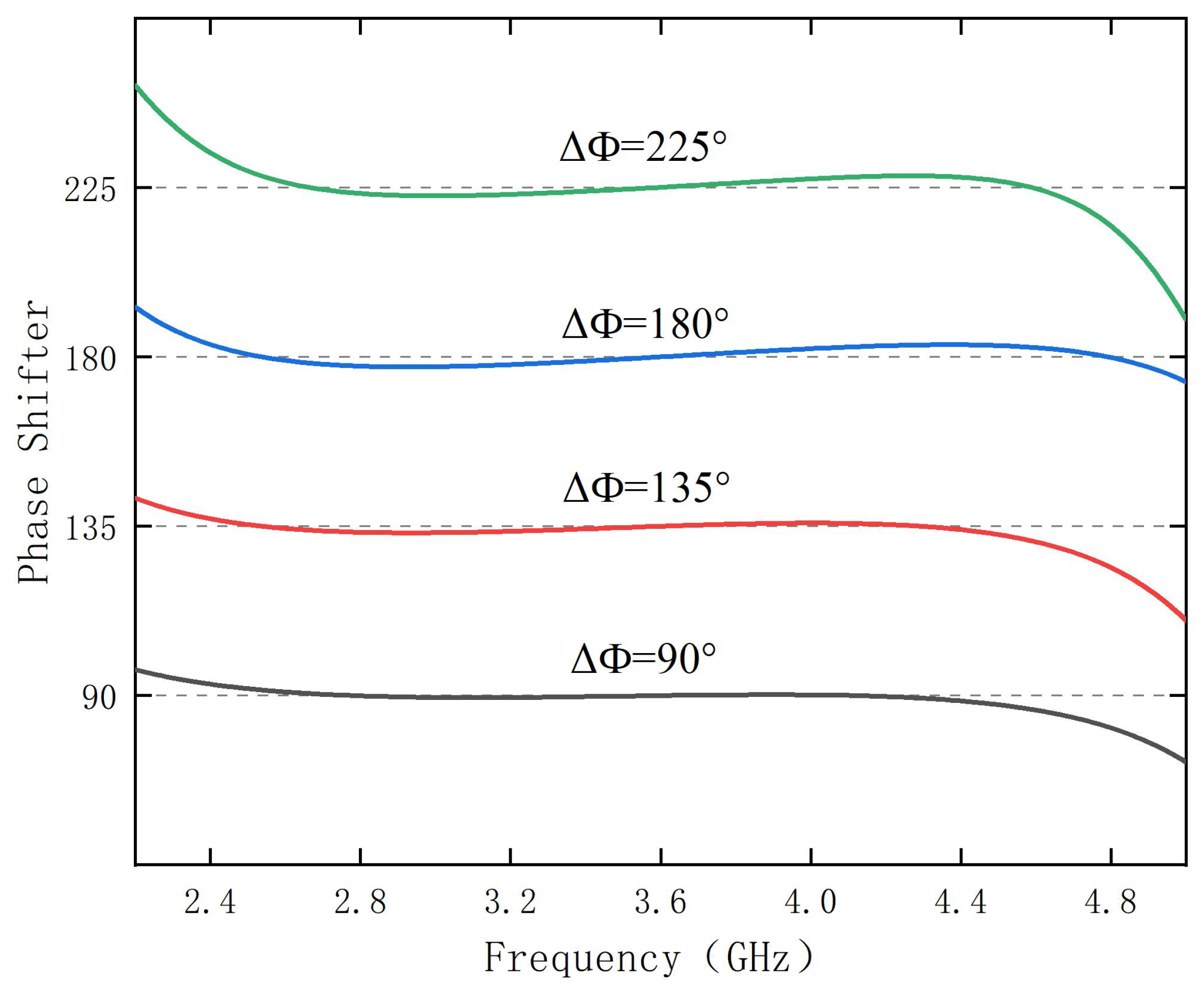
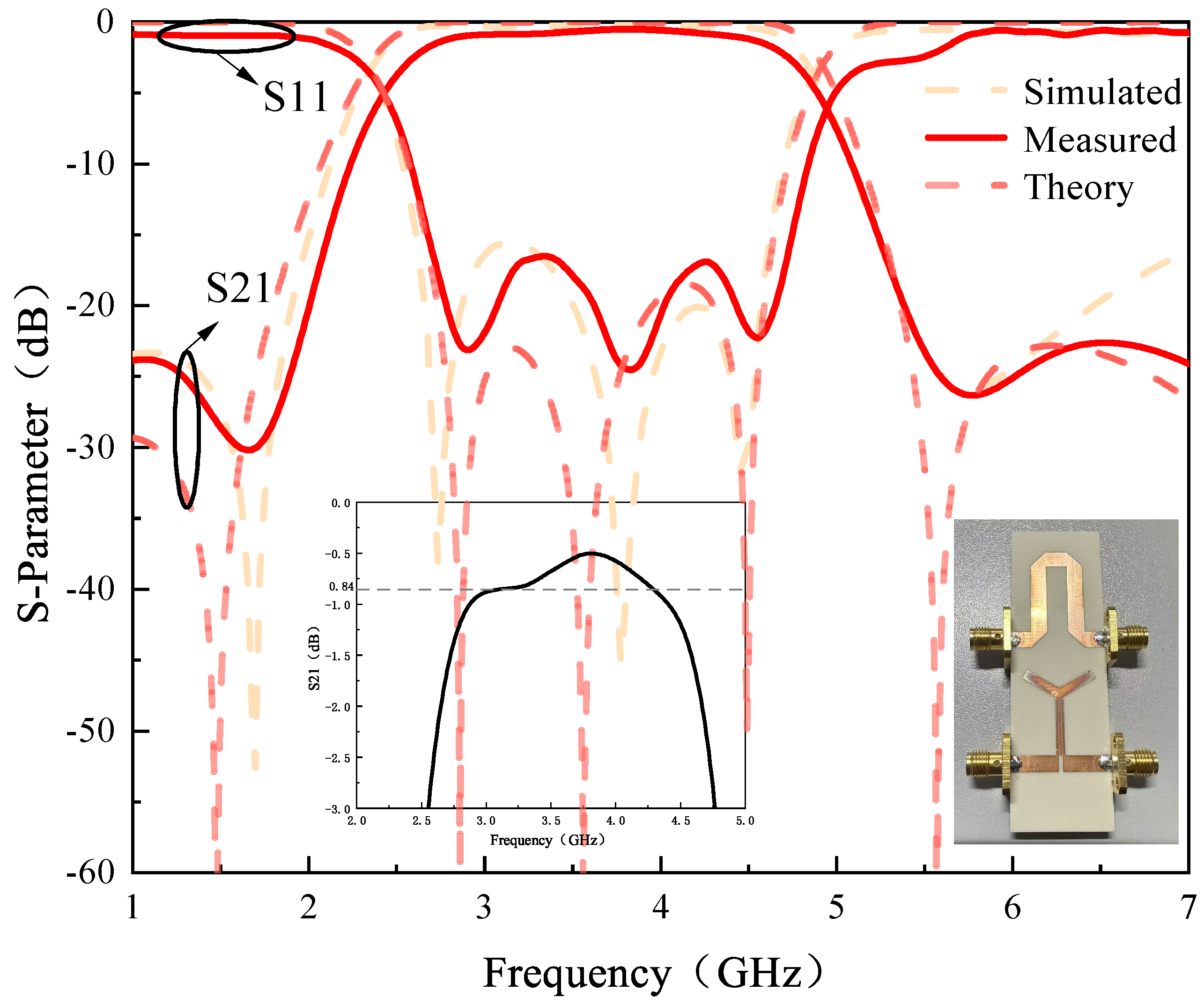
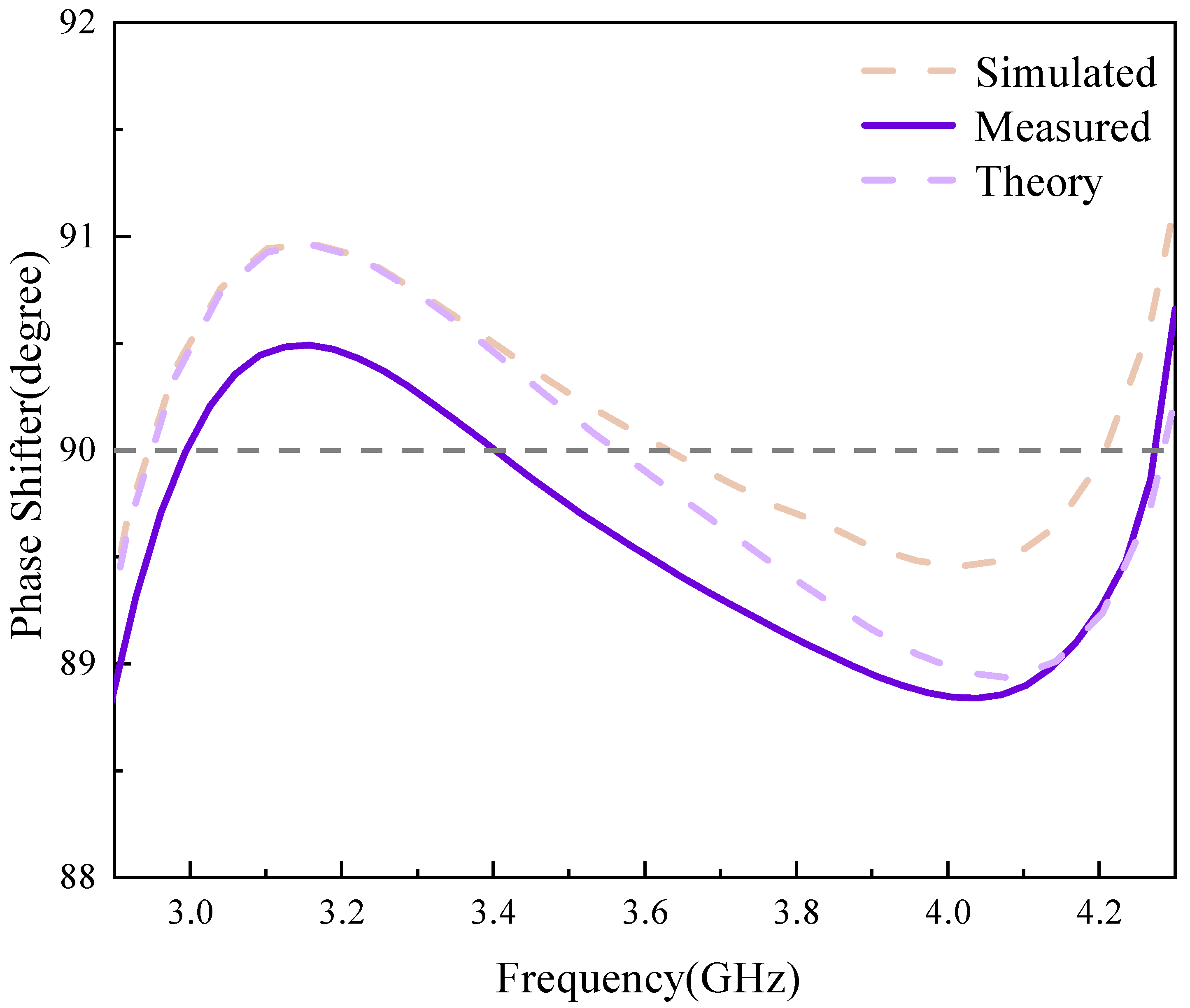
4. Result and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tolin, E.; Vipiana, F.; Litschke, O.; Bruni, S. Phase shifters design for scan range extension of Rotman lens beamforming based antenna arrays. In Proceedings of the 2018 IEEE International Symposium on Antennas and Propagation & USNC/URSI National Radio Science Meeting, Boston, MA, USA, 8–13 July 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 2129–2130. [Google Scholar]
- Kobayashi, A.; Imanishi, R.; Nosaka, H. Fast-Phase-Switching Current-Mode Phase Shifter for Beyond 5G Phased Array Antenna. In Proceedings of the 2023 53rd European Microwave Conference (EuMC), Berlin, Germany, 19–21 September 2023; IEEE: Piscataway, NJ, USA, 2023; pp. 26–29. [Google Scholar]
- Cao, J.Z.; Chen, F.C. Design of continuously steerable Nolen matrix-based beamforming networks using tunable phase shifters. In Proceedings of the 2022 International Conference on Microwave and Millimeter Wave Technology (ICMMT), Harbin, China, 12–15 August 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 1–3. [Google Scholar]
- Qiu, L.L.; Zhu, L. Synthesis design of filtering differential phase shifters of independently suppressed harmonics. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 2760–2764. [Google Scholar] [CrossRef]
- Xu, W.; Li, T.; Chen, X.; Chen, H.; Feng, L. Highly selective phase shifter using stub-loaded coupled-line resonators. Microw. Opt. Technol. Lett. 2023, 65, 3164–3170. [Google Scholar] [CrossRef]
- Tian, Y.; Gu, J.; Xu, H.; Liu, W.; Duan, Z.; Gao, H.; Yan, N. A 26-32GHz 6-bit bidirectional passive phase shifter with 14dBm IP1dB and 2.6° RMS phase error for phased array system in 40nm CMOS. In Proceedings of the 2023 IEEE/MTT-S International Microwave Symposium-IMS 2023, San Diego, CA, USA, 11–16 June 2023; IEEE: Piscataway, NJ, USA, 2023; pp. 195–198. [Google Scholar]
- Jang, Y.; Salman, M.; Jeong, Y.C.; Choi, K.S.; Han, S.M.; Ahn, D. Wideband phase shifter using 3 types of LC resonant circuits for phase slope alignment. In Proceedings of the 2019 PhotonIcs & Electromagnetics Research Symposium-Spring (PIERS-Spring), Rome, Italy, 17–20 June 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 4034–4039. [Google Scholar]
- Lyu, Y.P.; Zhu, L.; Cheng, C.H. Design and analysis of Schiffman phase shifter under operation of its second phase period. IEEE Trans. Microw. Theory Tech. 2018, 66, 3263–3269. [Google Scholar] [CrossRef]
- Feng, L.; Chen, J.; Yu, X.; Zhu, L.; Liu, H. A novel wideband 90° filtering phase shifter using broadside-coupled MSLs. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 2742–2746. [Google Scholar] [CrossRef]
- Zheng, B.; Tang, H.; Li, H.; Zhou, L.; Dong, Y.; Haerinia, M.; Zhang, H. Ultra-Wideband Schiffman Phase Shifter Designed with Deep Neural Networks. Authorea Prepr. 2023. [Google Scholar]
- Qiu, L.L.; Zhu, L.; Lyu, Y.P. Schiffman phase shifters with wide phase shift range under operation of first and second phase periods in a coupled line. IEEE Trans. Microw. Theory Tech. 2019, 68, 1423–1430. [Google Scholar] [CrossRef]
- Zheng, S.Y.; Chan, W.S. Differential RF phase shifter with harmonic suppression. IEEE Trans. Ind. Electron. 2013, 61, 2891–2899. [Google Scholar] [CrossRef]
- Yeung, S.H.; Xue, Q.; Man, K.F. Broadband 90 Differential Phase Shifter Constructed Using a Pair of Multisection Radial Line Stubs. IEEE Trans. Microw. Theory Tech. 2012, 60, 2760–2767. [Google Scholar] [CrossRef]
- Zheng, S.Y.; Chan, W.S.; Man, K.F. Broadband phase shifter using loaded transmission line. IEEE Microw. Wirel. Components Lett. 2010, 20, 498–500. [Google Scholar] [CrossRef]
- Tang, X.; Mouthaan, K. Phase-shifter design using phase-slope alignment with grounded shunt λ/4 stubs. IEEE Trans. Microw. Theory Tech. 2010, 58, 1573–1583. [Google Scholar] [CrossRef]
- Wang, Y.; Bialkowski, M.; Abbosh, A. Double Microstrip-Slot Transitions for Broadband 90° Microstrip Phase Shifters. IEEE Microw. Mireless Components Lett. 2012, 22, 58–60. [Google Scholar] [CrossRef]
- Lyu, Y.P.; Zhu, L.; Cheng, C.H. A new design of ultrawideband single-layer 90° phase shifter in the view of group delay. IEEE Microw. Wirel. Components Lett. 2019, 29, 376–378. [Google Scholar] [CrossRef]
- Dong, Q.; Wu, Y.; Zheng, Y.; Wang, W.; Liu, Y. A compact single-layer ultra-wideband phase shifter using weakly coupled lines. IEEE Access 2019, 7, 12575–12583. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, L.; Pan, J.; Guo, Y.; Dai, X. Design of a wideband phase shifter using loaded element. In Proceedings of the 2016 Progress in Electromagnetic Research Symposium (PIERS), Shanghai, China, 8–11 August 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 2153–2155. [Google Scholar]
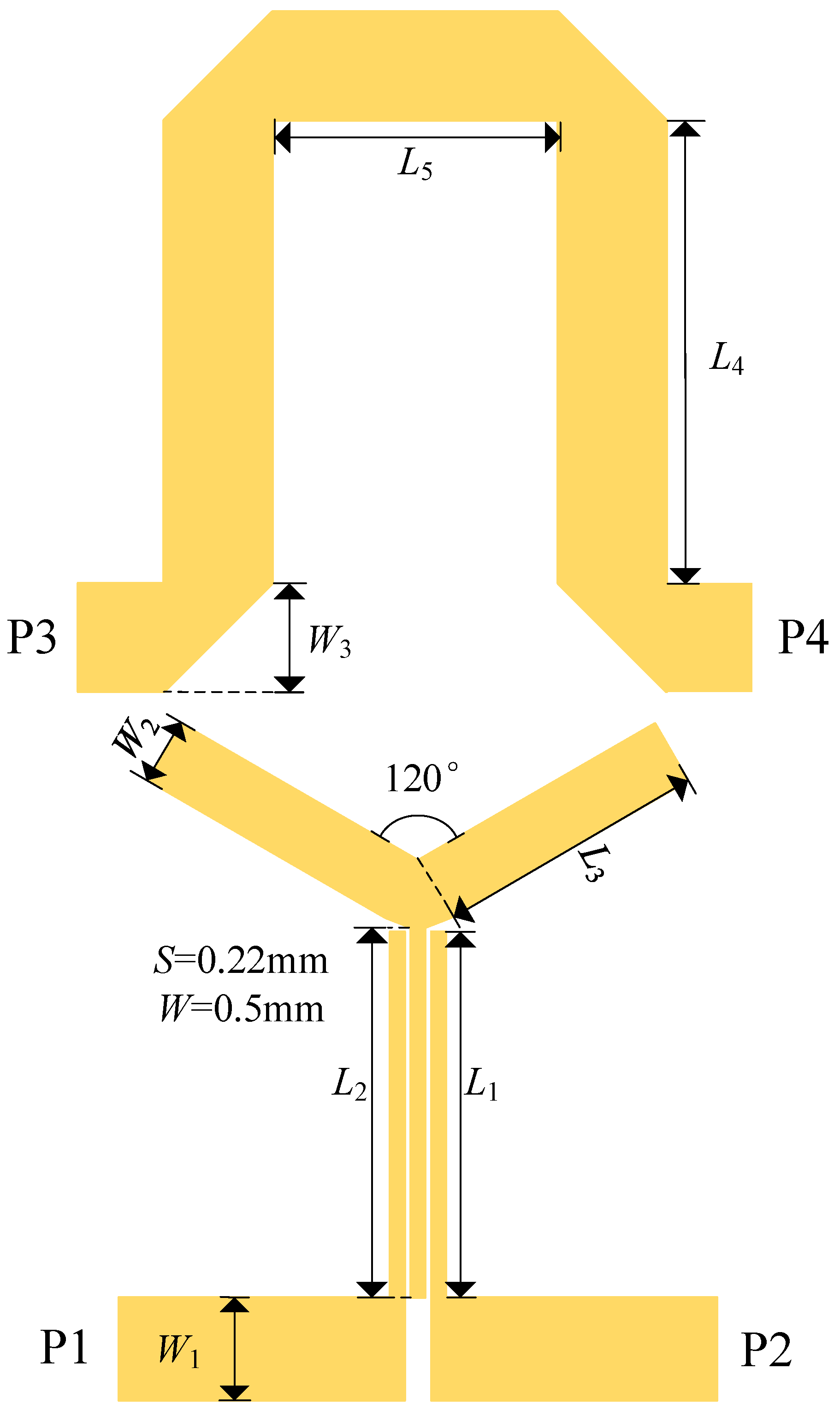
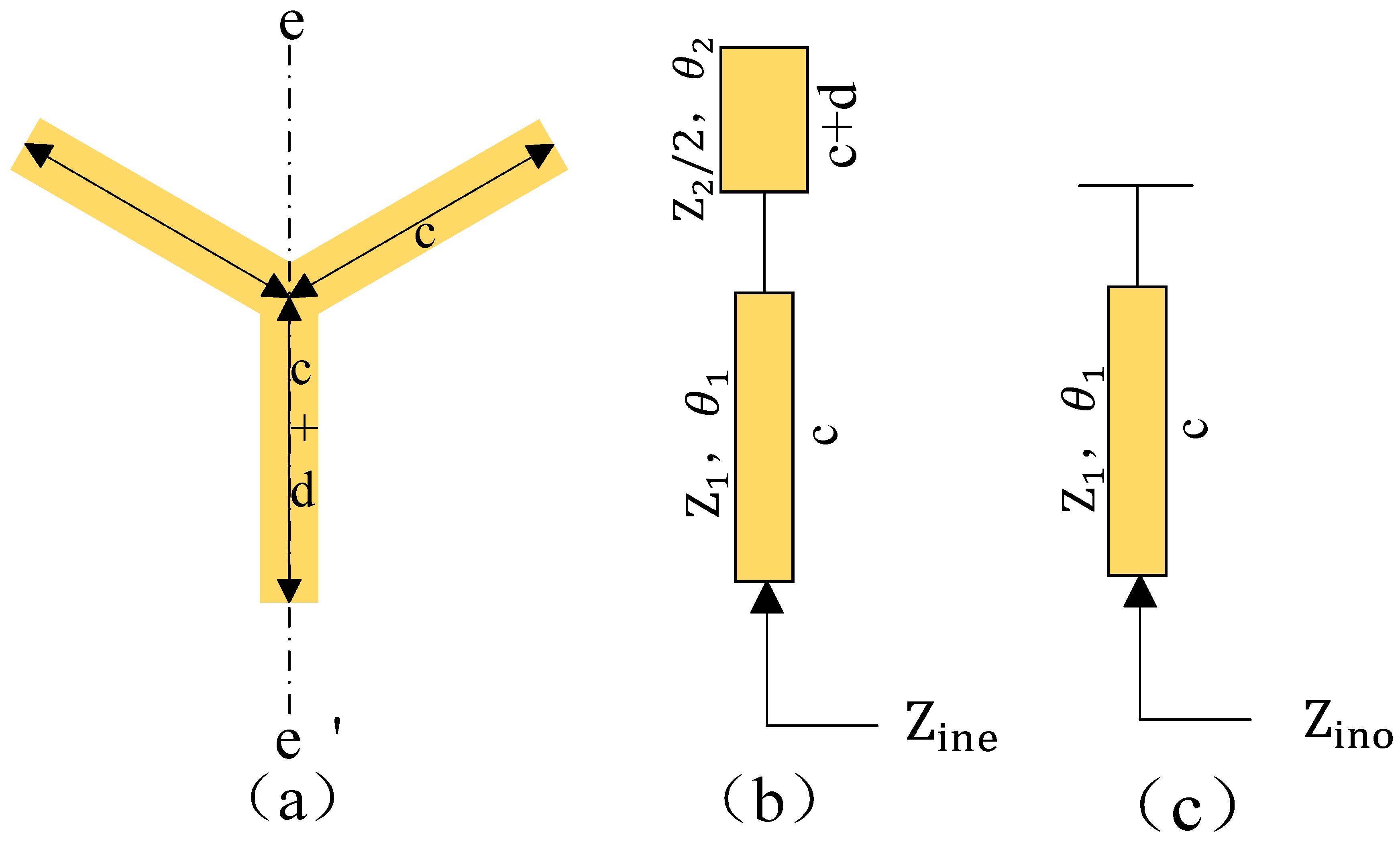
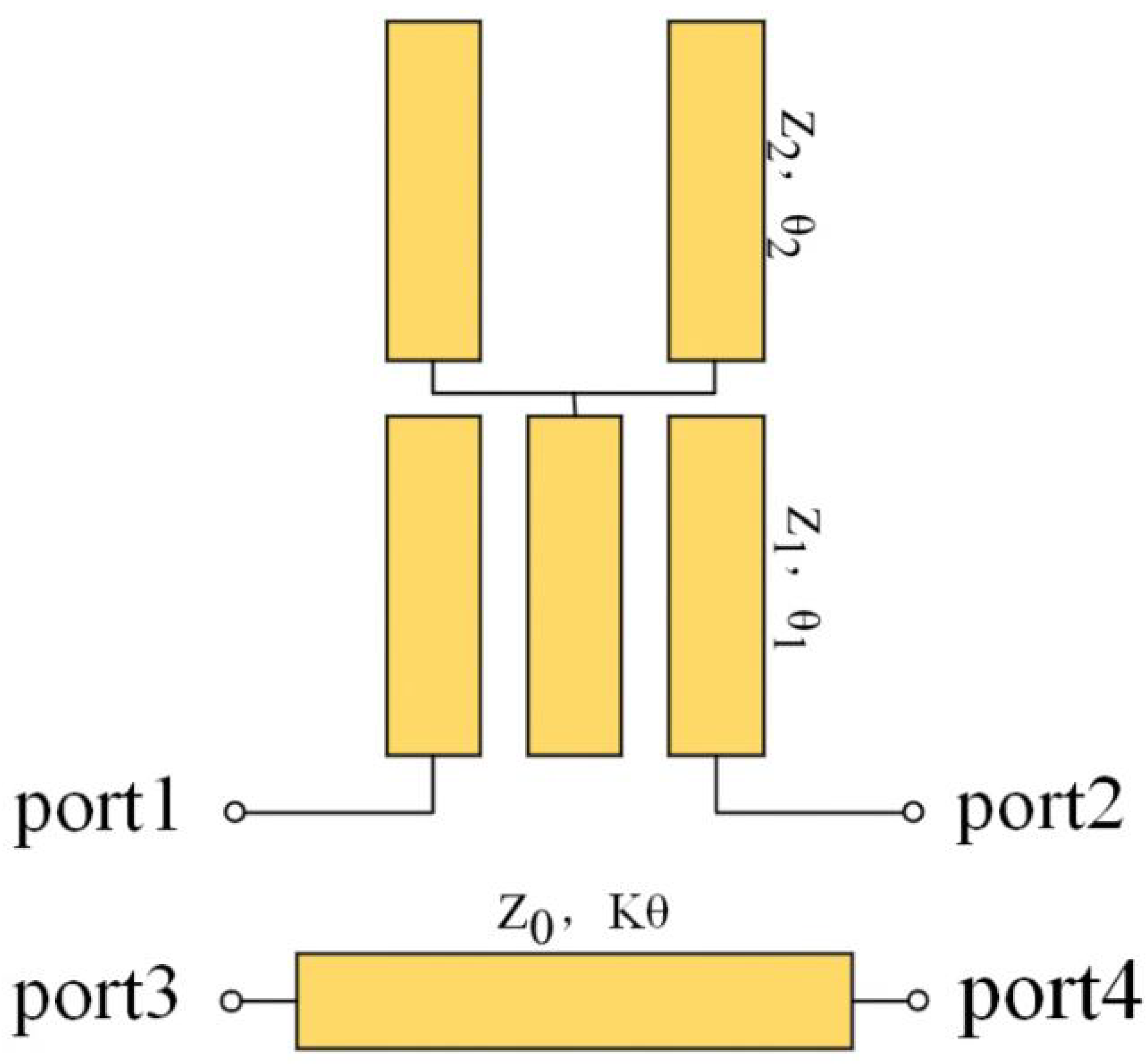


| Phase Shifter (°) | Equivalent Circuit Parameters (°\ohm) | Physical Dimensions (mm) |
|---|---|---|
| 90 | Z0 = 50; Z1 = 51.2; Z2 = 92.4; = 84; = 76.2; K = 0.482; = 226.1 | L1 = 12.8; L2 = 12.95; L3 = 9.6; L4 = 16.2; L5 = 10; W1 = 3.6; W2 = 2.3; W3 = 3.8; S = 0.22 |
| 135 | Z0 = 50; Z1 = 50.7; Z2 = 91.5; = 91.5; = 69.2; K = 0.469; = 141.4 | L1 = 15.5; L2 = 15.8; L3 = 10; L4 = 15.2; L5 = 10; W1 = 3.6; W2 = 1; W3 = 3.8; S = 0.15 |
| 180 | Z0 = 50; Z1 = 72.8; Z2 = 92.4; = 87.8; = 76.2; K = 0.403; = 176.1 | L1 = 14; L2 = 14.2; L3 = 11; L4 = 15.2; L5 = 10; W1 = 3.6; W2 = 1; W3 = 3.8; S = 0.09 |
| 225 | Z0 = 50; Z1 = 94.6; Z2 = 92.4; = 92; = 76.2; K = 0.412; = 226.1 | L1 = 14; L2 = 15.1; L3 = 11; L4 = 18.2; L5 = 10; W1 = 3.6; W2 = 2.3; W3 = 3.8; S = 0.205 |
| Reference | F0 (GHz) | PR (°) | MaxPD (°) | IL/RL (dB) | FBW (%) |
|---|---|---|---|---|---|
| 5 | 0.5 | 89.9 | ±1.5 | <1.4/>16 | 37.5 |
| 6 | 3.6 | 45–180 | ±5 | <0.6/>15 | 38.5 |
| 8 | 2.25 | 0–360 | ±10 | <0.5/>10 | 64 |
| 9 | 3 | 90 | ±2.8 | <1.2/>10 | 58 |
| 10 | 3 | 90 | ±3 | <0.46/>22 | 80 |
| 17 | 3 | 90 | ±3 | <0.97/>19.6 | 60.3 |
| This Work | 3.6 | 90–225 | ±1.2 | <0.84/>16.5 | 60.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, X.; Ma, Y.; Feng, L.; Li, T.; Xu, W.; Chen, H. Constructing a Microstrip Phase Shifter with Low Phase Error Using a Y-Resonator. Electronics 2024, 13, 5006. https://doi.org/10.3390/electronics13245006
Fan X, Ma Y, Feng L, Li T, Xu W, Chen H. Constructing a Microstrip Phase Shifter with Low Phase Error Using a Y-Resonator. Electronics. 2024; 13(24):5006. https://doi.org/10.3390/electronics13245006
Chicago/Turabian StyleFan, Xiangsuo, Yanfang Ma, Linping Feng, Tianfeng Li, Wenhao Xu, and Huajin Chen. 2024. "Constructing a Microstrip Phase Shifter with Low Phase Error Using a Y-Resonator" Electronics 13, no. 24: 5006. https://doi.org/10.3390/electronics13245006
APA StyleFan, X., Ma, Y., Feng, L., Li, T., Xu, W., & Chen, H. (2024). Constructing a Microstrip Phase Shifter with Low Phase Error Using a Y-Resonator. Electronics, 13(24), 5006. https://doi.org/10.3390/electronics13245006





