Active Power Dispatch of Renewable Energy Power Systems Considering Multiple Renewable Energy Station Short-Circuit Ratio Constraints
Abstract
1. Introduction
2. Multiple Renewable Energy Stations Short Circuit Ratio Constraint Based on Multivariate Least Squares Fitting
2.1. MRSCR Indicators
2.2. Constraint Conditions Based on the Short-Circuit Ratio of Multiple Renewable Energy Stations
2.3. Multivariate Least Squares Fitting of the MRSCR Constraint
2.3.1. Multivariate Least Squares Method
2.3.2. Multivariate Least Squares Fitting of the Short-Circuit Ratio Constraint
3. Short-Term Economic Dispatch Model for Wind-Thermal Power Coordination Considering MRSCR
3.1. Objective Function
3.2. Constraints
- Logical constraints on the operational status of dispatchable units:
- 2.
- Minimum startup and shutdown time constraints for generators:
- 3.
- Ramp rate limit constraints for generators:
- 4.
- Maximum and minimum generation capacity constraints for generators:
- 5.
- Power balance constraints:
- 6.
- Transmission line capacity constraints:
- 7.
- Power system reserve capacity constraints:
- 8.
- MRSCR Constraints:
4. Case Study
4.1. Experimental Parameters and Scenario Setup
4.1.1. Parameters of Dispatchable Thermal Power Units
4.1.2. Wind Power Plant Output Curve
4.1.3. Grid Load Curve
4.2. Fitting and Calculation of MRSCR Constraints
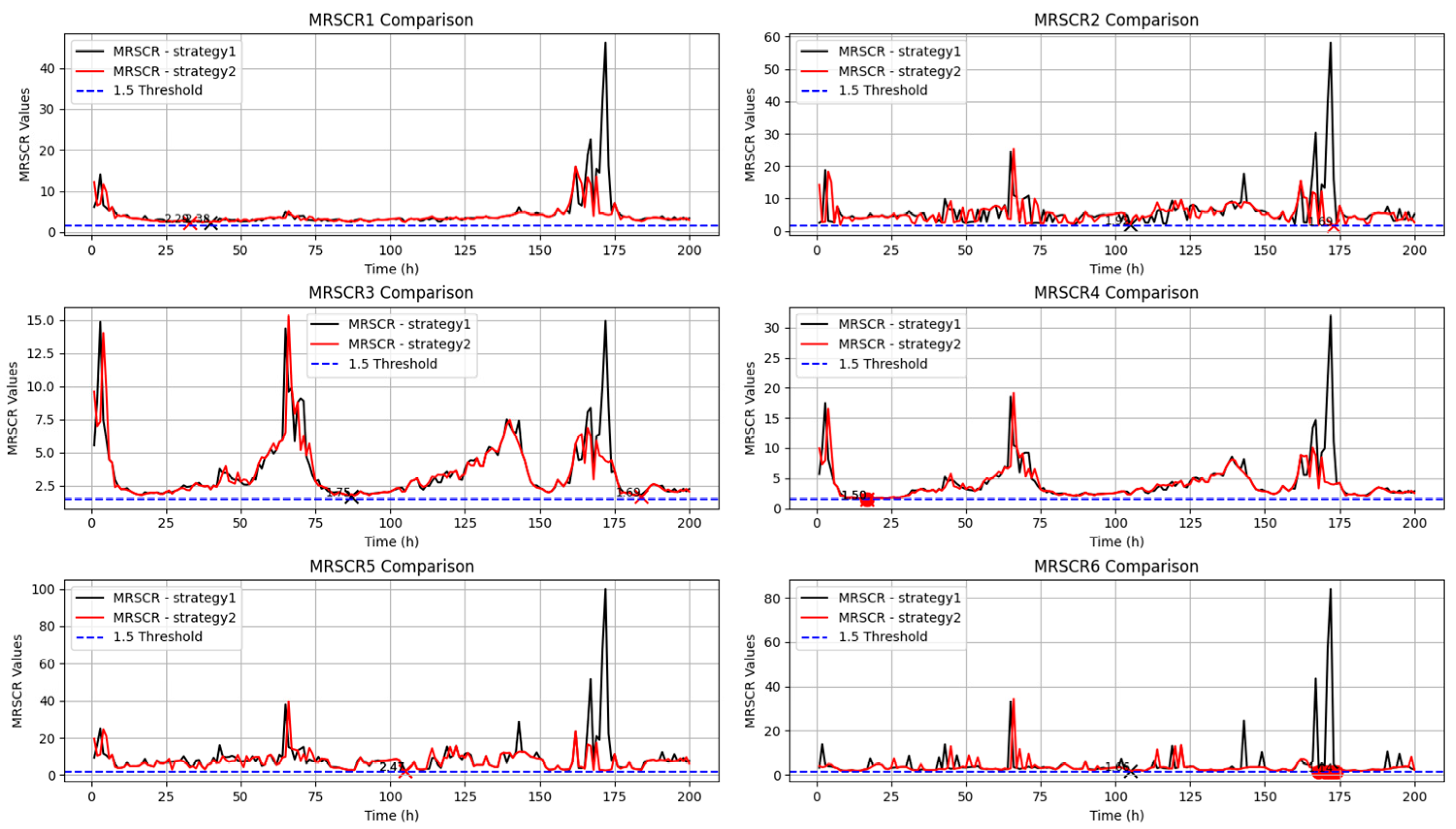
4.3. Experimental Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, X.; Lu, Z.; Liu, Y.; Chen, S. Development models and key technologies of future grid in China. Proc. CSEE 2014, 34, 4999–5008. (In Chinese) [Google Scholar]
- Sun, H.; Xu, T.; Guo, Q.; Li, Y.; Lin, W.; Yi, J.; Li, W. Analysis on blackout in Great Britain power grid on August 9th, 2019 and its enlightenment to power grid in China. Proc. CSEE 2019, 39, 6183–6192. (In Chinese) [Google Scholar]
- Ma, J.; Zhao, D.; Qian, M.; Zhu, L.; Yao, L.; Wang, N. Reviews of control technologies of large-scale renew-able energy connected to weakly-synchronized sending-end DC power grid. Power Syst. Technol. 2017, 41, 3112–3120. (In Chinese) [Google Scholar]
- Kang, C.; Yao, L. Key scientific issues and theoretical research framework for power systems with high proportion of renewable energy. Autom. Electr. Power Syst. 2017, 41, 2–11. (In Chinese) [Google Scholar]
- Sun, H.; Zhang, Z.; Lin, W.; Yang, Y.; Luo, X.; Wang, A. Analysis on serious wind turbine generators tripping accident in northwest China power grid in 2011 and its lessons. Power Syst. Technol. 2012, 36, 76–80. (In Chinese) [Google Scholar]
- Ebrie, A.S.; Kim, Y.J. Reinforcement learning-based optimization for power scheduling in a renewable energy connected grid. Renew. Energy 2024, 230, 120886. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, C.; Wang, Y. Cooperative game optimization scheduling of multi-region integrated energy system based on ADMM algorithm. Energy 2024, 302, 131728. [Google Scholar] [CrossRef]
- Zhu, X.; Hu, M.; Xue, J.; Li, Y.; Han, Z.; Gao, X.; Wang, Y.; Bao, L. Re-search on multi-time scale integrated energy scheduling optimization considering carbon constraints. Energy 2024, 302, 131776. [Google Scholar] [CrossRef]
- Zhang, Y.; Ma, C.; Yang, Y.; Pang, X.; Liu, L.; Lian, J. Study on Short-Term Optimal Operation of Cascade Hydro-Photovoltaic Hybrid Systems. Appl. Energy 2021, 291, 116828. [Google Scholar] [CrossRef]
- Guo, S.; Zheng, K.; He, Y.; Kurban, A. The Artificial Intelligence-Assisted Short-Term Optimal Scheduling of a Cascade Hydro Photovoltaic Complementary System with Hybrid Time Steps. Renew. Energy 2023, 202, 1169–1189. [Google Scholar] [CrossRef]
- Aguilar, D.; Quinones, J.J.; Pineda, L.R.; Ostanek, J.; Castillo, L. Optimal sched-uling of renewable energy microgrids: A robust multi-objective approach with machine learning-based probabilistic forecasting. Appl. Energy 2024, 369, 123548. [Google Scholar] [CrossRef]
- Gao, Y.; Chang, X.; Xue, Y.; Su, J.; Li, Z.; Sun, H. Interval Optimization Scheduling of Renewable Hydrogen Refueling Stations Considering Wind and Solar Uncertainties and Dynamic Hydrogen Prices. Power Syst. Technol. 2024, 1–13. [Google Scholar] [CrossRef]
- Dong, Z.; Zhang, Z.; Huang, M.; Yang, S.; Zhu, J.; Zhang, M.; Chen, D. Research on day-ahead optimal dispatching of virtual power plants considering the coordinated operation of diverse flexible loads and new energy. Energy 2024, 297, 131235. [Google Scholar] [CrossRef]
- Li, X.; Dong, Y.; Liu, H.; Yao, Z. Multi-Time-Scale Scheduling Model for Renewable Energy Grid Integration Considering the Generalized Short-Circuit Ratio. Mod. Electr. Power 2024, 41, 240–248. (In Chinese) [Google Scholar] [CrossRef]
- Vineeth, V.V.; Vijayalakshmi, V.J. A novel deep learning approach for estimating and classifying short-term voltage stability events in modern power systems with composite load and distributed energy resources. Electr. Eng. 2024, 1–13. [Google Scholar] [CrossRef]
- Paul, C.; Sarkar, T.; Dutta, S.; Hazra, S.; Roy, P.K. Optimal Power Flow of Multi-objective Combined Heat and Power with Wind-Solar-Electric Vehicle-Tidal Using Hybrid Evolutionary Approach. Process Integr. Optim. Sustain. 2024, 1–31. [Google Scholar] [CrossRef]
- Hong, H. Optimization Scheduling of Novel Power Systems Considering Frequency-Inertia Safety Constraints; Hangzhou Dianzi University: Hangzhou, China, 2024. (In Chinese) [Google Scholar] [CrossRef]
- Zhang, Y. Study on the Impact of UPFC and New Energy Devices on Power System Stability; North China Electric Power University: Beijing, China, 2017. (In Chinese) [Google Scholar]
- Lai, B. Optimization Operation and Small Disturbance Stability Analysis of New Energy Power Systems; North China Electric Power University: Beijing, China, 2016. (In Chinese) [Google Scholar]
- Jia, H.; Wang, L. Study on Small Disturbance Stability of Power Systems with Large-Scale Wind Farms. Power Syst. Technol. 2012, 36, 61–69. (In Chinese) [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, L.; Chen, S.; An, N. Impact of Large-Scale Wind Farms on the Small Disturbance Stability and Damping Characteristics of Power Systems. Power Syst. Technol. 2007, 13, 75–80. (In Chinese) [Google Scholar]
- GB 38755-2019; Code on Security and Stability for Power System. National Energy Agency: Beijing, China, 2019.
- Zhang, Y.; Huang, S.H.; Schmall, J.; Conto, J.; Billo, J.; Rehman, E. Evaluating system strength for large-scale wind plant integration. In Proceedings of the IEEE PES General Meeting, National Harbor, MD, USA, 27–31 July 2014. [Google Scholar]
- Report to NERC ERSTF for Composite Short Circuit Ratio (CSCR) Estimation Guideline; GE Energy Consulting: Sche-nectady, NY, USA, 2015.
- CIGRE. CIGRE Working Group B4.41. In Connection of Wind Farms to Weak AC Networks; CIGRE: Paris, France, 2016. [Google Scholar]
- Wu, D.; Li, G.; Javadi, M.; Malyscheff, A.M.; Hong, M.; Jiang, J.N. Assessing impact of renewable energy integration on system strength using site-dependent short circuit ratio. IEEE Trans. Sustain. Energy 2018, 9, 1072–1080. [Google Scholar] [CrossRef]
- Sun, H.; Xu, S.; Xu, T.; Guo, Q.; He, J.; Zhao, B.; Yu, L.; Zhang, Y.; Li, W.; Zhou, Y. Definition and Metrics of Multiple Renewable Energy Station Short-Circuit Ratio. Proc. CSEE 2021, 41, 497–506. (In Chinese) [Google Scholar] [CrossRef]


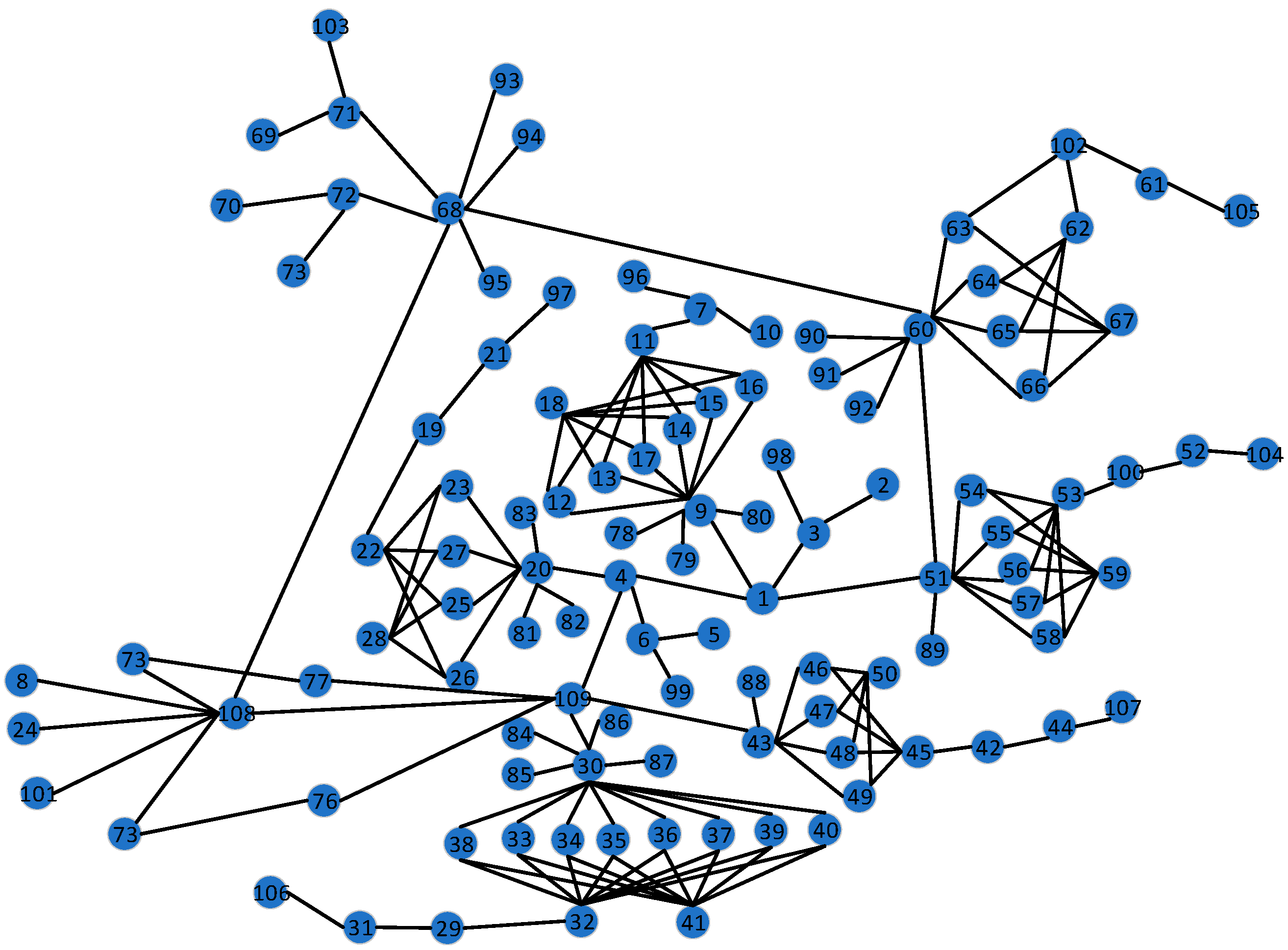

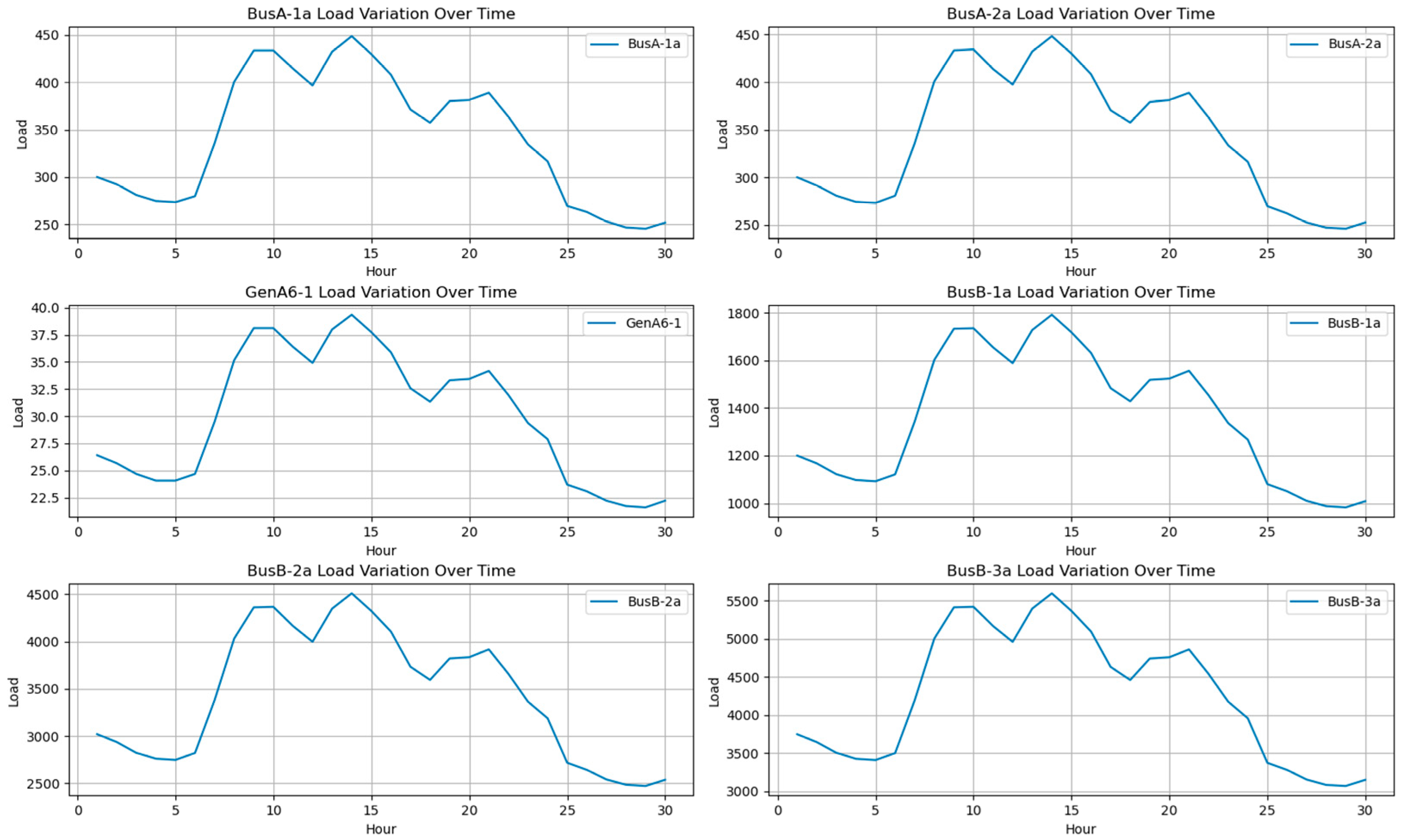
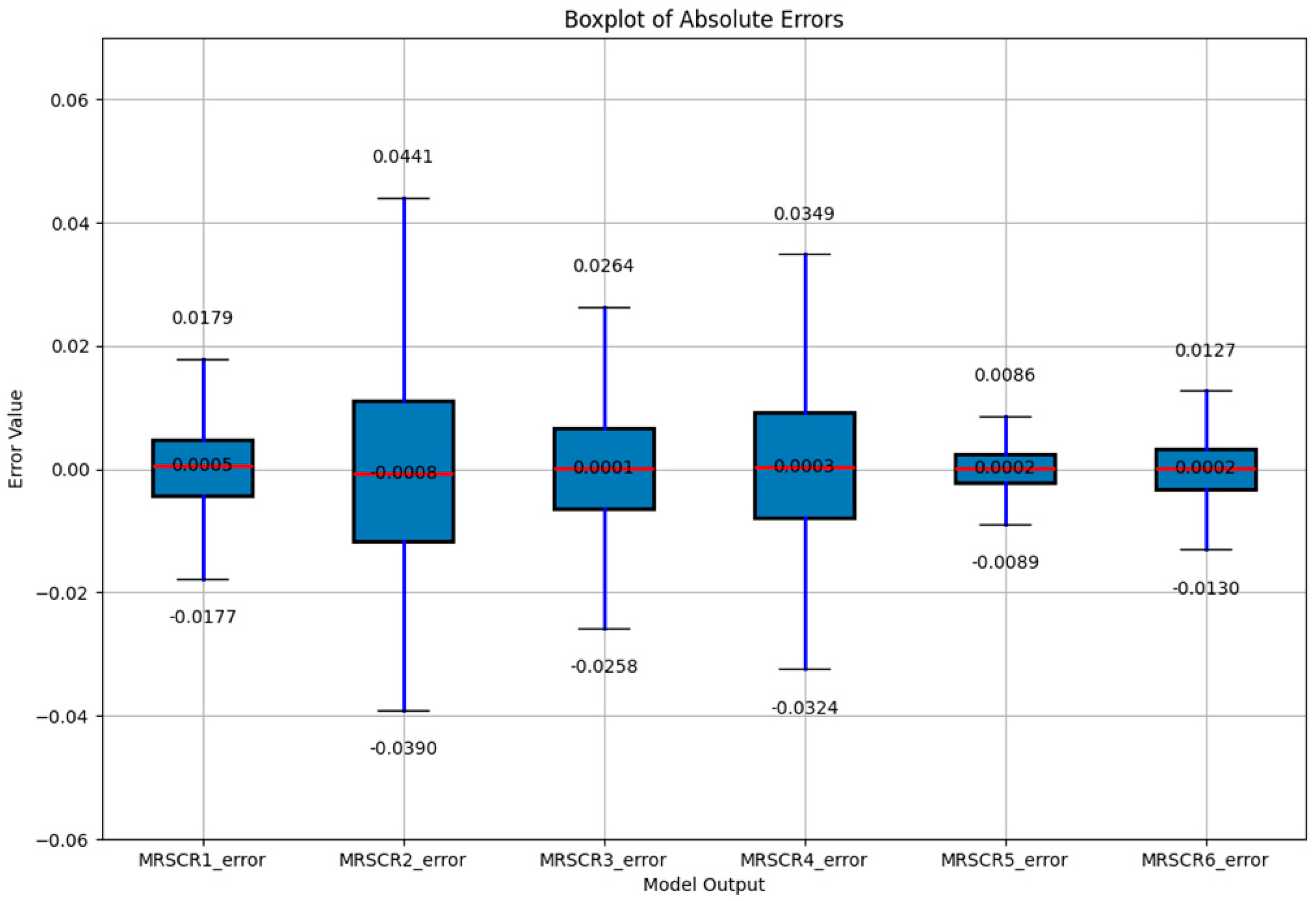

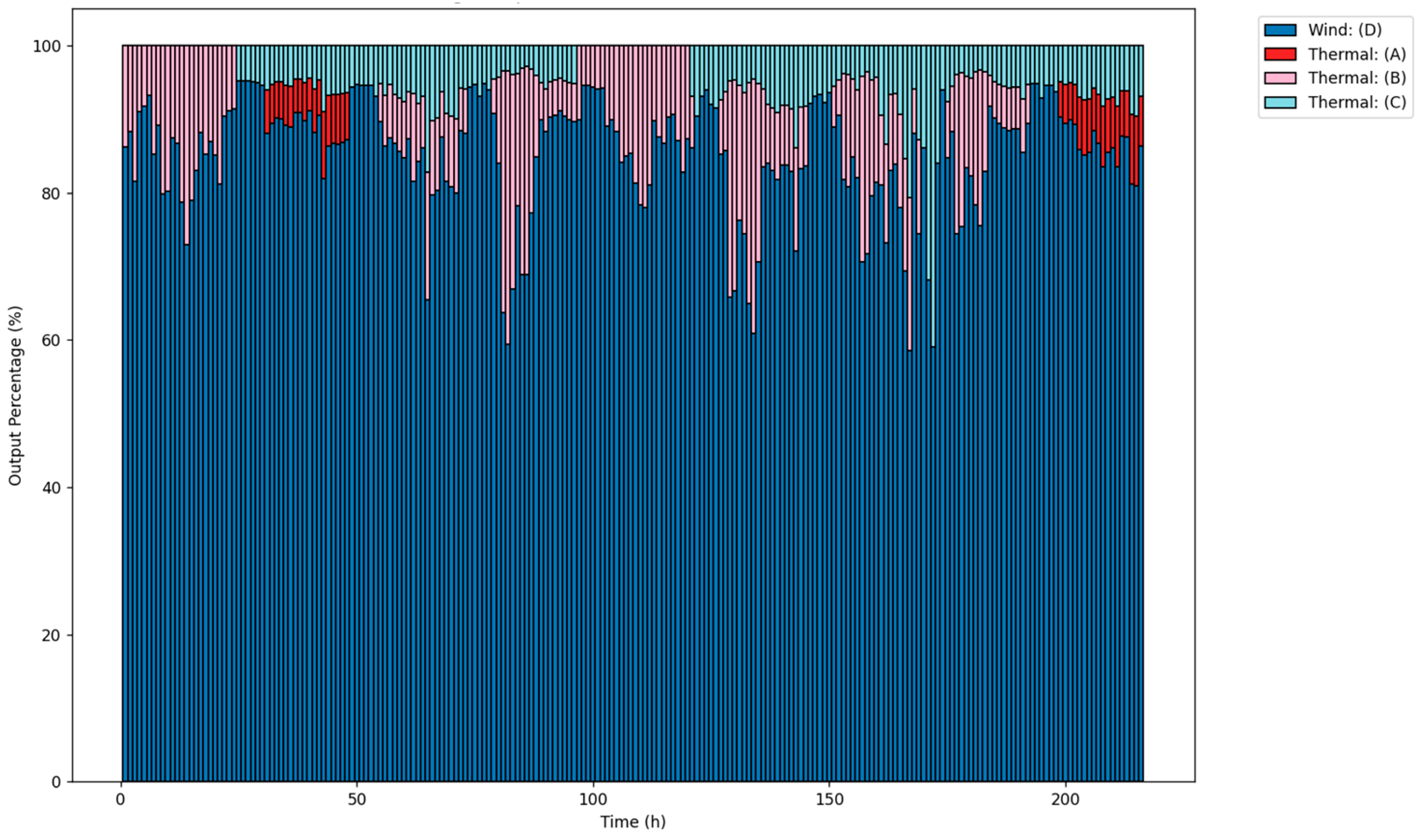

| Node | Maxcap (MW) | Mincap (MW) | heat_rate (MMBtu/MWh) | var_om ($/MWh) | fix_om ($) | st_cost ($/start) | Ramp (MW/h) | Minup (h) | Mindn (h) |
|---|---|---|---|---|---|---|---|---|---|
| 78 | 600 | 180 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 79 | 580 | 174 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 80 | 544 | 163 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 81 | 560 | 168 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 82 | 640 | 192 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 83 | 500 | 150 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 84 | 650 | 195 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 85 | 680 | 204 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 86 | 600 | 180 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 87 | 600 | 180 | 10.05 | 4.6 | 2.7 | 90 | 800 | 10 | 10 |
| 88 | 620 | 186 | 10.05 | 4.6 | 2.6 | 90 | 1000 | 9 | 11 |
| 89 | 580 | 174 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 90 | 600 | 180 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 91 | 560 | 168 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 92 | 640 | 192 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 93 | 600 | 180 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 94 | 620 | 186 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| 95 | 600 | 180 | 14.1 | 26 | 1 | 60 | 800 | 1 | 1 |
| TIME (h) | MRSCR1 | MRSCR2 | MRSCR3 | MRSCR4 | MRSCR5 | MRSCR6 |
|---|---|---|---|---|---|---|
| 166 | 18.85373 | 18.31047 | 8.08322 | 13.35676 | 26.45281 | 6.22232 |
| 166 | 13.40469 | 12.00423 | 6.87182 | 10.07883 | 16.48418 | 3.26143 |
| 167 | 22.62489 | 30.29640 | 8.38348 | 14.65219 | 51.59498 | 43.5647 |
| 167 | 11.67327 | 11.33970 | 6.20249 | 8.689696 | 15.78557 | 3.29426 |
| 168 | 5.510105 | 2.191812 | 3.85195 | 4.620816 | 8.062710 | 2.82580 |
| 168 | 3.927695 | 1.767404 | 2.99019 | 3.282451 | 2.722518 | 1.45523 |
| 169 | 15.44229 | 14.38219 | 6.24638 | 9.245981 | 20.99923 | 5.19328 |
| 169 | 13.51455 | 12.22165 | 5.901937 | 8.432638 | 17.41708 | 3.92059 |
| 170 | 14.45075 | 13.29922 | 6.384079 | 9.916256 | 18.88385 | 4.16951 |
| 170 | 4.574496 | 1.817756 | 4.781466 | 4.385137 | 2.801712 | 1.38901 |
| Approach | Objective Function | Computation Time | Metric Evaluation |
|---|---|---|---|
| MRSCR-Constrained Approach | 10,200,210.74 | 131.94 s | Better |
| MRSCR-Nonconstrained Approach | 10,200,209.73 | 95.356 s | Normal |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, L.; Xu, M.; Lin, J.; Xu, H.; Zheng, L. Active Power Dispatch of Renewable Energy Power Systems Considering Multiple Renewable Energy Station Short-Circuit Ratio Constraints. Electronics 2024, 13, 3811. https://doi.org/10.3390/electronics13193811
Wu L, Xu M, Lin J, Xu H, Zheng L. Active Power Dispatch of Renewable Energy Power Systems Considering Multiple Renewable Energy Station Short-Circuit Ratio Constraints. Electronics. 2024; 13(19):3811. https://doi.org/10.3390/electronics13193811
Chicago/Turabian StyleWu, Linlin, Man Xu, Jiajian Lin, Haixiang Xu, and Le Zheng. 2024. "Active Power Dispatch of Renewable Energy Power Systems Considering Multiple Renewable Energy Station Short-Circuit Ratio Constraints" Electronics 13, no. 19: 3811. https://doi.org/10.3390/electronics13193811
APA StyleWu, L., Xu, M., Lin, J., Xu, H., & Zheng, L. (2024). Active Power Dispatch of Renewable Energy Power Systems Considering Multiple Renewable Energy Station Short-Circuit Ratio Constraints. Electronics, 13(19), 3811. https://doi.org/10.3390/electronics13193811






