Aggregation Equivalence Method for Direct-Drive Wind Farms Based on the Excitation–Response Relationship
Abstract
1. Introduction
- (1)
- Using the amplitude and phase of the terminal voltage and the output active and reactive power as the boundary signals for the grid-connected PMSG model, an excitation–response relationship model of the voltage/power of the grid side converter (GSC) of the PMSG is established to describe the dynamic characteristics of the port of the PMSG. The eigenvalues of the new model are highly consistent with those of the state-space equation model.
- (2)
- Based on the unit model, an aggregation equivalent method for a direct-drive wind farm based on the excitation–response relationship is proposed. The proposed method takes into account the differences in operating conditions of PMSGs and the length of collecting power lines; therefore, it has good accuracy in small disturbance stability analysis of wind farm grid-connected systems, and the proposed method is simple and convenient for modification and analysis.
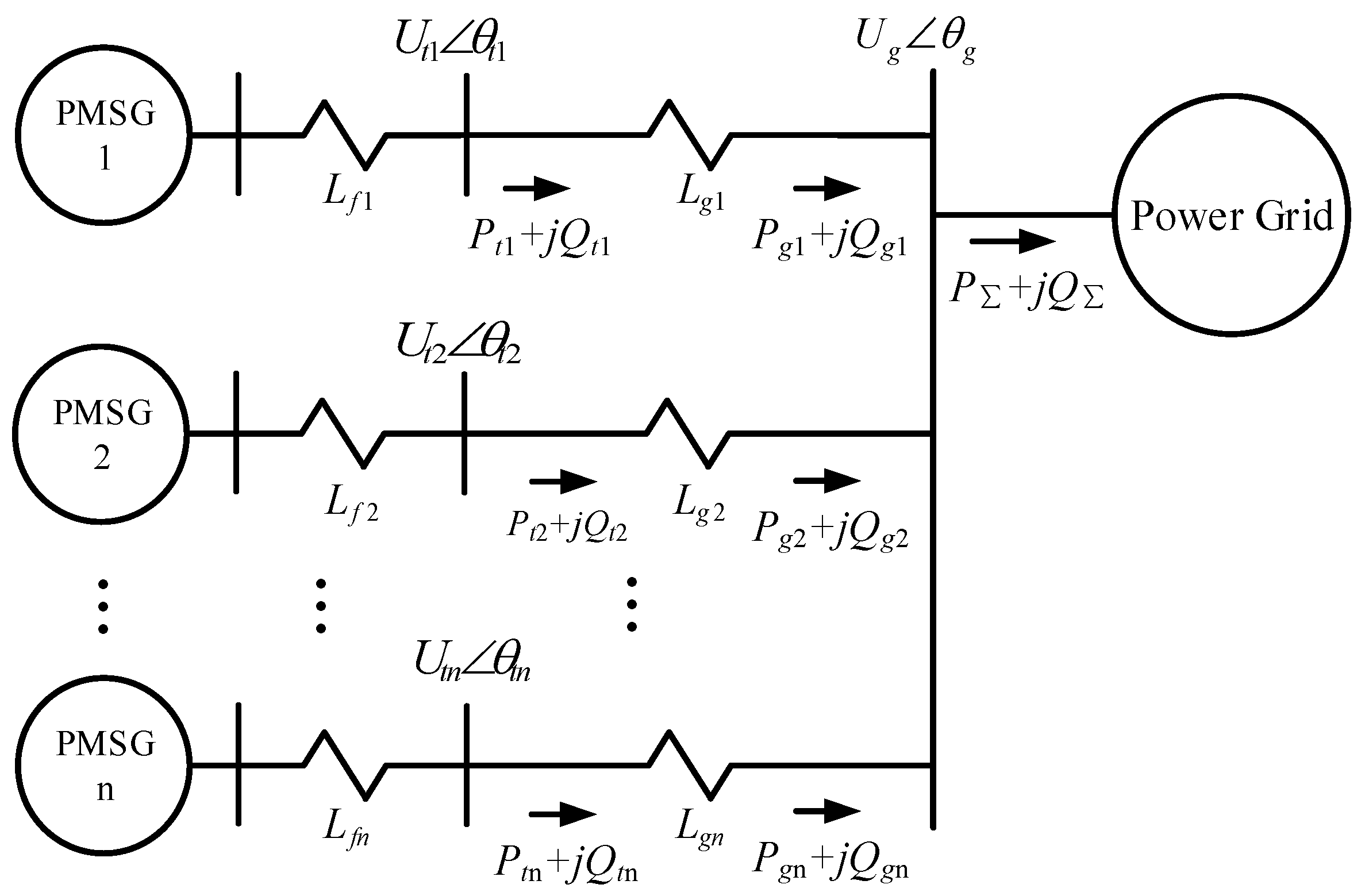
2. Excitation–Response Relationship Model of the Terminal Voltage/Output Power for the Grid-Connected PMSG System
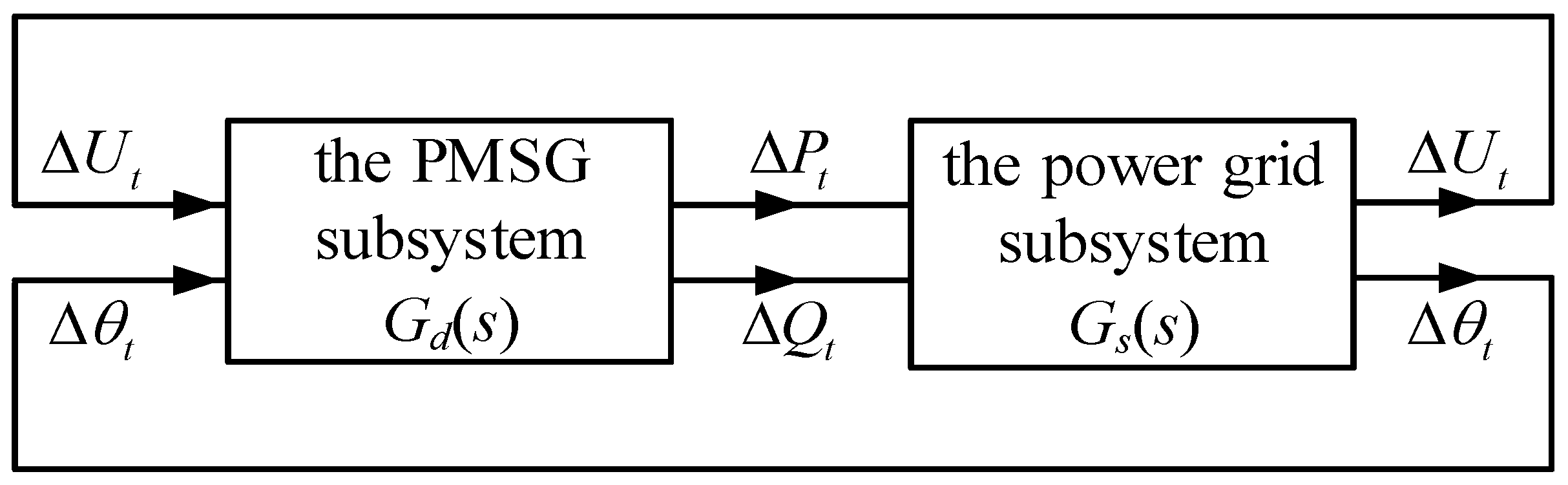
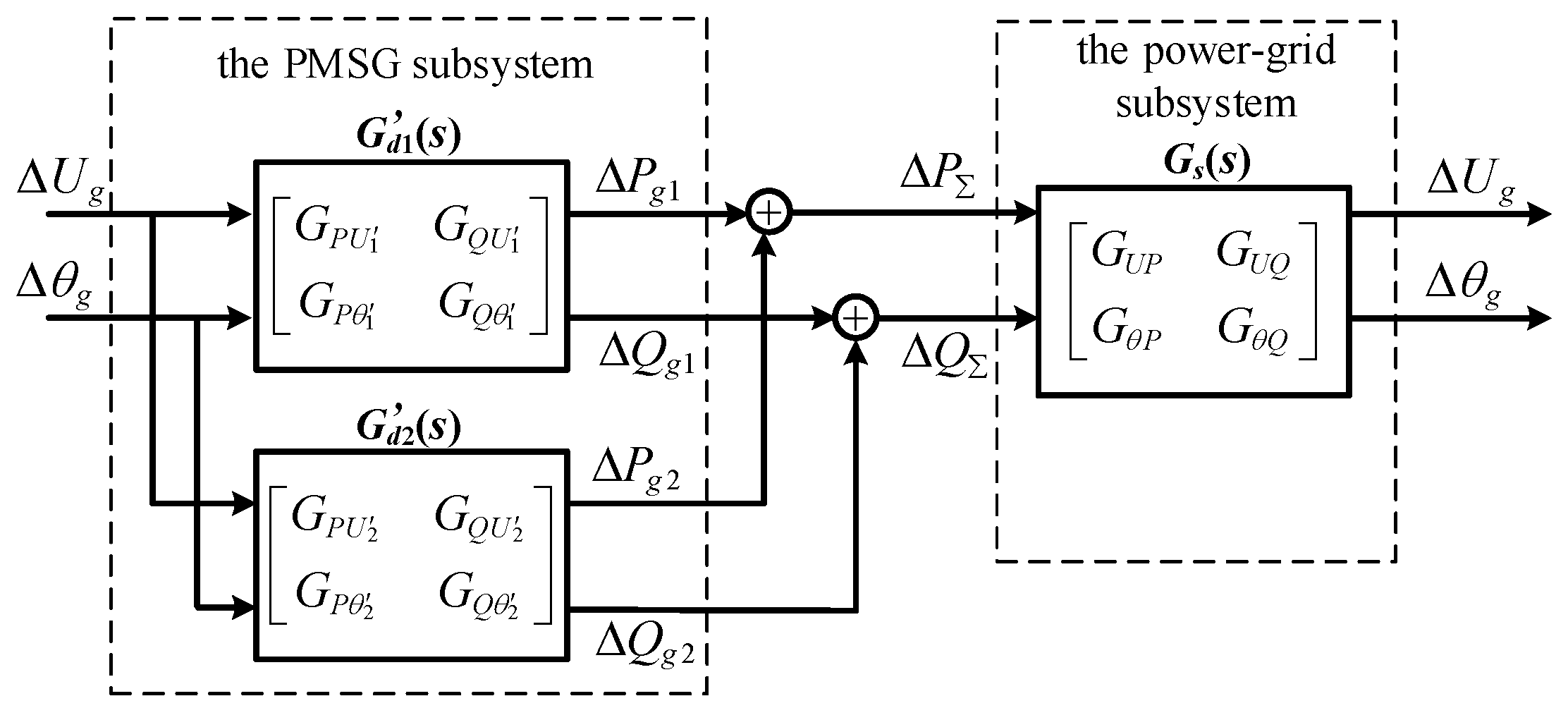
2.1. Model of the PMSG Subsystem
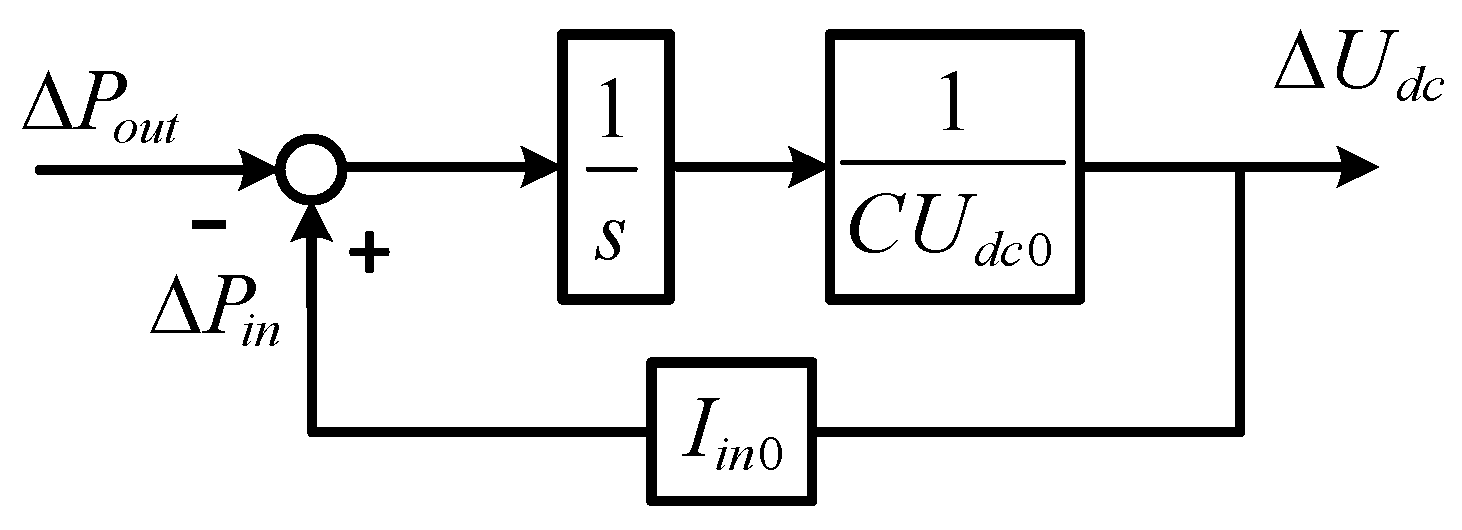
2.2. Model of the Power Grid Subsystem
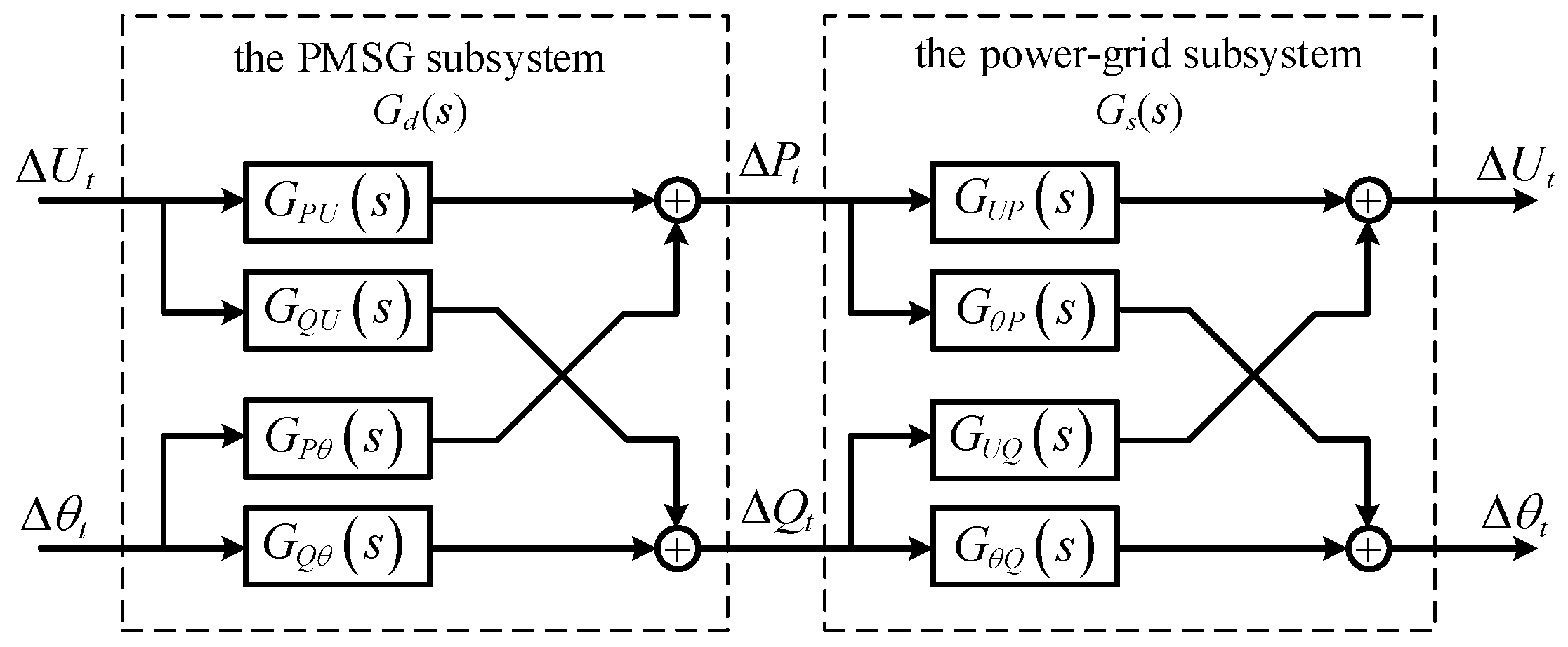
2.3. Model of the Grid-Connected PMSG System
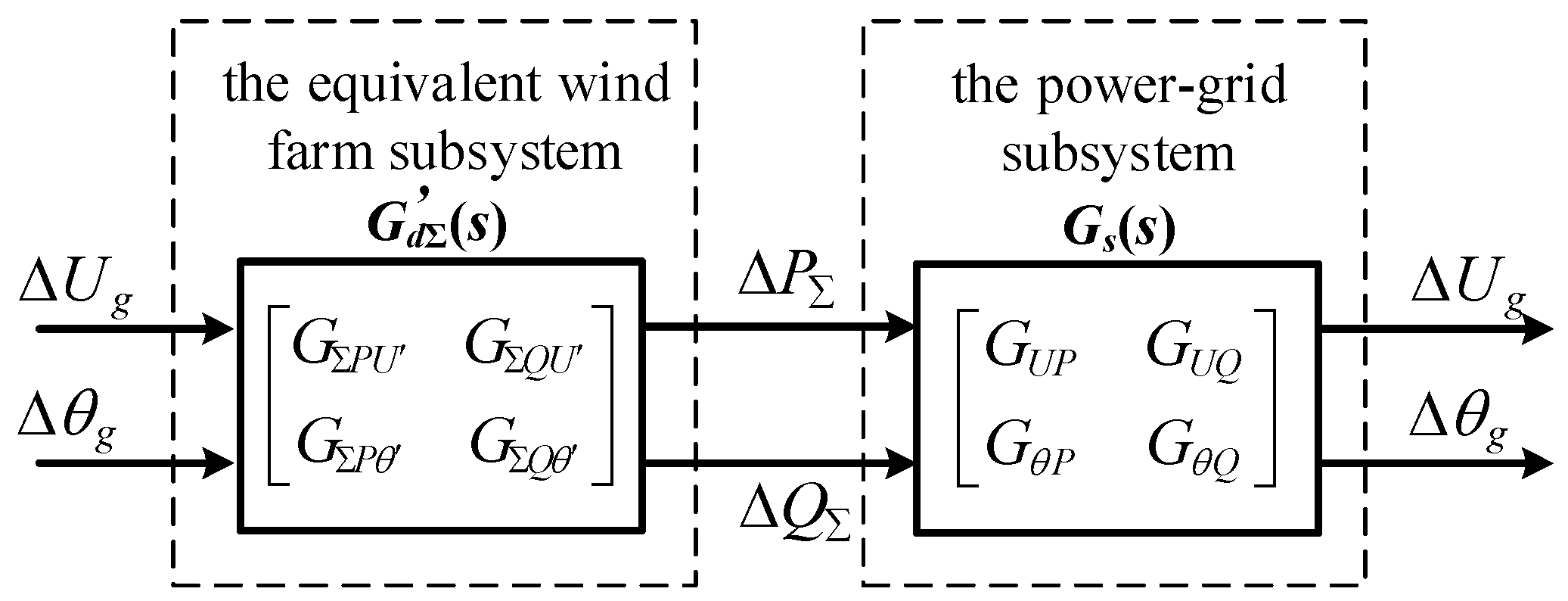
3. Excitation–Response Relationship between the Voltage / and the Output Power / at the Convergence Busbar for the PMSGs
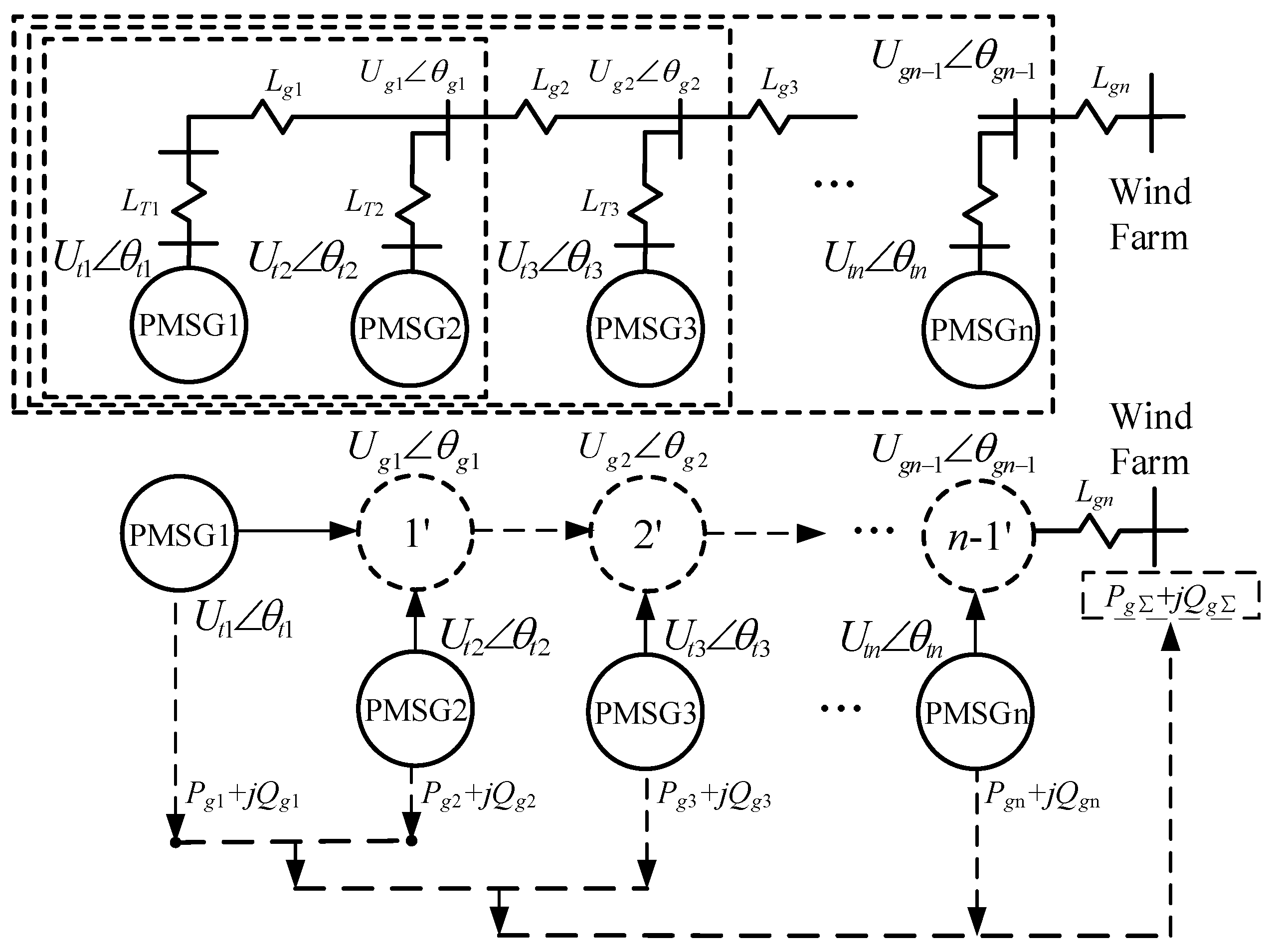
4. Aggregation Equivalence for Direct-Driven Wind Farms Based on the Excitation–Response Relationship
5. Model Verification and Analysis
5.1. Verification and Analysis of the Grid-Connected PMSG Model Based on the Excitation–Response Relationship
- Effect of operating conditions on the dominant oscillation modes
- 2.
- Influence of power grid strengths on the dominant oscillation modes
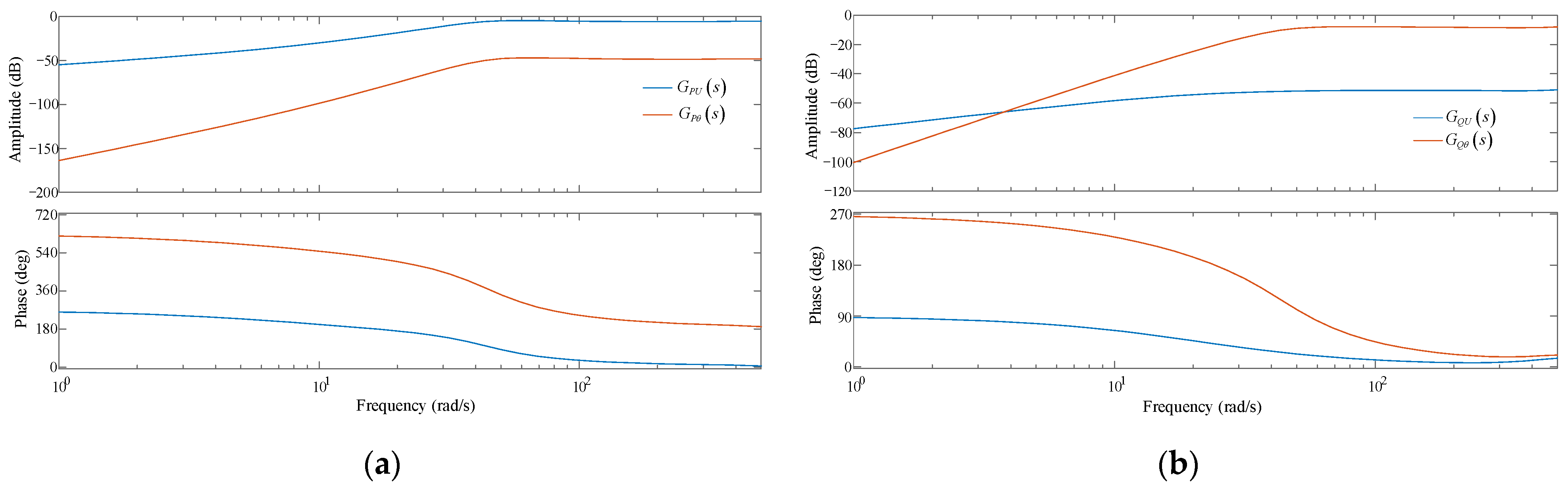
5.2. Active and Reactive Power Response Characteristics of Direct-Driven PMSG
5.3. Aggregation Equivalence Verification of Direct-Driven Wind Farms Based on the Excitation–Response Relationship
- Set voltage amplitude disturbance
- 2.
- Voltage phase disturbance
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
| Symbol | Variable | Numerical Value |
|---|---|---|
| Sbase | Rated power | 2 MW |
| Vbase | Rated voltage | 0.69 kV |
| fbase | Rated frequency | 50 Hz |
| Udcref | dc capacitance voltage reference value | 1.2 kV |
| Cdc | Direct current capacitance | 0.1 F |
| Lf | Filter inductance | 0.00015 H |
| (kpdc, kidc) | Voltage outer loop proportional integral parameters | (3.5, 140) |
| (kppll, kipll) | PLL proportional integral parameter | (50, 2000) |
| (kpi, kii) | Current inner loop proportional integral parameter | (0.3, 160) |
| Operating Condition | PMSG1 | PMSG2 | PMSG3 | PMSG4 | PMSG5 | PMSG6 |
|---|---|---|---|---|---|---|
| Operating condition (P0) | 0.1 p.u. | 0.1 p.u. | 0.2 p.u. | 0.2 p.u. | 0.4 p.u. | 0.4 p.u. |
| Feeder reactance | 0.2 mH | 0.4 mH | 0.2 mH | 0.4 mH | 0.2 mH | 0.4 mH |
Appendix C

References
- Zhou, X.X.; Chen, S.Y.; Lu, Z.X.; Huang, Y.H.; Ma, S.C. Technology features of the new generation power system in China. Proc. CSEE 2018, 38, 1893–1904+2205. [Google Scholar]
- Li, M.J.; Yu, Z.; Xu, T.; He, J.B.; Wang, C.; Xie, X.R.; Liu, C. Study of Complex Oscillation Caused by Renewable Energy Integration and Its Solution. Power Syst. Technol. 2017, 41, 1035–1042. [Google Scholar]
- Chen, G.P.; Li, M.J.; Xu, T.; Zhang, J.Y.; Wang, C. Practice and Challenge of Renewable Energy Development Based on Interconnected Power Grid. Power Syst. Technol. 2017, 41, 3095–3103. [Google Scholar]
- Xie, X.R.; He, J.B.; Mao, H.Y.; Li, Y.Z. New issues and classification of power system stability with high shares of renewables and power electronics. Proc. CSEE 2021, 41, 461–474. [Google Scholar]
- Du, W.J.; Wang, Y.J.; Wang, H.F.; Ren, B.X.; Xiao, X.Y. Small-disturbance stability limit of a grid-connected wind farm with PMSGs in the timescale of DC voltage dynamics. IEEE Trans. Power Syst. 2021, 36, 2366–2379. [Google Scholar] [CrossRef]
- Zhang, M.Y.; Xiao, S.W.; Tian, T.; Zhang, H.H.; Bi, T.S. Analysis of SSO Influencing Factors and Parameter Adjustment for Grid-Connected Full-Converter Wind Farm Based on Impedance Sensitivity. Power Syst. Technol. 2018, 42, 2768–2777. [Google Scholar]
- Sheng, Y.B.; Lin, T.; Chen, B.P.; Chen, R.S.; Guo, Z.Y.; Xu, X.L. Coordination and Optimization of Controller Parameters for Subsynchronous/Super-Synchronous Oscillation in New Energy Delivery Systems. Trans. China Electrotech. Soc. 2019, 34, 984–992. [Google Scholar]
- Ali, M.; IIie, I.S.; Milanović, J.V.; Chiccoet, G. Wind Farm Model Aggregation Using Probabilistic Clustering. IEEE Trans. Power Syst. 2013, 28, 309–316. [Google Scholar] [CrossRef]
- Zhang, J.; He, Y.G. Parameters Identification of Equivalent Model of Permanent Magnet Synchronous Generator Wind Farm Based on Analysis of Trajectory Sensitivity. Trans. China Electrotech. Soc. 2020, 35, 3303–3313. [Google Scholar]
- Li, H.; Yang, C.; Zhao, B.; Wang, H.S.; Chen, Z. Aggregated models and transient performances of a mixed wind farm with different wind turbine generator systems. Elecrt. Power Syst. Res. 2012, 92, 1–10. [Google Scholar] [CrossRef]
- Wang, Y.F.; Lu, C.; Zhu, L.P.; Zhang, G.L.; Li, X.; Chen, Y. Comprehensive modeling and parameter identification of wind farms based on wide-area measurement systems. J. Mod. Power Syst. Cle. 2016, 4, 383–393. [Google Scholar] [CrossRef]
- Jalili-Marandi, V.; Pak, L.F.; Dinavahi, V. Real-time simulation of grid-connected wind farms using physical aggregation. IEEE Trans. Ind. Electron. 2010, 57, 3010–3021. [Google Scholar] [CrossRef]
- Zhou, Y.H.; Zhao, L.; Lee, W.J. Robustness analysis of dynamic equivalent model of DFIG wind farm for stability study. IEEE Trans. Ind. Appl. 2018, 54, 5682–5690. [Google Scholar] [CrossRef]
- Zou, J.X.; Peng, C.; Xu, H.B. A fuzzy clustering algorithm-based dynamic equivalent modeling method for wind farm with DFIG. IEEE Trans. Energy Conver. 2015, 30, 1329–1337. [Google Scholar] [CrossRef]
- Cheng, X.Y.; Lee, W.J.; Sahni, M.; Cheng, X.Z.; Lee, L. Dynamic Equivalent Model Development to Improve the Operation Efficiency of Wind Farm. IEEE Trans. Ind. Appl. 2016, 52, 2759–2767. [Google Scholar] [CrossRef]
- Kong, M.Y.; Sun, D.; Nian, H. Modeling Method for Power Response Characteristics of DFIG-based Wind Turbine System. Autom. Electr. Power Syst. 2022, 46, 118–125. [Google Scholar]
- Chen, S.Y.; Wang, C.; Shen, H.; Gao, N.C.; Zhu, L.; Lan, H. Dynamic Equivalence for Wind Farms Based on Clustering Algorithm. Proc. CSEE 2012, 32, 11–19. [Google Scholar]
- Dong, W.K.; Wang, Y.; Wang, H.F. Single-machine Equivalent Model of a Group of Wind Turbine Generators for Small-signal Stability Analysis. Power Syst. Technol. 2021, 45, 1241–1250. [Google Scholar]
- Dong, W.K.; Du, W.J.; Wang, H.F. Dynamic equivalent model of a grid-connected wind farm for oscillation stability analysis. Proc. CSEE 2021, 41, 75–87+399. [Google Scholar]
- He, J.Y.; Zhou, Y.H.; Wang, K.; Li, L.; Li, Z.H.; Xin, H.H. Self-adaptive Equivalence Method for Wind Farm with Maintained Dominant Mode. Autom. Electr. Power Syst. 2021, 45, 28–36. [Google Scholar]
- Xie, X.R.; Liu, H.K.; He, J.B.; Liu, W. Small-signal Impedance Admittance Network Modeling for Grid-connected Renewable Energy Generation Systems. Autom. Electr. Power Syst. 2017, 41, 26–32. [Google Scholar]
- Wang, Y.P.; Yan, G.G.; Mu, G.; Yang, C. Research on Aggregation Modeling of Grid Connected VSC Under AC Current Control Scale. Proc. CSEE 2022, 42, 2900–2909. [Google Scholar]
- Wang, X.B.; Du, W.J.; Wang, H.F. Mechanism Analysis of Open-loop Modal Resonance on Subsynchronous Oscillations Caused by PLL in Power Systems With Integrated PMSGs. Proc. CSEE 2018, 38, 1935–1950. [Google Scholar]
- Xie, X.R.; Liu, H.K.; He, J.B.; Zhang, C.Y.; Qiao, Y. Mechanism and characteristics of subsynchronous oscillation caused by the interaction between full-converter wind turbines and AC systems. Proc. CSEE 2016, 36, 2366–2372. [Google Scholar]
- He, W.; Yuan, X.M.; Hu, J.B. Inertia provision and estimation of PLL-Based DFIG wind turbines. IEEE Trans. Power Syst. 2017, 32, 510–521. [Google Scholar] [CrossRef]
- Zhang, M.Q.; Yuan, X.M.; Hu, J.B. Inertia and primary frequency provisions of PLL-synchronized VSC HVDC when attached to islanded AC system. IEEE Trans. Power Syst. 2018, 33, 4179–4188. [Google Scholar] [CrossRef]
- Yuan, H.; Yuan, X.M.; Hu, J.B. Modeling of grid-connected VSCs for power system small-signal stability analysis in DC-link voltage control timescale. IEEE Trans. Power Syst. 2017, 32, 3981–3991. [Google Scholar] [CrossRef]
- Hu, Z.J.; Su, S.; Wang, R.P.; Liu, G.L.; Zhang, K.; Xie, X.P. Robust Distributed Load Frequency Control for Multi-area Wind Energy-Dominated Microgrids Considering Phasor Measurement Unit Failures. IEEE Internet Things 2024, in press. [Google Scholar] [CrossRef]
- Hu, Z.J.; Zhang, K.; Su, S.; Wang, R.P.; Li, Y.H. Robust Cooperative Load Frequency Control for Enhancing Wind Energy Integration in Multi-Area Power Systems. IEEE Trans. Autom. Sci. Eng. 2024, in press. [Google Scholar] [CrossRef]
- Zhao, Q.; Zhang, Y.J.; Xie, X.R.; Zhang, Y.Q.; Zhang, D.Z.; Chen, Z.W. Mitigation of Subsynchronous Oscillations Based on Renewable Energy Hydrogen Production System and Its Supplementary Damping Control. Proc. CSEE 2019, 39, 3728–3735. [Google Scholar]
- Shao, B.B.; Zhao, S.Q.; Yang, Y.H.; Gao, B.F.; Wang, L.Y.; Blaabjerg, F. Nonlinear Subsynchronous Oscillation Damping Controller for Direct-Drive Wind Farms With VSC-HVDC Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 2842–2858. [Google Scholar] [CrossRef]
- Yao, J.; Wang, X.W.; Li, J.W.; Liu, R.K.; Zhang, H.L. Sub-Synchronous Resonance Damping Control for Series-Compensated DFIG-Based Wind Farm With Improved Particle Swarm Optimization Algorithm. IEEE Trans. Energy Convers. 2019, 34, 849–859. [Google Scholar] [CrossRef]
- Liu, B.; Li, Z.; Dong, X.L.; Yu, S.S.; Chen, X.; Oo, A.M.T.; Lian, X.Q.; Shan, Z.Y.; Liu, X.D. Impedance Modeling and Controllers Shaping Effect Analysis of PMSG Wind Turbines. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 1465–1478. [Google Scholar] [CrossRef]
- Shao, B.B.; Zhao, S.Q.; Gao, B.F.; Shen, J.L.; Zhao, Z.M. Inside-wind-farm/Wind-farm-grid Sub-synchronous Oscillation Characteristics Analysis in Multiple D-PMSGs Interfaced with VSC-HVDC System. Proc. CSEE 2020, 40, 3835–3847. [Google Scholar]
- Ma, W.D.; Wang, X.T.; Xie, D. Characteristics of Subsynchronous Oscillation Power Propagation in the Large-scale Wind Farm Integrated System. CSEE 2020, 40, 5217–5229. [Google Scholar]
- Chen, W.H.; Wang, D.H.; Guo, X.L.; Chen, X.; Tan, L.N.; Shen, Y. Identifying modeling boundary using local propagation mechanism of subsynchronous oscillations. Proc. CSEE 2017, 37, 4999–5009. [Google Scholar]
- Chao, W.J.; Deng, C.P.; Huang, J.W.; Dai, L.Y.; Min, Y.X.; Cheng, Y.F.; Wang, Y.H.; Liao, J.Q. A Sub-Synchronous Oscillation Suppression Strategy Based on Active Disturbance Rejection Control for Renewable Energy Integration System via MMC-HVDC. Electronics 2023, 12, 2885. [Google Scholar] [CrossRef]
- Sahraoui, M.; Cardoso, A.J.M.; Ghoggal, A. The Use of a Modified Prony Method to Track the Broken Rotor Bar Characteristic Frequencies and Amplitudes in Three-Phase Induction Motors. IEEE Trans. Ind. Appl. 2015, 51, 2136–2147. [Google Scholar] [CrossRef]
- Yutthagowith, P.; Pattanadech, N. Improved Least-Square Prony Analysis Technique for Parameter Evaluation of Lightning ImpulseVoltage and Current. IEEE Tran. Power Del. 2016, 31, 271–277. [Google Scholar] [CrossRef]


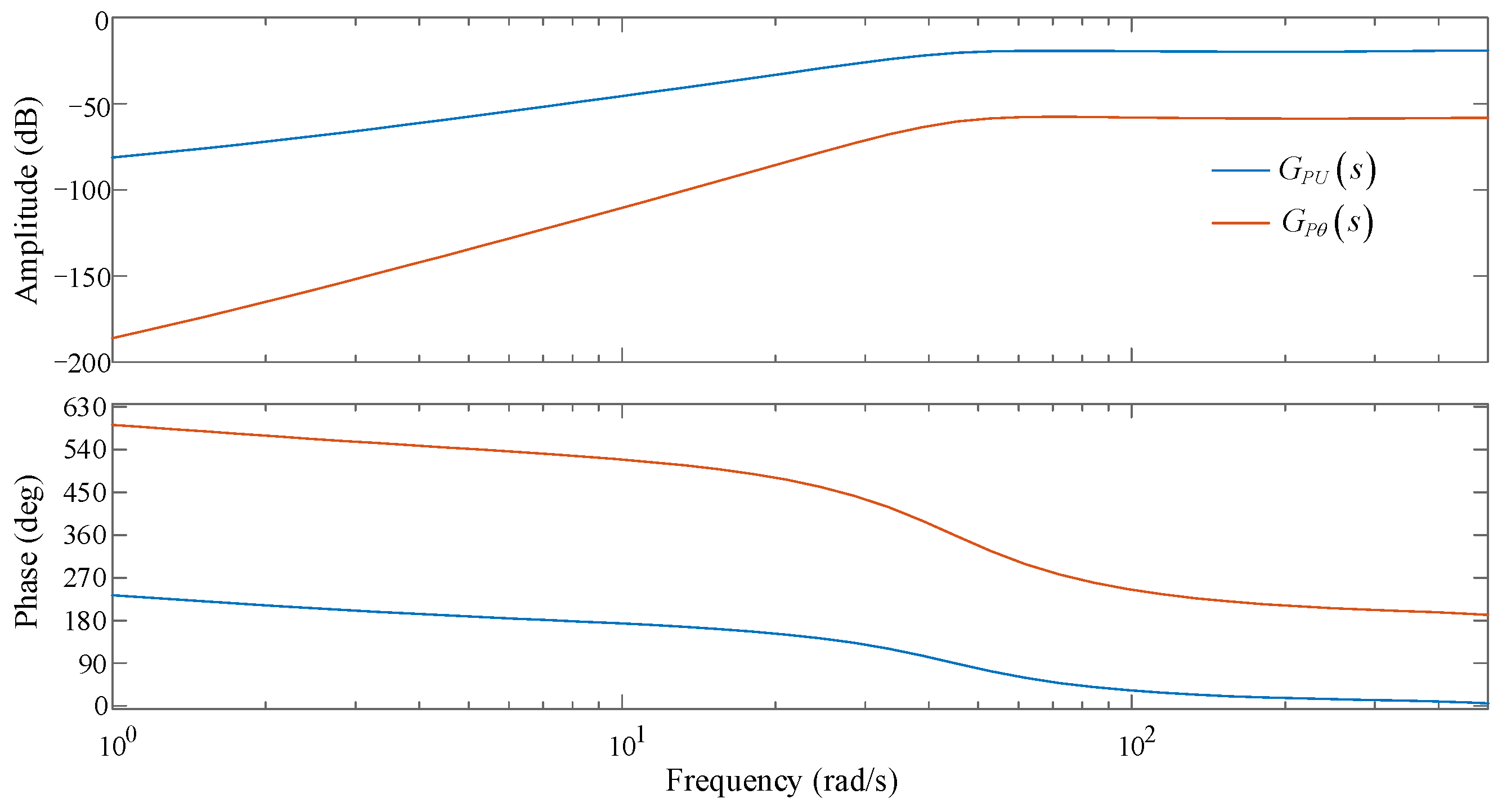
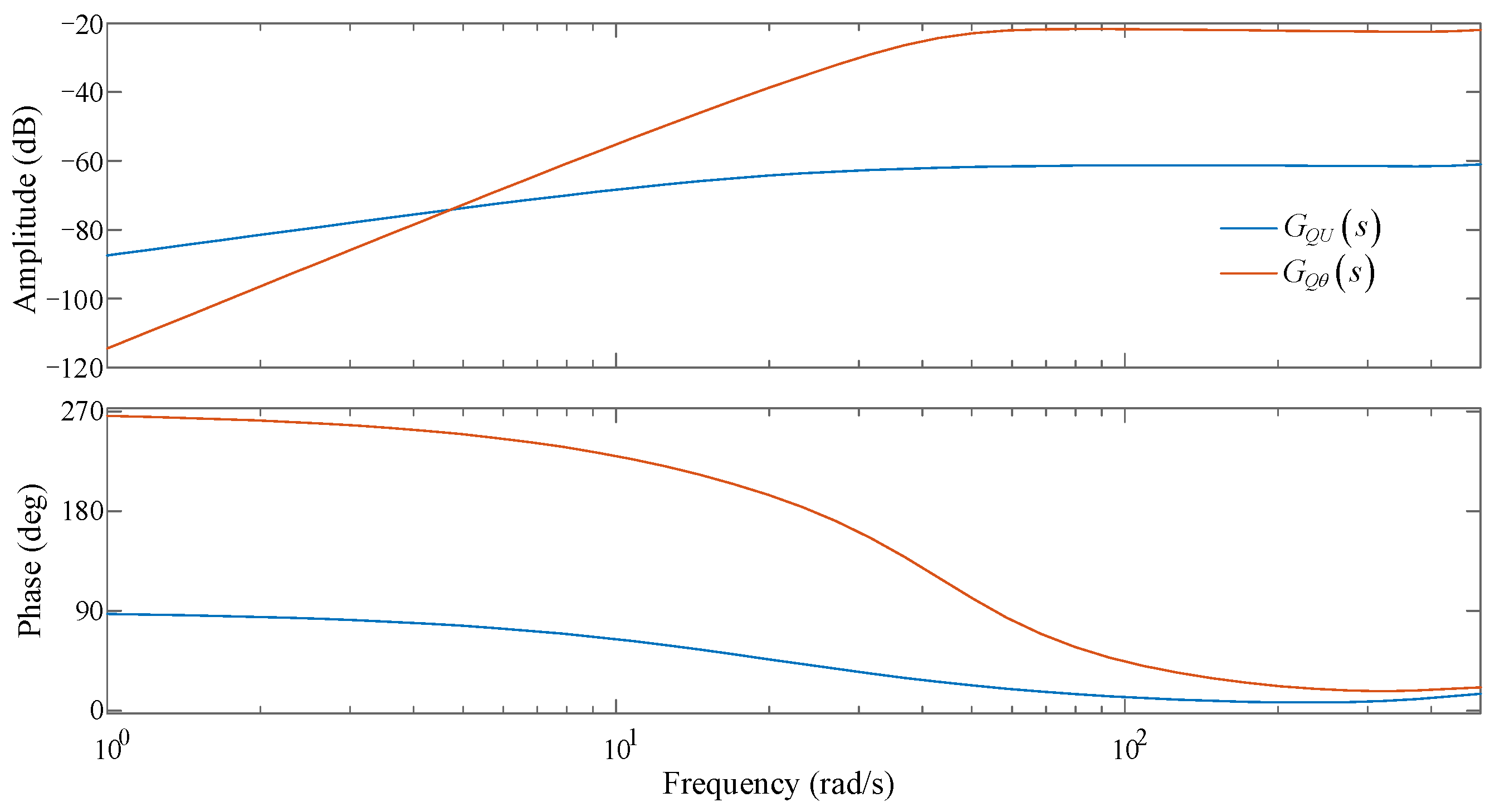

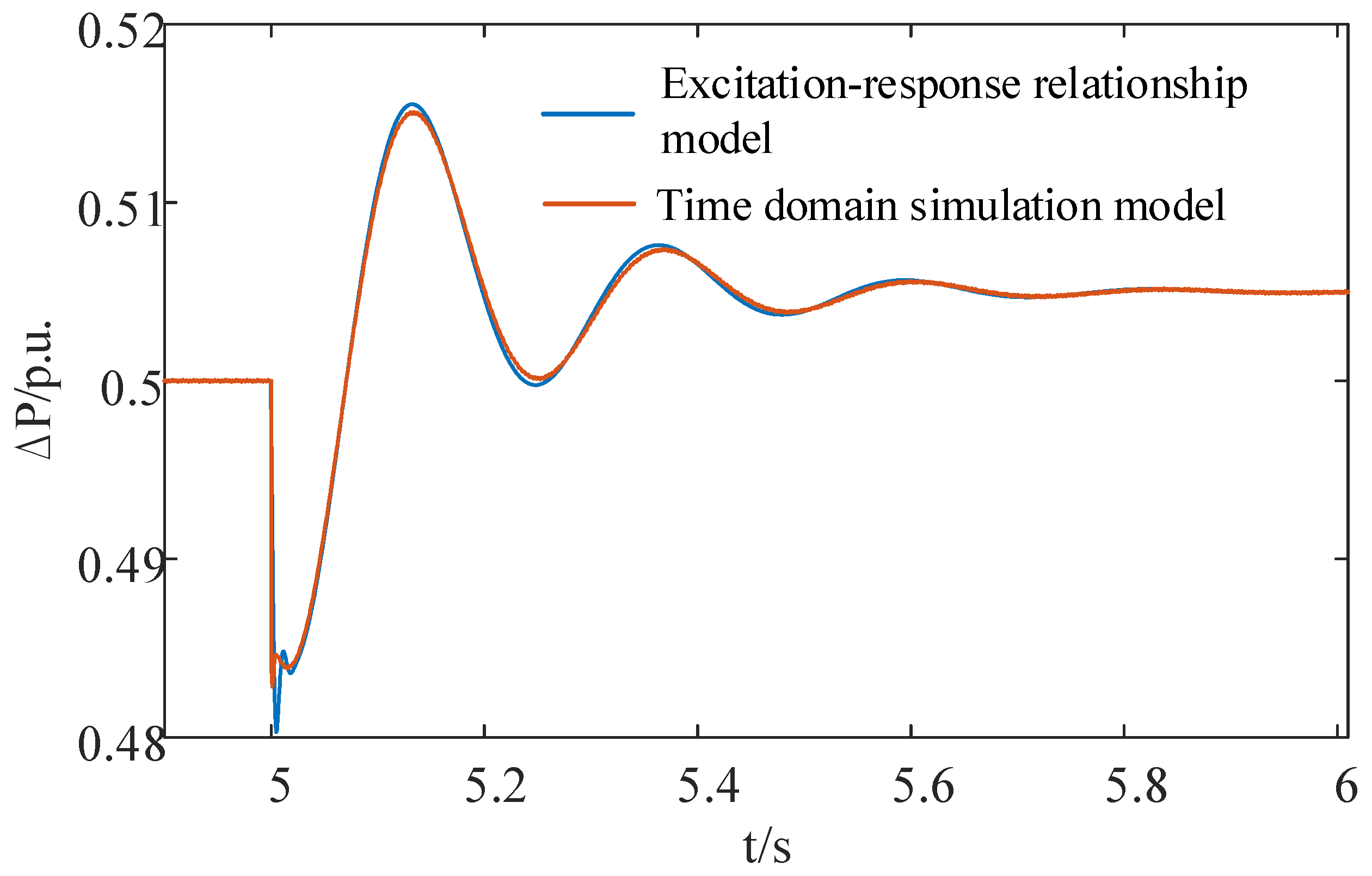
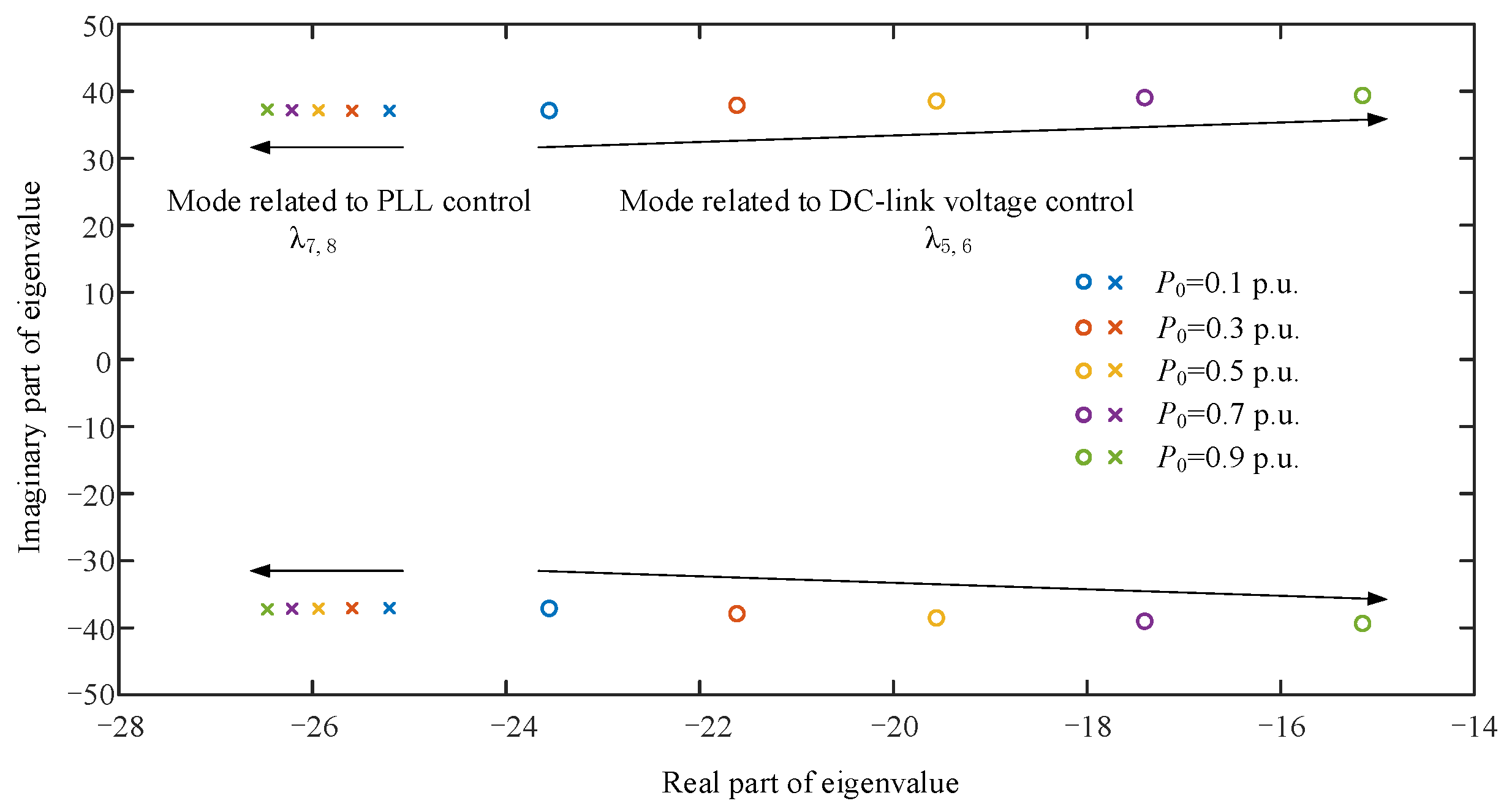


| Operating Conditions P0 (Iin0) | 0.1 p.u. | 0.5 p.u. | 0.9 p.u. |
|---|---|---|---|
| Eigenvalues of the state-space equation model | |||
| λ1,2 | −213.6 ± j 456.7 | −213.8 ± j 456.2 | −214.3 ± j 455.6 |
| λ3,4 | −290.5 ± j 486.9 | −290.5 ± j 486.9 | −290.4 ± j 487.0 |
| λ5,6 | −23.56 ± j 37.11 | −19.56 ± j 38.52 | −15.16 ± j 39.40 |
| λ7,8 | −25.20 ± j 37.07 | −25.94 ± j 37.14 | −26.43 ± j 37.21 |
| λ9 | −20.86 | −20.98 | −21.22 |
| Eigenvalues of small-signal model based on excitation–response relationship | |||
| λ1,2 | −213.9 ± j 456.5 | −214.2 ± j 456.1 | −214.5 ± j 455.6 |
| λ3,4 | −290.1 ± j 486.9 | −290.1 ± j 486.9 | −290.1 ± j 487.0 |
| λ5,6 | −23.58 ± j 37.02 | −19.57 ± j 38.34 | −15.18 ± j 39.31 |
| λ7,8 | −25.19 ± j 37.05 | −25.92 ± j 37.11 | −26.41 ± j 37.20 |
| λ9 | −20.52 | −20.65 | −21.03 |
| Detailed Model | Excitation-Response Relationship Model | Capacity Weighted Model | |
|---|---|---|---|
| Dominant eigenvalue | −6.38 ± j 27.748 | −6.46 ± j 27.753 | −6.02 ± j 26.881 |
| Frequency error | \(4.416 Hz) | 0.02% (4.417 Hz) | −3.12% (4.278 Hz) |
| Damping ratio error | \(0.224) | 1.28% (0.227) | −2.41% (0.218) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, G.; Wang, Y.; Fan, Y.; Yang, C.; Yue, L. Aggregation Equivalence Method for Direct-Drive Wind Farms Based on the Excitation–Response Relationship. Electronics 2024, 13, 2124. https://doi.org/10.3390/electronics13112124
Yan G, Wang Y, Fan Y, Yang C, Yue L. Aggregation Equivalence Method for Direct-Drive Wind Farms Based on the Excitation–Response Relationship. Electronics. 2024; 13(11):2124. https://doi.org/10.3390/electronics13112124
Chicago/Turabian StyleYan, Gangui, Yupeng Wang, Yuxing Fan, Cheng Yang, and Lin Yue. 2024. "Aggregation Equivalence Method for Direct-Drive Wind Farms Based on the Excitation–Response Relationship" Electronics 13, no. 11: 2124. https://doi.org/10.3390/electronics13112124
APA StyleYan, G., Wang, Y., Fan, Y., Yang, C., & Yue, L. (2024). Aggregation Equivalence Method for Direct-Drive Wind Farms Based on the Excitation–Response Relationship. Electronics, 13(11), 2124. https://doi.org/10.3390/electronics13112124





