Signal Classification Algorithms over Time Selective Channels
Abstract
:1. Introduction
2. Observation Model
3. Proposed Framework
- The value should be provided as a priori information to determine the upper bound on the number of processed samples per classifier, and then determine the appropriate length of each block. To this end, we assume that the receiver is equipped with a speed meter to measure the relative velocity between the transmitter and receiver, v. Hence, an estimate of is computed through the simple expression of , where is the transmission wavelength.
- We assume that the receiver has a rough estimation of the received signal bandwidth. Therefore, can be computed by using . The aforementioned assumptions can be easily carried out in practice.
4. STBCs Classification
4.1. Preliminaries
4.2. Proposed Classification Algorithm over Time Selective Channels
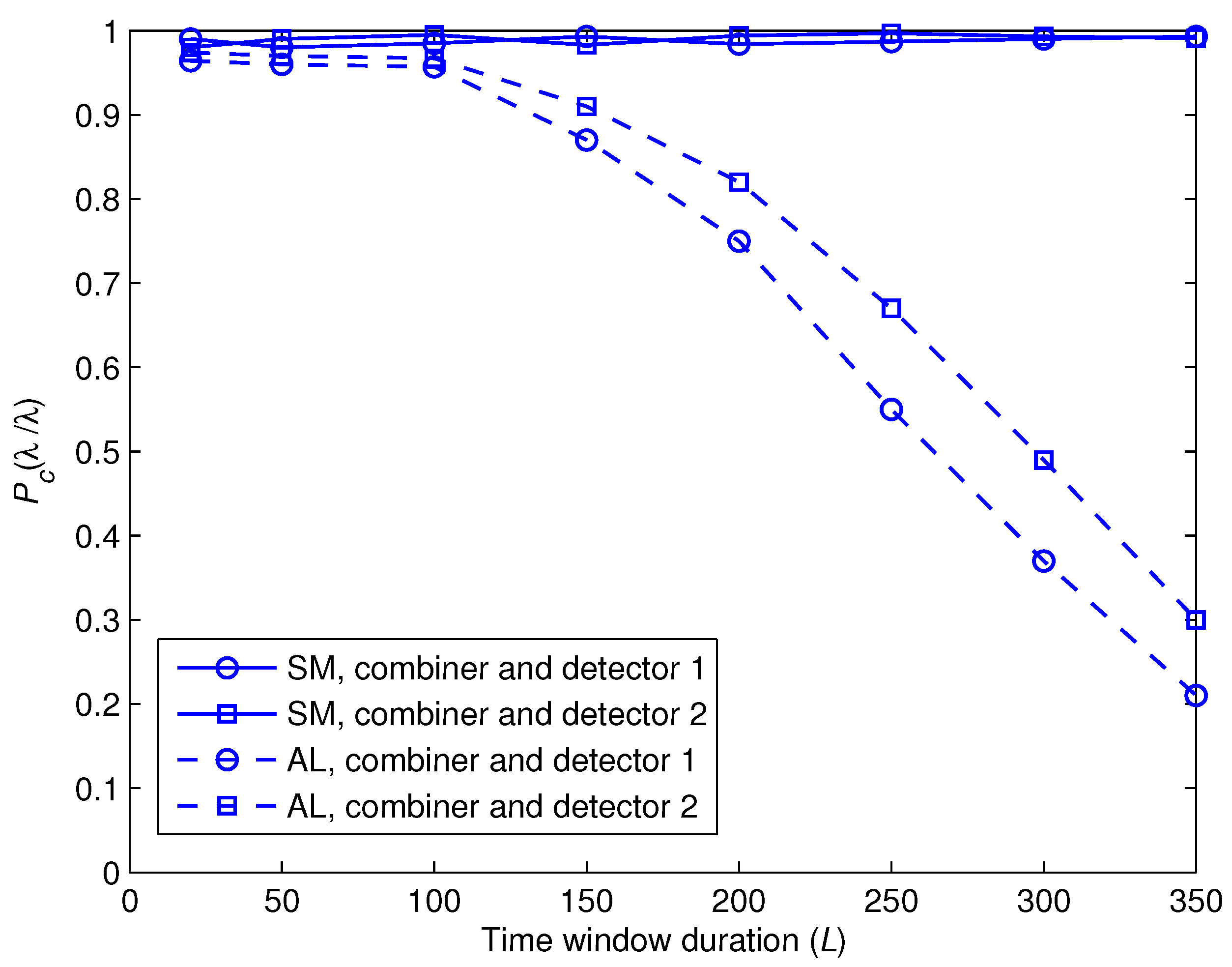
- Combiner and Detector 1
- Combiner and Detector 2
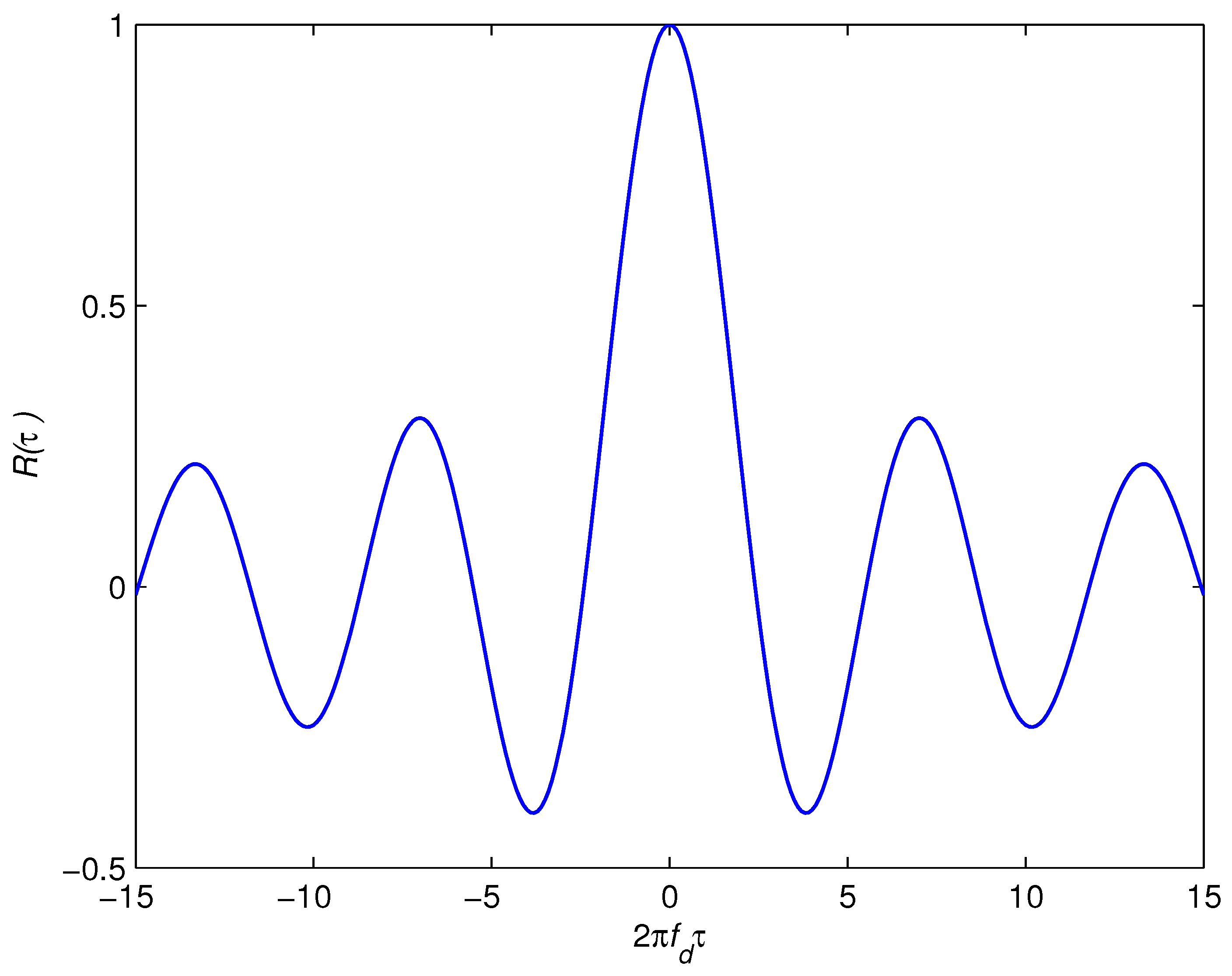
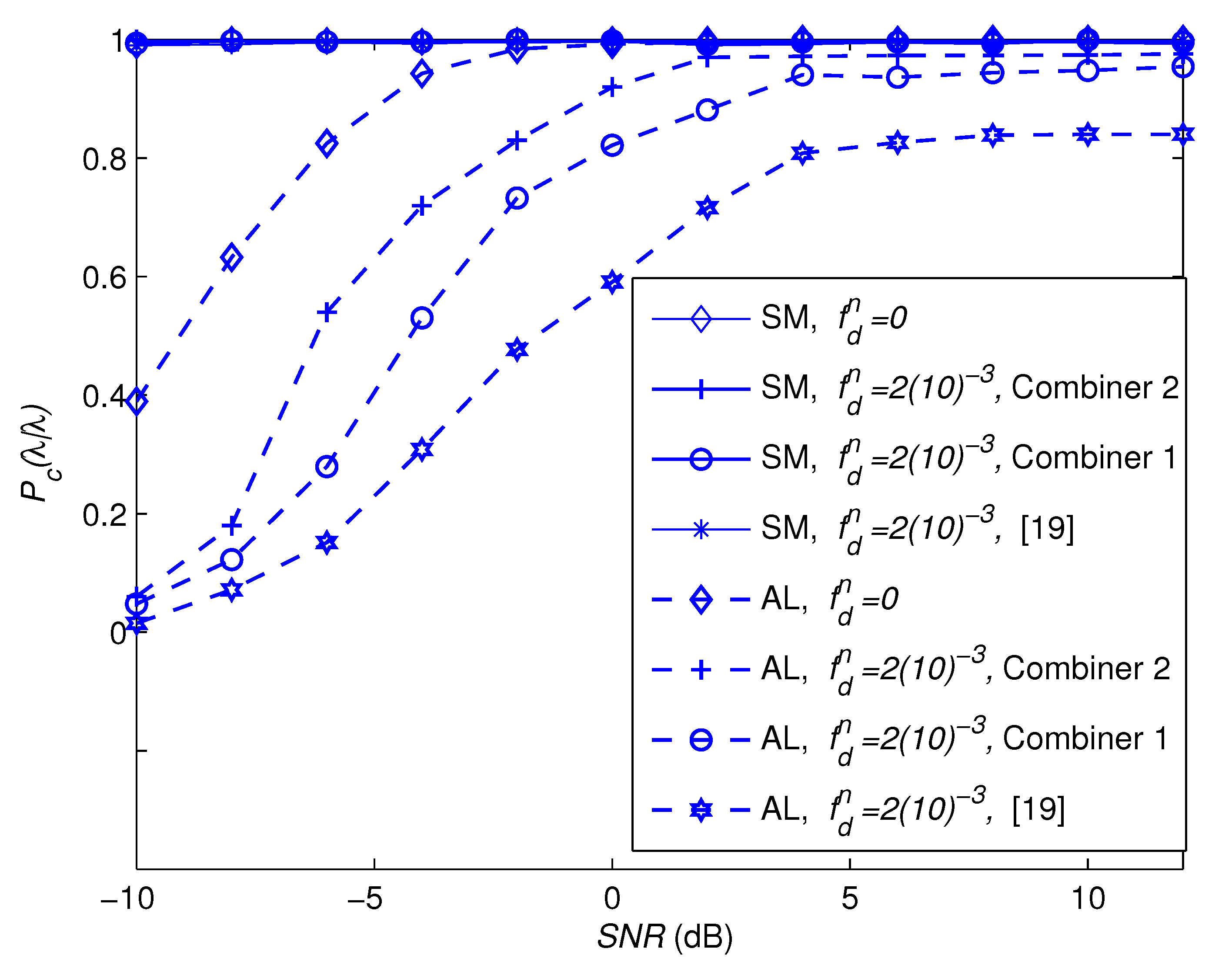
5. Simulation Results
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dobre, O.A. Signal Identification for Emerging Intelligent Radios: Classical Problems and New Challenges. IEEE Instrum. Meas. Mag. 2015, 18, 11–18. [Google Scholar] [CrossRef]
- Dobre, O.A.; Abdi, A.; Bar-Ness, Y.; Su, W. A survey of automatic modulation classification techniques: Classical approaches and new developments. IET Commun. 2007, 1, 137–156. [Google Scholar] [CrossRef] [Green Version]
- Chu, P.; Xie, L.; Dai, C.; Chen, Y. Automatic Modulation Recognition for Secondary Modulated Signals. IEEE Wirel. Commun. Lett. 2021, 10, 962–965. [Google Scholar] [CrossRef]
- Li, Y.; Wang, B.; Shao, G.; Shao, S. Automatic Modulation Classification for Short Burst Underwater Acoustic Communication Signals Based on Hybrid Neural Networks. IEEE Access 2020, 8, 227793–227809. [Google Scholar] [CrossRef]
- Zang, K.; Ma, Z. Automatic Modulation Classification Based on Hierarchical Recurrent Neural Networks with Grouped Auxiliary Memory. IEEE Access 2020, 8, 213052–213061. [Google Scholar] [CrossRef]
- Choqueuse, V.; Marazin, M.; Collin, L.; Yao, K.C.; Burel, G. Blind Recognition of Linear Space–Time Block Codes: A Likelihood-Based Approach. IEEE Trans. Signal Process. 2010, 58, 1290–1299. [Google Scholar] [CrossRef]
- Choqueuse, V.; Yao, K.; Collin, L.; Burel, G. Hierarchical Space-Time Block Code Recognition Using Correlation Matrices. IEEE Trans. Wirel. Commun. 2008, 7, 3526–3534. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.; Zhang, L.; Zhong, Z.; Liu, R. Blind Recognition of LDPC Codes Over Candidate Set. IEEE Commun. Lett. 2020, 24, 11–14. [Google Scholar] [CrossRef]
- Wu, Z.; Zhang, L.; Zhong, Z. A Maximum Cosinoidal Cost Function Method for Parameter Estimation of RSC Turbo Codes. IEEE Commun. Lett. 2019, 23, 390–393. [Google Scholar] [CrossRef]
- Haring, L.; Chen, Y.; Czylwik, A. Automatic Modulation Classification Methods for Wireless OFDM Systems in TDD Mode. IEEE Trans. Commun. 2010, 58, 2480–2485. [Google Scholar] [CrossRef]
- Su, W. Feature Space Analysis of Modulation Classification Using Very High-Order Statistics. IEEE Commun. Lett. 2013, 17, 1688–1691. [Google Scholar] [CrossRef]
- Liao, K.; Zhao, Y.; Gu, J.; Zhang, Y.; Zhong, Y. Sequential Convolutional Recurrent Neural Networks for Fast Automatic Modulation Classification. IEEE Access 2021, 9, 27182–27188. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, M.; Yang, J.; Sun, W. A Data Preprocessing Method for Automatic Modulation Classification Based on CNN. IEEE Commun. Lett. 2021, 25, 1206–1210. [Google Scholar] [CrossRef]
- Zhou, Y.; Lin, T.; Zhu, Y. Automatic Modulation Classification in Time-Varying Channels Based on Deep Learning. IEEE Access 2020, 8, 197508–197522. [Google Scholar] [CrossRef]
- Hassan, K.; Dayoub, I.; Hamouda, W.; Nzeza, C.; Berbineau, M. Blind Digital Modulation Identification for Spatially Correlated MIMO Systems. IEEE Trans. Wirel. Commun. 2012, 91, 683–693. [Google Scholar] [CrossRef]
- Proakis, J. Digital Communications, 4th ed.; McGraw-Hill: New York, NY, USA, 2000. [Google Scholar]
- Marey, M.; Dobre, O.A.; Inkol, R. Blind STBC Identification for Multiple Antenna OFDM Systems. IEEE Trans. Commun. 2014, 62, 1554–1567. [Google Scholar] [CrossRef]
- Marey, M.; Dobre, O.A.; Inkol, R. Classification of Space-time Block Codes Based on Second-order Cyclostationarity with Transmission Impairments. IEEE Trans. Wirel. Commun. 2012, 11, 2574–2584. [Google Scholar] [CrossRef]
- Marey, M.; Dobre, O.A.; Liao, B. Classification of STBC Systems over Frequency-selective Channels. IEEE Trans. Veh. Technol. 2015, 64, 2159–2164. [Google Scholar] [CrossRef]
- Ciuonzo, D.; De Maio, A.; Rossi, P.S. A Systematic Framework for Composite Hypothesis Testing of Independent Bernoulli Trials. IEEE Signal Process. Lett. 2015, 22, 1249–1253. [Google Scholar] [CrossRef]
- Goel, A.; Patel, A.; Nagananda, K.G.; Varshney, P.K. Robustness of the Counting Rule for Distributed Detection in Wireless Sensor Networks. IEEE Signal Process. Lett. 2018, 25, 1191–1195. [Google Scholar] [CrossRef]
- Aceto, G.; Ciuonzo, D.; Montieri, A.; Pescape, A. Multi-classification Approaches for Classifying Mobile App Traffic. J. Netw. Comput. Appl. 2018, 103, 131–145. [Google Scholar] [CrossRef]
- Zheng, Y.; Xiao, C. Simulation Models with Correct Statistical Properties for Rayleigh Fading Channels. IEEE Trans. Commun. 2003, 51, 920–928. [Google Scholar] [CrossRef]
- Kim, I.-L. Exact BER Analysis of OSTBCs in Spatially Correlated MIMO Channels. IEEE Trans. Commun. 2006, 54, 1365–1373. [Google Scholar]




| Parameter | Value |
|---|---|
| modulation | QPSK |
| U | 2000 |
| L | 100 |
| M | 20 |
| 1 | |
| 10 | |
| 85 | |
| 2 | |
| 2 | |
| No. of trials | 1000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marey, M.; Mostafa, H. Signal Classification Algorithms over Time Selective Channels. Electronics 2021, 10, 1714. https://doi.org/10.3390/electronics10141714
Marey M, Mostafa H. Signal Classification Algorithms over Time Selective Channels. Electronics. 2021; 10(14):1714. https://doi.org/10.3390/electronics10141714
Chicago/Turabian StyleMarey, Mohamed, and Hala Mostafa. 2021. "Signal Classification Algorithms over Time Selective Channels" Electronics 10, no. 14: 1714. https://doi.org/10.3390/electronics10141714
APA StyleMarey, M., & Mostafa, H. (2021). Signal Classification Algorithms over Time Selective Channels. Electronics, 10(14), 1714. https://doi.org/10.3390/electronics10141714






