Quantifying Risk Perception: The Entropy Decision Risk Model Utility (EDRM-U)
Abstract
1. Introduction
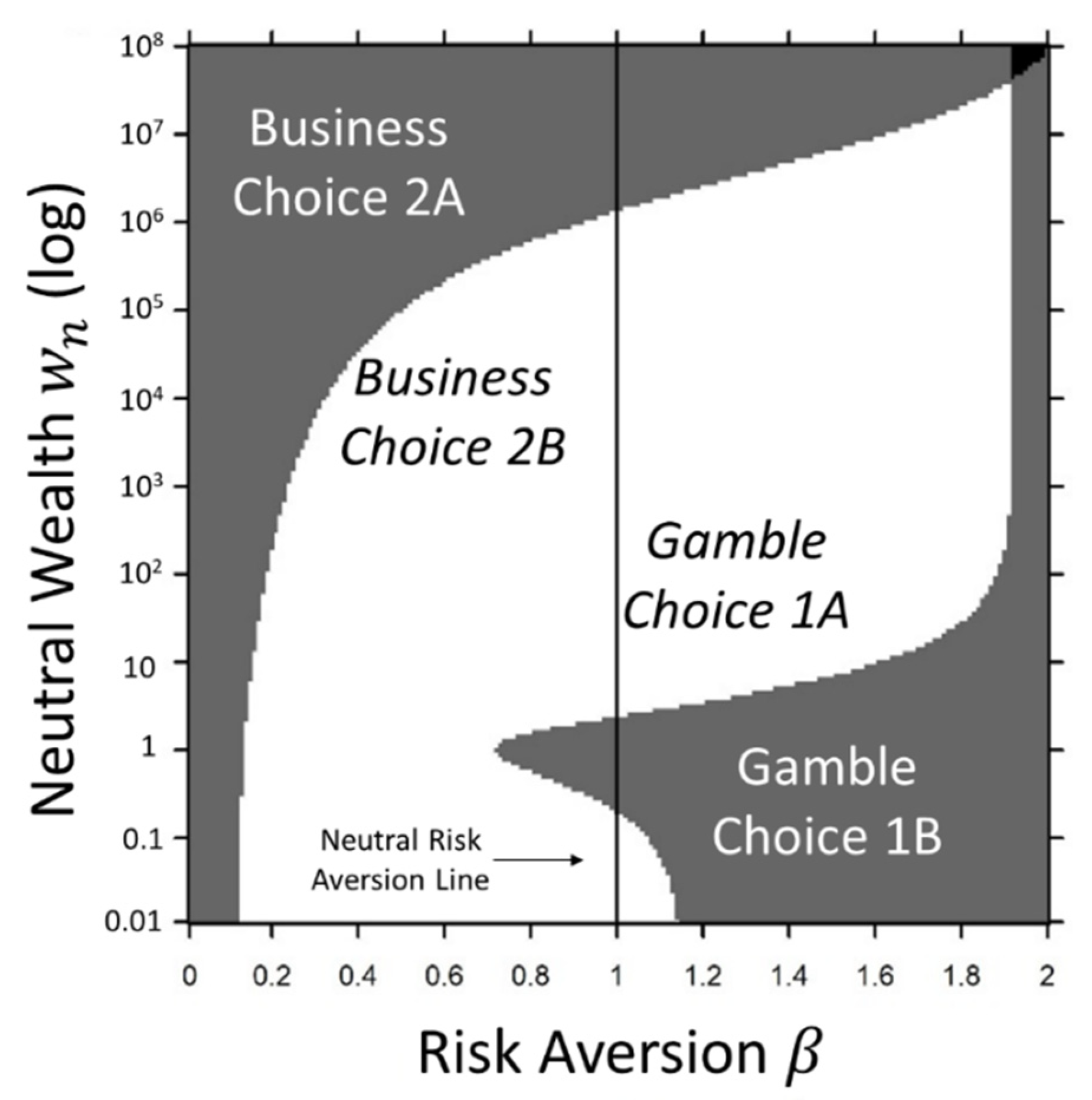
Practical Application Example (Notional)
- Part 1.
- Choice 1A: ($5, 0.5; −$1; −$1.11) (Selected) Choice 1B: (−$0.11).
- Part 2.
- Choice 2A: ($20M, 0.1; −$1M) Choice 2B: ($2M, 0.5; −$1M) (Selected).
2. Method
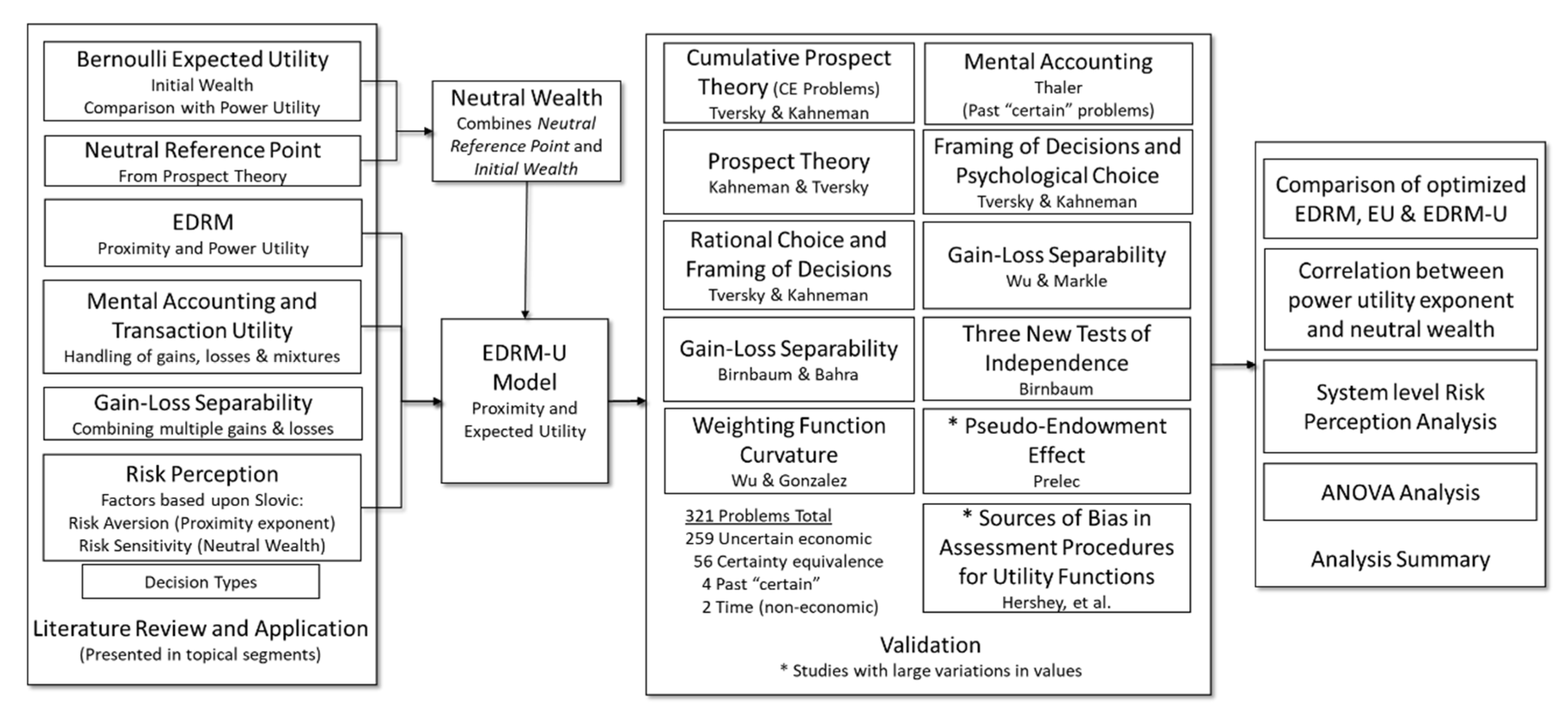
3. Literature Review and Application
3.1. Daniel Bernoulli’s Expected Utility
Comparison of Power Utility and Expected Utility
3.2. Neutral Reference Point
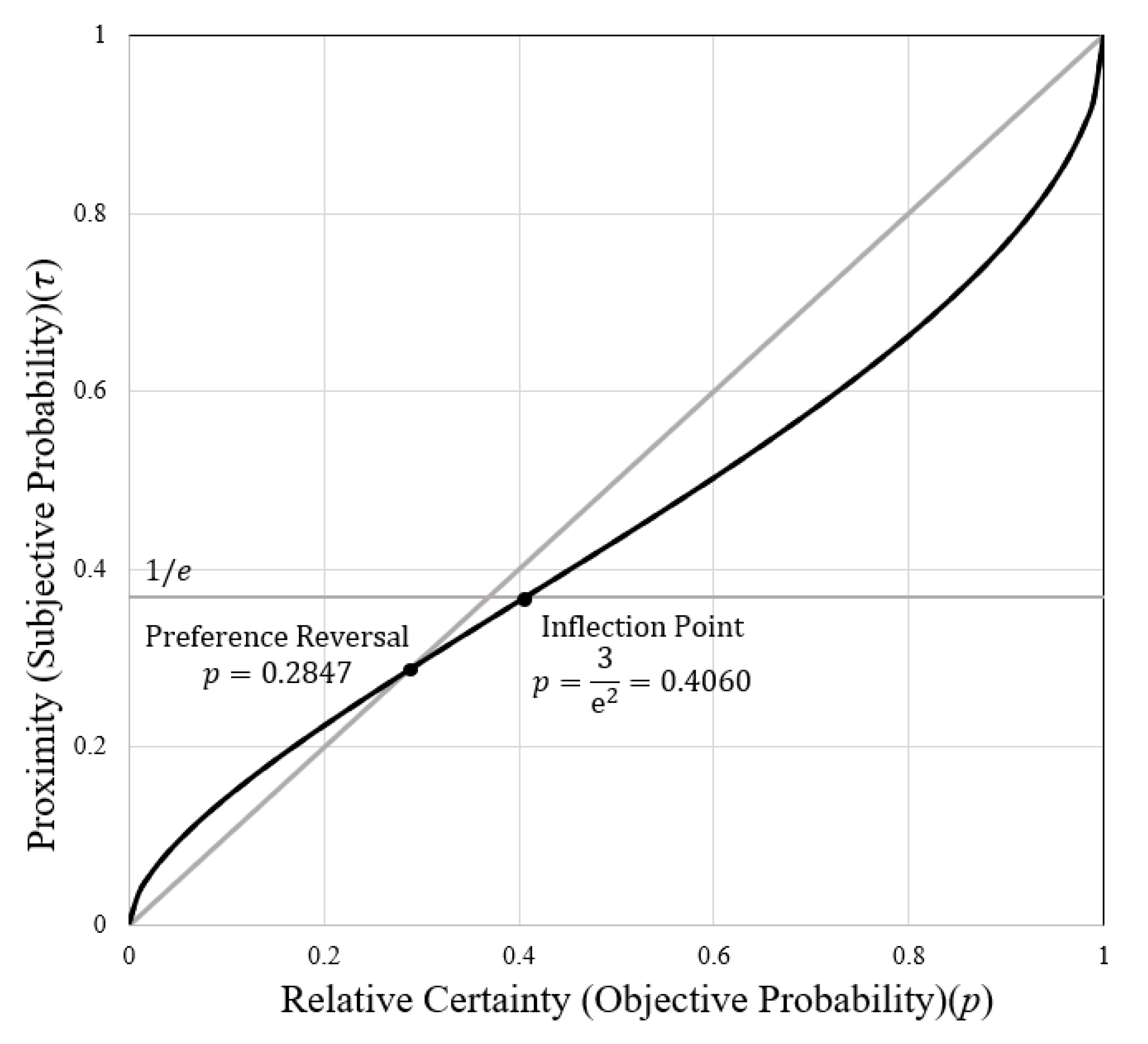
3.3. Entropy Decision Risk Model
- It accepts the use of the power utility ubiquitous to positive decision theory research to isolate the subjective-objective probability relationship, as shown in Equation (4);
- It assumes there is no difference in how the subjects valued gains or losses (i.e., that the value function exponent was constant for gains or losses and that no loss aversion was present).
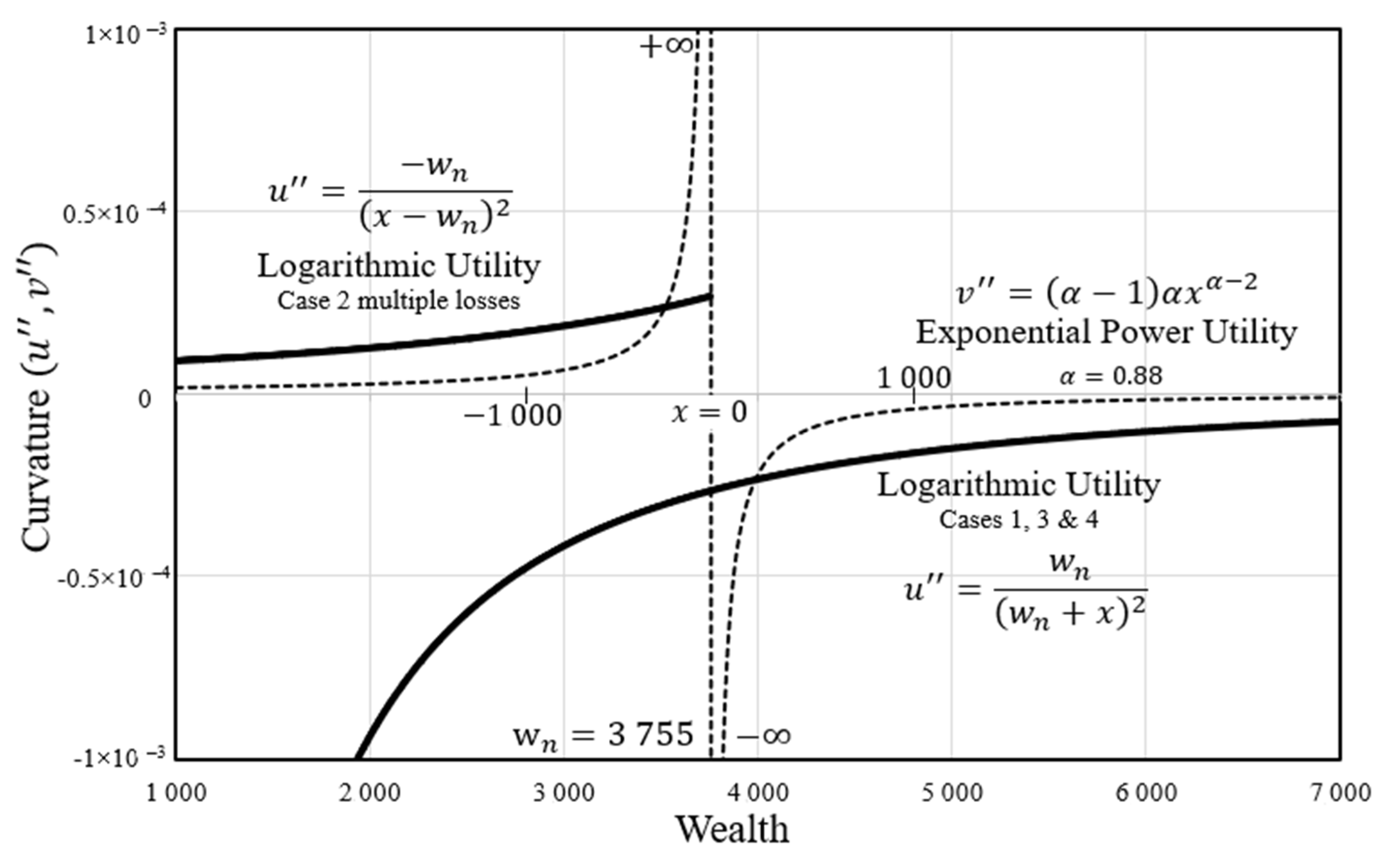
3.4. Mental Accounting and Transaction Utility
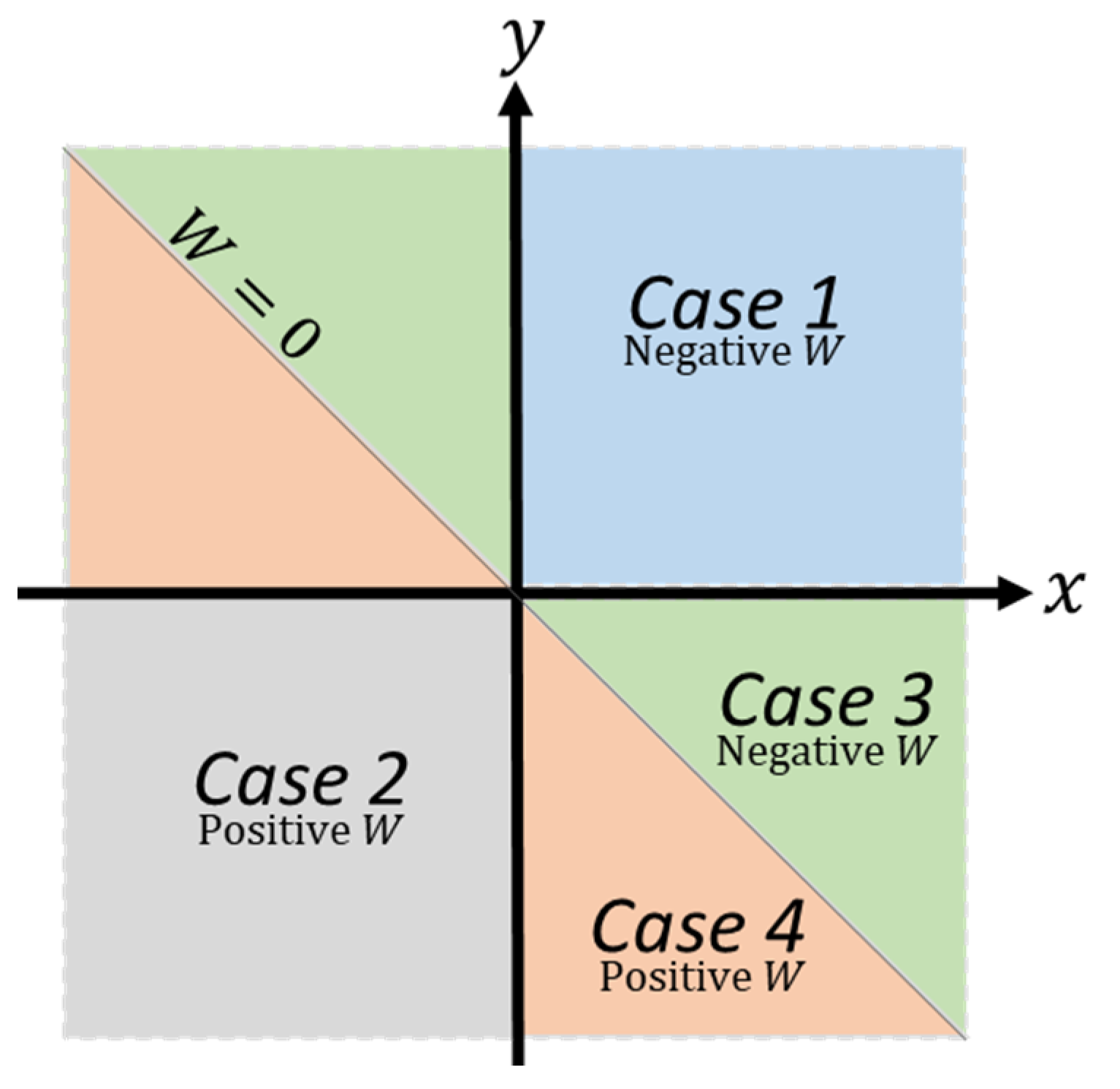
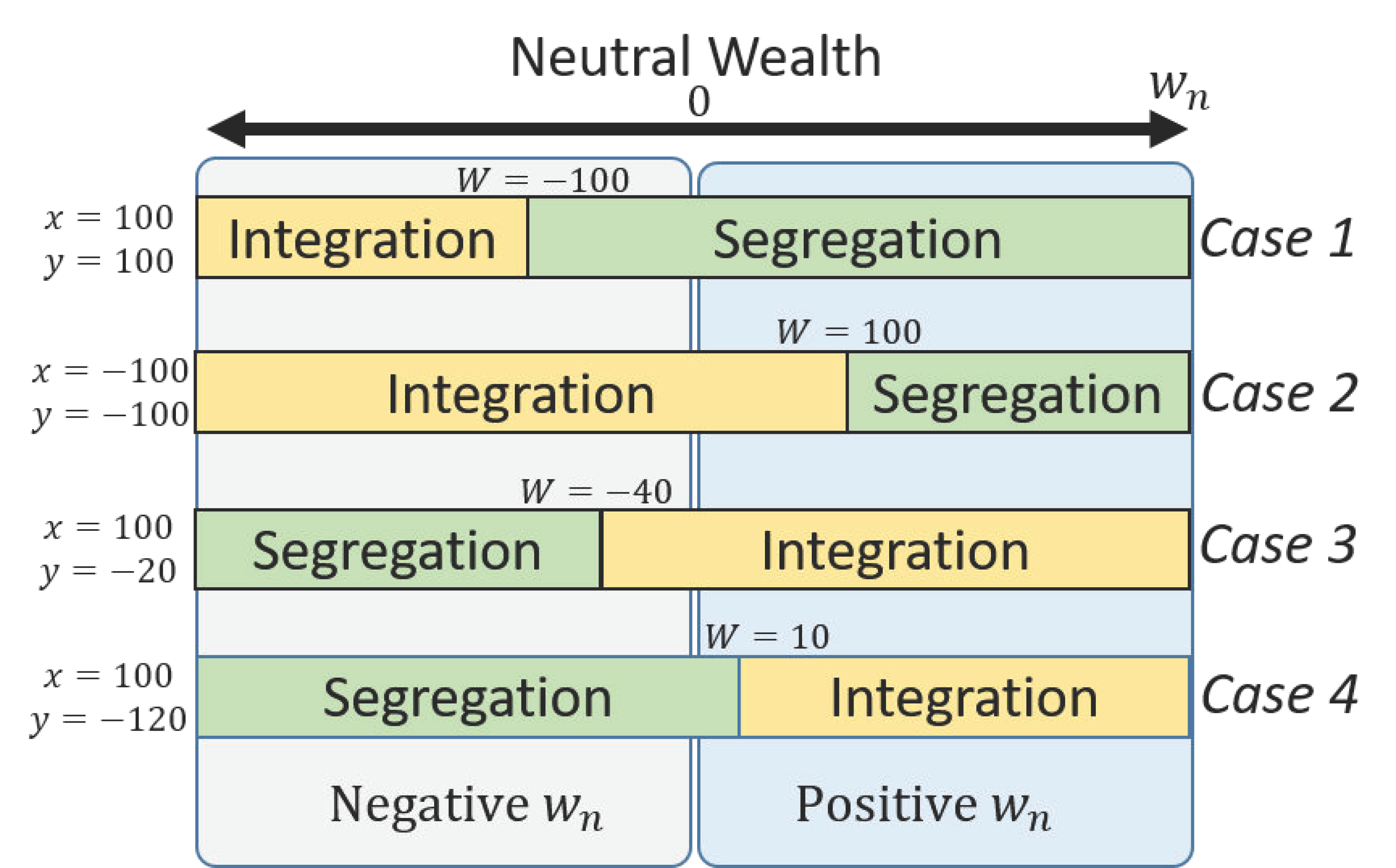
- Case 1
- Multiple gains (segregation): , which says that people prefer many smaller gains over a single larger gain (i.e., people like a greater number of smaller presents).
- Case 2
- Multiple losses (integration): ; affirms that subjects prefer grouped losses rather than separated ones (i.e., people prefer consolidated bills).
- Case 3
- Mixed gains (integration with cancellation): ; is always positive, but the losses more quickly cancel out gains due to loss aversion.
- Case 4
- Mixed losses (segregation or integration with cancellation): . Because is always negative and gains are felt less than commensurate losses, Thaler states that there is a point at which there is a shift between segregation and integration; the introduction of neutral wealth permits defining this shift point.
3.5. Gain-Loss Separability
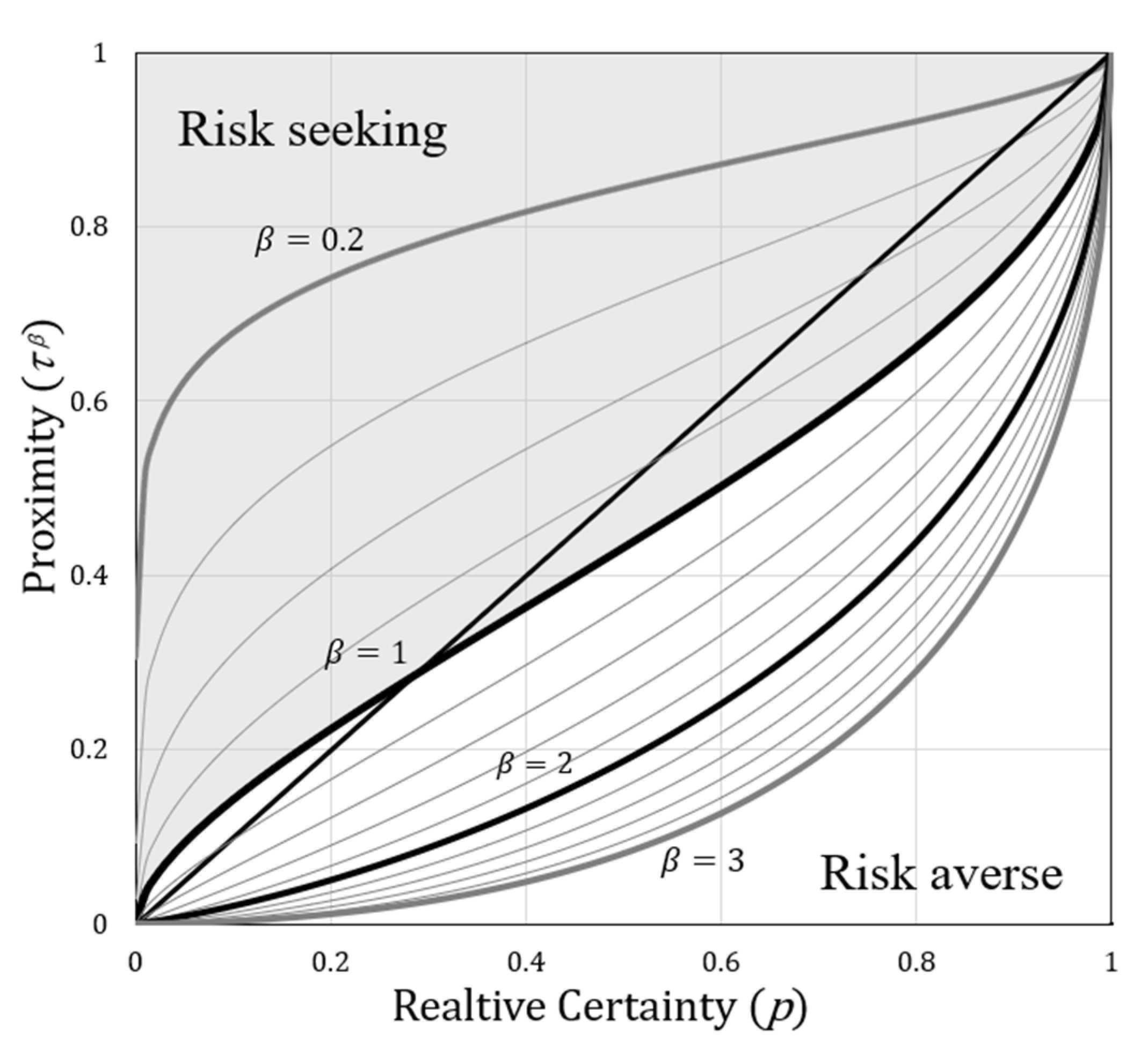
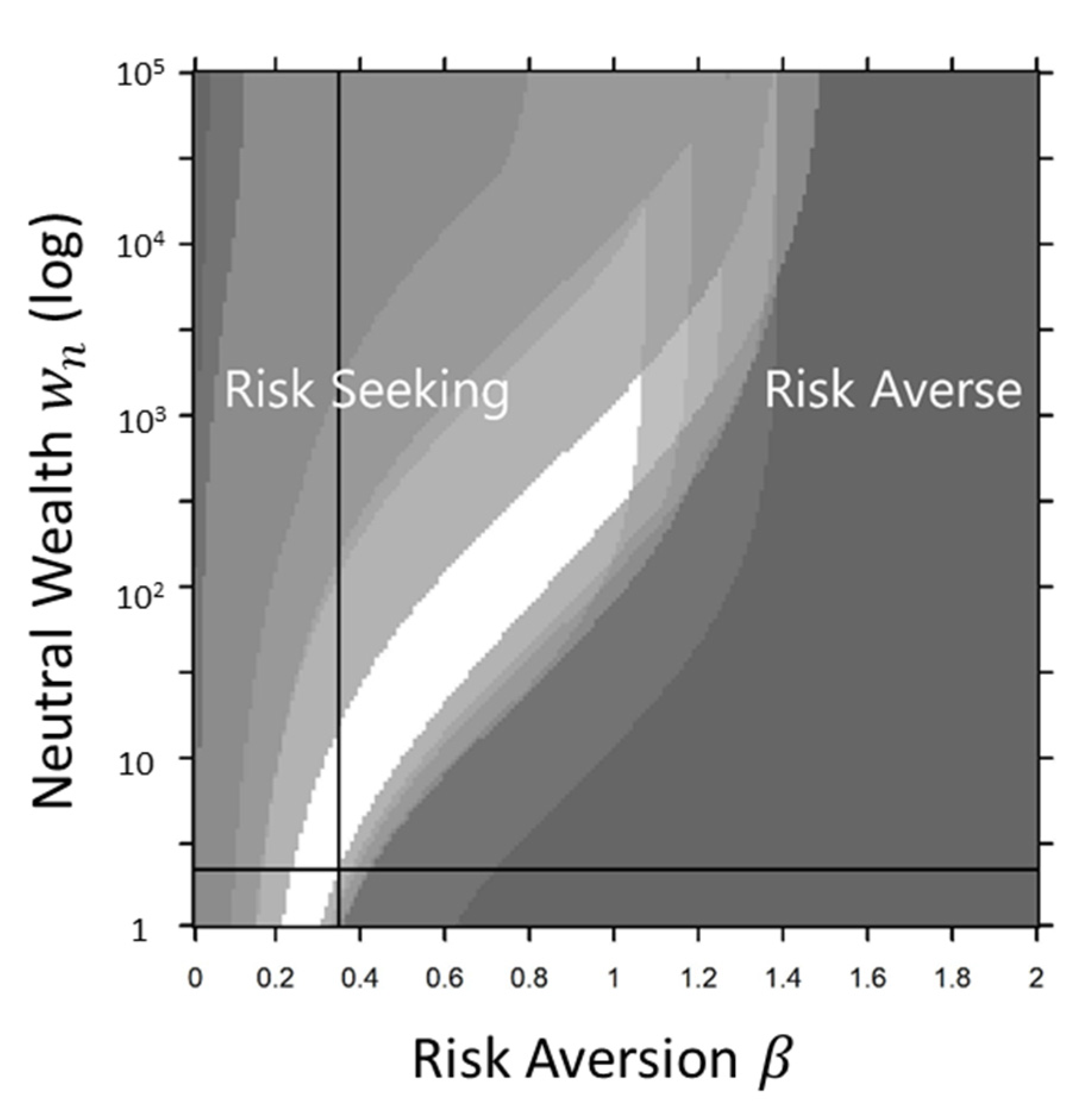
3.6. Risk Perception Measures: Risk Aversion and Risk Sensitivity
3.6.1. Risk Aversion (, Proximity Exponent)
3.6.2. Risk Aversion Evaluation and Introduction of Risk Sensitivity
4. EDRM-U Model
5. Validation
5.1. VNM Axiomatic Analysis
5.2. Probability Evaluation Model (PEM)
5.3. Cumulative Prospect Theory
5.4. Thaler Mental Accounting (Riskless, with no Uncertainty)
5.5. Prospect Theory
5.6. Framing of Decisions and Psychology of Choice
5.7. Rational Choice and the Framing of Decisions
- Survival Frame Choice A states: 68% survive to 1 year, 34% survive to 5 years, 90% survive surgery.
- Mortality Frame Choice A states: 32% die within 1 year, 66% die within 5 years, 10% die from surgery.
5.8. Wu and Markle Gain-Loss Separability
5.8.1. Gains Only
5.8.2. Losses Only
5.8.3. Mixed
5.9. Birnbaum and Bahra Gain Loss Separability
5.10. Birnbaum: Three New Tests of Independence That Differentiate Models of Risky Decision Making
- Safe: (100, 0.8; 44, 0.1; 40, 0.1) Risky: (100, 0.8; 96; 0.1; 4, 0.1).
5.11. Wu and Gonzalez Weighting Function Curvature
5.12. Prelec: A “Pseudo-Endowment” Effect, and Its Implications for Some Recent Nonexpected Utility Models
5.13. Hershey et al. Sources of Bias in Assessment Procedures for Utility Functions
5.13.1. Hershey et al. Loss Problems (Experiment 1)
5.13.2. Hershey et al. Large Value Gain Problems (Experiment 2)
6. Summary of Analyses
6.1. EDRM-U Comparison with EDRM and EU Using Averaged Test Results
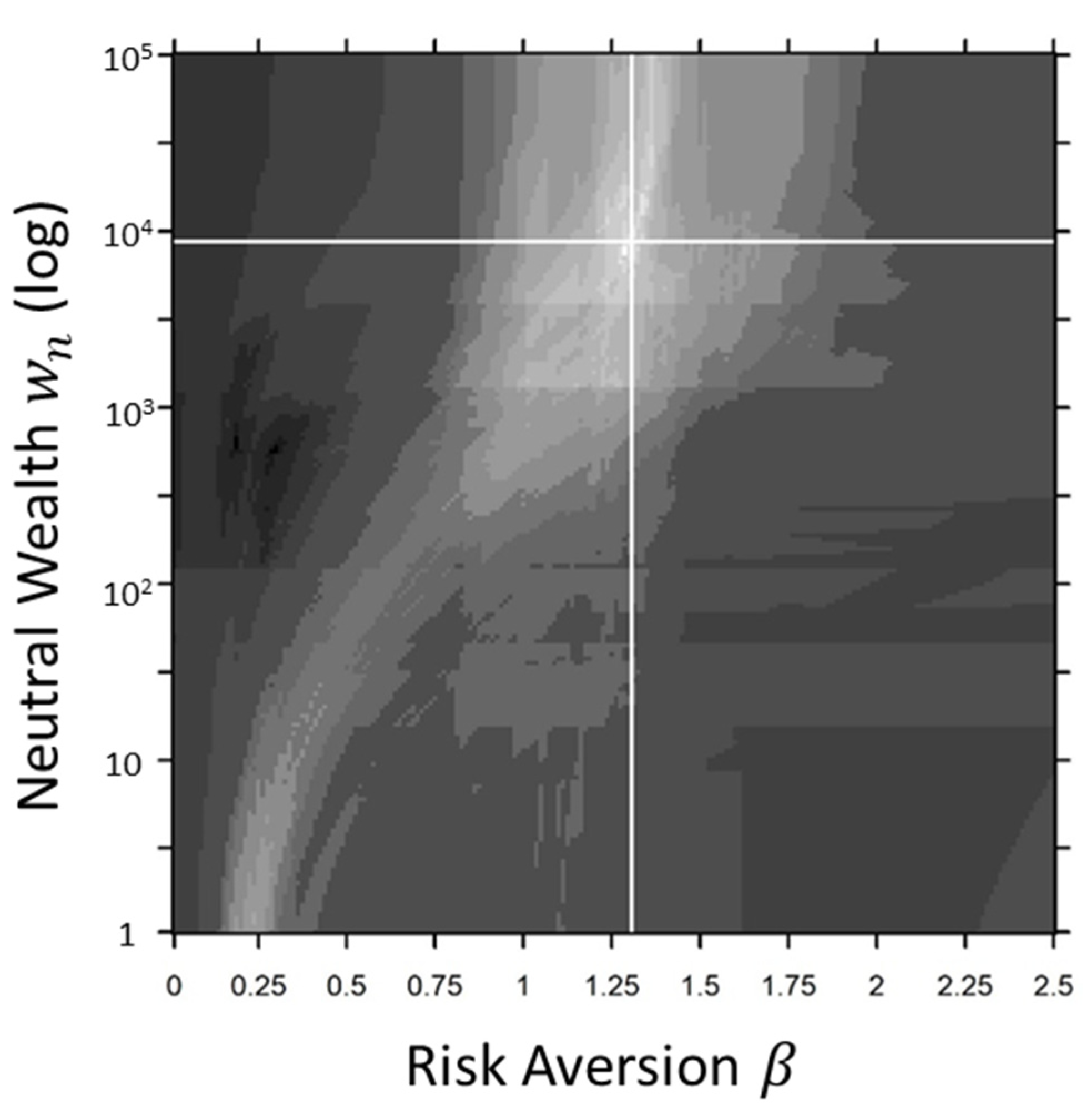
6.2. Correlation between Power Utility Exponent and Neutral Wealth
6.3. Risk Perception (EDRM versus EDRM-U)
6.4. ANOVA Analysis
7. Discussion
- Choices with large ranges of values;
- Choices involving mixtures of gains and losses;
- Treatment of risk aversion, which includes loss aversion.
Author Contributions
Funding
Conflicts of Interest
References
- Monroe, T.J.; Beruvides, M.G.; Tercero-Gómez, V.G. Derivation and application of the subjective-objective probability relationship from entropy: The Entropy Decision Risk Model (EDRM). Systems 2020, 8, 46. [Google Scholar] [CrossRef]
- Sinn, H.-W. Weber’s law and the biological evolution of risk preferences: The selective dominance of the logarithmic utility function, 2002 geneva risk lecture. Geneva Pap. Risk Insur. Theory 2003, 28, 87. [Google Scholar] [CrossRef]
- Allais, M. Le comportement de l’homme rationnel devant le risque: Critique des postulats et axiomes de l’ecole Americaine. Econometrica 1953, 21, 503–546. [Google Scholar] [CrossRef]
- Bentham, J. An Introduction to the Principles of Morals and Legislation; T. Payne and son: London, UK, 1789. [Google Scholar]
- Ellsberg, D. Risk, Ambiguity, and the Savage Axioms. Q. J. Econ. 1961, 75, 643–669. [Google Scholar] [CrossRef]
- Tapiero, C.S. Risk and Financial Management; John Wiley and Sons Ltd.: West Sussex, UK, 2004. [Google Scholar]
- Schumpeter, J.A. History of Economic Analysis; Oxford University Press: New York, NY, USA, 1954. [Google Scholar]
- Kahneman, D. Thinking, Fast and Slow, 1st ed.; Farrar, Straus and Giroux: New York, NY, USA, 2011. [Google Scholar]
- Bernoulli, D. Exposition of a new theory on the measurement of risk (1738). Econometrica 1954, 22, 23–36. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect theory—Analysis of decision under risk. Econometrica 1979, 47, 263–291. [Google Scholar] [CrossRef]
- Markowitz, H. The utility of wealth. J. Polit. Econ. 1952, 60, 151–158. [Google Scholar] [CrossRef]
- Thaler, R. Mental accounting and consumer choice. Mark. Sci. 1985, 4, 199–214. [Google Scholar] [CrossRef]
- Kahneman, D.; Knetsch, J.L.; Thaler, R.H. Experimental tests of the endowment effect and the coase theorem. J. Polit. Econ. 1990, 98, 1325–1348. [Google Scholar] [CrossRef]
- Hershey, J.C.; Kunreuther, H.C.; Schoemaker, P.J.H. Sources of bias in assessment procedures for utility functions. Manag. Sci. 1982, 28, 936. [Google Scholar] [CrossRef]
- Shannon, C.E.; Weaver, W. The Mathematical Theory of Communication; The Univeristy of Illinois Press: Urbana, IL, USA, 1949. [Google Scholar]
- Pisano, R.; Sozzo, S. A unified theory of human judgements and decision-making under uncertainty. Entropy 2020, 22, 738. [Google Scholar] [CrossRef]
- Ben-Naim, A. Entropy and information theory: Uses and misuses. Entropy 2019, 21, 1170. [Google Scholar] [CrossRef]
- Jaynes, E.T. Information theory and statistical mechanics. Phys. Rev. 1957, 106, 620–630. [Google Scholar] [CrossRef]
- Monroe, T.J.; Beruvides, M.G. Translating risk perception for standard risk analyses. In IISE Annual Conference; Cromarty, L., Shirwaiker, R., Wang, P., Eds.; Norcross, GA, USA, 2020; Available online: https://www.iise.org/Annual/details.aspx?id=6790 (accessed on 20 November 2020).
- Kullback, S. Information Theory and Statistics; Wiley: New York, NY, USA, 1959. [Google Scholar]
- Monroe, T.J. Entropy Decision Risk Model (Accepted Dissertation Proposal); Beruvides, M.G., Patterson, P.E., Smith, M.L., Tercero-Gómez, V.G., Eds.; Texas Tech University: Lubbock, TX, USA, 2019. [Google Scholar]
- Tversky, A.; Kahneman, D. Advances in prospect-theory—Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar] [CrossRef]
- Frigg, R. Probability in boltzmannian statistical mechanics. In Time, Chance and Reduction. Philosophical Aspects of Statistical Mechanics; Gerhard Ernst, G., Hüttemann, A., Eds.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Keynes, J.M. A Treatise on Probability; Macmillan and co., Limited: London, UK, 1921. [Google Scholar]
- Birnbaum, M.H.; Bahra, J.P. Gain-loss separability and coalescing in risky decision making. Manag. Sci. 2007, 53, 1016–1028. [Google Scholar] [CrossRef][Green Version]
- Sjöberg, L. The methodology of risk perception research. Qual. Quant. 2000, 34, 407–418. [Google Scholar] [CrossRef]
- Thompson, W. Electrical units of measure. Pop. Lect. Addresses 1889, I, 73–136. [Google Scholar]
- Perrow, C. Normal accident at three Mile Island. Society 1981, 18, 17–26. [Google Scholar] [CrossRef]
- Perrow, C. Normal Accidents; Princeton University Press: Princeton, NJ, USA, 1999. [Google Scholar]
- Rasmussen, N. The application of probabilistic risk assessment techniques to energy technologies. Annu. Rev. Energy 1981, 6, 123–138. [Google Scholar] [CrossRef]
- Slovic, P. Perception of risk. Science (Am. Assoc. Adv. Sci.) 1987, 236, 280–285. [Google Scholar] [CrossRef]
- Sharp, M.E.; Viswanathan, J.; Lanyon, L.J.; Barton, J.J.S. Sensitivity and bias in decision-making under risk: Evaluating the perception of reward, its probability and value. PLoS ONE 2012, 7, e33460. [Google Scholar] [CrossRef]
- Weber, E.U.; Blais, A.-R.; Betz, N.E. A domain-specific risk-attitude scale: Measuring risk perceptions and risk behaviors. J. Behav. Decis. Mak. 2002, 15, 263–290. [Google Scholar] [CrossRef]
- Man, S.S.; Chan, A.H.S.; Alabdulkarim, S. Quantification of risk perception: Development and validation of the construction worker risk perception (CoWoRP) scale. J. Saf. Res. 2019, 71, 25–39. [Google Scholar] [CrossRef]
- Stanovich, K.E.; West, R.F. Individual differences in reasoning: Implications for the rationality debate? Behav. Brain Sci. 2000, 23, 645. [Google Scholar] [CrossRef]
- Sjoberg, L. Factors in risk perception. Risk Anal. 2000, 20, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Wakker, P. Separating marginal utility and probabilistic risk aversion. Theory Decis. 1994, 36, 1–44. [Google Scholar] [CrossRef]
- Wakker, P.P.; Zank, H. A simple preference foundation of cumulative prospect theory with power utility. Eur. Econ. Rev. 2002, 46, 1253–1271. [Google Scholar] [CrossRef]
- Falmagne, J.-C. Elements of Psychophysical Theory; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Tversky, A.; Kahneman, D. The framing of decisions and the psychology of choice. Science 1981, 211, 453. [Google Scholar] [CrossRef] [PubMed]
- Bertalanffy, L.V. General System Theory; George Braziller Inc.: New York, NY, USA, 1968. [Google Scholar]
- von Neumann, J.; Morgenstern, O. Theory of Games and Economic Behavior; Princeton University Press: Princeton, NJ, USA, 2007. [Google Scholar]
- Department of the Treasury. Treasury Reporting Rates of Exchange as of 31 March 1978; U.S. Government Printing Office: Washington, DC, USA, 1978.
- Tversky, A.; Kahneman, D. Rational choice and the framing of decisions. (proceedings from a conference held October 13–15, 1985, at the University of Chicago). J. Bus. 1986, 59, S251. [Google Scholar] [CrossRef]
- Schelling, T. Choice and Consequence; Harvard University Press: Cambridge, MA, USA, 1984. [Google Scholar]
- Wu, G.; Markle, A.B. An empirical test of gain-loss separability in prospect theory. Manag. Sci. 2008, 54, 1322–1335. [Google Scholar] [CrossRef]
- Tversky, A. Elimination by aspects: A theory of choice. Psychol. Rev. 1972, 79, 281–299. [Google Scholar] [CrossRef]
- Wu, G.; Gonzalez, R. Curvature of the probability weighting function. Manag. Sci. 1996, 42, 1676–1690. [Google Scholar] [CrossRef]
- Prelec, D. The probability weighting function. Econometrica 1998, 66, 497–527. [Google Scholar] [CrossRef]
- Prelec, D. A “Pseudo-endowment” effect, and its implications for some recent nonexpected utility models. J. Risk Uncertain. 1990, 3, 247–259. [Google Scholar] [CrossRef]
- Hershey, J.; Schoemaker, P. Probability versus certainty equivalence methods in utility measurement: Are they equivalent? Manag. Sci. 1985, 31, 1213. [Google Scholar] [CrossRef]
- Tversky, A.; Kahneman, D. Loss aversion in riskless choice—A reference-dependent model. Q. J. Econ. 1991, 106, 1039–1061. [Google Scholar] [CrossRef]
- ISO. ISO 31000:2018 Risk Management—Guidelines; International Organization for Standardization: Vernier, Geneva, Switzerland, 2018. [Google Scholar]
| 1 | Prospect Theory was analyzed using Israeli Pounds (I£), which was replaced by the Shekel in 1980 and the New Shekel in 1985. |
| 2 | By convention, states with a value of zero are not explicitly mentioned. In this case, there is a 95% chance of no gain or loss. |
| 3 | First half of the Wu and Markle surveys and all of the Wu and Gonzalez surveys. |
| 4 | Problems: 2 Birnbaum (1–6 and 2–7), 2 Birnbaum–Bahra Gain-Loss (6 and 12). |
| 5 | Problems: 2 Birnbaum (1–6 and 2–7), 2 Birnbaum–Bahra Gain-Loss (6 and 12), 2 Prelec 3CD. |
| 6 | For EDRM, when the 18 Hershey et al. gains problems are removed from the data set, the remainder of the optimized EDRM analyses together are normal, with a Shapiro–Wilk p-value of 0.1531. This is consistent with the discussion of Section 5.13.2. which documents the significant differences between the calculated and actual results. |







| Positive Theory | Normative Theory | Combined |
|---|---|---|
| EDRM | EU | EDRM-U |
| Proximity (subj prob) | Objective Probability | Proximity (subj prob) |
| Power Utility | Logarithmic Utility | Logarithmic Utility |
| Pure Gain or Loss (No Cancellation) | Mixed (Cancellation) | |
|---|---|---|
| Segregation (e.g., prefer many separate gifts) | wn > W | wn < W |
| Integration (e.g., prefer a single billing statement) | wn < W | wn > W |
| Economic Decision Theories | Dual Process Theory | Risk Perception | Risk Perception | Risk Perception |
|---|---|---|---|---|
| Stanovich, et al. 2011 | Slovic & Peters 2006 | Sjöberg 2000 | This Research | |
| Positive, behavioral | Type 1 (intuition) | Risk as feelings | Risk attitude | Risk aversion (proximity exponent) |
| Normative, expectation | Type 2 (deliberate) | Risk as analysis | Risk sensitivity | Risk sensitivity (neutral wealth) |
| Type | Risky Choice (Value, Probability) | Safer Choice (Value, Probability) | Problem Source |
|---|---|---|---|
| 0 | (96, 0.45; 4, 0.45; 2, 0.1) | (58, 0.45; 56, 0.45; 2, 0.1) | Birnbaum 3–12 |
| 1 | (96, 0.45; 4, 0.45; 2, 0.1) | (44, 45; 40, 0.45; 2, 0.1) | Birnbaum 1–12 |
| 2 | (98, 0.85; 96; 0.05; 11, 0.1) | (99, 0.9; 14, 0.05; 12, 0.05) | Birnbaum 4–5 |
| 3 | (5000, 0.001) or (−5) | (5) or (−5000, 0.001) | Prospect Theory 14 or 14′ |
| 4 | (10,000, 0.01; 2000, 0.04) | (7000, 0.05)2 | Prelec 3AB |
| 5 | (1200, 0.3; −200, 0.7) | (400, 0.7; −800 0.3) | Wu-Markle 23 Mixed |
| Number matching | Number of binary matches and number of problems (used for optimization) |
| Binomial Prob (>50%) | Binomial probability of result being >50% (same as ratio) |
| Binomial p-Value | Resulting binomial p-value, values are acceptable |
| Matching Std dev () | Standard deviation of % difference of matches only (used for optimization) |
| All % Coeff of Det ( | Coefficient of determination between all actual and calculated percentages |
| All % Spearman rank () | Nonparametric rank test between all actual and calculated percentages |
| Unit: 1991 USD | EDRM | EDRM Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|
| Power utility exp () | 1 | 1 | ||
| Risk aversion () | 1 | 0.9473 | 1 | 0.9473 |
| Neutral Wealth () $ | ||||
| All Coeff of Det ( | 0.9971 | 0.9973 | 0.9971 | 0.9973 |
| All Spearman rank () | 0.9983 | 0.9985 | 0.9983 | 0.9985 |
| Unit: 1982 USD | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Problem (Values Only) | EDRM-U | Calc% | Actual% | Diff | Match | |||||
| Outcome A | Outcome B | A | B | A | B | Y/N | ||||
| 1 | (50, 25) | (75) | 55.08 | 46.00 | 82 | 18 | 78 | 22 | −4.2 | Yes |
| 2 | (−100, −50) | (−150) | −89.96 | −69.70 | 10 | 90 | 18 | 83 | 7.8 | Yes |
| 3 | (−20, 100) | (80) | 29.75 | 47.98 | 30 | 70 | 27 | 73 | −3.0 | Yes |
| 4 | (25, −200) | (−175) | −501.83 | −512.88 | 66 | 34 | 77 | 23 | 10.7 | Yes |
| Unit: 1983 USD | EDRM Uncorrected | EDRM Optimal | EDRM-U Optimal |
|---|---|---|---|
| Power utility exp () | 0.88 | 0.688 | |
| Neutral Wealth () $ | 50.61 | ||
| Number matching | 4/4 | 4/4 | 4/4 |
| Binomial Prob (>50%) | 1 | 1 | 1 |
| Binomial p-Value | 0.125 | 0.125 | 0.125 |
| Matching Std dev () | 13.297 | 6.476 | 7.539 |
| All % Coeff of Det ( | 0.962 | 0.960 | 0.948 |
| All % Spearman rank () | 1 | 1 | 1 |
| Unit: 1978 Israeli Pound | EDRM Uncorrected | EDRM Optimal | EU Optimal | EDRM-U at | EDRM-U Optimal | |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.87 | 0.853 | |||
| Risk aversion () | 1 | 1 | 0.9775 | 1 | 0.894 | |
| Neutral Wealth () I£ | 6750 | 3755 | ||||
| Number matching | 19/19 | 19/19 | 19/19 | 12/19 | 19/19 | 19/19 |
| Binomial Prob (>50%) | 1 | 1 | 1 | 0.632 | 1 | 1 |
| Binomial p-Value | 3.8 × 10−6 | 3.8 × 10−6 | 3.8 × 10−6 | 0.359 | 3.8 × 10−6 | 3.8 × 10−6 |
| Matching Std dev () | 10.868 | 10.825 | 10.821 | 10.844 | 9.903 | 9.642 |
| All % Coeff of Det ( | 0.859 | 0.859 | 0.859 | 0.519 | 0.882 | 0.888 |
| All % Spearman rank () | 0.697 | 0.701 | 0.701 | 0.645 | 0.705 | 0.699 |
| Unit: 1980 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.65 | 0.65 | |||
| Risk aversion () | 1 | 1 | 1 | 1 | 0.668 | |
| Neutral Wealth () $ | 17.8 | 0.315 | ||||
| Number matching | 8/8 | 8/8 | 8/8 | 7/8 | 8/8 | 8/8 |
| Binomial Prob (>50%) | 1 | 1 | 1 | 0.875 | 1 | 1 |
| Binomial p-Value | 0.008 | 0.008 | 0.008 | 0.070 | 0.008 | 0.008 |
| Matching Std dev () | 8.757 | 6.023 | 6.023 | 8.613 | 7.810 | 6.312 |
| All % Coeff of Det ( | 0.945 | 0.968 | 0.968 | 0.960 | 0.958 | 0.965 |
| All % Spearman rank () | 0.850 | 0.850 | 0.850 | 0.850 | 0.850 | 0.850 |
| Unit: Years | EDRM | EDRM-U | EDRM-U | ||
|---|---|---|---|---|---|
| Problem (Years, Probability) | |||||
| A-Surgery | B-Radiation | Act%-Calc% | Act%-Calc% | Act%-Calc% | |
| 1a (1 year) | (1, 0.68; 5, 0.34; 1, 0.9) | (1, 0.77; 5, 0.22) | 6% | 11% | 20% |
| 1b | (−1, 0.32; −5, 0.66) | (−1, 0.23; −5, 0.78) | 1% | 1% | 1% |
| 1a (5 years) | (1, 0.68; 5, 0.34; 5, 0.9) | (1, 0.77; 5, 0.22) | −13% | −17% | 0% |
| 1b | (−1, 0.32; −5, 0.66) | (−1, 0.23; −5, 0.78) | 1% | 1% | 1% |
| Unit: 1985 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.649 | 0.328 | |||
| Risk aversion () | 1 | 1 | 0.503 | 1 | 1.420 | |
| Neutral Wealth () $ | 11.885 | |||||
| Number matching | 12/14 | 14/14 | 14/14 | 11/14 | 14/14 | 14/14 |
| Binomial Prob (>50%) | 0.857 | 1 | 1 | 0.786 | 1 | 1 |
| Binomial p-Value | 0.013 | 1.2 × 10−4 | 1.2 × 10−4 | 0.057 | 1.2 × 10−4 | 1.2 × 10−4 |
| Matching Std dev () | 7.911 | 8.410 | 8.334 | 11.124 | 10.110 | 8.720 |
| All % Coeff of Det ( | 0.793 | 0.910 | 0.911 | 0.883 | 0.899 | 0.898 |
| All % Spearman rank () | 0.864 | 0.917 | 0.917 | 0.900 | 0.917 | 0.917 |
| Unit: 2006 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.557 | 0.557 | |||
| Risk aversion () | 1 | 1 | 1 | 1 | 1.530 | |
| Neutral Wealth () $ | 625 | 7599 | ||||
| Number matching | 23/34 | 31/34 | 31/34 | 31/34 | 29/34 | 31/34 |
| Binomial Prob (>50%) | 0.676 | 0.912 | 0.912 | 0.912 | 0.853 | 0.912 |
| Binomial p-Value | 0.058 | 7.7 × 10−7 | 7.7 × 10−7 | 7.7 × 10−7 | 3.9 × 10−5 | 7.7 × 10−7 |
| Matching Std dev () | 7.884 | 11.297 | 11.297 | 12.236 | 12.126 | 11.202 |
| All % Coeff of Det ( | 0.514 | 0.565 | 0.565 | 0.671 | 0.467 | 0.618 |
| All % Spearman rank () | 0.725 | 0.776 | 0.776 | 0.766 | 0.688 | 0.780 |
| Unit: 2006 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.581 | 1 | |||
| Risk aversion () | 1 | 1 | 1.721 | 1 | 1.721 | |
| Neutral Wealth () $ | 51.5 | |||||
| Number matching | 19/34 | 28/34 | 28/34 | 20/34 | 21/34 | 28/34 |
| Binomial Prob (>50%) | 0.559 | 0.824 | 0.824 | 0.588 | 0.618 | 0.824 |
| Binomial p-Value | 0.608 | 2.0 × 10−4 | 2.0 × 10−4 | 0.392 | 0.230 | 2.0 × 10−4 |
| Matching Std dev () | 10.205 | 12.237 | 12.237 | 8.976 | 10.086 | 12.237 |
| All % Coeff of Det ( | 0.475 | 0.505 | 0.505 | 0.084 | 0.042 | 0.505 |
| All % Spearman rank () | 0.816 | 0.678 | 0.678 | 0.241 | 0.354 | 0.678 |
| Unit: 2006 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.8205 | 1 | |||
| Risk aversion () | 1 | 1 | 1.208 | 1 | 1.39 | |
| Neutral Wealth () $ | 12975 | 4375 | ||||
| Number matching | 28/34 | 28/34 | 28/34 | 20/34 | 28/34 | 30/34 |
| Binomial Prob (>50%) | 0.824 | 0.824 | 0.824 | 0.588 | 0.824 | 0.882 |
| Binomial p-Value | 2.0 × 10−4 | 2.0 × 10−4 | 2.0 × 10−4 | 0.392 | 2.0 × 10−4 | 2.0 × 10−6 |
| Matching Std dev () | 8.818 | 8.603 | 8.361 | 12.919 | 9.309 | 8.557 |
| All % Coeff of Det ( | 0.352 | 0.380 | 0.384 | 0.135 | 0.305 | 0.456 |
| All % Spearman rank () | 0.703 | 0.687 | 0.697 | 3.4 × 10−11 | 0.725 | 0.624 |
| Unit: 2004 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.898 | 0.848 | |||
| Risk aversion () | 1 | 1 | 0.846 | 1 | 0.968 | |
| Neutral Wealth () $ | 993.27 | 995.75 | ||||
| Number matching | 14/18 | 14/18 | 14/18 | 13/18 | 17/18 | 17/18 |
| Binomial Prob (>50%) | 0.778 | 0.778 | 0.778 | 0.722 | 0.944 | 0.944 |
| Binomial p-Value | 0.031 | 0.031 | 0.031 | 0.096 | 1.5 × −4 | 1.5 × 10−4 |
| Matching Std dev () | 8.800 | 8.751 | 7.187 | 12.981 | 8.855 | 8.773 |
| All % Coeff of Det ( | 0.615 | 0.619 | 0.685 | 0.313 | 0.678 | 0.685 |
| All % Spearman rank () | 0.800 | 0.800 | 0.860 | 0.317 | 0.800 | 0.804 |
| Unit: 2003 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.5 | 0.5 | |||
| Risk aversion () | 1 | 1 | 0.93 | 1 | 1.001 | |
| Neutral Wealth () $ | 22.75 | 22.75 | ||||
| Number matching | 11/21 | 19/21 | 19/21 | 16/21 | 18/21 | 18/21 |
| Binomial Prob (>50%) | 0.524 | 0.905 | 0.905 | 0.762 | 0.857 | 0.857 |
| Binomial P-Value | 1 | 2.2 × 10−4 | 2.2 × 10−4 | 0.027 | 1.5 × 10−3 | 1.5 × 10−3 |
| Matching Std dev () | 8.257 | 7.290 | 6.975 | 7.376 | 5.841 | 5.850 |
| All % Coeff of Det ( | 0.244 | 0.746 | 0.731 | 0.571 | 0.712 | 0.713 |
| All % Spearman rank () | 0.250 | 0.880 | 0.885 | 0.719 | 0.878 | 0.878 |
| Unit: 1995 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.5885 | 0.387 | |||
| Risk aversion () | 1 | 1 | 1.220 | 1 | 1.221 | |
| Neutral Wealth () $ | 99 | 23.4 | ||||
| Number matching | 26/40 | 30/40 | 33/40 | 18/40 | 29/40 | 34/40 |
| Binomial Prob (>50%) | 0.650 | 0.750 | 0.825 | 0.450 | 0.725 | 0.850 |
| Binomial P-Value | 0.081 | 2.2 × 10−3 | 4.2 × 10−5 | 0.636 | 6.4 × 10−3 | 8.4 × 10−6 |
| Matching Std dev () | 5.998 | 7.191 | 9.031 | 8.290 | 7.110 | 7.990 |
| All % Coeff of Det ( | 0.473 | 0.482 | 0.393 | 1.0 × 10−5 | 0.499 | 0.474 |
| All % Spearman rank () | 0.449 | 0.484 | 0.645 | 0.114 | 0.522 | 0.712 |
| Unit: 1989 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.543 | 0.467 | |||
| Risk aversion () | 1 | 1 | 1.268 | 1 | 1.12 | |
| Neutral Wealth () $ | 4765 | 22170 | ||||
| Number matching | 5/9 | 7/9 | 9/9 | 7/9 | 8/9 | 9/9 |
| Binomial Prob (>50%) | 0.556 | 0.778 | 1 | 0.778 | 0.889 | 1 |
| Binomial P-Value | 1 | 0.180 | 3.9 × 10−3 | 0.180 | 0.039 | 3.9 × 10−3 |
| Matching Std dev () | 6.940 | 7.575 | 16.201 | 12.617 | 9.160 | 7.245 |
| All % Coeff of Det ( | 0.320 | 0.720 | 0.558 | 0.124 | 0.803 | 0.924 |
| All % Spearman rank () | 0.433 | 0.850 | 0.700 | 0.333 | 0.900 | 0.983 |
| Unit: 1980 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 0.250 | 1 | |||
| Risk aversion () | 1 | 1 | 4.003 | 1 | 4.003 | |
| Neutral Wealth () $ | 0 | |||||
| Number matching | 6/10 | 10/10 | 10/10 | 10/10 | 10/10 | 10/10 |
| Binomial Prob (>50%) | 0.6 | 1 | 1 | 1 | 1 | 1 |
| Binomial P-Value | 0.705 | 2.0 × 10−3 | 2.0 × 10−3 | 2.0 × 10−3 | 2.0 × 10−3 | 2.0 × 10−3 |
| Matching Std dev () | 15.484 | 9.586 | 9.586 | 10.231 | 10.704 | 9.586 |
| All % Coeff of Det ( | 0.206 | 0.272 | 0.272 | 0.181 | 0.191 | 0.272 |
| All % Spearman rank () | 0.345 | 0.564 | 0.564 | 0.394 | 0.418 | 0.564 |
| Unit: 1980 USD | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Power utility exp () | 0.88 | 1 | 0.891 | |||
| Risk aversion () | 1 | 1 | 0.748 | 1 | 0.325 | |
| Neutral Wealth () $ | 274.25 | 1.494 | ||||
| Number matching | 12/18 | 13/18 | 13/18 | 9/18 | 18/18 | 18/18 |
| Binomial Prob (>50%) | 0.667 | 0.722 | 0.722 | 0.500 | 1 | 1 |
| Binomial P-Value | 0.238 | 0.096 | 0.096 | 1 | 7.6 × 10−6 | 7.6 × 10−6 |
| Matching Std dev () | 14.877 | 14.304 | 13.849 | 7.254 | 7.829 | 6.332 |
| All % Coeff of Det ( | 0.075 | 0.116 | 0.151 | 0.584 | 0.869 | 0.913 |
| All % Spearman rank () | −0.044 | −0.055 | −0.064 | 0.717 | 0.890 | 0.956 |
| Weighted Averages | EDRM Uncorrected | EDRM | EDRM Optimal | EU Optimal | EDRM-U | EDRM-U Optimal |
|---|---|---|---|---|---|---|
| Number matching | 183/259 | 221/259 | 226/259 | 174/259 | 219/259 | 236/259 |
| Binomial Prob (>50%) | 0.707 | 0.853 | 0.873 | 0.672 | 0.846 | 0.911 |
| Matching Std dev () | 9.108 | 9.591 | 9.973 | 10.261 | 9.115 | 8.954 |
| All % Coeff of Det ( | 0.471 | 0.554 | 0.541 | 0.352 | 0.527 | 0.636 |
| All % Spearman rank () | 0.589 | 0.658 | 0.683 | 0.497 | 0.676 | 0.760 |
| Optimal EDRM | All | Gain Only | Loss Only | Mix Only |
|---|---|---|---|---|
| Power utility exp (α) | 0.7095 | |||
| Risk aversion () | 1.012 | 1.012 | 0.988 | 0.969 |
| Number matching | 202/259 | 120/154 | 52/61 | 33/44 |
| Optimal EU | ||||
| Initial wealth () $ | 24.90 | |||
| Number matching | 144/259 | 88/154 | 43/61 | 12/44 |
| Optimal EDRM-U | ||||
| ) $ | 8778.52 | |||
| Risk aversion () | 1.306 | 1.245 | 1.604 | 1.306 |
| Number matching | 209/259 | 120/154 | 52/61 | 39/44 |
| EDRM | EDRM-U | |||
|---|---|---|---|---|
| Actual-Calc % Diff (prob > F) | ||||
| Type (Gain, Loss, Mix) | 0.5160 | Not Significant | 0.9206 | Not Significant |
| Match (yes, no) | 0.1992 | Not Significant | 0.2364 | Not Significant |
| Interaction (Type and Match) | 0.0329 | Significant | 0.2785 | Not Significant |
| Magnitude (prob > F) (Corrected to 2020 USD) | ||||
| Act-Calc % Diff | 0.7101 | Not Significant | 0.4448 | Not Significant |
| Match (yes, no) | 0.9461 | Not Significant | 0.5930 | Not Significant |
| Interaction (% Diff and Match) | 0.0672 | Marginal | 0.6293 | Not Significant |
| Normality (p-value) | ||||
| Shapiro–Wilk | 8.21 × 10−4 | Not Normal6 | 0.6774 | Normal |
| Normality Plots | Normal, except at the lower extreme due to Hershey et al. gains (See Section 5.13.2). | Normal | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Monroe, T.; Beruvides, M.; Tercero-Gómez, V. Quantifying Risk Perception: The Entropy Decision Risk Model Utility (EDRM-U). Systems 2020, 8, 51. https://doi.org/10.3390/systems8040051
Monroe T, Beruvides M, Tercero-Gómez V. Quantifying Risk Perception: The Entropy Decision Risk Model Utility (EDRM-U). Systems. 2020; 8(4):51. https://doi.org/10.3390/systems8040051
Chicago/Turabian StyleMonroe, Thomas, Mario Beruvides, and Víctor Tercero-Gómez. 2020. "Quantifying Risk Perception: The Entropy Decision Risk Model Utility (EDRM-U)" Systems 8, no. 4: 51. https://doi.org/10.3390/systems8040051
APA StyleMonroe, T., Beruvides, M., & Tercero-Gómez, V. (2020). Quantifying Risk Perception: The Entropy Decision Risk Model Utility (EDRM-U). Systems, 8(4), 51. https://doi.org/10.3390/systems8040051






