Research on Safety Decision-Making Behavior in Megaprojects
Abstract
1. Introduction
2. Literature Review
2.1. Construction Megaprojects
2.2. Engineering Safety Management
2.3. Decision-Making Behavior in Megaprojects
3. Evolutionary Game Model Construction
3.1. Basic Assumptions
3.2. Establishment of Benefit Matrix
4. Evolutionary Game Analysis
4.1. Expected Return Function
4.2. Replication Dynamic Equations
4.3. Evolutionary Game Stability Analysis
- (1)
- Stability analysis of pure strategies for construction companies in megaprojects.
- (2)
- Stability analysis of pure strategies for supervision units in megaprojects.
- (3)
- Analysis of the asymptotic stability of behavioral strategies for construction companies and supervisory units in megaprojects.
5. Evolutionary Game Simulation Analysis
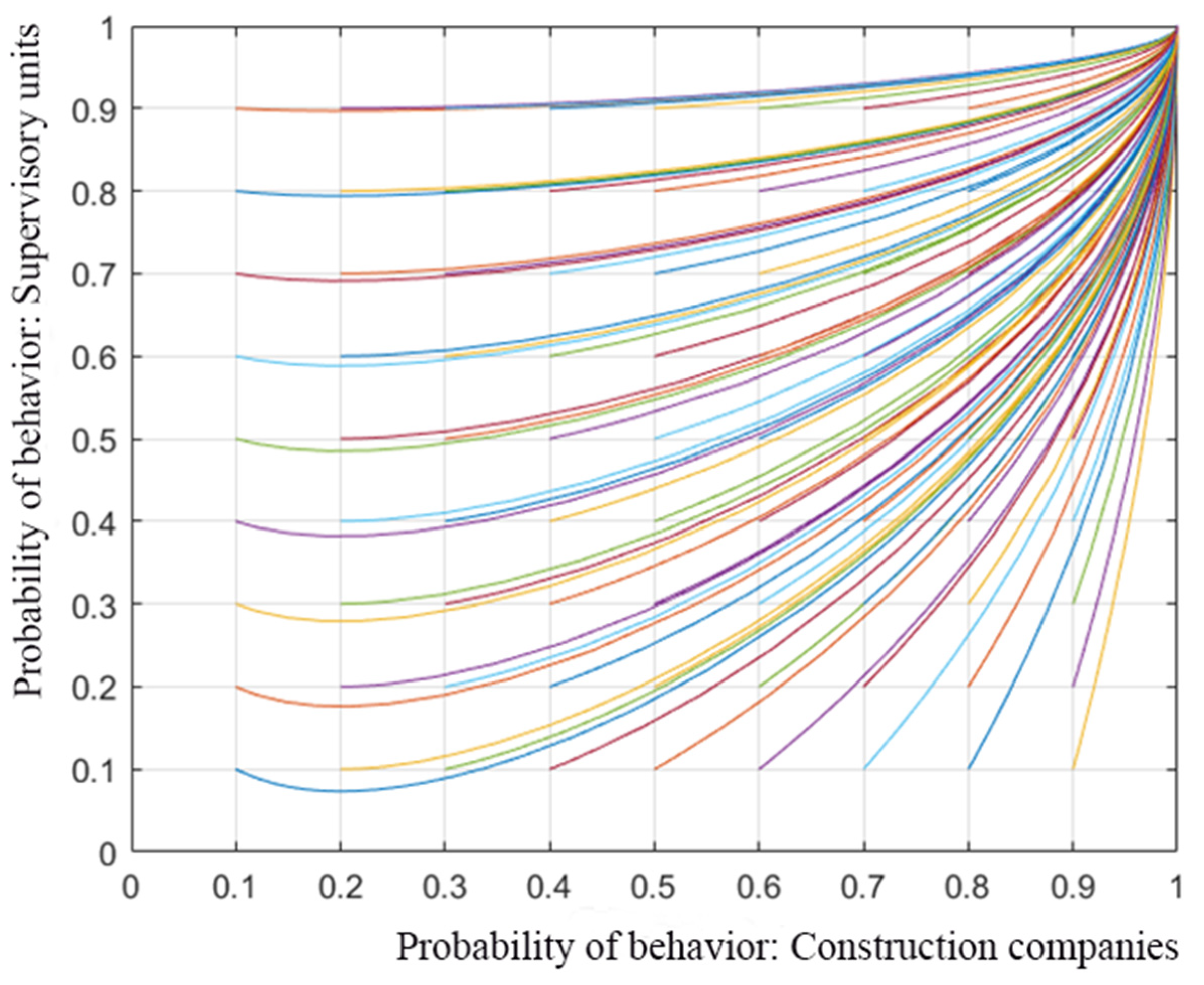
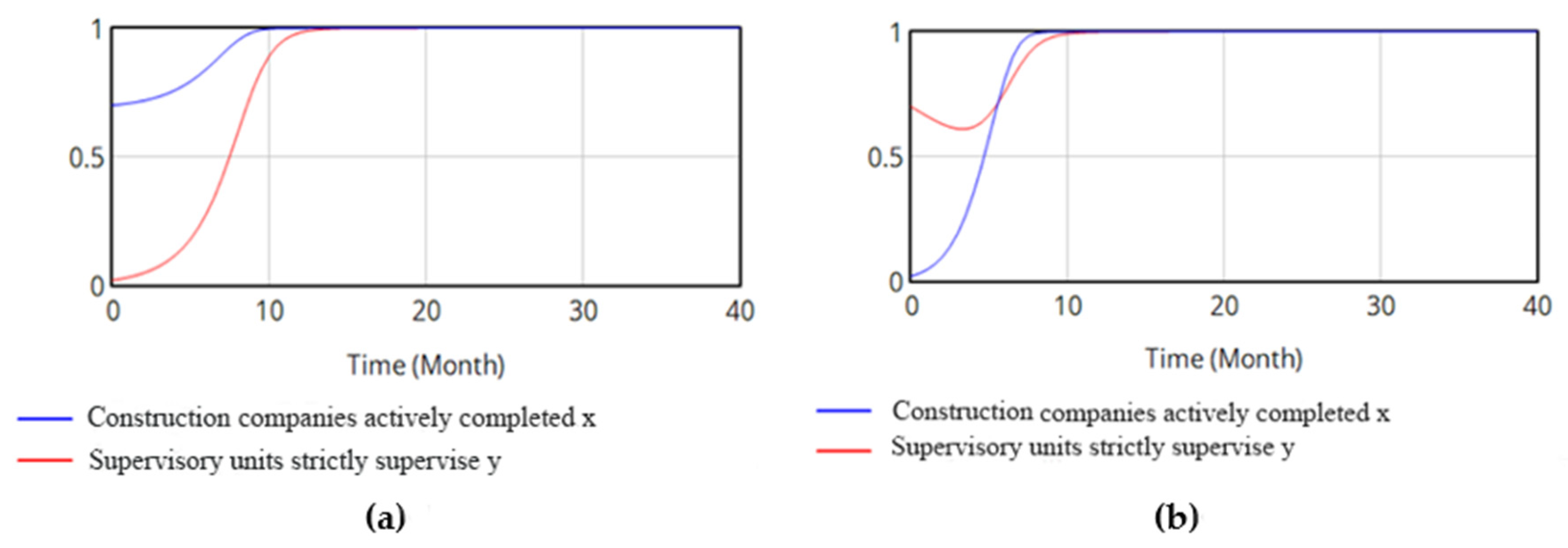
5.1. Evolution of the Game Dynamics of a Single Subject’s Independent Behavior on the Behavioral Choices of Both Parties
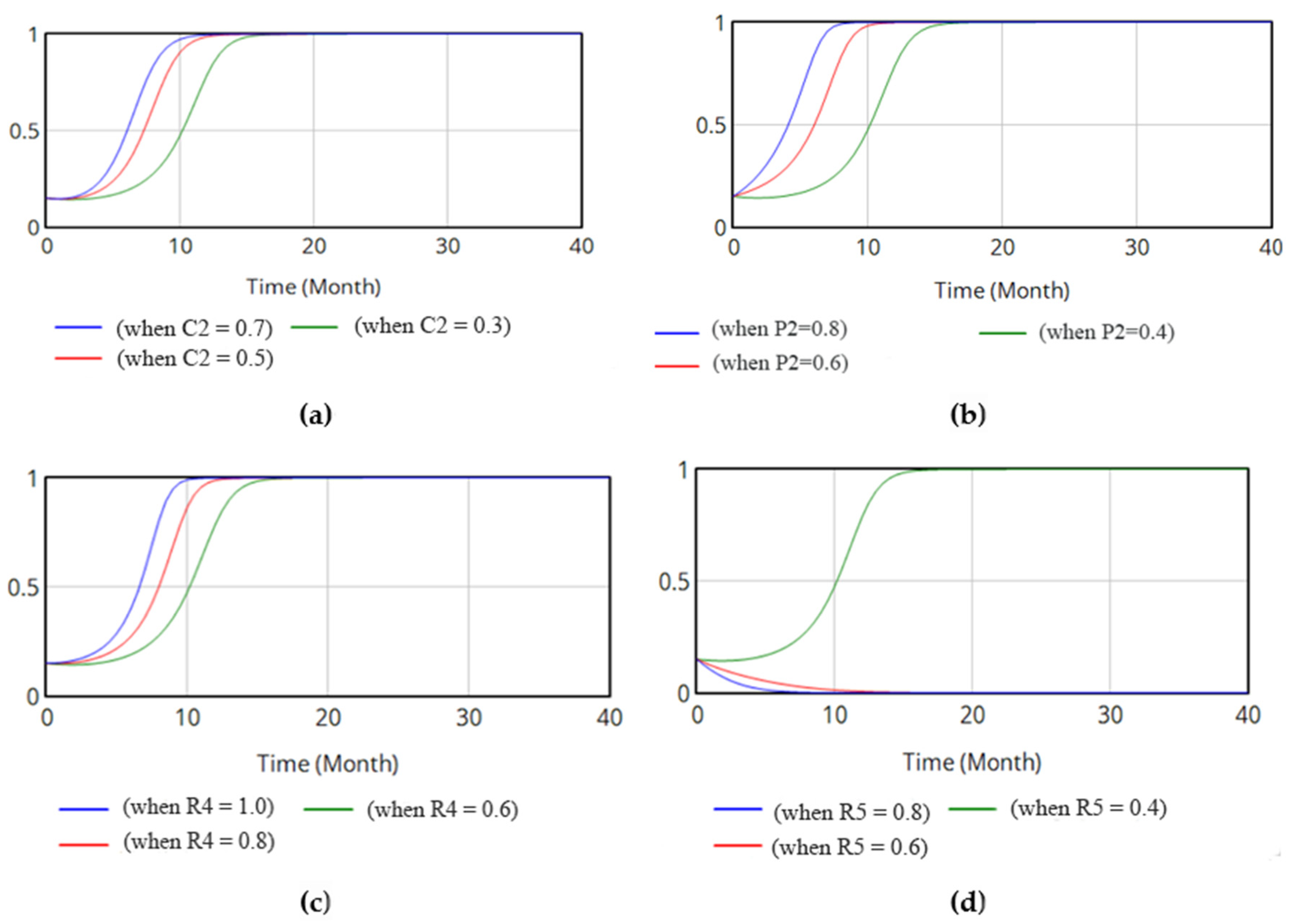
5.2. The Influence of Exogenous Variables on the Dynamic Evolution of the Game of Behavioral Strategies of a Single Subject
- (1)
- The influence of exogenous variables on the behavioral strategies of mega engineering construction companies.
- (2)
- The effect of exogenous variables on the safety behavioral strategies of supervisory units in megaprojects.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xie, L.; Lin, G.; Hon, C.; Xia, B.; Skitmore, M. Comparing the Psychosocial Safety Climate between Megaprojects and Non-Megaprojects: Evidence from China. Appl. Sci. 2020, 10, 8809. [Google Scholar] [CrossRef]
- Syn, T.; Ramaprasad, A. Megaprojects–symbolic and sublime: An ontological review. Int. J. Manag. Proj. Bus. 2019, 12, 377–399. [Google Scholar] [CrossRef]
- Zidane, Y.J.T.; Johansen, A.; Ekambaram, A. Megaprojects-Challenges and Lessons Learned. Procedia Soc. Behav. Sci. 2013, 74, 349–357. [Google Scholar] [CrossRef]
- Flyvbjerg, B.; Turner, J.R. Do classics exist in megaproject management? Int. J. Proj. Manag. 2018, 36, 334–341. [Google Scholar] [CrossRef]
- Ding, J.; Zhang, G.; Sun, M. Megaproject Governance Research in China: A Review and Visual Analysis from the Whole Life Cycle Perspective. Buildings 2023, 13, 1443. [Google Scholar] [CrossRef]
- Chatzimichailidou, M.; Whitcher, T.; Suzic, N. Complementarity and Compatibility of Systems Integration and Building Information Management. IEEE Syst. J. 2024, 18, 1198–1207. [Google Scholar] [CrossRef]
- Nik Bakht, M.; El-diraby, T. Ordinary people-extraordinary projects! From big data to knowledge in stakeholder management for construction megaprojects. eWork and eBusiness in Architecture, Engineering and Construction. In Proceedings of the 10th European Conference on Product and Process Modelling, Vienna, Austria, 17–19 September 2015. [Google Scholar] [CrossRef]
- Wijayasekera, S.C.; Hussain, S.A.; Paudel, A.; Paudel, B.; Steen, J.; Sadiq, R.; Hewage, K. Data Analytics and Artificial Intelligence in the Complex Environment of Megaprojects: Implications for Practitioners and Project Organizing Theory. Proj. Manag. J. 2022, 53, 485–500. [Google Scholar] [CrossRef]
- Sirisomboonsuk, P.; Gu, V.C.; Cao, R.Q.; Burns, J.R. Relationships between project governance and information technology governance and their impact on project performance. Int. J. Proj. Manag. 2018, 36, 287–300. [Google Scholar] [CrossRef]
- An, S.-M.; Woo, S.; Cho, C.-S.; Lee, S. Development of budget-constrained rescheduling method in mega construction project. KSCE J. Civ. Eng. 2016, 21, 85–93. [Google Scholar] [CrossRef]
- Locatelli, G.; Mikic, M.; Kovacevic, M.; Brookes, N.; Ivanisevic, N. The Successful Delivery of Megaprojects: A Novel Research Method. Proj. Manag. J. 2017, 48, 78–94. [Google Scholar] [CrossRef]
- Cepeda, D.M.; Sohail, M.; Ogunlowo, O.O. Understanding the critical success factors for delivery of megaprojects in Colombia. Proc. Inst. Civ. Eng. Manag. Procure. Law 2018, 171, 45–57. [Google Scholar] [CrossRef]
- Wang, G.; Locatelli, G.; Wan, J.; Li, Y.; Le, Y. Governing behavioral integration of top management team in megaprojects: A social capital perspective. Int. J. Proj. Manag. 2021, 39, 365–376. [Google Scholar] [CrossRef]
- Jiang, K.; Le, Y.; Zheng, X.; Zhang, X. Understanding Organizational Improvisation in Construction Megaprojects. Proc. Constr. Res. Congr. 2022, 2022, 855–862. [Google Scholar]
- Ma, T.; Ding, J.; Wang, Z.; Skibniewski, M.J. Governing Government-Project Owner Relationships in Water Megaprojects: A Concession Game Analysis on Allocation of Control Rights. Water Resour. Manag. 2020, 34, 4003–4018. [Google Scholar] [CrossRef]
- Al-Subaie, A.A.; Faisal, M.N.; Aouni, B.; Jabeen, F. Investigating the Role of Leadership Styles and Governance on Project Performance in Megaprojects. Pol. J. Manag. Stud. 2021, 23, 45–58. [Google Scholar] [CrossRef]
- Huda, M.S. Autocratic power? Energy megaprojects in the age of democratic backsliding. Energy Res. Soc. Sci. 2022, 90, 102605. [Google Scholar] [CrossRef]
- Liu, T.; Mao, Y.; Lu, S. Fostering Participants’ Collaborative Innovation Performance in Megaprojects: The Effects of Perceived Partners’ Non-Mediated Power. J. Constr. Eng. Manag. 2023, 149, 04022141. [Google Scholar] [CrossRef]
- Cantarelli, C.C. Innovation in megaprojects and the role of project complexity. Prod. Plan. Control 2020, 33, 943–956. [Google Scholar] [CrossRef]
- Jin, Z.; Zeng, S.; Chen, H.; Shi, J.J. Creating value from diverse knowledge in megaproject innovation ecosystems. Int. J. Proj. Manag. 2022, 40, 646–657. [Google Scholar] [CrossRef]
- Ansar, A. Old challenges, new solutions: Getting major projects right in the twenty-first century. Oxf. Rev. Econ. Policy 2022, 38, 217–223. [Google Scholar] [CrossRef]
- Ansar, A.; Flyvbjerg, B. How to solve big problems: Bespoke versus platform strategies. Oxf. Rev. Econ. Policy 2022, 38, 338–368. [Google Scholar] [CrossRef]
- Anna Wiewiora, K.C.D. Surfacing and responding paradoxes in megascale projects. Int. J. Proj. Manag. 2022, 40, 235–250. [Google Scholar] [CrossRef]
- Qiu, Y.; Chen, H.; Sheng, Z.; Cheng, S. Governance of institutional complexity in megaproject organizations. Int. J. Proj. Manag. 2019, 37, 425–443. [Google Scholar] [CrossRef]
- Shi, Q.; Hertogh, M.; Bosch-Rekveldt, M.; Zhu, J.; Sheng, Z. Exploring Decision-Making Complexity in Major Infrastructure Projects_ A Case Study From China. Proj. Manag. J. 2020, 51, 617–632. [Google Scholar] [CrossRef]
- Qiu, Y.; Chen, H.; Sheng, Z.; Zhang, J.; Cheng, S. Institutional Entrepreneurship and Megaproject: A Case of the Hong Kong–Zhuhai–Macau Bridge. IEEE Trans. Eng. Manag. 2022, 69, 3053–3067. [Google Scholar] [CrossRef]
- Hu, Y.; Chan, A.P.C.; Le, Y.; Jiang, W.-P.; Xie, L.-L.; Hon, C.H.K. Improving Megasite Management Performance through Incentives: Lessons Learned from the Shanghai Expo Construction. J. Manag. Eng. 2012, 28, 330–337. [Google Scholar] [CrossRef]
- Deng, L.; Zhong, M.; Liao, L.; Peng, L.; Lai, S. Research on Safety Management Application of Dangerous Sources in Engineering Construction Based on BIM Technology. Adv. Civ. Eng. 2019, 2019, 7450426. [Google Scholar] [CrossRef]
- Yang, Z.Z.; Feng, L. Application Study on Engineering Safety Management Base on Fractal Theory. In Proceedings of the International Conference on Engineering and Business Management (EBM2011), VOLS 1-6, Chengdu, China, 25–27 March 2011; pp. 1321–1324. [Google Scholar]
- Zhang, Y.; Tsai, S.-B. Safety Management of Civil Engineering Construction Based on Artificial Intelligence and Machine Vision Technology. Adv. Civ. Eng. 2021, 2021, 1–14. [Google Scholar] [CrossRef]
- Yap, J.B.H.; Lee, K.P.H.; Wang, C. Safety enablers using emerging technologies in construction projects: Empirical study in Malaysia. J. Eng. Des. Technol. 2021, 21, 1414–1440. [Google Scholar] [CrossRef]
- Liu, Y. Safety barriers: Research advances and new thoughts on theory, engineering and management. J. Loss Prev. Process. Ind. 2020, 67, 104260. [Google Scholar] [CrossRef]
- Qian, Q.; Lin, P. Safety risk management of underground engineering in China: Progress, challenges and strategies. J. Rock Mech. Geotech. Eng. 2016, 8, 423–442. [Google Scholar] [CrossRef]
- Xiong, Z.M.; Lu, H.; Wang, M.Y.; Qian, Q.H.; Rong, X.L. Research progress on safety risk management for large scale geotechnical engineering construction in China. Rock Soil Mech. 2018, 39, 3703–3716. [Google Scholar] [CrossRef]
- Feng, H.H.; Lu, L.; Liu, J. The Game Analysis of Quality Safety Behavior Subjects in Highway Engineering. Appl. Mech. Mater. 2014, 501–504, 2619–2622. [Google Scholar] [CrossRef]
- He, Y.J.Z. Analysis of Safety Management Measures in Civil Engineering Construction. In Proceedings of the 2019 International Conference on Education & Educational Research (ICEER 2019), New Orleans, LA, USA, 6–9 May 2019; pp. 19–23. [Google Scholar]
- Ouyang, J.; Ren, H. Game Analysis on Engineering Safety Management. In Proceedings of the 2008 International Conference on Risk Management & Engineering Management, Estoril, Portugal, 28–30 June 2008; pp. 61–64. [Google Scholar]
- Li, Y.; Suo, J. Safety Assessment of Construction Engineering Based on Combined Algorithms. In Proceedings of the 2009 International Conference on Intelligent Human-Machine Systems and Cybernetics, Hangzhou, China, 26–27 August 2009; pp. 47–50. [Google Scholar]
- Friedman, D. Evolutionary Games in Economics. Econometrica 1991, 59, 637. [Google Scholar] [CrossRef]
- Friedman, D. On economic applications of evolutionary game theory. J. Evol. Econ. 1998, 8, 15–43. [Google Scholar] [CrossRef]


| Subject of the Decision | Parameters | Explanation |
|---|---|---|
| Parameters of the construction companies | R1 | Proceeds to the construction companies on normal completion of megaprojects |
| R3 | Good image and reputational benefits gained by the construction companies when completed positively | |
| C1 | Additional costs incurred by the construction companies when actively completed | |
| P1 | Penalties in case of speculative behavior of construction companies | |
| C2 | Loss of image and reputation in case of speculative behavior of construction companies | |
| Parameters of the supervisory units | R2 | Proceeds to the supervisory units upon normal completion of the project |
| P2 | Penalties in case of lax supervision by the supervisory units | |
| C3 | Additional costs incurred when supervisory units are strictly supervised | |
| C4 | Costs incurred when supervisory units are lax in supervision | |
| Other parameters | R4 | Benefits of cooperation between the two parties from strict management by the supervisory units when the construction companies are actively completed |
| R5 | Other additional benefits from lax supervision by the supervisory units in the event of speculative behavior by the construction companies |
| Subjects of Decision | Supervisory Units | ||
|---|---|---|---|
| Strict Supervision (y) | Lax Supervision (1 − y) | ||
| Construction companies | Proactive completion (x) | (R1 − C1 + R3 + R4, R2 − C3 + R4) | (R1 − C1, R2 − P2 − C4) |
| Speculative behavior (1 − x) | (R1 − P1 − C2, R2 − C3) | (R1 − P1 − C2 + R5, R2 − P2 − C4 + R5) | |
| Equilibrium Point | det(J) | tr(J) |
|---|---|---|
| E1(0,0) | (C2 − C1 + P1 − R5)(C4 − C3 + P2 − R5) | C2 − C1 + P1 − R5+ C4 − C3 + P2 − R5 |
| E2(0,1) | (C2 − C1 + P1 + R3 + R4)(C3 − C4 − P2 + R5) | C2 − C1 + P1 + R3 + R4+C3 − C4 − P2 + R5 |
| E3(1,0) | (C1 − C2 − P1 + R5)(C4 − C3 + P2 + R4) | C1− C2 − P1 + R5+ C4 − C3 + P2 + R4 |
| E4(1,1) | (C1 − C2 − P1 − R3 − R4)(C3 − C4 − P2 − R4) | C1 − C2 − P1 − R3 − R4+ C3 − C4 − P2 − R4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haiyirete, X.; Gan, X.; Wang, J. Research on Safety Decision-Making Behavior in Megaprojects. Systems 2024, 12, 315. https://doi.org/10.3390/systems12080315
Haiyirete X, Gan X, Wang J. Research on Safety Decision-Making Behavior in Megaprojects. Systems. 2024; 12(8):315. https://doi.org/10.3390/systems12080315
Chicago/Turabian StyleHaiyirete, Xuekelaiti, Xiaochang Gan, and Jian Wang. 2024. "Research on Safety Decision-Making Behavior in Megaprojects" Systems 12, no. 8: 315. https://doi.org/10.3390/systems12080315
APA StyleHaiyirete, X., Gan, X., & Wang, J. (2024). Research on Safety Decision-Making Behavior in Megaprojects. Systems, 12(8), 315. https://doi.org/10.3390/systems12080315






