Decisions and Coordination of the Green Supply Chain with Retailers’ Fairness Concerns
Abstract
1. Introduction
1.1. Background and Motivation
1.2. Research Content and Questions
1.3. Contributions and Paper Arrangements
2. Literature Review
2.1. Decisions of the Green Supply Chain
2.2. Coordination of the Green Supply Chain
2.3. Fairness Concerns in the Supply Chain
2.4. Research Gaps
3. Model Description and Construction
3.1. Model Description
3.2. Notations
3.3. Assumptions and Model Building
3.3.1. Assumptions
3.3.2. Model Building
4. Decision-Making Model Analysis
4.1. Without Fairness Concerns
4.2. With Fairness Concerns of the Retailer
4.2.1. The Manufacturer Considers the Retailer’s Fairness Concerns
4.2.2. The Manufacturer Does Not Consider the Retailer’s Fairness Concerns
4.3. Sensitivity Analysis and Model Comparison
5. Coordination Model Analysis
5.1. Centralized Decision-Making
5.2. Two-Part Tariff Contract
6. Numerical Simulations and Discussions
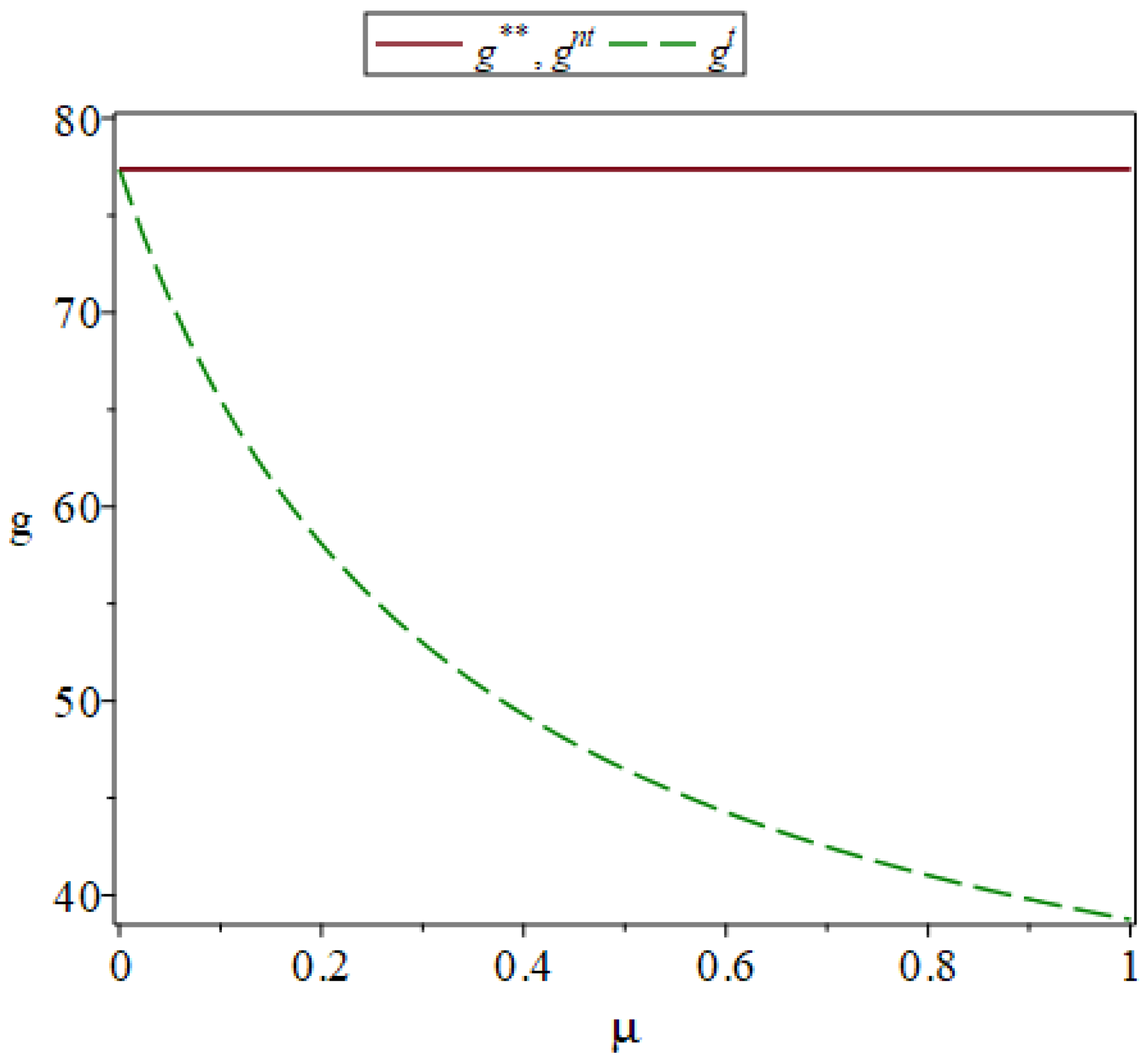
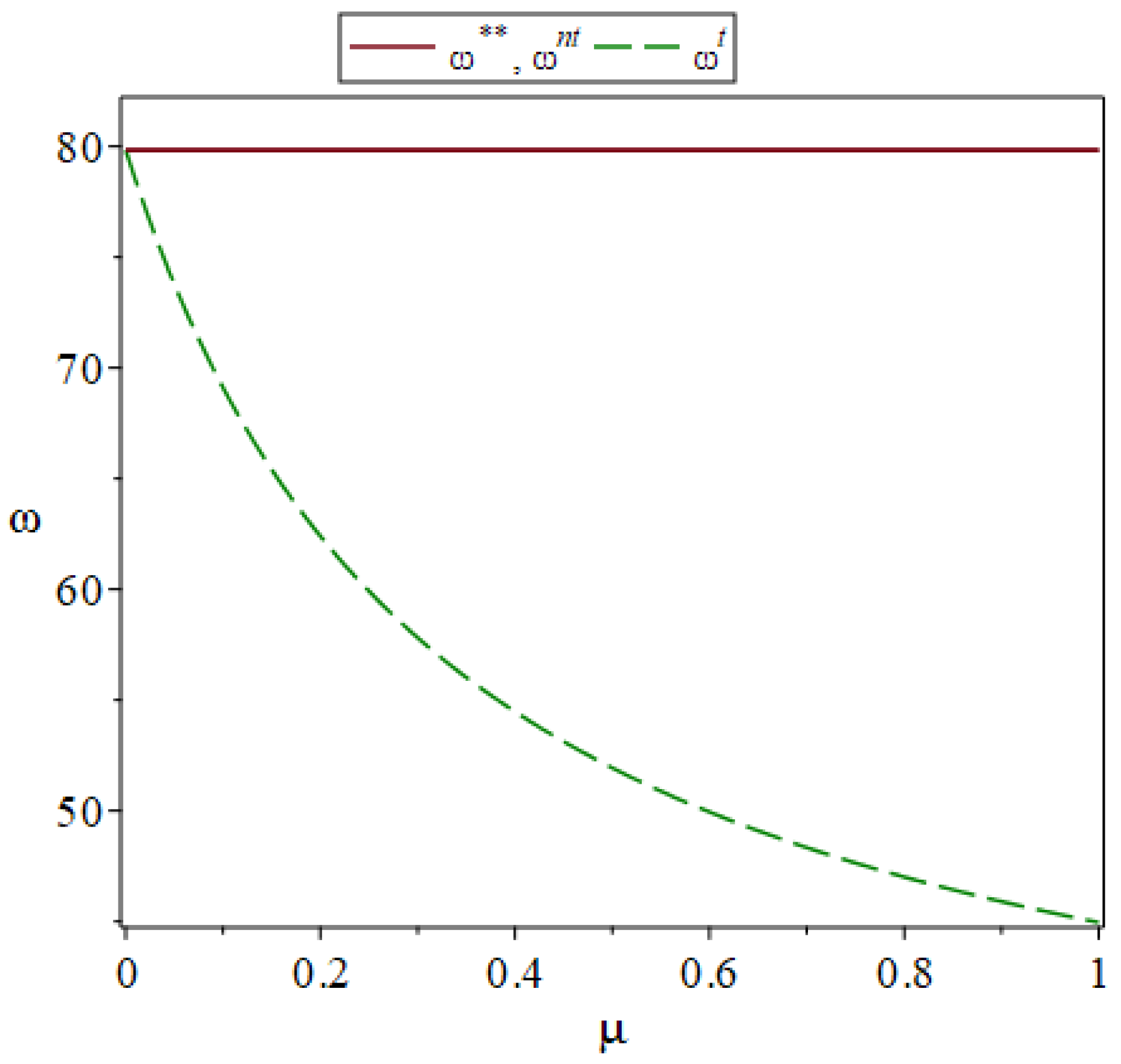
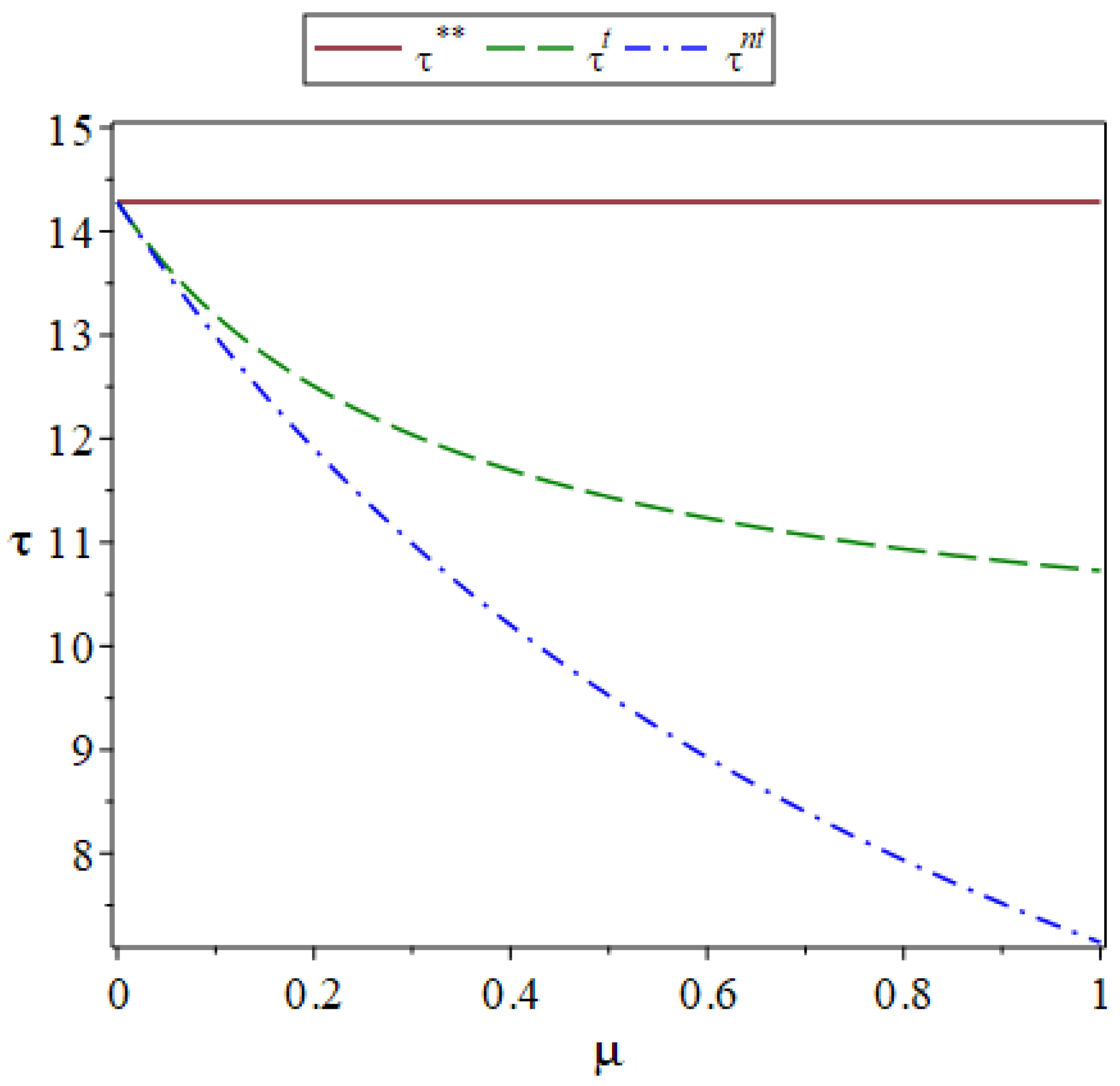
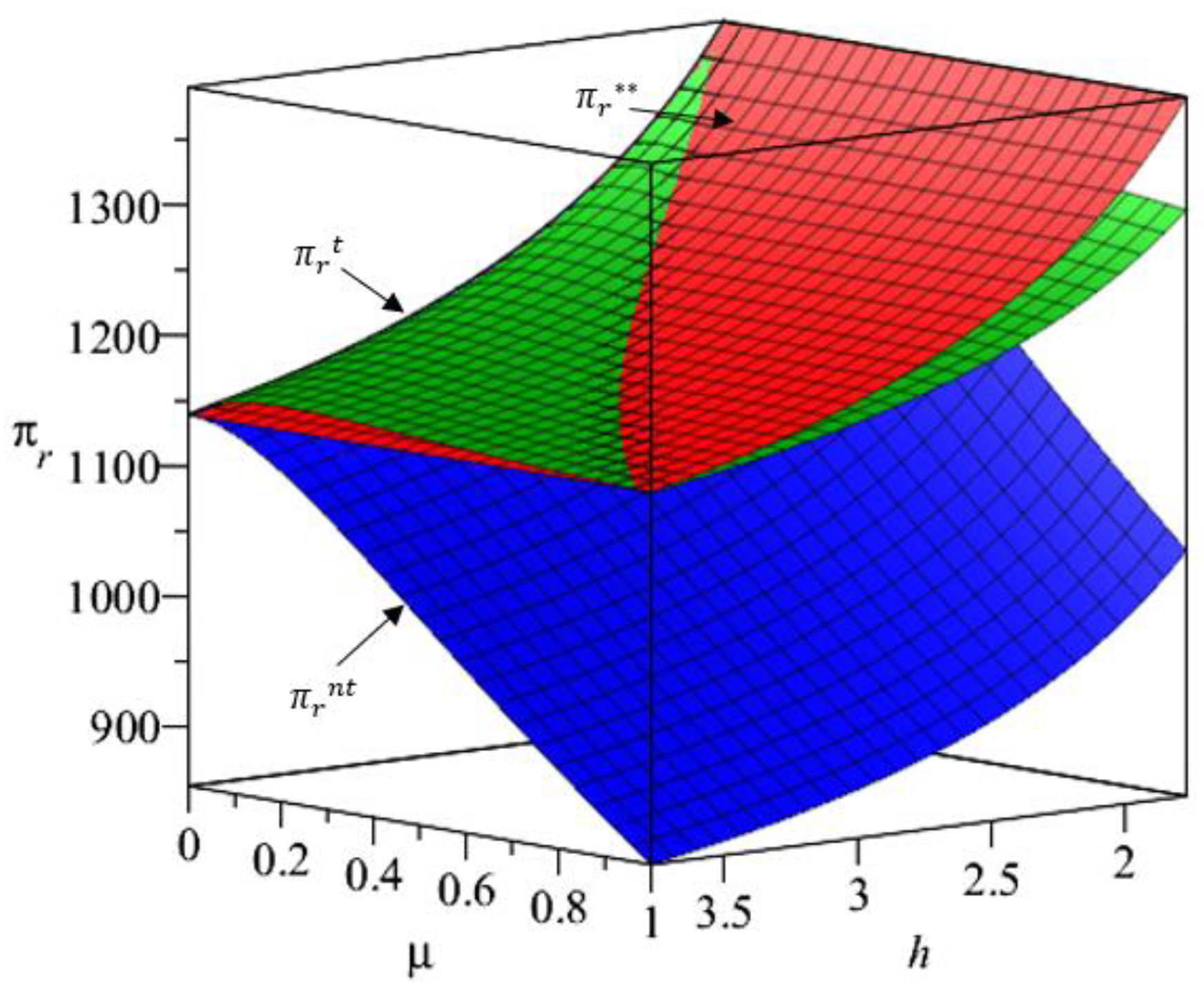
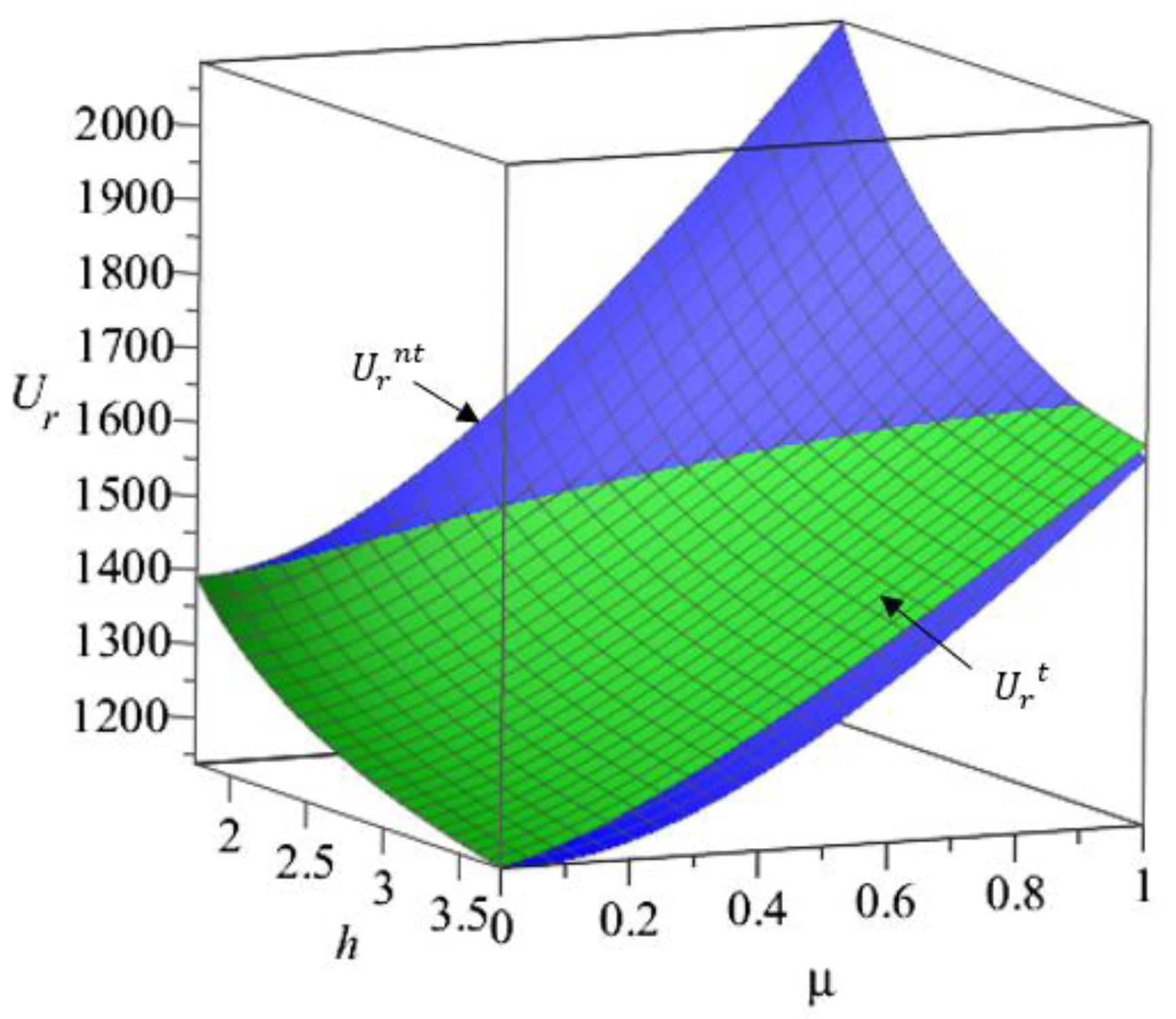
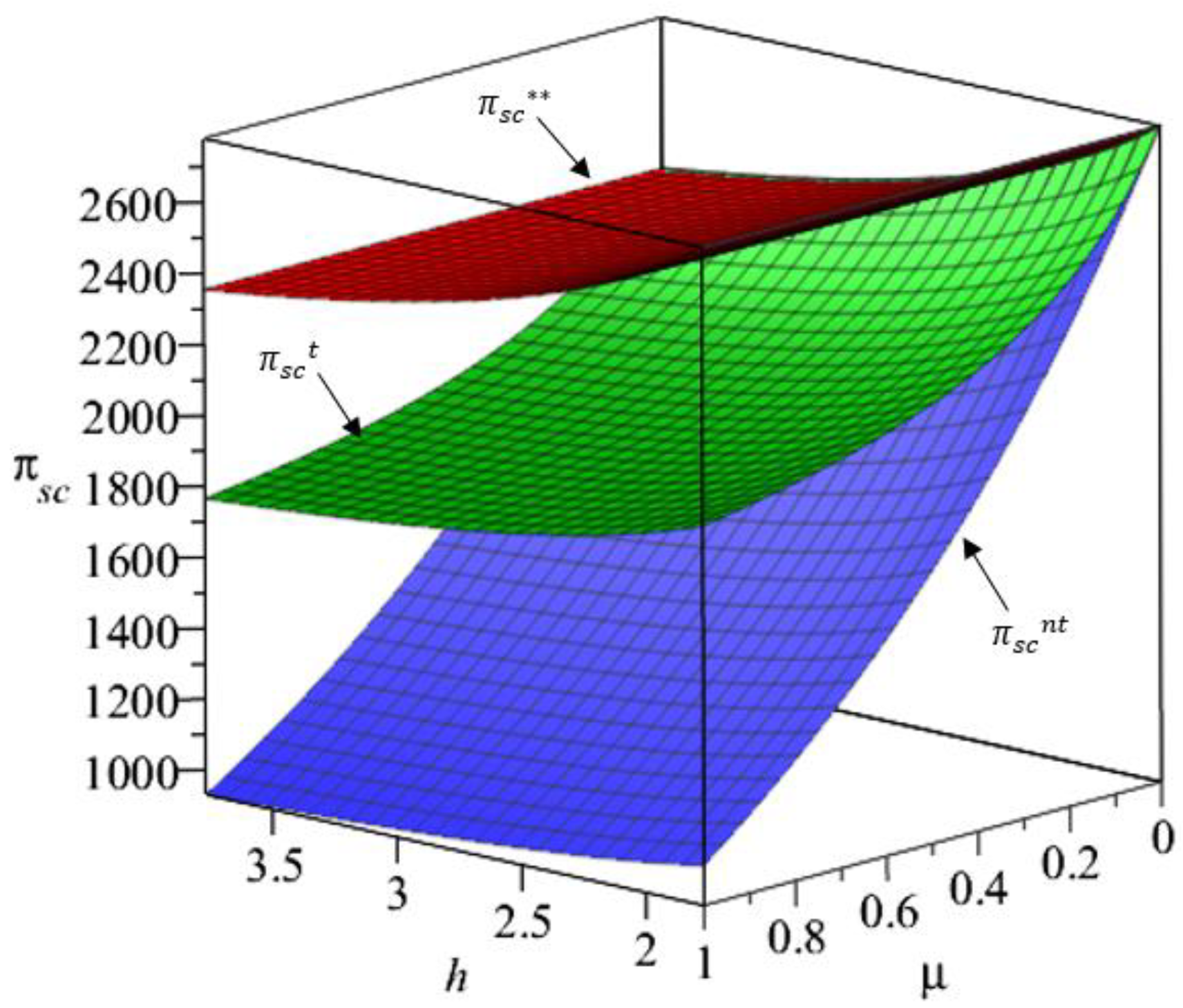
6.1. Impact of Fairness Concerns
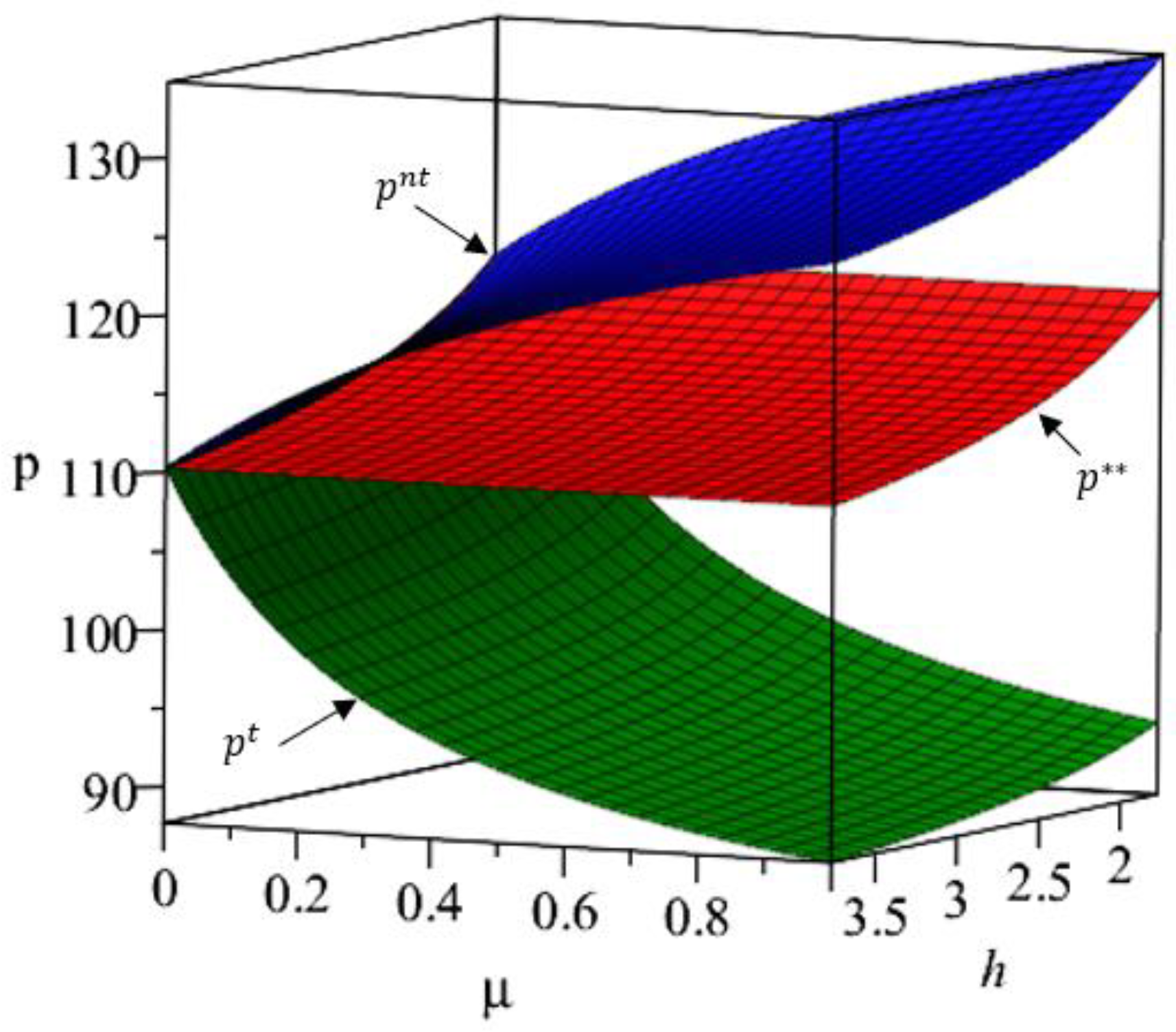
6.2. Coordination Contract
7. Conclusions, Managerial Implications and Limitations
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. The Proof of Theorem 1
Appendix A.2. The Proof of Theorem 2
Appendix A.3. The Proof of Theorem 3
Appendix A.4. The Proof of Corollary 1
Appendix A.5. The Proof of Corollary 2
Appendix A.6. The Proof of Corollary 3
Appendix A.7. The Proof of Corollary 4
Appendix A.8. The Proof of Corollary 5
Appendix A.9. The Proof of Corollary 6
Appendix A.10. The Proof of Corollary 7
Appendix A.11. The Proof of Corollary 8
Appendix A.12. The Proof of Theorem 4
Appendix A.13. The Proof of Theorem 5
Appendix A.14. The Proof of Proposition 1
References
- Gayialis, S.P.; Kechagias, E.P.; Papadopoulos, G.A.; Masouras, D. A review and classification framework of traceability approaches for identifying product supply chain counterfeiting. Sustainability 2022, 14, 6666. [Google Scholar] [CrossRef]
- Dey, B.K.; Yilmaz, I.; Seok, H. A Sustainable Supply Chain Integrated with Autonomated Inspection, Flexible Eco-Production, and Smart Transportation. Processes 2022, 10, 1775. [Google Scholar] [CrossRef]
- Kechagias, E.P.; Chatzistelios, G.; Papadopoulos, G.A.; Apostolou, P. Digital transformation of the maritime industry: A cybersecurity systemic approach. Int. J. Crit. Infr. Prot. 2022, 37, 100526. [Google Scholar] [CrossRef]
- Min, H. Blockchain technology for enhancing supply chain resilience. Bus. Horiz. 2019, 62, 35–45. [Google Scholar] [CrossRef]
- Li, G.; Li, L.; Choi, T.M.; Sethi, S.P. Green supply chain management in Chinese firms: Innovative measures and the moderating role of quick response technology. J. Oper. Manag. 2020, 66, 958–988. [Google Scholar] [CrossRef]
- Zhu, Q.; Sarkis, J. Relationships between operational practices and performance among early adopters of green supply chain management practices in Chinese manufacturing enterprises. J. Oper. Manag. 2004, 22, 265–289. [Google Scholar] [CrossRef]
- Xiaomi Group. 2020 Annual Report. 2020. Available online: https://cdn.cnbj1.fds.api.mi-img.com/staticsfile/csr/pdf/3.pdf (accessed on 1 June 2022).
- Wang, M. BYD will Invest 10.627 Billion Yuan in Research and Development in 2021, A Year-on-Year Increase of 24.2%. 2021. Available online: https://baijiahao.baidu.com/s?id=1728641710586085698&wfr=spider&for=pc (accessed on 1 June 2022).
- Roh, T.; Noh, J.; Oh, Y.; Park, K.-S. Structural relationships of a firm’s green strategies for environmental performance: The roles of green supply chain management and green marketing innovation. J. Clean. Prod. 2022, 356, 131877. [Google Scholar] [CrossRef]
- Hu, H.; Li, Y.; Li, M. Decisions and Coordination of Green Supply Chain Considering Big Data Targeted Advertising. J. Theor. Appl. Electron. Commer. Res. 2022, 17, 1035–1056. [Google Scholar] [CrossRef]
- Yan, K.; Liu, S.; Zuo, M.; Zheng, J.; Xu, Y. Dual-Channel Supply Chain Pricing Decisions under Discounted Advertising Value. Systems 2022, 10, 76. [Google Scholar] [CrossRef]
- Gurnani, H.; Erkoc, M.; Luo, Y. Impact of product pricing and timing of investment decisions on supply chain coopetition. Eur. J. Oper. Res. 2007, 180, 228–248. [Google Scholar] [CrossRef]
- Marketing Edge. Amazon Dethrones Procter & Gamble as World’s Largest Advertiser. 2020. Available online: https://marketingedge.com.ng/amazon-dethrones-procter-gamble-as-worlds-largest-advertiser/ (accessed on 1 June 2022).
- Corsten, D.; Kumar, N. Do suppliers benefit from collaborative relationships with large retailers? An empirical investigation of efficient consumer response adoption. J. Mark. 2005, 69, 80–94. [Google Scholar] [CrossRef]
- Loch, C.H.; Wu, Y. Social preferences and supply chain performance: An experimental study. Manag. Sci. 2008, 54, 1835–1849. [Google Scholar] [CrossRef]
- Katok, E.; Olsen, T.; Pavlov, V. Wholesale pricing under mild and privately known concerns for fairness. Prod. Oper. Manag. 2014, 23, 285–302. [Google Scholar] [CrossRef]
- Nie, T.; Du, S. Dual-fairness supply chain with quantity discount contracts. Eur. J. Oper. Res. 2017, 258, 491–500. [Google Scholar] [CrossRef]
- Jian, J.; Li, B.; Zhang, N.; Su, J. Decision-making and coordination of green closed-loop supply chain with fairness concern. J. Clean. Prod. 2021, 298, 126799. [Google Scholar] [CrossRef]
- Tsao, Y.; Sheen, G. Effects of promotion cost sharing policy with the sales learning curve on supply chain coordination. Comput. Oper. Res. 2012, 39, 1872–1878. [Google Scholar] [CrossRef]
- Hu, H.; Wu, Q.; Han, S.; Zhang, Z. Coordination of dual-channel supply chain with perfect product considering sales effort. Adv. Prod. Eng. Manag. 2020, 15, 192–203. [Google Scholar] [CrossRef]
- Zhang, R.; Ma, W.; Si, H.; Liu, J.; Liao, L. Cooperative game analysis of coordination mechanisms under fairness concerns of a green retailer. J. Retail. Consum. Serv. 2021, 59, 102361. [Google Scholar] [CrossRef]
- Zhang, L.; Zhou, H.; Liu, Y.; Lu, R. Optimal environmental quality and price with consumer environmental awareness and retailer’s fairness concerns in supply chain. J. Clean. Prod. 2019, 213, 1063–1079. [Google Scholar] [CrossRef]
- Wang, Y.; Fan, R.; Shen, L.; Jin, M. Decisions and coordination of green e-commerce supply chain considering green manufacturer’s fairness concerns. Int. J. Prod. Res. 2020, 58, 7471–7489. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, Z.; Pu, X.; Li, Y. Green manufacturing strategy considering retailers’ fairness concerns. Sustainability 2019, 11, 4646. [Google Scholar] [CrossRef]
- Yuan, X.; Tang, F.; Zhang, D.; Zhang, X. Green remanufacturer’s mixed collection channel strategy considering enterprise’s environmental responsibility and the fairness concern in reverse green supply chain. Int. J. Environ. Res. Public Health 2021, 18, 3405. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Shao, E.; Gong, Y.; Guan, X. Decision-making for green supply chain considering fairness concern based on trade credit. IEEE Access 2021, 9, 67684–67695. [Google Scholar] [CrossRef]
- Zhu, W.; He, Y. Green product design in supply chains under competition. Eur. J. Oper. Res. 2017, 258, 165–180. [Google Scholar] [CrossRef]
- Parsaeifar, S.; Bozorgi-Amiri, A.; Naimi-Sadigh, A.; Sangari, M.S. A game theoretical for coordination of pricing, recycling, and green product decisions in the supply chain. J. Clean. Prod. 2019, 226, 37–49. [Google Scholar] [CrossRef]
- Agi, M.; Yan, X. Greening products in a supply chain under market segmentation and different channel power structures. Int. J. Prod. Econ. 2020, 223, 107523. [Google Scholar] [CrossRef]
- Xia, Q.; Zhi, B.; Wang, X. The role of cross-shareholding in the green supply chain: Green contribution, power structure and coordination. Int. J. Prod. Econ. 2021, 234, 108037. [Google Scholar] [CrossRef]
- Agi, M.A.N.; Nishant, R. Understanding influential factors on implementing green supply chain management practices: An interpretive structural modelling analysis. J. Environ. Manag. 2017, 188, 351–363. [Google Scholar] [CrossRef]
- Jamali, M.B.; Rasti-barzoki, M. A game theoretic approach for green and non-green product pricing in chain-to-chain competitive sustainable and regular dual-channel supply chains. J. Clean. Prod. 2018, 170, 1029–1043. [Google Scholar] [CrossRef]
- Gao, J.; Xiao, Z.; Wei, H. Competition and coordination in a dual-channel green supply chain with an eco-label policy. Comput. Ind. Eng. 2021, 153, 107057. [Google Scholar] [CrossRef]
- Khosroshahi, H.; Dimitrov, S.; Hejazi, S.R. Pricing, greening, and transparency decisions considering the impact of government subsidies and CSR behavior in supply chain decisions. J. Retail. Consum. Serv. 2021, 60, 102485. [Google Scholar] [CrossRef]
- Li, Z.; Pan, Y.; Yang, W.; Ma, J.; Zhou, M. Effects of government subsidies on green technology investment and green marketing coordination of supply chain under the cap-and-trade mechanism. Energ. Econ. 2021, 101, 105426. [Google Scholar] [CrossRef]
- Li, B.; Wang, H.; Zheng, W. Who will take on green product development in supply chains? Manufacturer or online retailer. J. Clean. Prod. 2021, 314, 128000. [Google Scholar] [CrossRef]
- Xiang, Z.; Xu, M. Dynamic game strategies of a two-stage remanufacturing closed-loop supply chain considering Big Data marketing, technological innovation and overconfidence. Comput. Ind. Eng. 2020, 145, 106538. [Google Scholar] [CrossRef]
- Tian, G.; Liu, J.; Gong, Y.; Wang, Q.; Sun, H.; Chen, H. Multinational companies’ coordination mechanism for extending corporate social responsibility to Chinese suppliers. J. Clean. Prod. 2020, 267, 121896. [Google Scholar] [CrossRef]
- Peng, Y.; Wang, W.; Li, S.; Veglianti, E. Competition and cooperation in the dual-channel green supply chain with customer satisfaction. Econ. Anal. Policy 2022, 76, 95–113. [Google Scholar] [CrossRef]
- Pal, B.; Sarkar, A.; Sarkar, B. Optimal decisions in a dual-channel competitive green supply chain management under promotional effort. Expert. Syst. Appl. 2023, 211, 118315. [Google Scholar] [CrossRef]
- Meng, Q.; Li, M.; Liu, W.; Li, Z.; Zhang, J. Pricing policies of dual-channel green supply chain: Considering government subsidies and consumers’ dual preferences. Sustain. Prod. Consump. 2021, 26, 1021–1030. [Google Scholar] [CrossRef]
- Ling, Y.; Xu, J. Price and greenness competition between duopoly firms considering consumer premium payments. Environ. Dev. Sustain. 2021, 23, 3853–3880. [Google Scholar] [CrossRef]
- Liu, A.; Luo, S.; Mou, J.; Qiu, H. The antagonism and cohesion of the upstream supply chain under information asymmetry. Ann. Oper. Res. 2021, 1–46. [Google Scholar] [CrossRef]
- Manoj, U.V.; Sriskandarajah, C.; Wagneur, E. Coordination in a two-stage production system: Complexity, conflict and cooperation. Comput. Oper. Res. 2012, 39, 1245–1256. [Google Scholar] [CrossRef]
- Liu, G.; Yang, H.; Dai, R. Which contract is more effective in improving product greenness under different power structures: Revenue sharing or cost sharing? Comput. Ind. Eng. 2022, 148, 106701. [Google Scholar] [CrossRef]
- Shen, Y.; Willems, S.P.; Dai, Y. Channel selection and contracting in the presence of a retail platform. Prod. Oper. Manag. 2019, 28, 1173–1185. [Google Scholar] [CrossRef]
- Li, M.; Zhang, X. Information acquisition and its incentives in an E-Commerce supply chain under the offline showroom model. J. Theor. Appl. Electron. Commer. 2021, 16, 1791–1804. [Google Scholar] [CrossRef]
- Hong, Z.; Guo, X. Green product supply chain contracts considering environmental responsibilities. Omega 2019, 83, 155–166. [Google Scholar] [CrossRef]
- Xu, X.; He, P.; Xu, H.; Zhang, Q. Supply chain coordination with green technology under cap-and-trade regulation. Int. J. Prod. Econ. 2017, 183, 433–442. [Google Scholar] [CrossRef]
- Heydaria, J.; Govindanb, K.; Basiria, Z. Balancing price and green quality in presence of consumer environmental awareness: A green supply chain coordination approach. Int. J. Prod. Res. 2020, 59, 1957–1975. [Google Scholar] [CrossRef]
- Zhang, C.T.; Wang, H.X.; Ren, M.L. Research on pricing and coordination strategy of green supply chain under hybrid production mode. Comput. Ind. Eng. 2014, 72, 24–31. [Google Scholar] [CrossRef]
- Basiri, Z.; Heydari, J. A mathematical model for green supply chain coordination with substitutable products. J. Clean. Prod. 2017, 145, 232–249. [Google Scholar] [CrossRef]
- Cui, T.H.; Raju, J.S.; Zhang, Z.J. Fairness and channel coordination. Manag. Sci. 2007, 53, 1303–1314. [Google Scholar]
- Ho, T.H.; Zhang, J. Designing pricing contracts for boundedly rational customers: Does the framing of the fixed fee matter? Manag. Sci. 2008, 54, 686–700. [Google Scholar] [CrossRef]
- Toktas-Palut, P. An integrated contract for coordinating a three-stage green forward and reverse supply chain under fairness concerns. J. Clean. Prod. 2021, 279, 123735. [Google Scholar] [CrossRef]
- Mondal, C.; Giri, B.C. Investigating a green supply chain with product recycling under retailer’s fairness behavior. J. Ind. Manag. Optim. 2022, 18, 3641–3677. [Google Scholar] [CrossRef]
- Zhou, Q.; Li, Q.; Hu, X.; Yang, W.; Chen, W. Optimal contract design problem considering the retailer’s fairness concern with asymmetric demand information. J. Clean. Prod. 2021, 287, 125407. [Google Scholar] [CrossRef]
- Adhikari, A.; Bisi, A. Collaboration, bargaining, and fairness concern for a green apparel supply chain: An emerging economy perspective. Transp. Res. E-Log. 2020, 135, 101863. [Google Scholar] [CrossRef]
- Liu, J.; Wu, X.; Lei, J.L. The combined impacts of consumer green preference and fairness concern on the decision of three-party supply chain. J. Ind. Manag. Optim. 2022, 18, 2749–2782. [Google Scholar] [CrossRef]
- Wang, D.; Ge, G.; Zhou, Y.; Zhu, M. Pricing-decision analysis of green supply chain with two competitive manufacturers considering horizontal and vertical fairness concerns. Environ. Sci. Pollut. R 2022, 29, 66235–66258. [Google Scholar] [CrossRef]
- Hu, H.; Li, Y.; Li, M.; Zhu, W.; Chan, F. Optimal decision-making of green agricultural product supply chain with fairness concerns. J. Ind. Manag. Optim. 2022, 1–23. [Google Scholar] [CrossRef]
- Sun, H.; Zhong, Y. Carbon emission reduction and green marketing decisions in a two-echelon low-carbon supply chain considering fairness concern. J. Bus. Ind. Mark. 2022, 1–25. [Google Scholar] [CrossRef]
- Ma, P.; Li, K.W.; Wang, Z.J. Pricing decisions in closed-loop supply chains with marketing effort and fairness concerns. Int. J. Prod. Res. 2017, 55, 6710–6731. [Google Scholar] [CrossRef]
- Alizadeh-Basban, N.; Taleizadeh, A.A. A hybrid circular economy—Game theoretical approach in a dual-channel green supply chain considering sale’s effort, delivery time, and hybrid remanufacturing. J. Clean. Prod. 2020, 250, 119521. [Google Scholar] [CrossRef]
- Zhu, Q.; Li, X.; Zhao, S. Cost-sharing models for green product production and marketing in a food supply chain. Ind. Manag. Data. Syst. 2018, 118, 654–682. [Google Scholar] [CrossRef]
- Hu, H.; Li, Y.; Tian, M.; Cai, X. Evolutionary Game of Small and Medium-Sized Enterprises’ Accounts-Receivable Pledge Financing in the Supply Chain. Systems 2022, 10, 21. [Google Scholar] [CrossRef]
- Xie, B.; An, K.; Cheng, Y. Dynamic Research on Three-Player Evolutionary Game in Waste Product Recycling Supply Chain System. Systems 2022, 10, 185. [Google Scholar] [CrossRef]


| Literature | Decision Variables | Fairness Concerns | Coordination | ||||
|---|---|---|---|---|---|---|---|
| P | G | GM | W | C | N | ||
| [27] | ✓ | ✓ | ✓ | ||||
| [39] | ✓ | ✓ | ✓ | ||||
| [40] | ✓ | ✓ | ✓ | ||||
| [18] | ✓ | ✓ | ✓ | ✓ | |||
| [22] | ✓ | ✓ | ✓ | ✓ | |||
| [55] | ✓ | ✓ | ✓ | ✓ | ✓ | ||
| [21] | ✓ | ✓ | ✓ | ✓ | ✓ | ||
| [61] | ✓ | ✓ | ✓ | ✓ | ✓ | ||
| [62] | ✓ | ✓ | ✓ | ✓ | ✓ | ||
| This paper | ✓ | ✓ | ✓ | ✓ | ✓ | ✓ | ✓ |
| Notation Names | Notations | Descriptions |
|---|---|---|
| Decision-making variables | Wholesale price (CNY/unit) | |
| Retail price (CNY/unit) | ||
| Greenness level | ||
| Green marketing effort level | ||
| Model parameters | Cost of production (CNY/unit) | |
| Green investment coefficient of green products (CNY), | ||
| Green marketing effort cost coefficient of green products (CNY), | ||
| Basic demand scale | ||
| Green sensitivity coefficient of consumers, | ||
| Green marketing effort sensitivity coefficient of consumers, | ||
| Fairness concerns coefficient of the retailer, | ||
| Other notations | Green investment of the manufacturer (CNY) | |
| Green marketing effort cost of the retailer (CNY) | ||
| Market demand | ||
| Manufacturer’s profit (CNY) | ||
| Retailer’s profit (CNY) | ||
| Supply chain’s profit (CNY) | ||
| Retailer’s utility (CNY) |
| (CNY) | (CNY) | (CNY) | (CNY) | (CNY) | (CNY) | (CNY) |
|---|---|---|---|---|---|---|
| −222, 562, 197 | 1189 | 554,406 | 1375 | 1375 | 555,781 | 555,781 |
| −222, 462, 197 | 1189 | 454,406 | 1375 | 101,375 | 555,781 | 555,781 |
| −222, 362, 197 | 1189 | 354,406 | 1375 | 201,375 | 555,781 | 555,781 |
| −222, 262, 197 | 1189 | 254,406 | 1375 | 301,375 | 555,781 | 555,781 |
| −222, 162, 197 | 1189 | 154,406 | 1375 | 401,375 | 555,781 | 555,781 |
| −222, 008, 980 | 1189 | 1189 | 1375 | 554,592 | 555,781 | 555,781 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, H.; Li, Y.; Li, Y.; Li, M.; Yue, X.; Ding, Y. Decisions and Coordination of the Green Supply Chain with Retailers’ Fairness Concerns. Systems 2023, 11, 5. https://doi.org/10.3390/systems11010005
Hu H, Li Y, Li Y, Li M, Yue X, Ding Y. Decisions and Coordination of the Green Supply Chain with Retailers’ Fairness Concerns. Systems. 2023; 11(1):5. https://doi.org/10.3390/systems11010005
Chicago/Turabian StyleHu, Haiju, Yakun Li, Yingyue Li, Mengdi Li, Xiao Yue, and Yu Ding. 2023. "Decisions and Coordination of the Green Supply Chain with Retailers’ Fairness Concerns" Systems 11, no. 1: 5. https://doi.org/10.3390/systems11010005
APA StyleHu, H., Li, Y., Li, Y., Li, M., Yue, X., & Ding, Y. (2023). Decisions and Coordination of the Green Supply Chain with Retailers’ Fairness Concerns. Systems, 11(1), 5. https://doi.org/10.3390/systems11010005







