Stability Analysis of Low-Carbon Technology Innovation Cooperation under a Reward and Punishment Mechanism
Abstract
:1. Introduction
2. Review of the Literature
2.1. A Study of the Evolution of LCTI
2.2. Research Methodology of LCTI
3. Model Construction
3.1. Basic Assumptions
3.2. Model Establishment
3.3. Replicator Dynamic Analysis
3.3.1. Stability Analysis of Enterprise A
3.3.2. Stability Analysis of Enterprise B
3.3.3. Stability Analysis of Government
3.4. Stability Analysis of Equilibrium Points
4. Numerical Simulation and Results
4.1. The Dynamic Evolution of ESS
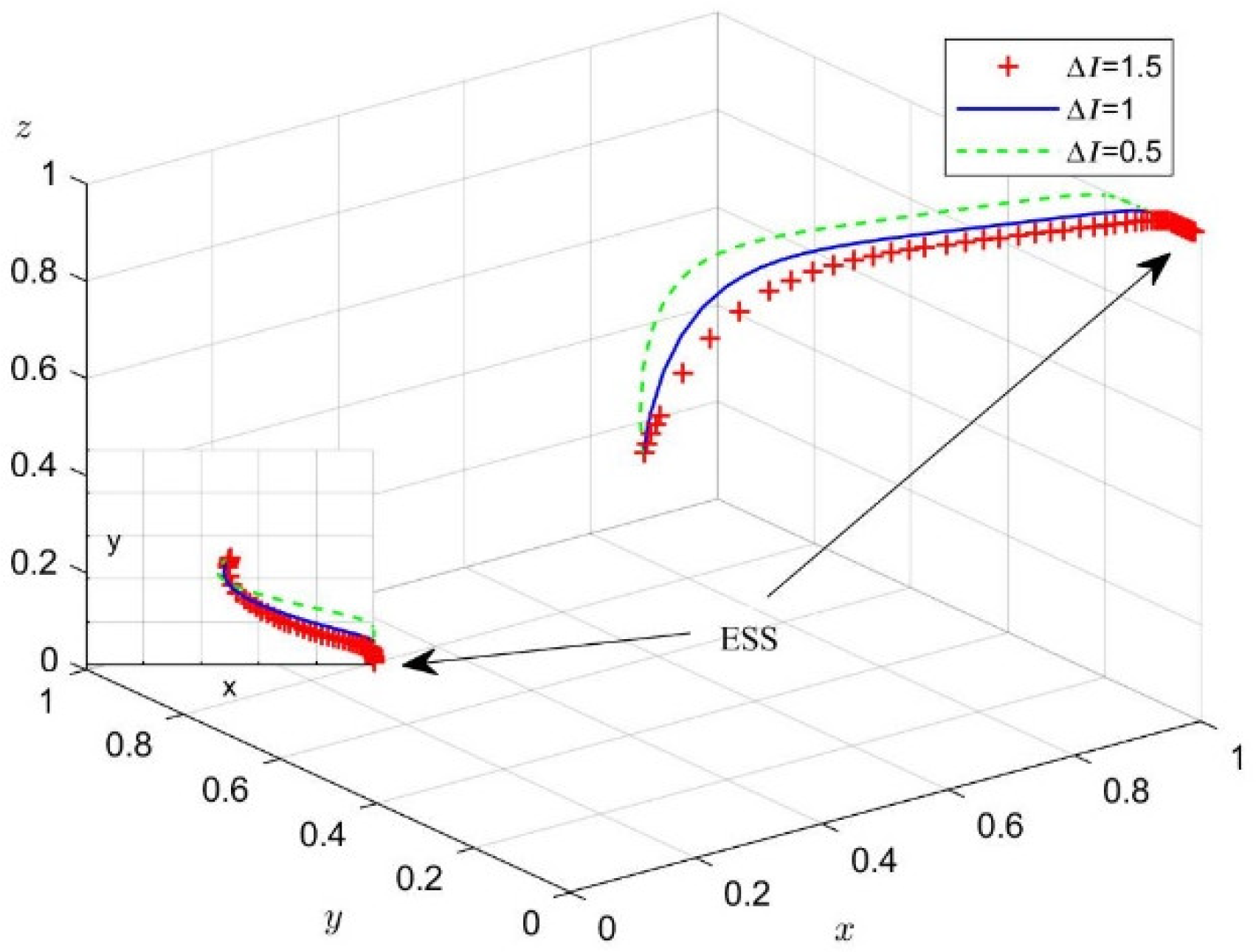
4.2. Punishment Analysis
4.3. Parametric Analysis of Coefficient Parameters
4.4. Reward Analysis
4.5. Cost Analysis
5. Discussion and Implications
5.1. Reward and Punishment Mechanism of LCTI
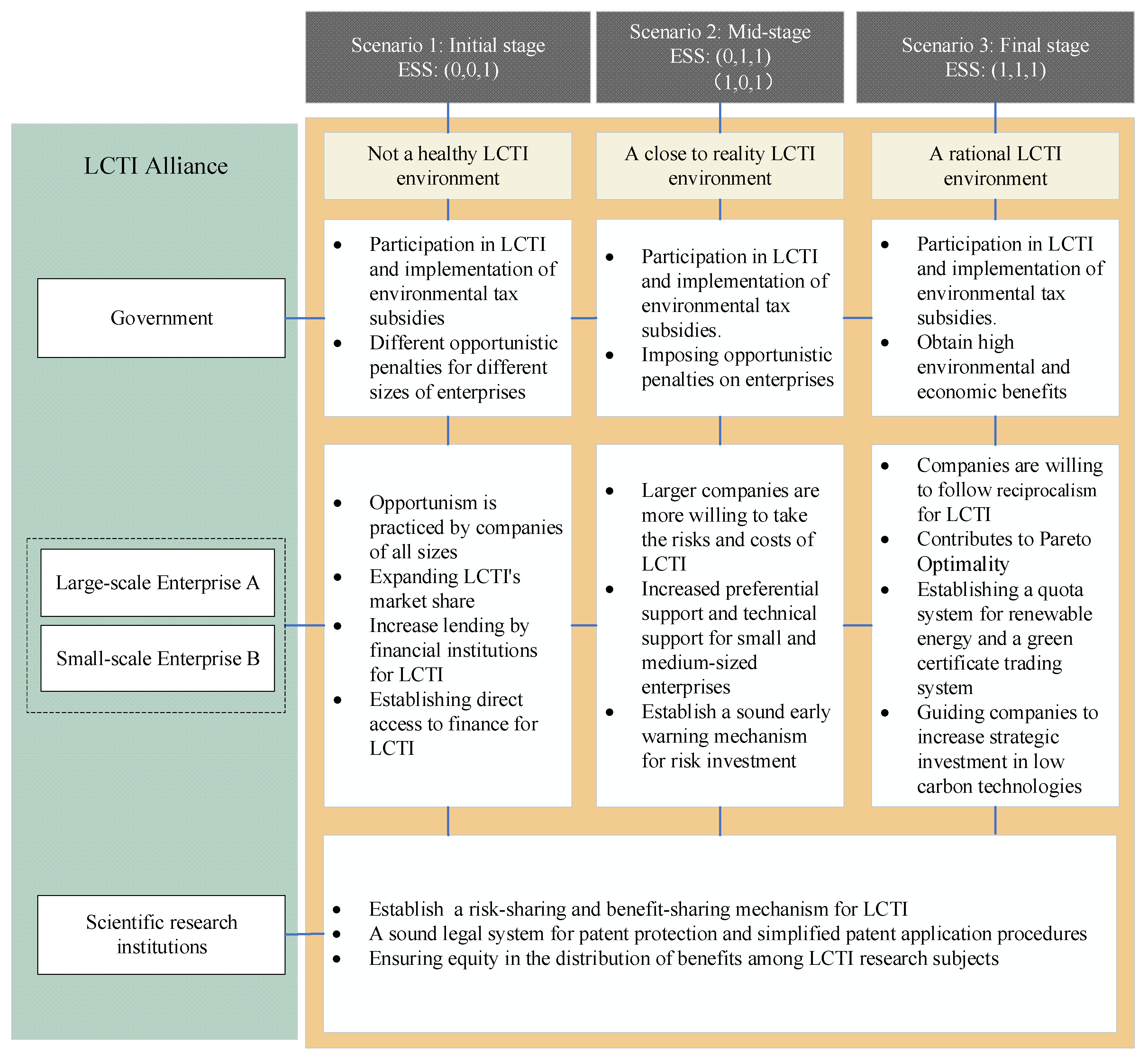
5.2. Policy Implications
6. Conclusions and Limitation
6.1. Conclusions
6.2. Limitation
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lai, X.D.; Zhan, W.L. Probing green technology innovation incentive mechanism: Gaming based on double information asymmetry. Shanghai Manag. Sci. 2022, 44, 43–48. [Google Scholar] [CrossRef]
- UNFCCC. Glasgow Climate Pact. Available online: https://unfccc.int/sites/default/files/resource/cma2021_L16_adv.pdf (accessed on 13 November 2021).
- World Meteorological Organization. State of the Global Climate 2021: WMO Provisional Report. 2021. Available online: https://library.wmo.int/doc_num.php?explnum_id=10859 (accessed on 18 May 2021).
- Shi, R.; Cui, Y.; Zhao, M. Role of low-carbon technology innovation in environmental performance of manufacturing: Evidence from OECD countries. Environ. Sci. Pollut. Res. 2021, 28, 68572–68584. [Google Scholar] [CrossRef] [PubMed]
- Liu, R.H.; Ding, M.L.; Wang, S.H. Thinking on the scientific and technological guidance of China’s carbon dioxide peak and carbon neutrality by European and American countries. Reform. Strategy 2022, 38, 1–14. [Google Scholar] [CrossRef]
- Shi, Y.; Han, B.; Zeng, Y. Simulating policy interventions in the interfirm diffusion of low-carbon technologies: An agent-based evolutionary game model. J. Clean. Prod. 2020, 250, 119449. [Google Scholar] [CrossRef]
- Gong, C.; Liu, J.; Chang, J. Evolutionary Game Analysis of the Innovation Behavior of High-Tech Enterprises with Government Participation. Math. Prob. Eng. 2021, 2021, 5528042. [Google Scholar] [CrossRef]
- Chen, W.; Hu, Z.H. Using evolutionary game theory to study governments and manufacturers’ behavioral strategies under various carbon taxes and subsidies. J. Clean. Prod. 2018, 201, 123–141. [Google Scholar] [CrossRef]
- Yang, N.; Song, Y.; Zhang, Y.; Wang, J. Dark side of joint R&D collaborations: Dependence asymmetry and opportunism. J. Bus. Ind. Mark. 2020, 35, 741–755. [Google Scholar] [CrossRef]
- Arıkan, A.T. Opportunism is in the eye of the beholder: Antecedents of subjective opportunism judgments. J. Bus. Ethics 2020, 161, 573–589. [Google Scholar] [CrossRef]
- Conner, K.R.; Prahalad, C.K. A resource-based theory of the firm: Knowledge versus opportunism. Organ. Sci. 1996, 7, 477–501. [Google Scholar] [CrossRef]
- Chai, L.; Li, J.; Clauss, T.; Tangpong, C. The influences of interdependence, opportunism and technology uncertainty on interfirm coopetition. J. Bus. Ind. Mark. 2019, 34, 948–964. [Google Scholar] [CrossRef]
- Das, T.K.; Rahman, N. Determinants of Partner Opportunism in Strategic Alliances: A Conceptual Framework. J. Bus. Psychol. 2010, 25, 55–74. [Google Scholar] [CrossRef]
- Indrawati, H.; Caska; Suarman. Barriers to technological innovations of SMEs: How to solve them? Int. J. Innov. Sci. 2020, 12, 545–564. [Google Scholar] [CrossRef]
- Wang, M.X.; Feng, X.L.; He, W.J. Economic policy of uncertainty and enterprise technological innovation: Mechanism analysis and empirical evidence. J. Southwest Univ. 2022, 48, 94–108. [Google Scholar] [CrossRef]
- Qian, H.X.; An, T.L. The Influence Mechanism of Financial Deepening on Technology Innovation in China. Nanjing J. Soc. Sci. 2022, 7, 50–60. [Google Scholar] [CrossRef]
- Guo, N.; Quan, Y. Practical exploration and thinking of local governments intervention in financial market construction in relatively poor counties—By taking inner mongolia autonomous region as an example. Inner Mongolia Soc. Sci. 2022, 43, 207–212. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, Y.; Wang, H.; Ouyang, X.; Xie, Y. Can green credit policy promote low-carbon technology innovation? J. Clean. Prod. 2022, 359, 132061. [Google Scholar] [CrossRef]
- Fan, R.; Chen, R.; Wang, Y.; Wang, D.; Chen, F. Simulating the impact of demand-side policies on low-carbon technology diffusion: A demand-supply coevolutionary model. J. Clean. Prod. 2022, 351, 131561. [Google Scholar] [CrossRef]
- Wang, D.; Huang, L.; Sun, Z.; Zhou, W.; Wang, W. The impact of environmental regulation on the innovation and diffusion of low-carbon technology in energy sector. IOP Conf. Ser. Earth Environ. Sci. 2021, 687, 012188. [Google Scholar] [CrossRef]
- Wang, W.; Lu, N.; Zhang, C. Low-carbon technology innovation responding to climate change from the perspective of spatial spillover effects. Chin. J. Popul. Resour. Environ. 2018, 16, 120–130. [Google Scholar] [CrossRef]
- Gao, S.; Wang, C. How to design emission trading scheme to promote corporate low-carbon technological innovation: Evidence from China. J. Clean. Prod. 2021, 298, 126712. [Google Scholar] [CrossRef]
- Zhang, X.; Geng, Y.; Tong, Y.W.; Kua, H.W.; Tian, X.; Wu, R.; Zhao, X.; Chiu, A.S. Spatial characteristics and its driving factors of low-carbon energy technology innovation in China: A gravity movement and exploratory spatial data analysis. J. Clean. Prod. 2021, 295, 126481. [Google Scholar] [CrossRef]
- Lyu, X.; Shi, A.; Wang, X. Research on the impact of carbon emission trading system on low-carbon technology innovation. Carbon Manag. 2020, 11, 183–193. [Google Scholar] [CrossRef]
- Ma, J.; Hu, Q.; Shen, W.; Wei, X. Does the low-carbon city pilot policy promote green technology innovation? based on green patent data of Chinese a-share listed companies. Int. J. Environ. Res. Public Health 2021, 18, 3695. [Google Scholar] [CrossRef]
- Cui, H.; Zhu, X.; Wang, H. Collaborative innovation of low-carbon technology from the triple helix perspective: Exploring critical success factors based on DEMATEL-ISM. Pol. J. Environ. Stud. 2020, 29, 1579–1592. [Google Scholar] [CrossRef]
- Jiang, Y.; Hu, Y.; Asante, D.; Ampaw, E.M.; Asante, B. The effects of executives’ low-carbon cognition on corporate low-carbon performance: A study of managerial discretion in China. J. Clean. Prod. 2022, 357, 132015. [Google Scholar] [CrossRef]
- Deng, Y.; You, D.; Zhang, Y. Research on improvement strategies for low-carbon technology innovation based on a differential game: The perspective of tax competition. Sustain. Prod. Consum. 2021, 26, 1046–1061. [Google Scholar] [CrossRef]
- Tang, F.C.; Ji, X.M.; Wang, H.Y. Governance Logic of Opportunism in Open Innovation—From the Perspective of Moral Decision. Forum Sci. Tech. Chin. 2021, 11, 69–77. [Google Scholar] [CrossRef]
- Liu, R.H.; Yang, Y.; Ding, M.L.; Wang, S.H. On construction of China’s green and low-carbon technology system and innovation path under the carbon peaking and carbon neutrality goals. Soc. Sci. Guangxi 2022, 4, 8–15. [Google Scholar] [CrossRef]
- Yang, X.; Guo, Y.; Liu, Q.; Zhang, D. Dynamic co-evolution analysis of low-carbon technology innovation compound system of new energy enterprise based on the perspective of sustainable development. J. Clean. Prod. 2022, 349, 131330. [Google Scholar] [CrossRef]
- Yuan, X.M.; Song, M.X.; Wei, F.L. Study on the evolution of innovation system based on industry-university-research knoeledge sharing. J. Yanshan Univ. 2022, 23, 87–96. [Google Scholar] [CrossRef]
- Dong, C.; Bi, K. On innovation performance of low-carbon technology breakthrough innovation network in manufacturing industry under the global value chain: A case study based on Chinese manufacturing industries. IEEE Access 2020, 8, 174080–174093. [Google Scholar] [CrossRef]
- Jiang, Y.; Chun, W.; Yang, Y. The effects of external relations network on low-carbon technology innovation: Based on the study of knowledge absorptive capacity. Sustainability 2018, 10, 155. [Google Scholar] [CrossRef]
- Zhang, X.; Geng, Y.; Tong, Y.W.; Kua, H.W.; Dong, H.; Pan, H. Trends and driving forces of low-carbon energy technology innovation in China’s industrial sectors from 1998 to 2017: From a regional perspective. Front. Energy 2021, 15, 473–486. [Google Scholar] [CrossRef]
- Lai, X.; Liu, J.; Shi, Q.; Georgiev, G.; Wu, G. Driving forces for low carbon technology innovation in the building industry: A critical review. Renew. Sustain. Energy Rev. 2017, 74, 299–315. [Google Scholar] [CrossRef]
- Zhao, X.; Bai, Y.; Ding, L. Incentives for personal carbon account: An evolutionary game analysis on public-private-partnership reconstruction. J. Clean. Prod. 2021, 282, 125358. [Google Scholar] [CrossRef]
- Yuan, X.; Zheng, C. Evolutionary game and simulation analysis of low-carbon technology innovation with multi-agent participation. IEEE Access 2022, 10, 11284–11295. [Google Scholar] [CrossRef]
- Zhou, K.; Ren, T. Low-carbon technology collaborative innovation in industrial cluster with social exclusion: An evolutionary game theory perspective. Chaos 2021, 31, 033124. [Google Scholar] [CrossRef]
- Friedman, D. Evolutionary games in economics. Econometrica 1991, 59, 637–666. [Google Scholar] [CrossRef]
- He, R.; Gonzalez, H. Numerical Synthesis of Pontryagin Optimal Control Minimizers Using Sampling-Based Methods. In Proceedings of the IEEE 56th Annual Conference on Decision and Control (CDC), Melbourne, Australia, 12–15 December 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 733–738. [Google Scholar]
- Xiao, H.J.; Yu, F.W.; Tang, H.L.; Peng, D.H.; Zhou, J.H. A study on the evolutionary game of low carbon environmental friendly technology under the collaborative innovation of government industry school research finance. Oper. Res. Manag. Sci. 2021, 30, 39–46. [Google Scholar] [CrossRef]
- Yuan, X.M.; Zheng, C.C. Research on evolutionary game of low-carbon technology collaborative innovation based on tripartite game. Math. Pract. Theory 2022, 52, 31–43. [Google Scholar]
- He, R.; Gonzalez, H. Zoned HVAC Control via PDE-Constrained Optimization. In Proceedings of the American Control Conference (ACC), Boston, MA, USA, 6–8 July 2016; IEEE: Piscataway, NJ, USA, 2016; Volume 7, pp. 587–592. [Google Scholar]
- Lv, B.L.; Zhao, G.B.; Zhang, Y. On the Existence of Mutual Benefit Cooperative Innovation of Industry-University-Research in China. Value Eng. 2019, 38, 87–88. [Google Scholar] [CrossRef]
- Yuan, C.; Yu, H.L.; Yang, D.L. Research on the Evolution of Enterprise Cooperative Innovation Behavior with Strong Reciprocity Theory. Oper. Res. Manag. Sci. 2017, 26, 169–175. [Google Scholar] [CrossRef]
- Li, F.; Tu, R.N.; Jia, M.X. Research on the implicit knowledge transfer incentive mechanism within the scientific research innovation team of colleges and universities under reciprocal preference. J. Jiangsu Univ. Sci. Tech. 2019, 33, 74–81. [Google Scholar] [CrossRef]
- Ji, L.Y.; Liu, L.C.; Zhang, Y.; Zhang, J.T. Evolutionary Game Analysis of Collaborative Innovation of Industry—University Cooperation under the Preference of Reciprocity. Sci. Tech. Manag. Res. 2018, 38, 74–78. [Google Scholar] [CrossRef]
- Yin, S. The Digital Green Innovation of Manufacturing Enterprise Cooperation Based on Reciprocity Theory: Research Status, Analytical Framework and Methodologies. J. Hebei Agric. Univ. 2022, 24, 21–31. [Google Scholar] [CrossRef]
- Zhang, S.; Zhu, L. Coregulation Supervision Strategy of Drug Enterprises under the Government Reward and Punishment Mechanism. Complexity 2021, 5865299. [Google Scholar] [CrossRef]
- Zhou, K.; Wang, Q.; Tang, J. Tripartite evolutionary game and simulation analysis of coal mining safe production supervision under the Chinese central government’s reward and punishment mechanism. Math. Probl. Eng. 2021, 2021, 5298890. [Google Scholar] [CrossRef]
- Xue, H.T. Utilization and management of carbon market revenue: European and American practice and its reference to China. J. Chin. Univ. Geosci. 2022, 22, 77–89. [Google Scholar] [CrossRef]


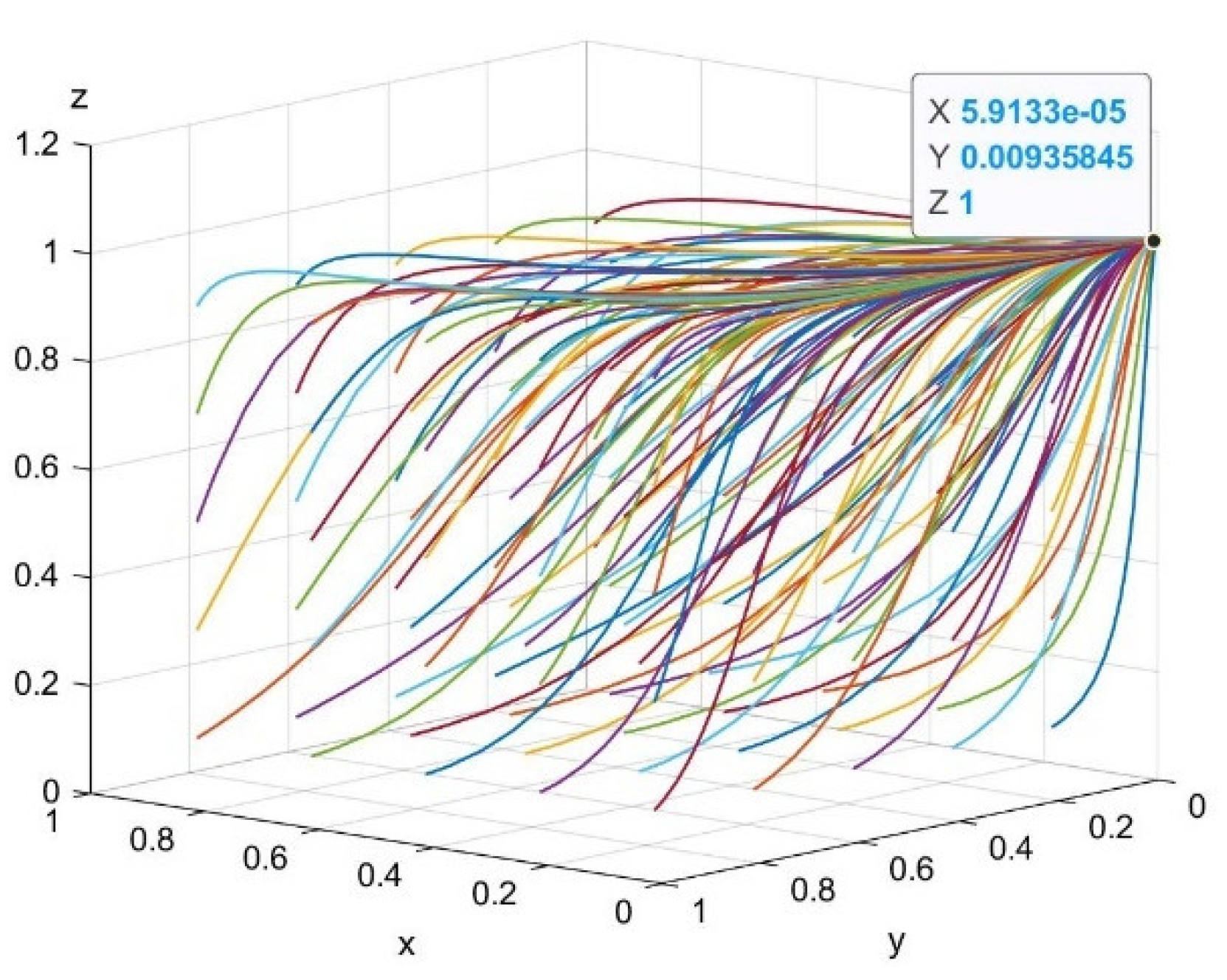

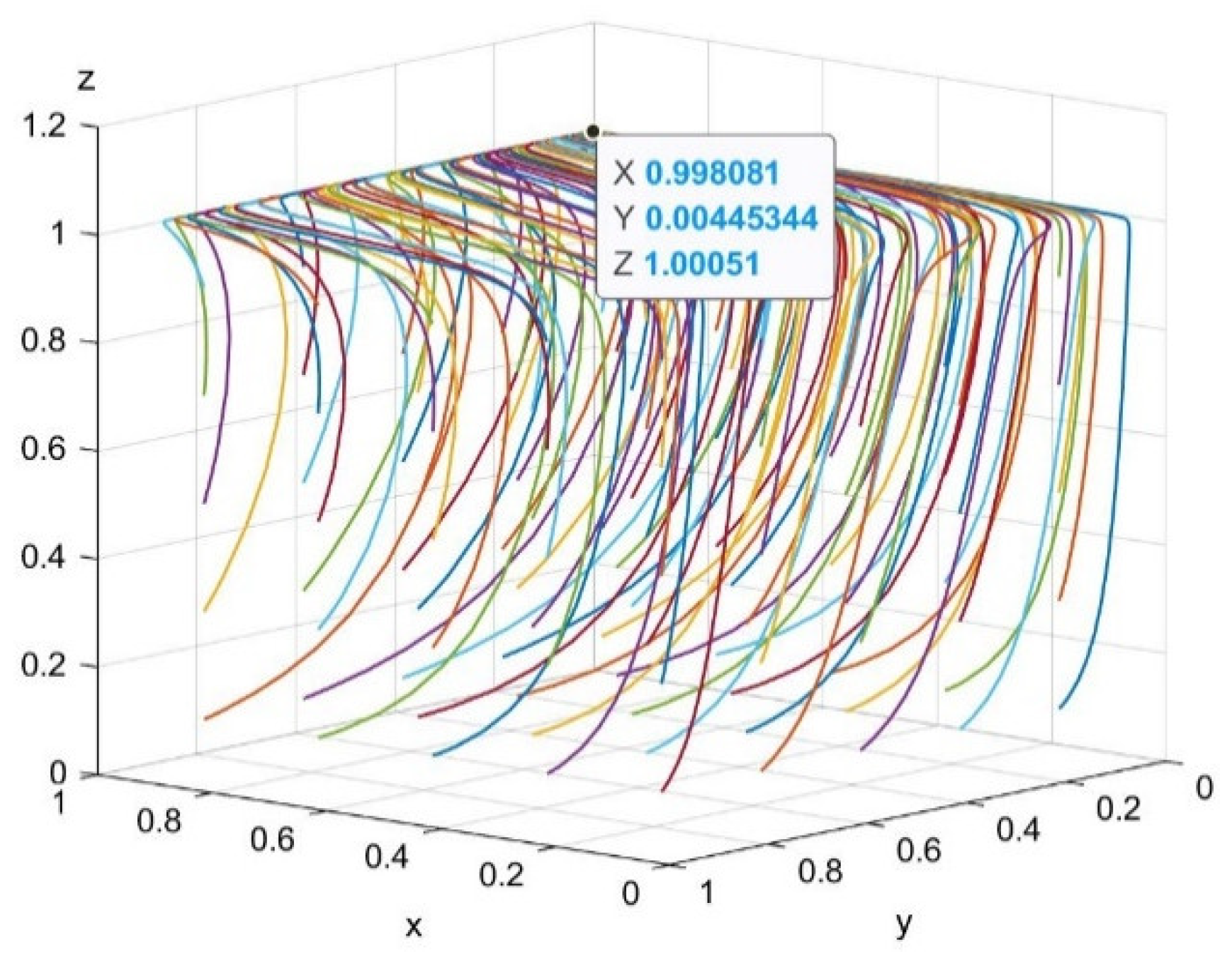
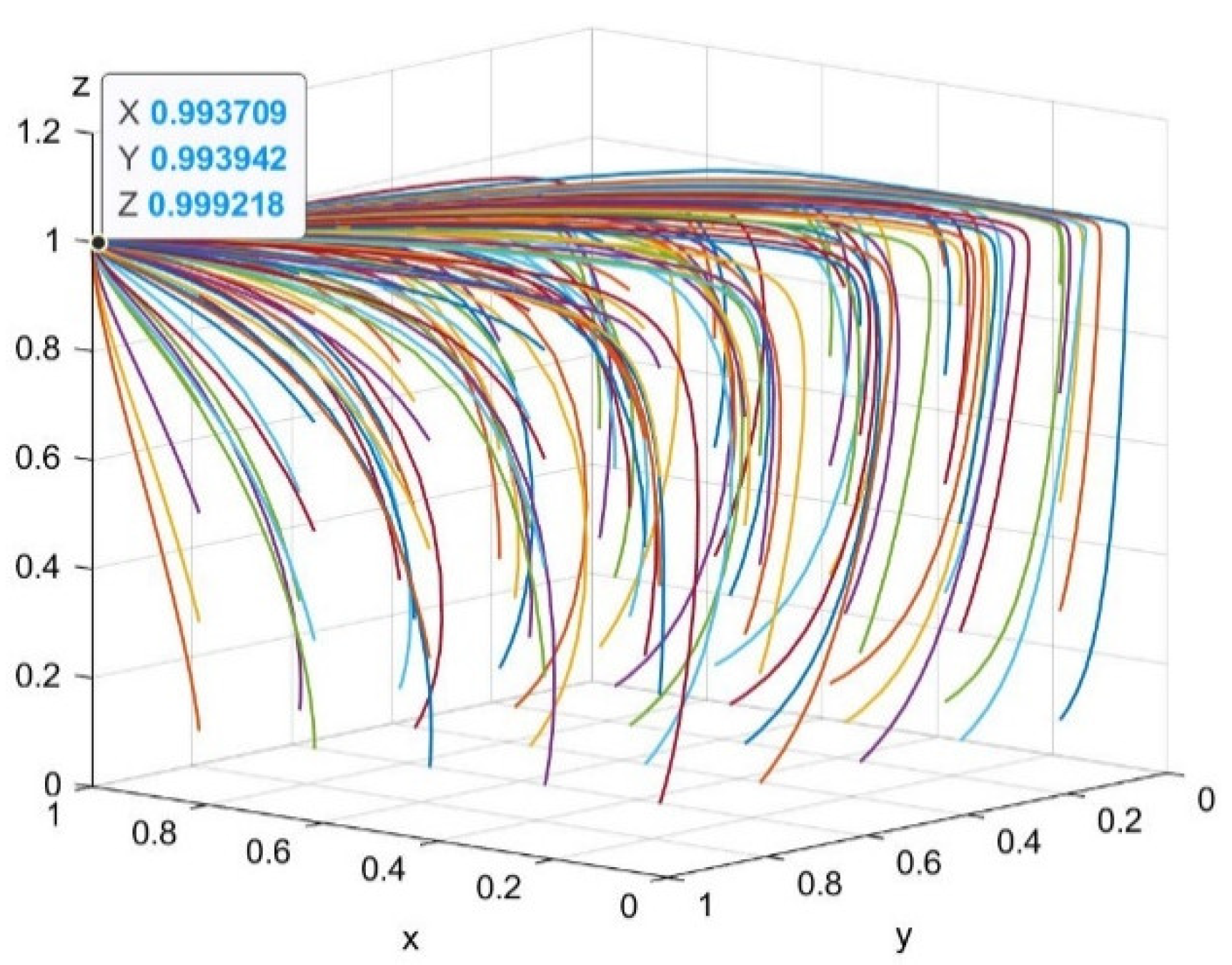
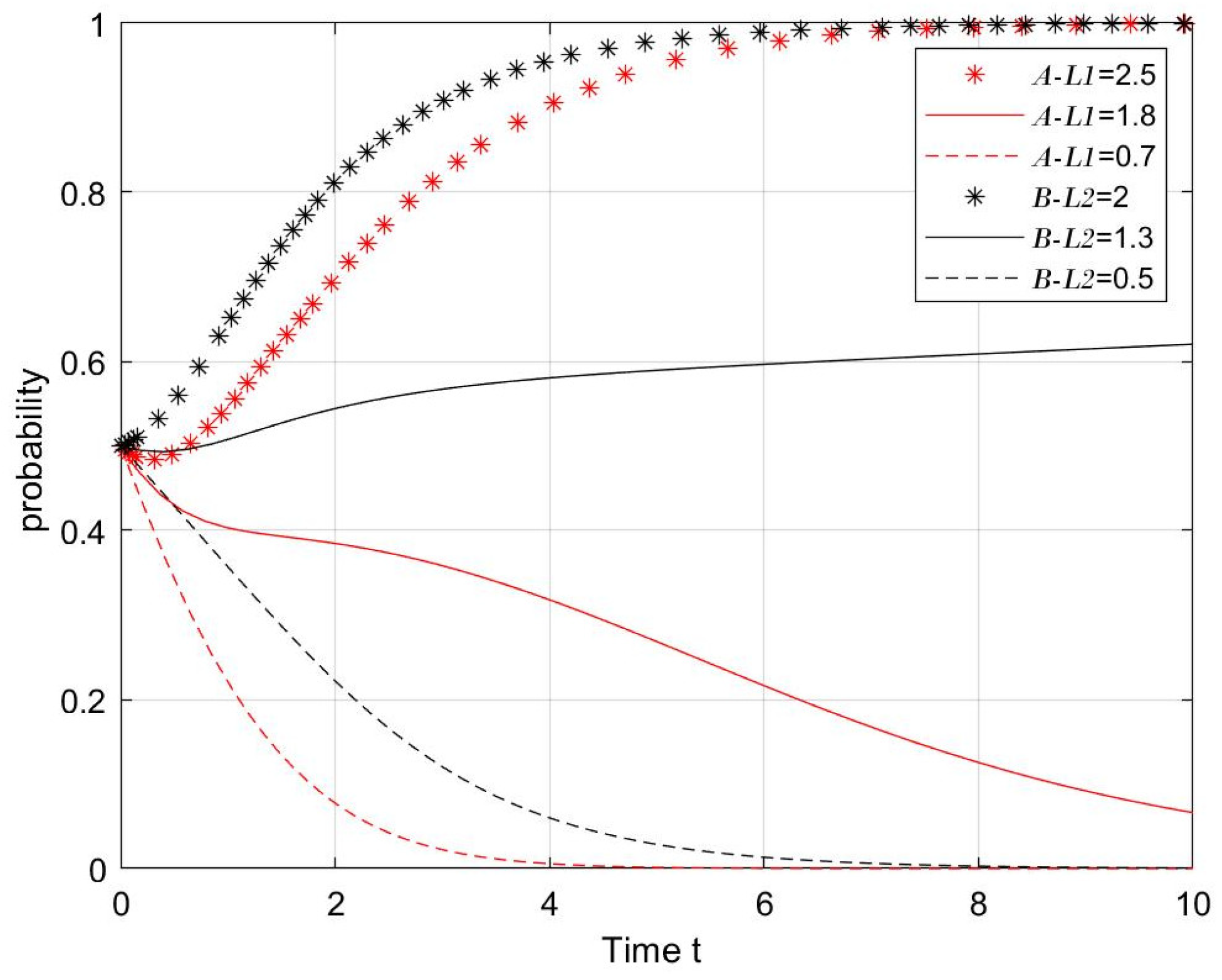


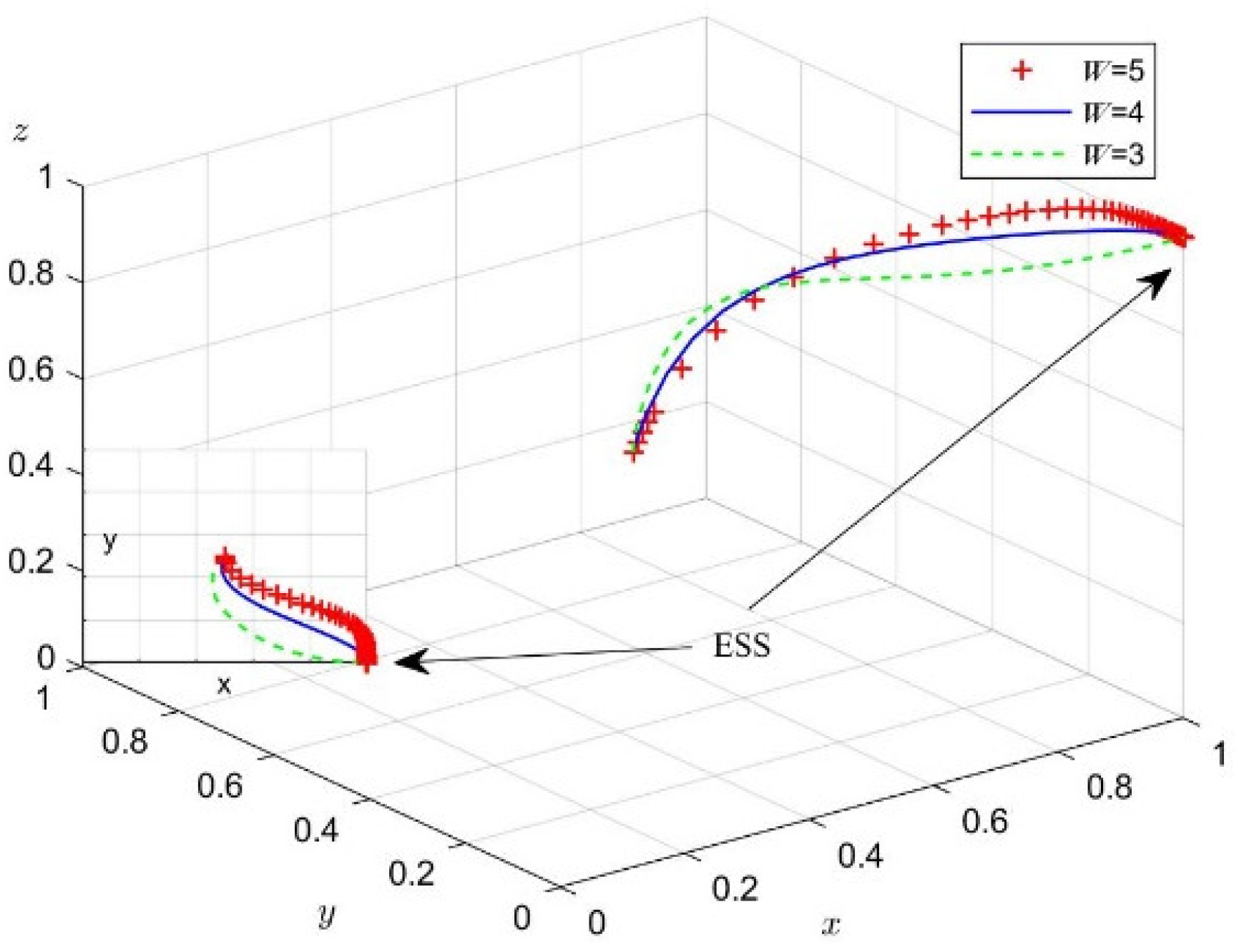

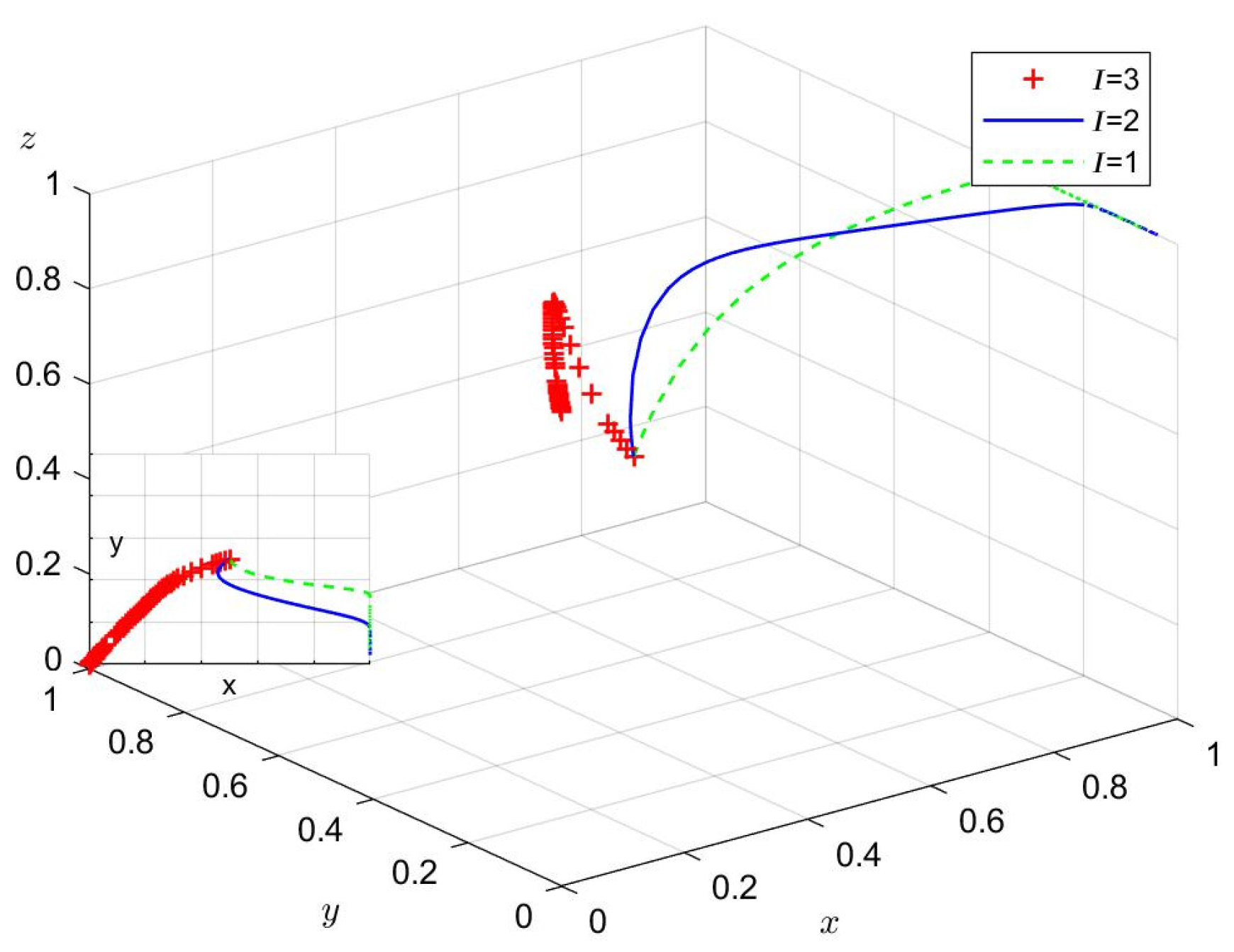
| Parameters | Meanings |
|---|---|
| Market value of LCTI. | |
| Extra gains earned by firms from total market returns when they choose opportunism (hence also the losses incurred by reciprocal firms). | |
| Total cost of investment required for corporate LCTI. | |
| Additional cost to companies when they choose reciprocity for LCTI. | |
| Proportion of the share of enterprise A in the market. | |
| Government’s carbon tax subsidy for LCTI. | |
| Probability of LCTI success under reciprocity. | |
| Probability of LCTI success under opportunism. | |
| Environmental and economic gains for the government when participating in LCTI. | |
| Additional benefits to the government when both enterprises A and B practice reciprocity. | |
| Additional benefits to the government when only one enterprise chooses reciprocity. | |
| Government penalties for enterprise A when it chooses opportunism. | |
| Government penalties for enterprise B when it chooses opportunism. | |
| Environmental and economic losses incurred by the government when one business chooses opportunism and the other reciprocity. | |
| Environmental and economic gains for the government when both enterprises choose opportunism. |
| Enterprise A | Enterprise B | Government | |
|---|---|---|---|
| Participation | Non-Participation | ||
| Reciprocity | Reciprocity | ||
| Opportunism | |||
| Opportunism | Reciprocity | ||
| Opportunism | |||
| Strategy Combination | Enterprise A | Enterprise B | Government |
|---|---|---|---|
| Equilibrium Points | Eigenvalues | Eigenvalue Symbol | State | Stability Condition |
|---|---|---|---|---|
| uncertain | Unstable point | — | ||
| uncertain | ||||
| uncertain | ESS | ① | ||
| uncertain | ||||
| uncertain | Unstable point | — | ||
| uncertain | ||||
| uncertain | ESS | ② | ||
| uncertain | ||||
| uncertain | Unstable point | — | ||
| uncertain | ||||
| uncertain | ESS | ③ | ||
| uncertain | ||||
| uncertain | Unstable point | — | ||
| uncertain | ||||
| uncertain | ESS | ④ | ||
| uncertain | ||||
| eigenvalues with different valence | Saddle point | |||
| Case 1 | 4 | 0.5 | 2 | 1 | 0.6 | 0.4 | 0.55 | 0.45 | 0.7 | 0.5 | 1 | 1 | 2 |
| Case 2 | 3.5 | 0.7 | 2 | 0.5 | 0.6 | 0.6 | 0.6 | 0.4 | 1.2 | 1 | 1 | 1 | 2 |
| Case 3 | 4 | 1.5 | 2 | 0.5 | 0.6 | 0.5 | 0.6 | 0.2 | 2.5 | 0.5 | 1 | 1 | 2 |
| Case 4 | 4 | 0.4 | 2 | 1.8 | 0.6 | 0.5 | 0.6 | 0.3 | 1.5 | 1 | 1 | 1 | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; He, R.; Ren, K.; He, Y.; Zhou, J. Stability Analysis of Low-Carbon Technology Innovation Cooperation under a Reward and Punishment Mechanism. Systems 2022, 10, 118. https://doi.org/10.3390/systems10040118
Wang M, He R, Ren K, He Y, Zhou J. Stability Analysis of Low-Carbon Technology Innovation Cooperation under a Reward and Punishment Mechanism. Systems. 2022; 10(4):118. https://doi.org/10.3390/systems10040118
Chicago/Turabian StyleWang, Min, Runxin He, Kai Ren, Youshi He, and Jianya Zhou. 2022. "Stability Analysis of Low-Carbon Technology Innovation Cooperation under a Reward and Punishment Mechanism" Systems 10, no. 4: 118. https://doi.org/10.3390/systems10040118
APA StyleWang, M., He, R., Ren, K., He, Y., & Zhou, J. (2022). Stability Analysis of Low-Carbon Technology Innovation Cooperation under a Reward and Punishment Mechanism. Systems, 10(4), 118. https://doi.org/10.3390/systems10040118





