The Significance of Multi-Size Carbon Fibers on the Mechanical and Fracture Characteristics of Fiber Reinforced Cement Composites
Abstract
:1. Introduction
2. Experimental Program
2.1. Materials
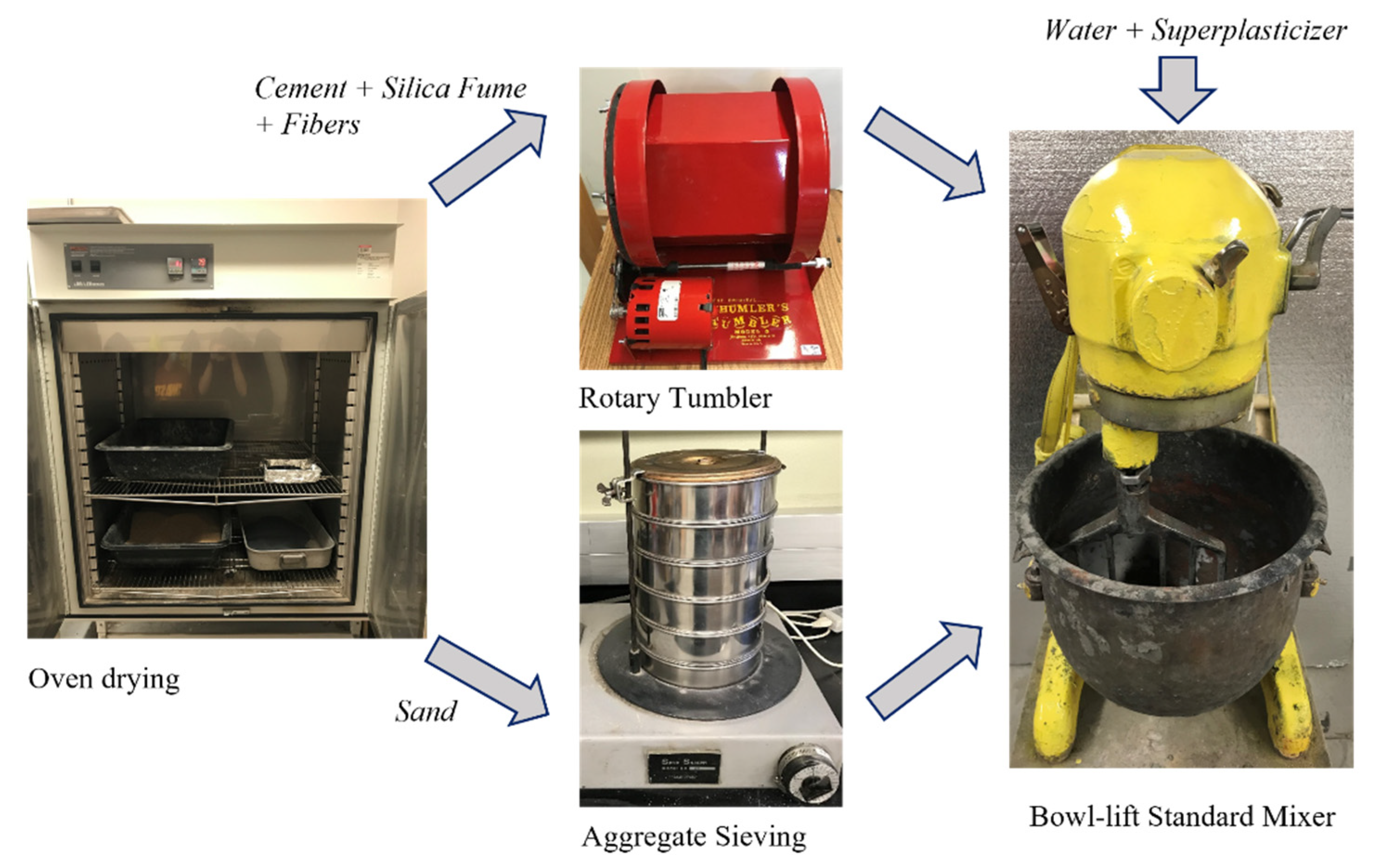
2.2. Mixing Protocol
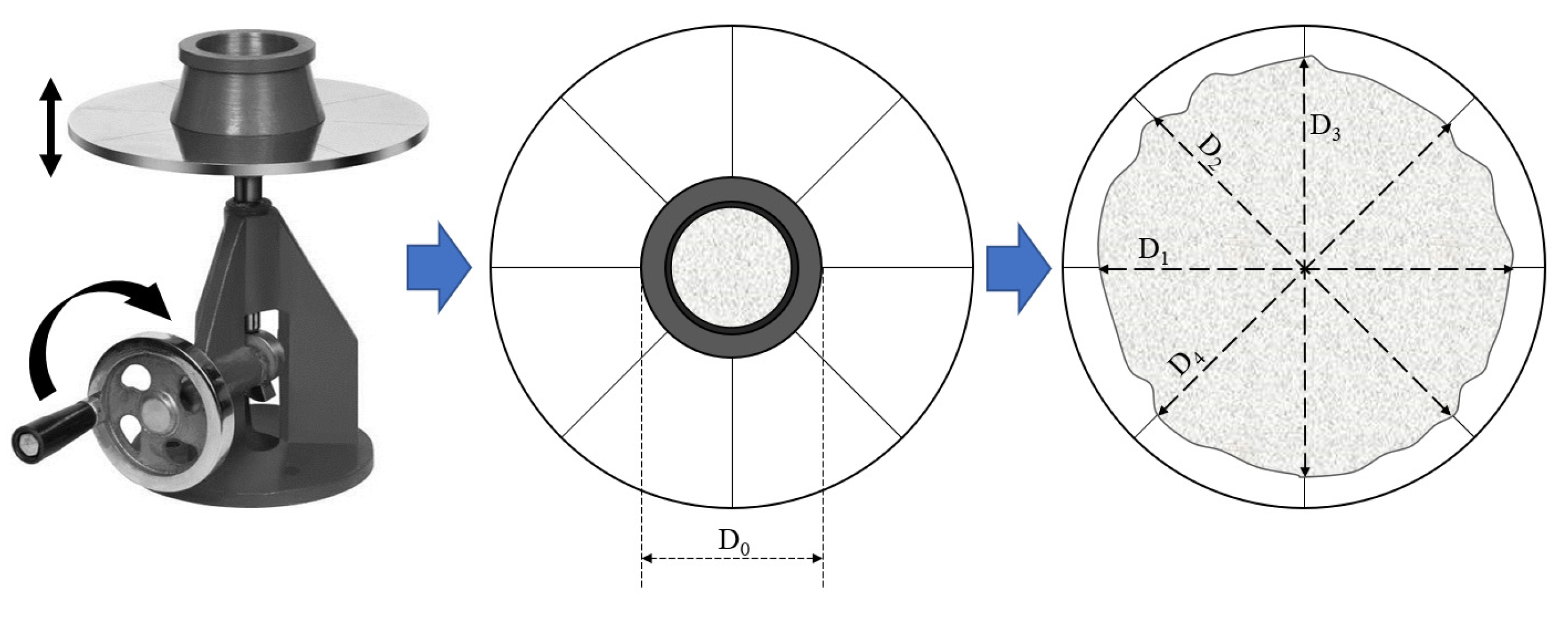
2.3. Flowability Test
2.4. Uniaxial Compression Test
2.5. Modulus of Rupture Test
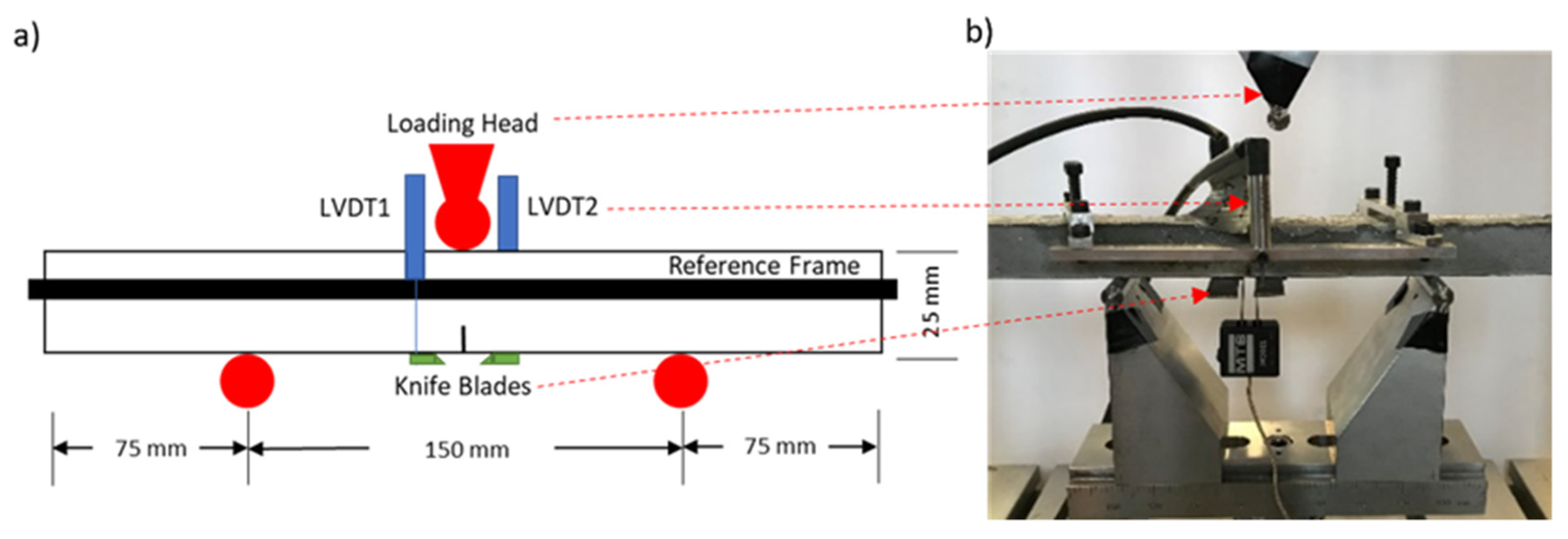
2.6. Fracture Toughness Test
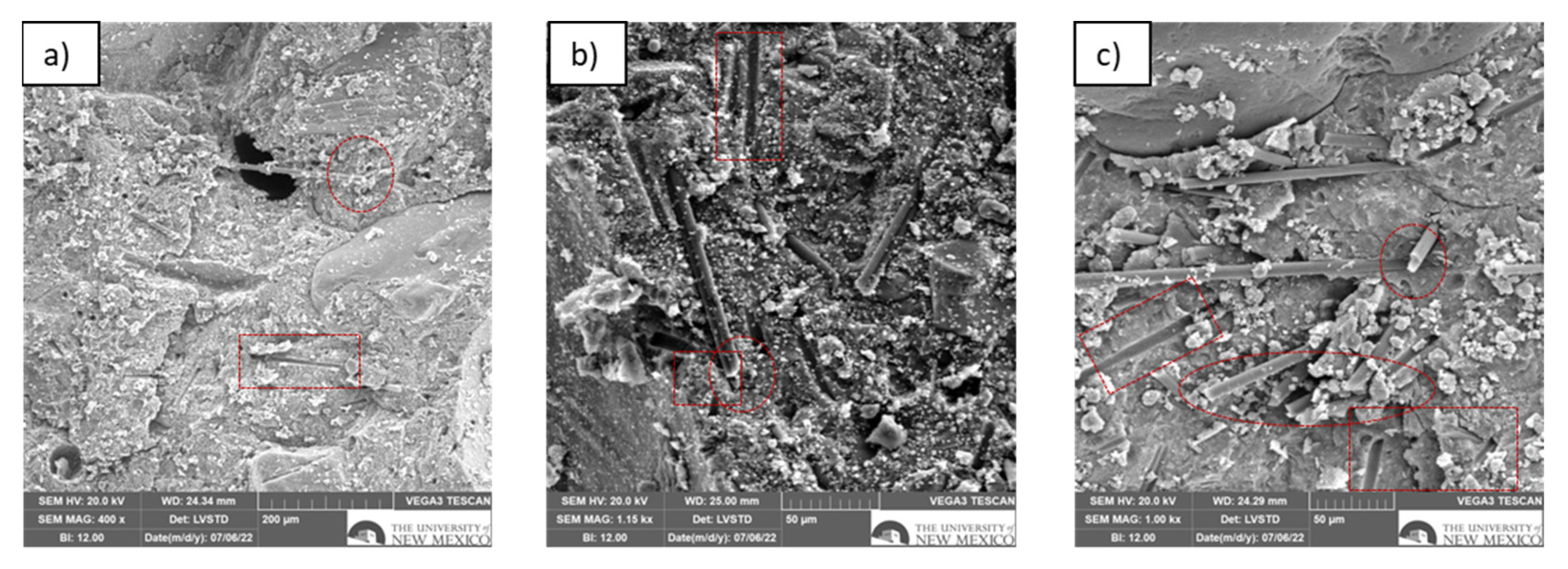
2.7. Scanning Electron Microscopy (SEM)
3. Results and Discussion
3.1. Flowability
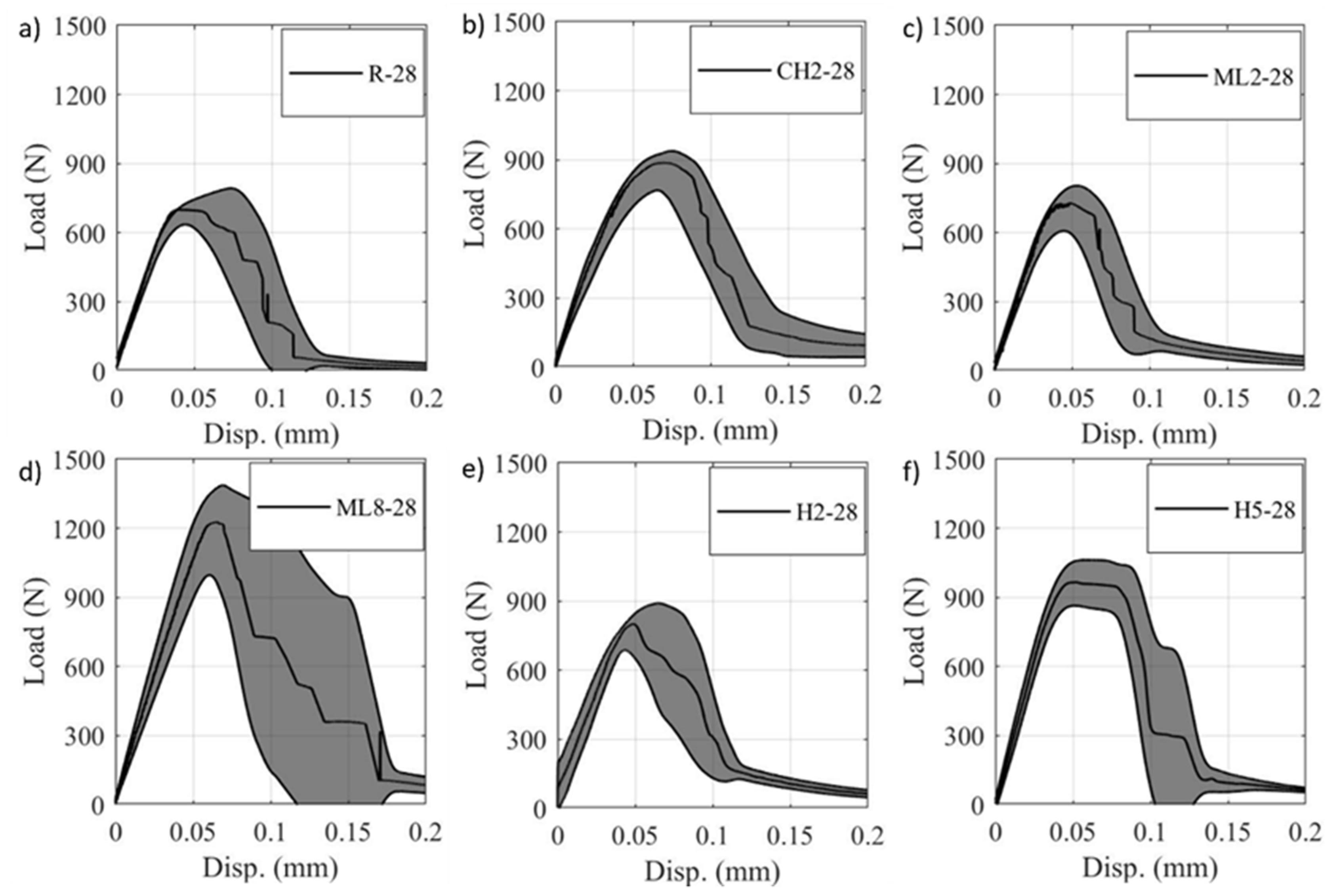
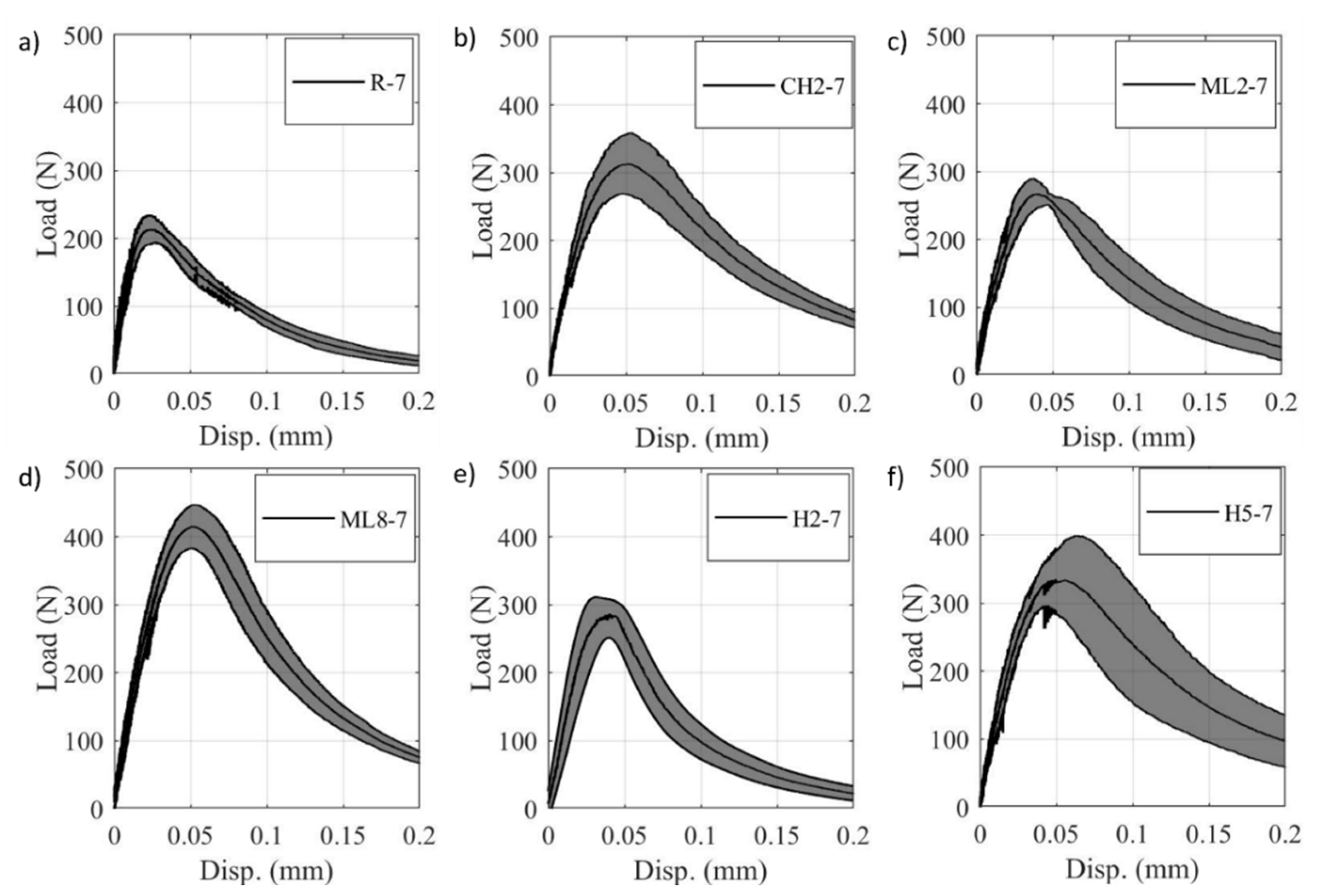
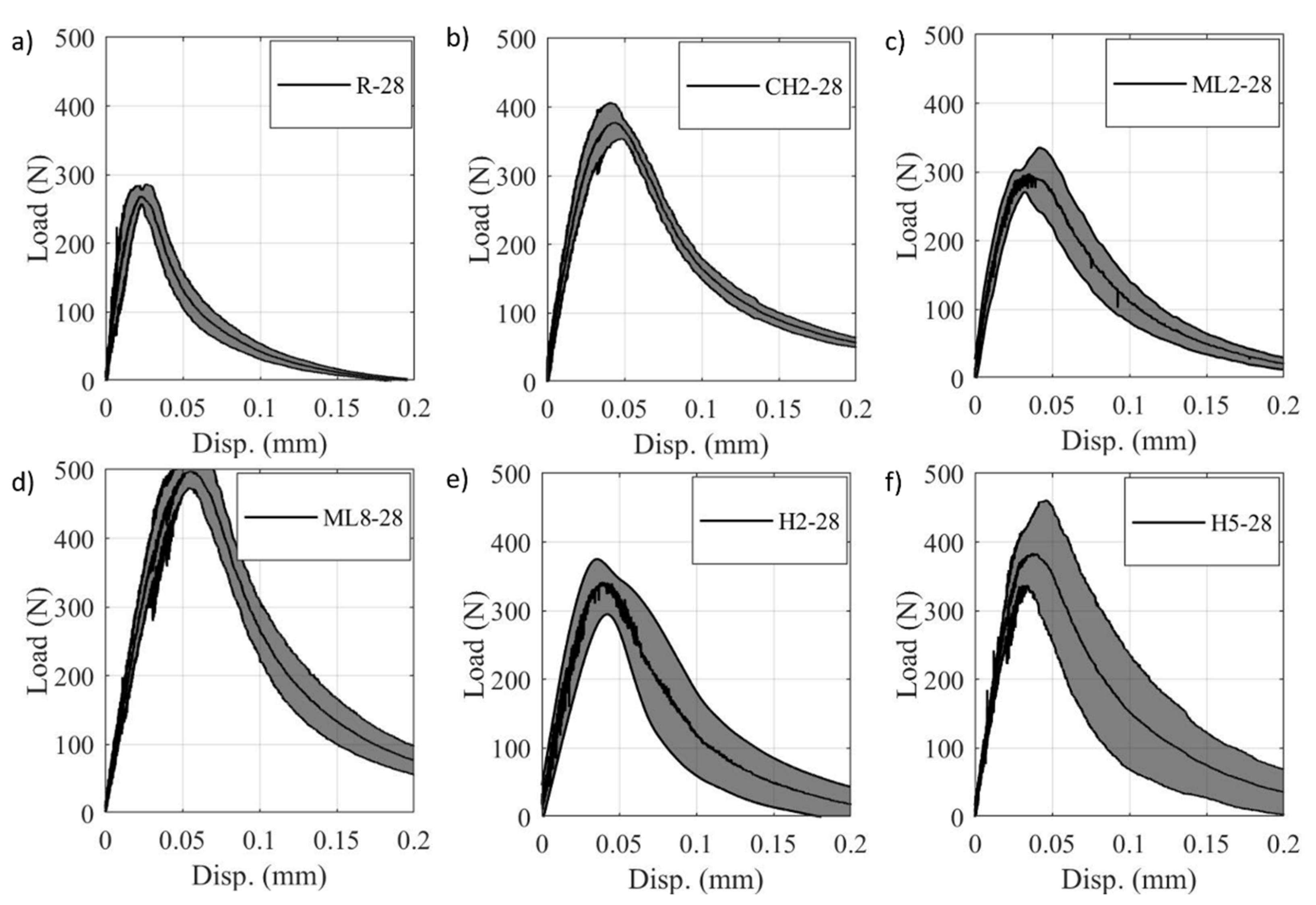
3.2. Mechanical Properties
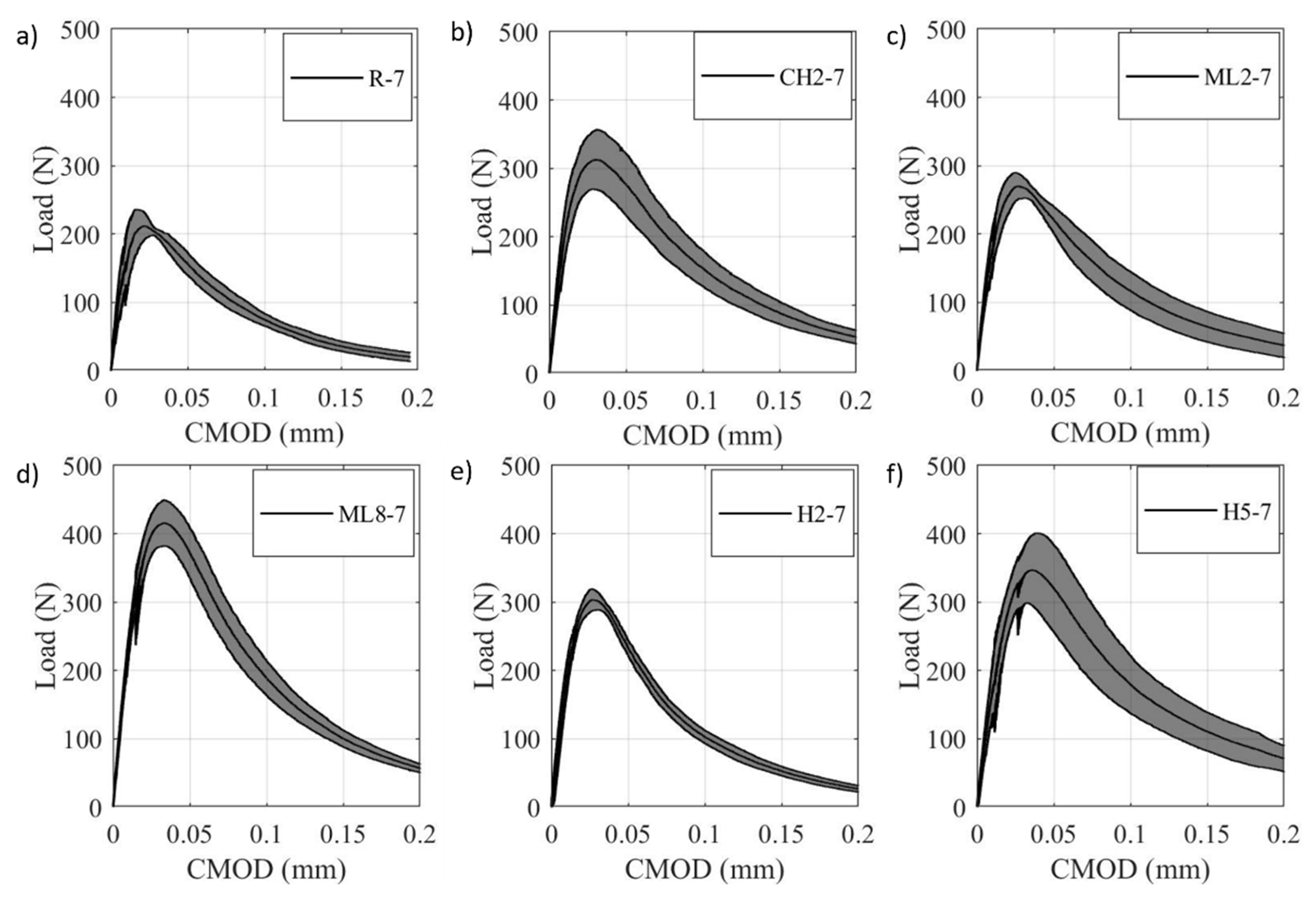
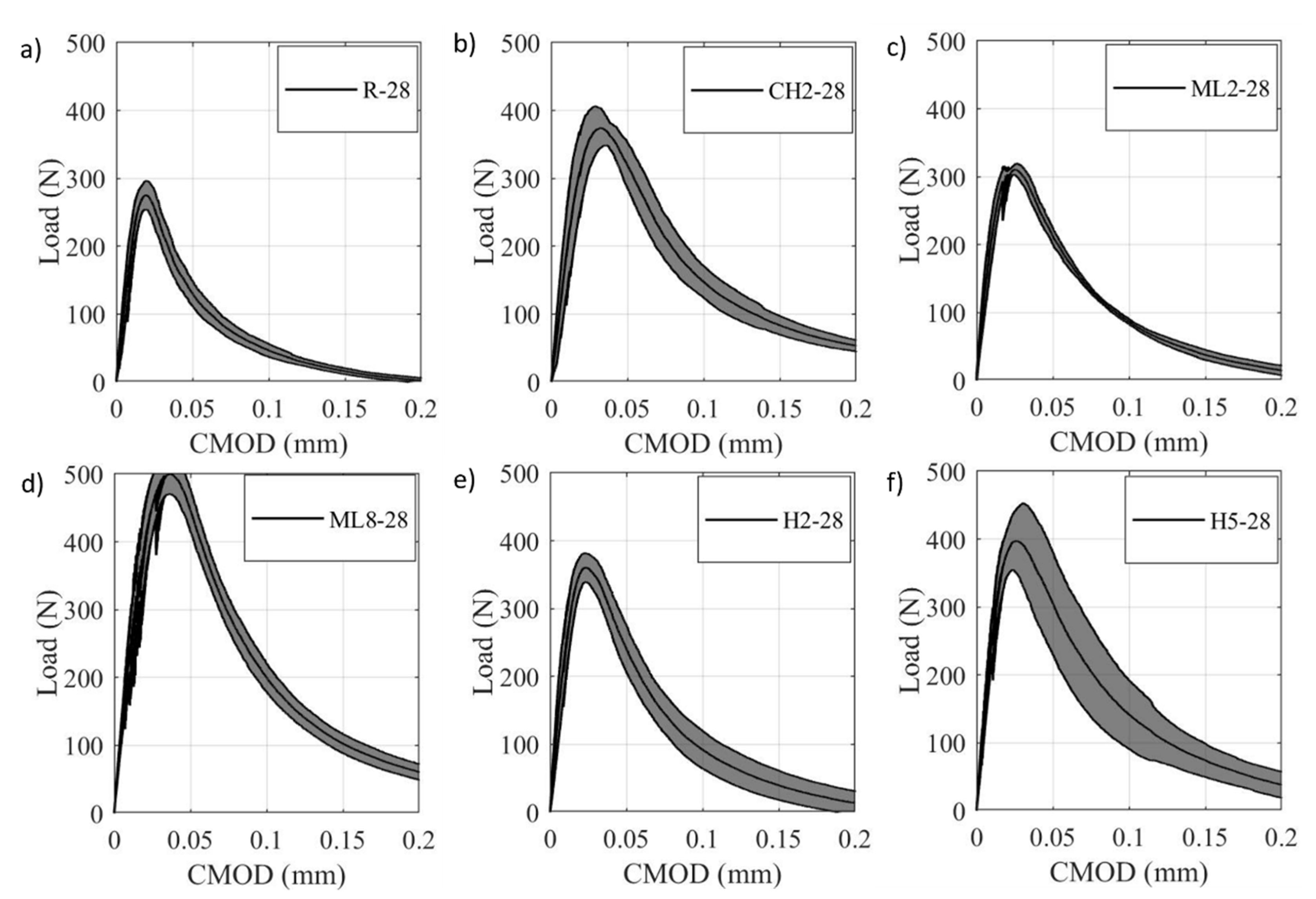
3.3. Fracture Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Shi, T.; Li, Z.; Guo, J.; Gong, H.; Gu, C. Research progress on CNTs/CNFs-modified cement-based composites—A review. Constr. Build. Mater. 2019, 202, 290–307. [Google Scholar] [CrossRef]
- Hogancamp, J.; Grasley, Z. The use of microfine cement to enhance the efficacy of carbon nanofibers with respect to drying shrinkage crack resistance of portland cement mortars. Cem. Concr. Compos. 2017, 83, 405–414. [Google Scholar] [CrossRef]
- Taha, M.M.R.; Shrive, N.G. Enhancing Fracture Toughness of High-Performance Carbon Fiber Cement Composites. ACI Mater. J. 2001, 98, 168–178. [Google Scholar] [CrossRef]
- Cao, J.; Chung, D. Improving the dispersion of steel fibers in cement mortar by the addition of silane. Cem. Concr. Res. 2001, 31, 309–311. [Google Scholar] [CrossRef]
- Chung, D. Comparison of submicron-diameter carbon filaments and conventional carbon fibers as fillers in composite materials. Carbon N. Y. 2001, 39, 1119–1125. [Google Scholar] [CrossRef]
- Lok, T.S.; Xiao, J.R. Flexural Strength Assessment of Steel Fiber Reinforced Concrete. J. Mater. Civ. Eng. 1999, 11, 188–196. [Google Scholar] [CrossRef]
- Saccani, A.; Manzi, S.; Lancellotti, I.; Lipparini, L. Composites obtained by recycling carbon fibre/epoxy composite wastes in building materials. Constr. Build. Mater. 2019, 204, 296–302. [Google Scholar] [CrossRef]
- Petersen; Link, R.; Eren, O.; Marar, K.; Çelik, T. Effects of Silica Fume and Steel Fibers on Some Mechanical Properties of High-Strength Fiber-Reinforced Concrete. J. Test. Eval. 1999, 27, 380. [Google Scholar] [CrossRef] [Green Version]
- Rieger, C.; Van Mier, J.G. Pullout of microfibers from hardened cement paste. In Advances in Cement-Based Materials; CRC Press: Boca Raton, FL, USA, 2009; pp. 67–73. [Google Scholar]
- Zheng, Z.; Feldman, D. Synthetic fibre-reinforced concrete. Prog. Polym. Sci. 1995, 20, 185–210. [Google Scholar] [CrossRef]
- Aveston, J.; Kelly, A. Theory of multiple fracture of fibrous composites. J. Mater. Sci. 1973, 8, 352–362. [Google Scholar] [CrossRef]
- Morris, A.; Garrett, G. A comparative study of the static and fatigue behaviour of plain and steel fibre reinforced mortar in compression and direct tension. Int. J. Cem. Compos. Light. Concr. 1981, 3, 73–91. [Google Scholar] [CrossRef]
- Jhatial, A.A.; Sohu, S.; Bhatti, N.-U.; Lakhiar, M.T.; Oad, R. Effect of steel fibres on the compressive and flexural strength of concrete. Int. J. Adv. Appl. Sci. 2018, 5, 16–21. [Google Scholar] [CrossRef]
- Barr, B.; Asghari, A.; Hughes, T. Tensile strength and toughness of FRC materials. Int. J. Cem. Compos. Light. Concr. 1988, 10, 101–107. [Google Scholar] [CrossRef]
- Hogancamp, J.R. The Use of Microfine Cement to Enhance the Efficacy of Carbon Nanofibers and Microfibers in Portland Cement Mortars. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2017. [Google Scholar]
- Tyson, B.M.; Abu Al-Rub, R.K.; Yazdanbakhsh, A.; Grasley, Z. Carbon Nanotubes and Carbon Nanofibers for Enhancing the Mechanical Properties of Nanocomposite Cementitious Materials. J. Mater. Civ. Eng. 2011, 23, 1028–1035. [Google Scholar] [CrossRef]
- Metaxa, Z.S.; Konsta-Gdoutos, M.S.; Shah, S.P. Carbon nanofiber cementitious composites: Effect of debulking procedure on dispersion and reinforcing efficiency. Cem. Concr. Compos. 2013, 36, 25–32. [Google Scholar] [CrossRef]
- Yazdanbakhsh, A.; Grasley, Z.; Tyson, B.; Abu Al-Rub, R. Challenges and Benefits of Utilizing Carbon Nanofilaments in Cementitious Materials. J. Nanomater. 2012, 2012, 371927. [Google Scholar] [CrossRef] [Green Version]
- Nasibulina, L.I.; Anoshkin, I.V.; Nasibulin, A.; Cwirzen, A.; Penttala, V.; Kauppinen, E. Effect of Carbon Nanotube Aqueous Dispersion Quality on Mechanical Properties of Cement Composite. J. Nanomater. 2012, 2012, 169262. [Google Scholar] [CrossRef] [Green Version]
- Sbia, L.A.; Peyvandi, A.A.; Soroushian, P.; Lu, J.; Balachandra, A.M. Enhancement of Ultrahigh Performance Concrete Material Properties with Carbon Nanofiber. Adv. Civ. Eng. 2014, 2014, 854729. [Google Scholar] [CrossRef]
- Konsta-Gdoutos, M.S.; Metaxa, Z.S.; Shah, S.P. Multi-scale mechanical and fracture characteristics and early-age strain capacity of high performance carbon nanotube/cement nanocomposites. Cem. Concr. Compos. 2010, 32, 110–115. [Google Scholar] [CrossRef]
- Metaxa, Z.S.; Seo, J.-W.T.; Konsta-Gdoutos, M.S.; Hersam, M.C.; Shah, S.P. Highly concentrated carbon nanotube admixture for nano-fiber reinforced cementitious materials. Cem. Concr. Compos. 2012, 34, 612–617. [Google Scholar] [CrossRef]
- Stynoski, P.; Mondal, P.; Marsh, C. Effects of silica additives on fracture properties of carbon nanotube and carbon fiber reinforced Portland cement mortar. Cem. Concr. Compos. 2015, 55, 232–240. [Google Scholar] [CrossRef]
- Zaheer, M.M.; Jafri, M.S.; Sharma, R. Effect of diameter of MWCNT reinforcements on the mechanical properties of cement composites. Adv. Concr. Constr. 2019, 8, 207–215. [Google Scholar] [CrossRef]
- Gdoutos, E.E.; Konsta-Gdoutos, M.S.; Danoglidis, P.A.; Shah, S.P. Advanced cement based nanocomposites reinforced with MWCNTs and CNFs. Front. Struct. Civ. Eng. 2016, 10, 142–149. [Google Scholar] [CrossRef]
- Musso, S.; Tulliani, J.-M.; Ferro, G.; Tagliaferro, A. Influence of carbon nanotubes structure on the mechanical behavior of cement composites. Compos. Sci. Technol. 2009, 69, 1985–1990. [Google Scholar] [CrossRef]
- Yazdanbakhsh, A.; Grasley, Z.; Tyson, B.; Abu Al-Rub, R.K. Distribution of Carbon Nanofibers and Nanotubes in Cementitious Composites. Transp. Res. Rec. J. Transp. Res. Board 2010, 2142, 89–95. [Google Scholar] [CrossRef] [Green Version]
- Brown, L.; Sanchez, F. Influence of carbon nanofiber clustering on the chemo-mechanical behavior of cement pastes. Cem. Concr. Compos. 2016, 65, 101–109. [Google Scholar] [CrossRef] [Green Version]
- Stephens, C.; Brown, L.; Sanchez, F. Quantification of the re-agglomeration of carbon nanofiber aqueous dispersion in cement pastes and effect on the early age flexural response. Carbon 2016, 107, 482–500. [Google Scholar] [CrossRef] [Green Version]
- Yazdanbakhsh, A.; Grasley, Z. Utilization of Silica Fume to Stabilize the Dispersion of Carbon Nanofilaments in Cement Paste. J. Mater. Civ. Eng. 2014, 26, 1–5. [Google Scholar] [CrossRef]
- Rossi, P. Influence of fibre geometry and matrix maturity on the mechanical performance of ultra high-performance cement-based composites. Cem. Concr. Compos. 2013, 37, 246–248. [Google Scholar] [CrossRef]
- Khandelwal, S.; Rhee, K.Y. Recent advances in basalt-fiber-reinforced composites: Tailoring the fiber-matrix interface. Compos. Part B Eng. 2020, 192, 108011. [Google Scholar] [CrossRef]
- Abu Al-Rub, R.; Tyson, B.M.; Yazdanbakhsh, A.; Grasley, Z. Mechanical Properties of Nanocomposite Cement Incorporating Surface-Treated and Untreated Carbon Nanotubes and Carbon Nanofibers. J. Nanomech. Micromech. 2012, 2, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Sanchez, F.; Zhang, L.; Ince, C. Multi-scale Performance and Durability of Carbon Nanofiber/Cement Composites. Nanotechnol. Constr. 2009, 3, 345–350. [Google Scholar] [CrossRef]
- Sbia, L.A.; Peyvandi, A.A.; Soroushian, P.; Balachandra, A.M. Optimization of ultra-high-performance concrete with nano- and micro-scale reinforcement. Cogent Eng. 2014, 1, 990673. [Google Scholar] [CrossRef]
- Finegan, I.C.; Tibbetts, G.G.; Glasgow, D.G.; Ting, J.-M.; Lake, M.L. Surface treatments for improving the mechanical properties of carbon nanofiber/thermoplastic composites. J. Mater. Sci. 2003, 38, 3485–3490. [Google Scholar] [CrossRef]
- Nasibulin, A.G.; Shandakov, S.D.; Nasibulina, L.I.; Cwirzen, A.; Mudimela, P.R.; Habermehl-Cwirzen, K.; Grishin, D.A.; Gavrilov, Y.V.; Malm, J.E.M.; Tapper, U.; et al. A novel cement-based hybrid material. New J. Phys. 2009, 11, 023013. [Google Scholar] [CrossRef]
- Nasibulin, A.; Koltsova, T.; Nasibulina, L.I.; Anoshkin, I.V.; Semencha, A.; Tolochko, O.V.; Kauppinen, E.I. A Novel Approach For Nanocarbon Composite Preparation. MRS Proc. 2012, 1454, 279–286. [Google Scholar] [CrossRef]
- Nasibulina, L.I.; Anoshkin, I.V.; Shandakov, S.D.; Nasibulin, A.G.; Cwirzen, A.; Mudimela, P.R.; Habermehl-Cwirzen, K.; Malm, J.E.M.; Koltsova, T.S.; Tian, Y.; et al. Direct Synthesis of Carbon Nanofibers on Cement Particles. Transp. Res. Rec. J. Transp. Res. Board 2010, 2142, 96–101. [Google Scholar] [CrossRef] [Green Version]
- Šmilauer, V.; Hlaváček, P.; Padevět, P. Micromechanical Analysis of Cement Paste with Carbon Nanotubes. Acta Polytech. 2012, 52, 22–28. [Google Scholar] [CrossRef]
- Thostenson, E.T.; Li, W.Z.; Wang, D.Z.; Ren, Z.F.; Chou, T.W. Carbon nanotube/carbon fiber hybrid multiscale composites. J. Appl. Phys. 2002, 91, 6034–6037. [Google Scholar] [CrossRef]
- Yazdanbakhsh, A.; Grasley, Z. The theoretical maximum achievable dispersion of nanoinclusions in cement paste. Cem. Concr. Res. 2012, 42, 798–804. [Google Scholar] [CrossRef]
- Yazdanbakhsh, A.; Grasley, Z.; Tyson, B.; Abu Al-Rub, R.K. Dispersion quantification of inclusions in composites. Compos. Part A Appl. Sci. Manuf. 2011, 42, 75–83. [Google Scholar] [CrossRef]
- Piggott, M.R. Theoretical estimation of fracture toughness of fibrous composites. J. Mater. Sci. 1970, 5, 669–675. [Google Scholar] [CrossRef]
- Shu, X.; Graham, R.K.; Huang, B.; Burdette, E.G. Hybrid effects of carbon fibers on mechanical properties of Portland cement mortar. Mater. Des. 2015, 65, 1222–1228. [Google Scholar] [CrossRef]
- Ahmed, H.; Bogas, J.A.; Guedes, M. Mechanical Behavior and Transport Properties of Cementitious Composites Reinforced with Carbon Nanotubes. J. Mater. Civ. Eng. 2018, 30, 1–14. [Google Scholar] [CrossRef]
- Pakravan, H.R.; Latifi, M.; Jamshidi, M. Hybrid short fiber reinforcement system in concrete: A review. Constr. Build. Mater. 2017, 142, 280–294. [Google Scholar] [CrossRef]
- Metaxa, Z.S.; Konsta-Gdoutos, M.S.; Shah, S.P. Mechanical Properties and Nanostructure of Cement-Based Materials Reinforced with Carbon Nanofibers and Polyvinyl Alcohol (PVA) Microfibers. ACI Spec. Publ. 2010, 270, 115–124. [Google Scholar] [CrossRef]
- Bashir, M.T.; Muhammad, S.; Butt, M.J.; Alzara, M.; El-Kady, M.S. Aspect ratio effect of multi-walled carbon nanotubes and carbon fibers on high-performance cement mortar matrices. Innov. Infrastruct. Solut. 2020, 5, 1–11. [Google Scholar] [CrossRef]
- Yao, W.; Li, J.; Wu, K. Mechanical properties of hybrid fiber-reinforced concrete at low fiber volume fraction. Cem. Concr. Res. 2003, 33, 27–30. [Google Scholar] [CrossRef]
- Hameed, R.; Turatsinze, A.; Duprat, F.; Sellier, A. A study on the reinforced fibrous concrete elements subjected to uniaxial tensile loading. KSCE J. Civ. Eng. 2010, 14, 547–556. [Google Scholar] [CrossRef]
- Bentur, A.; Mindess, S. Fibre Reinforced Cementitious Composites; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar] [CrossRef]
- C1437-13; Standard Test Method for Flow of Hydraulic Cement Mortar. ASTM: West Conshohocken, PA, USA, 2013.
- Senff, L.; Labrincha, J.A.; Ferreira, V.M.; Hotza, D.; Repette, W.L. Effect of nano-silica on rheology and fresh properties of cement pastes and mortars. Constr. Build. Mater. 2009, 23, 2487–2491. [Google Scholar] [CrossRef]
- Felekoglu, B.; Tosun-Felekoglu, K.; Ranade, R.; Zhang, Q.; Li, V.C. Influence of matrix flowability, fiber mixing procedure, and curing conditions on the mechanical performance of HTPP-ECC. Compos. Part B Eng. 2014, 60, 359–370. [Google Scholar] [CrossRef]
- C1231/C1231M-15; Use of Unbonded Caps in Determination of Compressive Strength of Hardened Cylindrical Concrete Specimens. ASTM: West Conshohocken, PA, USA, 2015.
- C39/C39M-20; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM: West Conshohocken, PA, USA, 2013.
- Douba, A.E.; Emiroğlu, M.; Tarefder, R.A.; Kandil, U.F.; Taha, M.R. Use of Carbon Nanotubes to Improve Fracture Toughness of Polymer Concrete. Transp. Res. Rec. J. Transp. Res. Board 2017, 2612, 96–103. [Google Scholar] [CrossRef]
- Douba, A.E. Mechanical Characterization of Polymer Concrete with Nanomaterials; The University of New Mexico: Albuquerque, NM, USA, 2017. [Google Scholar]
- C1609/C1609M-19a; Standard Test Method for Flexural Performance of Fiber-Reinforced Concrete (Using Beam With Third-Point Loading). ASTM: West Conshohocken, PA, USA, 2020.
- Taha, M.R.; Xiao, X.; Yi, J.; Shrive, N.G. Evaluation of flexural fracture toughness for quasi-brittle structural materials using a simple test method. Can. J. Civ. Eng. 2002, 29, 567–575. [Google Scholar] [CrossRef]
- Bažant, Z.P. Analysis of Work-of-Fracture Method for Measuring Fracture Energy of Concrete. J. Eng. Mech. 1996, 122, 138–144. [Google Scholar] [CrossRef] [Green Version]
- Bažant, Z.P. Concrete fracture models: Testing and practice. Eng. Fract. Mech. 2002, 69, 165–205. [Google Scholar] [CrossRef]
- Gettu, A.; Garcia-A’lvarez, R.; Aguado, V.O. Effect of aging on the fracture characteristics and brittleness of a high-strength concrete. Cem. Concr. Res. 1998, 28, 349–355. [Google Scholar] [CrossRef]
- Shrive, N.G.; El-Rahman, M. Understanding the cause of cracking in concrete: A diagnostic aid. Concr. Int. 1985, 7, 39–44. [Google Scholar]
- Hsu, T.T.; Slate, F.O.; Sturman, G.M.; Winter, G. Microcracking of plain concrete and the shape of the stress-strain curve. J. Proc. 1963, 60, 209–224. [Google Scholar]
- Wang, E.; Shrive, N. Brittle fracture in compression: Mechanisms, models and criteria. Eng. Fract. Mech. 1995, 52, 1107–1126. [Google Scholar] [CrossRef]
- Cusatis, G.; Bažant, Z.P.; Cedolin, L. Confinement-shear lattice CSL model for fracture propagation in concrete. Comput. Methods Appl. Mech. Eng. 2006, 195, 7154–7171. [Google Scholar] [CrossRef]
- Xu, S.; Liu, J.; Li, Q. Mechanical properties and microstructure of multi-walled carbon nanotube-reinforced cement paste. Constr. Build. Mater. 2015, 76, 16–23. [Google Scholar] [CrossRef]
- Hawreen, A.; Bogas, J.; Dias, A.P.S. On the mechanical and shrinkage behavior of cement mortars reinforced with carbon nanotubes. Constr. Build. Mater. 2018, 168, 459–470. [Google Scholar] [CrossRef]
- Gupta, S.; Kua, H.W. Carbonaceous micro-filler for cement: Effect of particle size and dosage of biochar on fresh and hardened properties of cement mortar. Sci. Total Environ. 2019, 662, 952–962. [Google Scholar] [CrossRef]
- Sharma, A.; Reddy, G.; Varshney, L.; Bharathkumar, H.; Vaze, K.; Ghosh, A.; Kushwaha, H.; Krishnamoorthy, T. Experimental investigations on mechanical and radiation shielding properties of hybrid lead–steel fiber reinforced concrete. Nucl. Eng. Des. 2009, 239, 1180–1185. [Google Scholar] [CrossRef]
- Glavind, M.; Aarre, T. High-Strength Concrete with Increased Fracture-Toughness. MRS Online Proc. Libr. Arch. 1990, 211, 39–46. [Google Scholar] [CrossRef]
- Dawood, E.; Ramli, M. High strength characteristics of cement mortar reinforced with hybrid fibres. Constr. Build. Mater. 2011, 25, 2240–2247. [Google Scholar] [CrossRef]
- Hsie, M.; Tu, C.; Song, P. Mechanical properties of polypropylene hybrid fiber-reinforced concrete. Mater. Sci. Eng. A 2008, 494, 153–157. [Google Scholar] [CrossRef]
- Park, S.H.; Kim, D.J.; Ryu, G.S.; Koh, K.T. Tensile behavior of Ultra High Performance Hybrid Fiber Reinforced Concrete. Cem. Concr. Compos. 2012, 34, 172–184. [Google Scholar] [CrossRef]
- Banthia, N.; Sappakittipakorn, M. Toughness enhancement in steel fiber reinforced concrete through fiber hybridization. Cem. Concr. Res. 2007, 37, 1366–1372. [Google Scholar] [CrossRef]
- Lawler, J.S.; Zampini, D.; Shah, S.P. Microfiber and Macrofiber Hybrid Fiber-Reinforced Concrete. J. Mater. Civ. Eng. 2005, 17, 595–604. [Google Scholar] [CrossRef]
- Kang, S.-T.; Choi, J.-I.; Koh, K.-T.; Lee, K.S.; Lee, B.Y. Hybrid effects of steel fiber and microfiber on the tensile behavior of ultra-high performance concrete. Compos. Struct. 2016, 145, 37–42. [Google Scholar] [CrossRef]
- Rashiddadash, P.; Ramezanianpour, A.A.; Mahdikhani, M. Experimental investigation on flexural toughness of hybrid fiber reinforced concrete (HFRC) containing metakaolin and pumice. Constr. Build. Mater. 2014, 51, 313–320. [Google Scholar] [CrossRef]
- Zhao, Z.; Qi, T.; Zhou, W.; Hui, D.; Xiao, C.; Qi, J.; Zheng, Z. A review on the properties, reinforcing effects, and commercialization of nanomaterials for cement-based materials. Nanotechnol. Rev. 2020, 9, 303–322. [Google Scholar] [CrossRef]
- Feldman, D.; Zheng, Z. Synthetic Fibres for Fibre Concrete Composites. MRS Online Proc. Libr. Arch. 1993, 305, 123–128. [Google Scholar] [CrossRef]
- Singh, S.P.; Singh, A.P.; Bajaj, V. Strength and flexural toughness of concrete reinforced with steel-polypropylene hybrid fibres. Asian J. Civ. Eng. 2010, 11, 495–507. [Google Scholar]
- Zhang, C.; Cao, M. Fiber synergy in multi-scale fiber-reinforced cementitious composites. J. Reinf. Plast. Compos. 2013, 33, 862–874. [Google Scholar] [CrossRef]
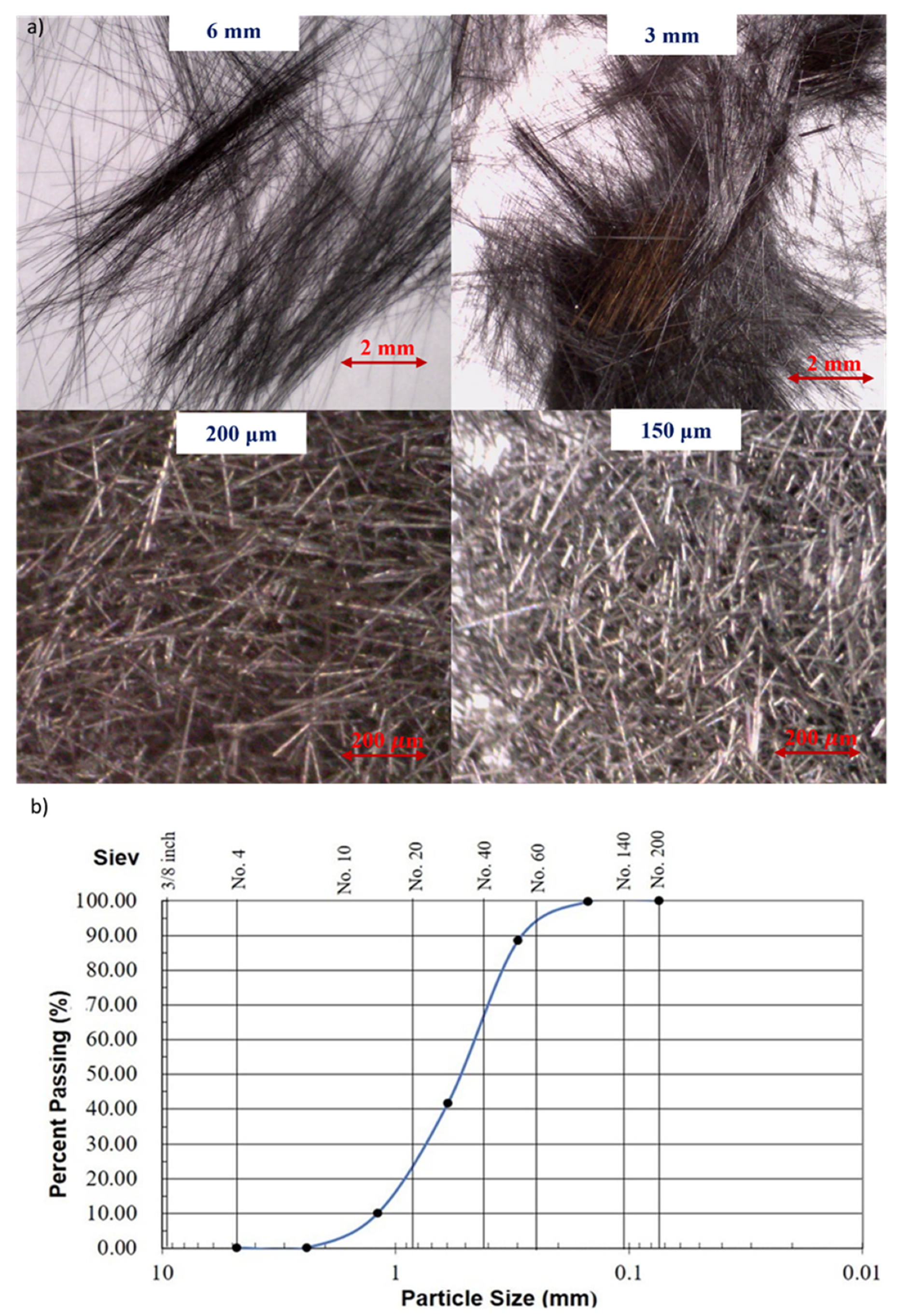
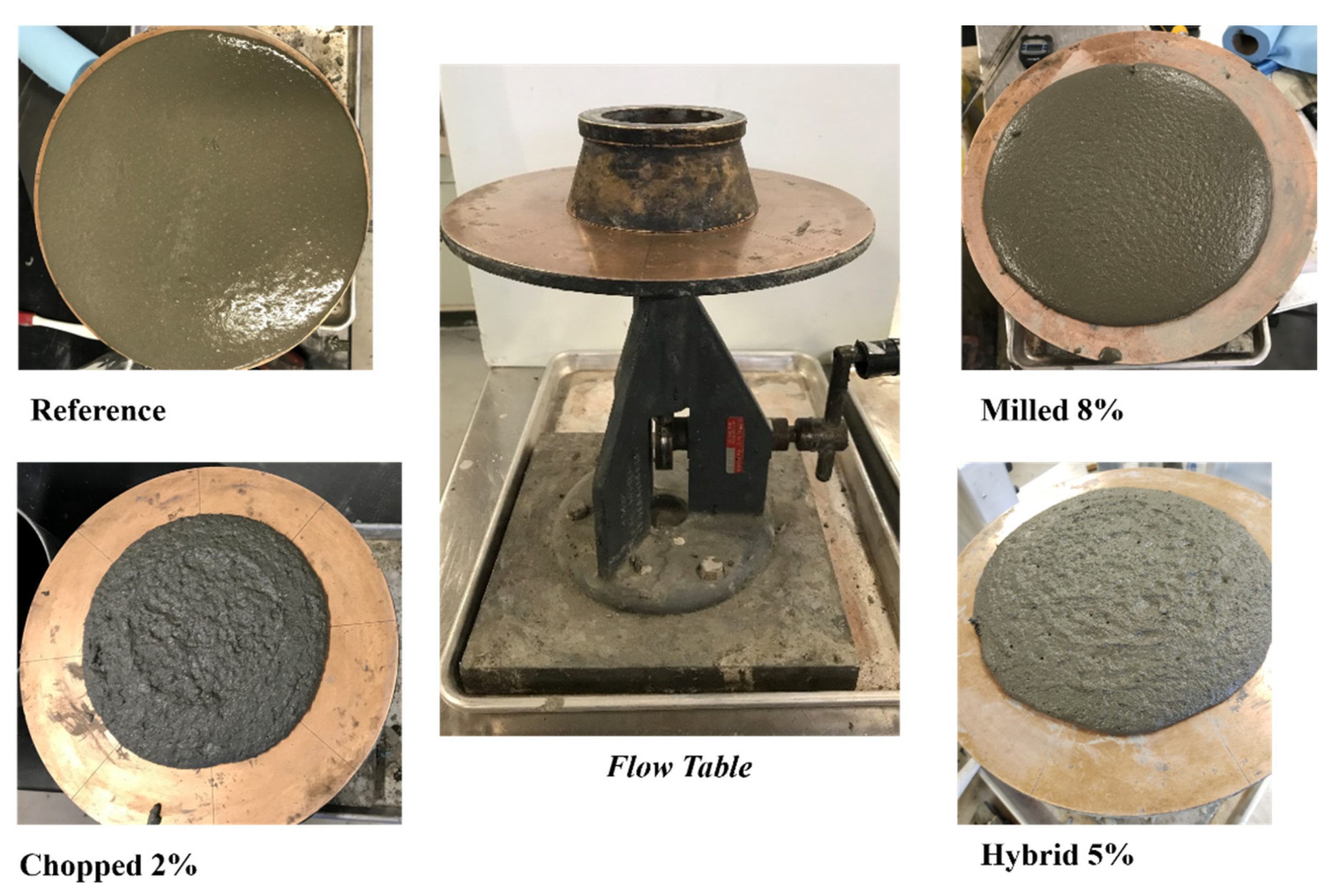




| Component | Reference (R) | Chopped 2% (CH2) | Milled 2% (ML2) | Milled 8% (ML8) | Hybrid 2% (H2) | Hybrid 5% (H5) | |
|---|---|---|---|---|---|---|---|
| Cement | 934.2 | ||||||
| Silica Fume | 93.4 | ||||||
| Sand | 934.2 | ||||||
| Water | 256.9 | ||||||
| Superplasticizer | 16.3 | ||||||
| CCMF (% wt. cement) | L = 3 mm | - | 1.0 | - | - | 0.2 | 0.5 |
| L = 6 mm | - | 1.0 | - | - | 0.2 | 0.5 | |
| MCMF (% wt. cement) | L = 150 μm | - | - | 1.0 | 4.0 | 0.8 | 2.0 |
| L = 200 μm | - | - | 1.0 | 4.0 | 0.8 | 2.0 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdellatef, M.; Heras Murcia, D.; Hogancamp, J.; Matteo, E.; Stormont, J.; Taha, M.M.R. The Significance of Multi-Size Carbon Fibers on the Mechanical and Fracture Characteristics of Fiber Reinforced Cement Composites. Fibers 2022, 10, 65. https://doi.org/10.3390/fib10080065
Abdellatef M, Heras Murcia D, Hogancamp J, Matteo E, Stormont J, Taha MMR. The Significance of Multi-Size Carbon Fibers on the Mechanical and Fracture Characteristics of Fiber Reinforced Cement Composites. Fibers. 2022; 10(8):65. https://doi.org/10.3390/fib10080065
Chicago/Turabian StyleAbdellatef, Mohammed, Daniel Heras Murcia, Joshua Hogancamp, Edward Matteo, John Stormont, and Mahmoud M. Reda Taha. 2022. "The Significance of Multi-Size Carbon Fibers on the Mechanical and Fracture Characteristics of Fiber Reinforced Cement Composites" Fibers 10, no. 8: 65. https://doi.org/10.3390/fib10080065
APA StyleAbdellatef, M., Heras Murcia, D., Hogancamp, J., Matteo, E., Stormont, J., & Taha, M. M. R. (2022). The Significance of Multi-Size Carbon Fibers on the Mechanical and Fracture Characteristics of Fiber Reinforced Cement Composites. Fibers, 10(8), 65. https://doi.org/10.3390/fib10080065







