Thermal Friction Contact Analysis of Graded Piezoelectric Coatings Under Conductive Punch Loading

Abstract

In this paper, we investigate the thermal friction sliding contact of a functionally graded piezoelectric material (FGPM)-coated half-plane subjected to a rigid conductive cylindrical punch. This study considers the effect of the thermal convection term in heat conduction. The thermo-electro-elastic material parameters of the coating vary exponentially along its thickness direction. Utilizing thermoelastic theory and Fourier integral transforms, the problem is formulated into Cauchy singular integral equations of the first and second kinds with surface stress, contact width, and electric displacement as the unknown variables. The numerical solutions for the contact stress, electric displacement, and temperature field of the graded coating surface are obtained using the least-squares method and iterative techniques. It can be observed that the thermo-electro-elastic contact behavior of the coating surface undergoes significant changes as the graded index varies from −0.5 to 0.5, the friction coefficient ranges from 0.1 to 0.5, and the sliding velocity changes from 0.01 m/s to 0.05 m/s. The results indicate that adjusting the graded index of the coating, the sliding speed of the punch, and the friction coefficient can improve the thermo-electro-elastic contact damage of the material’s surface.

1. Introduction

Piezoelectric composite materials are important intelligent materials widely used in smart structural devices, such as piezoelectric sensors, piezoelectric transducers, piezoelectric ultrasonic motors, and micro-robots [1,2,3,4]. With the increasing application and development of piezoelectric materials, piezoelectric intelligent components often operate in extremely harsh environments characterized by coupled thermal, electrical, and mechanical loads, such as linear ultrasonic piezoelectric motors and piezoelectric friction dampers [5,6,7]. Their working processes involve typical thermo-electro-mechanical coupling contact problems, where the thermal, electrical, and elastic properties of the materials interact and are coupled during contact. Studies of thermoelastic contact in piezoelectric materials indicate that stress and temperature concentrations occur at the edges of contact regions [8]. This implies that the edges of contact zones are sites for potential thermoelastic contact damage. Such damage can significantly impair the material’s ability to withstand contact loads and may lead to failure and damage. Therefore, it is essential to develop effective strategies to mitigate the occurrence of thermoelastic contact damage on the surfaces of piezoelectric materials.

Functionally graded material (FGM) exhibits material properties that vary gradually with spatial coordinates [9]. Functionally graded piezoelectric material (FGPM) is a novel intelligent composite material that integrates the advantages of piezoelectric and graded materials [10]. Ke et al. [11,12] first extended the contact mechanics of FGM to FGPM, taking into account the frictionless contact and sliding friction contact between a FGPM coated-structure and an insulating and conductive punch. The results show that the application of FGPM can improve the ability of piezoelectric materials to resist surface electro-elastic contact damage. Liu et al. [13,14] studied the axisymmetric frictionless/limited frictional contact between a conductive/insulating spherical punch and FGPM-coated substrate. Su et al. [15] developed an efficient least-squares method and iterative algorithm to solve the frictional contact problem between a rigid conductive cylindrical punch and an FGPM-coated structure. Çömez [16] investigated the frictional dynamic contact problem between a conductive rigid cylindrical punch and an FGPM-coated half-plane. Han et al. [17] analyzed the sliding friction and adhesive coupling contact problem between an FGPM-coated half-space and insulated punch under a plane strain state.

None of the aforementioned research considered the thermal effect; hence, when FGPM is subjected to high-temperature environments, the results may be invalid or require correction. To the best of the authors’ knowledge, there is limited research on the frictional thermal contact of FGPM. Kargarnovin et al. [18] used an exponential model to simulate FGPMs and investigated the two-dimensional thermal contact problem of FGPM under thermoelectric loads but did not consider the generated frictional heat. Zhou et al. [19] analyzed heat conduction in FGPM plates subjected to sliding punch loads and revealed the effects of non-Fourier heat conduction. Ma et al. [20,21] studied the two-dimensional sliding friction contact between functionally graded magneto-electro-elastic coating substrates and magneto-electro-elastic half-planes under the action of conductive magnetic punches. Çömez et al. [22] studied the steady-state thermoelastic frictional contact of FGPM-coated half-planes under the action of a rigid conductive cylindrical punch but neglected the effect of the thermal convection term in their heat conduction analysis. The above results indicate that the graded variation in material parameters significantly affects surface contact stress, temperature, and electric displacement distribution, which further demonstrates that FGPMs have high application value in mitigating friction contact damage.

In this paper, the thermal sliding friction contact problem between an FGPM-coated structure and a rigid conductive cylindrical punch is investigated. It is assumed that the material parameters of the coating vary exponentially in the thickness direction. The effects of frictional heat and thermal convection are also considered. Using the thermoelastic theory and Fourier integral transform, the problem is transformed into Cauchy singular integral equations. The effects of frictional heat and thermal convection are considered. The effects of the sliding velocity, friction coefficient, and graded index on the thermal friction contact characteristics are discussed in detail through parameter analysis.

2. Formulation of the Problem

The sliding thermal friction contact problem of an FGPM coating with thickness h under the action of a rigid conducting cylindrical punch is considered, as shown in Figure 1. It is assumed that the thermo-electro-elastic parameters of the FGPM vary along the thickness direction of the coating according to the following exponential function:

$$\left\{c_{il}\left(z\right),e_{il}\left(z\right),{\epsilon }_{il}\left(z\right),k_i\left(z\right),{\lambda }_i\left(z\right),{\alpha }_3\left(z\right),c\left(z\right)\right\}=\left\{c_{il0},e_{il0},{\epsilon }_{il0},k_{i0},{\lambda }_{i0},{\alpha }_{30},c_0\right\}{e}^{\beta z}, 0<z\le h.$$

(1)

where c_il(z), e_il(z), ε_il(z), k_i(z), λ_i(z), α₃(z), and c(z) represent the elastic constant parameters, piezoelectric constant parameters, dielectric constant parameters, heat conduction coefficient parameters, thermal expansion coefficient parameters, thermoelectric coefficient parameters, and specific heat capacity parameters. The subscript “0” corresponds to the thermal-electro-elastic parameters of the homogeneous piezoelectric half-plane and graded coating at z = 0. Assuming the mass density ρ is constant, it can be denoted as ρ₀.

It is assumed that the material parameters at interface z = 0 are continuous, ensuring a uniform distribution of interfacial stress even when material properties are mismatched. A cylindrical punch slides on the surface of a coating at a small sliding velocity V under the action of a normal pressure P, a tangential force Q, and a total charge Γ. The coordinate system (x, z) is established as shown in Figure 1, with the x-axis located at the interface z = 0 between the graded coating and the homogeneous half-plane, and the z-axis pointing upward along the coating thickness direction. It is also assumed that the piezoelectric material is polarized along the z-axis direction. Within the contact zone $-b\le x\le a$, the Coulomb friction criterion is satisfied, and the tangential distribution force q(x) is proportional to the normal contact pressure p(x) and the friction coefficient μ, i.e., $q\left(x\right)=\mu p\left(x\right)$.

In reality, the material parameters of FGMs vary arbitrarily with spatial position. Recently, models such as the uniform stratification model [23,24], linear stratification model [25,26], and exponential stratification model [27] have been extensively utilized to simulate FGMs with arbitrary material parameters. However, obtaining analytical solutions for each sublayer of the linear stratification model is challenging, limiting its applicability for simulating the material parameters of FGPMs. In this study, to simplify the problem, the thermo-electric-elastic parameters in the model were set with a uniform graded index. For future studies, a laminate model could be considered to simulate the arbitrary variation in thermo-electric-elastic material parameters.

2.1. Temperature Field

It is assumed that the rigid cylindrical punch is an ideal thermal insulator and electrical conductor, such that the heat flow generated by friction quasi-statically enters the graded piezoelectric coating surface. Additionally, the cylindrical punch maintains a constant potential φ in the contact area. The relationship among the surface heat flow Θ_z(x), the contact pressure p(x), the friction coefficient μ, and the sliding velocity V can be expressed as follows:

$${\mathrm{\Theta }}_{z1}\left(x\right)=\mu Vp\left(x\right),\hspace{1em}\hspace{1em}-b\le x\le a.$$

(2)

Considering the steady-state heat conduction, the heat conduction equation of the FGPM coating can be written as

$$k_{10}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_1}{\partial x^2}}+k_{30}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_1}{\partial z^2}}+k_{30}\beta {\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}={\displaystyle \frac{k_{30}}{\chi \left(z\right)}}V{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial x}},$$

(3)

where Ω is the temperature field; $\chi \left(z\right)=k_3\left(z\right)/\left[c\left(z\right){\rho }_0\right]$ is the thermal diffusion coefficient; the result is constant $\chi =k_{30}/\left(c_0{\rho }_0\right)$ after Equation (1) is brought in. Subscript “1” represents the FGPM coating, and subscript “0” represents the homogeneous half-plane. Therefore, the equation can be written as

$$k_{10}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_1}{\partial x^2}}+k_{30}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_1}{\partial z^2}}+k_{30}\beta {\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}={\displaystyle \frac{k_{30}}{\chi }}V{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial x}}.$$

(4)

In addition, the steady-state heat conduction equation of the homogeneous half-plane at $(z\le 0)$ is

$$k_{10}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_0}{\partial x^2}}+k_{30}{\displaystyle \frac{{\partial }^2{\mathrm{\Omega }}_0}{\partial z^2}}=c_0{\rho }_{0}V{\displaystyle \frac{\partial {\mathrm{\Omega }}_0}{\partial x}}.$$

(5)

Fourier transform is performed on Equations (4) and (5):

$${\displaystyle \frac{{\mathrm{d}}^2{\tilde{\mathrm{\Omega }}}_1}{\mathrm{d}{z}^2}}+\beta {\displaystyle \frac{\mathrm{d}{\tilde{\mathrm{\Omega }}}_1}{\mathrm{d}z}}-\left(s^2{\displaystyle \frac{k_{10}}{k_{30}}}+{\displaystyle \frac{\mathrm{i}sV}{\chi }}\right){\tilde{\mathrm{\Omega }}}_1=0,$$

(6)

$$-s^2{k}_{10}{\tilde{\mathrm{\Omega }}}_0+k_{30}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{\mathrm{\Omega }}}_0}{\mathrm{d}{z}^2}}=c_0{\rho }_{0}V\mathrm{i}s{\tilde{\mathrm{\Omega }}}_0.$$

(7)

where “~” represents Fourier integral transform, $\mathrm{i}=\sqrt{-1}$ is the imaginary unit, and $s$ is the integral transform parameter. The general solutions of Equations (6) and (7) are

$${\tilde{\mathrm{\Omega }}}_1=A_1{\mathrm{e}}^{m_{1}z}+A_2{\mathrm{e}}^{m_{2}z}, {\tilde{\mathrm{\Omega }}}_0=A_0{e}^{m_{0}z},$$

(8)

where A₁, A₂, and A₀ are unknown coefficients, and

$$m_{1,2}=\left[-\beta \pm \sqrt{{\beta }^2+4\left(s^2{k}_{10}/k_{30}+\mathrm{i}sV/\chi \right)}\right]/2, m_0=\sqrt{s^2{k}_{10}/k_{30}+\mathrm{i}s{c}_0{\rho }_{0}V/k_{30}}.$$

(9)

It is assumed that all the heat generated by friction is conducted from the surface of the coating down to the inside of the structure, and the area outside the contact area is thermally insulated. The surface heat flow Q(x) is proportional to the friction coefficient, sliding velocity, and contact pressure, i.e.,

$$k_{30}{e}^{\beta h}{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}=\mu Vp\left(x\right),\hspace{1em}\hspace{1em}\left(z=h\right),$$

(10)

Furthermore, the FGPM coating is perfectly bonded to the half-plane; therefore, the temperature satisfies the continuity conditions on the bonded interface z = 0, i.e.,

$${\mathrm{\Omega }}_1={\mathrm{\Omega }}_0, {\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}={\displaystyle \frac{\partial {\mathrm{\Omega }}_0}{\partial z}},\hspace{1em}\hspace{1em}\left(z=0\right).$$

(11)

By performing Fourier transform on the boundary condition Equations (10) and (11) and substituting the general solution Equation (8), we can obtain

$$m_1{A}_1{e}^{m_{1}h}+m_2{A}_2{e}^{m_{2}h}={\displaystyle \frac{\mu V}{k_{30}}}{e}^{-\beta h}\tilde{p}\left(s\right),\hspace{1em}\hspace{1em}\left(z=h\right),$$

(12a)

$$A_1+A_2=A_0, A_1{m}_1+A_2{m}_2=A_0{m}_0,\hspace{1em}\hspace{1em}\left(z=0\right).$$

(12b)

The above equations can be solved to obtain A₁, A₂, and A₀:

$$A_0=g_0{R}_0, A_1=g_0{R}_1, A_2=g_0{R}_2,$$

(13)

$$\begin{array}{l}{R}_1={\displaystyle \frac{\left(m_2-m_0\right)\tilde{p}(s)}{\left(m_2-m_0\right)m_1{e}^{m_{1}h}+\left(m_0-m_1\right)m_2{e}^{m_{2}h}}}, R_2={\displaystyle \frac{\left(m_0-m_1\right)\tilde{p}(s)}{\left(m_2-m_0\right)m_1{e}^{m_{1}h}+\left(m_0-m_1\right)m_2{e}^{m_{2}h}}},\\ R_0={\displaystyle \frac{\left(m_2-m_1\right)\tilde{p}(s)}{\left(m_2-m_0\right)m_1{e}^{m_{1}h}+\left(m_0-m_1\right)m_2{e}^{m_{2}h}}},\end{array}$$

(14)

where $g_0={\displaystyle \frac{\mu V}{k_{30}}}{e}^{-\beta h}$.

By substituting Equations (13) and (14) into Equation (9) and performing an inverse Fourier transform, the temperature field can be obtained as follows:

$${\tilde{\mathrm{\Omega }}}_1={\displaystyle \frac{g_0}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }(R_1{e}^{m_{1}z}+R_2{e}^{m_{2}z})e^{\mathrm{i}sx}\mathrm{d}s},$$

(15)

$${\tilde{\mathrm{\Omega }}}_0={\displaystyle \frac{g_0}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }{R}_0{e}^{m_{0}z}{e}^{\mathrm{i}sx}\mathrm{d}s}.$$

(16)

2.2. Thermal Piezoelectric and Elastic Field

In the plane strain state, the graded coating displacement satisfies the following equations [21]:

$$\begin{array}{l}{c}_{110}{\displaystyle \frac{{\partial }^2{u}_{x1}}{\partial x^2}}+c_{440}{\displaystyle \frac{{\partial }^2{u}_{x1}}{\partial z^2}}+\left(c_{130}+c_{440}\right){\displaystyle \frac{{\partial }^2{u}_{z1}}{\partial x\partial z}}+\left(e_{310}+e_{150}\right){\displaystyle \frac{{\partial }^2{\phi }_1}{\partial x\partial z}}\\ +\beta \left[c_{440}\left({\displaystyle \frac{\partial u_{z1}}{\partial x}}+{\displaystyle \frac{\partial {\tilde{u}}_{x1}}{\partial z}}\right)+e_{150}{\displaystyle \frac{\partial {\phi }_1}{\partial x}}\right]={\lambda }_{110}{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial x}},\end{array}$$

(17a)

$$\begin{array}{l}\left(c_{130}+c_{440}\right){\displaystyle \frac{{\partial }^2{u}_{x1}}{\partial x\partial z}}+c_{440}{\displaystyle \frac{\partial u_{z1}}{\partial x^2}}+c_{330}{\displaystyle \frac{{\partial }^2{u}_{z1}}{\partial z^2}}+e_{150}{\displaystyle \frac{{\partial }^2{\phi }_1}{\partial x^2}}+e_{330}{\displaystyle \frac{{\partial }^2{\phi }_1}{\partial z^2}}\\ +\beta \left[c_{130}{\displaystyle \frac{\partial u_{x1}}{\partial x}}+c_{330}{\displaystyle \frac{\partial u_{z1}}{\partial z}}+e_{330}{\displaystyle \frac{\partial {\phi }_1}{\partial z}}\right]={\lambda }_{330}\left[\beta {\mathrm{\Omega }}_1+{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}\right],\end{array}$$

(17b)

$$\begin{array}{l}\left(e_{150}+e_{310}\right){\displaystyle \frac{{\partial }^2{u}_{x1}}{\partial x\partial z}}+e_{150}{\displaystyle \frac{{\partial }^2{u}_{z1}}{\partial x^2}}+e_{330}{\displaystyle \frac{{\partial }^2{u}_{z1}}{\partial z^2}}-{\epsilon }_{110}{\displaystyle \frac{{\partial }^2{\phi }_1}{\partial x^2}}-{\epsilon }_{330}{\displaystyle \frac{{\partial }^2{\phi }_1}{\partial z^2}}\\ +\beta \left[e_{310}{\displaystyle \frac{\partial u_{x1}}{\partial x}}+e_{330}{\displaystyle \frac{\partial u_{z1}}{\partial z}}-{\epsilon }_{330}{\displaystyle \frac{\partial {\phi }_1}{\partial z}}\right]={\alpha }_{30}\left[\beta {\mathrm{\Omega }}_1+{\displaystyle \frac{\partial {\mathrm{\Omega }}_1}{\partial z}}\right].\end{array}$$

(17c)

Perform the Fourier integral transformation on Equation (17a–c) with respect to x, and substitute the temperature field Equation (15) to obtain

$$\begin{array}{l}-s^2{c}_{110}{\tilde{u}}_{x1}+c_{440}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{x1}}{\mathrm{d}{z}^2}}+\mathrm{i}s\left(c_{130}+c_{440}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{z1}}{\mathrm{d}z}}+\mathrm{i}s\left(e_{310}+e_{150}\right){\displaystyle \frac{\mathrm{d}{\tilde{\phi }}_1}{\mathrm{d}z}}\\ +\beta \left[c_{440}\left(\mathrm{i}s{\tilde{u}}_{z1}+{\displaystyle \frac{\mathrm{d}{\tilde{u}}_{x1}}{\mathrm{d}z}}\right)+\mathrm{i}s{e}_{150}{\tilde{\phi }}_1\right]=\mathrm{i}s{g}_0{\lambda }_{110}\tilde{p}\left(s\right)\left(R_1{e}^{m_{1}z}+R_2{e}^{m_{2}z}\right),\end{array}$$

(18a)

$$\begin{array}{l}\mathrm{i}s\left(c_{130}+c_{440}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{x1}}{\mathrm{d}z}}-s^2{c}_{440}{\tilde{u}}_{z1}+c_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{z1}}{\mathrm{d}{z}^2}}-s^2{e}_{150}{\tilde{\phi }}_1+e_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{\phi }}_1}{\mathrm{d}{z}^2}}\\ +\beta \left[\mathrm{i}s{c}_{130}{\tilde{u}}_{x1}+c_{330}{\displaystyle \frac{\mathrm{d}{\tilde{u}}_{z1}}{\mathrm{d}z}}+e_{330}{\displaystyle \frac{\mathrm{d}{\tilde{\phi }}_1}{\mathrm{d}z}}\right]\\ =g_0{\lambda }_{330}\tilde{p}\left(s\right)\left[\left(\beta +m_1\right)R_1{e}^{m_{1}z}+\left(\beta +m_2\right)R_2{e}^{m_{2}z}\right],\end{array}$$

(18b)

$$\begin{array}{l}\mathrm{i}s\left(e_{150}+e_{310}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{x1}}{\mathrm{d}z}}-s^2{e}_{150}{\tilde{u}}_{z1}+e_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{z1}}{\mathrm{d}{z}^2}}+s^2{\epsilon }_{110}{\tilde{\phi }}_1-{\epsilon }_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{\phi }}_1}{\mathrm{d}{z}^2}}\\ +\beta \left[\mathrm{i}s{e}_{310}{\tilde{u}}_{x1}+e_{330}{\displaystyle \frac{\mathrm{d}{\tilde{u}}_{z1}}{\mathrm{d}z}}-{\epsilon }_{330}{\displaystyle \frac{\mathrm{d}{\tilde{\phi }}_1}{\mathrm{d}z}}\right]\\ =g_0{\alpha }_{30}\tilde{p}\left(s\right)\left[\left(\beta +m_1\right)R_1{e}^{m_{1}z}+\left(\beta +m_2\right)R_2{e}^{m_{2}z}\right].\end{array}$$

(18c)

The general solutions of Equation (18a–c) in the transform domain are

$${\left\{{\tilde{u}}_{x1}\left(s,z\right), {\tilde{u}}_{z1}\left(s,z\right), {\tilde{\phi }}_1\left(s,z\right)\right\}}^{\mathrm{T}}=\left[T_{11}\left(s,z\right)\right]\left\{B_1\left(s\right)\right\}+\left\{T_{21}\left(s,z\right)\right\}\tilde{p}\left(s\right)$$

(19)

where $\{B_1(s)\}={\{B_{11}(s) B_{12}(s), B_{13}(s), B_{14}(s), B_{15}(s), B_{16}(s)\}}^{\mathrm{T}}$ is the undetermined unknown coefficient of the graded coating (the superscript “T” represents the transpose of the matrix); $[T_{11}(s,z)]$ and $\{T_{21}(s,z)\}$ are, respectively,

$$\left[T_{11}\left(s,z\right)\right]={\left\{T_{1i1}^a\left(s,z\right), T_{1i1}^b\left(s,z\right), T_{1i1}^c\left(s,z\right)\right\}}^{\mathrm{T}}, \left\{T_{21}\left(s,z\right)\right\}={\left\{T_{21}^a\left(s,z\right), T_{21}^b\left(s,z\right), T_{21}^c\left(s,z\right)\right\}}^{\mathrm{T}},$$

where

$$T_{1i1}^a\left(s,z\right)=e^{n_{i1}z}, T_{1i1}^b\left(s,z\right)={\overline{a}}_{i1}\left(s\right)e^{n_{i1}z}, T_{1i1}^c\left(s,z\right)={\overline{b}}_{i1}{e}^{n_{i1}z},$$

$$T_{21}^a\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2{e}^{m_{\delta }z}{F}_{1\delta }}, T_{21}^b\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2{e}^{m_{\delta }z}{F}_{2\delta }}, T_{21}^c\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2{e}^{m_{\delta }z}{F}_{3\delta }}.$$

The expressions $n_{i1}, {\overline{a}}_{i1}(s), {\overline{b}}_{i1}(s) (i=1,\cdots ,6)$ and $F_{v\delta }(v=1, 2, 3; \delta =1, 2)$ are shown in Appendix A.

According to the physical relationship, the stress component and electrical displacement of the graded coating in the transformation domain can be expressed in the following matrix form:

$${\left\{{\tilde{\sigma }}_{zz1}\left(s,z\right), {\tilde{\sigma }}_{xz1}\left(s,z\right), {\tilde{D}}_{z1}\left(s,z\right)\right\}}^{\mathrm{T}}=\left[T_{31}\left(s,z\right)\right]\left\{B_1\left(s\right)\right\}+\left\{T_{41}\left(s,z\right)\right\}\tilde{p}\left(s\right).$$

(20)

The expressions of matrix $[T_{31}(s,z)]$ and matrix $\{T_{41}(s,z)\}$ are

$$\left[T_{31}\left(s,z\right)\right]={\left\{T_{3i1}^a\left(s,z\right), T_{3i1}^b\left(s,z\right), T_{3i1}^c\left(s,z\right)\right\}}^{\mathrm{T}}, \left\{T_{41}\left(s,z\right)\right\}={\left\{T_{41}^a\left(s,z\right), T_{41}^b\left(s,z\right), T_{41}^c\left(s,z\right)\right\}}^{\mathrm{T}},$$

where

$$T_{3i1}^a\left(s,z\right)=\left[\mathrm{i}s{c}_{130}+n_{i1}{c}_{330}{\overline{a}}_{i1}\left(s\right)+n_{i1}{e}_{330}{\overline{b}}_{i1}\left(s\right)\right]e^{\left(n_{i1}+\beta \right)z},$$

$$T_{3i1}^b\left(s,z\right)=\left[\mathrm{i}s{c}_{440}{\overline{a}}_{i1}\left(s\right)+n_{i1}{c}_{440}+\mathrm{i}s{e}_{150}{\overline{b}}_{i1}\left(s\right)\right]e^{\left(n_{i1}+\beta \right)z},$$

$$T_{3i1}^c\left(s,z\right)=\left[\mathrm{i}s{e}_{310}+n_{i1}{e}_{330}{\overline{a}}_{i1}\left(s\right)-n_{i1}{\epsilon }_{330}{\overline{b}}_{i1}\left(s\right)\right]e^{\left(n_{i1}+\beta \right)z},$$

$$T_{41}^a\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2\left[\mathrm{i}s{c}_{130}{F}_{1\delta }\left(s\right)+m_{\delta }{c}_{330}{F}_{2\delta }\left(s\right)+m_{\delta }{e}_{330}{F}_{3\delta }\left(s\right)-{\lambda }_{330}{R}_{\delta }\right]e^{\left(m_{\delta }+\beta \right)z}},$$

$$T_{41}^b\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2\left[\mathrm{i}s{c}_{440}{F}_{2\delta }\left(s\right)+m_{\delta }{c}_{440}{F}_{1\delta }\left(s\right)+\mathrm{i}s{e}_{150}{F}_{3\delta }\left(s\right)\right]e^{\left(m_{\delta }+\beta \right)z}},$$

$$T_{41}^c\left(s,z\right)=g_0{\displaystyle \sum _{\delta =1}^2\left[\mathrm{i}s{e}_{310}{F}_{1\delta }\left(s\right)+m_{\delta }{e}_{330}{F}_{2\delta }\left(s\right)-m_{\delta }{\epsilon }_{330}{F}_{3\delta }\left(s\right)-{\alpha }_{30}{R}_{\delta }\right]e^{\left(m_{\delta }+\beta \right)z}}.$$

$$\left(i=1,\cdots ,6; \delta =1,2\right)$$

On the other hand, let u_x0, u_z0, φ₀, σ_zz0, σ_xz0, and D_z0, be the displacements, stress components, potential, and electric displacements of $(z\le 0)$ on the homogeneous piezoelectric half-plane, respectively. In the homogeneous piezoelectric half-plane, the displacement satisfies

$$c_{110}{\displaystyle \frac{{\partial }^2{u}_{x0}}{\partial x^2}}+c_{440}{\displaystyle \frac{{\partial }^2{u}_{x0}}{\partial z^2}}+\left(c_{130}+c_{440}\right){\displaystyle \frac{{\partial }^2{u}_{z0}}{\partial x\partial z}}+\left(e_{310}+e_{150}\right){\displaystyle \frac{{\partial }^2{\phi }_0}{\partial x\partial z}}={\lambda }_{110}{\displaystyle \frac{\partial {\mathrm{\Omega }}_0}{\partial x}},$$

(21a)

$$\left(c_{130}+c_{440}\right){\displaystyle \frac{{\partial }^2{u}_{x0}}{\partial x\partial z}}+c_{440}{\displaystyle \frac{\partial u_{z0}}{\partial x^2}}+c_{330}{\displaystyle \frac{{\partial }^2{u}_{z0}}{\partial z^2}}+e_{150}{\displaystyle \frac{{\partial }^2{\phi }_0}{\partial x^2}}+e_{330}{\displaystyle \frac{{\partial }^2{\phi }_0}{\partial z^2}}={\lambda }_{330}{\displaystyle \frac{\partial {\mathrm{\Omega }}_0}{\partial z}},$$

(21b)

$$\left(e_{150}+e_{310}\right){\displaystyle \frac{{\partial }^2{u}_{x0}}{\partial x\partial z}}+e_{150}{\displaystyle \frac{{\partial }^2{u}_{z0}}{\partial x^2}}+e_{330}{\displaystyle \frac{{\partial }^2{u}_{z0}}{\partial z^2}}-{\epsilon }_{110}{\displaystyle \frac{{\partial }^2{\phi }_0}{\partial x^2}}-{\epsilon }_{330}{\displaystyle \frac{{\partial }^2{\phi }_0}{\partial z^2}}={\alpha }_{30}{\displaystyle \frac{\partial {\mathrm{\Omega }}_0}{\partial z}}.$$

(21c)

Perform Fourier transform on Equation (21a–c) to obtain

$$-s^2{c}_{110}{\tilde{u}}_{x0}+c_{440}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{x0}}{\mathrm{d}{z}^2}}+\mathrm{i}s\left(c_{130}+c_{440}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{z0}}{\mathrm{d}z}}+\mathrm{i}s\left(e_{310}+e_{150}\right){\displaystyle \frac{\mathrm{d}{\tilde{\phi }}_0}{\mathrm{d}z}}=\mathrm{i}s{g}_0{\lambda }_{110}{R}_0{e}^{m_{0}z}\tilde{p}\left(s\right),$$

(22a)

$$\mathrm{i}s\left(c_{130}+c_{440}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{x0}}{\mathrm{d}z}}-s^2{c}_{440}{\tilde{u}}_{z0}+c_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{z0}}{\mathrm{d}{z}^2}}-s^2{e}_{150}{\tilde{\phi }}_0+e_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{\phi }}_0}{\mathrm{d}{z}^2}}=g_0{\lambda }_{330}{m}_0{R}_0{e}^{m_{0}z}\tilde{p}\left(s\right),$$

(22b)

$$\mathrm{i}s\left(e_{150}+e_{310}\right){\displaystyle \frac{\mathrm{d}{\tilde{u}}_{x0}}{\mathrm{d}z}}-s^2{e}_{150}{\tilde{u}}_{z0}+e_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{u}}_{z0}}{\mathrm{d}{z}^2}}+s^2{\epsilon }_{110}{\tilde{\phi }}_0-{\epsilon }_{330}{\displaystyle \frac{{\mathrm{d}}^2{\tilde{\phi }}_0}{\mathrm{d}{z}^2}}=g_0{\alpha }_{30}{m}_0{R}_0{e}^{m_{0}z}\tilde{p}\left(s\right).$$

(22c)

Consider that the displacement component and potential in the homogeneous piezoelectric half-plane satisfy the conditions at infinity, i.e., u_x0, u_z0, φ₀→0, when $\sqrt{x^2+z^2}\to \infty$. The general solution of Equation (22a–b) can be expressed as

$${\left\{{\tilde{u}}_{x0}\left(s,z\right), {\tilde{u}}_{z0}\left(s,z\right), {\tilde{\phi }}_0\left(s,z\right)\right\}}^{\mathrm{T}}=\left[T_{10}\left(s,z\right)\right]\left\{B_0\left(s\right)\right\}+\left\{T_{20}\left(s,z\right)\right\}\tilde{p}\left(s\right).$$

(23)

where $\left\{B_0\right\}={\left\{B_{01}, B_{02}, B_{03}\right\}}^{\mathrm{T}}$ are undetermined unknown coefficients. $[T_{10}(s,z)]$ and $\{T_{20}(s,z)\}$ are, respectively,

$$\left[T_{10}\left(s,z\right)\right]={\left[T_{1k0}^a\left(s,z\right), T_{1k0}^b\left(s,z\right), T_{1k0}^c\left(s,z\right)\right]}^{\mathrm{T}}, \left\{T_{20}\left(s,z\right)\right\}={\left\{T_{20}^a\left(s,z\right), T_{20}^b\left(s,z\right), T_{20}^c\left(s,z\right)\right\}}^{\mathrm{T}},$$

$$T_{1k0}^a\left(s,z\right)=e^{n_{k0}z}, T_{1k0}^b\left(s,z\right)={\overline{c}}_{k0}\left(s\right)e^{n_{k0}z}, T_{1k0}^c\left(s,z\right)={\overline{d}}_{k0}{e}^{n_{k0}z},$$

$$T_{20}^a\left(s,z\right)=g_0{e}^{m_{0}z}{F}_{10}, T_{20}^b\left(s,z\right)=g_0{e}^{m_{0}z}{F}_{20}, T_{20}^c\left(s,z\right)=g_0{e}^{m_{0}z}{F}_{30}.$$

The expressions of $n_{i1}, {\overline{c}}_{k0}(s), {\overline{d}}_{k0}(s) (k=1, 2, 3)$ and $F_{v0}(v=1, 2, 3)$ are shown in Appendix A. Then, the stress component and electrical displacement in the homogeneous piezoelectric half-plane can be expressed as follows:

$${\left\{{\tilde{\sigma }}_{zz0}\left(s,z\right), {\tilde{\sigma }}_{xz0}\left(s,z\right), {\tilde{D}}_{z0}\left(s,z\right)\right\}}^{\mathrm{T}}=\left[T_{30}\left(s,z\right)\right]\left\{B_0\left(s\right)\right\}+\left\{T_{40}\left(s,z\right)\right\}\tilde{p}\left(s\right),$$

(24)

where

$$\left[T_{30}\left(s,z\right)\right]={\left[T_{3k0}^a\left(s,z\right), T_{3k0}^b\left(s,z\right), T_{3k0}^c\left(s,z\right)\right]}^{\mathrm{T}}, \left\{T_{40}\left(s,z\right)\right\}={\left\{T_{40}^a\left(s,z\right), T_{40}^b\left(s,z\right), T_{40}^c\left(s,z\right)\right\}}^{\mathrm{T}},$$

$$T_{3k0}^a\left(s,z\right)=\left[\mathrm{i}s{c}_{130}+n_{k0}{c}_{330}{\overline{c}}_{k0}\left(s\right)+n_{k0}{e}_{330}{\overline{d}}_{k0}\left(s\right)\right]e^{n_{k0}z},$$

$$T_{3k0}^b\left(s,z\right)=\left[n_{k0}{c}_{440}+\mathrm{i}s{c}_{440}{\overline{c}}_{k0}\left(s\right)+\mathrm{i}s{e}_{150}{\overline{d}}_{k0}\left(s\right)\right]e^{n_{k0}z},$$

$$\mathrm{q}{T}_{3k0}^c\left(s,z\right)=\left[\mathrm{i}s{e}_{310}+n_{k0}{e}_{330}{\overline{c}}_{k0}\left(s\right)-n_{k0}{\epsilon }_{330}{\overline{d}}_{k0}\left(s\right)\right]e^{n_{k0}z},$$

$$T_{40}^a\left(s,z\right)=g_0\left[\mathrm{i}s{c}_{130}{F}_{10}\left(s\right)+m_0{c}_{330}{F}_{20}\left(s\right)+m_0{e}_{330}{F}_{30}\left(s\right)-{\lambda }_{330}{R}_0\right]e^{m_{0}z},$$

$$T_{40}^b\left(s,z\right)=g_0\left[\mathrm{i}s{c}_{440}{F}_{20}\left(s\right)+m_0{c}_{440}{F}_{10}\left(s\right)+\mathrm{i}s{e}_{150}{F}_{30}\left(s\right)\right]e^{m_{0}z},$$

$$T_{40}^c\left(s,z\right)=g_0\left[\mathrm{i}s{e}_{310}{F}_{10}\left(s\right)+m_0{e}_{330}{F}_{20}\left(s\right)-m_0{\epsilon }_{330}{F}_{30}\left(s\right)-{\alpha }_{30}{R}_0\right]e^{m_{0}z}.$$

$$\left(k=1, 2, 3\right)$$

2.3. Boundary Conditions

Assuming that the rigid cylindrical punch is an ideal conductor, it is satisfied at the contact surface z = h:

$${\sigma }_{zz}\left(x,h\right)=\left\{\begin{array}{cc}-p(x)\hfill & -b\le x\le a\hfill \\ 0\hfill & x<-b,x>a\hfill \end{array}\right. ,$$

(25a)

$${\sigma }_{xz}\left(x,h\right)=\left\{\begin{array}{cc}-q(x)\hfill & -b\le x\le a\hfill \\ 0\hfill & x<-b,x>a\hfill \end{array}\right.,$$

(25b)

$$D_z\left(x,h\right)=\left\{\begin{array}{cc}-g(x)\hfill & -b\le x\le a\hfill \\ 0\hfill & x<-b,x>a\hfill \end{array}\right..$$

(25c)

At the contact interface z = 0, the stress, displacement, and electrical displacement satisfy the following continuous conditions:

$$u_{x1}(x,0)=u_{x0}(x,0), u_{z1}(x,0)=u_{z0}(x,0),$$

(26a)

$${\sigma }_{zz1}(x,0)={\sigma }_{zz0}(x,0), {\sigma }_{z1}(x,0)={\sigma }_{zx0}(x,0),$$

(26b)

$$D_{z1}(x,0)=D_{z0}(x,0), {\phi }_1(x,0)={\phi }_0(x,0).$$

(26c)

Perform Fourier transform on the above boundary conditions (Equations (25) and (26)) and represent them in matrix form:

$$\left[T_{31}\left(s,h\right)\right]\left\{B_1\left(s\right)\right\}+\left\{T_{41}\left(s,h\right)\right\}\tilde{p}\left(s\right)=\left\{L\right\},$$

(27a)

$$\left[L_{11}\left(s,0\right)\right]\left\{B_1\left(s\right)\right\}+\left\{L_{21}\left(s,0\right)\right\}\tilde{p}\left(s\right)=\left[L_{10}\left(s,0\right)\right]\left\{B_0\left(s\right)\right\}+\left\{L_{20}\left(s,0\right)\right\}\tilde{p}\left(s\right).$$

(27b)

The expression for the unknown coefficient $\left\{B_1\left(s\right)\right\}$, $\left\{B_0\left(s\right)\right\}$ can be obtained by the transfer matrix:

$$\left\{B_1\left(s\right)\right\}=\left[C\right]\left\{B_0\left(s\right)\right\}+\left\{S\right\}\tilde{p}\left(s\right),$$

(28a)

$$\left\{B_0\left(s\right)\right\}={\left[V\right]}^{-1}\left\{L\right\}-{\left[V\right]}^{-1}〈\left[T_{31}\left(s,h\right)\right]\left\{S\right\}+\left\{T_{41}\left(s,h\right)\right\}〉\tilde{p}\left(s\right),$$

(28b)

where

$$\left[C\right]={\left[L_{11}\left(s,0\right)\right]}^{-1}\left[L_{10}\left(s,0\right)\right], \left\{L\right\}={\left\{-\tilde{p}(s), -\tilde{q}(s), -\tilde{g}(s)\right\}}^{\mathrm{T}},$$

$$\left\{S\right\}={\left[L_{11}\left(s,0\right)\right]}^{-1}〈\left\{L_{20}\left(s,0\right)\right\}-\left\{L_{21}\left(s,0\right)\right\}〉, \left[V\right]=\left[T_{31}\left(s,h\right)\right]\left[C\right],$$

$$\begin{array}{l}\left[L_{11}\left(s,0\right)\right]=\left[\begin{array}{c}{T}_{11}\left(s,0\right)\\ T_{31}\left(s,0\right)\end{array}\right], \left\{L_{21}\left(s,0\right)\right\}=\left\{\begin{array}{c}{T}_{21}\left(s,0\right)\\ T_{41}\left(s,0\right)\end{array}\right\}, \\ \left[L_{10}\left(s,0\right)\right]=\left[\begin{array}{c}{T}_{10}\left(s,0\right)\\ T_{30}\left(s,0\right)\end{array}\right], \left\{L_{20}\left(s,0\right)\right\}=\left\{\begin{array}{c}{T}_{20}\left(s,0\right)\\ T_{40}\left(s,0\right)\end{array}\right\}.\end{array}$$

By substituting Equation (28) into Equation (19) and performing Fourier inverse transformation, the displacement and potential of coating surface (z = h) can be obtained:

$${\left\{u_{xh}, u_{zh}, {\phi }_h\right\}}^{\mathrm{T}}={\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }\left[M_1\left(s,h\right)\right]\left\{L\right\}{e}^{\mathrm{i}sx}\mathrm{d}s+}{\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }\left\{M_2\left(s,h\right)\right\}\tilde{p}\left(s\right)e^{\mathrm{i}sx}\mathrm{d}s}.$$

(29)

where

$$\left[M_1\left(s,z\right)\right]=\left[T_{11}\left(s,h\right)\right]\left[C\right]{\left[V\right]}^{-1}, \left[\mathsf{\Phi }\right]=\left[T_{31}\left(s,h\right)\right]\left\{S\right\}+\left\{T_{41}\left(s,h\right)\right\},$$

$$\left\{M_2\left(s,z\right)\right\}=-\left[T_{11}\left(s,h\right)\right]\left[C\right]{\left[V\right]}^{-1}\left[\mathsf{\Phi }\right]+\left[T_{11}\left(s,h\right)\right]\left\{S\right\}+\left\{T_{21}\left(s,h\right)\right\}.$$

By performing asymptotic and parity analysis on matrices $\left[M_1(s,h)\right]$ and $\left\{M_2\left(s,h\right)\right\}$, it can be concluded that

$$\underset{s\to +\infty }{\lim }s\left[M_1\left(s,h\right)\right]=\left[\begin{array}{ccc}{f}_{11}^{\infty }& f_{12}^{\infty }& f_{13}^{\infty }\\ f_{21}^{\infty }& f_{22}^{\infty }& f_{23}^{\infty }\\ f_{31}^{\infty }& f_{32}^{\infty }& f_{33}^{\infty }\end{array}\right], \underset{s\to +\infty }{\lim }{s}^2\left[M_2\left(s,h\right)\right]=\left\{\begin{array}{c}{\theta }_1^{\infty }\\ {\theta }_2^{\infty }\\ {\theta }_3^{\infty }\end{array}\right\},$$

(30a)

$$f_{11}(-s)=-f_{11}(s), \hspace{0.33em}{f}_{12}(-s)=f_{12}(s), f_{13}(-s)=-f_{13}(s),$$

(30b)

$$f_{k1}(-s)=f_{k1}(s), f_{k2}(-s)=-f_{k2}(s), f_{k3}(-s)=f_{k3}(s),\hspace{0.33em}\hspace{0.33em}\hspace{0.33em}(k=2, 3),$$

(30c)

$${\theta }_1\left(-s\right)=-{\theta }_1\left(s\right), {\theta }_2\left(-s\right)={\theta }_2\left(s\right), {\theta }_3\left(-s\right)={\theta }_3\left(s\right).$$

(30d)

After separating the singularity term, Equation (29) can be expressed as

$$\begin{array}{cc}{\left\{u_{xh}, u_{zh}, {\phi }_h\right\}}^{\mathrm{T}}& ={\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }\left[{\mathsf{\Pi }}_1\right]\left\{L\right\}{e}^{\mathrm{i}sx}\mathrm{d}s}+{\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }\left\{\left[M_1\left(s,h\right)\right]-\left[{\mathsf{\Pi }}_1\right]\right\}\left\{L\right\}{e}^{\mathrm{i}sx}\mathrm{d}s}\hfill \\ & +{\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }\left\{{\mathsf{\Pi }}_2\right\}\tilde{p}\left(s\right)e^{\mathrm{i}sx}\mathrm{d}s}+{\displaystyle \frac{1}{2\pi }}{\displaystyle {\int }_{-\infty }^{+\infty }〈\left\{M_2\left(s,h\right)\right\}-\left\{{\mathsf{\Pi }}_2\right\}〉\tilde{p}\left(s\right)e^{\mathrm{i}sx}\mathrm{d}s},\hfill \end{array}$$

(31)

where

$$\left[{\mathsf{\Pi }}_1\right]={\displaystyle \frac{1}{s}}\left[\begin{array}{ccc}{f}_{11}^{\infty }& \mathrm{sign}(s)f_{12}^{\infty }& f_{13}^{\infty }\\ \mathrm{sign}(s)f_{21}^{\infty }& f_{22}^{\infty }& \mathrm{sign}(s)f_{23}^{\infty }\\ \mathrm{sign}(s)f_{31}^{\infty }& f_{32}^{\infty }& \mathrm{sign}(s)f_{33}^{\infty }\end{array}\right], \left\{{\mathsf{\Pi }}_2\right\}={\displaystyle \frac{1}{s^2}}{\left\{\mathrm{sign}\left(s\right){\theta }_1^{\infty },{\theta }_2^{\infty },{\theta }_3^{\infty }\right\}}^{\mathrm{T}}.$$

Because the rigid conductive punch slides slowly with a constant velocity on the coating surface, the contact pressure p(x) and tangential distributed force q(x) satisfy Coulomb’s friction law within the contact interval $-b\le x\le a$, i.e.,

$$p\left(x\right)=\mu q\left(x\right).$$

(32)

Take the derivative of Equation (32) with respect to x and use the following relation [28,29]:

$$\begin{array}{c}{\displaystyle {\int }_0^{+\infty }\sin \left[s\left(x-t\right)\right]}\mathrm{d}s={\displaystyle \frac{1}{x-t}}, {\displaystyle {\int }_0^{+\infty }\cos \left[s\left(x-t\right)\right]}\mathrm{d}s=\pi \delta \left(x-t\right),\\ {\displaystyle {\int }_0^{+\infty }\frac{\cos \left[s\left(x-t\right)\right]}{s}\mathrm{d}s=-\mathrm{In}\left|x-t\right|}, {\displaystyle {\int }_0^{+\infty }\frac{\sin \left[s\left(x-t\right)\right]}{s}\mathrm{d}s=\frac{\pi }{2}\mathrm{sign}\left(x-t\right)}.\end{array}$$

(33)

We can obtain

$$\begin{array}{cc}{\displaystyle \frac{\partial u_{xh}}{\partial x}}\hfill & =-\mathrm{i}{f}_{11}^{\infty }p\left(x\right)-\mathrm{i}{f}_{13}^{\infty }g\left(x\right)-{\displaystyle \frac{f_{12}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{\mu p\left(t\right)}{t-x}\mathrm{d}t}\hfill \\ & -{\displaystyle \frac{1}{\pi }}{\displaystyle {\int }_{-b}^a\left[p\left(t\right)K_{11}\left(x,t\right)+\mu p\left(t\right)K_{12}\left(x,t\right)+g\left(t\right)K_{13}\left(x,t\right)\right]}\mathrm{d}t\hfill \\ & -{\displaystyle \frac{\mathrm{i}{\theta }_1^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)\mathrm{In}\left|x-t\right|\mathrm{d}t}+{\displaystyle \frac{\mathrm{i}}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)K_{14}\left(x,t\right)\mathrm{d}t},\hfill \end{array}$$

(34a)

$$\begin{array}{cc}{\displaystyle \frac{\partial u_{zh}}{\partial x}}\hfill & =-\mathrm{i}{f}_{22}^{\infty }\mu p\left(x\right)-{\displaystyle \frac{f_{21}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{p\left(t\right)}{t-x}\mathrm{d}t}-{\displaystyle \frac{f_{23}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{g\left(t\right)}{t-x}\mathrm{d}t}\hfill \\ & -{\displaystyle \frac{1}{\pi }}{\displaystyle {\int }_{-b}^a\left[p\left(t\right)K_{21}\left(x,t\right)+\mu p\left(t\right)K_{22}\left(x,t\right)+g\left(t\right)K_{23}\left(x,t\right)\right]}\mathrm{d}t\hfill \\ \hfill & -{\displaystyle \frac{{\theta }_2^{\infty }}{2}}{\displaystyle {\int }_{-b}^{a}p\left(t\right)\mathrm{sign}\left(x-t\right)\mathrm{d}t}-{\displaystyle \frac{1}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)K_{24}\left(x,t\right)\mathrm{d}t},\end{array}$$

(34b)

$$\begin{array}{cc}{\displaystyle \frac{\partial {\phi }_h}{\partial x}}\hfill & =-\mathrm{i}\mu f_{32}^{\infty }p\left(x\right)-{\displaystyle \frac{f_{31}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{p\left(t\right)}{t-x}\mathrm{d}t}-{\displaystyle \frac{f_{33}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{g\left(t\right)}{t-x}\mathrm{d}t}\hfill \\ & -{\displaystyle \frac{1}{\pi }}{\displaystyle {\int }_{-b}^a\left[p\left(t\right)K_{31}\left(x,t\right)+\mu p\left(t\right)K_{32}\left(x,t\right)+g\left(t\right)K_{33}\left(x,t\right)\right]}\mathrm{d}t\hfill \\ & -{\displaystyle \frac{{\theta }_3^{\infty }}{2}}{\displaystyle {\int }_{-b}^{a}p\left(t\right)\mathrm{sign}\left(x-t\right)\mathrm{d}t}-{\displaystyle \frac{1}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)K_{34}\left(x,t\right)\mathrm{d}t}.\hfill \end{array}$$

(34c)

where $K_{ij}\left(i=1, 2, 3; j=1, 2, 3, 4\right)$ can be found in Appendix A. In addition, the total pressure P and total charge Γ with p(x) and g(x) satisfy the following equilibrium conditions:

$${\displaystyle {\int }_{-b}^{a}p\left(t\right)\mathrm{d}t=P},$$

(35a)

$${\displaystyle {\int }_{-b}^{a}g\left(t\right)}\mathrm{d}t=\mathrm{\Gamma }.$$

(35b)

3. Solution of Singular Integral Equations

In this study, the width of the contact area is much smaller than the radius of the conductive cylindrical punch, so the cylindrical shape can be approximated as a parabolic shape [30]. On the other hand, assuming that the surface potential ${\phi }_h$ is constant in the contact region, the partial derivatives of $u_{zh}$ and ${\phi }_h$ can be written as

$${\displaystyle \frac{\partial u_{zh}}{\partial x}}={\displaystyle \frac{x}{R}},\hspace{1em}\hspace{1em}{\displaystyle \frac{\partial {\phi }_h}{\partial x}}=0, \left(-b\le x\le a\right).$$

(36)

The normal contact pressure $p\left(x\right)$ is smooth at the edge of the contact region [11,31] $\left(x=-b, x=a\right)$. For a conductive cylindrical punch, charge g(x) can be written as

$$g\left(x\right)=g_1\left(x\right)+g_2\left(x\right).$$

(37)

where g₁(x) is the surface charge distribution caused by normal load P, and it is smooth at the edge of the contact region; g₂(x) is the surface charge distribution caused by the total potential $\phi$ and has a −1/2 singularity in the contact region. Furthermore, perform the following transforms on x and t:

$$t={\displaystyle \frac{a+b}{2}}\eta +{\displaystyle \frac{a-b}{2}}, x={\displaystyle \frac{a+b}{2}}\zeta +{\displaystyle \frac{a-b}{2}}, -b\le \left(t,x\right)\le a, -1\le \left(\eta ,\zeta \right)\le 1.$$

(38)

Then, Equation (34b,c) can be expressed as

$$\begin{array}{l}\mathrm{i}\mu f_{22}^{\infty }p\left(\zeta \right)+{\displaystyle \frac{f_{21}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{p\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+{\displaystyle \frac{f_{23}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{g_1\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+\\ {\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1\left[p\left(\eta \right)K_{21}\left(\zeta ,\eta \right)+\mu p\left(\eta \right)K_{22}\left(\zeta ,\eta \right)+g_1\left(\eta \right)K_{23}\left(\zeta ,\eta \right)\right]}\mathrm{d}\eta +\\ {\displaystyle \frac{{\theta }_2^{\infty }(a+b)}{4}}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)\mathrm{sign}\left(\zeta -\eta \right)\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)K_{24}\left(\zeta ,\eta \right)\mathrm{d}\eta }=-{\displaystyle \frac{\left(a+b\right)\zeta +\left(a-b\right)}{2R}},\end{array}$$

(39a)

$$\begin{array}{l}\mathrm{i}\mu f_{32}^{\infty }p\left(\zeta \right)+{\displaystyle \frac{f_{31}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{p\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+{\displaystyle \frac{f_{33}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{g_1\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+\\ {\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1\left[p\left(\eta \right)K_{31}\left(\zeta ,\eta \right)+\mu p\left(\eta \right)K_{32}\left(\zeta ,\eta \right)+g_1\left(\eta \right)K_{33}\left(\zeta ,\eta \right)\right]}\mathrm{d}\eta +\\ {\displaystyle \frac{{\theta }_3^{\infty }(a+b)}{4}}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)\mathrm{sign}\left(\zeta -\eta \right)\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)K_{34}\left(\zeta ,\eta \right)\mathrm{d}\eta }=0,\end{array}$$

(39b)

$${\displaystyle {\int }_{-1}^{1}p\left(\eta \right)\mathrm{d}\eta =\frac{2P}{a+b}},$$

(39c)

$${\displaystyle {\int }_{-1}^1{g}_1\left(\eta \right)\mathrm{d}\eta =\frac{2{\mathrm{\Gamma }}_1}{a+b}},$$

(39d)

$${\displaystyle \frac{f_{23}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{g_2\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1{K}_{23}\left(\zeta ,\eta \right)g_2\left(\eta \right)\mathrm{d}\eta }=0,$$

(39e)

$${\displaystyle {\int }_{-1}^1{g}_2\left(\eta \right)\mathrm{d}\eta }={\displaystyle \frac{2\left(\mathrm{\Gamma }-{\mathrm{\Gamma }}_1\right)}{a+b}}.$$

(39f)

It can be found that Equation (39a,b) are the Cauchy singular integral equations of the first kind for g₁(x) and the Cauchy singular integral equations of the second kind for p(x). Here, the coupled singular integral Equation (39a–d) are first solved to obtain p(x) and g₁(x). Then, the first Cauchy singular integral Equation (39e) is numerically solved to obtain g₂(x).

To solve the coupled Cauchy singular integral Equation (39a–d), Equation (39a,b) can be represented as follows:

$$\begin{array}{l}\mathrm{i}\mu f_{22}^{\infty }p\left(\zeta \right)+{\displaystyle \frac{f_{21}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{p\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1\left[p\left(\eta \right)K_{21}\left(\zeta ,\eta \right)+\mu p\left(\eta \right)K_{22}\left(\zeta ,\eta \right)\right]}\mathrm{d}\eta +\\ {\displaystyle \frac{{\theta }_2^{\infty }(a+b)}{4}}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)\mathrm{sign}\left(\zeta -\eta \right)\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)K_{24}\left(\zeta ,\eta \right)\mathrm{d}\eta }=H_1\left(\zeta \right),\end{array}$$

(40a)

$${\displaystyle \frac{f_{33}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{g_1\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }+{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1{g}_1\left(\eta \right)K_{33}\left(\zeta ,\eta \right)}\mathrm{d}\eta =H_2\left(\zeta \right),$$

(40b)

where

$$H_1=-{\displaystyle \frac{\left(a+b\right)\zeta +\left(a-b\right)}{2R}}-{\displaystyle \frac{f_{23}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{g_1\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }-{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1{g}_1\left(\eta \right)K_{23}\left(\zeta ,\eta \right)}\mathrm{d}\eta ,$$

$$\begin{array}{cc}{H}_2& =-{\displaystyle \frac{f_{31}^{\infty }}{\pi }}{\displaystyle {\int }_{-1}^1\frac{p\left(\eta \right)}{\eta -\zeta }\mathrm{d}\eta }-\mathrm{i}\mu f_{32}^{\infty }p\left(\zeta \right)-{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^1\left[p\left(\eta \right)K_{31}\left(\zeta ,\eta \right)+\mu p\left(\eta \right)K_{32}\left(\zeta ,\eta \right)\right]}\mathrm{d}\eta \\ & -{\displaystyle \frac{{\theta }_3^{\infty }(a+b)}{4}}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)\mathrm{sign}\left(\zeta -\eta \right)\mathrm{d}\eta }-{\displaystyle \frac{a+b}{2\pi }}{\displaystyle {\int }_{-1}^{1}p\left(\eta \right)K_{34}\left(\zeta ,\eta \right)\mathrm{d}\eta }.\hfill \end{array}$$

The second Cauchy singular integral Equation (40a) is solved using Krenk’s numerical method [32]. The contact pressure is expressed as

$$p\left(\eta \right)=w_1\left(\eta \right){\left(1-\eta \right)}^{{\delta }_1}{\left(1+\eta \right)}^{{\delta }_2}, \left(-1\le \eta \le 1\right),$$

(41)

where

$${\delta }_1={\displaystyle \frac{1}{\pi }}\arctan \left[{\displaystyle \frac{f_{21}^{\infty }}{\mathrm{i}\mu \left(-f_{22}^{\infty }\right)}}\right]+N_0, {\delta }_2=-{\displaystyle \frac{1}{\pi }}\arctan \left[{\displaystyle \frac{f_{21}^{\infty }}{\mathrm{i}\mu \left(-f_{22}^{\infty }\right)}}\right]+M_0.$$

N₀ and M₀ are arbitrary integers and satisfy

$$\kappa =-\left({\delta }_1+{\delta }_2\right)=-\left(N_0+M_0\right).$$

(42)

Then, Equation (39a,c) can be resolved into a set of linear equations [32,33]:

$${\displaystyle \sum _{l=1}^M{W}_l^M\left\{\frac{f_{21}^{\infty }}{{\eta }_l-{\zeta }_r}+\frac{a+b}{2}\left[\begin{array}{l}{K}_{21}\left({\zeta }_r,{\eta }_l\right)+\mu K_{22}\left({\zeta }_r,{\eta }_l\right)+\\ \frac{\pi {\theta }_2^{\infty }}{2}\mathrm{sign}\left({\zeta }_r-{\eta }_l\right)+K_{24}\left({\zeta }_r,{\eta }_l\right)\end{array}\right]\right\}}{w}_1\left({\eta }_l\right)=H_1\left({\zeta }_r\right),$$

(43a)

$${\displaystyle \sum _{l=1}^M{W}_l^M{w}_1\left({\eta }_l\right)=\frac{2P}{\left(a+b\right)\pi }}.$$

(43b)

where $M$ is the number of discrete points within the contact region (−1, 1), and ${\eta }_l$, ${\zeta }_r$, and $W_l^M$ are determined by the following equations, where $P_M^{\left({\delta }_1,{\delta }_2\right)}\left(\cdot \right)$ is the Jacobi polynomial, and $\mathrm{\Gamma }\left(\cdot \right)$ is the Gamma function:

$$P_M^{\left({\delta }_1,{\delta }_2\right)}\left({\eta }_l\right)=0, \left(l=1, 2,\cdots , M\right),$$

$$P_{M-\kappa }^{\left(-{\delta }_1,-{\delta }_2\right)}\left({\zeta }_r\right)=0, \left(r=1, 2,\cdots ,M-\kappa \right),$$

$$W_l^M=-2^{-\kappa }{\displaystyle \frac{\mathrm{\Gamma }\left({\delta }_1\right)\mathrm{\Gamma }\left(1-{\delta }_1\right)}{\pi }}{\displaystyle \frac{P_{M-\kappa }^{\left(-{\delta }_1,-{\delta }_2\right)}\left({\eta }_l\right)}{P_M^{{\left({\delta }_1,{\delta }_2\right)}^{\prime }}\left({\eta }_l\right)}}.$$

The numerical method of Erdogan and Gupta [34] was used to solve the first Cauchy singular integral Equation (40b), and we let $g_1\left(\eta \right)={\lambda }_1\left(\eta \right)\sqrt{1-{\eta }^2}$ as well as discrete Equations (39d) and (40b) be linear equations as follows:

$${\displaystyle \sum _{l=1}^M\frac{\left(1-{\eta }_l^2\right)}{M+1}\left\{\left[\frac{f_{33}^{\infty }}{{\eta }_l-{\zeta }_r}+\frac{a+b}{2}{K}_{33}\right]{\lambda }_1\left({\eta }_l\right)\right\}}=H_2\left({\zeta }_r\right),$$

(44a)

$${\displaystyle \sum _{l=1}^M\frac{\left(1-{\eta }_l^2\right)}{M+1}{\lambda }_1\left({\eta }_l\right)}={\displaystyle \frac{2{\mathrm{\Gamma }}_1}{\left(a+b\right)\pi }}.$$

(44b)

where $M$ is the number of discrete points within the contact interval (−1, 1), and

$${\eta }_l=\cos \left[l\pi /\left(M+1\right)\right], {\zeta }_r=\cos \left[\left(2r-1\right)\pi /2\left(M+1\right)\right], \left(r=1, 2, \cdots , M+1\right).$$

It can be found that there are $2M+4$ equations (Equation (44a,b)) and $2M+3$ unknowns w₁(η₁)…w₁(η_M), λ₁(η₁)…λ₁(η_M), a, b, and Γ₁. This is a typical super-qualitative system of equations, and it is difficult to obtain accurate solutions for the normal contact pressure and charge distribution. Here, the iterative method and least-squares numerical values in reference [15] are used to solve coupled Equations (43) and (44), and the optimal solutions of normal contact pressure p(x) and charge distribution g₁(x) are obtained. The specific solving steps are as follows:

(1)

Ignore the friction and solve the corresponding frictionless contact problem. Give the values of P and Γ, solve for frictionless problem Equation (46) with p₍₀₎(η) and g₁₍₀₎(η) as initial values for p(η) and g₁(η).

(2)

Solve the friction contact problem for the approximation value. Substituting the initial value of g₁₍₀₎(η) into Equation (43a) and keeping P constant, the contact zone dimensions a, b, and normal contact pressure p₍₁₎(η). Then, applying the least-squares method, substitute p₍₁₎(η) into Equation (44a) to solve for the approximation value g₁₍₁₎(η) of g₁(η).

(3)

Iterate for the final values. Repeatedly iterate step (2) until the relative error between the two successive iterations is no more than 0.1%. The final values are obtained as p_(n)(η) and g_1(n)(η).

After obtaining the normal contact pressure p(x) and charge g₁(x), continue solving Equation (39e,f) to obtain g₂(x). Using the numerical method of Erdogan and Gupta [34], assuming that $g_2\left(\eta \right)={\lambda }_2\left(\eta \right)/\sqrt{1-{\eta }^2}$, Equation (39e,f) can be discretized as

$${\displaystyle \frac{1}{M}}{\displaystyle \sum _{l=1}^M{\lambda }_2\left({\eta }_l\right)\left[\frac{f_{23}^{\infty }}{{\eta }_l-{\zeta }_r}+\frac{a+b}{2}{K}_{23}\left({\zeta }_r,{\eta }_l\right)\right]}=0,$$

(45a)

$${\displaystyle \frac{1}{M}}{\displaystyle \sum _{l=1}^M{\lambda }_2\left({\eta }_l\right)}={\displaystyle \frac{2\left(\mathrm{\Gamma }-{\mathrm{\Gamma }}_1\right)}{\left(a+b\right)\pi }}.$$

(45b)

where ${\eta }_l=\cos \left[\left(2l-1\right)\pi /2M\right], {\zeta }_r=\cos \left[r\pi /M\right], \left(r=1, 2,\cdots ,M-1\right)$; M is the total number of discrete points in interval (−1,1). By solving the system of linear equations in Equation (45a,b), the charge distribution on discrete points g₂(x₁)…g₂(x_M) can be obtained, and then the charge distribution in the contact region g₂(x) can be obtained via interpolation.

After obtaining the surface normal contact stress σ_zzh = σ_zz(x,h) = −p(x), charge distribution D_zh = D_z(x,h) = −g(x), and temperature Ω_h = Ω(x,h), the in-plane stress σ_xxh = σ_xx(x,h) and in-plane electrical displacement D_xh = D_x(x,h) of the coating contact surface can be expressed as

$$\begin{array}{cc}{\sigma }_{xxh}\left(x\right)& =-\left({\Delta }_1+\mathrm{i}{f}_{11}^{\infty }{\Delta }_3\right)p\left(x\right)-\left({\Delta }_2+\mathrm{i}{f}_{13}^{\infty }{\Delta }_3\right)g\left(x\right)-{\displaystyle \frac{\mu f_{12}^{\infty }{\Delta }_3}{\pi }}{\displaystyle {\int }_{-b}^a\frac{p\left(t\right)}{t-x}\mathrm{d}t}\\ & -{\displaystyle \frac{{\Delta }_3}{\pi }}{\displaystyle {\int }_{-b}^a\left[p\left(t\right)K_{11}\left(x,t\right)+\mu p\left(t\right)K_{12}\left(x,t\right)+g\left(t\right)K_{13}\left(x,t\right)\right]\mathrm{d}t}\hfill \\ & -{\displaystyle \frac{\mathrm{i}{\Delta }_3{\theta }_1^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)\ln \left|t-x\right|\mathrm{d}t}+{\displaystyle \frac{{\Delta }_3}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)K_{14}\left(x,t\right)\mathrm{d}t}+{\Delta }_4{\mathrm{\Omega }}_h\left(x\right),\hfill \end{array}$$

(46)

$$\begin{array}{cc}{D}_{xh}\left(x\right)& =-\left({\displaystyle \frac{e_{150}}{c_{440}}}-\mathrm{i}{f}_{32}^{\infty }{\Delta }_5\right)\mu p\left(x\right)+{\displaystyle \frac{{\Delta }_5{f}_{31}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{p\left(t\right)}{t-x}\mathrm{d}t}+{\displaystyle \frac{{\Delta }_5{f}_{33}^{\infty }}{\pi }}{\displaystyle {\int }_{-b}^a\frac{g\left(t\right)}{t-x}\mathrm{d}t}\\ & -{\displaystyle \frac{{\Delta }_5}{\pi }}{\displaystyle {\int }_{-b}^a\left[p\left(x\right)K_{31}\left(x,t\right)-\mu p\left(x\right)K_{32}\left(x,t\right)+g\left(x\right)K_{33}\left(x,t\right)\right]\mathrm{d}t}\hfill \\ & +{\displaystyle \frac{{\Delta }_5{\theta }_3^{\infty }}{2}}{\displaystyle {\int }_{-b}^{a}p\left(t\right)\mathrm{sign}\left(x-t\right)\mathrm{d}t}+{\displaystyle \frac{{\Delta }_5}{\pi }}{\displaystyle {\int }_{-b}^{a}p\left(t\right)K_{34}\left(x,t\right)\mathrm{d}t},\hfill \end{array}$$

(47)

where

$${\Delta }_1={\displaystyle \frac{e_{310}{e}_{330}+c_{130}{\epsilon }_{330}}{e_{330}^2+c_{330}{\epsilon }_{330}}}, {\Delta }_2={\displaystyle \frac{c_{130}{e}_{330}-c_{330}{e}_{310}}{e_{330}^2+c_{330}{\epsilon }_{330}}},$$

$${\Delta }_3=\left[{\displaystyle \frac{c_{110}{e}_{330}^2-c_{130}^2{\epsilon }_{330}+c_{330}{e}_{310}^2+c_{110}{c}_{330}{\epsilon }_{330}-2c_{130}{e}_{310}{e}_{330}}{e_{330}^2+c_{330}{\epsilon }_{330}}}\right]e^{\beta h},$$

$${\Delta }_4=\left[{\displaystyle \frac{c_{130}{\epsilon }_{330}{\lambda }_{330}-e_{330}^2{\lambda }_{110}+e_{130}{e}_{330}{\lambda }_{330}-{\epsilon }_{330}{\lambda }_{110}{c}_{330}+{\alpha }_{30}{e}_{330}{c}_{130}-e_{130}{\alpha }_{30}{c}_{330}}{e_{330}^2+c_{330}{\epsilon }_{330}}}\right]e^{\beta h},$$

$${\Delta }_5=\left({\displaystyle \frac{e_{150}^2}{c_{440}}}+{\epsilon }_{110}\right)e^{\beta h}.$$

4. Numerical Results and Discussion

Based on the above analysis, the numerical results of the stress component, electric displacement, and surface temperature are given in this section. The effects of graded index βh, friction coefficient μ, and punch sliding velocity V on the thermal friction contact behavior of the FGPM coating were investigated. It was assumed that the FGPM coating was composed of cadmium selenide material, and its thermo-electro-elastic parameters are shown in

Table 1. Thermal piezoelectric and elastic parameters of cadmium selenide [22].

c110 (GPa)	c130 (GPa)	c330 (GPa)	c440 (GPa)
74.1	39.3	83.6	13.2
e310 (C/m2)	e330 (C/m2)	e150 (C/m2)	ε110 (10−10 C/Vm)
−0.16	0.347	0.138	0.825
ε330 (10−10 C/Vm)	λ110 (106 Nm−2K−1)	λ330 (106 Nm−2K−1)	α30 (10−6 C/Km2)
0.902	0.621	0.551	22.94
k10 (W/Km)	k30 (W/Km)	c0 (Wskg−1K−1)	ρ0 (Kg/m3)
9	9	490	5816

[22]. Without a special state, the total pressure $P=2\times {10}^4 \mathrm{KN}/\mathrm{m}$, the total charge $\mathrm{\Gamma }=1.8\times {10}^{-4} \mathrm{C}/\mathrm{m}$, the cylinder radius R = 0.1 m, and the coating thickness h = 0.01 m. For the sake of discussion, let us define dimensionless quantities: ${\sigma }^{*}=P/h$, $D^{*}=\mathrm{\Gamma }/h$, and ${\mathrm{\Omega }}^{*}=k^{*}P/\left(c_0{\rho }_{0}h\right)$, in which $k^{*}=k_{30}/k_{10}$.

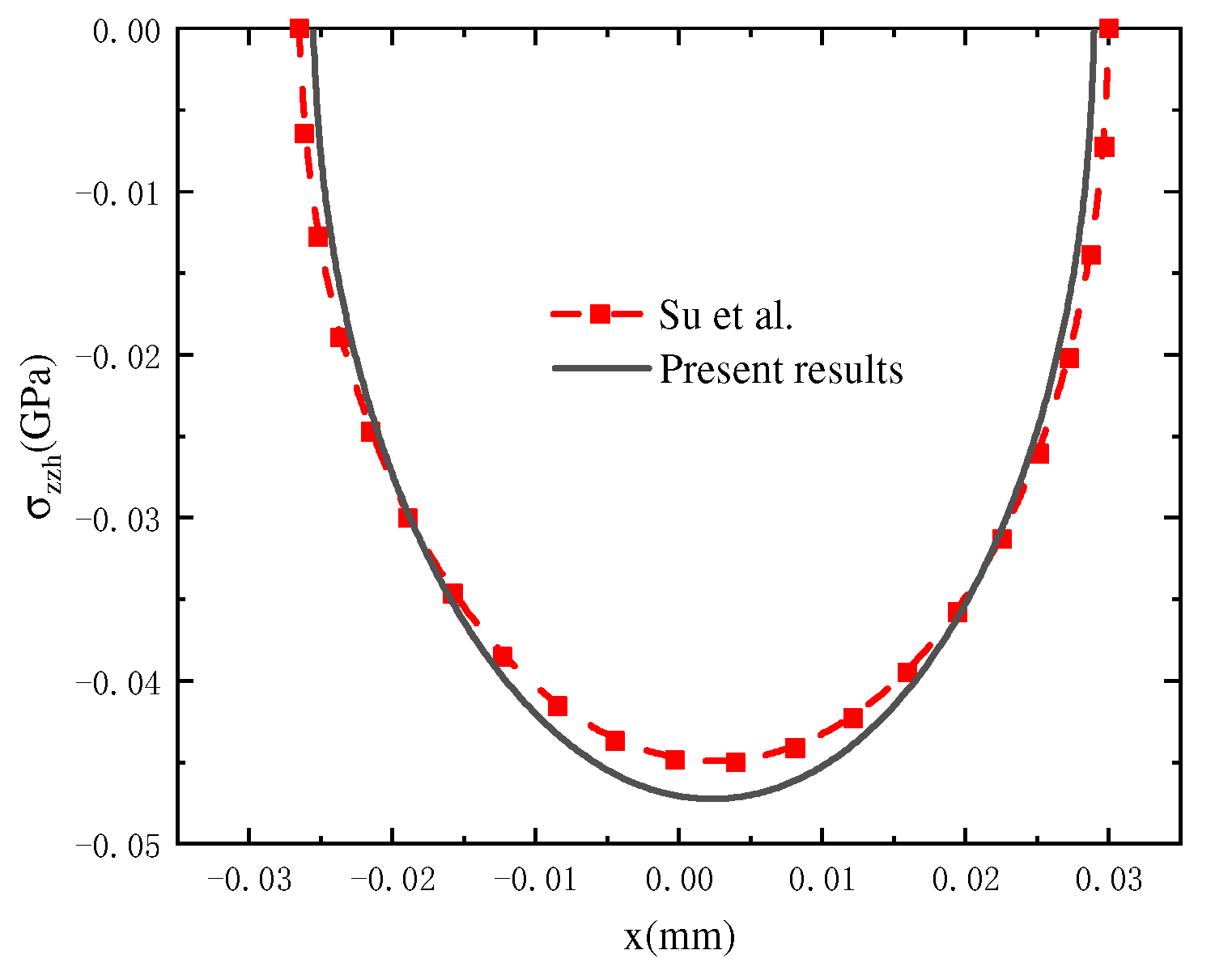
To verify the validity of this model, ignoring the frictional heat effect, the problem was reduced to the elastic frictional contact problem of an FGPM coating structure. Figure 2 shows the distribution of normal contact stress σ_zzh, electrical displacement D_zh, and in-plane stress σ_xxh on the surface of the FGPM coating with friction coefficient μ = 0.3, and cylindrical radius R = 0.06 m. The curves are the results of this paper, and the discrete points are the results of Su et al. [15]. It was found that the present results agree very well with Su et al.’s results.

Figure 3 compares the normal contact stress in the contact problem of a punch, considering and ignoring the friction heat effect. Specifically, it compares the present results with those of Su et al. The figure shows that the normal contact pressure increases due to the influence of friction heat. Therefore, the friction heat effect significantly influences the normal contact stress, making it necessary to consider friction heat in friction contact problems.

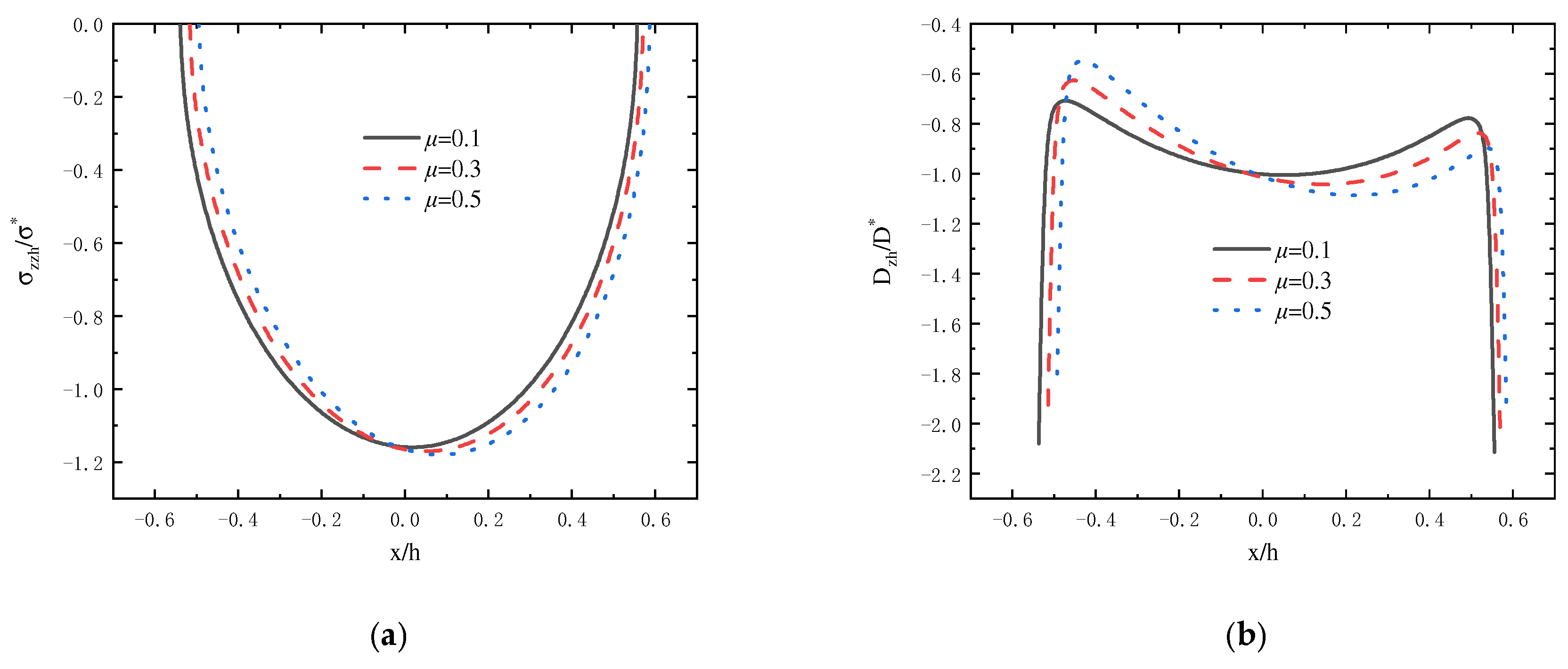
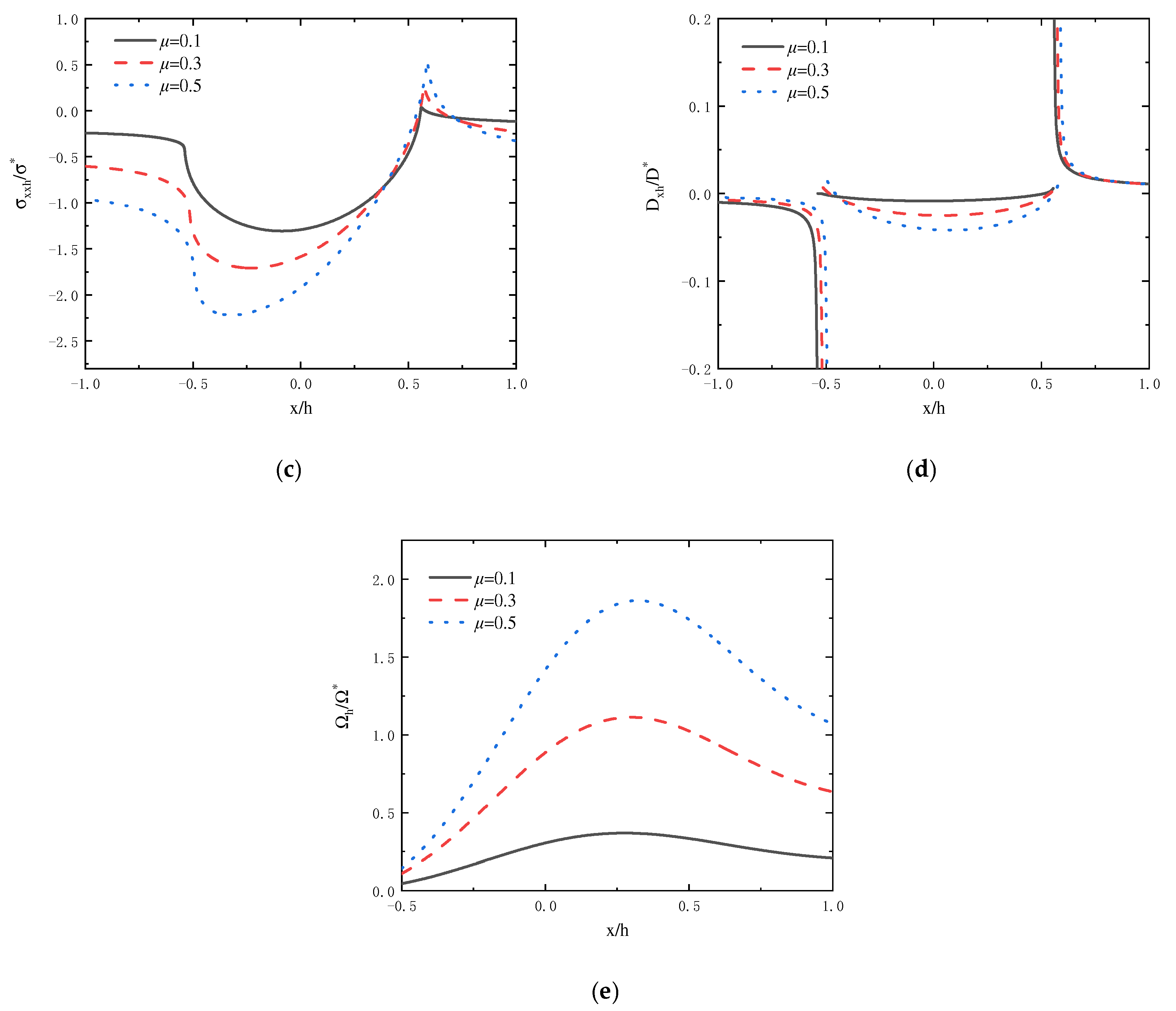
Figure 4 illustrates the impact of the friction coefficient μ on the thermal friction contact characteristics of the graded piezoelectric coating structure. The results indicate that both the maximum internal stress σ_xxh (Figure 4c) and the maximum surface temperature Ω_h (Figure 4e) at the tail end increase with the friction coefficient μ. The highest temperature is observed in the center of the contact region, attributed to the concentration of surface contact stress in the center of the cylindrical punch. The figure also shows that the friction coefficient μ affects the surface normal contact stress σ_zzh (Figure 4a), surface electrical displacement D_zh (Figure 4b), and in-plane electrical displacement D_xh (Figure 4d). Moreover, the distribution of σ_zzh and D_zh in the contact region is asymmetrical due to frictional effects. Reducing the friction coefficient can effectively mitigate surface contact damage.

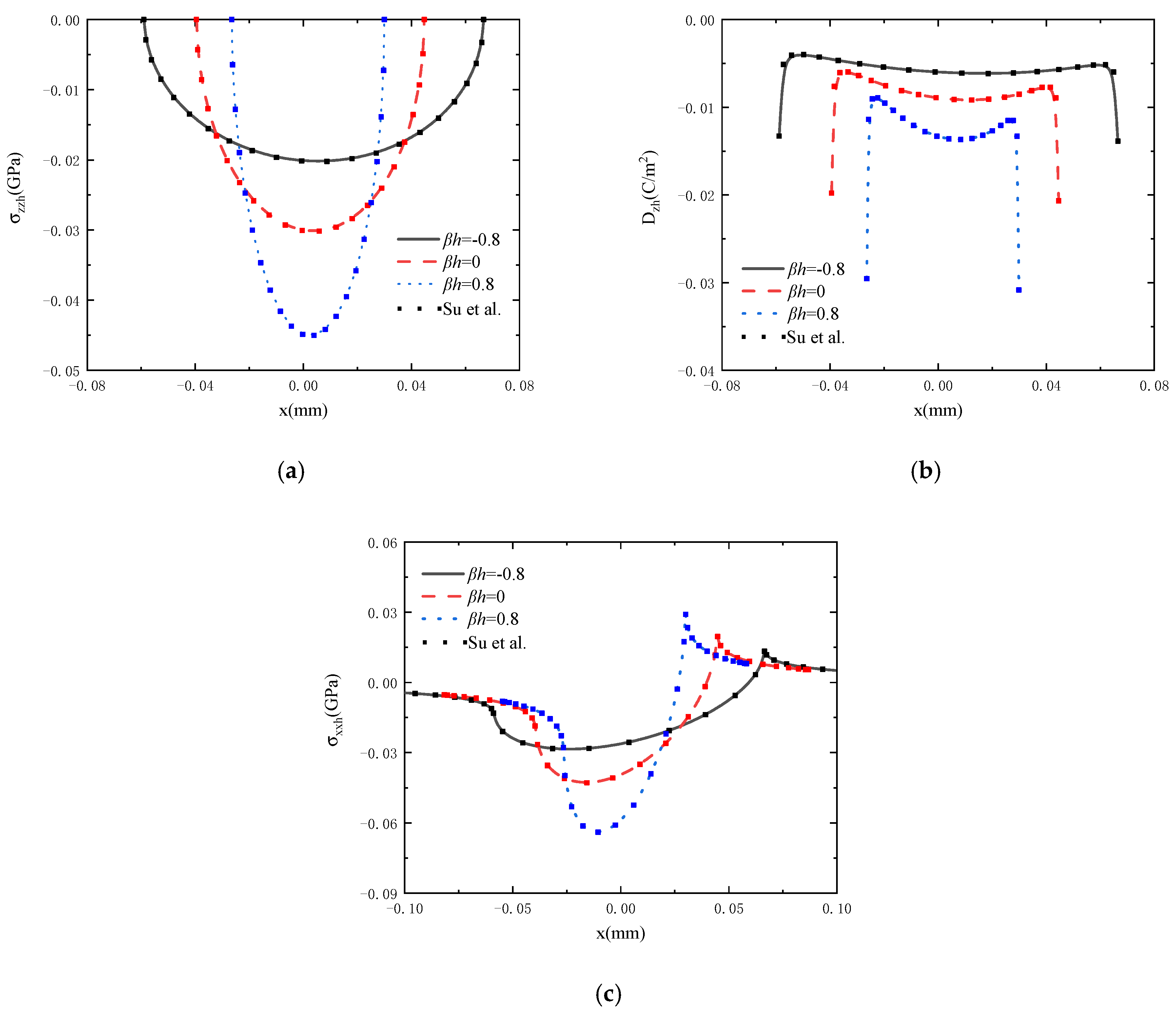
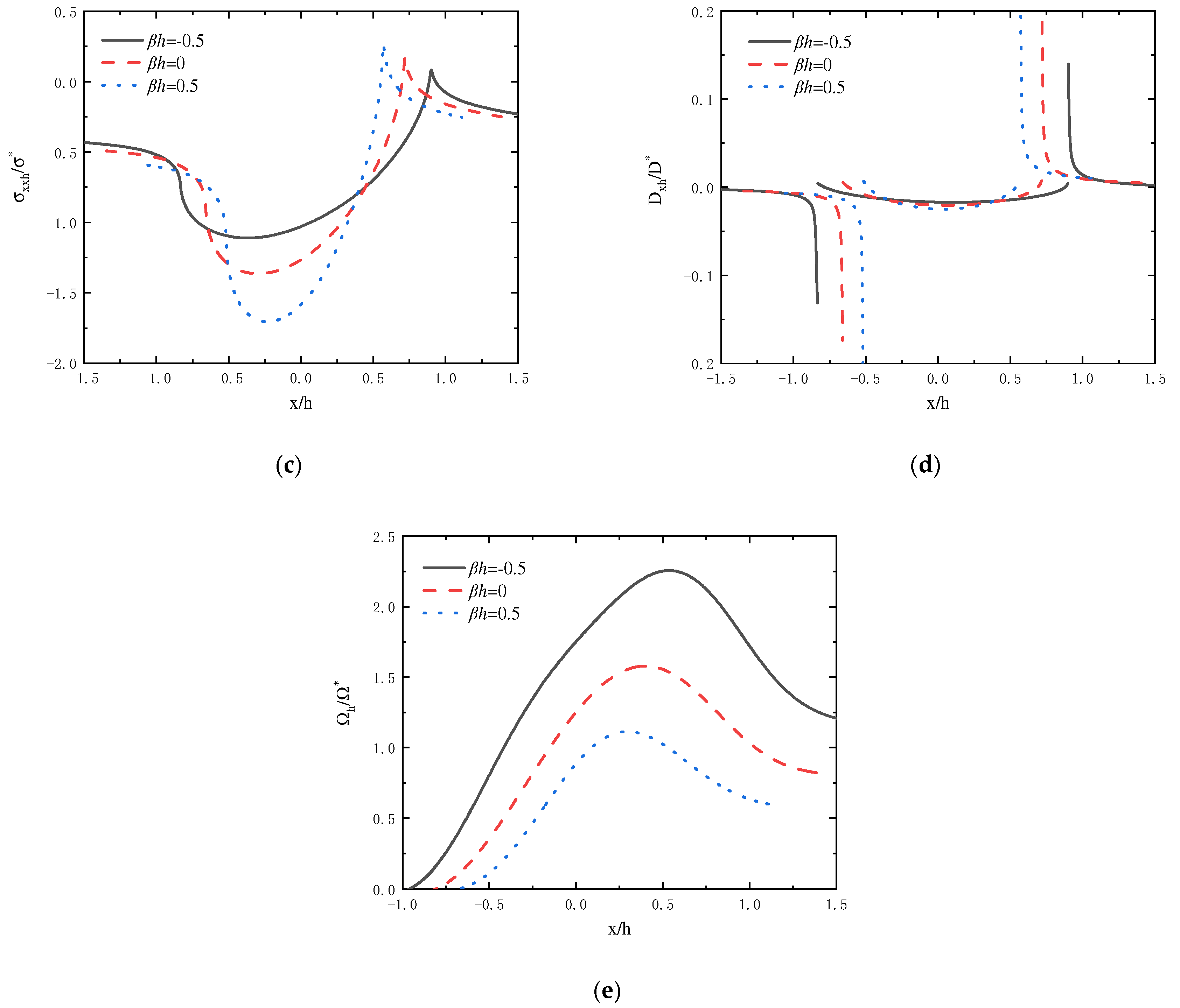
The graded index, a parameter reflecting material inhomogeneity [22], can be valued in three ways, determining the performance of FGPMs. A positive graded index (βh > 0) indicates in a coating that hardens in the thickness direction, while a negative index (βh < 0) indicates a softening coating. When βh = 0, the material is a uniform piezoelectric layer. Figure 5 demonstrates the effect of the graded index βh of the coated thermo-electro-elastic material on the thermal friction contact characteristics of the graded piezoelectric coating structure. As the graded index βh increases, the maximum values of σ_zzh (Figure 5a) and D_zh (Figure 5b) significantly increase, but the contact region size decreases. Notably, the maximum in-plane stress peak σ_xxh at the tail of the contact zone (Figure 5c) also increases with βh, potentially leading to fatigue crack generation. Figure 5e shows that the highest surface temperature Ω_h values occur in the contact center and decrease with increasing βh, as the normal contact stress largely determines the surface temperature field [21]. The in-plane electric displacement D_xh (Figure 5d) exhibits a singularity at the contact region edge, decreasing at the front but increasing at the tail with increasing βh. The results indicate that adjusting the graded index can enhance the surface resistance to contact damage.

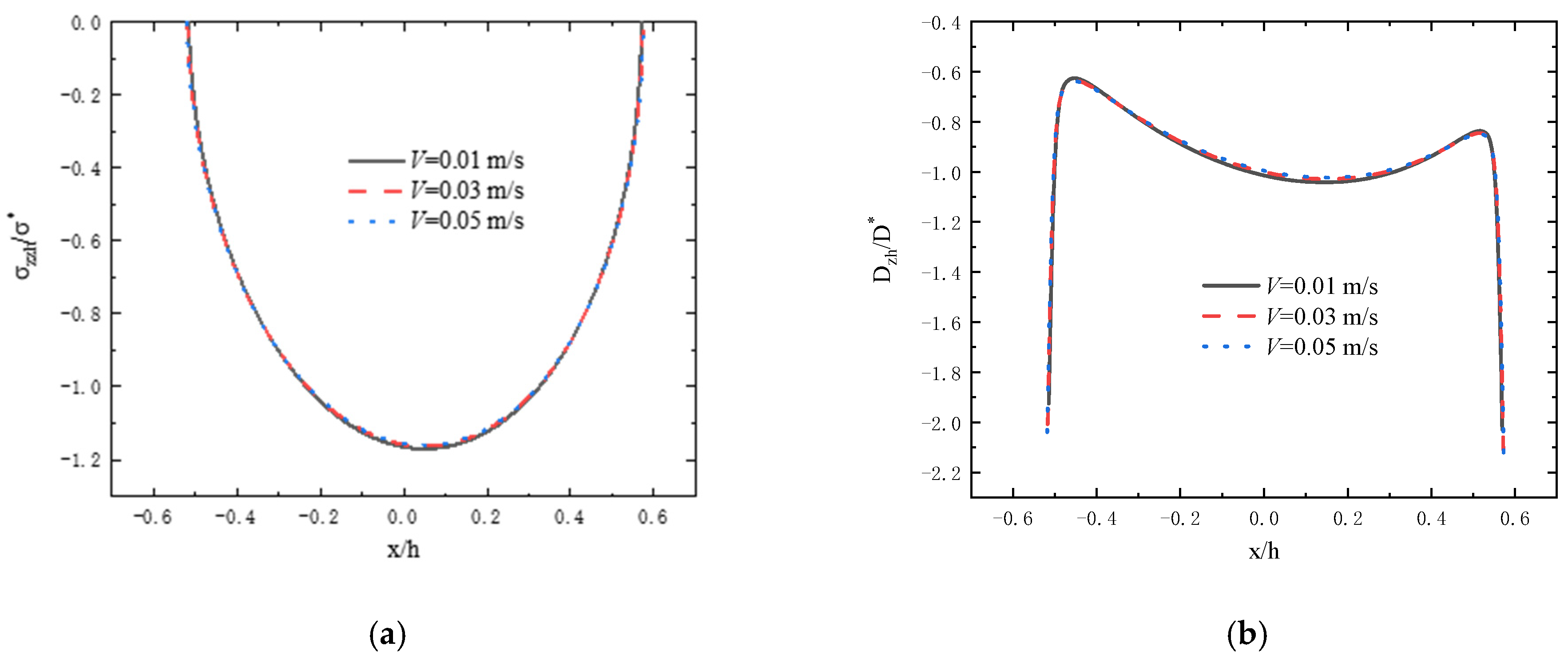
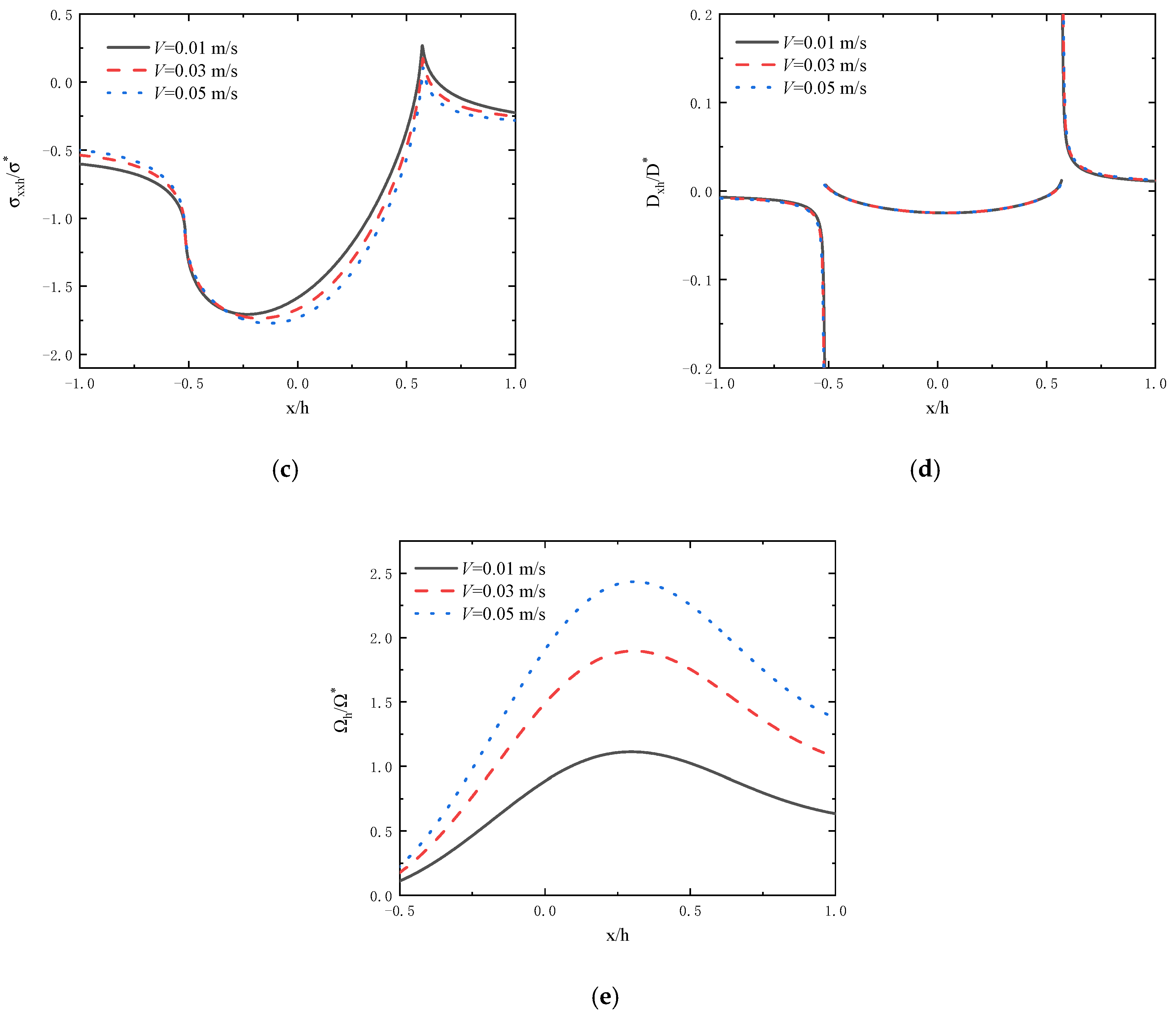
Figure 6 illustrates the effect of the punch sliding velocity V on the thermal friction contact characteristics of the graded piezoelectric coating structure. The sliding velocity V has minimal impact on the surface contact stress (Figure 6a) and electrical behavior (Figure 6b,d). However, the in-plane stress σ_xxh (Figure 6c) changes significantly at the end of the contact zone, with its maximum value decreasing as the sliding velocity increases. This is attributed to frictional heat weakening the surface in-plane stress, indicating a compressive effect of internal in-plane stress on the hot surface [35]. Additionally, the surface temperature field (Figure 6e) increases with the sliding velocity, as a higher velocity leads to increased heat flow from the contact zone. The results highlight that changes in sliding velocity most significantly affect the contact surface temperature.

5. Conclusions

In this paper, the sliding friction contact between an FGPM-coated half-plane and rigid conductive cylindrical punch was studied. It was assumed that the coating material parameters vary exponentially along the thickness direction. The effects of friction heat and thermal convection terms were considered. The distribution of contact stress, electric displacement, and surface temperature on the surface of FGPM coating was determined by using the least-squares and iterative methods. The effects of sliding velocity, friction coefficient, and graded index on the thermal friction contact characteristics of FGPM coating were discussed in detail. The conclusions are as follows:

• For the sliding contact problem of a rigid conductive cylindrical punch, the maximum in-plane stress occurs at the contact region’s end, indicating a potential damage site. This stress increases at higher graded indices and friction coefficients, suggesting that adjusting these parameters can mitigate the thermo-electro-elastic contact damage on frictional surfaces.
• The graded index of the coating material, varying from −0.5 to 0.5, significantly affects the distribution of the surface contact stress and electrical displacement. Thus, adjusting the coating gradient can alter these distributions, suggesting that graded coatings can mitigate thermo-electro-elastic contact damage.
• Varying the sliding velocity from 0.01 m/s to 0.05 m/s, the friction coefficient from 0.1 to 0.5, and the graded index from 0.5 to −0.5 increases the surface temperature. This is due to the increased heat generation from higher friction coefficients and sliding speeds. Thus, adjusting these parameters can reduce surface friction heat and suppress thermal contact damage.