PECVD Synthesis and Thermoelectric Properties of Thin Films of Lead Chalcogenides (PbTe)1−x(PbS)x
Abstract
1. Introduction
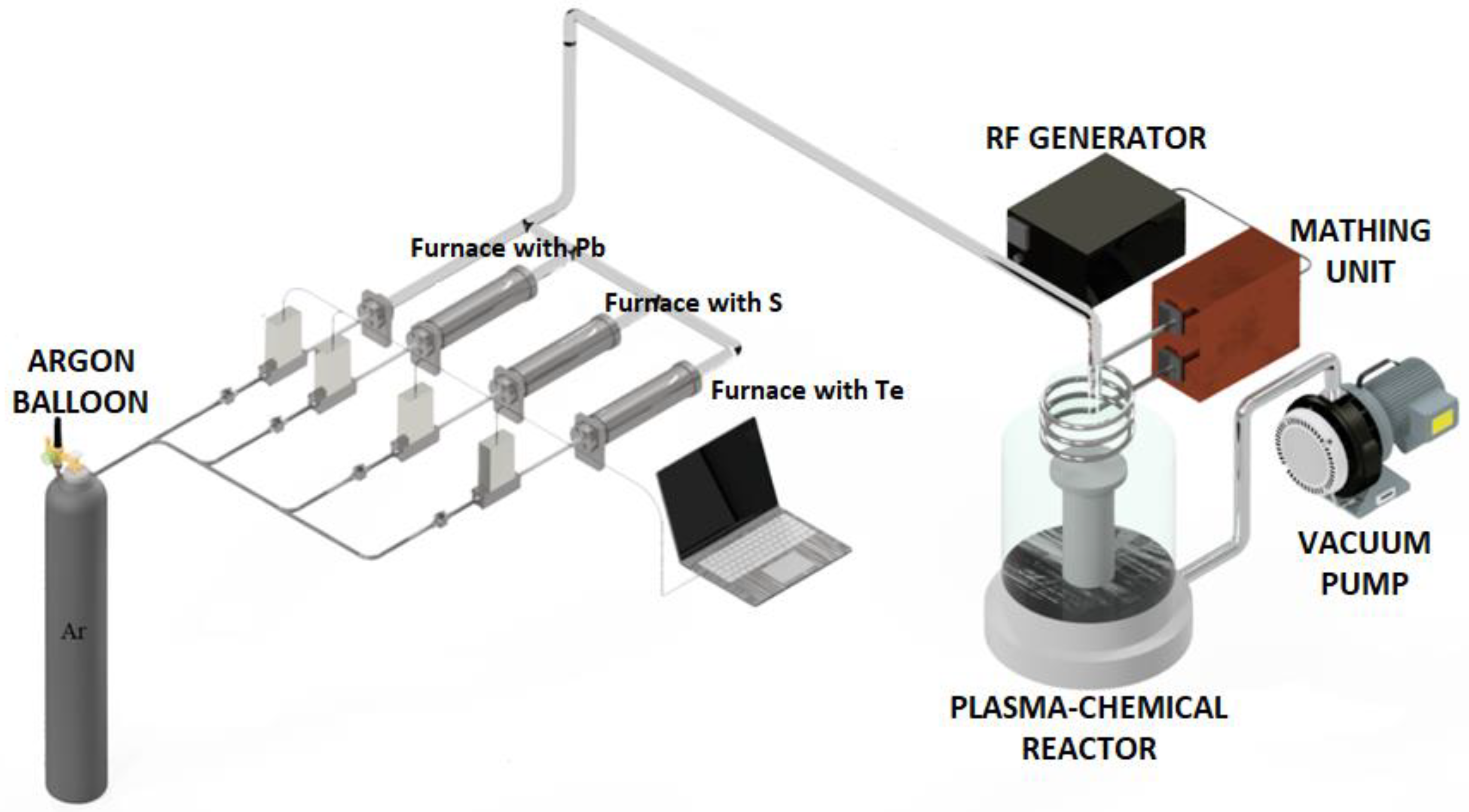
2. Materials and Methods
3. Results and Discussion
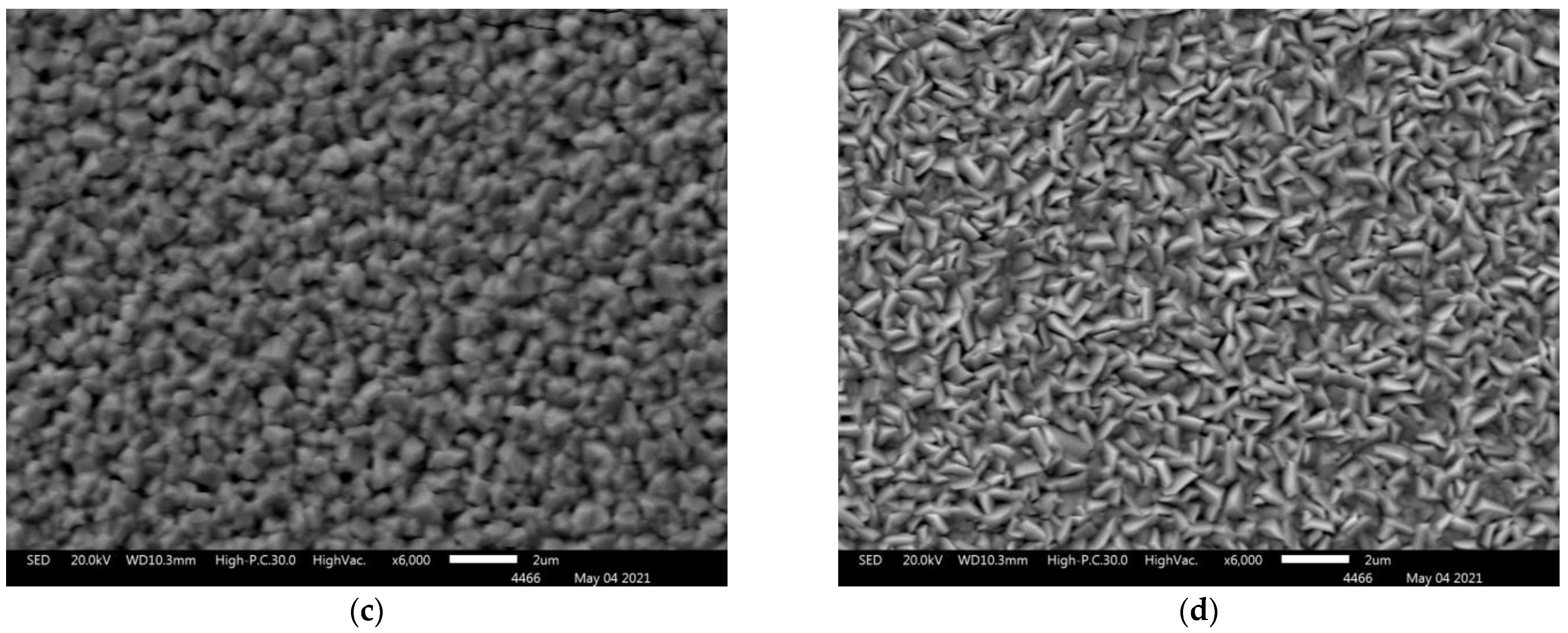
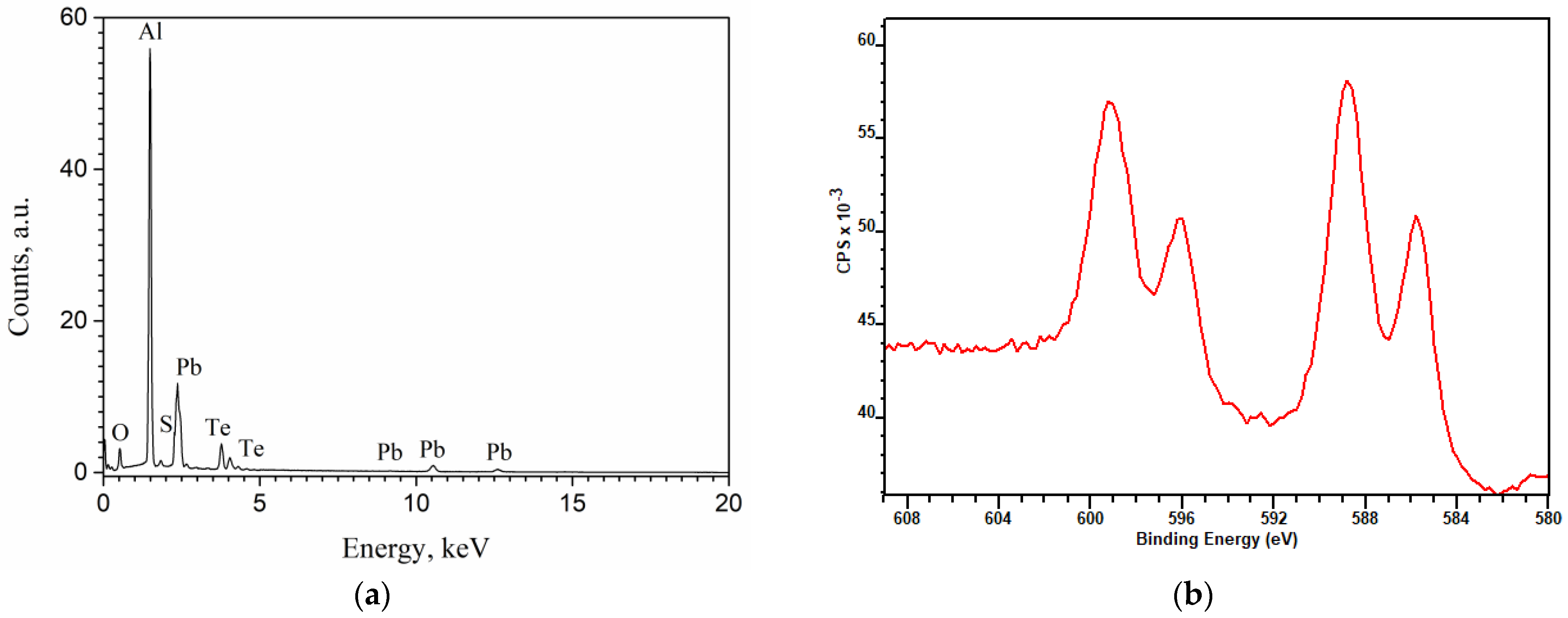
3.1. Elemental Analysis and Scanning Electron Microscopy
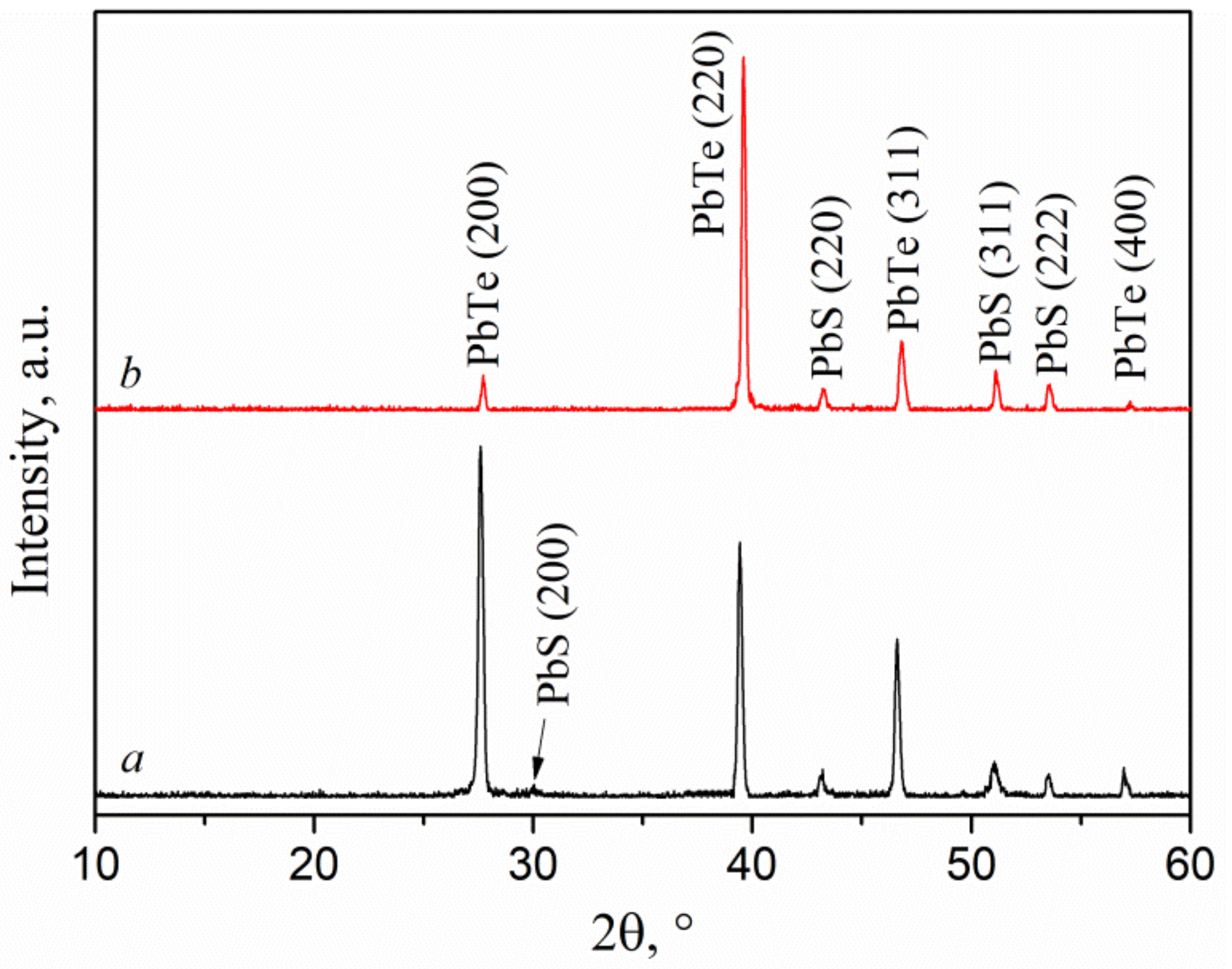
3.2. Results of XRD Phase Analysis
3.3. Thermoelectric Properties of the Films
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, B.; Zhong, S.P.; Zhang, Z.B.; Zheng, Z.Q.; Zhang, Y.P.; Zhang, H. Broadband photodetectors based on 2D group IVA metal chalcogenides semiconductors. Appl. Mater. Today 2019, 15, 115–138. [Google Scholar] [CrossRef]
- Xu, Y.; Li, R.; Bai, S.; Li, Y.; Jia, Z.; Yang, Y.; Cui, E.; Yao, F.; Wang, D.; Lei, C.; et al. Chalcogenide-based narrowband photodetectors for imaging and light communication. Adv. Funct. Mater. 2022, 33, 2212523. [Google Scholar] [CrossRef]
- Tayyab, M.; Liu, Y.; Liu, Z.; Xu, Z.; Yue, W.; Zhou, L.; Lei, J.; Zhang, J. A new breakthrough in photocatalytic hydrogen evolution by amorphous and chalcogenide enriched cocatalysts. Chem. Eng. J. 2023, 455, 140601. [Google Scholar] [CrossRef]
- Tshimangadzo, S.M.; Philiswa, N.N. Review on metal chalcogenides and metal chalcogenide-based nanocomposites in photocatalytic applications. Chem. Afr. 2023, 19, e01509. [Google Scholar]
- Ali, S.A.; Ahmad, T. Chemical strategies in molybdenum-based chalcogenides nanostructures for photocatalysis. Int. J. Hydrog. Energy 2022, 47, 29255–29283. [Google Scholar] [CrossRef]
- Li, J.; Jimenez-Calvo, P.; Paineau, E.; Ghazzal, M.N. Metal chalcogenides-based heterojunctions and novel nanostructures for photocatalytic hydrogen evolution. Catalysts 2020, 10, 89. [Google Scholar] [CrossRef]
- Yamini, S.A.; Wang, H.; Gibbs, Z.M.; Pei, Y.; Dou, S.X.; Snyder, G.J. Chemical composition tuning in quaternary p-type Pb-chalcogenides—A promising strategy for enhanced thermoelectric performance. Phys. Chem. Chem. Phys. 2014, 16, 1835–1840. [Google Scholar] [CrossRef]
- Yamini, S.A.; Wang, H.; Gibbs, Z.M.; Pei, Y.; Mitchell, D.R.G.; Dou, S.X.; Snyder, G.J. Thermoelectric performance of tellurium-reduced quaternary p-type lead–chalcogenide composites. Acta Mater. 2014, 80, 365–372. [Google Scholar] [CrossRef]
- Yamini, S.A.; Patterson, V.; Santos, R. Band-gap nonlinearity in lead chalcogenide (PbQ, Q = Te, Se, S) alloys. ACS Omega 2017, 2, 3417–3423. [Google Scholar] [CrossRef]
- Manettas, A.; Santos, R.; Ferreres, X.R.; Yamini, S.A. Thermoelectric performance of single-phase p-type quaternary (PbTe)0.65−x(PbSe)0.35(PbS)x alloys. ACS Appl. Energy Mater. 2018, 1, 1898–1903. [Google Scholar] [CrossRef]
- Hone, F.G.; Ampong, F.K.; Nkum, R.K.; Boakye, F. Band gap engineering in lead sulphur selenide (PbS1−xSex) thin films synthesized by chemical bath deposition method. J. Mater. Sci. Mater. Electron. 2017, 28, 2893–2900. [Google Scholar]
- Kudryashov, M.; Mochalov, L.; Nezdanov, A.; Kornev, R.; Logunov, A.; Usanova, D.; Mashin, A.; De Filpo, G.; Gogova, D. A novel plasma-based method for synthesis of As-Se-Te films: Impact of plasma parameters on the structure, composition, and optical properties. Superlattices Microstruct. 2019, 128, 334–341. [Google Scholar] [CrossRef]
- Mochalov, L.; Logunov, A.; Prokhorov, I.; Sazanova, T.; Kudrin, A.; Yunin, P.; Zelentsov, S.; Letnianchik, A.; Starostin, N.; Boreman, G.; et al. Plasma-chemical synthesis of lead sulphide thin films for near-IR photodetectors. Plasma Chem. Plasma Process. 2020, 41, 493. [Google Scholar] [CrossRef]
- Mochalov, L.; Logunov, A.; Markin, A.; Kitnis, A.; Vorotyntsev, V. Characteristics of the Te-based chalcogenide films dependently on the parameters of the PECVD process. Opt. Quantum Electron. 2020, 52, 197. [Google Scholar] [CrossRef]
- Kumanek, B.; Janas, D. Thermal conductivity of carbon nanotube networks: A review. J. Mater. Sci. 2019, 54, 7397–7427. [Google Scholar] [CrossRef]
- Tambasov, I.A.; Voronin, A.S.; Evsevskaya, N.P.; Kuznetsov, Y.M.; Luk’yanenko, A.V.; Tambasova, E.V.; Gornakov, M.O.; Dorokhin, M.V.; Loginov, Y.Y. Experimental study of the thermal conductivity of single-walled carbon nanotube-based thin films. Phys. Solid State 2020, 6, 1090–1094. [Google Scholar] [CrossRef]
- Dorokhin, M.V.; Kuznetsov, Y.M.; Lesnikov, V.P.; Zdoroveishchev, A.V.; Demina, P.B.; Erofeeva, I.V. Studies of thermoelectric properties of superlattices based on manganese silicide and germanium. Phys. Solid State 2019, 12, 2348–2352. [Google Scholar] [CrossRef]
- Erofeeva, I.V.; Dorokhin, M.V.; Lesnikov, V.P.; Kuznetsov, Y.M.; Zdoroveyshchev, A.V.; Pitirimova, E.S. Thermoelectric effects in nanoscale layers of manganese silicide. Semiconductors 2017, 11, 1403–1408. [Google Scholar] [CrossRef]
- Orlova, D.S.; Rogacheva, E.I. Galvanomagnetic properties of thin films of bismuth, doped with tellurium. Nanosyst. Nanomater. Nanotech. 2009, 2, 487–493. (In Russian) [Google Scholar]
- Park, N.-W.; Lee, W.-Y.; Yoon, Y.-S.; Kim, G.-S.; Yoon, Y.-G.; Lee, S.-K. Achieving out-of-plane thermoelectric figure of merit ZT = 1.44 in a p-type Bi2Te3/Bi0.5Sb1.5Te3 superlattice film with low interfacial resistance. ACS Appl. Mater. Interfaces 2019, 11, 38247–38254. [Google Scholar] [CrossRef]
- Zheng, Z.-H.; Shi, X.-L.; Ao, D.-W.; Liu, W.-D.; Li, M.; Kou, L.-Z.; Chen, Y.-X.; Li, F.; Wei, M.; Liang, G.-X.; et al. Harvesting waste heat with flexible Bi2Te3 thermoelectric thin film. Nat. Sustain. 2022, 6, 180–191. [Google Scholar] [CrossRef]
- Zheng, D.; Jin, H.; Liao, Y.; Ji, P. Bi2Te3 nanowires tuning PEDOT:PSS structure for significant enhancing electrical transport property. Mater. Lett. 2023, 338, 134019. [Google Scholar] [CrossRef]
- Lach-hab, M.; Papaconstantopoulos, D.A.; Mehl, M.J. Electronic structure calculations of lead chalcogenides PbS, PbSe, PbTe. J. Phys. Solids 2002, 63, 833–841. [Google Scholar] [CrossRef]
- Pei, Y.; LaLonde, A.; Iwanaga, S.; Snyder, G.J. High thermoelectric figure of merit in heavy hole dominated PbTe. Energy Environ. Sci. 2011, 4, 2085–2089. [Google Scholar] [CrossRef]
- Heremans, J.P.; Jovovic, V.; Toberer, E.S.; Saramat, A.; Kurosaki, K.; Charoenphakdee, A.; Yamanaka, S.; Snyder, G.J. Enhancement of thermoelectric efficiency in PbTe by distortion of the electronic density of states. Science 2008, 321, 554–557. [Google Scholar] [CrossRef] [PubMed]
- Rogacheva, E.I.; Krivulkin, I.M.; Nashchekina, O.N.; Sipatov, A.Y.; Volobuev, V.V. Effect of oxidation on the thermoelectric properties of PbTe and PbS epitaxial films. Appl. Phys. Lett. 2001, 78, 1661–1663. [Google Scholar] [CrossRef]
- Yang, D.; Lu, C.; Yin, H.; Herman, I.P. Thermoelectric performance of PbSe quantum dot films. Nanoscale 2013, 5, 7290–7296. [Google Scholar] [CrossRef]
- Yan, Q.; Chen, H.; Zhou, W.; Hng, H.H.; Boey, F.Y.C.; Ma, J. A simple chemical approach for PbTe nanowires with enhanced thermoelectric properties. Chem. Mater. 2008, 20, 6298–6300. [Google Scholar] [CrossRef]
- Rogacheva, E.I.; Nashchekina, O.N.; Vekhov, Y.O.; Dresselhaus, M.S.; Cronin, S.B. Effect of thickness on the thermoelectric properties of PbS thin films. Thin Solid Films 2003, 423, 115–118. [Google Scholar] [CrossRef]
- Geethu, R.; Jacob, R.; Shripathi, T.; Okram, G.S.; Ganesan, V.; Tripathi, S.; Fatima, A.; Sreenivasan, P.V.; Urmila, K.S.; Pradeep, B.; et al. Optoelectronic and thermoelectric properties in Ga doped β-PbS2 nanostructured thin films. Appl. Surf. Sci. 2012, 258, 6257–6260. [Google Scholar] [CrossRef]
- Mochalov, L.A.; Kuznetsov, Y.M.; Dorokhin, M.V.; Fukina, D.G.; Knyazev, A.V.; Kudryashov, M.A.; Kudryashova, Y.P.; Logunov, A.A.; Mukhina, O.V.; Zdoroveyshchev, A.V.; et al. Thermoelectrical properties of ternary lead chalcogenide plumbum-selenium-tellurium thin films with excess of tellurium prepared by plasma-chemical vapor deposition. Thin Solid Films 2020, 752, 139244. [Google Scholar] [CrossRef]
- Chen, X.; Zhou, Z.; Lin, Y.-H.; Nan, C. Thermoelectric thin films: Promising strategies and related mechanism on boosting energy conversion performance. J. Mater. 2020, 6, 494–512. [Google Scholar] [CrossRef]
- Ibanez, M.; Zamani, R.; Gorsse, S.; Fan, J.; Ortega, S.; Cadavid, D.; Morante, J.R.; Arbiol, J.; Cabot, A. Core-shell nanoparticles as building blocks for the bottom-up production of functional nanocomposites: PbTe-PbS thermoelectric properties. ACS Nano. 2013, 7, 2573–2586. [Google Scholar] [CrossRef] [PubMed]
- Dorokhin, M.V.; Kuznetsov, Y.M.; Demina, P.B.; Erofeeva, I.V.; Zavrazhnov, A.Y.; Boldin, M.S.; Lantsev, E.A.; Popov, A.A.; Boryakov, A.V.; Zdoroveyshchev, A.V.; et al. High-efficiency spark plasma sintered Ge0.3Si0.7:P thermoelectric energy converters with silicone phosphide as a source of phosphorus doping. Nanoscale Microscale Thermophys. Eng. 2023, 27, 125–134. [Google Scholar] [CrossRef]
- Othman, M.S. Mechanical response of PbSSe, PbSTe ternary and PbSnSTe quaternary alloys at high pressure. ARO-Sci. J. Koya Univ. 2020, 8, 29–33. [Google Scholar] [CrossRef]
- Boryakov, A.V.; Surodin, S.I.; Kryukov, R.N.; Nikolichev, D.E.; Zubkov, S.Y. Spectral fit refinement in XPS analysis technique and its practical applications. J. Electron Spectrosc. Relat. Phenom. 2018, 50, 1443–1448. [Google Scholar] [CrossRef]
- Crist, B.V. Handbooks of Monochromatic XPS Spectra: The Elements and Native Oxides; XPS International Inc.: Ames, IA, USA, 1999; Volume 1. [Google Scholar]
- Crist, B.V. Handbooks of Monochromatic XPS Spectra: Commercially Pure Binary Oxides and a Few Common Carbonates and Hydroxides; XPS International LLC: Mountain View, CA, USA, 2005; Volume 2. [Google Scholar]
- Shyju, T.S.; Anandhi, S.; Sivakumar, R.; Gopalakrishnan, R. Studies on lead sulfide (PbS) semiconducting thin films deposited from nanoparticles and Its NLO application. Int. J. Nanosci. 2014, 1, 1450001. [Google Scholar] [CrossRef]
- Li, W.; Fang, C.; Dijkstra, M.; Huis, M. The role of point defects in PbS, PbSe and PbTe: A first principles study. J. Phys. Condens. Matter. 2015, 27, 355801. [Google Scholar] [CrossRef]
- Bala, M.; Gupta, S.; Tripathi, T.S.; Varma, S.; Tripathi, S.K.; Asokan, K.; Avasthi, D.K. Enhancement of thermoelectric power of PbTe:Ag nanocomposite thin films. RSC Adv. 2015, 5, 33. [Google Scholar] [CrossRef]
- Gupta, S.; Agarwal, D.C.; Tripathi, S.K.; Neeleshwar, S.; Panigrahi, B.K.; Jacquot, A.; Lenoir, B.; Avasthi, D.K. Superiority of ion irradiation over annealing for enhancing the thermopower of PbTe thin films. Radiat. Phys. Chem. 2013, 86, 6–9. [Google Scholar] [CrossRef]
- Karthikeyan, V.; Surjadi, J.U.; Wong, J.C.K.; Kannan, V.; Lam, K.-H.; Chen, X.; Lu, Y.; Roy, V.A.L. Wearable and flexible thin film thermoelectric module for multi-scale energy harvesting. J. Power Sources 2020, 455, 227983. [Google Scholar] [CrossRef]
- Ahmad, K.; Almutairi, Z.; Wan, C. Thermoelectric properties of PbTe-based graphene nanocomposite. J. Mater. Sci. Mater. Electron. 2020, 31, 20996–21004. [Google Scholar] [CrossRef]




| № | P (W) | Substrate | Compound, at. % | |||
|---|---|---|---|---|---|---|
| Pb | S | Te | ||||
| 1 | 60 | Al2O3 | 50 ± 1 | 3 ± 1 | 47 ± 1 | (PbTe)0.94(PbS)0.06 |
| 2 | 60 | Si | 50 ± 1 | 5 ± 1 | 45 ± 1 | (PbTe)0.90(PbS)0.10 |
| 3 | 100 | Al2O3 | 50 ± 1 | 10 ± 1 | 40 ± 1 | (PbTe)0.80(PbS)0.20 |
| 4 | 100 | Si | 50 ± 1 | 12 ± 1 | 38 ± 1 | (PbTe)0.76(PbS)0.24 |
| No. | Composition | ρ, μOhm·m | μ, cm2/V∙s | p, 1018 cm−3 |
|---|---|---|---|---|
| 1 | PbTe | 627 ± 6 | 150 ± 5 | 0.67 ± 0.02 |
| 2 | (PbTe)0.9(PbS)0.1 | 332 ± 3 | 72.6 ± 2.2 | 2.59 ± 0.08 |
| 3 | (PbTe)0.8(PbS)0.2 | 200 ± 2 | 50.3 ± 1.5 | 6.21 ± 0.19 |
| 4 | (PbTe)0.7(PbS)0.3 | 176 ± 2 | 49.2 ± 1.5 | 7.22 ± 0.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuznetsov, Y.M.; Mochalov, L.A.; Dorokhin, M.V.; Fukina, D.G.; Kudryashov, M.A.; Kudryashova, Y.P.; Zdoroveyshchev, A.V.; Zdoroveyshchev, D.A.; Kalentyeva, I.L.; Kriukov, R.N. PECVD Synthesis and Thermoelectric Properties of Thin Films of Lead Chalcogenides (PbTe)1−x(PbS)x. Coatings 2023, 13, 1030. https://doi.org/10.3390/coatings13061030
Kuznetsov YM, Mochalov LA, Dorokhin MV, Fukina DG, Kudryashov MA, Kudryashova YP, Zdoroveyshchev AV, Zdoroveyshchev DA, Kalentyeva IL, Kriukov RN. PECVD Synthesis and Thermoelectric Properties of Thin Films of Lead Chalcogenides (PbTe)1−x(PbS)x. Coatings. 2023; 13(6):1030. https://doi.org/10.3390/coatings13061030
Chicago/Turabian StyleKuznetsov, Yurii Mikhailovich, Leonid Alexandrovich Mochalov, Mikhail Vladimirovich Dorokhin, Diana Georgievna Fukina, Mikhail Alexandrovich Kudryashov, Yuliya Pavlovna Kudryashova, Anton Vladimirovich Zdoroveyshchev, Daniil Antonovich Zdoroveyshchev, Irina Leonidovna Kalentyeva, and Ruslan Nikolayevich Kriukov. 2023. "PECVD Synthesis and Thermoelectric Properties of Thin Films of Lead Chalcogenides (PbTe)1−x(PbS)x" Coatings 13, no. 6: 1030. https://doi.org/10.3390/coatings13061030
APA StyleKuznetsov, Y. M., Mochalov, L. A., Dorokhin, M. V., Fukina, D. G., Kudryashov, M. A., Kudryashova, Y. P., Zdoroveyshchev, A. V., Zdoroveyshchev, D. A., Kalentyeva, I. L., & Kriukov, R. N. (2023). PECVD Synthesis and Thermoelectric Properties of Thin Films of Lead Chalcogenides (PbTe)1−x(PbS)x. Coatings, 13(6), 1030. https://doi.org/10.3390/coatings13061030






